Abstract
Electrical resistances of roots and stems of hydroponically raised willows (Salix schwerinii) were studied and related to root morphology. Willow cuttings with and without roots were set in a constant electric field (effective voltage of 0.1 V, sine-AC, 128 Hz) in a hydroponic solution. The electrical resistance of different components in the measurement system was measured and analysed in relation to root surface area in contact with the cultivation solution. Axial resistivities of single root segments and of stems were measured. The results showed that the resistance decreased in relation to an increase in the contact surface area of the roots with the solution. The resistance depended strongly on the contact area of the stem with the solution, however, thus causing bias in the evaluation of root surface area. This work is a new contribution for the understanding of current pathways in the root system as exposed to an external electric field and for developing a non-destructive method to study plant roots accordingly. It may be concluded that the electrical resistance method is a useful non-destructive method to study roots and their physiological properties. Electrical analogues for roots and stem comprising resistors are discussed in relation to in situ measurements.
Keywords: Earth impedance, hydroponic, non-destructive, resistivity, root, root surface area
Introduction
One of the key functions of plant roots is to supply water and nutrients to the shoot (Clothier and Green, 1997). An increasing number of environmental issues, such as climate change, water management, and soil pollution have given an impetus to study roots and their function (Cudlín et al., 2007). A major obstacle in such studies has been the difficulty to investigate roots in situ. Conventional methods such as soil cores, monoliths, and in-growth cores are destructive and thus not useful for the continuous monitoring of growth, mass, and surface area of roots in field conditions.
New non-destructive techniques, such as minirhizotron imaging, ground penetrating radar (GPR), isotopes, X-ray imaging, and magnetic resonance imaging (MRI) have been used for observing roots and their function at micro and macro scales (Segal et al., 2008; Hirano et al., 2009). These non-destructive methods have their own limitations in their application, however. Minirhizotron imaging, for example, may be used for monitoring root growth dynamics and root longevity, but it gives a limited scope of the whole root system only (Majdi, 1996). Ground penetrating radar (GPR) has been used to map the whole root systems but it may detect clearly coarse roots only (greater than 19 mm in diameter). Root water content also affects GPR results (Hirano et al., 2009). New non-destructive methods are needed for in situ studies of roots and their function.
One of the non-destructive methods for studying roots is based on the electrical properties of roots. Measurements of root electrical capacitance have been particularly interesting (Chloupek, 1972, 1977; Dalton, 1995; Rajkai et al., 2005). In these studies, the root morphology (e.g. fresh or dry mass, length, surface area) from various plant species (maize, onion, tomato, clover, alfalfa, poplar) and even from different genotypes were related with electrical capacitance measured at 1 kHz (Chloupek, 1977; Preston et al., 2004; McBride et al., 2008). Capacitance obtained at a single 1 kHz frequency was assumed to be a measure of active root area and the extent of root system (Dalton, 1995). However, the moisture of the root–soil system and the position of a stem electrode had a strong effect on the capacitance measurements (Chloupek, 1977; Dalton, 1995; van Beem et al., 1998).
The electrical impedance spectroscopy (EIS) is widely used in clinical studies with a large range of frequency (Grimnes and Martinsen, 2008). In plant sciences, EIS has been used to demonstrate the response of detached plant tissues to cold acclimation, freeze–thaw injury, and ozone and carbon dioxide exposure at cellular level (Repo et al., 1994, 2000, 2004; Ryyppö et al., 1998). EIS has been used for studying the properties of intact root systems in only a few studies (Ozier-Lafontaine and Bajazet, 2005; Rajkai et al., 2005; Repo et al., 2005).
In a simple version of the EIS, termed the earth impedance method, measurement is run at a single low frequency, and the absorbing root surface area (ARSA) is assessed accordingly (Aubrecht et al., 2006; Čermák et al., 2006). In this method, the soil–root–stem system is connected to an electric circuit, and a low frequency current from an external source is driven to the roots through electrodes set in the stem and soil. According to the theory, the current would pass from the root to the soil predominantly at the root tips (Aubrecht et al., 2006). The resistance is assumed to be related to absorbing root surface area (ARSA). The value of ARSA depends on the longitudinal electrical resistivity of the root and the mean extension of the roots from the trunk in field conditions. The resistivity of roots is difficult to assess in field conditions. Therefore, it is assumed to be approximately the same as in the stem and assessed on the stem accordingly. Recently, electrical resistance tomography (ERT) of soil was applied to detect the spatial distribution of the roots (Amato et al., 2009).
It was found previously that the contact of stem of young maize seedlings with the nutrient cultivation solution did not affect the electrical properties, i.e. capacitance and resistance, of the overall system composing of a piece of stem, roots, and the solution (Walker, 1965). Some recent studies on woody plants suggest that the current would not pass to soil through the roots but at the root collar, however (J Urban and R Bequet, personal communication). Therefore, with regard to the applications of the earth impedance method, more studies are needed on the current pathways and the equivalent circuits of soil–root systems (Dalton, 1995).
The aim of this study was to measure electrical properties of the roots in relation to their morphology in hydroponically grown willows and to assess the effect of the stem on electrical current pathways. The hypotheses were: (i) In a constant electric field, the current through the root–solution interface and the resistance accordingly is related to the root surface area in contact with the solution. (ii) The resistance between the plant and the solution is affected, besides roots, by the contact of the cutting with the solution too. This contact would cause bias in evaluation of the properties of the root system.
Materials and methods
Dormant willow cuttings (Salix schwerinii) were collected in January, 2009 from a plantation in Siikasalmi (62°30′ N, 29°30′ E) in eastern Finland, and stored in water containers at a cold room (4 °C). Thirty-two cuttings (18–20 mm in diameter, 250 mm in length) were cultivated for 12 d in aerated tap water containers (size 500×390×250 mm) in a growth chamber (PGW36, Conviron, Winnipeg, Canada) with the following conditions: the air temperature 20 °C, the photon flux density 320 μmol m−2 s−1, the photoperiod 18/6 h (day/night), and a relative humidity of 80%. The cuttings were embedded in the water solution half way by using floating pads. Water was refreshed at 3–4 d intervals. Two days before the electrical measurements, the tap water was replaced by a nutrient solution (10 mg N in 1.0 l of water with macro- and micronutrients; Riddoch et al., 1991). The conductivity of the hydroponic solution was 54.2 μS cm−1. After 12 d of growth, the specimens were moved within the same containers from the growth chamber to laboratory (23 °C) to start the measurements next day.
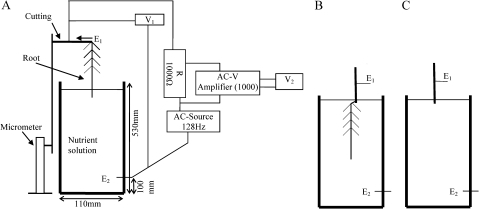
One brightly coloured and non-wounded root of each cutting was isolated from neighbouring roots and connected to an electric circuit (Fig. 1). Other roots were wrapped in a clean plastic sheet and isolated from the measurement circuitry. Constant electric field (effective voltage of 0.1 V, sine-AC, 128 Hz) (TG215, TTi, UK) was applied to study the electric resistance of single roots at different immersion depths in the nutrient solution. The depth was adjusted by a micrometer starting from the root apex until the whole intact root was immersed. Voltage and current electrodes (Ag-needles, 0.5 mm in diameter) were inserted in the stem of the cutting and at the bottom of the solution container (Fig. 1). Voltage and current was assessed at each immersion depth (Fluke 8022A Multimeter, John Fluke Inc., Washington, USA). The electrical current passing through the root–stem continuum (IA) and the total resistance (RA) were calculated by equations 1 and 2, respectively.
| (1) |
| (2) |
where V2 and V1 (V1=0.1 V) are the voltages, Rcon is a known resistance (1000 Ω), and 103 is the amplifier gain factor (Fig. 1).
Fig. 1.
Diagram of the experimental set-ups for the measurement of electrical resistance of willow roots. (A) Root apex of a single intact root in contact with the solution. (B) Whole root with a piece of stem in the solution. (C) A piece of stem in the solution. V1 and V2 are voltage meters. E1 and E2 refer to the silver electrodes. Immersion depth of the root in the solution is adjusted by the micrometer. Note: The measurement circuitry in the (B) and (C) is the same as in (A).
The resistance of the root with a piece of stem included, RB, was measured at different immersion depths until the stem contacted the solution (Fig. 1B). Then the root was dissected from the cutting, and the resistance of the stem, RC, was measured as immersed at the same depth as with the root (Fig. 1C).
After the electrical measurements of the whole root, it was scanned (Epson Expression 1640XL, Epson America, Inc., USA) to assess the root surface area and the number of lateral roots using image analysis (WinRhizo, Régent Instruments Inc, Québec, Canada).
Distribution of axial electrical resistivity along the root profile was assessed after scanning. Root segments (lroot was 8 mm) were excised from the root tip over the free lateral root area. The specimens were set longitudinally between two Ag/AgCl electrodes (RCI, WPI Ltd., Sarasota, USA) with an electrode gel in-between (Signa gel, Parker Labs Inc., Fairfield, USA) (Repo, 1994). The electrodes were connected to an LCR-analyser (HP-4284A, Hewlett-Packard, Santa Clara, USA) and the axial resistance of the root was measured at 0.1 V and 128 Hz. Subsequently, each root segment was scanned to assess the average diameter (Droot) and to calculate the resistivity. The roots were kept in the same nutrient solution during scanning and transporting. Axial electrical resistivity (proot in unit Ωm) of each root segment was calculated by equation 3.
| (3) |
where Rroot is the electrical resistance of each root segment, Droot is the average diameter of the root segment, and lroot is the length of the root segment (8 mm).
The resistivity of the stem was obtained by a classic method of electricity (Aubrecht et al., 2006). Four electrodes within the same distance (lstem=10 mm) were inserted into the stem and connected to a grounding tester (Fluke 1625, John Fluke Inc., Washington, USA) to record resistance (Rstem) at 128 Hz. The diameter (Dstem) of the stem was measured, and the stem resistivity (pstem in unit Ωm) was calculated by applying equation 3.
Statistical analysis
Relationships between electrical resistance and root morphology (root surface area and the number of lateral roots) were determined by using Person correlation analysis and a general linear and non-linear regression analysis. The differences of axial electrical resistivity of the root segments, and the resistance of the cuttings with and without the roots were tested by one-way ANOVA. The Tukey procedure was used in multiple comparisons. Statistical significance was assessed at the level of 0.05. Statistical analyses were performed by using SPSS software (ver.15.0, SPSS Inc., IL, USA).
Results
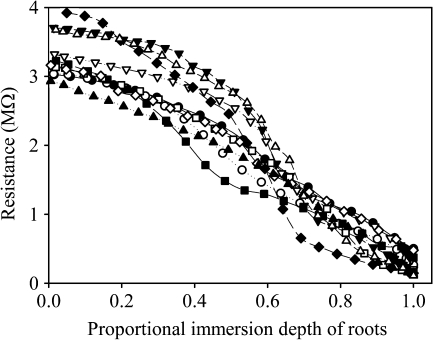
The total electrical resistance (RA) was considerably high (3–4 MΩ) when the root apex was in contact with the nutrient solution only. When the distal part of the root was gradually immersed into the solution, the resistance decreased with an increasing immersion depth. When the whole root was immersed in the nutrient solution, the electrical resistance ranged between 100–700 kΩ depending on the size of the root (Fig. 2).
Fig. 2.
Ten examples (out of 31 samples in total) of the root electrical resistance as determined by gradual immersion of the root at different depths in the nutrient solution. The x-axis scale is normalized according to the immersion depth as recorder on the micrometer (see Fig. 1). Each curve represents a single root.
When correlation was calculated for individual roots separately, there was a significant correlation (P <0.01) between electrical resistance and root morphology, i.e. surface area and number of lateral roots. The electrical resistance had a significant negative correlation with its corresponding root surface area (r= –0.93) and with the number of lateral roots (r= –0.91). Meanwhile, the root surface area positively correlated with the number of lateral roots and root length, respectively (r=0.98 for both).
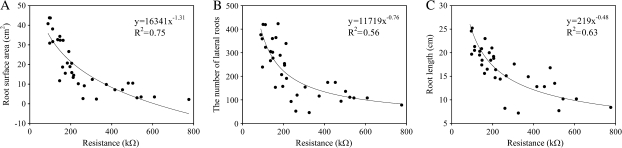
The resistance and root morphological characteristics (surface area, length, and number of lateral roots) were significantly correlated when whole roots of different plants were immersed as a whole in the solution (proportional immersion depth=1 in Fig. 2). The resistance decreased non-linearly (Fig. 3) with an increase of the root size. A significant linear relation was found between the root surface and the number of lateral roots, and with root length (R2=0.62 and R2=0.69, respectively).
Fig. 3.
Relation between the root electrical resistance and root surface area (A), the number of lateral roots (B), and the root length (C) for the intact willow roots as immersed in the nutrient solution (n=31).
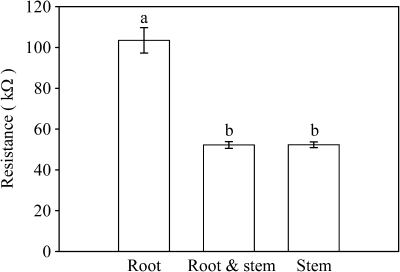
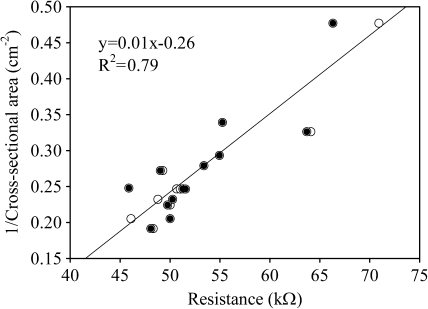
Contact of the stem with the solution had a significant effect on the resistance. The resistance halved (Fig. 4) when the root and a piece of the stem (Fig. 1B) were immersed in the solution as compared with the root in the solution only (Fig. 1A). However, no difference was observed when the stem was in contact with the solution with or without the root (Fig. 4). A significant linear relation was found between cross-sectional area of the stem and the resistance of the stem as immersed with or without the root (RB, RC, respectively) immersed in the solution (Fig. 5).
Fig. 4.
The electrical resistance of intact willow root (Root), root and a piece of stem (Root and stem), and the stem only with the root dissected (Stem) as immersed in the nutrient solution (see Fig. 1A, B, and C, respectively). Bars indicate standard error of the mean (n=16). The same letter indicates no significant difference at the level of 0.05.
Fig. 5.
Relation between the electrical resistances of willow cuttings (with and without root) and the cross-sectional area of the stems as immersed in the nutrient solution (see Fig. 1B and C, respectively). Open and dots closed symbols refer to the stems with and without root, respectively.
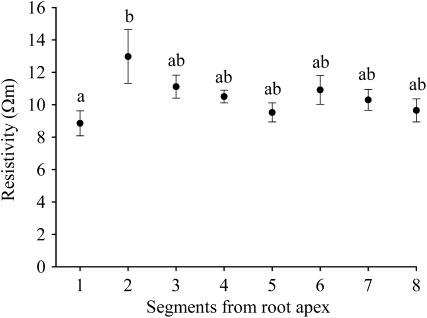
For 8 mm long samples of fine root, axial electrical resistivity (proot) varied between 8.9 and 13.0 Ωm along the root profile from the apex to a distance of 64 mm. The mean value was 10.5 Ωm (Fig. 6). The lowest resistivity was at the root apex (segment 1) and the highest next to the root apex (segment 2). No significant difference occurred between any other root segments. The stem resistivity (pstem) ranged between 73.9 to 134.8 Ωm, and the mean was 108.3 Ωm.
Fig. 6.
The axial electrical resistivity of the 8 mm long segments along the root starting from the apex. Bars indicate the standard error of the mean (n=31). The segments with the same letter code are not significantly different (Tukey multiple comparisons test at the significance level of 0.05).
Discussion
A constant electric field was applied to study the electrical resistance of roots of willow cuttings in a nutrient solution. The results indicate that the electrical resistance method offers good opportunities to investigate the relationship between electrical resistance and the root morphology (root surface area, the number of lateral roots, and root length) of willows. The study also showed the limitations of this method because the contact of the cut surface of the stem with the growing medium caused a large current passage through the cutting cylinder.
When the root was gradually immersed in the solution (Fig. 1A), a significant correlation was found between electrical resistance and proportion of the root in the solution (Fig. 2). This indicates that the contact surface area between the roots and the substrate has an important role in the overall electrical resistance of the root–soil interface. There is a reason to believe that the absorbing root surface area for the uptake of water and nutrients increased accordingly (Segal et al., 2008). Meanwhile, and even more importantly, a significant relationship was found between electrical resistance and root morphology when the whole intact root of different size was immersed in the solution (Fig. 5). These results agree with the assumption of the earth impedance method that the electrical resistance is related to the root area (Aubrecht et al., 2006; Čermák et al., 2006).
The resistance halved when, in addition to roots, a part of the stem was immersed in the solution (Fig. 4). Moreover, the electrical resistance remained approximately the same when the stem with and without the root was in contact with the solution (Fig. 4). This suggests that, in this particular case, with willow cuttings the roots would play a minor role in the total electrical resistance of stem and root in the solution. In addition to the outer surface of the stem, the cut surface probably formed a good contact with the solution through the phloem and xylem of the stem. This seemed to provide a high passage of current (as compared to roots) between the stem and the solution, resulting in low electrical resistance accordingly, the latter being linearly related with the cross-sectional area of the stem (Fig. 5). This conclusion is further supported by the observation that there was no difference in the relationship between resistance and cross-sectional area of the stem with or without the roots (Fig. 5). A similar linear relationship was observed in maize plants in a nutrient solution (Walker, 1965) and in coniferous and broadleaf woody species under field conditions (Čermák et al., 2006). Further studies are needed to test if the stem contact would have as strong an effect on the overall resistance in seed-originated intact plants as in the cuttings of this study.
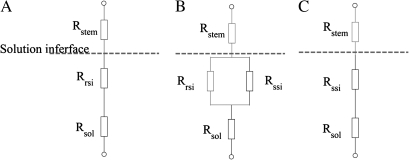
The results of this study may be considered in terms of electrical circuit analogues formed of resistors with corresponding equations (see Appendix). In each the electrical analogues, there are two resistances that remain approximately the same, i.e. the stem and solution. They may be estimated as known parameters through the resistivity of the stem (mean value 108.3 Ωm) and the conductivity of the solution (54.2 μS cm−1 corresponding to the resistivity of 184.5 Ωm), respectively. The resistance of the system function, i.e. the solution and the E2-electrode without plant, was far less than 10 kΩ being much less than the resistance of the root immersed in the solution (cf. Fig. 2). The root–solution and the stem–solution interfaces result in variability in the parameters of the electrical analogues accordingly. When the stem with the root was immersed in the solution (Fig. 1B), a parallel electrical circuit was formed of the root–solution interface and the stem–solution interface (equation A2, see Appendix), and the former having larger resistance than the latter (equations A1 and A3, see Appendix), respectively. Due to this parallel circuitry there was no significant difference in the total resistance of the stem with and without the root immersed in the solution. According to the measurement at a single low frequency a detailed impedance analysis of the system that would require an electrical impedance spectroscopic (EIS) approach cannot be run (Ozier-Lafontaine and Bajazet, 2005; Repo et al., 2005). The EIS would allow an estimation of the resistances and capacitances of the affecting components in the circuitry, i.e. the electrode–solution interface, solution, root, stem, and electrode–stem interface (Dalton, 1995).
Electrode polarization impedance commonly occurs at low frequencies, decreasing with increasing the frequency depending of the electrodes (Geddes and Baker, 1989; Grimnes and Martinsen, 2008). The influence of the electrode polarization can be minimized by using reversible electrodes, e.g. Ag/AgCl electrodes (Repo, 1994; Dalton, 1995; Ozier-Lafontaine and Bajazet, 2005). In our measurements, at the frequency of 128 Hz, the resistance due to polarization at the electrode–stem and electrode–solution interfaces was small but not fully absent as compared with other components in the circuit (Y Cao, unpublished data). The solution electrode was fixed in the same position and just the stem electrode was changed from plant to plant. Thus, it may be assumed that the bias due to the polarization resistance was additively the same in different measurements, and would not affect the conclusion concerning the role of roots.
The experiment was carried out in the hydroponic solution where two important factors causing variability in the field measurements were fixed, i.e. soil moisture content and soil type. In the field measurements, the conductivity of soil will vary depending on the water content and highly mobile ions, such as K+, and Cl– (Dvorak et al., 1981). Soil properties, i.e. soil moisture, salinity, and soil texture, have been observed to affect the capacitance and impedance measurements (Dalton, 1995) Therefore, it has been recommended that the measurements should be run at a soil moisture content high enough, i.e. the field capacity (Dalton, 1995, van Beem et al., 1998).
The position of the stem electrodes should be considered in the earth impedance measurements. The position of the stem electrode affects the proportion of the stem in the circuit and the resistance accordingly. The stem electrode should be set close to primary lateral roots and it should remain in the same position during measurements (Dalton, 1995, Preston et al., 2004; Rajkai, 2005). In beans, for instance, raising the electrode 10 cm upwards from the root collar leads to a linear increase in resistance from below 1 kΩ to above 150 kΩ, and to a non-linear decrease in capacitance from 70 nF to less than 5 nF (Dalton, 1995). With regard to the position of the soil electrode, preliminary studies have shown that the distance and depth of the soil electrode would not have any effect on the capacitance and resistance (van Beem et al., 1998; Preston et al., 2004; Ozier-Lafontaine and Bajazet, 2005). In the earth impedance method, however, the mean extension of the roots is determined according to the resistance when the soil electrode is moved apart from the stem (Čermák et al., 2006). These contradictory results may be due to the four-electrode technique in the earth impedance and two-electrode technique in other measurements.
Root axial resistivity changed along the root profile being the lowest at the root tips and the highest next to the root tips. This agrees with a study on corn roots (Anderson and Higinbotham, 1976). Near the root tip there is a hydraulically isolated zone with immature metaxylem and a mature protoxylem which is non-functional for water absorption. This part, therefore, can be minimized in a consideration of the water flow into the vascular tissue (Steudle and Peterson, 1998). It may be assumed that the high axial electrical resistance next to the root tip is related with the ineffective zone for transport water (Frensch and Steudle, 1989).
In conclusion, the electrical resistance method is a promising and non-destructive method for studying roots and their function. It is possible to apply this non-destructive method for studying the dynamics of roots and their function. However, the role of the stem in contact with the growth substrate should be considered. More research is required on the calibration of the method with intact plants and, in particular, in field conditions, and on the role of additional components such as mycorrhizas. It may be considered as a complementary non-destructive method to ground penetrating radar (GPR) and electrical resistance tomography (ERT).
Acknowledgments
This work was supported by the China Scholarship Council (CSC, China), the Centre for International Mobility (CIMO, Finland), and the Faculty of Forest Sciences, University of Joensuu.
We thank Mr Unto Pieviläinen (Department of Physics and Mathematics, University of Eastern Finland) for his expertise in technical issues, Mr Josef Urban (Mendel University of Agriculture and Forestry, Czech Republic) for his comments on the manuscript ,and Dr Saija Kaskinen (language centre, University of Eastern Finland) for the English revision of the manuscript.
Appendix
Electrical resistance circuit analogs for the three major measurement set-ups, i.e. root, root and stem, and stem only immersed in the solution.
The equations for the total resistance for the three major measurement set-ups (Fig. A1).
| (A1) |
| (A2) |
| (A3) |
RA is the total resistance when the whole intact root was immersed in the solution (see Fig. 1A); RB is the total resistance when the whole intact root and a piece of the stem was immersed in the solution (see Fig. 1B); RC is the total resistance when a piece of the stem with the root dissected was immersed in the solution (see Fig. 1C).
Fig. A1.
Three electrical resistance analogues corresponding to the three measurement set-ups in Fig. 1A, B, and C, respectively. Symbols: Rrsi the resistance of root-solution interface; Rssi the resistance of stem-solution interface; Rsol the resistance of the solution; Rstem the resistance of the stem above solution interface.
References
- Amato M, Bitella G, Rossi R, Gomez JA, Lovelli S, Gomes JF. Multi-electrode 3D resistivity imaging of alfalfa root zone. European Journal of Agronomy. 2009;31:213–222. [Google Scholar]
- Anderson WP, Higinbotham N. Electrical resistances of corn root segments. Plant Physiology. 1976;57:137–141. doi: 10.1104/pp.57.2.137. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Aubrecht L, Stanek Z, Koller J. Electrical measurement of the absorption surface of tree roots by the earth impedance method. 1. Theory. Tree Physiology. 2006;26:1105–1112. doi: 10.1093/treephys/26.9.1105. [DOI] [PubMed] [Google Scholar]
- Čermák J, Ulrich R, Stanek Z, Koller J, Aubrecht L. Electrical measurement of the absorption surface of tree roots by the earth impedance method. 2. Verification based on allometric relationships and root severing experiments. Tree Physiology. 2006;26:1113–1121. doi: 10.1093/treephys/26.9.1113. [DOI] [PubMed] [Google Scholar]
- Chloupek O. The relationship between electric capacitance and some other parameters of plant roots. Biologia Plantarum. 1972;14:227–230. [Google Scholar]
- Chloupek O. Evaluation of the size of a plant's root system using its electrical capacitance. Plant and Soil. 1977;48:525–532. [Google Scholar]
- Clothier BE, Green SR. Roots: the big movers of water and chemical in soil. Soil Science. 1997;162:534–543. [Google Scholar]
- Cudlín P, Kieliszewska-Rokicka B, Rudawska M, et al. Fine roots and ectomycorrhizas as indicators of environmental change. Plant Biosystems. 2007;141:406–425. [Google Scholar]
- Dalton FN. In situ root extent measurements by electrical capacitance methods. Plant and Soil. 1995;173:157–165. [Google Scholar]
- Dvorak M, Cernohorska J, Janacek K. Characteristics of current passage through plant tissue. Biologia Plantarum. 1981;23:306–310. [Google Scholar]
- Frensch J, Steudle E. Axial and radial hydraulic resistance to roots of maize (Zea mays L.) Plant Physiology. 1989;91:719–726. doi: 10.1104/pp.91.2.719. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Geddes LA, Baker LE. Principles of applied biomedical instrumentation. 3rd edn. New York: John Wiley and Sons; 1989. [Google Scholar]
- Grimnes S, Martinsen OG. Bioimpedance and bioelectricity basics. 2nd edn. San Diego: Academic Press; 2008. [Google Scholar]
- Hirano Y, Dannoura M, Aono K, Igarashi T, Ishii M, Yamse K, Makita N, Kanazawa Y. Limiting factors in the diction of tree roots using ground-penetrating radar. Plant and Soil. 2009;319:15–24. [Google Scholar]
- Majdi H. Root sampling methods: applications and limitations of the minirhizotron technique. Plant and Soil. 1996;185:255–258. [Google Scholar]
- McBride R, Candido M, Ferguson J. Estimating root mass in maize genotypes using the electrical capacitance method. Archives of Agronomy and Soil Science. 2008;54:215–226. [Google Scholar]
- Ozier-Lafontaine H, Bajazet T. Analysis of root growth by impedance spectroscopy (EIS) Plant and Soil. 2005;277:299–313. [Google Scholar]
- Preston GM, McBride RA, Bryan J, Candido M. Estimating root mass in young hybrid poplar trees using the electrical capacitance method. Agroforestry Systems. 2004;60:305–309. [Google Scholar]
- Rajkai K, Vegh KR, Nacsa T. Electrical capacitance of roots in relation to plant electrodes, measuring frequency and root media. Acta Agronomica Hungarica. 2005;53:197–210. [Google Scholar]
- Repo T. Influence of different electrodes and tissues on the impedance spectra of Scots pine shoots. Electro- and Magnetobiology. 1994;13:1–14. [Google Scholar]
- Repo T, Laukkanen J, Silvennoinen R. Measurement of tree root growth using electrical impedance spectroscopy. Silva Fennica. 2005;39:159–166. [Google Scholar]
- Repo T, Oksanen E, Vapaavuori E. Effects of elevated concentration of ozone and carbon dioxide on the electrical impedance of leaves of silver birch (Betula pendula) clones. Tree Physiology. 2004;24:833–843. doi: 10.1093/treephys/24.7.833. [DOI] [PubMed] [Google Scholar]
- Repo T, Zhang MIN, Ryyppö A, Vapaavuori E, Sutinen S. Effects of freeze–thaw injuring on parameters of distributed electrical circuits of stems and needles of Scots pine seedlings at different stages of acclimation. Journal of Experimental Botany. 1994;45:823–833. [Google Scholar]
- Repo T, Zhang G, Ryyppö A, Rikala R. The electrical impedance spectroscopy of Scots pine (Pinus sylvestris L.) shoots in relation to cold acclimation. Journal of Experimental Botany. 2000;51:2095–2107. doi: 10.1093/jexbot/51.353.2095. [DOI] [PubMed] [Google Scholar]
- Riddoch I, Letho T, Grace J. Photosynthesis of tropical tree seedlings in relation to light and nutrient supply. New Phytologist. 1991;119:137–147. doi: 10.1111/j.1469-8137.1991.tb01017.x. [DOI] [PubMed] [Google Scholar]
- Ryyppö A, Repo T, Vapaavuori E. Development of freezing tolerance in roots and shoots of Scots pine seedlings at non-freezing temperatures. Canadian Journal of Forest Research. 1998;28:557–567. [Google Scholar]
- Segal E, Kushnir T, Mualem Y, Shai Uri. Water uptake and hydraulica of the root hair rhizosphere. Vadose Zone. 2008;7:1027–1034. [Google Scholar]
- Steudle E, Peterson CA. How does water get through roots? Journal of Experimental Botany. 1998;322:775–788. [Google Scholar]
- van Beem J, Smith ME, Zobel RW. Estimating root mass in maize using a portable capacitance meter. Agronomy Journal. 1998;90:566–570. [Google Scholar]
- Walker JM. Electrical A. C. resistance and capacitance of Zea mays L. Plant and Soil. 1965;23:270–274. [Google Scholar]