Dynamics of lipid domain formation: Fluctuation analysis

Summary

Scanning-fluctuation correlation spectroscopy was used to detect sub resolution organizational fluctuations in the lipid liquid-crystalline phase for single lipid model systems. We used the fluorescent probe Laurdan which is sensitive to the amount of water in the membrane to show that there is a spatial heterogeneity on the scale of few pixels (the size of the pixel is 50 nm). We calculated the pixel variance of the GP function and we found that the variance has a peak at the phase transition for 3 different samples made of pure lipids. The pixel variance has an abrupt change at the phase transition of the membrane and then it slowly decreases at higher temperature. The relatively large variance of the GP indicates that the liquid phase of the membrane is quite heterogeneous even several degrees higher than the phase transition temperature. We interpreted this result as evidence of an underlying micro scale structure of the membrane in which water is not uniformly distributed at the micron scale. Imaging of these microstructures shows that the pixels with different GP tend to concentrate in specific domains in the membrane. In the case of single lipid membrane, the statistical and fluctuation analysis of the GP data shows that even such simple lipid systems are capable of generating and maintaining stable structural and organizational heterogeneities.

Introduction

Lipid molecular interactions have been the subject of extensive biophysical investigation due to their relevance in the understanding of functioning and dynamics of biological membranes. It is now established that in addition to their structural role, lipids and their interactions with other lipids, peptides and molecules are crucial for many biomembrane functions, such as signaling, homeostasis, and cell adhesion [1–3, 4]. Fluctuations in lipid organization have been associated with the concept of lipid rafts. Recent investigations using fluorescence based techniques such as single particle tracking, confocal and STED Fluorescence Correlation Spectroscopy, Foster Resonance Energy Transfer have revealed that lipid phase domains in biological membranes are small and transient in nature [5–9]. For this reason high spatial and temporal resolution is needed for these studies. Fluorescence Correlation Spectroscopy has been used to gather quantitative local information on lipid domain/rafts diffusion and protein partition into lipid phases in both model and biological membranes. However, the spatial correlations of these fluctuations have not been studied in detail.

From a biophysical point of view, spatial and temporal variations in lipid organization lead to local heterogeneity in the physicochemical properties of the membrane such as electric potential, viscosity and hydration. These parameters determine membrane structure, ion trafficking, correct protein folding, interactions, diffusion, and insertion in the membrane and therefore play a very important role in the functioning of biomembranes. Of particular interest is the phase behavior of both biological and model membrane as it not only affects the biophysical properties of the membrane, but also directly reflects the interactions of lipid molecules with each other and with the surrounding solvent.

Due to the complexity of biological membranes, model membranes have been used to mimic the structure of biological membranes while providing a chemically and biophysically controllable system. Model systems are extremely valuable for the study of lipid interactions and membrane biophysical properties. Each particular model system has advantages and disadvantages and could be more or less adequate to answer specific questions. Among the available model membrane systems, Giant Unilamellar Vesicles (GUVs) are extremely useful for light microscopy experiments due to their cell-like geometry and size which allows direct visualization of extended membrane portions at sub micron resolution under the microscope. Moreover, these systems provide a free standing bilayer thus eliminating possible artifacts due to the interaction with the substrate observed in supported bilayers, which can hinder investigation of processes such as lipid phase transition and lipid diffusion [10]. The use of GUVs has allowed visualizing lipid phase separation for a variety of lipid mixtures and has provided valuable information on binary and ternary mixtures phase diagram, tie lines, miscibility critical points [11, 12]. As opposed to biological membranes, in GUVs the two leaflets of the bilayer have the same lipid composition and lipid phase domains have been shown to span both leaflet of the bilayer [13]. The problem of leaflet coupling is interesting especially in relation to signaling mechanisms [14–17], and the study of leaflet coupling in simple lipid systems can shed light on the possible molecular interactions at the basis of transmembrane lipid coupling in biological membranes.

Despite the large number of studies about to lipid phase separation and phase transition in biological and model membranes at the steady state, a full understanding of the mechanisms at the base of lipid domain formation, phase fluctuations and leaflet coupling is still lacking even for simple system such as model lipid membranes. Although molecular dynamics simulations have been extremely useful in the study of this problem [18, 19], more experimental work is still needed. FRET studies on binary systems undergoing a sudden thermal quench by [20] gave indirect evidence of domain size growth until equilibrium was reached after hours. Fluorescence Correlation Spectroscopy (FCS) has been used to follow membrane dynamics as a function of sterol and sphingomyelin content [21, 22].

In this paper we discuss the experimental approach followed by our laboratory to study the spatio-temporal dynamic of lipid phase fluctuation and domain formation and its applications to the investigation of lipid organization in the liquid disordered phase. We exploited the spectral properties of the environmentally sensitive fluorescent probe Laurdan [23, 24] and fluorescence fluctuation analysis to follow the morphological and organizational development of lipid gel domains. Laurdan is sensitive to the polarity of the solvent surrounding it and in the case of lipid membranes it directly reports on the state of hydration of the membrane. This allowed us to study the membrane organization from the point of view of the water molecules hydrating the membrane which is directly related to the lipid density of particular interest.

The use of an environment sensitive probe, as opposed to dyes that partition in different phases, allows detecting fluctuations in the membrane physical organization independently from the local chemical composition and probe concentration and it is therefore needed for the investigation of fast transient phase fluctuations, especially in the case of single species lipid membranes. Most partitioning dyes only give a response depending on their concentration on a given phase. If the surrounding of the probe suddenly changes, the partitioning dyes must migrate to different locations before the change is reported by their change in local concentration. Instead, Laurdan has the ability to yield information on intermediate transient phase states as well.

Laurdan spectral response to lipid phase is quantified by the GP function introduced by Parasassi and Gratton [25–28] which has been widely used in microscopy to provide morphological and quantitative information on the membrane lateral organization [13, 25, 29–32]. In Laurdan GP imaging statistical analysis of the GP pixel distribution is used to obtain information on the local organization of the membrane. The GP average value reports on the level of packing of the lipids, while the width of the GP distribution is used as an indicator of the level of heterogeneity in the lipid organization as discussed by [25, 33]. In these two papers, the authors reported a widening of the GP distribution in the liquid-crystalline phase for single phosphocholine model membrane and proposed a model based on the presence of water cavities of different sizes at the membrane glycerol backbone to explain the finding. This model, while providing an explanation for the broader GP distribution at the molecular level, does not exclude the presence of larger supermolecular lipid organizational fluctuations in the lipid liquid- crystalline phase. Indirect experimental evidence for such fluctuations was reported by [34] in a study of diffusion of beads on 14:0 14:0-PC (DMPC) free standing bilayer. Due to the transient nature of the lipid phase fluctuations in the lipid fluid phase at the onset of phase transition, high temporal resolution is necessary. To detect both spatial and temporal GP correlations between different locations on the membrane with high temporal resolution, we performed circular scanning experiments. In these experiments a region of the membrane is scanned by a laser beam moving along an orbit in the plane of the membrane while data are acquired. Circular scanning measurements provide higher temporal resolution than raster-scan imaging while still retaining spatial information which is lost in single point fluorescence correlation spectroscopy (single point FCS) measurements. Correlation analysis of the GP signal reveals the presence of stable lipid phase heterogeneity in both single lipid (this paper) and binary model systems in the liquid-crystalline phase [43]. In the case of binary mixtures displaying phase separation, the analysis of the fluctuations of Laurdan GP along a line spanning different domains revealed the spatial correlations of the fluctuations and the movement of the borders between domains [43].

LAURDAN

The use of fluorescence microscopy in membranes studies allows performing fluorescence spectroscopy measurements while providing high spatial and temporal resolution of membrane morphology and lipid molecular behavior (lipid motility, hydration, local viscosity etc.) at the single “vesicle” (or cell) level.

Many of the fluorescence microscopy studies of lipid domain morphology and dynamics employ fluorescent dyes that preferentially partition in one of the lipid phases. These dyes have been employed to gather visualization of membrane domain morphology that complements the thermodynamic information obtained in classical bulk studies and it has been used to build phase diagrams for several binary and ternary lipid mixtures [11, 12, 35], and to detect nanodomains in model membranes using FRET [6, 8, 36, 37]. This approach, however, does not directly provide information on the local fast dynamics of membrane domains but rather on the local chemical environment of the lipid domains [38].

Environmentally sensitive dyes, on the other hand, allow direct investigation of the biophysical properties of the membrane. Initially designed by Gregorio Weber, Laurdan belongs to a class of fluorescent dyes – including Prodan, Prolan, Danca etc. [31, 39]- used to study the phenomena of fluorophores relaxation in polar solvent [23, 24]. Several properties of this dye make it a perfect tool for lipid phase behavior studies. First of all, its chemical structure (a fluorescent moiety attached to a 12 carbon hydrophobic tail) comports low solubility in water and high affinity for lipid membranes thus minimizing background fluorescence.

Laurdan location in the membrane

The location of Laurdan in the lipid membrane is another useful property for the visualization of lipid phase domains. The 12 carbon hydrophobic chain of the dye aligns parallel to the hydrophobic lipid tails anchoring the fluorescent moiety of the probe at the lipid glycerol backbone, with the dye excitation dipole oriented perpendicular to the membrane plane. In the gel phase, the tight packing causes an almost perfect alignment of the Laurdan transition dipole perpendicular to the vesicle surface. If the membrane is oriented parallel to the plane of light polarization, lipid gel phase domains can be identified as regions of low or nil fluorescence intensity. This property is usually referred as photoselection effect.

Spectral behavior

Both Laurdan excitation and emission spectra are sensitive to the polarity of the environment. In solvents of higher polarity, such as water, both the emission and excitation spectra of Laurdan are red shifted [26]. In aqueous environment, the dye excitation occurs on a time scale much shorter than that of the dipolar relaxation of the surrounding water molecules. When the excited state is reached, part of the excited dipole energy is used to realign the surrounding water molecules, until the dipole decays to the ground state emitting the fluorescence signal. During the lifetime of the excited state, dipolar relaxation changes the average emission wavelength of Laurdan [23, 24]. In lipid membrane, the spectral behavior of Laurdan reflects the extent of the dipolar relaxation of water molecules surrounding the dye fluorescent moiety and it is therefore strongly dependent on the lipid phase. The dye excited state lifetime, in fact, is on the same order of the relaxation times of the water molecules penetrating to the glycerol backbone and the trans-gauche isomerization of the lipid acyl chains. In the gel phase the emission spectrum is centered at 440 nm and at 490 nm in the liquid crystalline phase. This is a consequence of the difference in water penetration in the membrane between the gel and liquid crystalline phases.

Partition in lipid phases

Laurdan partitions equally in liquid crystalline and gel lipid phases. This represents an advantage over partitioning dyes whose fluorescence properties are insensitive to the membrane phase state, as the dye spatial distribution is sufficient to determine the lipid phase. It is important to point out that Laurdan spectral behavior and photoselection effect are consequence of two independent properties of the probe (its fluorescent moiety and orientation in the membrane respectively) and therefore report independently on two different aspects of the lipid organization.

The use of Laurdan in microscopy studies was made possible by the introduction of two photon excitation. Thanks to the Laurdan spectral sensitivity to lipid phases, microscopy measurements using this dye only require the use of two spectrally resolved channels in the emission path. Two band pass filters centered at the two emission maxima of Laurdan in the lipid gel and liquid-crystalline phases, 440nm and 490nm respectively, are generally used to detect the lipid phase related dye spectral changes.

Generalized Polarization

GP function

In order to quantify the lipid phase dependent Laurdan spectral shift, Parasassi et al. introduced the Generalized Polarization function [40] defined as:

$$\mathit{\text{GP}}=\frac{I_{440\mathit{\text{nm}}}-I_{490\mathit{\text{nm}}}}{I_{440\mathit{\text{nm}}}+I_{490\mathit{\text{nm}}}}$$ (1)

where I_440nm and I_490nm are the fluorescence intensities at 440 and 490 nm respectively. High GP values correspond to the scarcely hydrated lipids in the gel ordered phase at low temperatures and low GP values to lipids in the liquid-crystalline phase at high temperature.

The Laurdan GP function has been used in both steady state and time-resolved studies on lipid phase behavior and especially phase coexistence. Time resolved cuvette studies on lipid binary mixtures [27] [39] pointed out the presence of lipid phase separation as seen by the fast interconversion rates of Laurdan molecules between the two phases, which is on the order of 40–100 ns. These studies also showed that vesicles composed by binary lipid mixtures in the phase coexistence region do not display the properties expected from the simple addition of the two lipid phase. Rather, one phase influences the other.

Almost ten years later, GP microscopy measurements allowed direct visualization of lipid phase domains on GUVs [29] composed of binary and ternary lipid mixtures of defined composition. In microscopy experiments using GUVs, a portion of the vesicle membrane is imaged and a GP image is constructed from the intensities collected in the two channels thus providing immediate visualization of the membrane local phase state. A GP pixel distribution can be calculated for specific Regions of Interest (ROI) on each image. The GP average value indicates the level of packing and therefore the phase of the lipids in the ROI, while the width of the GP distribution can be used as an indicator of the local heterogeneity in lipid organization (ref). GP imaging of membranes displaying phase separation combines morphological and organizational information thus providing valuable insight about the miscibility of the lipid components and its relation with the hydrophobic tails mismatch [38].

GP of coexisting lipid phases

The liquid crystalline and gel phases are characterized by specific GP values for each lipid species. Any intermediate value between these two extremes originates by either a homogeneous phase with intermediate packing characteristics or by the coexistence of lipid phase domains below the microscope spatial resolution (about 400 nm).

In the case of non interacting coexisting lipid phases the intensities in the blue and green channel can be written as:

$$\begin{array}{c}{I}^B=\alpha I_{\mathit{\text{gel}}}^B+\beta I_{\mathit{\text{liquid}}}^B\hfill \\ I^G=\alpha I_{\mathit{\text{gel}}}^G+\beta I_{\mathit{\text{liquid}}}^G\hfill \end{array}$$ (2)

where α and β are the fractions of lipid in the gel and liquid phases respectively. Assuming that α+β=1 (as we have for instance in cuvette experiments), we have that:

$$\mathit{\text{GP}}=\frac{\alpha S_{\mathit{\text{gel}}}·{\mathit{\text{GP}}}_{\mathit{\text{gel}}}+(1-\alpha )S_{\mathit{\text{liquid}}}·{\mathit{\text{GP}}}_{\mathit{\text{liquid}}}}{\alpha S_{\mathit{\text{gel}}}+(1-\alpha )S_{\mathit{\text{liquid}}}}$$ (3)

where GP_gel and GP_liquid are the GP values typical of the gel and liquid – crystalline phases and S_gel and S_liquid are the sum of the green and blue intensities in the gel and liquid-crystalline phases respectively. In cuvette experiments, where the measurement is the result of an average over the whole sample αS_gel + (1 − α)S_liquid = I_t =constant, (where I_t is the total intensity collected in the two channels), and the GP has a linear dependence on α. In the case of measurements on single GUVs, however, only one section of the membrane is imaged and, due to the photoselection effect, the denominator in eq 3 is not constant. In particular, on the polar region of the vesicle, a lower total intensity will be observed in the lipid gel phase due to the alignment of the dye molecules perpendicularly to the light polarization plane. An exhaustive discussion on the Laurdan intensity and GP variations in the different lipid phases on different regions of the GUVs can be found in [38]. The relationship between lipid alignment (as detected by the fluorescence intensity) and lipid packing (as detected by the GP) can be exploited to gather information on the dynamics of lipid domain formation and leaflet coupling.

From eq. 2 we have that: (In all following formulas we change the subscript gel and liquid with the single letter g and l, respectively)

$$\alpha =\frac{I^G-I_l^G}{I_g^G-I_l^G}$$ (4)

Note that, in this case, I_g/l^B and I_g/l^G represent the intensity in the blue and green channel respectively of a pixel in the pure gel and liquid phase respectively.

Substituting this expression for α in equation 4, we find an expression for the GP as a function of the intensity in the green channel:

$$\mathit{\text{GP}}=\frac{I^G(I_g^B-I_l^B-I_g^G+I_l^G)-(I_l^G{I}_g^B-I_g^G{I}_l^B)}{I^G(I_g^B-I_l^B+I_g^G-I_l^G)-(I_l^G{I}_g^B-I_g^G{I}_l^B)}$$ (5)

Eq. 5 provides an analytical expression that links the GP to the fluorescence intensity in each of the channels in the case of coexisting non interacting lipid phases.

Notice that, in this case, I^B_g/l and I^G_g/l represent the intensity in the blue and green channel of a pixel in the pure gel and liquid phase, respectively. From the experimental data in the limiting conditions in which we have a pure gel phase and a pure liquid phase we estimate the values of I^B_g/l and I^G_g/l. By inversion of Eq. 5 we can link a given pair of GP and I^G to the fraction of molecules in the gel phase.

2D histograms

To estimate the unknown parameters in Eq. . 5 I^B_g/l and I^G_g/l, and to calculate fraction α of gel phase in a pixel using Eq 4; we construct a 2D histogram from the imaging data which represents how many points in the data set have a given value of GP and intensity in one of the two channels. This type of representation allows for the investigation of the relation between the packing of the lipids (as reported by the GP) and the level of alignment of the lipid (as reported by the fluorescence intensity in each channel which is related to the alignment of the Laurdan excitation dipole). In fact, if the lipids are perfectly aligned (Laurdan is aligned along the lipids) the fluorescence intensity (not the GP) will be low, because photo-selection forbids excitation of these molecules that are aligned perpendicular to the bilayer surface, when we illuminate the top or the bottom of the GUV.

Note that the 2D histograms represent a 2D distribution of the data point in the GP/intensity space. We developed a “best score” method which allows comparing the experimental 2D distribution to the two-state approximation curve of Eq. 5. The parameters of Eq. 5 are entered in the data analysis program and the GP versus green (blue) intensity is calculated using Eq. 5. A score, determined by the number of points at a user-determined distance from the curve, is calculated by the data analysis program. The unknown parameters are manually varied until the best (highest) score is obtained. There are four unknown parameters in Eq. 5. However, they are linked by the condition that the GPs of the liquid and gel phases are equal to a specific value determined separately for the pure gel and the pure liquid samples. This approach was use to study the dynamics of leaflet coupling and lipid phase interactions in the case of binary lipid mixtures [41].

Circular scanning

The use of circular scanning allows higher temporal resolution than imaging while still providing spatial information. Circular scanning data provide visual information on the lipid phase state, while allowing the use of fluctuation correlation analysis to detect average domain diffusion, size and number. Experimentally, scanning FCS, which was introduced in microscopy by [42], was used to circumvent the shot noise problem. It involves collecting the data in periodic fashion. This method has proven very useful for membrane studies due to the necessity of physically scanning the lipid membrane as quickly as possible, thus giving a good blend of both spatial information and temporal resolution. Moreover, the fast scanning of the laser beam on the membrane minimizes artifacts due to photobleaching of the dye, which are particularly vexing in single point FCS on 2 dimensional membranes [22, 43, 44].

In circular scanning mode, the laser beam is scanned in a circular orbit on the sample while the fluorescent signal is collected in the two channels at a given sampling frequency. In the case of random diffusion of particles in 2D and of a 3D Gaussian PSF, the autocorrelation function of the signal can be expressed as the product of two terms: the diffusive term G(τ), and the scan envelope S(τ) [42].

$$\begin{array}{c}{G}_s(\tau )=S(\tau )\times G(\tau )\hfill \\ S(\tau )=\text{exp}\left(\frac{-4A^2(1-\text{cos}(\omega \tau ))}{(1+(8D\tau /w_0^2))w_0^2}\right)\hfill \\ G(\tau )=\frac{\gamma }{N}{\left(1+\frac{4D\tau }{w_0^2}\right)}^{-1}\hfill \end{array}$$ (6)

Where D is the diffusion coefficient of the particles, w_0,z are the radial and axial shape factors of the PSF respectively, γ is a factor which depends on the excitation volume and N is the number of molecules in the excitation volume. We see that equation 6 contains information on the spatial correlation as well. In the case of no diffusion (D=0), the shape of S(τ) will depend on the spatial correlation between the position of the PSF on the orbit. The mathematical basis for the separation of the temporal and spatial information from scanning measurements are discussed in [45, 46]. In the two papers the authors derive the mathematical form of the temporal and spatial autocorrelation functions expressed in terms of the temporal and spatial increments in the case of linear scanning of free diffusion of fluorescent molecules.

GP autocorrelation function

Fluctuation correlation spectroscopy (FCS) is a non-perturbative method used to gain molecular information from stochastic processes such as Brownian motion. Usually this technique is applied to fluorescence signals. FCS has been extensively employed to study diffusion of single fluorophores and fluorescently labeled lipid domains on model and biological membranes [21, 47]. Circular Scanning FCS has been used to study the dynamics of lipid-protein interactions on model membranes [44]. Recently, the combination of FCS and STED has revealed a cholesterol dependent heterogeneous diffusion of sphingomyelin fluorescent analogs in biological membranes.

Fluorescence Correlation analysis can be applied to any fluctuating signal, including the generalized polarization (GP) function. The autocorrelation curve is a representation of the self-similarity of a signal at some time point with itself at some time τ later. The equation can be written as:

$$G(\tau )=\frac{<S(t)S(t+\tau )>}{<S(t){>}^2}-1$$ (7)

where S(t) is the signal intensity at time t, τ is some absolute time separation, and the angle brackets denote a time average. In this equation, as τ approaches infinity, for a stochastic, stationary system, the numerator approaches the product of the averages, and therefore G(τ) approaches zero. Note that the analytical form of the autocorrelation function given in eq (6) makes the assumption that the fluctuating entity is small compared to the observation volume. Of course this could not be true for the fluctuations of GP, which could extend for sizes comparable or larger than the illumination volume.

The two main components of a correlation curve are its amplitude, G(0), and average decay time,τ. The average decay time yields the time scale of the fluctuation process(es). Strictly speaking, the G(0) term contains the detector noise. Therefore it is customary in FCS to use the extrapolated (to 0) value of the G(τ). The extrapolated G(0) is inversely proportional to the number of fluctuating ‘entities’. In the case of fluorescence the entities are dye molecules. The G(0) is depressed for decreasing signal to background percentage. The analysis of the G(0) in the case of the GP autocorrelation curves, is particularly challenging as the average GP value can be a small number (depending on the lipid phase for instance). Note that in the GP case a small average value does not imply a poor signal to noise ratio, but a specific property of the studied system. When the GP average value is close to zero, we will therefore observe a large extrapolated G(0) which does not necessarily reflect a physical number fluctuation. In the case of circular scanning data, the autocorrelation function has the typical oscillating structure [42, 48] where the decay of the periodic peaks indicates the temporal decay of the correlation while the shape of the peaks reflects the presence of structures in the scanned orbit. Therefore the GP-autocorrelation curve calculated from circular scanning data contains both spatial and temporal information. The mathematical basis for the separation of the temporal and spatial information is discussed in the literature [45, 46]. We have used the GP autocorrelation curves as a qualitative indicator of the self similarity of the GP signal to study the dynamics of domain formation in model lipid membranes [41]. For GP fluctuations, as well as for intensity fluctuations the initial amplitude of the autocorrelation curve (G(0)) is related inversely to the number of objects (lipid phase domains) fluctuating on the scanned orbit. For the GP fluctuations (but not for intensity fluctuations) the extrapolated value of G(0) is directly related to the contrast between the GP signal from the domains and the background. The shape (particularly the width) of the first decay (of the scanning FCS curve) yields information on the characteristic size of the structures in the scanned orbit. i.e., we make no assumption about the size of the fluctuating object.

Lipid phase fluctuations

GP imaging and correlation analysis has been employed by our group to investigate the dynamics of lipid phase organization, using single lipid and binary systems [41]. Lipid phase fluctuations were induced by driving the system trough the L_α to L_β phase transition by varying the temperature. Particular interest was devoted to lipid phase fluctuations in the liquid crystalline phase, to the dynamics of leaflet coupling and the level of interaction of the two lipid phases with each other.

Dynamic of lipid domain formation

A DPPC:DLPC 1:1binary mixture was used in order to amplify the lipid phase fluctuations and the GP signal to noise ratio as the DPPC phase transition (41 °C) temperature was approached as described in [41]. The DPPC:DLPC 1:1 mixture has been well studied and characterized. Work by [29] using Laurdan and [47] using DiI-C20 and Bodipy have shown that below the phase separation temperature, large, interconnected, linear-shaped gel domains exist on the membrane. The domain visualization using partitioning dyes shows chemical segregation of the two species, while the Laurdan GP measurements show high level of alignment and tight packing of the lipids in the gel phase. Both studies have shown that the gel domains span both leaflets of the bilayer. The linear shape of the domains and the relatively small difference in the GP of the gel and liquid domains indicates a low line tension between the two phases which is related to the small mismatch of their hydrophobic tails.

Above the phase separation temperature (41 °C), both the GP and intensity traces acquired by circular scanning measurements appear homogeneous. GP correlation analysis of data collected before the onset of phase separation show the presence of micron scale correlation which increases in size (from about 1 µm at 45.6 °C to 8 µm at 41.2 °C) and temporal stability as the temperature is decreased towards the DPPC phase transition. The fact that while the GP correlation analysis shows micron size correlation, no GP domain larger than the PSF is observed above the DPPC main phase transition suggests the presence of sub diffraction lipid domains that then coalesce to form larger domains. For this binary mixture, phase separation occurs once the system is left to equilibrate at a temperature of 41 °C. Laurdan GP imaging and GP correlation analysis shows that phase separation occurs by formation of sub micron sized domains which increase in average number and size up to few microns and then coalesce to form larger linear shaped domains [41]. Domain diffusion is observed in the initial stages of phase separation and is concurrent with an increase in the average number of gel domains. After one hour from the onset of phase separation, the system reaches equilibrium; the domains become interconnected and their motion stops.

2D histogram analysis of these data was used to calculate the average fraction of lipids in the gel phase and gather information on the dynamics of leaflet coupling and of interaction between the two lipid phases. This analysis revealed that while leaflet coupling starts already 2 minutes after the onset of phase separation and it is completed after 5 minutes, domains that are confined to only one leaflet of the bilayer exist up to 20 minutes after the onset of phase separation, at which time the lipid molecules in the gel phase become perfectly aligned in both leaflets. This analysis also shows that in the initial stages of phase separation the two lipid phases follow a two state model, i.e. the two lipid phases do not influence each other. Only after the domains became interconnected and linear in shape, deviation from the two-state model is observed suggesting that pools of DLPC have been entrapped by the coalescing gel domains which induce alignment in the DLPC molecules while not excluding water from the membrane. While these studies show the existence of different coexisting phases at temperatures below the main phase transition of DPPC, it is unclear whether this phase separation could be observed in single lipid vesicles.

MATERIALS AND METHODS

Materials

1,2-Dipalmitoyl-3-sn-glycero-phosphocholine (DPPC), 1,2-Dimerystoiyl-3-sn-glycero-phosphocholine (DMPC) and 1,2-ditridecanoyl-3-sn-glycerophosphocholine (DEPC) dissolved in chloroform were purchased from Avanti Polar Lipids (Alabaster, AL). Laurdan in powder was purchased from Molecular Probes (Eugene, OR).

Two-photon fluorescence microscopy

The microscope setup is the same used in reference [43]. Briefly, the microscope is home-built around the body of an Axiovert microscope (Carl Zeiss, Jena, Germany). The laser source is a tunable mode-locked Titanium-Sapphire laser (model No. 900, Mira, Coherent) pumped by a 10W Verdi (Coherent, Santa Clara, CA). The excitation wavelength was set at 780 nm. A quarter-wave plate was used to circularly polarize the excitation light. The power measured before the laser enters the microscope was 70 mW. The estimated power at the sample was about 10 mW. The beam is scanned in a circular orbit using a sine and a cosine waveforms for the x and y axis, respectively by a set of galvanometric mirrors. A dichroic mirror reflects the excitation light into a 0.4 N.A. air objective (LD Achroplan R, Carl Zeiss). The waist of the point spread function (PSF) of the system was measured to be 0.6 µm. The emitted fluorescence light is splits in two channels centered at 446 nm with a bandwidth of 50nm and at 499 nm bandwidth of 46 nm and detected by two photomultiplier tubes. The data from the two channels are collected and stored in a PC. The data acquisition/analysis program SimFCS (http:// www.lfd.uci.edu/) controls the motion of the mirrors and the integration time of each data point in addition to processing and analyzing the data in real time. For the circular scanning measurement, the center of the orbit was placed at the top of the GUV. The radius was 2 µm for a total length of the orbit of 12.5 µm. The orbital period was 1 ms and 256 points were acquired per orbit.

Sample preparation

The electro formation method was used to generate the GUVs as previously described in [43]. Briefly, about 3 ml of a 0.2 mg/ml solution of lipids in chloroform were spread on each of two platinum electrodes of the electroformation chamber and the excess chloroform was removed using a N2 gas stream. The chamber is then filled with deionized nanopure water and then the electric field is applied until the GUVs have formed. Since the GUVs are still attached to the wire in the chamber, their movement is inhibited.

Results

GP fluctuation analysis reveals lipid organization heterogeneity in the lipid liquid crystalline phase in single lipid and binary systems

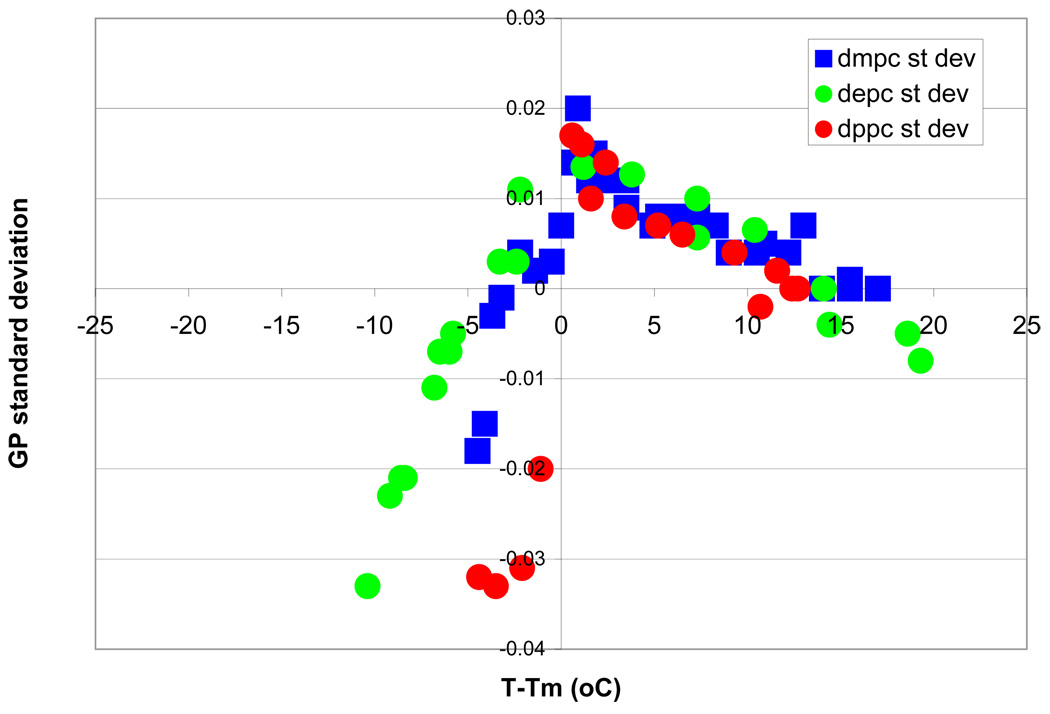
GP imaging measurements of the lipid liquid crystalline phase reveal a broader GP distribution than that observed in the lipid gel phase [13, 25]. More specifically, a broadening of the GP distribution is observed as the system temperature is lowered towards the main lipid phase transition temperature independently of the specific lipid specie under investigation as shown in figure 1 where the variation of GP pixel standard deviation is plotted against the normalized temperature for three different phosphocholines: DPPC, DMPC and DEPC (16:0 16:0; 14:0 14:0; 13:0 13:0-PC respectively).

Figure 1.

Standard deviation behaviors for three lipid species. The change in GP standard deviation is plotted versus the normalized temperature for DPPC (red circles), DMPC (blue squares), DEPC (green circles). The three curves reach a maximum at the phase transition midpoint (T-Tm= 0).

Figure 1 illustrates how all three lipids studied (DMPC, DEPC and DPPC) have the same pre-transitional (0<T-T_m<5) behavior. In the first part of the cooling cycle, (T-T_m>0) the three curves lie on top of each other. In all three cases, a maximum is reached at T-T_m=0. As the temperature is further decreased (T-T_m<0), the standard deviation drops faster for the longer acyl chain lipid (DPPC) as a consequence of the higher cooperativity of the phase transition for longer chains molecules [49–51].

The GP distribution broadening towards the phase transition can be explained by the water cavity model proposed by [25]. This model is based on the existence of water cavities of different sizes at the membrane glycerol backbone in the liquid-crystalline phase at high temperatures. Small variations in the number of water molecules present at the glycerol backbone can determine significant variations in the emission of Laurdan, especially if the average number of water molecules is small. NMR studies show that the average number of free water molecules at the glycerol backbone of phosphocholine membranes is small and between 2 and 6 [52–55]. When the temperature is decreased towards the phase transition, the average number of water molecules surrounding the dye decreases due to the average increase in lipid density. This causes fluctuations in the emission spectra of Laurdan that are larger than those at high temperatures where the high average number of molecules at the glycerol backbone assures that dipolar relaxation occurs always.

GP-fluctuation analysis reveals stable organizational fluctuations in the liquid-crystalline phase

The water cavity model provides an explanation for the GP broadening on the molecular level, but does not exclude the presence of super molecular fluctuations in lipid organization close to the phase transition temperature. Circular scanning measurements with their high spatial and temporal resolution provide a useful tool to investigate the presence, size and stability of such fluctuations.

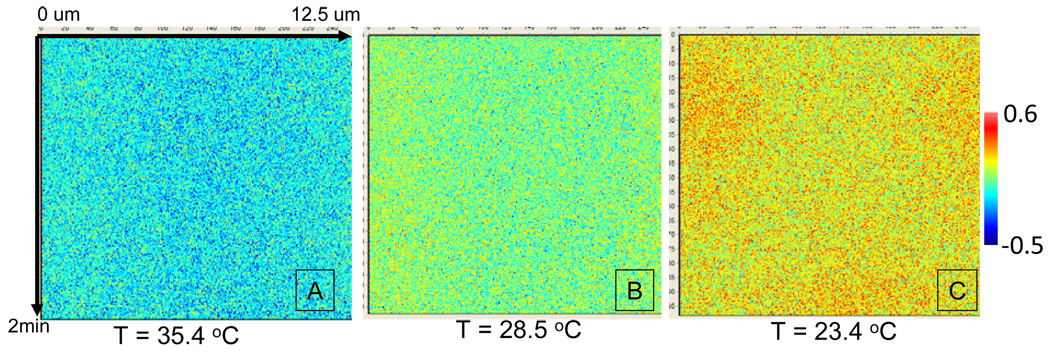
Circular scanning measurements performed on the polar region of single DMPC lipid GUVs showed the presence of micron sized spatial and temporal heterogeneity close to the main phase transition temperature as shown in figure 2. Micron sized areas of higher average GP are visualized on the GP traces as a function of space and time particularly in figure 2b and 2c.

Figure 2.

DMPC Circular scanning GP pseudo images at different temperatures. Each of these images represents the time evolution of the circular trajectory described by the laser beam. The horizontal axis in the different panels indicates the spatial extension of the orbit and the vertical axis represents the time. The image in c corresponds to the phase transition temperature for this mixture. In each image the pixel fluctuation and the large scale inhomogeneity can be sees. The color scale shows the range of the GP values.

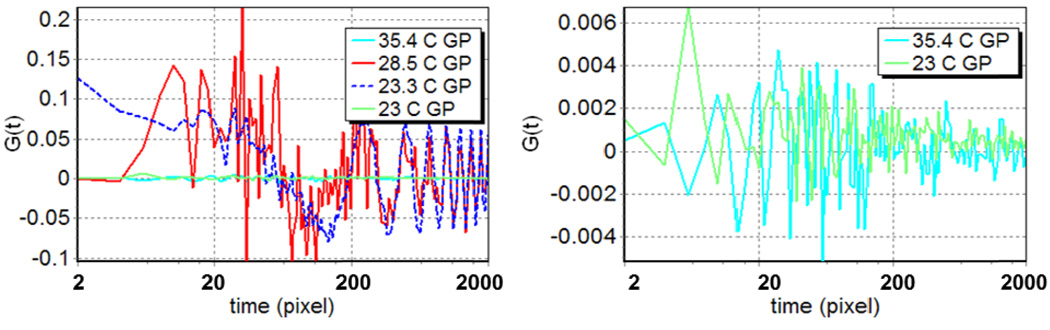
We calculated the circular scanning autocorrelation functions for the GP curves (Figure 3). We expressed the (spatio)-temporal coordinate in pixels. Temporally, one pixel corresponds to 100µs. In circular scanning FCS experiments, the spatial and temporal coordinates are linked as explained in [46]. Here the spatial increment between two adjacent pixels corresponds to a displacement of the center of the PSF of about 50 nm on the scanned orbit. In the autocorrelation curves calculated from the DMPC circular scanning data, the spatial information is contained in the first 256 points. Later times give information on the temporal evolution of the signal.

Figure 3.

GP autocorrelation functions. The curves in the graph were calculated from scanning data acquired on DMPC vesicles at different temperatures. The legend is shown on the right side of each figure. The image on the right side of the figure shows the detail of the high and low temperature GP autocorrelation curves in the left panel where we do not observe correlation.

The GP autocorrelation curves of points along the orbit show a dramatic difference between the data acquired at 35.4 °C and those acquired at 28.5 °C and lower (Figure 3). Already at 5 °C above the phase transition we observe a strong correlation at 400 nm (8 pixels), while no correlation is observed at higher temperatures. The general shape of the autocorrelation curve for the data acquired at the phase transition (23.3 °C) is similar to that at 28.5 °C. The two curves differ by the fact that the 28.5 °C curve appears to decay faster than the 23.3 indicating the presence of smaller structures at higher temperatures. An important characteristic of these two curves is that the amplitude of the periodic oscillations of the 28.5 °C curve decreases over time, while that of the 23.3 °C curve remain constant (Figure 3). This indicates that, at the phase transition the micron size structures are very stable and remain in the orbit path for all the acquisition time. Curves acquired immediately after the phase transition (at 23.1 and 23 °C) resemble those acquired at high temperature: i.e. do not show correlation (figure 3, right panel)

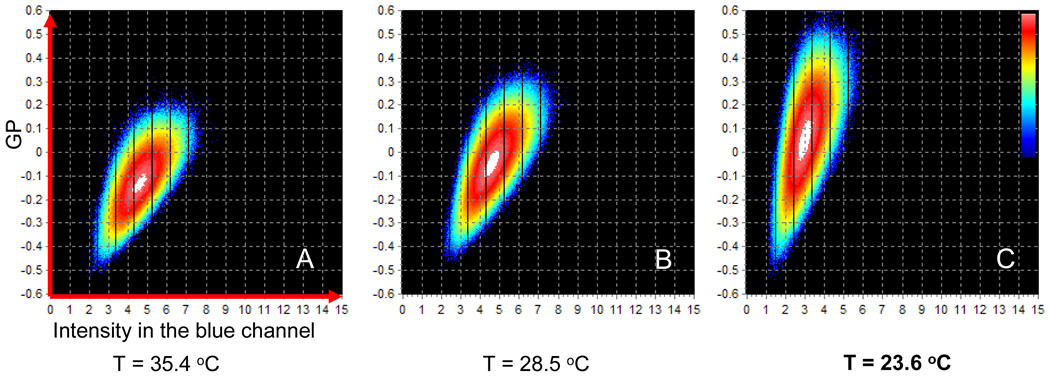
Figure 4 shows the 2D histograms calculated from DMPC circular scanning data in order to analyze the distribution of the GP value as a function of the intensity in the blue hannel. The distributions in figure 4 stretches towards higher values of GP as the temperature is decreased towards the phase transition temperature. This shows again an increase in the GP fluctuations near the phase transition. It is interesting that, while the average GP values increase as the phase transition is approached, we still find pixels with low GP values. Also, note how in all the 2D histograms in Figure 4 we find a direct proportionality between GP and blue intensity: high GP values are associated with higher blue intensity. This is expected if we consider the Laurdan spectral shift towards lower wavelengths in the lipid gel phase. However, as explained above, due to the fact that the lipids in the gel phase tend to be radially aligned, we could expect to observe high GP values to be correlated to photoselection effect, i.e. lower intensity on the polar region of the vesicle, as in the case of binary mixtures in the phase coexistence region reported in [41]. The absence of photoselection effect therefore implies a small degree of alignment also for the lipid molecules with higher GP.

Figure 4.

Time evolution of circular scanning 2D histograms of GP vs Intensity in the blue channel. As the temperature is decreased the center of the GP distribution changes from −0.13 at 35.4C to 0.05 at 23.6C. simultaneously with this change, the average intensity also decreases form 5.5 counts at 35.4C to 3 counts at 23.6C. The scale on the z-axes (frequency of counts) is logarithmic. The color scale is shown in image c.

As the phase transition temperature is approached, the slope of the 2D pixel distribution (defined as: (GP_max−GP_min)/(I_max−I_min)) becomes steeper (figure 4C) showing a gradual increase in the alignment of the higher GP areas. After the phase transition is reached, at temperatures lower than 23 °C the lipid molecules are better radially aligned in both leaflets of the bilayer and the fluorescence intensity signal drops drastically.

Discussion and Conclusions

The scanning-FCS methodology described in this paper allowed detecting sub resolution organizational fluctuations in the lipid liquid-crystalline phase for single lipid model systems. Heterogeneity on the micron scale is detected at about 5 °C above the phase transition temperature and can be directly visualized on GP pseudo image once the phase transition temperature is reached. These sub-micron size organizational fluctuations are never larger than two or three pixels (100–150 nm) at any temperature higher than the transition midpoint. This is true for both pixels with high GP (gel) and pixels with low GP (liquid disordered). The high GP pixels, although scattered in the whole pseudo image, tend to be concentrated in specific areas of the trajectory. We ascribe this observation to the cooperative nature of the transition: it is easier for the lipid molecules to assume a given configuration if the surrounding molecules are in that configuration. We conclude that the size of the cooperative unit does not increase above the size of the point spread function (about 600nm) at any temperature, as observed in the case of binary mixtures as well [43]. However, stable, macroscopic regions with different properties (different average lipid density) are formed by aggregation of sub micron sized objects. The difference in the average percentage of molecules in the gel phase can be calculated using eq 4. The difference in the fraction of molecules in the gel phase between the two regions calculated using equation 4 was only 7% on average. This, however, is a significant result which implies the presence of defined regions on the membrane with different characteristics. Moreover, the stability of such regions is very interesting from the biophysical point of view as it shows that basic structural properties of the lipid molecules can generate different environments on the membrane. From the autocorrelation curves we determine that long range organization exists away from the phase transition (blue curve in Figure 3). It is interesting that at every temperature higher than the phase transition we find pixels with GP values corresponding to the liquid-crystalline phase (<GP> = −0.5) in the GP pseudo images. We relate this observation to the presence of “pools” of water that remain in the membrane at any temperature higher than the phase transition.

In the case of single lipid membrane, the statistical and fluctuation analysis of the GP data shows that even such simple lipid systems are capable of generating and maintaining stable structural and organizational heterogeneities. It is plausible that the presence of sterols and surfactants serve mainly to modulate this inherent characteristic.

Abbreviations

DMPC

1,2 di-myristoyl phosphatidyl choline

POPC

1-palmitoyl-2-oleylphosphatidylcholine

DLPC

1,2 di-lauroyl phosphatidyl choline

DOPC

1,2 dioleylphosphatidylcholine

DPPC

1, 2-dipalmitoylphatidylcholine

PSF

point spread function