Discrimination of Intra- and Extracellular ²³Na⁺ Signals in Yeast Cell Suspensions Using Longitudinal Magnetic Resonance Relaxography

Abstract

This study tested the ability of MR Relaxography (MRR) to discriminate intra- (Na_i⁺) and extracellular (Na_e⁺) ²³Na⁺ signals using their longitudinal relaxation time constant (T₁) values. Na⁺-loaded yeast cell (Saccharomyces cerevisiae) suspensions were investigated. Two types of compartmental ²³Na⁺ T₁ differences were examined: a selective Na_e⁺ T₁ decrease induced by an extracellular relaxation reagent (RR_e), GdDOTP⁵⁻; and, an intrinsic T₁ difference. Parallel studies using the established method of ²³Na MRS with an extracellular shift reagent (SR_e), TmDOTP⁵⁻, were used to validate the MRR measurements. With 12.8 mM RR_e, the ²³Na_e⁺ T₁ was 2.4 ms and the ²³Na_i⁺ T₁ was 9.5 ms (9.4T, 24°C). The Na⁺ amounts and spontaneous efflux rate constants were found to be identical within experimental error whether measured by MRR/RR_e or by MRS/SR_e. Without RR_e, the Na⁺-loaded yeast cell suspension ²³Na MR signal exhibited two T₁ values, 9.1 (± 0.3) ms and 32.7 (± 2.3) ms, assigned to ²³Na_i⁺ and ²³Na_e⁺, respectively. The Na_i⁺ content measured was lower, 0.88 (± 0.06); while Na_e⁺ was higher, 1.43 (± 0.12) compared with MRS/SR_e measures on the same samples. However, the measured efflux rate constant was identical. T₁ MRR potentially may be used for Na_i⁺ determination in vivo and Na⁺ flux measurements; with RR_e for animal studies and without RR_e for humans.

Introduction

²³Na MR is the only technique that can potentially provide minimally invasive compartmental Na⁺ content measurement in intact organisms. Accurate in vivo intracellular Na⁺ concentration ([Na_i⁺]) measurement could provide a biomarker of cellular viability [1]. In the heart, it is known that [Na_i⁺] increases in ischemia [2; 3; 4] and, there is evidence that [Na_i⁺] increases in both hypertrophy and in failure [5]. This is important because [Na_i⁺] can have profound effects on cardiac function and arrhythmogenesis. In the brain, stroke increases total tissue [Na⁺] from values of less than 45 mM to approximately 70 mM [6]. Total tumor Na⁺ concentration was increased to 1.5 times that in contra-lateral normal brain [7]. A study by Sharma et al. of breast tumors employed an inversion recovery (IR) ²³Na MRI sequence to null or minimize signals with the larger T₁ values, which were presumed to be mostly ²³Na_e⁺[8]. The resulting image intensity therefore arose from magnetization with smaller T₁ values. The increased tumor ²³Na MRI intensity was thus attributed to increased ²³Na_i⁺.

The ²³Na_i⁺ and ²³Na_e⁺ resonances exhibit the same resonance frequency (ν) in biological samples. Some time ago, we and others introduced membrane impermeable extracellular shift reagents (SR_es) to selectively change (“shift”) the Na_e⁺ ν value [9; 10; 11; 12]. These SR_es were originally applied to study [Na_i⁺] in cell suspensions and perfused hearts [13; 14; 15]. There are two SR_e, DyTTHA³⁻ and TmDOTP⁵⁻, that have proven suitable for use in living animals[16; 17; 18; 19]. The use of ²³Na MRS with SR_e currently provides the best [Na_i⁺] measurements in isolated organs or intact animals. The prospects for the use of these SR_e in human studies are quite dim, however, because of the high doses required. This high [SR_e] requirement will probably remain even if new SR_es are developed.

The goal of this study was to develop and test MR methods for the measurement of Na_i⁺ that do not require SR_e. It is possible to discriminate cation resonances using their relaxation properties. This has been demonstrated for the longitudinal relaxation rate constant (R₁ = T₁⁻¹) values of ³⁹K⁺ resonances in perfused salivary gland [20] and in the isolated heart [21]. In those studies, the intrinsic T₁ values of the K_i⁺ and K_e⁺ resonances (8 and 68 ms, respectively; B₀ = 8.5T) were sufficiently different that inversion recovery (IR) pulse sequences could be used to selectively null the K_e⁺ resonances. Bi-exponential T₂ relaxation was selectively detected using a double quantum filter pulse sequence and this edited for the dog red blood cell ²³Na_i⁺ resonance [22]. These methods require that compartmental ²³Na R_x values (x = 1, 2) be sufficiently different. Alternatively, a (usually larger) R_x value difference can be induced with a relaxation reagent (RR). For example, Degani and Elgavish used GdEDTA⁻ as an extravesicular RR to measure equilibrium Na⁺ and Li⁺ transport kinetics across phosphatidylcholine vesicle membranes catalyzed by the ionophore monensin [23]. An IR pulse sequence was used to null the ²³Na_e⁺ or ⁷Li_e⁺ signal, which had a smaller T₁ value and observe mostly ²³Na_i⁺ or ⁷Li_i⁺, with the larger T₁.

The present study used ²³Na longitudinal (T₁) MR Relaxography (MRR) to discriminate and measure Na_i⁺ and Na_e⁺. T₁ relaxation decay data were subjected to an Inverse Laplace transform (ILT) (see Appendix 1 for a list of Abbreviation used) to produce a relaxogram, the apparent relaxation time constant (T₁′) distribution. The relaxogram can have multiple peaks, depending on the number of spin populations. Relaxation decay data were also analyzed using appropriate exponential functions. The ²³Na MRR measurements were validated with ²³Na MRS in the presence of SR_e. Na⁺-loaded yeast cell suspensions, which exhibit spontaneous Na⁺ efflux [13; 24], were used as model systems. Two methods for T₁ relaxographic ²³Na_i⁺ and ²³Na_e⁺ discrimination were investigated. The first employed GdDOTP⁵⁻ as an extracellular RR (RR_e) to selectively increase ²³Na_e⁺ R₁. The second used intrinsic ²³Na_i⁺ and ²³Na_e⁺ R₁ differences.

Results

Measurement of Na⁺-loaded yeast suspension Na⁺ content and Na_i⁺ efflux by ²³Na MRS with SR_e

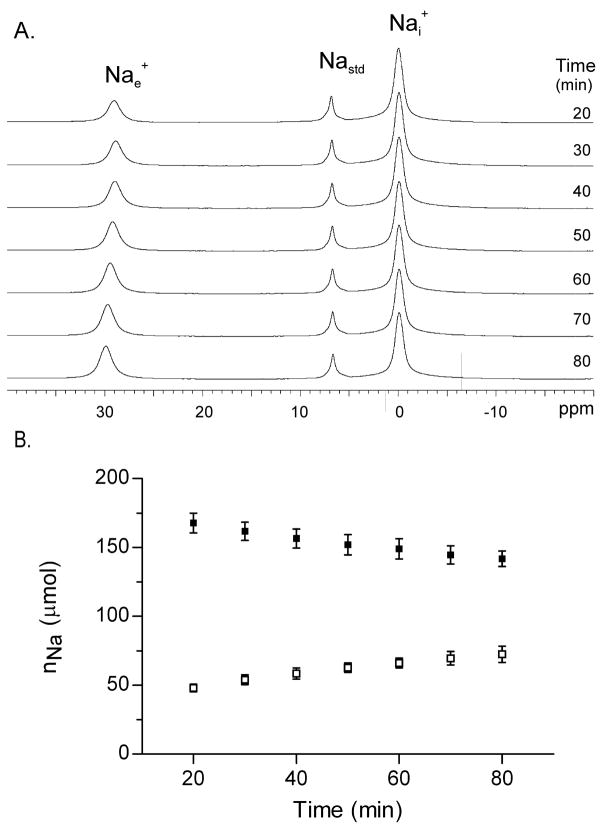
A stacked plot of ²³Na MR spectra from a Na⁺-loaded yeast suspension with 12.8 mM TmDOTP_e⁵⁻ is shown in Figure 1A. Inspection of the shifted ²³Na_e⁺ peak reveals an increase in the area and frequency with time after re-suspension. The increase in ²³Na_e⁺ peak area results from the slow Na_i⁺ efflux; the increase in ²³Na_e⁺ peak frequency may result from an uptake of divalent cations during the Na_i⁺ efflux. The n_Nai and n_Nae amounts were derived from the spectral peak areas (Figure 1B). The n_Nai decreased and the n_Nae increased with time.

Figure 1. Na⁺ efflux from Na⁺ loaded yeast ²³Na MR spectroscopy.

Panel A: Stacked ²³Na MR spectra obtained from a suspension of Na⁺ loaded yeast in which the SR_e, [TmDOTP_e⁵⁻] was 12.8 mM. The 3 ²³Na resonances are identified as follows (left to right) (1), the extracellular Na (Na_e⁺); (2), the Na standard (Na_std), which contains TmDOTP⁵⁻; and, (3), the intracellular Na (Na_i⁺).

Panel B: The Na⁺ contents, n_Nai (filled square) and n_Nae (open square), of suspensions of Na⁺ loaded yeast (n=4) measured by MRS with SR_e. Mean ± (SD) values are plotted.

Measurement of Na⁺-loaded yeast suspension Na⁺ contents and Na_i⁺ efflux by ²³Na T₁ MRR with RR_e

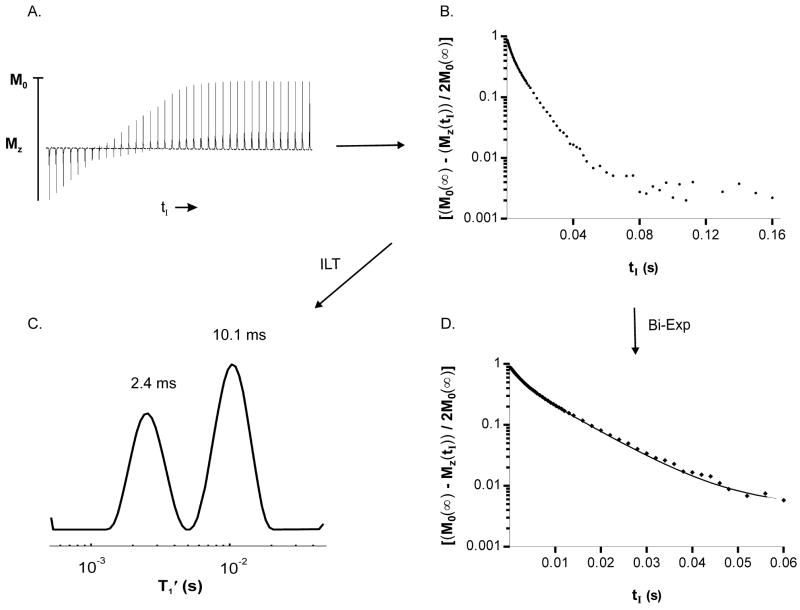
Figure 2 illustrates the T₁ MRR data acquisition and processing used in this study (Methods). The IR spectral peak areas (panel A) obtained from a yeast sample 74 minutes after suspension of the yeast in medium with 12.8 mM GdDOTP_e⁵⁻ recover with t_I. The IR peak area measurements plotted as the t_I -dependence of [(M_Z(∞) − M_Z(t_I))/(2M_Z(∞))] are shown in panel B. The ILT of this decay is the ²³Na T₁′ relaxogram (panel C), which exhibits two distinct T₁′ peaks, centered at 2.4 and 10.1 ms. With good quality relaxography the peak positions (T₁′) and areas, (a_i and a_e), are reasonably reliable. Because the peak widths are subject to ILT regularization effects they are not very physically meaningful. The peak widths do, however, absorb some of the errors of the ILT process [25; 26; 27; 28]. Artifactual relaxographic peaks, which occasionally are present at very small or very large T₁′ values, can distort a_i and a_e values. The t_I decay may also be fitted with an empirical bi-exponential (Bi-exp) function, (panel D) to determine a_i and a_e. The T′_1e and T′_1i values obtained from the relaxogram were entered into the Bi-exp expression (Methods) and fixed; only a_i, a_e, and noise constant C values were varied. Two linear segments are evident at t_I values less than 0.02 s (panel D). The small contribution at greater t_I values is almost exclusively from the noise term C. The a_i and a_e values obtained from such bi-exponential fittings more closely matched MRS/SR_e measured a_i and a_e values and are used in this report. The relaxogram aids in visualization.

Figure 2. ²³Na MRR data acquisition and processing.

Panel A:f A plot of 37 ²³Na IR spectra (of a total of 74 t_I values, 0.2 ms to 300 ms) acquired from a suspension of Na⁺ loaded yeast containing 12.8 mM [GdDOTP_e⁵⁻]; Panel B: A semi log plot of [(M_Z(∞) − M_Z(t_I))/2M_Z(∞)] derived from integration of the ²³Na yeast IR data; only the t_I values to 160 ms are shown. Panel C: The ILT of the [(M_Z(∞) − M_Z(t_I))/2M_Z(∞)] decay yields the ²³Na T₁ relaxogram, i.e. the T₁′ distribution with the Na_e T₁′ peak centered at 2.4 ms, a_e/(a_i + a_e) = 0.337 and the Na_i T₁′ peak centered at 10.1 ms, a_i/(a_i + a_e) = 0.663. Panel D: A semi log plot of [(M_Z(∞) − M_Z(t_I))/2M_Z(∞)] with a fit of a biexponential equation (Bi-Exp), a_eexp(−t_I/T′_1e) + a_iexp(−t_I/T′_1i) + C using the T₁′ values obtained from the relaxogram: a_e/(a_i + a_e) = 0.353 and a_i/(a_i + a_e) = 0.647; all t_I data were fit, only the t_I values to 60 ms are shown.

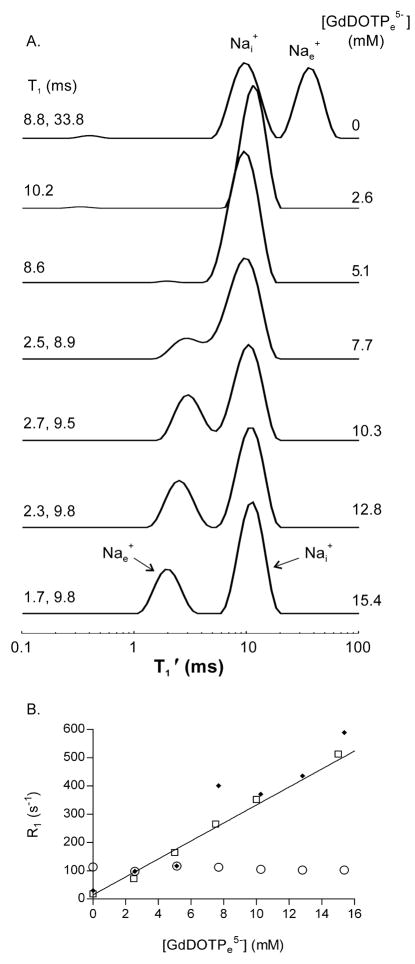
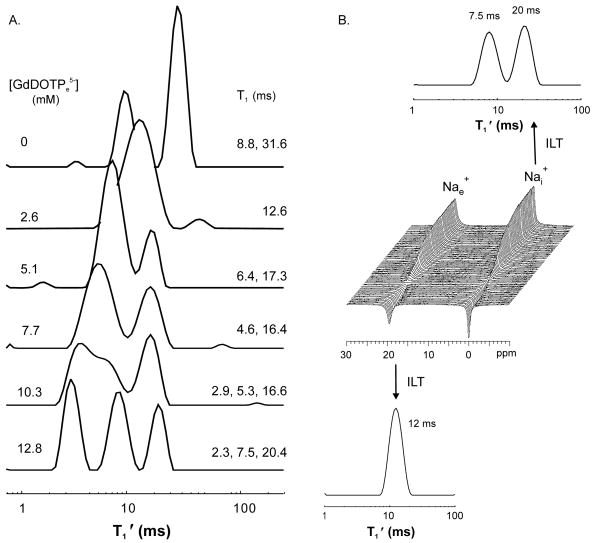
Figure 3A displays a stacked plot of relaxograms from a 50% wt/vol suspension of D273-10B Na⁺-loaded yeast with increasing [GdDOTP_e⁵⁻]. The [RR_e] values increase from top to bottom, and are given at the right sides of the relaxograms. One peak remains at ~10 ms in all relaxograms. Because the LnDOTP⁵⁻ complexes do not enter cells [15; 29], this peak is assigned to ²³Na_i⁺. The other peak moves continually to smaller T₁′ values with increasing [RR_e] passing through the 10 ms peak when [RR_e] is between 2 and 6 mM. By 7.7 mM [RR_e] two relaxographic peaks begin to re-emerge. With greater [RR_e] the resolution of these improves and the peak with the smaller T₁′ (eventually less than 3 ms) value is assigned to Na_e⁺. Whenever there are two distinct peaks in these relaxograms, the equilibrium transcytolemmal Na⁺ exchange system is in the slow-exchange-regime (SXR), the slow-exchange-limit (SXL), or the no-exchange-limit (NXL) condition. If one peak is present the system may be in the fast-exchange-regime (FXR) or the fast-exchange-limit (FXL) condition [30].

Figure 3. ²³Na T₁ MRR titration with [RR_e].

Panel A: A stacked plot of relaxograms obtained from Na⁺ loaded yeast suspended in medium with increasing concentrations of the extracellular relaxation reagent (RR_e) for Na⁺, GdDOTP⁵⁻. The [GdDOTP_e⁵⁻] are shown on the right; The T₁ values obtained for the peaks are shown on the left.

Panel B: The R₁ (= T₁⁻¹) parameters obtained from relaxographic peaks (Fig 2A) as a function of the [GdDOTP_e⁵⁻] are shown. The (open circle) reports the R₁ of the peak at T₁ ~ 9 – 10 ms, which is assigned to Na_i⁺; the (filled circles) report the R₁ of the peak assigned to Na_e⁺. The R₁ for a yeast cell free minimal medium solution containing 12.6 mM NaCl (open square) is also shown. The relaxivity of GdDOTP for 12.6 mM Na⁺ in cell free medium (^Nar_1CF ) obtained from the slope of the line fit to the R₁ was 31.8 (± 1.2) s⁻¹ mM⁻¹, R² = 0.99. The ^Nar_1CF was 24.9 (± 0.52) s⁻¹ mM⁻¹ in 50mM Na⁺ medium and, 17.9 (± 0.2) s⁻¹ mM⁻¹ for 150mM Na⁺ medium (not shown).

Figure 3B shows the relaxivity plot, R₁′ vs. [RR_e], for the yeast suspension of Fig 3A. The Na_i⁺ R₁′ (filled circles) is constant as [RR_e] increased, which is consistent with an SXL or NXL condition. The Na_e⁺ R₁′ (open circles) [RR_e] dependence is reasonably linear. The data for a cell-free (CF) minimal medium solution with 12.6 mM Na⁺ (open squares) is also shown. The data show the linear behavior expected: the Na⁺ + RR⁵⁻ ↔ NaRR⁴⁻ system is in the FXL. The slope of the straight line, 31.8 (± 1.2) mM⁻¹ s⁻¹, is the CF GdDOTP⁵⁻ relaxivity for ²³Na (^Nar_1CF). The RR relaxivities for water (r_1CF), although strictly proportional to [RR]/[H₂O], are effectively [H₂O] independent because of the very large H₂O concentration (~50 M). This is not the case for ^Nar_1CF values, which are quite dependent on both the [RR] numerator and the [Na⁺] denominator in [RR]/[Na⁺]. The ^Nar_1CF values for 50 and 150 mM Na⁺ in minimal medium are decreased to 24.9 (± 0.52) and 17.9 (± 0.2) mM⁻¹s⁻¹, respectively (data not shown). Furthermore, since the interaction of the RR anion with the Na cation is that of an ion pair, the ^Nar_1CF value is sensitive to the concentrations of competitive cations (e.g., Ca²⁺), anions, and the solution ionic strength. This is in addition to the B₀ and temperature dependences that affect r_1CF values. The general agreement of the R₁′ for yeast suspension Na_e⁺ and for Na⁺ in the CF minimal medium solution data is gratifying. The fact that the Fig. 3 Na_i⁺ T₁′ remains essentially [RR_e]-independent confirms the robust nature of the ILT and is encouraging for the general quantification of the MRR/RR_e approach.

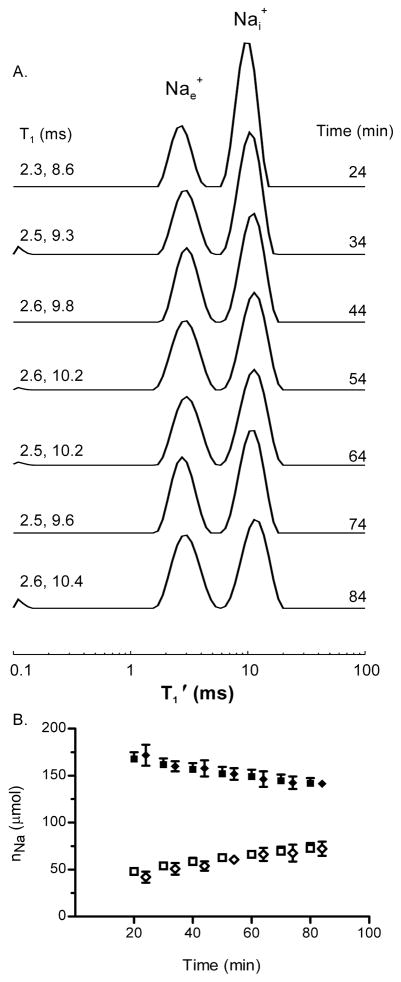
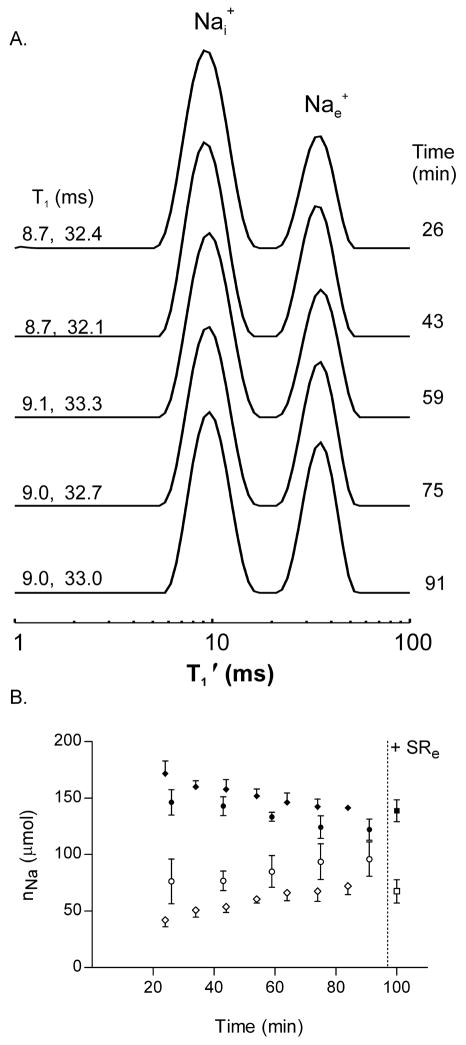
Figure 4 displays a stacked plot of sequential ²³Na T₁ relaxograms obtained from a yeast suspension containing 12.8 mM GdDOTP_e⁵⁻. A decrease of the relaxographic Na_i⁺ peak area and an increase of the Na_e⁺ peak area are evident over time. The n_Nai and n_Nae values are plotted (diamonds) in Figure 4B, with the MRS/SR_e n_Nai and n_Nae values (Fig. 1B) re-plotted (as squares) for comparison. The agreement is outstanding.

Figure 4. Na⁺ efflux from Na⁺ loaded yeast ²³Na T₁ MRR with RR_e.

Panel A: A stacked plot of Na⁺ T₁ relaxograms obtained from a suspension of Na⁺ loaded yeast with 12.8 mM [GdDOTP_e⁻⁵] in the medium. The T₁ values obtained for the relaxogram peaks are shown on the left, the elapsed time to the middle of the relaxogram acquisition is shown on the right.

Panel B: The time dependence of the Na⁺ contents, n_Nai (filled diamond) and n_Nae (open diamond), of suspensions of Na⁺-loaded yeast (n = 4) derived from the ²³Na MRR/RR_e measurements. Also shown for comparison are the MRS/SR_e measured n_Nai (filled square) and n_Nae (open square) content of suspensions of Na⁺ loaded yeast (Fig. 1B). Mean ± (SD) values are plotted.

Measurement of Na⁺-loaded yeast suspension Na⁺ contents and Na_i⁺ efflux by intrinsic ²³Na T₁ MRR

The potential for discrimination based on apparent intrinsic ²³Na_i⁺ and ²³Na_e⁺ T₁′ differences is apparent in the 0 mM RR_e relaxogram (Fig. 3A, top). ²³Na T₁′ relaxograms obtained from Na⁺-loaded yeast cells in RR_e-free minimal medium are shown in Figure 5A. The relaxogram peak assignments are reversed from those obtained with RR_e (Fig. 4A). These assignments for Fig. 5A are made on the basis of the RR_e titration (Fig 3A); and, on the basis of T₁ measurements made of the Na_i⁺ resonances, T₁′ = 9.5 ± (0.4 ms), made in the presence of SR_e (not shown). A temporal decrease of the Na_i⁺ peak area and an increase of the Na_e⁺ peak are evident.

Figure 5. Na⁺ efflux from Na⁺ loaded yeast intrinsic T₁ ²³Na MRR.

Panel A: A stacked plot of Na T₁ relaxograms of a suspension of Na⁺ loaded yeast is shown. No RR_e was added to the sample; the relaxogram peaks represent the distribution of intrinsic T₁ values in the sample. The T₁ values obtained from each relaxogram peak are shown on the left; the elapsed time to the middle of the relaxogram acquisition is shown on the right.

Panel B: The time dependence of the Na⁺ contents, n_Nai (filled circle) and n_Nae (open circle), of suspensions of Na⁺-loaded yeast (n=4) derived from ²³Na intrinsic T₁ MRR measures. At 97 min SR was added to the suspension and the MRS/SR_e measured n_Nai (filled square) and n_Nae (open square) values were obtained. For comparison the MRR/RR_e n_Nai (filled diamond) and n_Nae (open diamond) amounts (Fig 4B) are shown. All values are mean ± (SD).

The n_Nai and n_Nae values derived from intrinsic T₁ MRR are shown in Figure 5B (circles). Inspection reveals that the n_Nai and n_Nae amounts are different from those found in the other studies (Figs. 1B and 3B). To validate these ²³Na intrinsic T₁ MRR measurements, at 97 min SR was added to each suspension for measurements by ²³Na MRS (Fig 5B). The Na_i⁺ and Na_e⁺ MRS/SR_e a_i and a_e values report that the intrinsic T₁ MRR Na_i⁺ is 0.88 (± 0.06) of the MRS Na_i⁺ value; and, the MRR Na_e⁺ is 1.43 (± 0.12) of the MRS Na_e⁺ value. Thus, the Na_i⁺ amount is 12% too low and, the Na_e⁺ amount is 43% too high. A similar finding can be observed in the relaxograms obtained during the RR_e titration (Fig. 3A). The ratio of Na_i⁺ peak area in the 0 mM RR_e relaxogram to that in the 12.8 mM RR_e is 0.55/0.68 = 0.81 while the analogous ratio of the Na_e⁺ peak areas is 0.45/0.32 = 1.4. Possible reasons for this are considered in the Discussion section. Whatever the cause, multiplying the intrinsic T₁ MR n_Nai values by 1.14 and the intrinsic T₁ MR n_Nae values by 0.70 (not shown) gives results that are in good agreement with the n_Nai MRR/RR_e (filled diamonds) and the n_Nae MRR/RR_e (open diamonds), Fig. 5B.

Na⁺ Efflux kinetics

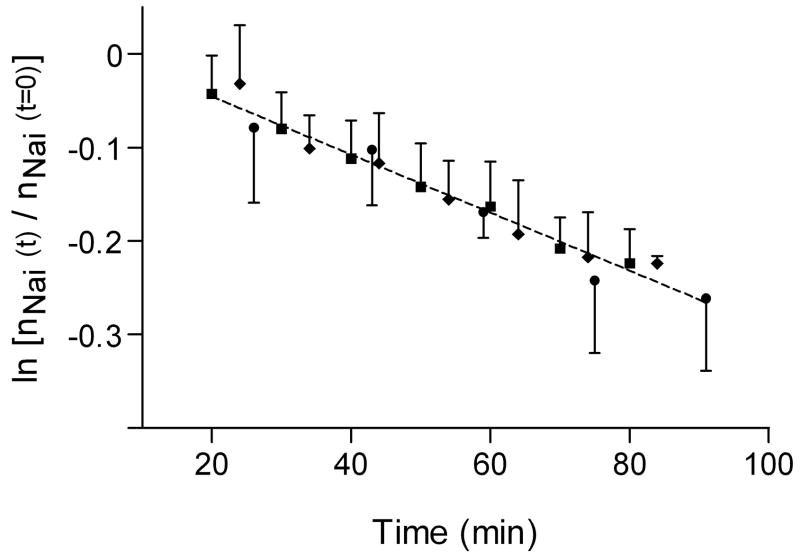
The transcytolemmal Na⁺ efflux was modeled as a first-order kinetic process (Methods). The ln[n_Nai(t)/n_Nai(0)] values for the MRS/SRe (squares), MRR/RR_e (diamonds) and intrinsic T₁MRR (circles) are shown in Figure 6. The data are clearly linear for the measurements of all three groups. The data sets were each individually fitted to lines with a slope of − 3.1 (± 0.3) ×10⁻³ min⁻¹. No better fitting solution was found in fitting the three data sets to lines with different slopes. The pseudo-first order rate constant for Na_i⁺ efflux was 3.1 (± 0.3) ×10⁻³ min⁻¹. Thus, for changes in relative Na_i⁺ (and presumably Na_e⁺) amounts, the intrinsic T₁ MRR approach returned a Na⁺ flux equal to that using MRS/SR_e or MRR/RR_e. This is an extremely encouraging result given the issue with the under-estimation of Na_i⁺.

Figure 6. Na⁺ Efflux kinetics.

A plot of the time dependence of ln[n_Nai(t)/n_Nai(t=0)] from the MRS/SRe results (filled squares), MRR/RR_e results (filled diamonds) and intrinsic T₁ MRR results (filled circles). All three data sets were fit to straight lines. The hypothesis was tested that the slope was different for the three lines individually fit to each set of data (p = 0.98). Thus, the slopes are equal for all three data sets. The common slope was 3.1 (± 0.3) ×10⁻³ min⁻¹; thus, the k = 3.1 (± 0.3) ×10⁻³ min⁻¹. A single line with an intercept of 0.016 (± 0.015) and the above slope fit to the three sets of data (R² = 0.67) is shown.

²³Na MR T₁ Relaxography can detect multiple Na_i⁺ populations in Na⁺-loaded yeast

Multiple Na_i⁺ relaxographic peaks were observed from suspensions of a Na⁺-loaded Baker’s yeast strain. This phenomenon was not seen with suspensions of D273 or Red Star Bakers Na⁺-loaded yeast. Figure 7A shows that during GdDOTP_e⁵⁻ titration of this Na⁺-loaded Baker’s yeast suspension, three relaxographic peaks are resolved with RR_e ≥ 10.3 mM. In addition to the 2.3 ms ²³Na_e⁺ peak at 12.8 mM RR_e, two other peaks are observed at 7.5 and 20.4 ms. Apparently, these two peaks report two different Na_i⁺ (or RR_e-inaccessible) populations. Although a single tissue Na⁺ population can exhibit two T₁ values (i.e., relax bi-exponentially) when the ²³Na system is out of the extreme narrowed condition, quantum mechanical constraints require that their relative contributions be 4:1 (component with smaller T₁: component with larger T₁) [31]. That is not the case here. To test the Na_i⁺ assignment of the larger T₁ peaks, ²³Na IR measurements were made on another Na⁺-loaded Baker’s yeast suspension with SR_e. The IR spectra are shown in the stacked plot (Fig. 7B). The IR data of the individual ²³Na_e⁺ and ²³Na_i⁺ resonances, discriminated by SR_e, were subjected to ILT. The relaxogram of the SR_e-inaccessible ²³Na_i⁺ resonance clearly exhibits two peaks (7.5 and 20 ms), while that for SR_e-accessible ²³Na_e⁺ only one.

Figure 7. ²³Na T₁ MRR with [RR_e] detects multiple Na_i⁺ populations in Na⁺-loaded Bakers yeast.

Panel A: A stacked plot of relaxograms obtained from a suspension of Na⁺ loaded Bakers yeast during serial addition of increasing amounts of the RR_e for Na⁺, GdDOTP⁵⁻. The [GdDOTP_e⁵⁻] are shown on the left. The T₁s determined from the center of the peaks are shown on the right. In the bottom relaxogram (12.8 mM RR_e) the 2.3 ms peak reports Na_e⁺. The peaks at 7.5 and 20.4 ms report Na_i⁺.

Panel B: A stacked plot of T₁ IR spectra obtained from a suspension of Na⁺ loaded bakers yeast with SR_e in the medium (center) with relaxograms resulting from an ILT of the IR data from each resonance. The relaxogram (upper) from the Na_i⁺ resonance exhibits two peaks at 7.5 ms and 20 ms, which matches the T₁ values found from the Na_i⁺ peaks in the bottom relaxogram (12.8 mM [GdDOTP_e⁵⁻]) of panel A.

[Na_i⁺] and [Na_e⁺] calculations

The intra- and extracellular volumes inside the MR coil sensitive volume were estimated to be 0.53 and 3.06 mL, respectively for a 50% Na⁺-loaded yeast suspension (Methods). Using n_Nai and n_Nae amounts linearly extrapolated to t = 0 in Fig. 1B yields [Na_i⁺]_t=0 =175 (± 3) μmol/0.53 mL = 330 (± 6) mM and [Na_e⁺]_t=0 = 42 (± 2) μmol/3.06 mL = 13.5 (± 0.6) mM. The [Na_e⁺] estimation is quite accurate: the minimal medium [Na_e⁺] was 13 mM.

Discussion

The results of this study demonstrate that ²³Na T₁ MRR can discriminate and quantify Na_i⁺ and Na_e⁺ contents in Na⁺-loaded yeast suspensions. We are not aware that this has been previously reported. ²³Na T₁ MRR with RR_e can report accurate absolute Na_i⁺ and Na_e⁺ contents, while intrinsic MRR reports accurate relative changes in Na_i⁺ and Na_e⁺ but not accurate absolute amounts.

In vivo ²³Na T₁ MRR measurements with RR_e for Na⁺ would require an RR_e that enabled differentiation of T_1e and T_1i at reasonable [RR_e] values. For the RR_e, GdDOTP⁵⁻, used in this study, RR_e concentrations greater than 10 mM were required to resolve the T_1e and T_1i peaks. The ratio of [Na_e⁺]/[GdDOTP_e⁵⁻] was approximately 1, which would be problematic for in vivo use. The relaxivity of GdDOTP⁵⁻ for ²³Na⁺ is sensitive to the [Na⁺] value and to the presence of other cations, such as Mg²⁺, present in the medium that compete for the chelate Na⁺ binding site(s) and anions that compete for Na⁺. Unfortunately, the requirement for relatively high [RR_e] may be hard to avoid. Gd(III) complexes that function as efficient RRs for ¹H₂O have a water molecule binding site within the Gd³⁺ inner coordination sphere. This close proximity to the Gd³⁺ atom results in efficient T₁ relaxation of the ¹H₂O signal. Being also a cation, Na⁺ is unlikely to occupy a site so near Gd³⁺ inner coordination sphere. Na⁺ is more likely to bind to or be attracted to negatively charged sites on the ligand, the outer coordination sphere. Consequently, Na⁺ will be farther from the paramagnetic ion and, due to the dipolar nature of the interaction, not experience such large relaxation enhancement. Also, ²³Na R₁ values are inherently large because of the quadrupolar nature of this I = 3/2 nucleus. These characteristics will increase the [RR_e] required for effective ²³Na⁺ relaxation enhancement.

More promising for in vivo use, our results also demonstrate that intrinsic ²³Na T₁ MRR can differentiate Na_i⁺ and Na_e⁺ in Na⁺-loaded yeast suspensions. This approach accurately measures Na⁺ efflux kinetics and this suggests that intrinsic ²³Na T₁ MRR can accurately measure relative Na_i⁺ and Na_e⁺ amounts. However, there are issues in the accurate quantification of the absolute Na_i⁺ and Na_e⁺ amounts. The Na_i⁺ content is underestimated while that of Na_e⁺ is overestimated. We present three general hypotheses that could explain this discrepancy.

The first hypothesis is related to the fact that the ILT is an “ill-conditioned” operation [25; 26; 28; 32]; it is not conducted analytically. It is accomplished numerically, usually with a smoothed (“regularized”) discretized (“grid”) method, which is effectively a multi-exponential analysis [25; 28; 32]. The numerical methods employed can cause errors when the apparent relaxation time constants of the two spin populations to be discriminated are too similar. The MRS/SR_e validation experiments demonstrate that MRR/RR_e is perfectly quantitative for the Na⁺ populations if the [RR_e] value is great enough to cause a sufficiently large R′_1e/R′_1i ratio. The R′_1e/R′_1i is 4.3 when [RR_e] = 12.8 mM and MRR/RR_e is quantitative. Thus, one might suspect that the intrinsic R′_1i/R′_1e0 value (R′_1i is here greater than R′_1e0, the RR_e-free value of R′_1e) is insufficiently large to allow accurate determination of the apparent Na_i⁺ and Na_e⁺ populations. However, the intrinsic R′_1i/R′_1e0 value is reduced only to 3.6 (Fig. 5A). Simulations suggest that it is the smaller R′₁ that is underestimated by ILT when the R′₁ ratio is ≤ 5 [32]. Since we measure a_i and a_e using bi-exponential fitting with fixed ILT T₁′ values, any ILT T₁′ errors could propagate into our analysis.

The second hypothesis is as follows. In the absence of RR_e the equilibrium transcytolemmal Na⁺ exchange system is not in the SXL condition. This would apply if the rate constant for exchange k is not sufficiently smaller that the applicable shutter speed: T ⁻¹ ≡ |R_1e − R_1i|, where the R₁’s are the intrinsic values (i.e. those in the absence of exchange). There may be some evidence for this in the nature of the RR_e titration relaxograms (Fig. 3A). Even though k [≡ k_ie + k_ei ≈ k_ie] would appear to be very small, the exchange system is forced to depart slower domains and pass through the FXL condition [T⁻¹ ≪ k] during the RR_e titration. This can be seen by expanding the T ⁻¹ expression as |^Nar_1e[RR_e] + R_1e0 − R_1i|. Since R_1i > R_1e0, T⁻¹ must pass through zero at some [RR_e] value (≈ 3 mM in Fig. 3B). When 2SX systems are in the FXR or FXL conditions, it is the component with the greater R₁ whose relative contribution is diminished by the exchange [31]. After the Na_e⁺ peak has passed through the Na_i⁺ peak and emerged well on the small T₁ (large R₁) side ([RR_e] = 15.4 mM; Fig. 3A, bottom), the fractional relaxographic Na_i⁺ area (a_i) is essentially the same as the MRS value 20 minutes after re-suspension (Fig. 1B), so the system must be in the SXL. After the Na_e⁺ peak passes through the Na_i⁺ peak ([RR_e] > 8 mM), however, its relative area (a_e) is noticeably diminished. This is consistent with a shutter-speed (Na_e⁺ ↔ Na_i⁺ exchange) effect [31]. When two peaks emerge from relaxographic coalescence, the smaller T₁ component fractional peak area (a_S) is less than the mole fraction of the population that it represents (p_e, in this case) unless the equilibrium intercompartmental exchange system is in the actual SXL condition [T⁻¹ ≫ k], or the NXL. Since k seems so small in this case (Fig. 6; k = 3.1 × 10⁻³ min⁻¹ or 5.2 × 10⁻⁵ s⁻¹), the reversion to the FXR and FXL conditions would have to occur within a very tiny [RR_e] range. Perhaps there are concomitant faster transcytolemmal cycling processes that result in equilibrium Na⁺ exchange, but do not contribute to the slower net Na⁺ efflux. A two-site-exchange analysis [33] suggests that the k_ie′ value resulting from faster equilibrium processes would have to be 5 s⁻¹ (i.e., much greater than k_ie for the net efflux) to account for a 12% n_Nai underestimation.

Thus, the departure of the transcytolemmal Na⁺ exchange ²³Na MR system from the SXL or NXL condition could explain why the a_i and a_e values from the RR_e-free suspension relaxogram are not equal to the p_i and p_e values. If so, this could provide a way to determine p_i and p_e from a_i and a_e.

The third hypothesis is that ²³Na MR intrinsic T₁ relaxography inherently underestimates the smaller T₁ component (Na_i⁺) and overestimates the larger T₁ component (Na_e⁺). Using a phantom consisting of a sphere inside an NMR tube with compartmental Na⁺ T₁s and a range of compartmental Na⁺ populations similar to those encountered in Na⁺ loaded yeast suspensions, we found the following: the sphere Na⁺ (modeling Na_i⁺) population was underestimated 1–6%; and, the tube Na⁺ (modeling Na_e⁺) population was overestimated 2 –4 %. Thus, under ideal conditions ²³Na intrinsic T₁ MRR areas do not equal the ²³Na MRS determined populations. This population discrepancy was observed using all relaxographic analysis options: ILT, Bi-exp with T₁s input from ILT (used in the analysis in this work), and a Bi-exp that determined both T₁ and population. In general, the Bi-exp that determined T₁ and population tended to be slightly more accurate. Although the Na_i⁺ underestimation in the sphere/tube phantom (1–6%) was less than that observed in the yeast suspension (~12%) it probably accounts for 25 to 50% of that underestimation. The remaining discrepancy (~6 to 9%) could be due to more “noise” in the yeast suspension measurements and/or some of the equilibrium exchange effects outlined in the second hypothesis.

Despite the discrepancy between the RR_e-free suspension relaxogram a_i and a_e values and the p_i and p_e values, the efflux kinetics are still accurately measured. This is evidenced by the excellent agreement shown in the Fig. 6 plot of the intrinsic MRR results with those from the MRS/SR_e and MRR/R_e studies. The intrinsic MRR approach measures relative n_Nai values (and presumably also relative n_Nae values) with high accuracy.

The capacity of intrinsic ²³Na T₁ MRR to discriminate Na_i⁺ and Na_e⁺ amounts, albeit with possibly reduced accuracy compared with the use of RR_e, indicates the potential for application of this method in vivo, and even with human subjects. It may be possible to correct the Na_i⁺ and Na_e⁺ amounts, assuming that the content determination error is reproducible. Using the sphere/tube phantom described above we found that Na_i⁺ of 5 to 19% of the total Na⁺, a reasonable range for Na_i⁺ in vivo, was underestimated ~12% by MR relaxography. While this is greater than the underestimation observed at Na_i⁺ of 50% in the same phantom it indicates that Na_i⁺ can be discriminated and estimated in tissues in vivo. Since ²³Na intrinsic T₁ MRR accurately measures relative changes in Na_i⁺ (Fig. 6), Na_i⁺ contents of a region-of-interest may be compared with those of an appropriate control (reference) regions.

The ability of ²³Na intrinsic T₁ MRR to discriminate Na_i⁺ and Na_e⁺ will depend on the T₁ values of the two populations, as well as the relative sizes of the two populations. Bansal and co-workers reported that in the in vivo rat liver (intact animal) Na_i⁺ T₁ = 21 ms in presence of SR_e and Na_e⁺ = 41 ms (estimated from measurements without SR_e) [34]. When an IR ²³Na MRI sequence was used with t_I = 25–30 ms to null signals with T₁ values of 36 – 43 ms, which were presumed to be ²³Na_e⁺, two fold increases in ²³Na MRI intensity were observed in breast tumors in vivo [8]. This image intensity arose from magnetization with smaller T₁ values (T₁ ~ 20 ms) and was attributed to increased ²³Na_i⁺. Thus, there are indications from in vivo studies that Na_i⁺ T₁ ≈ 20 ms and Na_e⁺ ≈ 40 ms. MRR simulations with p_i ≈ 0.15, Na_i⁺ T₁ ≈ 20 ms, and Na_e⁺ = 40 ms indicate that ²³Na intrinsic T₁ MRR will detect both populations.

The attractiveness of intrinsic T₁ MRR is that it does not require use (or development) of an RR_e for ²³Na⁺. This could be especially valuable in neurological ²³Na MR studies, where RR access to the extracellular interstitial space is limited by low blood-brain barrier permeability.

The observation of two different relaxographic T₁′ peaks for SR_e-inaccessible Na⁺ populations in suspensions of Na⁺-loaded Baker’s yeast cells also results from intrinsic T₁ differences. These two T₁ peaks represent Na⁺ populations for which the exchange system is in the SXR or SXL condition. This means a k ≪ 30 s⁻¹ (from the difference in the R₁′ values). It is possible that these are two Na_i⁺ populations separated by membranes, i.e., Na⁺ in two different compartments, such as, the cytoplasm and vacuoles. Gupta, et al, reported an intracellular Na⁺ resonance with two T₁ components in the ²³Na MR spectrum of a Rana oocyte suspension [35]. As Na⁺ efflux from this strain of Baker’s yeast proceeded, the area of the T₁ ~ 8 ms peak generally remained relatively constant, while the area of the T₁ ~ 18 ms peak decreased, at least up until the two peaks merged into one.

²³Na MR spectroscopy and relaxography measure Na⁺ amounts. To determine [Na_i⁺] and [Na_e⁺] values, the intracellular and extracellular volumes must also be measured or estimated. Labadie et. al. demonstrated that ¹H₂O T₁ MR relaxography with an RR_e (or contrast reagent), GdDTPA²⁻, allows discrimination of the intra- (¹H₂O_i) and extracellular (¹H₂O_e) signals [25]. The two peak relaxogram measures the volume fractions, if the shutter-speed effects of equilibrium transcytolemmal water exchange kinetics are accounted for and quantified. Using this method to determine the volumes enabled us to estimate [Na_i⁺]_, and [Na_e⁺] values and, thus, the Na⁺ concentration gradient. Immediately upon re-suspension, [Na_i⁺]/[Na_e⁺] was 24 for Na⁺-loaded yeast cells. After one hour of spontaneous efflux, the [Na_i⁺]/[Na_e⁺] ratio had decreased to 13.

The potential diagnostic utility of Na_i⁺ measurements has led to the development of other Na⁺ compartmental discrimination methods. As described above, ²³Na MRS/SR_e currently provides the best [Na_i⁺] measurements in isolated organs or intact animals. The most extensively studied alternative method has been the ²³Na multiple-quantum MR coherence filters. The Na_i⁺ discrimination is, however, usually not as complete as with SR_e. This, combined with the more than 90% signal intensity reduction from that of single quantum coherences used here, has limited the usefulness of the multi-quantum-filter [31; 36; 37]. Studies of perfused heart concluded that Na_i⁺ content may be reliably determined from SR-free triple-quantum-filtered spectra when the Na_e⁺ contribution does not vary appreciably, such as during constant pressure perfusion [38]. Studies of the liver in vivo, which used SR_e to aid interpretation, found that Na_e⁺ contributed significantly to the total triple-quantum-filtered signal in live animals, and that the intensity of this signal did not change postmortem. However, the triple-quantum-filtered Na_i⁺ signal increased by approximately 380% over a one hour postmortem period, whereas the single quantum Na_i⁺ increased by only 90% [29]. Thus, it is difficult to quantify Na_i⁺ in ²³Na multi-quantum-filtered spectra.

This study explored the potential for compartmental T₁ differences to allow Na⁺ discrimination. The findings demonstrate that ²³Na MRR can discriminate and measure Na_i⁺ and Na_e⁺ on this basis. Two types of ΔT₁ situations were investigated. The first imposed a T_1e reduction with an RR_e. This method was accurate but required relatively large [RR_e] values. With a more effective Na_e⁺ RR_e, this method might be used in vivo. The second method exploited intrinsic Na_i⁺ and Na_e⁺ T₁ differences in the yeast cell suspension. This method was less accurate in Na_i⁺ content determination but did accurately measure the spontaneous Na_i⁺ efflux rate constant. It offers the potential advantage of not requiring the use of exogenous agents to enable relaxographic discrimination in ²³Na MRI, and could thus be used for human studies.

Experimental

Na⁺-loaded yeast cells were prepared as follows. Typically, 16 g of yeast cells, either D273-10B (American Type Culture Collection, Manassas, VA) or baker’s yeast (obtained from a local bakery or Red Star yeast from a supermarket) were added to 800 mL of a 0.2 M Na₃citrate, 5% weight/volume (wt/vol) glucose solution and bubbled with 95% O₂/5% CO₂. This mixture was stirred at room temperature for 2 h (± 15 min) [24]. After Na⁺ loading, the yeast cells were centrifuged (T = 4°C) and washed twice with 50 mL of cold (T = 0°C) minimal medium containing: 4 mM MgSO₄, 13 mM KCl, 13 mM Na⁺ (added as NaOH to adjust pH to 6.6), and 50 mM 3-N-morpholino-propanesulfonic acid. After the second washing the centrifuged yeast pellet was re-suspended in minimal medium to make up the experimental samples to be 50% wet wt/vol. Where noted stock solutions containing 100 mM Tris₄HTmDOTP (a ²³Na⁺ SR[15]) or Tris₄HGdDOTP (a ²³Na⁺ RR) were added to the minimal medium before making up the 50% wt/vol yeast suspension. The final total suspension volume required to bring cell density to 50% wt/vol varied slightly but was 10 mL or greater. The final extracellular SR or RR concentrations were ~12.8 mM unless otherwise noted.

In studies where [RR_e] was varied, the following steps occurred after each set of MR measurements: (1) the yeast suspension was centrifuged; (2) the supernatant was discarded; (3) the cell pellet was re-suspended in 50 mL of cold minimal medium and re-centrifuged; and (4) the packed cells were re-suspended in ~10 mL minimal medium containing necessary RR stock volume to achieve the next [RR_e] value.

Yeast suspension MR measurements were conducted in 20 mm o.d. NMR tubes (Wilmad-Labglass, Buena, NJ). Before positioning in the magnet, the tube was fitted with a home-made apparatus consisting of two lengths of Clay Adams PE 90 tubing (Becton-Dickinson, Franklin Lakes, NJ) that extended into the tube to near its bottom. The 95% N₂/5% CO₂ gas flowed constantly through the tubing. The gas flow was adjusted to keep the yeast cells suspended during the MR measurements.

²³Na MR measurements

²³Na MR free-induction-decays (FIDs) were acquired at 105.5 MHz (9.4T) using a 20 mm Broad Band probe (Nalorac Inc., Martinez, CA) in a Varian Inova spectrometer (Varian Inc., Palo Alto, CA). For ²³Na MRS one-pulse measurements, 208 FIDs were acquired and averaged over a 1 min period using 90° RF pulses and a recycle time of 0.266 s: the time between the end of the pulse and the start of FID digitization (the dead time) was 115 μs. ²³Na MR T₁ relaxation time constants of yeast suspension in presence of RR were measured using an IR pulse sequence with the following parameters: 16 FIDs were averaged for each incremented time from inversion (t_I) between the 180° and 90° pulses; there were 74 t_I values (minimum, 0.2 ms; maximum, 300 ms); the recycle time minimum was 0.306 s; the dead time was 115 μs; and the total time was 7 min. In studies that measured intrinsic ²³Na T₁ relaxation time constants, 8 FIDs were averaged for each of 74 t_I values (minimum, 0.5 ms and maximum, 600 ms); the recycle time was 0.6 s; the dead time was 115μs; and the total time was 7min. The mid-point of the spectral acquisition was taken as the measurement time. Time zero was defined as that of the re-suspension of the yeast in cold minimal medium to a 50% wt/vol cell density. The MR probe air temperature was 24 (± 1) °C.

Na⁺ amounts from ²³Na MR

The areas of MRS ²³Na⁺ resonances were measured using both the Varian software frequency spectral integration routine (VNMR 6.1c) and Bayesian FID Analysis (Bayesian Analysis Software, G.L. Bretthorst Washington University, St. Louis, MO). The Bayesian Analysis yields the FID amplitude for each resonance [39], which we term the Bayes number. With the same spectrometer settings, the Bayes numbers report the relative signal sizes (Na⁺ amounts) in different samples. The absolute Na⁺ content was calculated as follows. A capillary containing 8.425 μmol of Na₅Dy(PPP)₂, a SR[11], was placed inside a 20 mm NMR tube containing Na⁺-free minimal medium (KOH was used to alter pH). The capillary was contained completely within the RF coil sensitive volume. Bayesian analysis of the one pulse MRS FID of this standard sample provided a conversion factor, μmol Na⁺/Bayes number. Thus, for each MRS/SR_e measurement, the Bayes numbers for the Na_i⁺ and Na_e⁺ resonances were converted to amounts (μmol Na⁺). For MRR studies, the Bayes number of a one-pulse MRS FID was obtained to yield the total Na⁺ amount (n_Na). One-pulse and IR measurements were interleaved throughout the study.

²³Na Relaxographic data analysis

In the IR data sets, integrated using Varian software, the longitudinal magnetization (M_z) values at the three longest t_I values were averaged to estimate the Boltzmann equilibrium magnetization [<M_Z(t_I)> = M_Z(∞)]. The t_I-dependent quantity [(M_Z(∞) − M_Z(t_I))/(2M_Z(∞))] was then calculated from the IR data and processed using a 1D ILT algorithm [28; 40] written in Matlab (TwoDLaplaceInverse, Magritek Limited, Wellington, New Zealand). The output of the ILT is the apparent relaxation time constant distribution, or T₁′ relaxogram. A two peak relaxogram yielded presumed Na_i⁺ T₁′ (T′_1i) and Na_e⁺ T₁′ (T′_1e) values. Relaxograms were plotted and analyzed to obtain the T₁′ (center of a peak) and relative area values using Matlab routines (MathWorks Inc, Natick, MA).

The T₁′ values obtained from the relaxogram were used in a least squares fitting of an empirical (phenomenological) bi-exponential expression, a_eexp(−t_I/T′_1e) + a_iexp(−t_I/T′_1i) + C, to the [(M_Z(∞) − M_Z(t_I))/(2M_Z(∞))] IR decay (GraphPad Prism version 5.0 for Windows, GraphPad Software, San Diego, CA). Only the apparent mole fraction, a_i and a_e and noise constant, C, values were varied. The Na_i⁺ (n_Nai) and Na_e⁺ (n_Nae) amounts were then calculated as follows:

$${\text{n}}_{\text{Nai}}={\text{n}}_{\text{Na}}\times {\text{a}}_{\text{i}}/({\text{a}}_{\text{i}}+{\text{a}}_{\text{e}});\text{and}{\text{n}}_{\text{Nae}}={\text{n}}_{\text{Na}}-{\text{n}}_{\text{Nai}}.$$ [1]

Yeast suspension intra- and extracellular volumes

Total sample intracellular and extracellular volumes (V_i and V_e, respectively) were calculated as follows. ¹H₂O MRR (398.8 MHz; 9.4T) with GdDTPA_e²⁻ and two-site-exchange (2SX) analysis [25] for equilibrium transcytolemmal water interchange in a 50% wt/vol Na⁺-loaded yeast suspension found a ¹H₂O_i mole fraction (population) [p_i(w)] of 0.148 and a ¹H₂O_e mole fraction [p_e(w)] of 0.852.

The yeast cell pellet dry to wet weight ratio was 0.17 (± 0.02). Thus, 5 g (wet wt) yeast contains 0.85 g dry wt. The final yeast suspension sample volume was 10 mL. Correcting for yeast cell mass gives water mass (10 − 0.85 = 9.15 g) - assuming unit density, this is 9.15 mL; 9.15 × 0.852 = 7.796 mL ≈ 7.8 mL = V_e. This V_e was used to calculate [SR_e] and [RR_e].

The [Na_i⁺] and [Na_e⁺] values were calculated using the intra- and extracellular volume fractions detected, ^dV_i and ^dV_e. The RF coil sensitive volume was 3.92 mL; thus, 0.392 of the total sample volume. Therefore, 0.333 g (dry wt) yeast was inside the RF coil and, 3.92 mL RF coil volume − 0.333 mL yeast = 3.59 mL H₂O total inside the RF coil volume. This results in the following detected volumes, 3.59 mL H₂O × p_i (0.148) = 0.53 mL = ^dV_i; and, ^dV_e = 3.06 mL.

Kinetics

After re-suspension, Na⁺ spontaneously exits the Na⁺-loaded yeast cells. We analyze this by assuming that transcytolemmal Na⁺ efflux is an effectively irreversible first-order kinetic process: that is k_ei ≪ k_ie, where k_ie is first-order rate constant for Na⁺ efflux and k_ei is that for Na⁺ influx. If so a plot of ln[n_Nai(t)/n_Nai(0)] vs. t will yield a straight line with slope equal to − k_ie. The n_Nai(0) values were estimated by fitting n_Nai (t) with an effective straight line.

Statistical analysis

Results are presented as the mean (± one standard deviation (SD)) values. GraphPad Prism version 5.0 for Windows, GraphPad Software, San Diego, CA was used for graphs and data fittings and comparison of data fitting parameters. Differences were declared statistically significant if p < 0.05.

Appendix 1

Abbreviations

a_i

fractional peak area of intracellular Na⁺ obtained by relaxography

a_e

fractional peak area of extracellular Na⁺ obtained by relaxography

a_L

fractional area of peak with larger T₁ obtained from relaxography

a_S

fractional area of peak with smaller T₁ obtained from relaxography

Bi-exp

bi-exponential function (a_eexp(−t_I/T′_1e) + a_iexp(−t_I/T′_1i) + C)

FXR

Fast eXchange Regime

FXL

Fast eXchange Limit ([T⁻¹ ≪ k])

ILT

Inverse Laplace Transform, the apparent relaxation time constant (T₁′) distribution

MRR

Magnetic Resonance Relaxography

n_Nae

amount of extracellular Na⁺

n_Nai

amount of intracellular Na⁺

NXL

No eXchange Limit

p_e

fractional population of extracellular Na⁺

p_i

fractional population of intracellular Na⁺

^Nar_1CF

Relaxivity of Na⁺ in cell free solution

R_1e0

longitudinal relaxation rate extracellular Na⁺ in absence of RR_e

R_1e

longitudinal relaxation rate of extracellular Na⁺ (in the absence of exchange)

R_1i

longitudinal relaxation rate of intracellular Na⁺ (in the absence of exchange)

Relaxogram

the apparent relaxation time constant (T₁′) distribution produced by ILT

RR_e

extracellular relaxation reagent

SR_e

extracellular shift reagent

SXL

Slow eXchange Limit ([T⁻¹ ≫; k])

SXR

Slow eXchange Regime

T⁻¹

shutter speed ≡ |R_1e − R_1i|