A phenomenological model that predicts forces generated when electrical stimulation is superimposed on submaximal volitional contractions

Abstract

Superimposition of electrical stimulation during voluntary contractions is used to produce functional movements in individuals with central nervous system impairment, to evaluate the ability to activate a muscle, to characterize the nature of fatigue, and to improve muscle strength during postsurgical rehabilitation. Currently, the manner in which voluntary contractions and electrically elicited forces summate is not well understood. The objective of the present study is to develop a model that predicts the forces obtained when electrical stimulation is superimposed on a volitional contraction. Quadriceps femoris muscles of 12 able-bodied subjects were tested. Our results showed that the total force produced when electrical stimulation was superimposed during a volitional contraction could be modeled by the equation T = V + S[(MaxForce − V)/MaxForce]^N, where T is the total force produced, V is the force in response to volitional contraction alone, S is the force response to the electrical stimulation alone, MaxForce is the maximum force-generating ability of the muscle, and N is a parameter that we posit depends on the differences in the motor unit recruitment order and firing rates between volitional and electrically elicited contractions. In addition, our results showed that the model predicted accurately (intraclass correlation coefficient ≥0.97) the total force in response to a wide range of stimulation intensities and frequencies superimposed on a wide range of volitional contraction levels. Thus the model will be helpful to clinicians and scientists to predict the amount of stimulation needed to produce the targeted force levels in individuals with partial paralysis.

electrical stimulation is used to elicit muscle contractions via stimulation of the intact or partially intact peripheral nervous system. One clinical application of electrical stimulation is functional electrical stimulation (FES) and involves the electrical activation of skeletal muscles to assist individuals with central nervous system impairments to produce functional movements (4, 5, 21, 24). During FES, an electrically elicited muscle contraction is commonly superimposed over a voluntary contraction to augment force generation by a weak muscle (4, 21, 24). Superimposition of electrical stimulation during voluntary contractions also can be used to evaluate an individual's ability to activate a skeletal muscle (12, 23), to characterize the nature of fatigue (7), or to improve muscle strength during postsurgical rehabilitation (22, 26) and in individuals with muscle weakness due to upper motor neuron injuries (31). Although electrical stimulation is commonly superimposed on volitional contractions during clinical applications, the manner in which volitionally and electrically elicited forces summate has not been clearly identified.

For accurate generation of targeted joint motions during FES applications, it is crucial that the appropriate amount of joint torque be generated by the combination of electrical and volitional contraction forces (24). For instance, in individuals who have sustained a stroke, electrical stimulation is commonly delivered to the ankle dorsiflexor muscles during the swing phase of gait to augment volitional force production and generate sufficient ankle dorsiflexion for the foot to clear the ground (13, 16, 21, 34). During FES-assisted correction of foot drop in poststroke individuals, generation of too little dorsiflexor force can result in inability of the foot to clear the ground; generation of too much dorsiflexor force can result in rapid onset of muscle fatigue. Thus, to design optimal FES systems that generate targeted joint motions with minimum muscle fatigue, it is important to understand how electrically induced muscle forces summate with concurrent volitional forces.

The manner in which volitional force production is augmented by electrical stimulation may depend on a variety of biophysical factors such as differences in the order and rate of fiber recruitment between electrically and volitionally induced contractions (9, 15, 29), distance of electrodes and electric field from target nerve fibers (28), and tissue conductivity (28). Frigo et al. (8), while developing an EMG-based FES controller, assumed that during concurrent volitional and electrical activation of muscle, the output force is the resultant of simple linear summation of the two components. In contrast, other experimental studies indicated that a simple summation of electrical and volitional forces did not hold true, at least during maximal voluntary contractions where the addition of electrical stimulation offered zero force augmentation to nonimpaired volitional muscle contractions (32, 33). Furthermore, a series of studies by Langzam and colleagues (18–20) found a linear summation when volitional contractions ranging from 0 to 30% of the maximum voluntary contraction were superimposed on electrically elicited contractions using a 20-Hz stimulation train with intensities set to produce forces ranging from 0 to 30% of the maximum voluntary contraction. Interestingly, however, these studies also showed that at low current intensities, force augmentation was greater than the electrically induced force obtained without a concurrent volitional contraction (18–20). In contrast, at higher intensities, the combined torque of the two components was smaller than the simple summation of the voluntary and electrically induced contractions (18–20). Herbert and Gandevia (11) modeled the effect of superimposing a single supramaximal electrical pulse during a range of volitional activation levels and found that the amplitude of the simulated interpolated twitch declined linearly with increase in voluntary force. These studies, however, tested only a narrow range of stimulation and volitional intensities and a single stimulation frequency (18–20).

The present study examines the additional force produced by superimposition of electrical stimulation onto submaximal volitional contractions by using combinations of a wide range of volitional and electrical contraction intensities and electrical stimulation frequencies. The objective of this study is to develop a mathematical model that can accurately predict the total isometric forces obtained when electrical stimulation is superimposed on volitional contractions.

MATERIALS AND METHODS

Subjects.

Twelve able-bodied subjects (6 women and 6 men, age 28 ± 5 yr, height 171 ± 12 cm, weight 69 ± 14 kg) were recruited for this study. Each subject participated in one testing session. Data collected from the first six subjects (4 men and 2 women) were used to develop a mathematical model to predict the forces generated when submaximal levels of electrical stimulation are superimposed on submaximal levels of volitional contractions. Additional data, which were not used for model development, were collected from the remaining six subjects (2 men and 4 women) to validate the model. All subjects signed an informed consent form. The study protocol and consent form were reviewed and approved by the Human Subjects Review Board of the University of Delaware. All subjects recruited for this study had previously participated in studies involving electrical stimulation and were, therefore, accustomed to electrical stimulation.

Equipment and experimental setup.

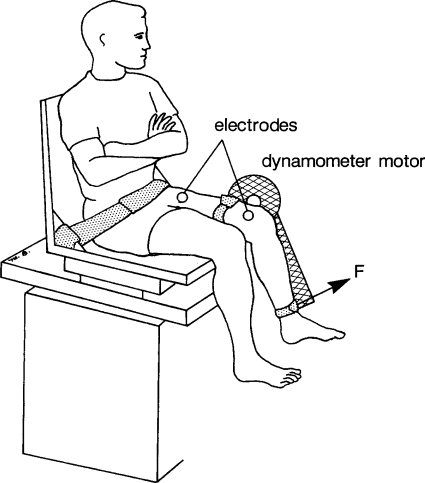
Subjects were seated on a computerized controlled force dynamometer (KinCom III 500–11; Chattecx, Chattanooga, TN) with their hips flexed to 75° and their knee flexed to 90° (Fig. 1) (3). The axis of the dynamometer was aligned with the knee joint axis, and the force transducer was positioned against the anterior portion of the tibia, ∼4 cm proximal to the lateral malleolus (3). Electrical stimulation was delivered to the quadriceps femoris muscle via two surface electrodes using a bipolar electrode setup (7.6 × 12.7 cm; CONMED, Utica, NY). The anode was positioned over the proximal portions of the rectus femoris and vastus lateralis, whereas the cathode was positioned over the distal portion of the thigh, over the vastus medialis and distal portion of the rectus femoris (1). The trunk, pelvis, and thigh of the leg being tested were each stabilized with inelastic straps. A Grass S48 stimulator with an SIU8T stimulus isolation unit (Grass Instruments, West Warwick, RI) was used to stimulate the quadriceps femoris muscle of the subject's dominant leg. The stimulator was controlled by a computer using a custom-written LabVIEW program (National Instruments, Austin, TX). Data collected from the force transducer were sampled at 200 Hz using an analog-to-digital board. The data were then analyzed using a custom-written LabVIEW program (25).

Fig. 1.

Schematic representation of the experimental setup (see text for details). F, force measured by the dynamometer's transducer.

At the beginning of the experimental session, each subject performed a 3- to 5-s maximum voluntary isometric contraction (MVIC). During the contraction, a supramaximal stimulation train with amplitude of 135 V, pulse duration of 600 μs, frequency of 100 Hz, and train duration of 100 ms was superimposed on the contracting muscle ∼2 s into the volitional contraction (30) to determine the central activation ratio (CAR) and the maximum force-generating ability of the muscle (MaxForce). The CAR was calculated by dividing the subject's MVIC by the total force elicited when a burst of electrical pulses was delivered during an MVIC and was used to determine the level of voluntary activation (12, 32). A CAR of 1.0 represents full activation, and a CAR <1.0 corresponds to incomplete activation. If a subject's CAR was <0.95, the procedure was repeated after a 5-min rest. Each subject was given three attempts to reach a CAR of 0.95; if unable to do so, the subject was either rescheduled or excluded from the study. For this study we defined MaxForce as the maximum force that was produced by the burst of electrical pulses during the CAR testing. This MaxForce was the “best estimate” of the subject's maximum force-generating ability. After the CAR testing, subjects were given a 10-min rest before continuing the study.

Experimental procedure for model development phase.

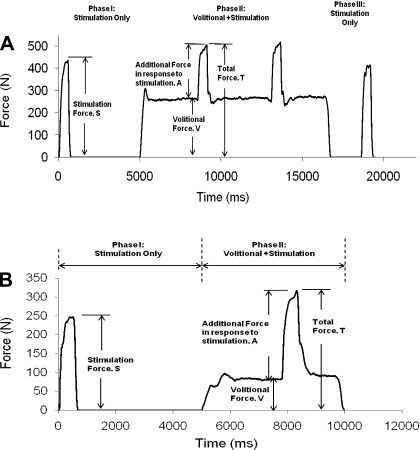
Each subject performed 12 trials during which electrical stimulation was superimposed on volitional contractions. Specifically, electrical stimulation that produced 10, 20, 30, and 40% of the MaxForce was superimposed on volitional contractions of 10, 20, and 30% of the MaxForce. The order of testing of the 12 trails was randomized for each subject. For each of the selected trial, using a 30-Hz, 500-ms train with each pulse fixed at a duration of 600 μs, we varied the stimulation amplitude to produce the targeted stimulation force level. Next, the muscle was potentiated with fifteen 14-Hz, 770-ms trains, and a rest time of 5-s was provided between each train. Immediately following potentiation, we manually readjusted (within 2–3 attempts) the stimulation amplitude with a 30-Hz, 500-ms train to produce the targeted stimulation force level of 10, 20, 30, or 40% of the MaxForce. Finally, using the visual display of the dynamometer, we set a line that represented the targeted force level (10, 20, or 30% of the MaxForce) the subjects were instructed to reach volitionally. Within less than 2 min of potentiating the muscle, testing at the selected trial of electrical stimulation and volitional contraction was carried out. Each trial contained three phases of muscle activation: 1) phase I force production by electrical stimulation only, 2) phase II electrical stimulation superimposed on a volitional contraction, and 3) phase III force production by electrical stimulation alone (see Fig. 2A for details of the timing of each phase of contraction). The same stimulation train was repeated both during and after the volitional contractions to determine whether muscle fatigue or potentiation occurred. A rest period of 5 min was provided before testing the next trial. The entire procedure was then repeated for testing each of the 12 trials.

Fig. 2.

A: the 3 phases of muscle activation for a typical subject tested during model development. In phase I, using the predetermined stimulation amplitude, subjects were stimulated with a 30-Hz, 500-ms train to reach the targeted maximum force-generating ability of the muscle (%MaxForce); subjects then relaxed for ∼4.5 s after the stimulation was delivered. In phase II, subjects were instructed to contract volitionally and maintain the targeted volitional %MaxForce level for ∼8 s; the 30-Hz stimulation train with a predetermined stimulation amplitude (same as phase I) was superimposed at ∼3 and ∼6 s during the volitional contraction. In phase III, after completing the volitional contraction, subjects rested for ∼2 s before the first train was repeated. B: the 2 phases of muscle activation for a typical subject tested during model validation. In phase I, using the predetermined stimulation amplitude and frequency, subjects were stimulated with a 500-ms train to reach the targeted %MaxForce level; subjects then relaxed for ∼4.5 s after the stimulation was delivered. In phase II, subjects were instructed to contract volitionally and maintain the targeted volitional %MaxForce level for ∼5 s; a single stimulation train (same as phase I) was superimposed at ∼3 s during the volitional contraction.

Data analysis for the model development phase.

Peak forces were determined in response to stimulation alone (stimulation force, S) and when stimulation was delivered to the contracting muscle (total force, T; see Fig. 2A). Data were averaged within a 100-ms window just before electrical stimulation was superimposed on the volitional contraction to identify the force during volitional contractions alone (volitional force, V). Absolute percent errors between the two occurrences of S, V, and T normalized to the first occurrence of S, V, and T, respectively, were calculated for each subject at each level of stimulation and volitional contraction (see Fig. 2A). Because previous studies from our laboratory have shown that delivering the same train twice within a session gave ∼15% error due to physiological variance, the effects of muscle fatigue or potentiation were considered only if the errors were >15%. If a particular combination of stimulation and volitional contraction had an error >15% when the two values of S, V, or T were compared, the forces in response to that particular stimulation and volitional combination for that subject were excluded from further data analyses. For combinations that had their force error ≤15%, the two values of S, V, and T were averaged for each subject.

A number of custom linear and nonlinear equations were developed to describe mathematically the force generated by the muscle when stimulation was superimposed on a volitional contraction. Each equation had to satisfy the following two boundary conditions for the case of a stimulated force superimposed on a volitional force: 1) if no volitional force is being generated (V = 0), the total force must equal the force produced by the stimulation (T = S); and 2) if the volitional force is equal to the maximum force-generating ability of the muscle (V = MaxForce), then the total force must equal the volitional force (T = V). Measured total force data were plotted first as a function of the measured volitional force; then, with the use of MATLAB's curve-fitting toolbox, the plotted data were fit at each of the four levels of electrical stimulation (10, 20, 30, and 40% of the MaxForce). Goodness of fit between the measured and modeled forces was evaluated using coefficients of determination (R²). The equation that satisfied the boundary conditions and had the best R² value was chosen.

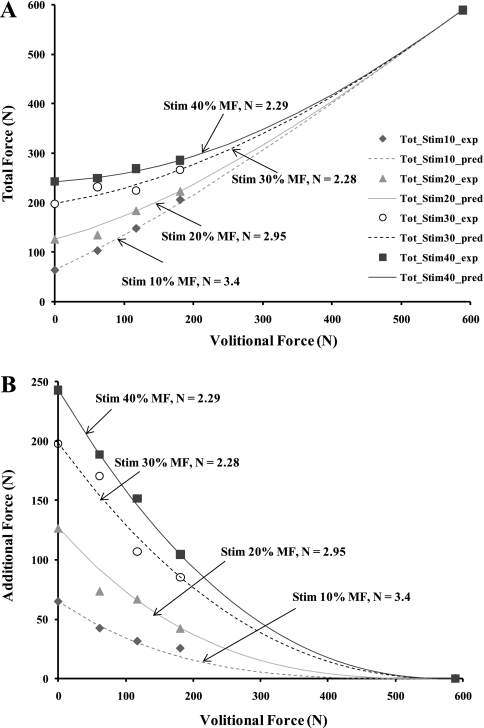
Typical force responses to stimulation alone, to volitional contraction alone, and when stimulation was superimposed during a volitional contraction are as shown in Fig. 2A. The mean absolute errors between the two occurrences of S, V, and T for each trial were <15% for all the subjects tested (Table 1), suggesting that little fatigue or potentiation occurred during testing. When the total force was plotted as a function of the volitional force for each of the 12 combinations of volitional and electrical stimulation forces, we saw a nonlinear relationship between the total force and volitional force for each of the levels of electrical stimulation (see Fig. 3A). We also plotted the additional forces produced when electrical stimulation was superimposed on a volitional contraction versus the volitional force to further analyze this nonlinear relationship (see Fig. 3B). Based on these data sets, we observed that the total force was not a simple linear summation of the volitional force and the force in response to stimulation alone. Rather, it was a function of the level of volitional contraction, the level of electrical stimulation, and the interaction between the levels of volitional and electrical stimulation. Thus we identified the following equation that described the nonlinear relationship between T and V at each stimulation level and satisfied the boundary conditions:

$$\text{T}=\text{V}+\text{S}{\left(\frac{\text{MaxForce}-\text{V}}{\text{MaxForce}}\right)}^N$$ 1

Table 1.

Mean absolute percent error averaged across six subjects

	Stimulation Level
	10% MF			20% MF			30% MF			40% MF
Volitional Level	S	V	T	S	V	T	S	V	T	S	V	T
10% MF	3.1 ± 2.5	1.4 ± 0.2	4.8 ± 1.3	3 ± 2.3	3.1 ± 1	4.2 ± 0.9	6.8 ± 0.1	1.9 ± 0	3.0 ± 1	4.2 ± 0.4	3.3 ± 2.6	2.4 ± 1.8
20% MF	3.9 ± 6.6	2.4 ± 2.2	5.8 ± 1.9	4.1 ± 0.5	1.9 ± 3.5	3.4 ± 0.7	5.1 ± 0.1	3.2 ± 1.3	1.6 ± 1.8	3.2 ± 0.5	1.7 ± 0.3	1.9 ± 1.7
30% MF	9.6 ± 5.2	2.6 ± 0.9	3.8 ± 0.7	7.6 ± 1.8	2.2 ± 0.4	2.3 ± 4.9	2.7 ± 0.9	2.4 ± 1.2	3.8 ± 2.4	4.5 ± 0.7	3.5 ± 1.8	1.3 ± 1.3

Value are mean (±SE) absolute percent error averaged across 6 subjects. Percent errors were calculated between the 2 occurrences of forces in response to stimulation alone (S), volitional contraction alone (V), and when stimulation was delivered to the contracting muscle (total force, T) for different combinations of stimulation and volitional contraction levels. MF, maximum force-generating ability of muscle.

Fig. 3.

A: plots of total force (T) vs. volitional force as a function of the 4 stimulation levels. Symbols represent the measured total force, and the lines represent the modeled total force. Total force is the sum of the volitional force and the additional force in response to stimulation that is superimposed on the volitional force (i.e., Stim 10% MF represents a stimulation amplitude set to produce 10% of the MaxForce). B: plots of additional force vs. volitional force as a function of the 4 stimulation levels. Data in A and B are from a typical subject. MaxForce for this subject was 588 N.

In Eq. 1 we posit that N depends on the differences in the motor unit recruitment order and firing rates between volitional and electrically elicited contractions. The value of N was identified for each subject at each stimulation level (Fig. 3A and Table 2). The term S[(MaxForce − V)/MaxForce]^N is the modeled stimulation force (Fig. 3B). For each subject, Eq. 1 fit the data at each stimulation level with R² values ≥0.99 (Table 2). We performed a two-way ANOVA on the values of N to test the main effects of subjects and stimulation levels to see whether the values of N varied between subjects and across stimulation levels for each subject. Results of the test showed that there was a significant variation in the values of N across subjects (P = 0.0013) and no significant difference across stimulation levels (P = 0.52). Thus the stimulation level to best identify the value of N that would predict the total forces across stimulation levels was determined for each subject. Using a systematic approach, we found that the stimulation level of 30% of the MaxForce had the smallest averaged mean error across all subjects and stimulation levels (Table 3) and was therefore used to identify the value of N to predict the total force at other stimulation levels for each subject.

Table 2.

Values of parameter N and their corresponding R² values for six subjects tested during the development phase of the study

	Subject 1		Subject 2		Subject 3		Subject 4		Subject 5		Subject 6
Stimulation Level	N	R2	N	R2	N	R2	N	R2	N	R2	N	R2
10% MF	3.59	0.99	1.57	0.99	2	1	2.41	0.99	1.33	0.99	3.4	0.99
20% MF	3.21	1	1.96	0.99	1.68	0.99	1.35	0.99	2.11	0.99	2.95	0.99
30% MF	2.74	0.99	1.89	0.99	1.4	0.99	3.29	0.99	1.54	0.99	2.28	0.99
40% MF	3.18	0.99	1.38	0.99	0.9	0.99	2.17	0.99	1.73	0.99	2.29	1

Values are parameter N and the corresponding correlation coefficient (R²) values for the 6 subjects tested at the 4 stimulation intensities during the development phase of the study.

Table 3.

Mean percent errors between the model and experimental peak total force normalized to experimental peak total force

	Stimulation Level at Which N is Determined
	10% MF							20% MF							30% MF							40% MF
Stimulation Level	S1	S2	S3	S4	S5	S6	Avg	S1	S2	S3	S4	S5	S6	Avg	S1	S2	S3	S4	S5	S6	Avg	S1	S2	S3	S4	S5	S6	Avg
10% MF	1.4	0.4	1.9	3.6	2.0	2.0	1.88	2.3	0.4	3.5	6.1	4.6	2.5	3.22	3.1	1.4	5.2	4.4	2.3	4.7	3.52	2.4	2.1	8.8	4.1	2.6	4.7	4.12
20% MF	4.5	4.0	3.5	8.1	6.8	5.5	5.40	4.8	2.5	2.8	6.2	1.5	4.7	3.75	5.4	2.8	3.1	13	4.7	9.7	6.45	4.9	6.8	8.1	6.7	3.0	9.7	6.53
30% MF	5.2	3.8	8.4	6.6	3.1	11.0	6.35	4.0	1.7	5.2	19.0	5.6	6.9	7.06	3.4	1.7	2.3	3.3	1.8	4.2	2.78	3.9	7.3	4.2	9.2	2.9	4.2	5.29
40% MF	10.0	4.4	12.0	4.0	6.1	12.0	8.08	9.9	8.5	8.3	12.0	5.1	7.5	8.55	10.0	7.3	5.1	8.3	4.4	0.8	5.98	9.8	2.4	1.9	3.3	3.0	0.7	3.52
Grand Avg							5.43							5.65							4.68							4.87

Mean percent errors between the model and experimental peak total force normalized to the experimental peak total force and measured at volitional levels of 10%, 20%, and 30% MF for each subject at each stimulation level. The mean percent errors are averaged (Avg) for all of the 6 subjects tested, and then a grand average is calculated at each stimulation level for which the value of N is determined.

Experimental procedure for model validation phase.

To validate Eq. 1, we collected data from six additional subjects in response to a wide range of stimulation frequencies, stimulation intensities, and volitional contraction levels. Data were collected at 30 Hz to determine the value of N and at two new frequencies of 20 and 60 Hz (Table 4). With the use of the 30-Hz stimulation frequency, a stimulation level of 30% of the MaxForce was superimposed on volitional contraction levels of 10, 30, and 50% of the MaxForce. With the use of a 20-Hz stimulation frequency, stimulation levels of 10 and 30% of the MaxForce were superimposed on volitional contraction levels of 10 and 50% of the MaxForce. With the use of a 60-Hz stimulation frequency, a stimulation level of 10% of the MaxForce was superimposed on volitional contraction levels of 10 and 50% of the MaxForce, a stimulation level of 30% of the MaxForce was superimposed on volitional contraction levels of 10, 50, and 70% of the MaxForce, and a stimulation level of 40% of the MaxForce was superimposed on a volitional contraction level of 70% of the MaxForce. Each subject was tested with a total of 13 trials that contained the above combinations of volitional and electrically elicited contractions. These trials were randomized with a rest time of 5 min between each trial. Before testing at each combination of volitional and electrical stimulation, the muscle was potentiated with fifteen 14-Hz, 770-ms trains with a 5-s delay between each train. Because data from the model development phase showed that there was no muscle fatigue or potential during each trial, subjects were stimulated only once during phase II and the second testing with electrical stimulation only (i.e., phase III) was omitted (Fig. 2B) during each trial.

Table 4.

Combination of stimulation and volitional contraction levels tested for the model validation phase of the study

	Stimulation Level
Volition Level	10% MF	30% MF	40% MF
10% MF	20, 30, 60	20, 30, 60
30% MF		30
50% MF	20, 30, 60	20, 30, 60
70% MF		60	60

Values represent stimulation frequencies (in Hz) at which the indicated combination of stimulation and volitional contraction levels were tested for the model validation phase of the study.

Data analysis for model validation phase.

The value of parameter N in Eq. 1 was identified for each subject by fitting the measured total force data in response to the 30-Hz stimulation train with a stimulation intensity set to produce 30% of MaxForce and superimposed on the volitional contraction levels of 10%, 30%, and 50% of the MaxForce. The identified value of N was then used in Eq. 1 to predict the total forces for 20- and 60-Hz frequencies at each of the volitional and electrical stimulation combinations for each subject. The measured and predicted total forces for each subject were first normalized with respect to his or her MaxForce and then compared at each of the volitional and electrical stimulation combinations to test the predictive ability of our nonlinear model (Eq. 1). Intraclass correlation coefficients (ICCs) were used to determine how well the model (Eq. 1) predicted the normalized total force for the 20- and 60-Hz stimulation frequencies for the six subjects for all combinations tested. The ICC is an index that provides an estimate of both consistency and average agreement between two or more data sets while accounting for offsets in the data (27). In addition, for the 20- and 60-Hz frequencies, the measured total force values were plotted against the predicted total force values for the six subjects for all the combinations tested. Slopes of trend lines with the intercepts set at zero were used to evaluate how well the predicted and measured total forces matched. An ICC of 1 and a trend line slope of 1 would suggest a perfect prediction by the model.

We also compared the ability of a simple linear model (i.e., T = V + S) versus our nonlinear model (Eq. 1) to predict the measured total forces. We used 95% confidence intervals of the normalized measured total forces to determine the accuracy of the two models. Thus the normalized forces were averaged across subjects at each stimulation and volitional contraction level combination. If the averaged, normalized total force predicted by the two models fell within the 95% confidence interval of the averaged, normalized measured total force, then each of the model's predictions was accepted as accurate.

In Eq. 1, because we posited that N depends on the differences in the motor unit recruitment order and firing rates between volitional and electrically elicited contractions, we varied the value of N to evaluate its effects on the predicted additional forces produced {S[(MaxForce − V)/MaxForce]^N} when electrical stimulation was superimposed on a volitional contraction. Specifically, we varied the value of N from N = 0 to N >>1, and we plotted modeled additional forces versus the volitional forces for different values of N.

RESULTS

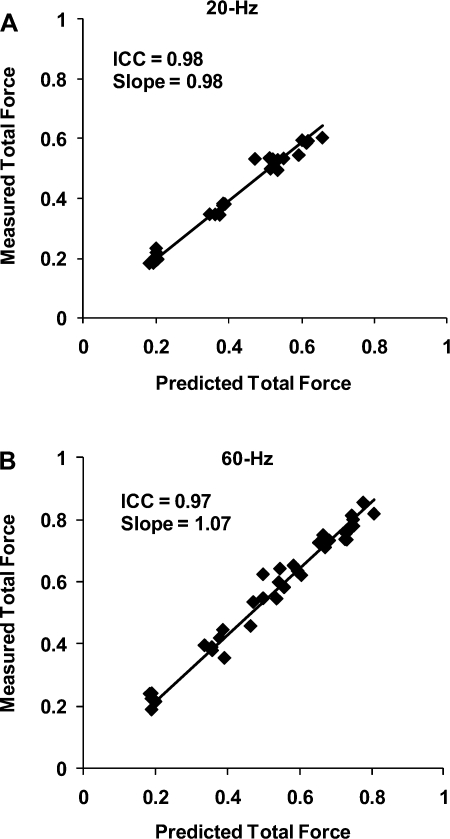
Complete data were collected for all six subjects from the validation phase of the study. For each subject tested, Eq. 1 fit the 30-Hz data with R² values ≥0.97 (Table 5). Similar to results seen during the model development phase, the values of the parameter N varied between subjects during the model validation phase (Table 5). ICCs comparing the normalized measured and predicted total forces showed ICC values of 0.98 and 0.97 for 20- and 60-Hz frequencies, respectively. In addition, scatter plots of predicted vs. measured total forces were plotted, and slopes of the trend lines with intercepts set at zero were calculated. A perfect model would have ICC values and trend line slopes equal to one. In the present study, the trend line slopes at 20- and 60-Hz frequencies were 0.98 and 1.07, respectively (Fig. 4).

Table 5.

Values of parameter N and their corresponding R² values for the six subjects tested during the validation phase of the study

	Subject 7		Subject 8		Subject 9		Subject 10		Subject 11		Subject 12
Stimulation Intensity	N	R2	N	R2	N	R2	N	R2	N	R2	N	R2
30% MF	1.16	0.99	0.95	0.97	2.03	0.99	1.35	0.99	1.51	0.98	1.16	0.99

Values are parameter N and the corresponding R² values for the 6 subjects tested during the validation phase of the study. The value of N was identified by fitting the measured total force data in response to a 30-Hz stimulation train with its intensity set at 30% MF and superimposed on volitional contraction levels of 10, 30, and 50% MF.

Fig. 4.

Plots of measured vs. predicted total forces at the validation frequencies of 20 (A) and 60 Hz (B) for 6 subjects tested during the validation phase of study. The total forces were normalized with respect to each subject's MaxForce. Intraclass correlation coefficients (ICCs) for agreement between measured and predicted data and the slopes of the trend lines (with intercepts set at 0) are reported at top left of each plot.

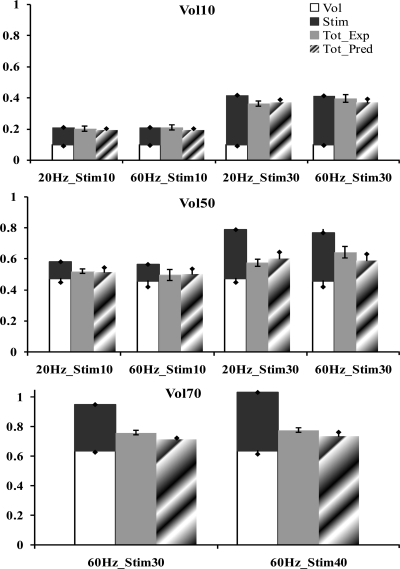
At a volitional contraction level of 10% of the MaxForce, our nonlinear model predicted the total force within the 95% confidence interval of the measured force for all the validation stimulation frequencies and intensities tested (Fig. 5A). The predictions of the simple linear model were also accurate at the 10% MaxForce volitional contraction level for all the frequencies and intensities tested, except at 30% MaxForce stimulation level and 20-Hz stimulation frequency, where the simple linear model overestimated the force by 5.2% (Fig. 5A). At volitional recruitment levels of 50 and 70% of the MaxForce, the simple linear model overestimated the total force at all the validation stimulation frequencies and intensities tested (Fig. 5, B and C). Specifically, at 50% MaxForce volitional level with the stimulation levels of 10 and 30% MaxForce, the average errors were 6.5 and 17%, respectively (Fig. 5B), and at 70% MaxForce volitional level with the stimulation levels of 30 and 40% MaxForce, the average errors were 19 and 25.6%, respectively (Fig. 5C). Our nonlinear model predicted the total forces within the 95% confidence interval of the measured forces at 50% MaxForce volitional level for all the validation frequencies and intensities tested, except at the 60-Hz stimulation frequency at a stimulation level of the 30% MaxForce, where the model underestimated the force by 5.2% (Fig. 5B). At 70% MaxForce volitional level, the nonlinear model underestimated the total force with an average error of 4.2% for the two stimulation intensities tested (Fig. 5C).

Fig. 5.

Bar graphs show the comparison of the mean predicted, normalized total forces of the linear model and our nonlinear model with respect to the mean measured, normalized total forces for 6 subjects tested during the validation phase of the study at a volitional level of 10% of MaxForce (Vol10; A), 50% of MaxForce (Vol50; B), and 70% of MaxForce (Vol70; C). Each x-axis represents the characteristics of the stimulation train (i.e., 20Hz_Stim10 represents a stimulation train with a frequency of 20 Hz and a stimulation intensity that produces 10% of MaxForce). Errors bars on the measured total force data (Tot_Exp; shaded bars) define the 95% confidence interval, and error bars on the remaining bars represent the standard deviations. The predictions of the linear model are shown as stacked bars of the forces in response to stimulation only (solid bars) and volitional contraction only (open bars). Tot_Pred, mean predicted, normalized total force data of nonlinear model (hatched bars).

In our model, with a value of N = 0, the term [(MaxForce − V)/MaxForce]^N = 1 and the force added onto the volitional contraction was equal to the stimulated force (Fig. 6A). Thus, for N = 0, the total force was given by a simple linear summation of V and S (T = V + S) as long as the total force did not exceed the MaxForce. Therefore, in Fig. 6A, the model predictions for N = 0 are depicted only for the condition of V + S ≤ MaxForce. Alternatively, for values of N >> 1 in Eq. 1, the term S[(MaxForce − V)/MaxForce]^N approached zero, so the additional force due to the stimulation declined rapidly until the volitional force was less than or equal to the stimulated force, at which point the additional force was equal to zero (Fig. 6A). In contrast, with a value of N =1, the additional force was S − (SV/MaxForce) and showed a linear decline as the volitional contraction level and followed the diagonal line in Fig. 6A.

Fig. 6.

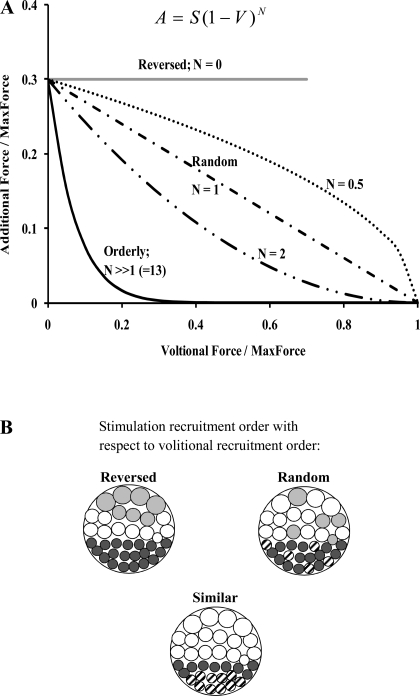
Schematic representation of the effect of various values of parameter N from Eq. 1 (A) on the additional force produced by the electrical stimulation onto the volitional contraction. The additional force, A, the stimulation force, S, and the volitional force, V, are normalized with respect to the MaxForce. The beach ball pattern shown in B represents the interaction of the activation in response to stimulation and volitional contraction. The volitional activation always follows the size principle and recruits progressively from small to large motor units, and volitionally activated motor units in A are represented by solid circles. For a reversed recruitment order of motor units by electrical stimulation with respect to volitional contractions, the motor units are recruited from large to small units and are shown as shaded circles. For a random recruitment order of motor units by electrical stimulation, the motor units shown as hatched circles represent those motor units already activated by volitional contraction and cannot be activated by electrical stimulation. For the case where the recruitment order of motor units by electrical stimulation is similar the volitional recruitment order, if the force produced by electrical stimulation is less than the volitional contraction, then no additional force is produced. Under these conditions, in the beach ball labeled “similar,” all the motor units recruited by electrical stimulation are shown as hatched circles.

DISCUSSION

In this study we developed a nonlinear mathematical model to predict the total force generated by healthy human quadriceps femoris muscles when submaximal levels of electrical stimulation are superimposed on submaximal levels of volitional contractions. In our model (Eq. 1), only one parameter, N, needed to be identified for each subject. Our model then predicted accurately (R² = 0.97) the total force in response to a wide range of stimulation intensities and frequencies that were superimposed on a wide range of volitional contractions. In comparison, a simple linear model, which predicted the total force as a sum of the forces in response to electrical stimulation and volitional contractions, overestimated the measured forces, especially at higher levels of volitional contractions.

Because the term S[(MaxForce − V)/MaxForce]^N from Eq. 1 describes the additional force produced when electrical stimulation is superimposed on a volitional contraction, we posit that the parameter N can be used to provide insights into the interactions between electrical stimulation and volitional contractions. Interaction between superimposed electrical stimulation and volitional activation arise from the motor unit recruitment order of electrical stimulation with respect to volitional activation and the firing rates of motor units driven by electrical stimulation versus volitional activation. Although the recruitment of motor units by volitional activation follows the Henneman size principle with progressive recruitment from smaller to larger motor units (10), the order of recruitment of motor units in response to electrical stimulation is controversial (9, 15, 29). If the order of recruitment by electrical stimulation was reversed compared with volitional activation (i.e., large to small motor units), as posited by a few experimental studies (6, 17, 29), the force added onto the volitional contraction by electrical stimulation would be equal to the stimulated force as long as the sum of the volitional and stimulated forces was less than or equal to the MaxForce (Fig. 6). In our model, with a value of N = 0, force added onto the volitional contraction would be equal to the stimulated force (Fig. 6). Thus, for N = 0, our model would predict responses consistent with a reversed recruitment order of electrical stimulation with respect to volitional contraction, and the total force would be given by a simple linear summation of V and S (T = V + S) as long as the total force did not exceed the MaxForce.

Alternatively, if the recruitment of motor units by electrical stimulation was the same as the volitional recruitment, the additional force produced by electrical stimulation would decline proportional to the volitional contraction, reaching zero when the volitional contraction was greater than or equal to the force produced by electrical stimulation (Fig. 6). For values of N >> 1, the additional force due to the stimulation declined rapidly until the volitional force was less than or equal to the stimulated force, at which point the additional force was equal to zero (Fig. 6). Thus, for N >> 1, Eq. 1 described an orderly recruitment of electrical stimulation that was similar to volitional contractions. In contrast, if the recruitment order in response to electrical stimulation is random, as has been suggested by previous studies (2, 14, 15), the number of commonly activated motor units should be proportional to the product of stimulation and volitional forces, and the additional force produced by electrical stimulation should decline linearly as the volitional contraction level is increased (Fig. 6). In Eq. 1, the random order of recruitment by electrical stimulation was modeled with a value of N =1 so that the additional force follows the diagonal line in Fig. 6. In summary, as the value of N goes from zero to one, the recruitment order of electrical stimulation goes from a reversed to a more random order of recruitment with respect to that during volitional contractions, and as the value of N goes from one to much larger values, the recruitment order of electrical stimulation goes from a more random recruitment order to one similar to that of volitional contractions.

Although parameter N may help to provide valuable insights into the recruitment order of motor units by electrical stimulation with respect to volitional activation, it is difficult to use parameter N to explain the interaction of the firing rates of motor units in response to simultaneous electrical stimulation and volitional activation. During electrical stimulation, action potentials in response to stimulation will propagate along the motor neurons in both the orthodromic and antidromic direction. The orthodromic action potentials from the stimulation will cause the recruited motor units to fire at the electrical stimulation frequency. When electrical stimulation is superimposed during a volitional contraction, the antidromic action potentials may collide with voluntarily produced action potentials and reduce the motor neuron discharge rate immediately after the stimulus (11). Herbert and Gandevia (11) mathematically modeled the effect of superimposing a single pulse during volitional activation and showed that due to antidromic collisions, the modeled additional force generated by the single pulse declined linearly with increases in volitional force. In contrast, without antidromic collisions, the decline in the modeled additional force was less rapid and more nonlinear (see Fig. 8B in Ref. 10). In the present study, based on the results from Herbert and Gandevia, we posit that for N > 0, there is overlap in the motor units recruited by volitional activation and electrical stimulation, and that the antidromic collisions serve to reduce the additional force produced by electrical stimulation (Fig. 6A). In contrast, for N = 0, there is no overlap in the motor units and hence no antidromic collisions and no reduction in the additional force produced by electrical stimulation (Fig. 6A).

For the 12 subjects tested in the current study, at 30-Hz stimulation frequency with a stimulation intensity level of 30% of the MaxForce, the average value of N was 1.78 (±0.67), suggesting that recruitment order due to electrical stimulation was relatively random with respect to volitional contraction (see Tables 2 and 3; Fig. 6). In addition, our results showed that the random recruitment order of motor units in response to electrical stimulation held true for a range of stimulation intensities (see Table 2) and frequencies tested. Our finding of a random order of recruitment with electrical stimulation is consistent with previous studies (2, 14, 15) and with the data presented in the review article by Gregory and Bickel (9) showing that electrical stimulation is “nonselective and that muscle fibers are recruited without obvious sequencing related to fiber types.”

Our model accurately predicted the interaction between submaximal volitional activation and submaximal activation in response to electrical stimulation when a burst of stimulation (20, 30, and 60 Hz; 500 ms) was superimposed on a volitional contraction. Although Herbert and Gandevia (11) accurately modeled the force produced by a single or doublet supramaximal stimulus superimposed on a range of voluntary contractions, their study did not model the effect of superimposing a submaximal stimulation train on submaximal volitional contractions. Langzam and colleagues (18, 19) used EMG to determine the volitional component from the total force generated when volitional activation is superimposed on the force produced by electrical stimulation during isometric contractions of the human tibialis anterior muscle. Their results showed that the total force produced is a linear combination between the volitional and stimulation forces. Our results, however, indicate that the total force produced is a nonlinear function of the volitional and stimulation forces. The reasons for the discrepancy in describing the total force between the study by Langzam and colleagues (18, 19) and ours could be attributed to the type of muscle tested (tibialis anterior vs. quadriceps femoris) and differences in methodology (volitional activation superimposed on force generated by electrical stimulation vs. electrical stimulation superimposed on volitional activation). However, we believe that the primary reason why Langzam and colleagues (18, 19) were able to use a linear model to describe their data was because lower total forces (20–30% of the MaxForce) were generated during their experiments, and at these lower force levels even our data showed that a linear model would suffice to describe the data (see Fig. 5A). At higher force levels explored (e.g., 30–85% of the MaxForce), because there is an increasing overlap in the motor unit pool recruited by volitional activation and electrical stimulation, a nonlinear model such as ours would be required to describe the data accurately (Fig. 5, B and C).

In the present study, Eq. 1 was validated on healthy quadriceps femoris muscle at one particular muscle length when the muscle was potentiated but not fatigued. It would be interesting to see whether the form of Eq. 1 remains valid for other muscles and physiological conditions such as changes in muscle length and fatigue. In conclusion, we have proposed a simple nonlinear model that describes the forces produced by superimposition of electrical stimulation onto submaximal volitional contractions, using a wide range of stimulation intensities and frequencies. We believe that our model (Eq. 1) may be helpful to clinicians and scientists to allow them to predict the amount of stimulation needed to produce the targeted force levels in individuals with partial paralysis.

GRANTS

This study was supported by National Institutes of Health Grants NR010786 and HD038582.

DISCLOSURES

No conflicts of interest, financial or otherwise, are declared by the author(s).