Frequency-Velocity Mismatch: A Fundamental Abnormality in Parkinsonian Gait

Abstract

Gait dysfunction and falling are major sources of disability for patients with advanced Parkinson's disease (PD). It is presently thought that the fundamental defect is an inability to generate normal stride length. Our data suggest, however, that the basic problem in PD gait is an impaired ability to match step frequency to walking velocity. In this study, foot movements of PD and normal subjects were monitored with an OPTOTRAK motion-detection system while they walked on a treadmill at different velocities. PD subjects were also paced with auditory stimuli at different frequencies. PD gait was characterized by step frequencies that were faster and stride lengths that were shorter than those of normal controls. At low walking velocities, PD stepping had a reduced or absent terminal toe lift, which truncated swing phases, producing shortened steps. Auditory pacing was not able to normalize step frequency at these lower velocities. Peak forward toe velocities increased with walking velocity and PD subjects could initiate appropriate foot dynamics during initial phases of the swing. They could not control the foot appropriately in terminal phases, however. Increased treadmill velocity, which matched the natural PD step frequency, generated a second toe lift, normalizing step size. Levodopa increased the bandwidth of step frequencies, but was not as effective as increases in walking velocity in normalizing gait. We postulate that the inability to control step frequency and adjust swing phase dynamics to slower walking velocities are major causes for the gait impairment in PD.

INTRODUCTION

Gait dysfunction with falling is a major source of disability for patients with advanced Parkinson's disease (PD) and is not adequately controlled with currently available medical or surgical therapies. Current thinking suggests that the gait dysfunction is largely due to an inability to regulate stride length. That is, the steps taken during walking are short and do not lengthen when PD subjects try to increase their walking speed (Giladi et al. 1997; Morris et al. 1996). Training and visual cues can transiently normalize stride length, but when attention is diverted, gait promptly reverts to the shortened stride lengths and higher step frequencies (Knutsson 1972; Morris et al. 1996, 1998). It has been proposed that step rate increases to compensate for the shortened stride length (Morris et al. 1996, 1998). However, if increases in step frequency were simply compensating for limited stride length, then they should decrease at slower walking velocities. Instead, when PD subjects walked slowly on a treadmill, their step frequencies remained high (Cho 2008). This suggests that the high PD step frequencies are not a compensatory response. Thus although shortened stride length is an undeniable kinematic deficiency of PD gait (Cho 2008; Cho et al. 2006b; Morris et al. 1996, 1998), the underlying abnormalities that result in truncated stride length and high step frequency are still not clear.

During treadmill locomotion, the dynamic and kinematic parameters of locomotion in normal subjects are highly conserved, indicating that the timing of the steps is correlated with both walking velocity and step frequency (Osaki et al. 2007, 2008). Although linked during normal gait (Hirasaki et al. 1999; Winter 1983, 1989), step frequency and walking velocity can be dissociated by auditory and visual pacing (Osaki et al. 2008). When walking velocity is held constant but step frequency varies, peak forward velocity of the foot during the swing phases is fixed and does not vary with alterations in stride frequency. When step frequency is held constant and walking velocity is varied, the size of the foot movement is fixed, but the peak velocity of the forward swing increases. The main sequence (peak velocity vs. amplitude) relationships and the shape of the phase-plane trajectories of the swing phases reflect these changes.

Based on these observations, a model was developed that uses an internal representation of step frequency to modulate a feedback control mechanism that governs the dynamics of the swing, whereas peak velocity during the swing is determined by walking velocity (Osaki et al. 2007). This simple model accurately predicted forward step dynamics over a wide range of walking velocities and step frequencies in normal subjects (Osaki et al. 2008). Based on this model, we postulated that the shortened stride length in PD at low walking velocities is due to an alteration in central pacing, causing dissociation between step frequency and walking velocity (Cho et al. 2006a). In the present study, we examined step frequency and its effect on foot dynamics as PD subjects walked on a treadmill over a range of velocities and when they were paced over a range of auditory frequencies. We also determined the effect of levodopa (LD) on step dynamics.

METHODS

Subjects

Ten moderately advanced PD subjects and seven healthy normal controls participated in this study. Each person signed an informed consent approved by the Mount Sinai School of Medicine Institutional Review Board. The PD subjects were diagnosed as having PD by a movement disorders specialist and each had difficulty with gait. Motor function was evaluated using part III of the Unified Parkinson Disease Rating Scale (UPDRS III) and the modified Hoehn and Yahr (H&Y) staging system (Fahn et al. 2003). The UPDRS III has scores ranging from 0 to 108, with higher scores representing more severe disease. The baseline demographics, disease duration, LD equivalence given, and presence of dyskinesias during examination are listed in Table 1. The UPDRS III, gait subscores (items 26–30 on the UPDRS), lower extremity rigidity scores, freezing score (item 14 on the UPDRS), and Hoehn and Yahr scale are tabulated in Table 2. PD subjects were examined in the practically defined “off” state (∼12 h after the last dose of LD) and in the best “on” state (∼45 min after receiving 150% of their usual morning dose of LD). The data from all PD subjects were pooled in the graphs shown in the figures from which the analysis and conclusions were drawn. Control subjects (four males, three females, ranging in age from 30 to 58 yr; mean: 36.6 yr) had no evidence of neurological disease.

Table 1.

The PD subject, age, gender, disease duration, LD equivalence, and presence of dyskinesias

Subject	Age, years	Gender	Disease Duration, years	LD Equivalence Given, mg	Dyskinesias at Testing
1	78	F	3	100	No
2	48	M	7	300	No
3	47	M	5	150	Yes
4	66	M	10	225	No
5	65	M	9	150	Yes
6	61	M	13	200	No
7	54	M	9	300	Yes
8	75	M	7	200	No
9	68	M	13	300	Yes
10	67	M	2	75	No

Table 2.

Clinical scores

Subject	UPDRS III		Gait Score		Lower Extremity Rigidity Score		Freezing Score		Hoehn and Yahr (H&Y) Stage
	Off State	On State	Off State	On State	Off State	On State	Off State	On State	Off State	On State
1	28	21	8	7	3	2	0	0	3.0	3.0
2	15	3	7	0	0	0	0	0	3.0	2.0
3	28.5	17	6	4	2	2	1	0	3.0	2.0
4	49	38.5	9.5	8	5	3.5	0	0	3.0	3.0
5	41.5	27.5	11	4	3	2.5	3	1	3.0	2.0
6	36	25	5.5	2.5	1	0	0	0	2.5	2.0
7	12.5	2	3	0	0	0	1.5	0	2.5	1.0
8	54	43.5	13.5	9.5	1.5	1.5	1.5	0	3.0	3.0
9	34	21	11.5	6.5	0	0	3	1	4.0	3.0
10	22.5	17.5	3	1.5	0	0	1	0	2.0	2.0

The “Freezing Score” is item 14 in the Unified Parkinson Disease Rating Scale (UPDRS).

Experimental protocol

Subjects walked on a motor-driven linear treadmill (Q55, Quinton Instrument, Bothell, WA) at up to six velocities: 0.6, 0.9, 1.2, 1.5, 1.8, and 2.1 m/s. All subjects were safely able to walk ≤1.2 m/s. Subjects watched a visual target 1.0 m from the head at eye level. Each trial contained ≥10 complete stride cycles. At each treadmill speed, subjects walked with their most comfortable cadence. Six of the 10 PD subjects walked at step frequencies that were paced by auditory stimuli. Kinematic and dynamic parameters were evaluated using Froude numbers to normalize the data (Hof 1996; Kuo 2001; Minetti 2001a,b).

Data acquisition

Infrared light-emitting diodes (LEDs) were used to track foot movements. Movements of the right foot were recorded using the OPTOTRAK 3020 video motion analysis system (Northern Digital, Waterloo, Ontario, Canada; for a complete description of the OPTOTRAK characteristics, see Hirasaki et al. 1999).

Marker placement and measurement coordinate system

Foot movements were determined in three dimensions by combining groups of markers into rigid bodies. Foot markers were placed on the right shoe over the fifth metatarsal (toe) and the lateral calcaneus (heel) and over the lateral malleolus (ankle). A marker placed on the greater trochanter (hip) was used to determine the length of the leg. Other reference markers were placed on the head, trunk, thigh, and lower leg (for a complete description, see Osaki et al. 2007, 2008).

Four markers embedded 24 cm apart in a plastic plate attached to the side of the treadmill were used to determine the spatial coordinate frame for the foot movements. The X-axis (positive forward) was along the direction of walking on the treadmill, the Z-axis was spatially vertical (upward positive), and the Y-axis was horizontal relative to the direction of walking (leftward positive), forming a right-handed coordinate system.

Normalization of length and temporal vectors

The data were normalized and transformed into dimensionless quantities, which normalized the durations of the gait cycles for subjects with different body sizes and leg lengths (Hof 1996). Length was normalized to individual leg length l₀, acceleration to the acceleration of gravity a_g, and time to the period of a naturally oscillating leg (2π $\sqrt{l/a_g}$), where l is the distance from the hip to the center of mass of the leg (0.4l₀) (Chandler et al. 1975). The average leg length for all subjects was 0.84 m; thus the normalization time for a gait cycle was about 1.16 s, referred to as a “leg-period” in this study.

Froude numbers have been used extensively in gait kinematics to normalize velocity (Minetti 2001a,b). Some authors use l₀g₀, which is the square of velocity, to represent a Froude number of 1.0 (Kram et al. 1997; Vaughan and Malley 2005). Since many of our plots are related to velocity, however, we used $\sqrt{l_0{a}_g}$ to represent a Froude number of 1.0 (Osaki et al. 2007, 2008). Based on our normalization, the actual velocity in meters/s was about threefold the Froude number. Table 3 gives the relationship between the Froude numbers and the walking velocities in meters/s and also gives a qualitative descriptor to help identify the nature of the gait. Angular deviations are dimensionless quantities and do not require normalization.

Table 3.

Relationship between dimensionless normalized walking velocities (Froude numbers) and walking velocities for an average-height individual (5′10″) in m/s

Velocity of Walking (Froude Number)	Velocity of Walking for an Average-Height Individual, m/s	Velocity Descriptor
0.21	0.6	Very slow sauntering gait
0.31	0.9	Slow gait
0.42	1.2	Medium gait
0.52	1.5	Brisk gait
0.63	1.8	Fast gait
0.73	2.1	Very fast gait—close to running

At each walking velocity, a “Velocity Descriptor” is included to classify the briskness of the gait in qualitative terms.

Methods of data analysis and phase-plane trajectory assessment

Stride length, stride frequency, stance duration, and swing duration (Morris et al. 2005; Nutt and Thompson 1993; Osaki et al. 2007, 2008; Winter 1983, 1989) were based on the relationships that step frequency is equal to twice the stride frequency and that the product of stride frequency and stride length is equal to walking velocity

$$\text{Walking}\text{velocity}=\left(\text{stride}\text{length}\right)\times \left(\text{stride}\text{frequencey}\right)$$ (1)

Since the duration of the stride is inversely proportional to stride frequency, Eq. 1 would imply that there is an approximate inverse relationship between duration of the stance and swing phases with walking velocity. Therefore relationships between duration and velocity of both stance and swing phases were approximated as hyperbolic functions (Osaki et al. 2007, 2008).

Both step length and step frequency cannot be linearly related to walking velocity (Eq. 1); however, step frequency in PD subjects was confined to a limited range and stride length was approximately linearly related to walking velocity within this range. Therefore linear regression analysis was used to compare the slopes and intercept of the regression curves among normal subjects and PD subjects on and off antiparkinsonian medication.

Analyses were performed to assess peak Toe-X velocity versus range of Toe-X positions (Fig. 4, A and B). This is referred to as a phase-plane analysis and gives important information about the dynamics of the foot during the swing and stance phases (Osaki et al. 2007, 2008; Xiang et al. 2007). We also analyzed the Z-axis position of the foot versus percentage of gait cycle to determine information about toe clearance. Within the PD group, comparisons were also performed during the on- and off-LD states.

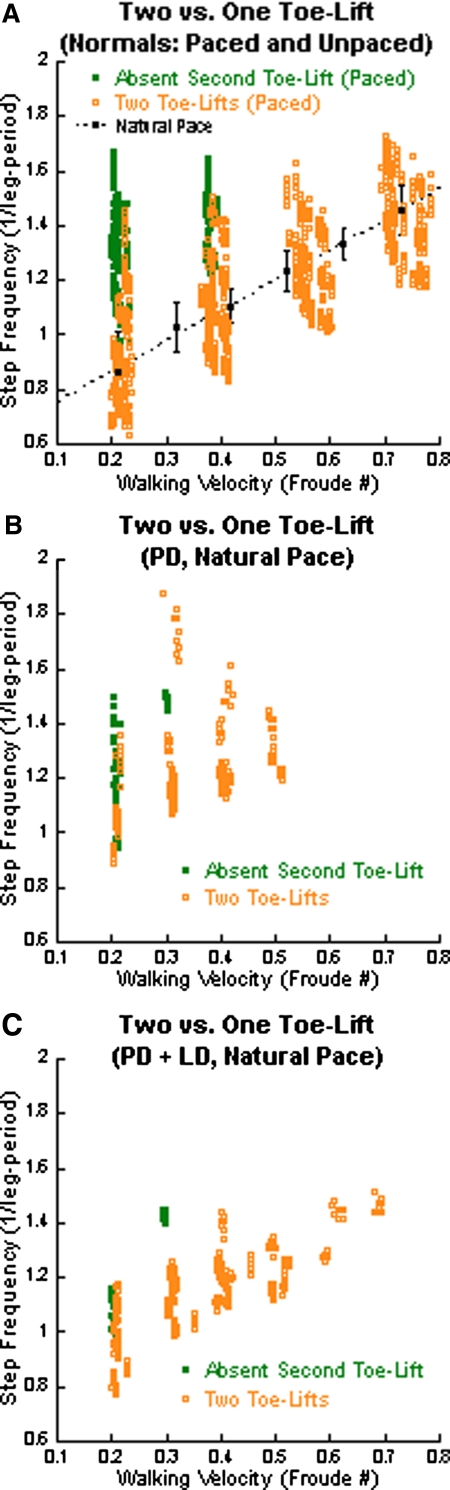
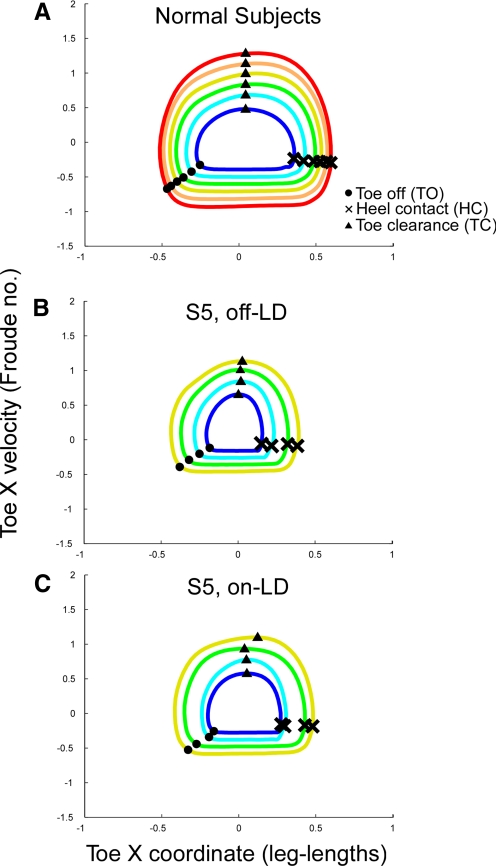
Fig. 4.

Absence of the second toe lift as a function of the frequency-velocity dissociation. The green solid circles represent gait cycles without the second toe lift and the orange open circles represent gait cycles with 2 toe lifts. A: paced data for normal subjects (green and orange circles). The black circles and error bars represent the mean ± SD of data from normal subjects at their natural pace. The black-dotted line is the linear regression. Note the loss of the second toe lift at higher frequencies of pacing at lower walking velocities. B: loss of second toe lift in PD subjects walking at their natural pace (green dots) and reappearance when walking faster (orange dots). C: reduced loss of second toe lift in PD + LD subjects walking at their natural pace.

Accuracy and variance of pacing

Accuracy of pacing was defined as the difference between the subject's step frequency and auditory pacing stimulus frequency. Positive values of accuracy corresponded to step frequencies that were higher than stimulus frequency, whereas negative values of accuracy had step frequencies that were lower than the stimulus frequency. The variance was the absolute value of the SD in step frequency for a given pacing frequency and walking velocity.

Statistical analysis

F-tests, t-tests, and Welch tests were done using the statistical package in Octave 3.0, an open source Matlab equivalent. We evaluated the regression of dependent variables as a function of an independent variable for different treatment conditions using indicator variables (Kirkwood and Sterne 2003).

We tested for coincidence of the regressions for pairs of the three classes (normal, off-LD, on-LD) by fitting the data to a general linear model with an indicator variable z, which was set to either 0 or 1, dependent on the class to which the data belonged. ANOVA was used to determine the significance level of whether the data could be fit by two different regressions or were coincident (Kirkwood and Sterne 2003). Programs were written in Octave to perform the statistical evaluation and return the P values for the level of significance.

RESULTS

Temporal properties of PD gait

PD subjects had a small range of walking velocities and stride frequencies (Fig. 1, A–D, blue solid lines and shaded area) relative to normal subjects (black-dotted lines). Their swing durations were approximately equal to those of normal subjects at about 0.4 Froude. The largest differences in stance and swing durations between normal and PD subjects were at the lowest walking velocity. The PD stance and swing phases were 0.54 and 0.35 leg-periods, whereas the normal stance and swing phases were 0.77 and 0.42 leg-periods, respectively. Despite these differences, the fundamental hyperbolic relationships between stance and swing durations and walking velocities were maintained in the PD subjects. Thus although they never achieved high walking velocities, the curves for both swing and stance durations of the PD subjects, when extrapolated, reached the same asymptotes as those of the normal subjects (Fig. 1A).

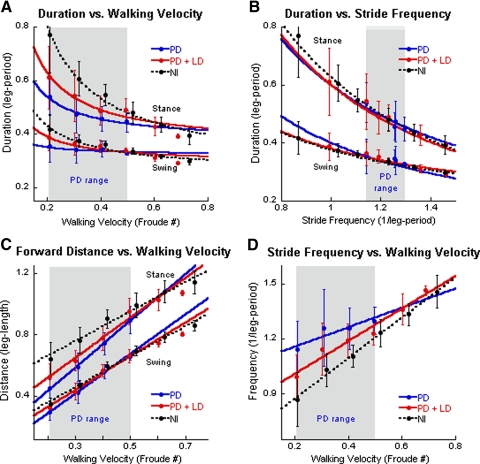
Fig. 1.

Analysis of gait as a function of walking velocity and stride frequency in Parkinson's disease (PD), PD + levodopa (LD), and in normal subjects. A: duration of stance and swing phases vs. walking velocity. In this and in subsequent figures, the blue symbols and lines represent data from PD subjects without medication, the red symbols and lines represent data from PD subjects with LD (see methods for details), and the black symbols and dotted lines represent data from normal subjects. The filled circles are means and the vertical lines ±1SD. The gray areas show the range of data from the PD subjects off LD. B: duration of stance and swing phases vs. stride frequency. C: distance covered in stance and swing phases as a function of walking velocity. D: stride frequency as a function of walking velocity. The top 3 traces represent stance phases and the bottom 3 traces represent swing phases in A–C.

Durations of the stance and swing phases of the PD subjects were also hyperbolically related to stride frequency (Fig. 1B, blue solid line). The mean duration of the stance and swing phases fell along the regression curve for normal subjects (Fig. 1B, black-dotted line). The range of stride frequencies for PD subjects was restricted to 1.14–1.30 leg-period⁻¹ (Fig. 1B, blue data points in shaded area), highlighting a severely limited range of stride frequencies in PD gait.

Spatial properties of PD gait

Since the body is stationary in space on the treadmill, the distance covered re the treadmill during stance and swing phases is equal to the treadmill velocity multiplied by the duration of the corresponding stance and swing phases (Osaki et al. 2007, 2008). Stance distances were most affected at lower walking velocities. In this range, the distances covered by PD subjects were 0.45 leg-length and by normal subjects were 0.64 leg-length. At higher velocities, the amplitudes of the stance phases of the PD subjects (0.90 leg-length) were close to those of the normal subjects (0.99 leg-length). This reflected the steeper slope of the distance versus velocity relationship for the stance phases in PD (Fig. 1C, Stance; P < 0.001). The PD swing distances were close to those of normal subjects across all walking velocities. Thus stride length was significantly shorter in PD than that in normal subjects (Morris et al. 1996, 1998, 2005), but only at lower walking velocities. At higher walking velocities, PD stance and swing distances were similar to those of normal subjects (Fig. 1C).

A striking finding was that stride frequencies in PD subjects were significantly higher than those in normal subjects at lower walking velocities (Fig. 1D, PD, blue solid line; Nl, black-dotted line), but the differences were minimal at walking velocities of about 0.5 Froude. The slope of the stride frequency/walking velocity relationship (1·leg-period⁻¹·Froude⁻¹) was 0.53 in PD subjects compared with 1.1 in normal subjects (Fig. 1D). Variances around the means were larger at the lower walking velocities in both PD and normal subjects. In summary, the gait of PD subjects had a restricted range of stride frequencies and shorter stride distances at lower walking velocities than in normal subjects.

Effect of LD on PD gait

Administration of LD improved the relationships between stance and swing duration (Fig. 1A), stride length (Fig. 1B), and stride frequency (Fig. 1D) with respect to walking velocity in PD subjects (cf. red data points and red lines with black-dotted and blue lines), but did not completely normalize the gait in any sphere. The largest degree of improvement was in the duration of the stance and swing phases at the lowest walking velocity (i.e., at 0.2 Froude; Fig. 1A). At higher velocities, the stance and swing durations approached normal values and, at about 0.4 and 0.6 Froude, the swing and stance durations of the PD + LD were comparable to those of normal subjects (see Table 3 for comparison between velocity in meters/s and duration in leg periods and Froude numbers). Stance distances were closer to normal values (Fig. 1C), but remained significantly shorter in the PD + LD subjects compared with those of normal subjects.

LD broadened the range of stride frequency to include lower step frequencies (from 1.00 to 1.50 leg-period⁻¹ (Fig. 1B, compare red plots to blue plots), while maintaining the relationship between stance and swing durations and stride frequency (Fig. 1B, red solid and black-dotted lines). Over this range, there was no significant difference between the normal, PD, and PD + LD subjects, suggesting that the dynamic relationships between stance and swing durations and stride frequency were the same. Despite the broadening effect of LD on the range of stride frequencies, PD + LD subjects still had a restricted range at the lower stride frequencies compared with that of normal subjects.

LD also increased the distances that the body traversed re the treadmill during the stance phase (Fig. 1C). The duration of the stance was longer after administration of LD at the lower walking velocities and the duration/stride frequency relationship approximated that of the normal subjects more closely (Fig. 1B). However, there were still substantial differences between stance phase durations, stance distances, and stride frequency as a function of walking velocity (Fig. 1, A, C, and D).

Pacing of step frequencies in PD subjects

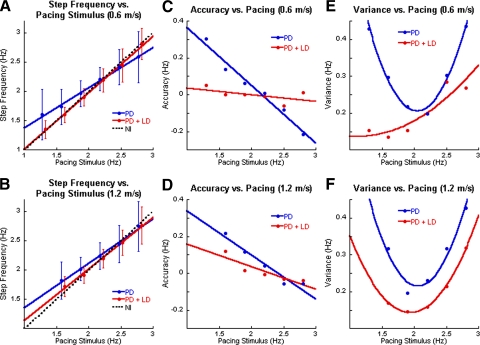
We next considered whether the high step frequency of PD subjects at low walking velocities was a primary abnormality or a consequence of the shortened step length. This was tested by giving auditory pacing cues at frequencies from 1.3 to 2.8 Hz at treadmill velocities of 0.6 and 1.2 m/s (Osaki et al. 2008). Subjects were instructed to synchronize the pace of their steps to the frequency of the external auditory stimulus. The slope of the PD step frequency/pacing stimulus frequency was 0.69 at 0.6 m/s (Fig. 2A) and 0.76 at 1.2 m/s (Fig. 2B) and the slopes of the data intersected the unity slope of the normal subjects for pacing stimuli between 2.2 and 2.5 Hz. This showed that the PD step frequencies were higher than those of normal subjects at lower pacing frequencies and lower at higher frequencies at both walking velocities.

Fig. 2.

A and B: step frequency (ordinate) as a function of auditory pacing stimulus (abscissa) when walking at 0.6 m/s (A, C, E) and at 1.2 m/s (B, D, F). As in Fig. 1, the blue and red symbols represent data from PD subjects off and on LD, and the black-dotted lines in A and B represent data from normal subjects. Filled circles and error bars are means ± 1SD. C and D: accuracy of stepping (ordinate) as a function of the pacing stimulus (abscissa). E and F: variance as a function of auditory pacing.

The accuracy of matching step frequency to the pacing frequency was defined as the difference between the subject's step frequency and the pacing stimulus frequency. Accuracy declined linearly at both walking velocities (Fig. 2, C and D). The linear regression of the PD subjects was significantly different from that of normal subjects, which was a line with a zero slope that indicated perfect accuracy (Osaki et al. 2008; Fig. 2, C and D). The most accurate step frequency at 0.6 m/s was 2.2 Hz with a difference of 0.039 Hz. At 1.2 m/s, the most accurate step frequency was at 2.5 Hz with a difference of 0.007 Hz.

The variance indicated how well subjects could maintain a consistent step frequency. The variances changed parabolically with changes in the pacing stimuli and had a minimum at around 2.1 Hz at 0.6 and 1.2 m/s (Fig. 2, E and F). These data show that another deficiency of PD locomotion is an inability to maintain a constant step frequency that is different from their natural step frequency. The accuracy of following the pacing stimulus was independent of walking velocity.

Effects of LD on pacing

With LD, the step frequency more closely matched the frequency of the auditory stimulus at both 0.6 and 1.2 m/s, with slopes that were 0.96 and 0.88, respectively (Fig. 2, A and B). LD improved the accuracy of the linear regression of step frequency as a function of pacing frequency (Fig. 2, C and D), with slopes of −0.036 at 0.6 m/s and −0.122 at 1.2 m/s (ANOVA using indicator variables; P < 0.001), and decreased the variance of step frequency at all pacing stimuli and at both low and high velocities (Fig. 2, E and F). At 0.6 m/s, the minimal variance was shifted to the lower pacing frequencies, with a minimum at <1.5 Hz (Fig. 2E). As the pacing stimulus frequency increased, the variance increased parabolically. There was a more distinct minimum at 1.9 Hz for walking at 1.2 m/s (Fig. 2F), with a symmetric increase in variance at 1.2 m/s. Although the parabolic relationship was similar to the variances without LD, the overall values were lower across all pacing frequencies and at both low and high velocities.

Kinematics and dynamic of foot movement: Z-axis movements of normal subjects

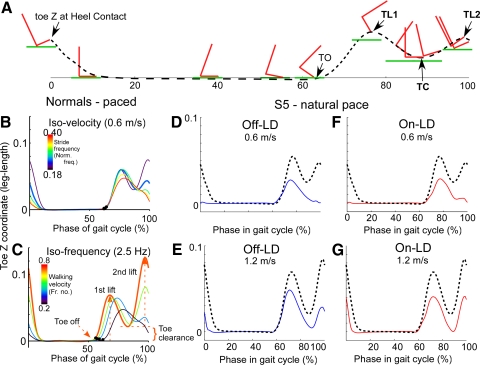
Z-axis motion of the foot during the swing phases is closely correlated to stride length in normal subjects (Osaki et al. 2007). Swing phases begin with a rise in the toe after the heel is elevated at the end of stance (Fig. 3A, toe off [TO]), with a peak in the Toe-Z movement at the point when the knee starts to extend (Fig. 3A, toe lift 1 [TL1]). The foot then swings forward along the X-axis, with the peak Toe-X velocity occurring at the point of toe clearance, which we defined as the point at which the toe has minimal distance to the walking surface (Fig. 3A, toe clearance [TC]). This occurs in normal subjects as the swing leg passes the stance leg (Winter and Rogers 1992). The toe is elevated again just before the heel makes contact with the ground, which is the second toe lift (Fig. 3A, toe lift 2 [TL2]).

Fig. 3.

A: pictorial representation of X-axis and Z-axis translation of the foot and toe (red) relative to the walking surface (green) during the stride cycle. The stance phase begins when the toe is elevated from the surface and the foot makes heel contact (0%). TO, toe off; TL1, first toe lift; TC, toe clearance; TL2, second toe lift. The black dashed line shows the trajectory of the toe at various phases of the stride cycle. B, D, and F: Toe-Z variation over the stride cycle during auditory pacing at different frequencies while walking at a constant velocity of 0.6 m/s in normal subjects (B), PD subjects off (D) and on LD (F). The bar on the left of the graph in B is a color representation of step frequency with lower frequencies shown as purple and higher shown as red. The black-dotted lines in D and F are from normal subjects, shown for comparison. Note the absence of the second toe lift in normal subjects in B, when the step frequency was high, and the absent second toe lift in D and F when PD subjects walked at 0.6 m/s. C, E, and G: Toe-Z variation over the stride cycle while normal subjects (C) walked at different velocities at a constant step frequency of 2.5 Hz. The bar on the left of the graph in C is a color representation of walking velocity with lower velocities shown as purple and higher shown as red. E and G: at 1.2 m/s, the PD subjects off (E) and on LD (G) had a second toe lift. The black-dotted lines represent data from normal subjects walking at 1.2 m/s.

The height of toe lift 2 is proportional to step length (Osaki et al. 2007) and, if walking velocity is held constant, the second toe lift diminishes as step frequency increases. At the highest step frequency, there is no toe lift 2 or toe clearance at the end of the swing phases (Fig. 3B, red curve; Osaki et al. 2008). Similarly, if walking velocity is decreased at a fixed step frequency (2.5 Hz), there is also no toe lift 2 or toe clearance at the lowest velocity (Fig. 3C, purple curve).

Both stride frequency and walking velocity had an effect on toe lift in normal subjects (Fig. 4A). The black-dotted line represents the linear fit of normal subjects walking at a natural pace. Second toe lifts were absent when stride frequency was paced too high for a given velocity (Fig. 4A, green solid dots). In contrast, both toe lifts and the toe clearance were present when walking was paced at lower frequencies (Fig. 4A, orange open circles). Two toe lifts were always present under walking conditions with higher velocities.

Z-axis movements: PD subjects

Typical derangements in vertical toe movements in PD subjects, walking at lower velocities, was a small first toe lift and an absent second toe lift (Fig. 3D, blue solid line). The second toe lift and the toe clearance became larger with increases in walking velocity (Fig. 3E). These patterns were observed in all PD subjects while walking at their natural pace at various velocities.

The second toe lift was significantly diminished or absent in PD subjects when they walked at low velocities (Fig. 4B, green solid dots). At these velocities, step frequencies were higher than those in normal subjects (see also Fig. 1D). By increasing walking velocity, the step frequency/walking velocity relationship was closer to that of normal subjects, with an improved second toe lift and toe clearance. Furthermore, the absent second toe lift in PD subjects at low velocities was observed in normal subjects when the step frequency was high and the walking velocity was low (Osaki et al. 2008) (Fig. 3B, red line; Fig. 3C, purple line).

The small increase in the second toe lift and toe clearance became more apparent with LD at both velocities (compare blue line of Fig. 3D to the red line of Fig. 3F and the blue line of Fig. 3E to the red line of Fig. 3G). However, increases in walking velocity had a greater impact than LD on increasing the size of the second toe lift (compare Fig. 3, D to E, blue lines; and Fig. 3, F to G, red lines). PD subjects treated with LD had fewer gait cycles with only one toe lift (Fig. 4C, green solid dots, one toe lift; orange open circles, two toe lifts). LD also helped to lower the natural step frequency at lower walking velocities, increasing the proportion of gait cycles with two toe lifts (Fig. 4C, orange open circles). Two toe lifts and the toe clearance were present at higher velocities (Fig. 4, B and C, orange open circles). Thus LD broadened the natural step frequency to include lower frequencies at lower walking velocities, preserving toe clearance and the second toe lift of PD gait cycles.

Forward-motion phase-plane dynamics

The dynamics of the forward swing phases in normal and PD subjects was compared using phase-plane plots, i.e., the relationship between toe velocity relative to Toe-X translation. In normal subjects, the swing phase (from TO to HC) of the phase-plane plots have an approximately circular shape (Fig. 5A). With increasing velocity, the radius of the swing phase trajectory increases. PD subjects had similar shapes of the forward-motion phase-plane trajectory of the swing phases (e.g., S5 in Fig. 5B). The peak Toe-X velocity during the swing was approximately equal to that of normal subjects, but the Toe-X translation was truncated (Fig. 5B). This prematurely ended the swing phase and caused early initiation of the swing of the other foot. In turn, this shortened the stance phase of the contralateral foot. This eccentricity was partially improved by LD (Fig. 5C).

Fig. 5.

Comparison of phase-plane trajectories of normal (A) and PD subject, S5, off- (B) and on-LD (C). The colors of the traces represent different walking velocities, with red representing the highest velocity and purple representing the lowest velocity. A: normal subjects had a close to circular trajectory during the swing phase, and the peak velocity and step size increased with increases in walking velocity. B: PD subject off-LD had an eccentric phase-plane trajectory, in which the increase in peak velocity with walking velocity was higher relative to step size than that in the normal subjects. C: LD partially normalized the relative shape of the trajectory.

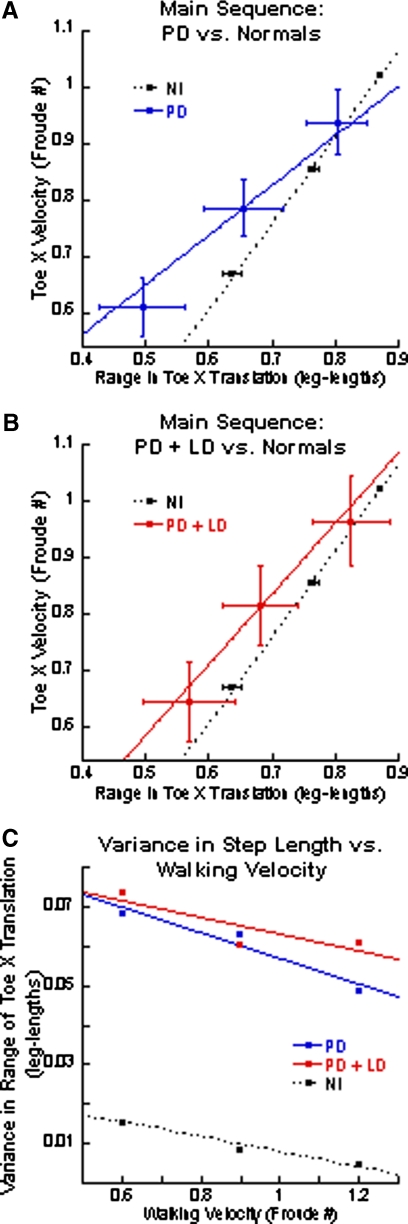
Main sequence relationships in the forward (X) direction

Differences in the phase-plane trajectories were reflected in main sequence relationships comparing peak forward toe velocity and Toe-X translation. The PD subjects had peak Toe-X velocities that were close to, but lower than, those in normal subjects (Fig. 6A, blue line). The Toe-X translations, however, were significantly shorter in PD subjects. When compared with those in normal subjects, the reductions in the X translations were most prominent at low walking velocities, corresponding to the diminished second toe lifts at slower velocities (Fig. 3B). With increasing treadmill velocity, differences between the Toe-X translations of PD subjects and normal subjects decreased (Fig. 6B). The variance in Toe-X translation was higher in PD than that in normal subjects, but decreased with increasing walking velocity (Fig. 6C).

Fig. 6.

Main sequence relationship of peak forward velocity and range of Toe-X translation during the swing phases. A: the blue solid line represents the regression line for PD subjects and the black-dotted line represents the regression for normal subjects. B: the red solid line represents the regression line for PD + LD subjects and the black-dotted line represents the regression line for normal subjects. For both A and B, the horizontal and vertical lines are the SDs for the range in toe translation and velocity, respectively. C: variance of X-translation for the normal (black), PD (blue), and PD + LD (red) subjects.

LD helped to normalize the peak toe velocities. The range of Toe-X translation was partially lengthened, but remained shorter than that in normal subjects, especially at lower walking velocities (Fig. 6B). Variances were unaffected by LD.

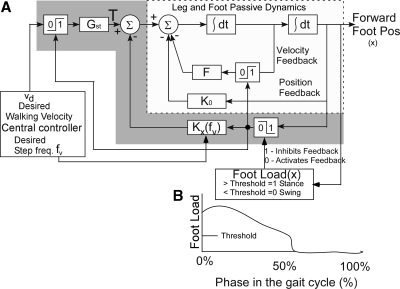
Model-based analysis of the locomotor deficit in PD

Modeling the forward step during locomotion has identified an active feedback control mechanism that governs the swing phase of locomotion (Fig. 7) (Osaki et al. 2007, 2008). Forward motion is controlled by a central command “Desired Velocity” (v_d) that is transmitted through a switch, which is activated when the load on the stance foot exceeds a threshold [Foot Load (x) = 1] (Fig. 7A). The dynamics of the stance phase is determined by the passive damping feedback F, which is also activated by the load on the stance foot exceeding a threshold [Foot Load (x) = 1] (Fig. 7). The parameter K₀ represents the natural elastic feedback when the leg is moved back, but is also present during the forward swing (Fig. 7B). At the end of stance phase when the load on the foot drops below threshold [Foot Load (x) = 0] (Fig. 7B), an active feedback parameter K_x “switches on.” The summation of the passive and active feedback parameters (K = K₀ + K_x) determines the dynamics of the swing phases (Fig. 7A). The active feedback parameter K_x is a function of desired stride frequency (f_d). The stride frequency is related to the square root of the parameter K, divided by the forward inertia of the leg J, given by ω = $\sqrt{\text{K}/\text{J}}$ (Osaki et al. 2007). During normal locomotion, f_d is coupled and related to v_d, giving rise to circular patterns in the phase-plane dynamics (Osaki et al. 2007). When desired walking velocity and frequency are decoupled by auditory or visual pacing stimuli, the phase-plane dynamics is more elliptical (Osaki et al. 2008). The model simulates the stance and swing phases of normal subjects over a wide range of walking velocities and step frequencies (Osaki et al. 2008).

Fig. 7.

Model of forward foot movement as a function of walking and frequency. See text for description.

Relationship of the frequency of oscillation of leg to forward step dynamics

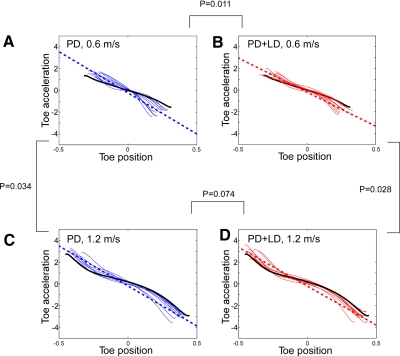
Acceleration and position of the foot during the swing phases were related to model parameters to explain the deficiencies during PD. When forward toe acceleration was plotted versus toe position, the slopes declined more steeply in PD subjects (Fig. 8, A and C, blue curves) than those in normal subjects (Fig. 8, A and C, black curves). The accelerations were higher in PD subjects at the onset of the swing phases and lower at the terminal portions. The largest differences in the slopes and variances occurred at the lowest walking velocity (Fig. 8A, blue curves and blue-dotted line). With increasing velocity, the slopes did not change significantly (compare Fig. 8, A with C, blue curves and blue-dotted lines). With LD, the slopes were partially normalized, especially at lower velocities, and the variances were smaller than those from gait cycles without LD (Fig. 8B, red curves and dotted line). However, the slopes remained steeper than those in normal subjects (not shown). The steeper slope represented a higher value of K, suggesting a higher resonant frequency—i.e., higher step frequency in the PD subjects off LD.

Fig. 8.

Forward toe acceleration as a function of toe position during the swing phases. The blue traces represent data from PD subjects and the red traces represent data from PD + LD subjects. The dotted lines are linear regressions of the PD and PD + LD subjects. The black traces represent the average Toe-X acceleration during the swing phase in normal subjects. A and C: data from PD subjects at 0.6 m/s (A) and 1.2 m/s (C). B and D: data from PD + LD subjects at 0.6 m/s (B) and 1.2 m/s (D).

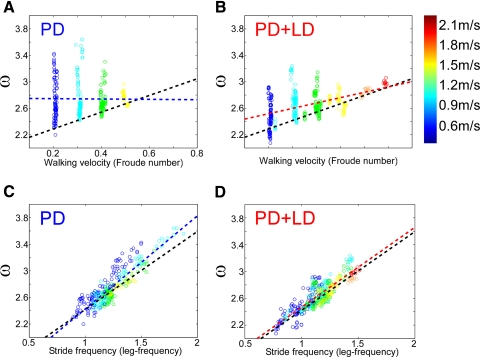
The variable ω, the slope of the forward toe acceleration versus toe position, was plotted as a function of walking velocity to determine whether the feedback parameter K was correlated with velocity. There was no correlation of ω with walking velocity in PD or PD + LD subjects (Fig. 9, A and B). The relationship between ω and stride frequency was not significantly affected in PD subjects (Fig. 9C, R = 0.88) (Osaki et al. 2007, 2008). However, the slope of ω versus stride frequency was higher and the distributions were spread throughout a wider range of ω and stride frequencies with respect to walking velocities in the untreated patients. LD changed the slope of ω from 1.40 to 1.19 rad/leg-frequency (Fig. 9, C and D) and reduced the spread of the data at each walking velocity (Fig. 9D), while maintaining the correlation between ω and stride frequency (Fig. 9D, R = 0.86). Thus the ability to coordinate leg swing from frequency information was preserved in PD subjects, although they lost the ability to coordinate step frequency with walking velocity.

Fig. 9.

Slopes of the Toe-X acceleration (ω) vs. toe position of PD subjects as a function of treadmill velocities of 0.6 m/s (A, B) and 1.2 m/s (C, D), off (A, C, blue) and on (B, D, red) LD. The black-dotted lines are linear regressions of the relationship of normal subjects. The heavy blue and red lines are linear regressions of data from PD and PD + LD subjects, respectively. The open circles are data from individual gait cycles. The different colors represent the different walking velocities, with purple being the lowest and red being the highest walking velocities (see color bar to the right). The data on the accelerations from Fig. 8 and the slopes of the toe acceleration as a function of toe position determine the feedback control parameter for the model shown in Fig. 7 during the swing phase.

DISCUSSION

The major findings of this study are that PD subjects with gait difficulties walk at low velocities and have step frequencies that are inappropriately high (close to 2.2 steps/s). They also have no toe clearance during the terminal portions of the swing phases. These factors shorten the swing phases, leading to early termination of the stance phase in the contralateral leg. This results in less displacement in the −X direction before starting the swing phase that, in turn, affects the following swing phase, inducing festination. In contrast, normal subjects tune the frequency of stepping to their walking velocity. Thus they generate toe clearance and steps that change frequency and length with walking velocity (Osaki et al. 2007, 2008). We hypothesize that there is a centrally generated, step frequency-walking velocity mismatch that accounts for much of the difficulty in PD gait. Independent control of velocity was critical in unmasking the frequency-velocity mismatch. Although “bottom-up” input, absence of visual flow, and lack of forward momentum are factors that may limit the generalizability of treadmill data, overground walking would not allow for precise control of velocity and would limit the duration and distance of the walking trials. LD broadened the bandwidth of step frequency and partially improved stepping dynamics at lower velocities, but did not fully normalize the deficits in PD gait.

Our view that abnormalities in PD gait are explained by a step frequency-walking velocity mismatch is supported by a model of gait that activates the foot with a signal related to “desired walking velocity.” This signal drives the foot backward and the body forward during the stance phase, subsequently generating forward swing phases when the load on the stance foot falls below a threshold (Osaki et al. 2007, 2008). The stance phase dynamics is governed by the passive damping and elasticity of the leg. The swing phase dynamics that drives the pendular-like motion of the leg is governed by active feedback control whose feedback gain is largely dependent on step frequency. The peak velocity of the swing phase is governed by the magnitude of the walking velocity, which alters the initial position of the foot at the start of the swing. When the walking velocity and step frequency are matched during normal gait, there is appropriate toe clearance during the swing and a normal second toe lift and heel strike at the conclusion of the swing. If the step frequency is too high for a given walking velocity, there is no time for the Z-axis motion to clear the surface and to generate the second toe lift that completes the swing phase (Fig. 3) (Osaki et al. 2007, 2008), prematurely shortening the stance phase of the contralateral foot.

The dynamics of the swing phases of PD subjects was similar to the predictions of the model when desired walking velocity and step frequency were decoupled with external pacing. The inability to coordinate walking velocity with step frequency resulted in the abnormal vertical toe movements observed in PD.

Our model-based explanation of the PD gait deficiency is different from the prevalent view, which assumes that increased step frequency compensates for a primary scaling defect in PD locomotion (Hausdorff et al. 2007; Morris et al. 1994, 1996, 1998, 2005). This conclusion was primarily based on the smaller slope of the stride length/step frequency relationship in PD than that in normal subjects (Morris et al. 1996). Other studies that examined the kinematics of walking found a reduced walking velocity in late-stage PD accompanied by reduced stride length, but no significant alterations in cadence (Arias and Cudeiro 2008; Bello et al. 2008; Fernandez-del-Olmo and Cudeiro 2005; Lewis et al. 2000). These studies focused on step length as the most significant factor in affecting gait velocity and stride-to-stride variability as a major fall risk factor, but they did not examine the dynamics of the step.

Our data demonstrated that PD subjects had a preferred step frequency, regardless of walking velocity, and could not lower the step frequencies to match the lower walking velocities. This could be due to the impaired control of the central pattern generator for locomotion. The relatively fixed step frequency was probably responsible for the larger deficit in step length compared with the step length of normal subjects at lower walking velocities. Improvement in step length and step dynamics and the reappearance of the second toe lift simply by increasing walking velocity provide compelling support for our hypothesis that the inability to scale frequency to velocity is a primary driver in PD gait impairment.

The accuracy and variance of step frequency in response to auditory pacing also support our frequency-velocity mismatch hypothesis. Step frequencies of PD subjects were uniformly higher than the pacing stimulus when the stimulus was <2 Hz. Were the higher cadence in PD simply a consequence of shortened stride length, pacing accuracy should have improved at lower walking velocities, but it was just at the slower walking velocities at which the inaccuracies in step frequency and pacing frequencies were largest (compare Fig. 2, A to B) and the swing phase dynamics was most affected. Furthermore, at higher walking velocities, where impairments from scaling deficits should have been more prominent, PD subjects had longer stride lengths and better gait dynamics. In addition, the range of UPDRS scores and physical characteristics could contribute to the variance, but cannot explain the decrease in variance with increased velocity across the population.

The inability to generate low frequencies of stepping at low velocities could be contributing to the difficulties with turns (Willems et al. 2007), since turning requires reduction in the speed of the forward trajectory. The inadequate toe clearance in PD may increase the risk of falls during turns.

Based on the model, desired walking velocity determines both the forward velocity during the stance phase and the peak velocity of the phase-plane trajectory of the swing phases. Peak velocities of the forward trajectories in the phase-plane plots were close to normal (Fig. 5), suggesting that PD subjects were processing the desired walking velocity command for appropriately generating the swing phases, but the pathways that drive the foot during the stance phases were compromised, leading to a premature initiation of the swing phase. This is consistent with the finding that the durations and the distance covered during the stance phases were substantially reduced in the PD subjects and that the stance phases were more affected than the swing phases (Fig. 1, A and C).

Administration of LD partially improved PD gait by restoring the relationship between step frequency and walking velocity at lower velocities, which resulted in a terminal toe lift and a longer stride length. LD partially broadened the bandwidth of step frequency, which limited the improvement in the accuracy and the variance of stepping. Based on the UPDRS score (Table 1), LD reduced rigidity and helped remove a potential confounding effect in measuring the step frequency-velocity mismatch. In some cases, rigidity was not present after frequency-velocity 12 h without medication, yet a mismatch was present. There was no clinical evidence of weakness in the lower limbs in any of the subjects. Therefore the frequency-velocity mismatch cannot be explained simply by rigidity or lack of force generation.

What is equally important, however, is that LD did not fully normalize PD gait. This included the duration of stepping in the low-velocity range at which PD subjects normally walk (Fig. 1), the accuracy and variance of stepping during auditory pacing (Fig. 3), and the dynamics of stepping (Figs. 5, 6, 8, and 9). There was also little effect of LD on stride frequency when PD subjects walked at higher velocities (Fig. 1D). These deficiencies suggest that nondopaminergic pathways that are responsible for the velocity, frequency, and feedback control of the locomotion generator are compromised in PD. This could explain why LD does not provide meaningful benefit for the gait dysfunction and postural instability in advanced disease (Olanow et al. 2009). In normal subjects, higher level control signals deriving from the dopaminergic neurons in the substantia nigra and nondopaminergic, extrastriatal pathways are able to regulate step frequency, thereby enabling appropriate foot dynamics (Brown 1911; Orlovsky et al. 1999). PD subjects lack the higher-level control to regulate step frequency, causing them difficulty at the low walking velocities. Thus although LD partially reduces gait dysfunction, it does not cure it—the supraspinal control of the locomotor generator must be understood better to treat PD gait abnormalities more rationally and effectively.

GRANTS

This work was supported by National Institutes of Health Grants DC-05222 and EY-04148 to T. Raphan and DC-05204 and EY-01867 to B. Cohen; The Bendheim Parkinson Center; and grants from The Bachmann–Strauss Dystonia and Parkinson Foundation, The Parkinson Alliance, and a pilot K-12 Career Development Award from the Mount Sinai School of Medicine to C. Cho.