Skip to main content
PMC home page
NIHPA Author Manuscripts logoLink to NIHPA Author Manuscripts
. Author manuscript; available in PMC: 2010 Jun 24.
Published in final edited form as: Int J Body Compos Res. 2008 Sep 2;6(3):114–121.

Can we use the Jackson and Pollock equations to predict body density/fat of obese individuals in the 21st century?

AM Nevill 1, GS Metsios 1, AS Jackson 2, J Wang 3, J Thornton 3, D Gallagher 3,4
PMCID: PMC2891061  NIHMSID: NIHMS180805  PMID: 20582331

Abstract

Objective

Jackson and Pollock’s (JP) ground-breaking research reporting generalized body density equations to estimate body fat was carried out in the late 1970s. Since then we have experienced an ‘obesity epidemic’. Our aim was to examine whether the original quadratic equations established by Jackson and co-workers are valid in the 21st century.

Methods

Reanalyzing the original JP data, an alternative, more biologically sound exponential power-function model for body density is proposed that declines monotonically, and hence predicts body fat to rise monotonically, with increasing skin-fold thicknesses. The model also remains positive irrespective of the subjects’ sum-of-skinfold thicknesses or age.

Results

Compared to the original quadratic model proposed by JP, our alternative exponential power-function model is theoretically and empirically more accurate when predicting body fat of obese subjects (sums of skinfolds >120mm). A cross-validation study on 14 obese subjects confirmed these observations, when the JP quadratic equations under estimated body fat predicted using dual energy x-ray absorptiometry (DXA) by 2.1% whereas our exponential power-function model was found to underestimate body fat by less than 1.0%. Otherwise, the agreement between the DXA fat (%) and the two models were found to be almost identical, with both coefficients of variation being 10.2%.

Conclusions

Caution should be exercised when predicting body fat using the JP quadratic equations for subjects with sums of skinfolds>120 mm. For these subjects, we recommend estimating body fat using the tables reported in the present manuscript, based on the more biologically sound and empirically valid exponential power-function model.

Keywords: Body density, monotonic decline, percentage body fat, skinfold thickness, Body mass index, body composition

Introduction

The prevalence of human obesity has dramatically increased in the western world. Some authors refer to this trend as an ‘obesity epidemic’ [1–3], intensifying the need to monitor these systematic changes in fatness using reliable and valid measures of adiposity. In population studies, the three most commonly used field measures of body composition to monitor obesity are: (1) the body mass index (BMI=body mass/stature2) where body mass and stature are recorded in kilograms (kg) and meters (m) respectively; (2) estimation of body fat using bioelectrical impedance equipment (BIA), and (3) estimates of percentage body fat based on the sum of skinfold thickness.

Each of the aforementioned methods has both advantages and limitations. BMI can be considered the simplest method (compared to skinfold and BIA) for prediction of body fat, as it only requires the assessment of weight and height. However, the major limitation of BMI is its inability to differentiate between muscle and fat, and hence it is incapable of accurately assessing the adiposity of both athletic and non-athletic populations [4, 5]. In addition, the strength and robustness of its relationship to adverse health outcomes has been frequently criticized [6, 7]. BIA is also widely used because it requires very little time for assessment, is easy to administer, requires neither specialized training nor practice and is non-invasive. Limitations of this method include its greater cost compared to BMI and skinfold methods, its prediction inaccuracy in various populations [8, 9] as well as the fact that various BIA equipment estimate fat and/or fat-free mass based on non-validated equations. Similar to BIA, methods involving skinfolds are less expensive, less time-consuming and do not require formally qualified trained personnel, compared to either underwater weighing or dual-energy X-ray absorptiometry (DXA). For these reasons, skinfolds are widely used by researchers as a method to predict body fat with a reasonable degree of accuracy (prediction error ranging from 4.9 to 8.4% against underwater weighing [10]). The main disadvantages of the skinfold method are a) the inability of calliper jaws to fit the large folds of obese individuals resulting in reduced precision in predicting body fat and b) the increased difficulty to find the appropriate landmarks [11]. However, based on the current citation of all of these methods (ISI Web of Knowledge, April 2008), the most frequently used method for prediction of body fat in the field setting still adopts the sum of skinfolds.

The most commonly cited articles to estimate body composition using the sum of skinfolds are by Jackson and Pollock [12] for men, and by Jackson et al. [13] for women, both having been cited 713 and 593 times to date (ISI Web of Knowledge, April 2008). The Jackson and Pollock (JP) equations [12, 13] are multiple regression equations with functions to estimate body density (BD) from the sum of skinfolds and age. Once BD is known, the Siri [14] two-component model is used to convert BD to percentage body fat. It is important to highlight, that these publications [11, 12] have received more than 45 citations within the last eight months, implying that, despite the limitations of skinfold assessment, it is a method widely used in research. Moreover, this method is also used currently by some researchers in the area of obesity-related research [15–17].

The original work of Jackson and colleagues was carried out in the 1970s prior to the current ‘obesity epidemic’. In a recent cross-validation study, Jackson and associates [18] reported that the correlations between DXA percent body fat and JP percent fat were high, 0.87 and 0.95 for women and men, but the equations underestimated DXA percent fat. The purpose of the present article is to examine whether the original equations established by Jackson and co-workers are likely to be accurate in the 21st century, in particular, when predicting overweight and obese subjects of modern western populations, and if found deficient, to provide a more biologically sound model to estimate body density for overweight and obese men and women.

Methods

The curvilinear quadratic model proposed by Jackson and Pollock [19] [referred to as BD(M-2)] to predict BD is given by:

BD=a−b⋅S+c⋅S2−d⋅age (Eq. 1)

where S = the sum of skinfolds (chest, abdomen and thigh for men: triceps, thigh, and suprailium for women) and ‘age’ is in years. A limitation of this model is that although BD will initially decline with increasing sum of skinfolds, BD will eventually plateau and then begin to rise as sum of skinfolds increase further. The model is therefore only safe to predict BD and hence percentage body fat, within the range of observations used to establish the original models.

An alternative curvilinear power-function model to describe body density that will overcome this limitation and hence decline monotonically with increasing sum of skinfolds is given by:

BD=a−b⋅Sk−d⋅age (Eq. 2)

Although the model will now decline monotonically with increasing sum of skinfolds, in its present form, BD will eventually become negative as both skinfolds and age increase further. Once again, the model would be limited to predict BD only within the range of observations used to establish the models. Of course, the same is true for the body density model proposed by Durnin and Womersley [20], given by BD= c – m·log (S), where c and m are both fitted constants.

A simple biologically-sound solution that will ensure the model (Eq. 2) will remain positive for all sum-of-skinfolds and age is to define the power-function model (Eq. 2) within an exponential term. This will ensure that BD will remain positive for all sum-of-skinfolds and age. The proposed exponential model becomes:

BD=exp(a−b⋅Sk−d⋅age) (Eq. 3)

The original Jackson and Pollock [12] quadratic model (Eq. 1) can be fitted using ordinary least-squares multiple regression, whilst the exponential model (Eq. 3) can be fitted using the non-linear least-squares regression program as implemented in SPSS (see Appendix 1). For comparative purposes, both models will be fitted to the men’s [12] and women’s [13] BD data. The quality of fit will be assessed by examining the residuals plotted against the predicted values as recommended in standard texts (e.g [21]).

Participants

The original JP data [12, 13] came from two general sources: students, faculty and staff at Wake Forest University (Winston-Salem, NC) and patients and research volunteers at the Cooper Institute (Dallas, TX). All subjects gave written informed consent consistent with the ethics procedures of these organizations. The race/ethnic composition of the JP men and women is not known, but nearly all were non-Hispanic white. The men’s and women’s physical characteristics are given in Table 1. These data have been previously published in Jackson and Pollock [12] for men and Jackson et al. [13] for women, or together in Jackson and Pollock [19].

Table 1.

General and physical characteristics (mean±s) of the male and female subjects.

Men (n=402) Women (n=283)
Mean s Mean s
General characteristics:
Age (yr) 32.8 11.0 31.8 11.5
Height (cm) 179.0 6.4 168.6 5.8
Mass (kg) 78.2 11.7 57.5 7.4
Body mass index (kg/m2) 24.4 3.2 20.2 2.2
Laboratory determined:
Body density (gm/ml) 1.058 0.018 1.044 0.018
Percentage body fat (%) 17.9 8.0 24.4 7.2
Skinfolds (mm):
Chest 15.2 8.0 12.6 4.8
Axilla 173.0 8.7 13.0 61.0
Triceps 14.2 6.1 18.2 5.9
Subscapula 16.0 7.0 14.2 6.4
Abdomen 25.1 10.8 24.2 9.6
Suprailium 16.2 8.9 14.0 7.1
Thigh 18.9 7.7 29.5 8.0
Sum of skinfolds (mm):
All seven 122.9 52.0 125.6 42.0
Chest, abdomen and thigh 59.2 24.5
Tr iceps, s uprailium, thigh 81.8 19.0
Open in a new tab

Cross-validation study

We cross validated the above models (Eqs 1 and 3) using 14 obese subjects from the Body Composition Unit, St. Luke’s-Roosevelt hospital, New York City [3 male; mean (±s) age=62.7 (8.5) yrs, height=175.2 (6.3) cm, weight=108.9 (6.3) kg and 11 female; mean (±s) age=62.7 (16.0) yrs, height=162.4 (7.6) cm, weight=84.5 (11.8) kg] whose mean (±s) sum of skinfolds were 132.0 (11.3) mm, range 120–152 mm [22]. The success of the cross validation was assessed by comparing the agreement between the estimates of percentage body-fat using the sum-of-skinfolds based on both the quadratic model (Eq. 1) and the exponential power function model (Eq. 3) against actual body fat using DXA (DPX, Lunar Corp., Madison, WI with software version 3.6Y) as previously described [23]. Agreement was reported as the mean within-subject difference (bias) and the standard deviation of the differences, the 95% limits of agreement, and the coefficient of variation [24–26]. Although DXA is widely used in research for the assessment of body composition, we recognise that when assessing the body fat of heavy and obese subjects, the DXA estimates of body fat are likely to be influenced by ‘trunk thickness’ with the associated error increasing as the subject’s trunk thickness increases [27].

Results

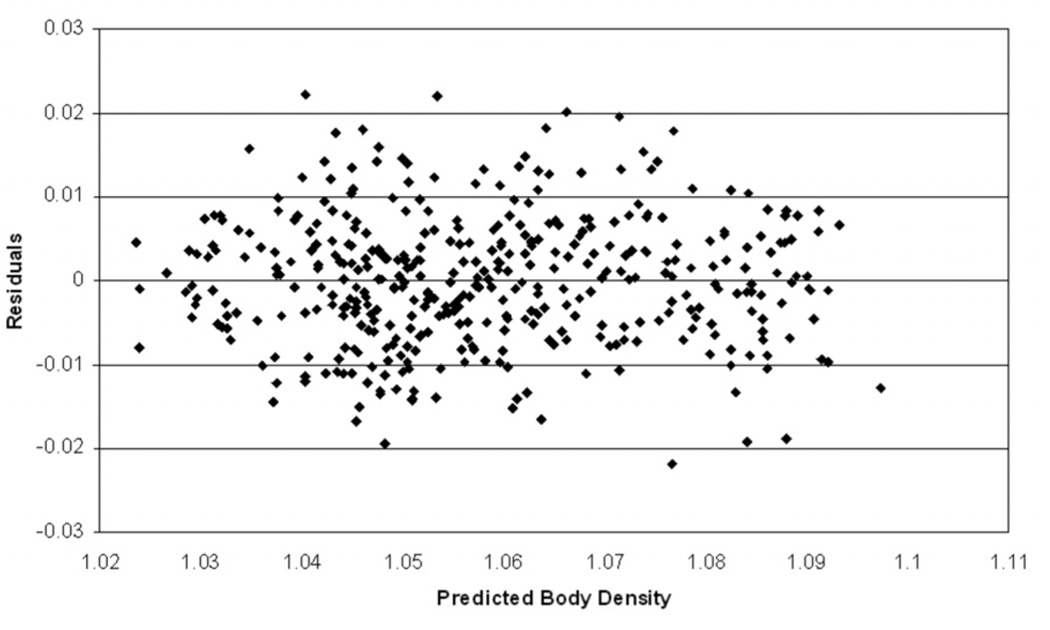
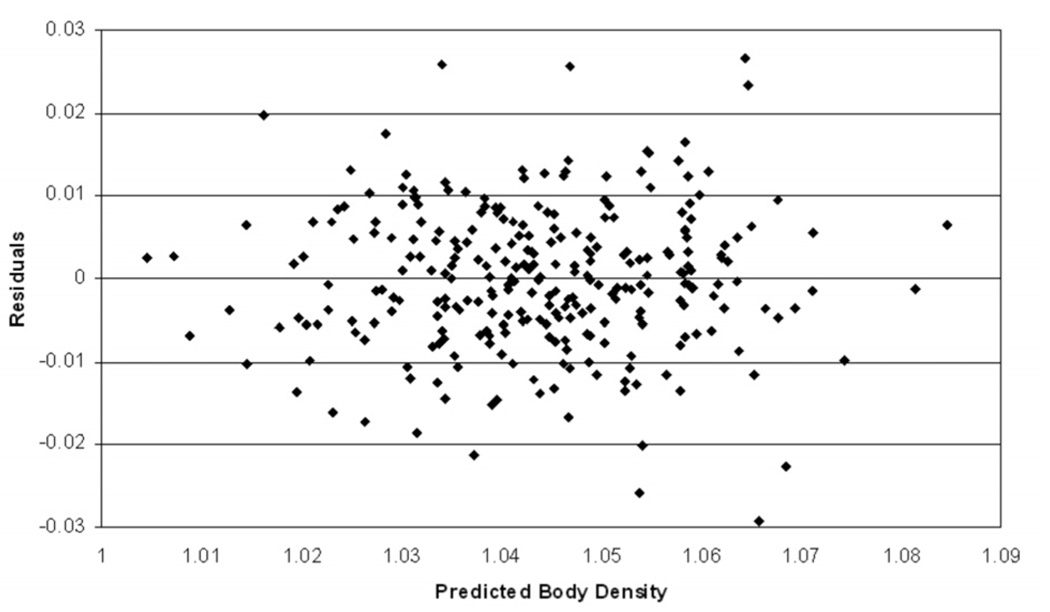
Table 2 shows the results from fitting the quadratic BD prediction equation (Eq. 1) and the exponential power-function model (Eq. 3) to the men’s [12] and women’s [13] data. The quality of fit was assessed with the help of residuals versus predicted BD plots for men and women, given in Figure 1 and Figure 2 respectively.

Table 2.

The quadratic and exponential body-density regression equations for males and females.

Regression equations R SE
Males
BD = 1.10938 − 0.0008267 S +0.0000016 S2 − 0.000257 age 0.91 0.008
BD = exp (0.109648 − 0.0021745 S0.747 − 0.0002516 age) 0.91 0.008
Females
BD = 1.1099421 – 0.0009929 S +0.0000023 S2 – 0.0001392 age 0.84 0.009
BD = exp (0.120936 – 0.0084087 S0.532 – 0.0001178 age) 0.84 0.009
Open in a new tab

Figure 1.

Figure 1

Open in a new tab

Residuals versus predicted body density using the exponential power function model for men.

Figure 2.

Figure 2

Open in a new tab

Residuals versus predicted body density using the exponential power function model for women.

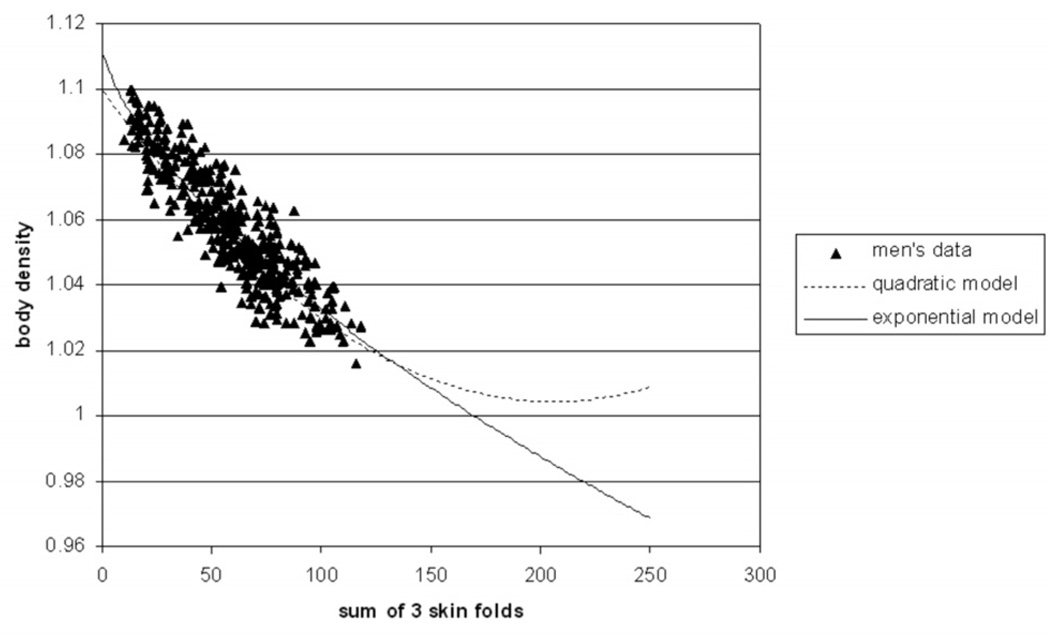
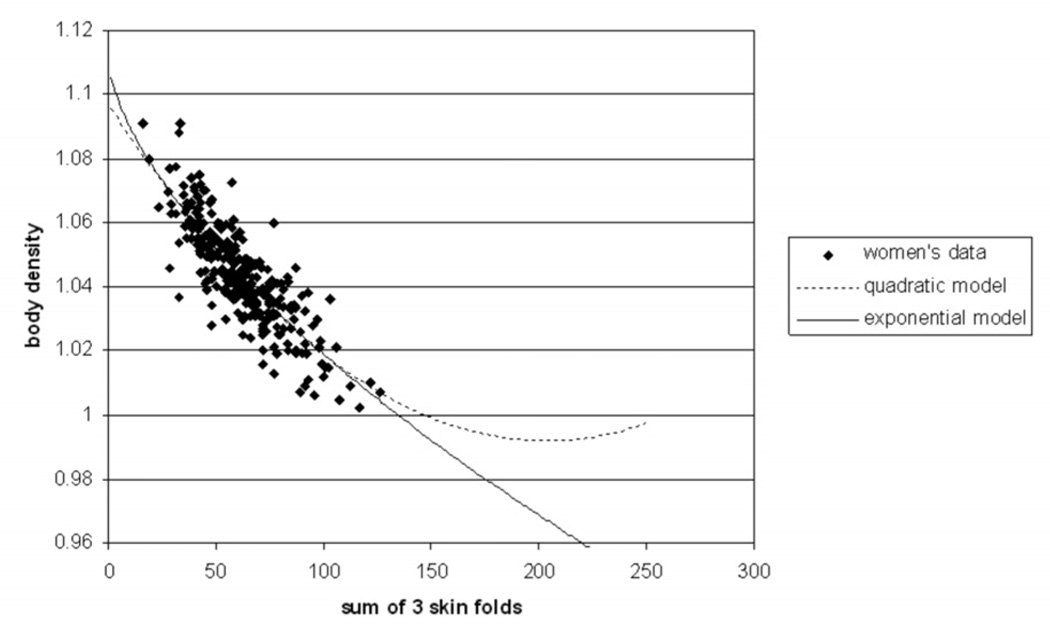
We plotted the BD versus the sum of three skinfolds for men and women in Figure 3 and Figure 4 respectively. Again, for comparative purposes, we have superimposed the quadratic model together with the proposed alternative exponential power-function model in both figures.

Figure 3.

Figure 3

Open in a new tab

The quadratic model and proposed alternative exponential power-function model for men.

Figure 4.

Figure 4

Open in a new tab

The quadratic model and proposed alternative exponential power-function model for women.

Table 3 and Table 4 provide estimates of body fat based on the exponential power-function model (Eq. 3) for men and women respectively.

Table 3.

Estimates of body fat based on the exponential power-function model (Eq. 3) for men.

Age
Sum of Skinfolds(mm) under 22 23–27 28–32 33–37 38–42 43–47 48–52 53–57 0ver 57
8–12 1.3 1.8 2.4 3.0 3.6 4.1 4.7 5.3 5.8
13–17 3.2 3.8 4.4 4.9 5.5 6.1 6.7 7.2 7.8
18–22 5.0 5.6 6.2 6.7 7.3 7.9 8.5 9.0 9.6
23–27 6.7 7.3 7.8 8.4 9.0 9.6 10.2 10.7 11.3
28–32 8.3 8.9 9.5 10.0 10.6 11.2 11.8 12.4 12.9
33–37 9.8 10.4 11.0 11.6 12.2 12.8 13.3 13.9 14.5
38–42 11.3 11.9 12.5 13.1 13.7 14.3 14.8 15.4 16.0
43–47 12.8 13.4 14.0 14.5 15.1 15.7 16.3 16.9 17.5
48–52 14.2 14.8 15.4 16.0 16.6 17.1 17.7 18.3 18.9
53–57 15.6 16.2 16.8 17.4 18.0 18.5 19.1 19.7 20.3
58–62 17.0 17.5 18.1 18.7 19.3 19.9 20.5 21.1 21.7
63–67 18.3 18.9 19.5 20.1 20.7 21.3 21.8 22.4 23.0
68–72 19.6 20.2 20.8 21.4 22.0 22.6 23.2 23.8 24.4
73–77 20.9 21.5 22.1 22.7 23.3 23.9 24.5 25.1 25.7
78–82 22.2 22.8 23.4 24.0 24.6 25.2 25.8 26.4 27.0
83–87 23.4 24.0 24.6 25.2 25.8 26.4 27.0 27.6 28.2
88–92 24.7 25.3 25.9 26.5 27.1 27.7 28.3 28.9 29.5
93–97 25.9 26.5 27.1 27.7 28.3 28.9 29.5 30.1 30.7
98–102 27.1 27.7 28.3 28.9 29.5 30.1 30.7 31.3 31.9
103–107 28.3 28.9 29.5 30.1 30.7 31.3 31.9 32.6 33.2
108–112 29.5 30.1 30.7 31.3 31.9 32.5 33.1 33.8 34.4
113–117 30.7 31.3 31.9 32.5 33.1 33.7 34.3 34.9 35.6
118–122 31.9 32.5 33.1 33.7 34.3 34.9 35.5 36.1 36.7
123–127 33.0 33.6 34.2 34.8 35.5 36.1 36.7 37.3 37.9
128–132 34.2 34.8 35.4 36.0 36.6 37.2 37.8 38.5 39.1
133–137 35.3 35.9 36.5 37.2 37.8 38.4 39.0 39.6 40.2
138–142 36.5 37.1 37.7 38.3 38.9 39.5 40.1 40.8 41.4
143–147 37.6 38.2 38.8 39.4 40.0 40.7 41.3 41.9 42.5
148–152 38.7 39.3 39.9 40.6 41.2 41.8 42.4 43.0 43.6
153–157 39.8 40.4 41.1 41.7 42.3 42.9 43.5 44.1 44.8
158–162 40.9 41.5 42.2 42.8 43.4 44.0 44.6 45.3 45.9
163–167 42.0 42.6 43.3 43.9 44.5 45.1 45.8 46.4 47.0
168–172 43.1 43.7 44.4 45.0 45.6 46.2 46.9 47.5 48.1
173–177 44.2 44.8 45.5 46.1 46.7 47.3 48.0 48.6 49.2
178–182 45.3 45.9 46.5 47.2 47.8 48.4 49.0 49.7 50.3
183–187 46.4 47.0 47.6 48.2 48.9 49.5 50.1 50.8 51.4
188–192 47.4 48.1 48.7 49.3 50.0 50.6 51.2 51.8 52.5
193–197 48.5 49.1 49.8 50.4 51.0 51.7 52.3 52.9 53.6
198–202 49.6 50.2 50.8 51.5 52.1 52.7 53.4 54.0 54.6
Open in a new tab

Table 4.

Estimates of body fat based on the exponential power-function model (Eq. 3) for women.

Age
Sum of Skinfolds(mm) under 22 23–27 28–32 33–37 38–42 43–47 48–52 53–57 0ver 57
8–12 2.4 2.7 2.9 3.2 3.5 3.8 4.0 4.3 4.6
13–17 5.5 5.8 6.1 6.3 6.6 6.9 7.2 7.4 7.7
18–22 8.2 8.5 8.8 9.0 9.3 9.6 9.8 10.1 10.4
23–27 10.6 10.9 11.2 11.4 11.7 12.0 12.3 12.5 12.8
28–32 12.8 13.1 13.4 13.6 13.9 14.2 14.5 14.7 15.0
33–37 14.9 15.1 15.4 15.7 15.9 16.2 16.5 16.8 17.0
38–42 16.8 17.0 17.3 17.6 17.9 18.1 18.4 18.7 19.0
43–47 18.6 18.8 19.1 19.4 19.7 20.0 20.2 20.5 20.8
48–52 20.3 20.6 20.9 21.1 21.4 21.7 22.0 22.2 22.5
53–57 21.9 22.2 22.5 22.8 23.1 23.3 23.6 23.9 24.2
58–62 23.5 23.8 24.1 24.4 24.7 24.9 25.2 25.5 25.8
63–67 25.1 25.3 25.6 25.9 26.2 26.5 26.8 27.0 27.3
68–72 26.6 26.8 27.1 27.4 27.7 28.0 28.2 28.5 28.8
73–77 28.0 28.3 28.6 28.8 29.1 29.4 29.7 30.0 30.2
78–82 29.4 29.7 30.0 30.2 30.5 30.8 31.1 31.4 31.7
83–87 30.7 31.0 31.3 31.6 31.9 32.2 32.5 32.7 33.0
88–92 32.1 32.4 32.6 32.9 33.2 33.5 33.8 34.1 34.4
93–97 33.4 33.7 33.9 34.2 34.5 34.8 35.1 35.4 35.7
98–102 34.6 34.9 35.2 35.5 35.8 36.1 36.4 36.6 36.9
103–107 35.9 36.2 36.5 36.7 37.0 37.3 37.6 37.9 38.2
108–112 37.1 37.4 37.7 38.0 38.3 38.5 38.8 39.1 39.4
113–117 38.3 38.6 38.9 39.2 39.5 39.7 40.0 40.3 40.6
118–122 39.5 39.8 40.1 40.3 40.6 40.9 41.2 41.5 41.8
123–127 40.6 40.9 41.2 41.5 41.8 42.1 42.4 42.7 43.0
128–132 41.8 42.1 42.3 42.6 42.9 43.2 43.5 43.8 44.1
133–137 42.9 43.2 43.5 43.8 44.1 44.3 44.6 44.9 45.2
138–142 44.0 44.3 44.6 44.9 45.2 45.4 45.7 46.0 46.3
143–147 45.1 45.4 45.7 46.0 46.2 46.5 46.8 47.1 47.4
148–152 46.1 46.4 46.7 47.0 47.3 47.6 47.9 48.2 48.5
153–157 47.2 47.5 47.8 48.1 48.4 48.7 49.0 49.3 49.6
158–162 48.2 48.5 48.8 49.1 49.4 49.7 50.0 50.3 50.6
163–167 49.3 49.6 49.9 50.2 50.5 50.7 51.0 51.3 51.6
168–172 50.3 50.6 50.9 51.2 51.5 51.8 52.1 52.4 52.7
173–177 51.3 51.6 51.9 52.2 52.5 52.8 53.1 53.4 53.7
178–182 52.3 52.6 52.9 53.2 53.5 53.8 54.1 54.4 54.7
183–187 53.3 53.6 53.9 54.2 54.5 54.8 55.1 55.4 55.7
188–192 54.3 54.5 54.8 55.1 55.4 55.7 56.0 56.3 56.6
193–197 55.2 55.5 55.8 56.1 56.4 56.7 57.0 57.3 57.6
198–202 56.2 56.5 56.8 57.1 57.4 57.7 58.0 58.3 58.6
Open in a new tab

The results of the cross-validation study are as follows: the mean body fat (%) for the 14 subjects (estimated using DXA) was 44.62 %. The mean estimated body fat (%) using the quadratic and exponential models were found to be 42.55% and 43.65% respectively. The mean within-subject differences (±s) between the DXA estimated fat (%) and the quadratic (Eq. 1) and exponential power-function (Eq. 3) models were 2.07% (4.5%) and 0.97% (4.5%) respectively. The 95% limits of agreement for the quadratic (Eq. 1) and exponential power-function (Eq. 3) models were 2.07% (8.9%) and 0.97% (8.9%) respectively, confirming the greater bias with the quadratic model but otherwise similar ranges of agreement associated with the two models. The coefficient of variation (CV) also confirmed the same precision associated with the two models, both having a CV =10.2%.

Discussion

There can be little doubt, the Jackson and Pollock [12] body fat equations for men, and the Jackson et al. [13] equations for women are both accurate and valid methods of estimating body fat (%) for subjects taken from a representative population of adults observed during the 1970s. However, a representative population of adults in the 21st century will be considerably heavier and fatter [28].

If we examine the consequences of using the Generalized Body Density equations recommended by Jackson and colleagues to predict the body fat of overweight or obese subjects from today’s population, there is a serious danger of under estimation. Figure 3 and Figure 4 illustrate this problem. Clearly, the quadratic models for BD begin to decline at a slower rate, and then begin to rise with additional sum-of-skinfolds. This characteristic of the quadratic models is not biologically sound, and suggests the need to exercise caution when using the generalized BD equations for overweight or obese subjects (with sum of skinfolds >120 mm) in the 21st century.

A more biologically sound model to describe the decline of BD with increasing sum of skinfolds is given by the exponential power-function model (Eq. 3). Not only does the model fit the data equally well as the original quadratic models proposed by Jackson and Pollock (see the multiple correlations, R, in Table 2), the model declines monotonically with increasing skinfold thicknesses. Note that by examining the residual plots in Figure 1 and Figure 2, all evidence of systematic curvature in BD has been successfully removed. Clearly, this is an important principle or characteristic of any model that relates BD with skinfold thicknesses. Also, unlike the power-function model (Eq. 2) and the log-transformed model proposed by Durnin and Womersley [20] to predict body density, the model remains positive for all skinfold thicknesses and age.

As reported by Astrand and Rodahl [29], BD (mass/volume) is theoretically dimensionless (L−3M=L0) where L is a linear of body size and M=mass. Hence, it was no surprise to find that the exponents for the sum of skinfolds in the exponential power function (Eq. 3) were both less than 1, found to be k=0.747 (with standard errors, SE=0.092) and k=0.532 (SE=0.173) for men and women respectively. Based on these standard errors, both parameters are significantly greater that zero but less that unity (a linear dimension of body size). However, as yet, there is no obvious theoretical or dimensional argument to explain these fitted exponents.

The estimates of body fat between the quadratic and the exponential models are extremely similar for sum of skinfolds less than 120 mm. However, as anticipated, when we observe the estimates of body fat for larger sum of skinfolds, for example, greater than 120 mm, the Jackson and Pollock’s [19] equations appears to systematically underestimate the body fat (%), especially for females. To illustrate, consider a 60 year old female subject whose sum of skinfolds was 130 mm. Jackson and Pollock’s equations predict the body fat as 41.8%. The equivalent prediction from Table 4, using the exponential power-function model, estimates body fat to be 44.1%. Indeed, when we examine all sums of skin-folds greater than 120 mm, JP equations underestimate body fat between 1% to 3%, an underestimation that will increase further the greater the skinfold thicknesses and age. Although this underestimation may not have an acute clinical implication (since the management of obesity would be similar in an obese individual with either 41% or 44.1% body fat), the application of the existing JP equation will lead to consistent underestimation of participants/patients body fat (%) with sum of skinfold >120 mm.. Furthermore, despite the limitations of skinfold method to predict body fat in obese subjects, researchers still persist in using the JP equation to predict body fat in different subject areas, eg older healthy, diseases and obese populations [15–17]. Given the wide utilization of these equations (ie large number of previous and recent citations), it seems increasingly likely that researchers will be recruiting a proportion of overweight and obese subjects (as part of a wider sample) and subsequently adopting JP skinfold methods to predict body fat.

The results from the cross-validation study confirmed the above observations. Using a reliable method of estimating body fat (%) (DXA) of a group of 14 obese subjects (sum of skinfold >120 mm), we found that the JP quadratic model (Eq.1) under estimated the body fat (%) by 2.1%. When the proposed exponential power-function model was used to estimate the same subjects’ body fat (%), the model underestimated the body fat by less than 1%. Apart from the tendency for the JP quadratic models to under estimate the body fat, the agreement associated between the DXA estimated fat (%) and the two models would appear to be very similar, with similar within-subject standard deviation of differences (s=4.5%) resulting in the same coefficient of variations given by CV=10.2.

The quadratic models used to build the JP equations provided an accurate fit for that population, certainly equal to the quality of fit obtained using the proposed exponential power function model. However, the body composition characteristics of the JP men and women are not representative of today’s population. A comparison of BMI-defined overweight and obese JP men and women with the 1999–2002 NHANES American men and women [28] identifies substantial body composition changes that have occurred in the United States. The proportion of JP men and women who were overweight or obese (BMI ≥ 25 kg.m2) was 42% and 7% respectively. In contrast, the percentage of today’s American men and women with a BMI ≥ 25 kg.m2 is substantially higher, 67% and 62%. These comparative data also document the increase in overweight and obesity was greater in women than men.

By observing Figure 3 and Figure 4 we can clearly see the danger of predicting BD, and hence body fat, using the JP quadratic equations beyond the range of the original 1970–80s observations. The model is biologically implausible given that it predicts BD to rise, and hence body fat (%) to decrease, as the sum of skinfolds exceeds 200 mm. Recognizing that in 21st century, obesity is a serious problem and that JP equations continue to be widely used for research (as noted above), this systematic underestimation will become an increasingly important issue that needs to be, at least acknowledged, and if possible, corrected. A more biologically sound model is proposed that offers one such alternative. The exponential power-function model for body density declines monotonically and hence predicts body fat (%) to rise monotonically with increasing skinfold thicknesses. The model also remains positive irrespective of either the sum of skinfold thickness or age, unlike the log-transformed model proposed by Durnin and Womersley [20].

In conclusion, despite the limitations of using sums of skinfolds described above, many researchers and health scientists still adopt the sums of skinfolds as their preferred method to estimate body fat (%). For these researchers, we recommend great caution when predicting body fat using the tables reported by Jackson and Pollock for subjects with sums of skinfolds >120 mm. For these subjects, we recommend estimating percentage body fat using the tables reported in the present manuscript, based on the more biologically sound and empirically valid exponential power-function model (Eq. 3).

Appendix 1

SPSS Syntax file to fit the exponential power-function model (Eq. 3) for body density (bd) using sum-of-three skinfolds (sum3) and age as predictors.

* NonLinear Regression.

MODEL PROGRAM a=.1 b=.003 k=.7 c=.003.

COMPUTE PRED_ = exp(a - b *sum3**k - c *age).

NLR bd/OUTFILE=’C:\DOCUME~1\in6581\SPSSFNLR.TMP’/PRED PRED_/CRITERIA SSCONVERGENCE 1E-8 PCON 1E-8.

References

  • 1.Dave RC. The obesity epidemic: too much food for thought? Br J Sports Med. 2004;38:360–3. doi: 10.1136/bjsm.2003.007443. discussion 363. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 2.Jeffreys M, McCarron P, Gunnell D, McEwen J, Smith GD. Body mass index in early and mid-adulthood, and subsequent mortality: a historical cohort study. Int J Obes Relat Metab Disord. 2003;27:1391–1397. doi: 10.1038/sj.ijo.0802414. [DOI] [PubMed] [Google Scholar]
  • 3.Popkin BM. The nutrition transition and obesity in the developing world. J Nutr. 2001;131 doi: 10.1093/jn/131.3.871S. 871S–3S. [DOI] [PubMed] [Google Scholar]
  • 4.Nevill AM, Stewart AD, Olds T, Holder RL. The relationship between adiposity and body size reveals the limitations of BMI. Am J Phys Anthropol. 2006;129:151–156. doi: 10.1002/ajpa.20262. [DOI] [PubMed] [Google Scholar]
  • 5.Stavropoulos-Kalinoglou A, Metsios GS, Koutedakis Y, Nevill AM, Douglas KM, Jamurtas A, et al. Redefining overweight and obesity in rheumatoid arthritis patients. Ann Rheum Dis. 2007;66:1316–1321. doi: 10.1136/ard.2006.060319. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 6.Michels KB, Greenland S, Rosner BA. Does body mass index adequately capture the relation of body composition and body size to health outcomes. Am J Epidemiol. 1998;147:167–172. doi: 10.1093/oxfordjournals.aje.a009430. [DOI] [PubMed] [Google Scholar]
  • 7.Blair SN, Church TS. The fitness, obesity, and health equation: is physical activity the common denominator? JAMA. 2004;292:1232–1234. doi: 10.1001/jama.292.10.1232. [DOI] [PubMed] [Google Scholar]
  • 8.Buchholz AC, Bartok C, Schoeller DA. The validity of bioelectrical impedance models in clinical populations. Nutr Clin Pract. 2004;19:433–446. doi: 10.1177/0115426504019005433. [DOI] [PubMed] [Google Scholar]
  • 9.Pateyjohns IR, Brinkworth GD, Buckley JD, Noakes M, Clifton PM. Comparison of three bioelectrical impedance methods with DXA in overweight and obese men. Obesity (Silver Spring) 2006;14:2064–2070. doi: 10.1038/oby.2006.241. [DOI] [PubMed] [Google Scholar]
  • 10.Scherf J, Franklin BA, Lucas CP, Stevenson D, Rubenfire M. Validity of skinfold thickness measures of formerly obese adults. Am J Clin Nutr. 1986;43:128–135. doi: 10.1093/ajcn/43.1.128. [DOI] [PubMed] [Google Scholar]
  • 11.Watts K, Naylor LH, Davis EA, Jones TW, Beeson B, Bettenay F, et al. Do skinfolds accurately assess changes in body fat in obese children and adolescents. Med Sci Sports Exerc. 2006;38:439–44. doi: 10.1249/01.mss.0000191160.07893.2d. [DOI] [PubMed] [Google Scholar]
  • 12.Jackson AS, Pollock ML. Generalized equations for predicting body density of men. Br J Nutr. 1978;40:497–504. doi: 10.1079/bjn19780152. [DOI] [PubMed] [Google Scholar]
  • 13.Jackson AS, Pollock ML, Ward A. Generalized equations for predicting body density of women. Med Sci Sports Exerc. 1980;12:175–181. [PubMed] [Google Scholar]
  • 14.Siri WE. Body composition from fluid spaces and density: Analysis of methods in techniques for measuring body composition. Washington DC: National Academy of Science, National Research Council; 1961. pp. 223–244. [Google Scholar]
  • 15.Dixon JB, Strauss BJ, Laurie C, O’Brien PE. Smaller hip circumference is associated with dyslipidemia and the metabolic syndrome in obese women. Obes Surg. 2007;17:770–777. doi: 10.1007/s11695-007-9142-y. [DOI] [PubMed] [Google Scholar]
  • 16.Jacob AN, Salinas K, Adams-Huet B, Raskin P. Weight gain in type 2 diabetes mellitus. Diabetes Obes Metab. 2007;9:386–393. doi: 10.1111/j.1463-1326.2006.00622.x. [DOI] [PubMed] [Google Scholar]
  • 17.Pongchaiyakul C, Kosulwat V, Rojroongwasinkul N, Charoenkiatkul S, Thepsuthammarat K, Laopaiboon M, et al. Prediction of percentage body fat in rural thai population using simple anthropometric measurements. Obes Res. 2005;13:729–738. doi: 10.1038/oby.2005.82. [DOI] [PubMed] [Google Scholar]
  • 18.Jackson AS, Mcfarlin BJ, Ellis K, Bray MS. Accuracy of generalized body composition equation with diverse men and women. Med Sci Sports Exerc. 2007;39:S35. [Google Scholar]
  • 19.Jackson AS, Pollock ML. Practical assessment of body composition. Phys Sportsmed. 1985;13:76–90. doi: 10.1080/00913847.1985.11708790. [DOI] [PubMed] [Google Scholar]
  • 20.Durnin JV, Womersley J. Body fat assessed from total body density and its estimation from skinfold thickness: measurements on 481 men and women aged from 16 to 72 years. Br J Nutr. 1974;32:77–97. doi: 10.1079/bjn19740060. [DOI] [PubMed] [Google Scholar]
  • 21.Christensen R. Analysis of variance, design and regression: Applied statistical methods. London: Chapman and Hall; 1996. [Google Scholar]
  • 22.Wang J, Thornton JC, Kolesnik S, Pierson RN., Jr Anthropometry in body composition. An overview. Ann N Y Acad Sci. 2000;904:317–326. doi: 10.1111/j.1749-6632.2000.tb06474.x. [DOI] [PubMed] [Google Scholar]
  • 23.Wu CH, Heshka S, Wang J, Pierson RN, Jr, Heymsfield SB, Laferrere B, et al. Truncal fat in relation to total body fat: influences of age, sex, ethnicity and fatness. Int J Obes (Lond) 2007;31:1384–1391. doi: 10.1038/sj.ijo.0803624. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 24.Bland JM, Altman DG. Statistical methods for assessing agreement between two methods of clinical measurement. Lancet. 1986;1:307–310. [PubMed] [Google Scholar]
  • 25.Atkinson G, Nevill AM. Statistical methods for assessing measurement error (reliability) in variables relevant to sports medicine. Sports Med. 1998;26:217–238. doi: 10.2165/00007256-199826040-00002. [DOI] [PubMed] [Google Scholar]
  • 26.Nevill AM, Atkinson G. Assessing agreement between measurements recorded on a ratio scale in sports medicine and sports science. Br J Sports Med. 1997;31:314–318. doi: 10.1136/bjsm.31.4.314. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 27.Roubenoff R, Kehayias JJ, Dawson-Hughes B, Heymsfield SB. Use of dual-energy x-ray absorptiome-try in body-composition studies: not yet a ‘gold standard’. Am J Clin Nutr. 1993;58:589–91. doi: 10.1093/ajcn/58.5.589. [DOI] [PubMed] [Google Scholar]
  • 28.Flegal KM, Troiano RP. Changes in the distribution of body mass index of adults and children in the US population. Int J Obes Relat Metab Disord. 2000;24:807–818. doi: 10.1038/sj.ijo.0801232. [DOI] [PubMed] [Google Scholar]
  • 29.Astrand P-O, Rodahl K. Textbook of work physiology. 3rd edn. New York: McGraw-Hill; 1986. [Google Scholar]

RESOURCES