Use of thin filament reconstituted muscle fibres to probe the mechanism of force generation

Abstract

The technique of selective removal of the thin filament by gelsolin in bovine cardiac muscle fibres, and reconstitution of the thin filament from isolated proteins is reviewed, and papers that used reconstituted preparations are discussed. By comparing the results obtained in the absence/presence of regulatory proteins tropomyosin (Tm) and troponin (Tn), it is concluded that the role of Tm and Tn in force generation is not only to expose the binding site of actin to myosin, but also to modify actin for better stereospecific and hydrophobic interaction with myosin. This conclusion is further supported by experiments that used a truncated Tm mutant and the temperature study of reconstituted fibres. The conclusion is consistent with the hypothesis that there are three states in the thin filament: blocked state, closed state, and open state. Tm is the major player to produce these effects, with Tn playing the role of Ca²⁺ sensing and signal transmission mechanism. Experiments that changed the number of negative charges at the N-terminal finger of actin demonstrates that this part of actin is essential to promote the strong interaction between actin and myosin molecules, in addition to the well-known weak interaction that positions the myosin head at the active site of actin prior to force generation.

Thin filament reconstituted muscle fibre system

For the purpose of understanding the cross-bridge interaction with actin and the role of regulatory proteins in this interaction, we reconstituted the thin filament in cardiac muscle fibres. This method is schematically represented in Fig. 1. The thin filament is first removed by gelsolin (Fig. 1A to 1B), followed by sequential reconstitution with G-actin (Fig. 1C), and regulatory proteins, tropomyosin (Tm) and troponin (Tn) (Fig. 1D). Gelsolin is an actin and thin filament severing protein, hence its action is specific and it does not disrupt other sarcomeric structure. However, overtreatment with gelsolin would disrupt the Z-line structure, because actin is one of the main constituents in the Z-line. The thin filament reconstitution method was initially developed at Ishiwata’s laboratory and the key properties of the reconstituted fibres, such as the recovery of isometric tension, the tension–pCa relationship, and the function of spontaneous oscillatory contraction, were examined on skeletal fibres (Funatsu et al. 1994), followed by cardiac fibres (Fujita et al. 1996; Fujita and Ishiwata 1998, 1999; for brief review see Ishiwata et al. 1998). In these works, it was demonstrated that bovine cardiac fibres were successful as the reconstituted system, in which actin, Tm and Tn can be replaced with those prepared from other muscles, for example, rabbit skeletal muscle proteins (Fujita et al. 1996; Fujita and Ishiwata 1999). The reconstitution method in cardiac fibres was successfully applied at Kawai’s laboratory to study the role of regulatory proteins (Fujita et al. 2002, 2004; Fujita and Kawai 2002; Lu et al. 2003) and the role of N-terminal negative charges of actin (Lu et al. 2005) in cross-bridge kinetics.

Fig. 1.

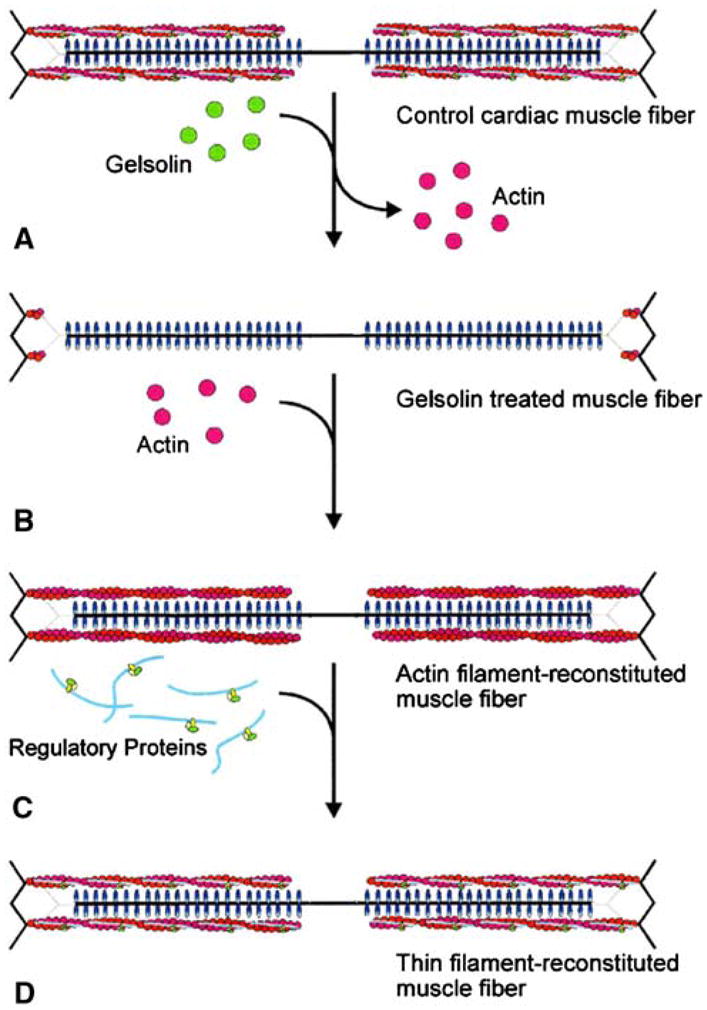
Schematic diagram illustrating the removal and reconstitution protocol of the thin filament. The thin filament in cardiac muscle fibres (A) is removed by gelsolin, yielding thin filament-free fibres (B). (C) The actin filament is reconstituted by adding exogenous G-actin under the polymerizing condition. The thin filament is further reconstituted by adding nTm that contains both Tm and Tn (D). Dotted lines represent connectin (titin) that anchors the thick filament to the Z-line. Redrawn from Biophysical Journal (Fujita et al. 1996; Fujita and Ishiwata 1998)

Because the most of the work on thin filament reconstitution was performed on bovine cardiac fibres, we focus on this preparation in this review. From the bovine heart of 1–2 years of age, one can find ~80% of the time a nice trabecular muscle that is thin (2–4 mm) and slender (10–30 mm) with two ends suspended in the right ventricle but otherwise freely floating, from which long muscle bundles (about 0.5 mm in diameter, 10 mm in length) can be dissected. This is skinned for 7–14 days in the solution that contains (mM) 10 EGTA, 2 MgATP, 5 ATP, 122 KPropionate (Prop), 2 DTT, 30 BDM (2,3-butanedione 2-monoxime), and 10 MOPS (pH 7.0) at 2°C, and stored in the storage solution (50% glycerol is included in the skinning solution) at −20°C. Propionate has been used at Kawai’s laboratory for muscle fibre studies for more than 20 years, however, it may not have been the best choice of anion (see Andrews et al. 1991). A fibre with the diameter of ~90 μm is dissected from a skinned bundle on the day of an experiment, and two ends of the preparation are respectively attached to a length driver and a tension transducer by nail polish. The fibre is then bathed at 2°C in the relaxing solution that contains (mM) 6 EGTA, 2.2 MgATP, 5 ATP, 8 phosphate (Pi), 41 NaProp, 75 KProp, 10 MOPS, and 200 ionic strength (pH 7.0). The pCa of this solution is >9 when contaminating Ca is considered (Reuben et al. 1971). The volume of the muscle chamber is 80 μl. A small volume is advantageous because the quantity of proteins used for reconstitution is usually limited. 90 μm is the optimal diameter of the preparation, which is important for good reproducibility of the results. The fibre is further skinned with 1% Triton-X100 in the relaxing solution for 20 min at 25°C. If a diffraction pattern is visible with He–Ne laser, the sarcomere length is adjusted to 2.0 μm. It is often difficult to obtain a good diffraction pattern in cardiac fibres, in which case the fibre is stretched slightly to remove the slack and to witness the sign of a tension rise. With this method the sarcomere length was shown to be in the range of 1.9–2.1 μm by confocal microscopy after staining with rhodamine-conjugated phalloidin (Fujita et al. 1996, 2002; Fujita and Ishiwata 1998, 1999). At this stage, the fibre length (L₀) is determined, which ranges 2–3 mm. The preparation is activated briefly for initial control tension at 25°C with the activating solution (5S0P) that contains (mM) 6 CaEGTA, 5.8 MgATP, 1.4 ATP, 15 creatine phosphate (CP), 1 NaProp, 92 KProp, 10 NaN₃, 10 MOPS, and 320 unit/ml creatine kinase (CK) (ionic strength 200 mM, pCa 4.66, pH 7.0), and relaxed immediately (Fig. 2A). The activation has to be brief or deterioration in the fibre may occur, which causes undesirable results in the subsequent reconstitution.

Fig. 2.

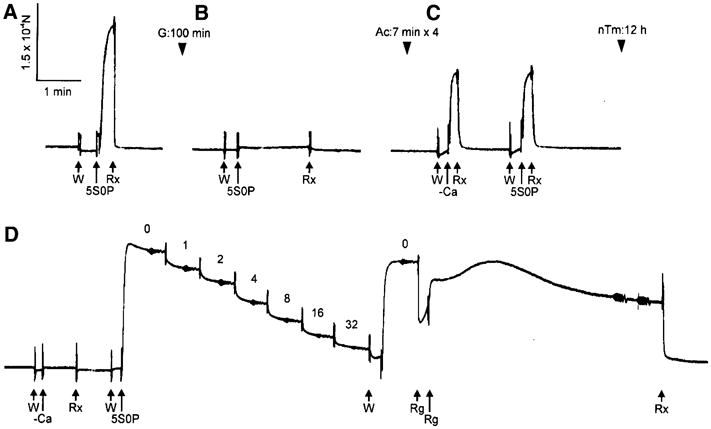
A slow pen trace of isometric tension at each stage of removal and reconstitution of the thin filament. A, Control myocardium; B, after gelsolin treatment (G) for 100 min; C, after actin filament-reconstitution (Ac); and D, after thin filament-reconstitution (nTm). Control myocardium was first activated in the 5S0P solution (pCa 4.66) at 25°C (A) to test for isometric tension. After gelsolin treatment, myocardium was immersed in the 5S0P solution to confirm the removal of thin filament (B). After reconstitution of actin filament (Ac) in C, myocardium was immersed in the solution with Ca²⁺ (5S0P) and without Ca²⁺ (–Ca) at 25°C. The relaxation was attained in the solution containing 40 mM BDM (Rx). After reconstitution of regulatory proteins (nTm) in D, active tension did not develop without Ca²⁺ (–Ca), but it developed with Ca²⁺ (5S0P). In D, the complex modulus data were collected at seven different Pi concentrations (0–32 mM as indicated), and the initial activation at 0 mMPi was repeated to detect any deterioration. All activations including rigor were performed at 25°C, and all records in this figure were taken from the same preparation. Reproduced from Biophysical Journal (Fujita et al. 2002) with permission

To perform reconstitution, the thin filament is first extracted in the solution that contains (mM) 2 CaEGTA, 2.2 ATP, 121 KCl, 4.25 MgCl₂, 2 leupeptin, 2 diisopropyl fluorophosphates (DFP), 40 BDM, 20 MOPS (pCa 4.66, pH 7.0) and ~0.3 mg/ml gelsolin at 2°C (Fig. 1A → B). Gelsolin has an apparent molecular weight of ~86 kD, and it is purified from bovine plasma by the method developed by Kurokawa et al. (1990); its purity is checked by SDS-PAGE. To inhibit the trace amount of contaminating proteases, leupeptin and DFP are used so that other contractile structures are not destroyed. Ca²⁺ is a cofactor of gelsolin action, hence it is essential to include this ion in the extraction solution. Because Ca²⁺ activates the muscle fibre system, 40 mM BDM is added to prevent force generation (Blanchard et al. 1990; Herrmann et al. 1992; Zhao and Kawai 1994b). Generally, 60–120 min of extraction time is needed at 2°C, depending on the strength of gelsolin. The temperature can be raised if the extraction time exceeds 120 min. The degree of extraction is judged by a brief active tension at 25°C (Fig. 2B, 5S0P). If the tension is < 10% of the control tension, the extraction is stopped by washing out the gelsolin with the relaxing solution. It is important to know that overtreatment of fibres with gelsolin is not good for subsequent reconstitution, because a short segment of the thin filament remaining at the Z-line (Fig. 1B) is essential to nucleate the growth of the actin filament (Fujita et al. 1996).

The thin filament extracted fibre is then subjected to actin filament reconstitution (Fig. 1B → C) from G-actin under the polymerizing condition in the solution that contains (mM) 4 EGTA, 4 MgATP, 8 KCl, 80 KI, 40 BDM, 20 Pi (pH 7.0), and 1 mg/ml G-actin. A problem here is that F-actin may nucleate everywhere in the muscle chamber and form quickly. The F-actin formation outside the fibre can be seen as clouding of the solution which turns into a gel shortly. To delay this nucleation and to use actin fragments remaining at the Z-line as the polymerization seeds, 80 mM KI is used instead of KCl (Funatsu et al. 1994; Fujita et al. 1996) for the reconstitution solution, which is freshly made each time, and the duration of reconstitution is limited to 7 min each and performed at 0°C. Because actin elongation is not adequate with the short time interval (7 min), the reconstitution procedure is repeated. Four treatments will usually recover 50–70% of initial tension. If the tension recovery is less than this, the treatment may be repeated for 2–4 more times. The reconstitution is carried out in the presence of 40 mM BDM, because the reconstituted actin filament is spontaneously active owing to the lack of regulatory proteins. Therefore, the “active tension” is induced by deletion of BDM and by elevating the temperature to 25°C (Fig. 2C), and “relaxation” is induced by the solution that adds 40 mM BDM to the relaxing solution and by lowering the temperature to 2°C. As it has been known for some time, active tension increases with temperature in mammalian muscles (Goldman et al. 1987; Ranatunga et al. 1987; Bershitsky and Tsaturyan 1992; Zhao and Kawai 1994a). Figure 2C demonstrates that the active tension is not sensitive to Ca²⁺, as expected.

The actin filament reconstituted fibre is further reconstituted with regulatory proteins in the relaxing solution that contains both Tm and Tn (Fig. 1C → D). These regulatory proteins can be added together as native Tm (nTm) that contains both Tm and Tn in the stoichiometric amount (Fujita et al. 1996, 2002), or added sequentially (Fujita and Ishiwata 1999; Fujita et al. 2004). This reconstitution takes over night (12–15 h) at 2°C, because one Tm molecule binds 7 actin monomers, and neighbouring Tm molecules have to make the head-to-tail interaction. Any misaligned Tm molecules should be realigned by spontaneous unbinding/rebinding reaction that takes some time (Ishiwata 1973; Ishiwata and Kondo 1978). Once regulatory proteins are reconstituted, the fibre becomes fully Ca²⁺ sensitive; at this point the active tension is tested (Fig. 2D). This tension is 1.3–2× larger than tension with the actin filament alone (Fig. 2C), and close to the initial control tension (Fig. 2A). With repeated activations, tension increases somewhat further, presumably because the repeated activation cuts the actin/thin filament that may have grown in undesirable directions.

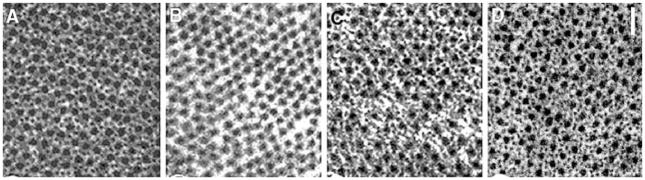
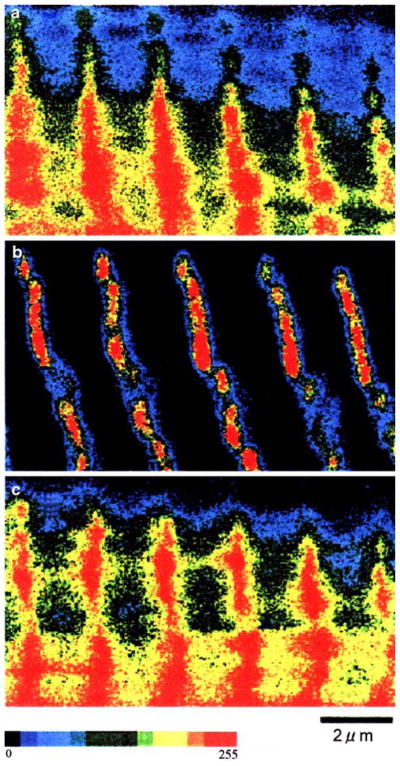
The extracted and/or reconstituted preparations are examined by electron microscopy (Fig. 3), confocal microscopy (Fig. 4), SDS-PAGE (Fig. 5), in addition to isometric tension (Fig. 2) and cross-bridge kinetics by using sinusoidal analysis technique (Figs. 6, 7). These results demonstrate that the extraction and reconstitution are satisfactory: the thin filament is extracted with gelsolin (Figs. 2B, 3B, 4B, 5B), the functional actin filament is formed (Figs. 2C, 3C, 4C, 5C, 6B), and the functional thin filament is formed (Figs. 2D, 3D, 5D, 6C).

Fig. 3.

Electron microscopic images of cross section of myocardium at each stage of extraction and reconstitution. (A) Control myocardium, (B) after gelsolin treatment, (C) after actin filament reconstitution, and (D) after the thin filament reconstitution with Tm and Tn. The scale bar is 100 nm. Reproduced from Journal of Physiology (Lu et al. 2003) with permission

Fig. 4.

Confocal fluorescence micrographs of cardiac fibres at each step of reconstitution showing the concentration of actin. (A) Control cardiac fibre, (B) after gelsolin treatment, (C) after reconstitution of the actin filament. The images have been pseudocolored using a linear scale (shown underneath) of fluorescence intensity of Rh-phalloidin that labelled actin. The highest intensity (red) corresponds to the Z-line. Calibration bar is 2 μm. Reproduced from Biophysical Journal (Fujita et al. 1996) with permission

Fig. 5.

SDS-PAGE of control (lane A), gelsolin-treated (lane B), actin filament-reconstituted (lane C), and thin filament-reconstituted (lane D) myocardium. Lane A corresponds to the myocardium in Fig. 2A, lane B to Fig. 2B, lane C to Fig. 2C, and lane D to Fig. 2D. HC = myosin heavy chain, α-Ac = α-actinin, A = actin, TnT = troponin T, Tm = tropomyosin, TnI = troponin I, LC1 = myosin light chain 1, LC2 = myosin light chain 2. The gelsolin band is visible just below α-actinin band in lane B. 8–16% gradient gelwas used and stained by Coomassie brilliant blue R-250. Reproduced from Biophysical Journal (Fujita et al. 2002) with permission

Fig. 6.

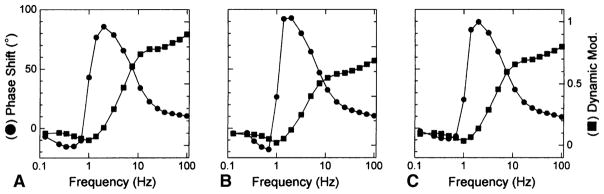
A: Complex modulus Y(f) of the control bovine myocardium activated as in Fig. 2A. B: Complex modulus of actin filament-reconstituted myocardium activated as in Fig. 2C. C: Complex modulus of thin filament-reconstituted myocardium activated as in Fig. 2D. The complex modulus is shown in the dynamic modulus (= |Y(f)|, ■) and phase shift (= arg{Y(f)}, ●) vs. frequency. The unit of the dynamic modulus is MPa. The 5S8P activating solution (mM: 6 CaEGTA, 5.8 MgATP, 1.36 ATP, 15 CP, 8 Pi, 1 NaProp, 73 KProp, 10 NaN₃, 10 MOPS, 200 ionic strength, 320 U/ml CK, pCa 4.66, pH 7.0) was used. Temperature of the experiment was 25°C

Fig. 7.

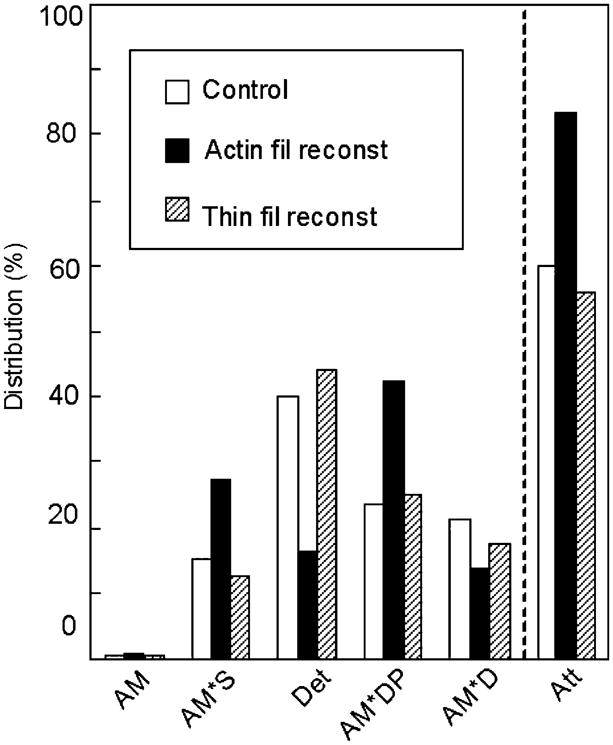
Cross-bridge distribution in native cardiac fibres (white bars), actin filament-reconstituted fibres (black bars), and thin filament-reconstituted fibres (hatched bars) at 5 mM MgATP and 8 mM Pi (25°C). Calculation is based on the kinetic constants reported in Fujita et al. (2002) and Eq. 18 of Kawai and Halvorson (1991). Att = sum of all strongly attached states (AM, AM*S, AM*DP, AM*D). Det = sum of detached states (MS, MDP) and weakly attached states (AMS, AMDP). The distribution of AM is very small (< 1%), because K₁[MgATP] ≫ 1. Because K₀ is not measured, the distribution of the AMD state is not entered here, but it is about the same or less than the distribution of the AM state. This is because [MgADP]≈0.01 mM in the presence of CP/CK, and K₀[MgADP] ≤ 1 in bovine myocardium (Lu et al. 2003). Redrawn from Biophysical Journal (Fujita et al. 2002)

Methods of studying cross-bridge kinetics

Once all components are reconstituted, the preparation is ready for mechanical analysis to test for its functions. Active isometric tension is the first parameter to be studied (Fig. 2D). In addition, there are several methods to study the cross-bridge kinetics in muscle fibres. Classically, force–velocity measurements were carried out, from which maximum force (P₀) and the maximum velocity of shortening (V_max) were studied (Huxley 1957; Edman 1979). More recently, the rate constant of force recovery (k_tr) is measured when the fibre is quickly shortened for a large distance (10–20% L₀) and restretched to the original length (Brenner and Eisenberg 1986; Metzger et al. 1989). The k_tr measurement has been also used to study the kinetics in single myofibrils (for review, see Poggesi et al. 2005). These methods require many cross-bridge cycles, hence the slowest step (rate limiting step) influences the measured parameters most significantly. These methods are comparable to the ATP hydrolysis rate measurement, because its rate is also limited by the slowest step in the cross-bridge cycle (Zhao and Kawai 1994a).

We implemented the sinusoidal analysis method (Kawai and Brandt 1980), which changes the muscle length in sinewaves of varying frequency (0.13–700 Hz); historically, this method was used by Pringle’s group on insect flight muscles (Pringle 1967; White and Thorson 1974). The amplitude of the length change is small (0.125% L₀), which corresponds to 1.25 nm per half sarcomere when the sarcomere length is 2 μm. Because of the presence of series compliance in the thin filament and in other sarcomeric structures (Oosawa et al. 1972; Huxley et al. 1994; Wakabayashi et al. 1994; Higuchi et al. 1995), ~0.6 nm of the length change is applied at the cross-bridge level. This value is very much smaller than the step size (5.3 nm; Kitamura et al. 1999), hence there is a possibility that elementary steps of the cross-bridge cycle can be studied.

Theoretically, the sinusoidal analysis method is complementary to the more commonly used step analysis method (Huxley and Simmons 1971; Heinl et al. 1974; Abbott and Steiger 1977), which changes the length of muscle fibres in small steps. The above mentioned frequency range corresponds to 0.8–4400 s⁻¹ in step analysis, because of the 2π factor that is multiplied to the frequency value for the frequency-to-time domain conversion. In rabbit psoas muscle fibres, the distortion of periodic force response to sinusoidal length change is small, and the total nonlinear power is < 1%, linear power is >99%, hence the regression coefficient is >0.995 when the force time course is fitted to sinusoidal waveform (Kawai and Brandt 1980). In other words, the force response is almost perfectly linear in response to sinusoidal length changes under our experimental conditions. This is in contrast to step analysis, where the rate constant changes significantly with the step size as demonstrated by Huxley and Simmons (1971) on frog semitendinosus fibres, or Abbot and Steiger (1977) on rabbit psoas fibres.

Both sinusoidal and step analysis methods are a subset of perturbation analysis, that modifies an experimental condition, and follow the subsequent time course in tension. Other examples are temperature jump (Goldman et al. 1987; Bershitsky and Taturyan 1992), pressure release (Fortune et al. 1991), and a use of caged compounds which releases a specific ligand on photoflash (Goldman et al. 1984; Dantzig et al. 1992; Araujo and Walker 1996). The released ligand binds to a contractile protein to initiate a transient. All of these methods apply a quick change to an experimental condition, hence the speed of the change must be faster than the rate constants to be observed. The perturbation causes an instability in the cross-bridge cycle, which otherwise is at the steady-state, and results in a redistribution of cross-bridges among various states. The redistribution takes time, hence it can be observed as “tension transients”, and analysed by a series of exponential functions to obtain the rate constants (called “apparent” or “observed” rate constants) in the exponential time course. For the same system (muscle fibres), the deduced rate constants are the same with any of the perturbation analysis methods employed. The purpose of this analysis is to construct a kinetic model of the cross-bridge cycle (Kawai 2003). There are several advantages in the sinusoidal analysis method including high resolution, covers a large frequency range, etc., but above all, it is worthwhile to point out that this method is simple to implement yet produces significant amounts of information. It is also a method that applies a minimal intervention to the fibres and no overall force change occurs, which is an advantage because no time is spent for stretching in-series compliance on a force increase, or no sarcomere dynamics (inhomogeneity or wave propagation) ensue that may occur with a force relaxation as observed in single myofibrils (Poggesi et al. 2005; Stehle et al. 2005). It is noteworthy that the sinusoidal analysis method is by far the least expensive method, hence the dollar amounts spent for each publication is minimal. In the last 28 years, Kawai’s laboratory’s peer reviewed publication cost on the average $60,000 per paper (direct cost), much of which (80%) was spent on personnel expenses.

To correlate the apparent rate constants with intrinsic rate constants of elementary steps of the cross-bridge cycle, it is necessary to study the MgATP effect, the MgADP effect and the phosphate (Pi) effect on the apparent rate constants (Kawai and Halvorson 1991). An example of the Pi study is shown in Fig. 2D, in which the numbers above the pen trace indicate the mM concentration of Pi added. From these studies, we succeeded in deducing a cross-bridge model with six states (Scheme 1 below) together with the rate constants (k₂, k₋₂, k₄, k₋₄) and the association constants (K₀, K₁, K₅) that characterize the elementary steps. These constants are as a whole called the “kinetic constants”.

Scheme 1.

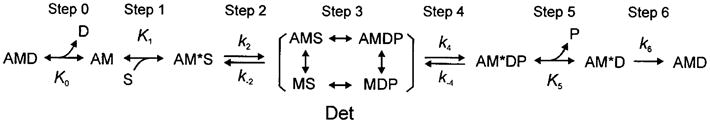
In this scheme, S = MgATP, D = MgADP, P = phosphate, A = actin, and M = myosin. With the sinusoidal analysis method, we characterized the cross-bridge scheme in rabbit psoas fibres (Kawai and Halvorson 1991), soleus slow twitch fibres (Wang and Kawai 1997), ferret cardiac fibres (Kawai et al. 1993), porcine cardiac fibres (Zhao and Kawai 1996), bovine cardiac fibres (Fujita et al. 2002), and other fast twitch skeletal muscle fibres from the rabbit (Galler et al. 2005). The above Scheme 1 is consistent to those deduced from solution studies (Taylor 1979; Geeves et al. 1984) of isolated and reconstituted contractile proteins, except that in solution studies the strongly bound AM*DP state has not been identified, the Pi release step (step 5) is practically irreversible (Taylor 1979), and the ATP isomerization step (step 2) is irreversible (k₋₂ = 0) in the interaction of myosin subfragment 1 and MgATP (Bagshaw and Trentham 1974). The irreversibility (extremely large K) means that the free energy reduction is large because of the relationship: ΔG° = −RT ln K, which means that perhaps half of the ATP hydrolysis energy is dissipated at step 2 and another half at step 5 in the case of solution studies. In the muscle fibre system, step 5 is known to be reversible, presumably because the hydrolysis energy is retained in the myosin head as the potential energy (force development). For step 2, the data fit better to the reversible model in muscle fibres, and the cause of its difference with solution data may reside in the vast difference in the experimental materials and conditions. Steps 1–2 are consistent with those deduced by using caged ATP (Goldman et al. 1984), and steps 4–5 are consistent with those deduced by using caged Pi (Dantzig et al. 1992; Araujo and Walker 1996) or pressure-release (Fortune et al. 1991) on rabbit psoas fibres.

Experiments with reconstituted fibres

Figure 3A is an electron micrograph of the control fibre showing the cross section in which both thick and thin filaments can be seen in hexagonal lattice. Figure 3B is after gelsolin treatment, where the thin filament is lost and often left with empty space behind. Figure 3C is after actin filament reconstitution, and Fig. 3D is after Tm and Tn reconstitution. It is seen that the actin filament (Fig. 3C) and the thin filament (Fig. 3D) are formed and they occupy the proper strategic position for interaction with the thick filament. With confocal fluorescence microscopy (Fig. 4), the longitudinal periodic pattern of muscle fibres before (Fig. 4A) and after (Fig. 4B) the gelsolin treatment, and after the reconstitution of the actin filament (Fig. 4C) can be seen (Fujita et al. 1996). Figure 5A is an SDS-PAGE of the control fibres. In Fig. 5B, almost all the thin filament proteins (actin, Tm, TnT and TnI) are removed after gelsolin treatment, but thick filament proteins (MHC, MLC1 and MLC2) and a Z-line protein (α-actinin) are left behind. A small amount of actin observed in Fig. 5B can be attributed to actin fragments remaining at the Z-line together with α-actinin. In this gel, TnC is not visualized. In Fig. 5C, actin is seen to have come back after the actin filament reconstitution. In Fig. 5D, Tm, TnT and TnI are seen to have come back after reconstitution of regulatory proteins. In Fig. 5B, the thin band below α-actinin is gelsolin which remained in fibres.

In Fig. 2A, the control active tension can be seen. In Fig. 2B, there is hardly any active tension after gelsolin treatment for 100 min. When the actin filament is reconstituted (Fig. 2C), tension develops irrespective of the presence of Ca²⁺. This tension is only about 70% of the control tension (Fig. 2A). In Fig. 2D, it is seen that the Ca²⁺ sensitivity and full tension recover after overnight treatment with nTm. With our experiments (Fujita et al. 2002), tension recovery was 107 ± 4% (±SEM, N = 26) compared to initial control (Fig. 2A), and the degree of the recovery is controlled by the number of times of actin filament reconstitution (7 min interval). In some fibres, tension recovered up to 140% (Fujita et al. 1996; Ishiwata et al. 1998) presumably because, in cardiac fibres, the in situ length of the thin filament may not make a full overlap with the thick filament, and the extra length of reconstituted thin filament can generate additional force by interacting with myosin cross-bridges. At this stage, sinusoidal analysis is performed, which can be seen as small oscillations in the tension trace in Fig. 2D. Figure 6 compares amplitude and phase shift of control (in A), actin filament reconstituted (in B), and thin filament reconstituted (in C) preparations. The frequency profile is similar in all preparations, which indicates that there is no large change in cross-bridge kinetics. From each profile, two apparent rate constants (2πb and 2πc) can be extracted (Kawai and Brandt 1980; Wannenburg et al. 2000; Fujita et al. 2002). Our experiments demonstrate that reproducibility of 2πb is 98 ± 6%, and 2πc is 92 ± 4% after the thin filament reconstitution. These observations indicate that the thin filament reconstitution in cardiac fibres is at its near perfection in terms of the structure, proteins incorporated, tension generating ability, and the cross-bridge dynamics.

Effect of regulatory proteins on force per cross-bridge, and positive allosteric effect

As mentioned, tension in the absence of regulatory proteins (Fig. 2C) is only about 70% of control (Fig. 2A). Tension increases 1.5× when Tm and Tn are reconstituted (Fig. 2D) (Fujita et al. 2002). There are two possibilities to account for this increase: (1) an increase in the number of force generating cross-bridges, and (2) an increase in force/cross-bridge. For this reason, we performed sinusoidal analysis during maximal activation, changed MgATP, MgADP and Pi concentrations, and determined the kinetic constants of elementary steps based on the six state model (Scheme 1) for (a) control fibres, (b) actin filament reconstituted fibres, and (c) thin filament reconstituted fibres (Fujita et al. 2002). The kinetic constants of (a) and (c) agreed within an experimental error, which demonstrates a good functional reconstitution. When comparing (a) and (b), the rate constants of steps 2 and 4 differed by 2×, and their equilibrium constants differed as much as by 4×. The Pi association constant K₅ differed by 3×. Thus, there were substantial changes in the kinetic constants of the elementary steps with the addition of regulatory proteins. From these kinetic constants, we calculated the cross-bridge distribution at various intermediate states (Fig. 7). The results show that there is actually a decrease in the number of strongly bound cross-bridges (bars labelled Att in Fig. 7) by 27% when the regulatory proteins are added. In Scheme 1, strongly bound cross-bridges are identified as AM*DP, AM*D, AMD, AM and AM*S (Kawai and Zhao 1993). From these results, we concluded that the force/cross-bridge is increased by addition of the regulatory proteins by the factor of ~2 (Fujita et al. 2002). It is hypothesized that the reason for this increase is that the regulatory proteins in the presence of Ca²⁺ apply a positive allosteric effect on actin so that the actin–myosin interaction becomes stronger. In other words, Tm and Tn modify the actin–myosin interface for better stereospecific interaction so that the efficiency of the energy transduction mechanism in myosin is improved. Such a mechanism was proposed based on solution studies (Tobacman and Butters 2000). This mechanism is another view of the well known cooperative activation mechanism of the thin filament: Ca²⁺ binding to TnC shifts the equilibrium from the “blocked state” to the “closed state”, and the cross-bridge binding shifts the equilibrium further to the “open state” (McKillop and Geeves 1993). In solution, it has been known that the cross-bridge binding to actin makes the Tm binding to actin stronger (Chalovich 1992). Thus, it is not surprising that Tm binding to the actin filament increased actin binding to myosin. The effect of regulatory proteins and the underlining mechanisms are generally consistent with studies that used single molecules (Gordon et al. 1998; VanBuren et al. 1999; Bing et al. 2000; Homsher et al. 2000; Kawai et al. 2006).

Negative allosteric effect

If the role of regulatory proteins is to apply an allosteric effect on the actin–myosin interaction, it may be possible to reverse the effect by using a Tm mutant. We used Δ23Tm (Hitchcock-DeGregori and An 1996; Landis et al. 1997) that lacks region 2 and 3 of 7 quasi repeat units of the Tm molecule (Hitchcock-DeGregori and Varnell 1990), and reconstituted together with normal Tn. Our result shows that the reconstituted fibre is Ca²⁺ sensitive, but active tension is only 40% of the control tension which is less than the tension generated by the actin filament without regulatory proteins (Lu et al. 2003). However, the number of strongly attached cross-bridges increased by 15%, indicating that tension/cross-bridge is decreased by the mutant Tm. This is an example of negative allosteric effect and supports the hypothesis that Δ23Tm diminishes the actin–myosin interaction or efficiency of transduction. Thus, we conclude that there is an allosteric signal sent from Tm to actin, which can be positive or negative depending on the character of the Tm molecule.

Tropomyosin or troponin?

The next question is whether this allosteric effect is caused by Tm or by both Tm and Tn. The overall effect is large because a change of up to 4× in the equilibrium constants was observed (Fujita et al. 2002). This question can be answered by reconstituting Tm and Tn sequentially. We carried out this experiment and compared the kinetic constants at each step of reconstitution. Our results show that active tension increased gradually, but all the kinetic constants, except for K₁, resumed the control value as soon as Tm was reconstituted without Tn (Fujita et al. 2004). K₁ resumed the control value when both Tm and Tn were reconstituted. These experiments demonstrate that Tm is the main player for the positive allosteric effect of the regulatory proteins on the actin–myosin interaction and subsequent energy transduction. This conclusion is further supported by the experiments showing that Tm but not Tn is responsible for the pH dependence of isometric tension (Fujita and Ishiwata 1999). From previous experiments we performed, we have known that K₁ is influenced by tension (Zhao et al. 1996), therefore by strain. That is, K₁ may change as a result of change in isometric tension. It was reported that the ATP binding step (K₁) may be influenced by loop 1 of myosin, because the charge distribution on the loop 1 and K₁ may be correlated (Wang and Kawai 2001); loop 1 comes close to the ATP binding site. It is possible that the position of loop 1 changes according to the strain on the myosin head, and this change in turn alters the ATP binding to the myosin head.

Temperature effect and hydrophobic interaction

There are two major categories of interaction between molecules. One is ionic and the other is hydrophobic. The initial interaction between actin and myosin is weak and thought to consist of the ionic interaction (Brenner et al. 1982). This interaction works over a long distance, helping the myosin head search for its binding site on actin. The interaction takes place between the N-terminal finger of actin, where four negative charges are found, and the loop 2 of myosin, where 5 positive charges are found. The subsequent interaction is strong and thought to consist of the hydrophobic interaction (Tonomura et al. 1962; Highsmith 1977; Rayment et al. 1993; Zhao and Kawai 1994a). This interaction is only possible when two molecules are close together and stereospecifically matched. The responsible site for actin is Leu¹⁴⁰, Tyr¹⁴³, Ile³⁴¹, Ile³⁴⁵, Leu³⁴⁹ and Phe³⁵² of a short helix-turn (140–149) and a long helix (337–351); the responsible site for myosin is (in chicken sequence) Pro⁵²⁹, Met⁵³⁰, Glu⁵³⁸, Met⁵⁴¹, Phe⁵⁴² and Pro⁵⁴³ of a helix-turn-helix (526–559) in the lower 50K domain (Holmes et al. 2004).

The hydrophobic interaction is endothermic (ΔH° > 0: absorbs heat), because a thin layer of structured water around the hydrophobic residues must be destroyed, a situation analogous to the melting of ice. Liberated water molecules go into the solution phase to gain thermal motion resulting in an increase in the entropy (ΔS° > 0). Because interacting proteins lose the thin layer of structured water, their combined heat capacity decreases (ΔC_P < 0). ΔH° > 0 and ΔS° > 0 have been observed on actomyosin interaction in solution (Tonomura et al. 1962; Highsmith 1977) and in myofibrils (Ishiwata et al. 1986). ΔH° > 0, ΔS° > 0 and ΔC_P < 0 have been observed in rabbit psoas fibres (Zhao and Kawai 1994a; Murphy et al. 1996) and soleus slow twitch fibres (Wang and Kawai 2001) on the step that generates force. This mechanism is consistent with the well known observation that isometric tension increases with an increase in the temperature both in mammalian skeletal (Goldman et al. 1987; Ranatunga et al. 1987; Zhao and Kawai 1994a; Coupland et al. 2001; Wang and Kawai 2001) and cardiac (Ranatunga 1999; Fujita and Kawai 2002) muscle fibres, and reviewed by Kawai (2003).

When the above two mechanisms are combined, then it follows that the tension increase with temperature is larger in the presence of regulatory proteins than in their absence. We performed this exact experiment, and obtained expected results. We found that the slope of the temperature–tension plot is much reduced in the absence of the regulatory proteins (Fujita and Kawai 2002). This experiment further supports the hypothesis that the regulatory proteins promote hydrophobic interaction between actin and myosin beyond what is known currently (see above). That is, the crystal structure of the actin and myosin interaction may be incomplete unless regulatory proteins and Ca²⁺ are added.

Negative charges of actin’s N-terminus

The N-terminal finger of actin is negatively charged across the phylogenic tree (Vandekerckhove and Weber 1978), and in muscles, this finger is known to make the weak (ionic) interaction with loop 2 of myosin (Sutoh 1982a, b; DasGupta and Reisler 1989; Furch et al. 1998); the loop 2 joins 50K and 20K domains and has 5 Lys residues. There is a line of evidence that the N-terminal finger activates S1 ATP hydrolysis rate in solution (Sutoh et al. 1991; Cook et al. 1993), which may suggest that the N-terminal finger also activates the strong (hydrophobic) interaction. For this reason, we used yeast (Saccharomyces cerevisiae) actin mutants (Cook et al. 1993) that vary in the number of N-terminal negative charges and asked a question whether the number of negative charges affects the strong interaction between actin and myosin molecules.

Yeast mutant actin molecules that vary N-terminal negative charges from 2 to 4 were raised and purified in Dr. Peter Rubenstein’s laboratory at the University of Iowa, and used for actin filament reconstitution (Lu et al. 2005). We observed that active tension was 10% and minimal in 2Ac (2 N-terminal negative charges), 23% in 3Ac (3 N-terminal negative charges), and 44% in 4Ac (4 N-terminal negative charges) actins. The same pattern was observed in rigor stiffness, indicating that the actin–myosin interaction becomes stronger with a larger number of N-terminal negative charges. During activation, we characterized the elementary steps, and concluded that there is no large reapportionment of cross-bridges among the six states (Scheme 1), indicating that an increase in the N-terminal negative charge enhances tension generated by each cross-bridge (Lu et al. 2005). This result is consistent with the hypothesis that N-terminal negative charges affect strong interaction between actin and myosin molecules, presumably by changing the actomyosin interface (Cook et al. 1993; Joel et al. 2001). When rabbit skeletal actin (4 N-terminal negative charges) was used, tension increased further to 77%, indicating that there are still significant functional differences between yeast and rabbit actins, although they have 87% sequence identity. These experiments were carried out in the absence of regulatory proteins.

Capping of the pointed end

The reconstituted thin filament does not have tropomodulin, which is the pointed end cap of the thin filament (Fowler et al. 1993; Littlefield and Fowler 1998). The lack of tropomodulin may suggest that actin polymerization and depolymerization would occur, hence the actin/thin filament may be unstable (Ishiwata and Funatsu 1985). In fact, we demonstrated previously that the length of the thin filament in the I-Z-I brush (the structure composed of the Z-line and thin filaments on both sides) spontaneously decreased even in physiological ionic strength at the rate of about 0.05 μm/h (Funatsu et al. 1988). The I-Z-I brush was obtained from skeletal myofibrils (1–2 μm in diameter) in which the thick filament was removed by high salt solution, which also removes the P-end capping protein, now known as tropomodulin (Ishiwata and Funatsu 1985).

In actuality, however, the absence of the capping protein does not appear to affect the stability of actin/thin filament under our experimental conditions, judging from the fact that the length of thin filaments does not change during the reconstitution procedure with the regulatory proteins for 12–15 h (Fujita and Ishiwata 1999; Fujita et al. 2004). Its stability is further confirmed by experimental results which show that the isometric tension of reconstituted fibres does not decrease with repeated activations (Fujita et al. 2002). Thus, spontaneous actin/thin filament depolymerization at the P-end seems not to occur in our actin/thin filament reconstituted fibres, probably because of the presence of the thick filament which interacts weakly with the actin/thin filament during relaxation. It is also possible that G-actin at the critical concentration of polymerization may exist in the core of the filament lattice in muscle fibres, but not in myofibrils, because of their thickness difference. In fact, we have been finding that the reconstituted fibres are more stable than native fibres, and they can survive a larger number of repeated Ca²⁺ activations than native fibres. This unexpected finding could be explained if extra actin/thin filament is formed to stabilize the sarcomere structure but that it may not contribute to active force generation.

Reconstitution of the actin/thin filament in skeletal muscles

The thin filament extraction and reconstitution protocol works well for the cardiac preparations. The same method was tried with rabbit psoas fibres, but the tension reproduced was limited to 30% (Funatsu et al. 1994). Even this degree of recovery is remarkable, because such reconstituted fibres may offer numerous possibilities in testing hypotheses. It is possible that the presence of nebulin in skeletal muscle (Wang and Wright 1988) may hinder the formation of the actin filament. Nebulin wraps the thin filament in skeletal muscles, and it may be difficult to experimentally reproduce the correct actin–nebulin and actin–myosin interaction at the same time. Instead of nebulin, nebulette is present in cardiac preparations (Moncman and Wang 1995), but since its molecular weight is small (107 kD) and it is localized close to the Z-line, the interaction between nebulette and actin molecules may become normal with the actin/thin filament reconstitution. Connectin (titin) (Maruyama et al. 1976; Wang et al. 1979) does not appear to interfere with the actin/thin filament reconstitution, primarily because it wraps only around the thick filament in the A-band area, and it runs in parallel with the actin/thin filament in the I-band area, except at a region near the Z-line (Funatsu et al. 1990, 1993). Connectin is depicted as the thin line in Fig. 1. With an EM examination, cardiac muscles have a robust-appearing Z-line that is thicker than the Z-line of fast twitch skeletal muscles. It is probable that the thicker Z-line may be the reason for the higher stability against the gelsolin treatment (Fujita et al. 1996). In rabbit psoas fibres the structure of the Z-line appears to be disorganized by partial removal of the thin fragment, one of main constituents of the Z-line structure (Funatsu et al. 1994).

Future direction

The advantage of the thin filament reconstitution method is that any thin filament protein can be replaced with a mutant protein, force and force transients can be studied, and the kinetic constants that characterize the six state cross-bridge model can be deduced, hence the structure–function relationship can be established. We are currently studying the functional difference of α-Tm, β-Tm, and their phosphorylated form (Lu et al. 2006). In the future, any mutant protein of the thin filament origin that causes familial hypertrophic cardiomyopathy (Blanchard et al. 1999; Wolska and Wieczorek 2003) or dilative cardiomyopathy (Chang and Potter 2005) can be studied to elucidate the structure–function relationship of these diseases.