A Modular Geometric Mechanism for Reorientation in Children

Abstract

Although disoriented young children reorient themselves in relation to the shape of the surrounding surface layout, cognitive accounts of this ability vary. The present paper tests three theories of reorientation: a snapshot theory based on visual image-matching computations, an adaptive combination theory proposing that diverse environmental cues to orientation are weighted according to their experienced reliability, and a modular theory centering on encapsulated computations of the shape of the extended surface layout. Seven experiments test these theories by manipulating four properties of objects placed within a cylindrical space: their size, motion, dimensionality, and distance from the space's borders. Their findings support the modular theory and suggest that disoriented search behavior centers on two processes: a reorientation process based on the geometry of the 3D surface layout, and a beacon-guidance process based on the local features of objects and surface markings.

Introduction

When humans or animals become disoriented, how do they regain their sense of direction? Research in developmental and comparative psychology, behavioral ecology, cognitive neuroscience, and neurobiology reveals an impressive sensitivity to surface layout geometry in guiding reorientation. In behavioral studies, both humans and a variety of nonhuman animals, including monkeys, rats, chicks, pigeons, fish, and even ants (Wystrach & Beugnon, 2009), use the overall shape of their environment to reorient themselves (for review, see Cheng & Newcombe, 2005). For example, when children as young as 18 months old observe the hiding of a toy in one corner of an empty rectangular testing arena and then are disoriented, they concentrate their search at the arena's two geometrically correct corners, avoiding the remaining corners with incorrect relations between the lengths and lateral positions of the walls that bound them (Hermer & Spelke, 1994, 1996; Learmonth, Nadel, & Newcombe, 2002; Learmonth, Newcombe, & Huttenlocher, 2001). By distinguishing between a longer or shorter wall on their left or right, children reveal their sensitivity to the fundamental Euclidean properties of distance and direction. But do extended surfaces and their geometric properties play a privileged role in children's reorientation?

Children and other animals also use objects and nongeometric features such as wall coloring to guide their search under disorientation (e.g., Cheng, 1986; Hermer & Spelke, 1996), but their use of these features is variable across species, environments, and tasks (Cheng & Newcombe, 2005; Cheng, 2008). When children are disoriented in a rectangular room with one uniquely colored wall, for example, they base their search on both the shape of the room and the position of the colored wall when the room is large (2.4 m by 3.7 m), the target is hidden directly at one of its corners (Learmonth et al., 2001; Learmonth et al., 2002), and they can explore the space freely (Learmonth, Newcombe, Sheridan, & Jones, 2008), but they base their search only on the shape of the room when it is small (1.2 m by 1.8 m), when the target is spatially displaced from its walls, and when they are confined to a small area that restricts their exploration of the whole room (Hermer & Spelke, 1994, 1996; Learmonth et al., 2002; Learmonth et al., 2008). Other animals show similar effects of the size of the room and the proximity of a target to a distinctively colored wall (e.g., Maes, Fontanari & Regolin, 2009). Such findings have animated an extended, ongoing debate over the specificity and organization of the mechanisms underlying spatial reorientation.

At the present time, three theories are most prominent. One theory proposes that reorientation depends on an encapsulated geometric module that functions automatically and largely independently of other processes (Cheng, 1986). On this view, disoriented animals reorient themselves only in accord with the shape of their surroundings, but they can also use objects and surface markings as beacons to guide their navigation. A second theory proposes that reorientation depends on the adaptive combination of all perceptible sources of environmental information, with each source weighted in accord with its salience and learned validity (Newcombe & Ratliff, 2007). On this view, the surface layout guides reorientation more consistently than do movable objects or surface markings because it is large, salient and has been experienced as a highly reliable cue to orientation. A third theory proposes that reorientation depends on an image-matching process operating on panoramic 2D retinal projections of the current and remembered environment (Cheng, 2008). Layout geometry and landmarks are not even represented by the reorientation system, on this view, but the surface layout has strong effects on disoriented animals' behavior because it projects distinctive and salient edges in panoramic images of the environment (Stürzl, Cheung, Cheng, & Zeil, 2008).

Here we attempt to test these three theories through studies of young children. We report a series of seven experiments that investigated the search performance of 3-year-old children in a simple and controlled environment: a circular arena with two asymmetrically placed, featurally indistinguishable objects. Across experiments, we manipulated the size, movability, and dimensionality of these potential cues to orientation so as to test the three competing theories. Before turning to these experiments, we first consider each of the theories in relation to the existing evidence to elucidate both their continued viability and their contrasting predictions for the present experiments.

Three theories of reorientation

The geometric module was proposed by Cheng and Gallistel following their observations of the behavior of foraging, disoriented rats. In their studies, untrained, disoriented rats relied primarily on the shape of their surroundings (a rectangular room) to relocate hidden food, while failing to use other available cues such as odors, 2D contrast patterns, and wall color (Cheng & Gallistel, 1984). After training, the rats learned to use the non-geometric features of the room to find the food (Cheng, 1986). Nevertheless, a persisting dissociation between the use of geometry and features was revealed in the trained rats' search behavior: the rats initially headed toward one of the two geometrically appropriate corners--either the correct corner or the diagonally opposite one--and then they continued on their way if the corner had the correct non-geometric features and reversed course otherwise. Based on these findings, Cheng (1986) argued that disoriented search behavior depends on at least two independent processes, operating on distinct representations of the environment. First, a reorientation process uses the global shape of the surrounding surface layout to indicate the animal's position and heading. This use of environmental terrain excludes information about objects and surface markings and involves a geometric computation of congruence between the shape of the current perceived layout and that of the remembered layout prior to disorientation. Second, a beacon guidance process uses representations of nearby, visible objects or surface markings as direct cues to locations of hidden objects, when the animal has been trained or otherwise induced to attend to them. This use of objects and their features does not involve the computation of one's position and heading, relative to other locations in the environment. Thus, only the modular, geometric process serves to reestablish the animal's orientation.

Cheng's observations have been replicated in a variety of other species, including ants who, after training, show a dramatic dissociation between processing of layout geometry, which guides their navigation toward a geometrically correct corner of a rectangular space, and processing of patterns at the room's corners, which then guide their choice of which corner to enter (Wystrach & Beugnon, 2009). Cheng and Gallistel's theory was extended to humans after young children were found to show similar, dissociable use of layout geometry and of non-geometric information (wall color) in reorientation tasks (Hermer & Spelke, 1996; Lee, Shusterman & Spelke, 2006; Wang, Hermer, & Spelke, 1999).

Thus, one of the key concepts characterizing the modular process of reorientation is that it involves a computation that is specifically sensitive to 3D surface layouts. In accord with Fodor's (1983) original description of cognitive modules, this computation is posited to be automatic, specific, and encapsulated from other cognitive processes. The distinctive prediction of a modular theory concerns the specificity and automaticity of the reorientation process. While reorientation by 3D surface layouts should exhibit high sensitivity to the geometric information of surface distance and sense relations, it should be impervious to other functional cues. In particular, a modular geometry-based reorientation process should spontaneously respond to layout geometry even when the navigating child or animal confronts an environment in which layout geometry is shown to be unreliable. Independent of this modular reorientation process, disoriented children and animals may use distinctive objects, colored walls, or other non-geometric cues to guide their behavior, but only as beacons that signal the locations of specific targets. These beacons may allow disoriented navigators to locate hidden objects, but they do not reestablish the navigator's sense of orientation.

An influential alternative to the modular view of reorientation by geometry is the adaptive combination theory of Newcombe (Newcombe & Ratliff, 2007).¹ The adaptive combination theory was motivated by the evidence that disoriented children's search is guided by non-geometric information such as the color of a wall when they are tested in a large room with an object hidden directly in one of its corners (Learmonth et al., 2001). According to this theory, all perceptible properties of the environment can be used for reorientation, and these properties are weighted according to their experienced validity: “Development of spatial reorientation capabilities may be conceptualized as the acquisition of the ability to encode relevant aspects of the environment with precision, and the learning of an optimal mix of weights to attach to these kinds of environmental information based on their past usefulness.” (Newcombe & Ratliff, 2007, p. 70). Because learning is influenced both by stimulus salience and by innate biases on encoding, the adaptive combination view allows for a role of innate predispositions in modulating this learning process. Crucially, however, disoriented navigators' use of landmarks and featural information depends on a single computation for spatial reorientation, performed on all types of available cues (Learmonth et al., 2001; Cheng & Newcombe, 2005).

The adaptive combination view provides a natural explanation for children's use of wall color in large rooms, because geometric representations of the environment have no special status within the reorientation process, relative to representations of other environmental properties. It also can explain why wall color is less useful in small rooms, because these environments are less familiar and exploring them is therefore less natural. Finally, it explains why the geometry of the large-scale layout appears to play a special role in behavioral studies on animals and children, because “research so far has mainly examined the relative use of geometry and features in situations in which geometry is instantiated in a very powerful fashion and features are less powerfully instantiated.” (Newcombe & Ratliff, 2007, p. 69). In particular, the features that children often ignore are small, movable objects that have previously been experienced as unreliable cues to reorientation. In contrast, the geometry that children reorient by typically come from walls or large objects that are distal, stable, and salient; children and animals learn that such potential landmarks are reliable cues for navigation and assign high weights for their use in the task of reorienting (Newcombe & Ratliff, 2007). If the geometry of the surrounding layout were made subtle or unreliable, on this view, its influence on children's reorientation should diminish or disappear.

A third alternative to both of the above theories has recently joined the fray. According to the image-matching theory (Cheng, 2008; Stürzl et al., 2008; Wystrach & Beugnon, 2009), reorientation depends on a coarse-grained visual-matching system based on snapshot representations (i.e., images) similar to those that guide some aspects of oriented search by insects (Cartwright & Collett, 1982). This theory was motivated by the findings of recent studies of trained animals, whose search for food sources and other significant locations has been shown to be guided strongly, under some conditions, by the contrasting brightness of the walls of the chamber (e.g., Pearce, Graham, Good, Jones & McGregor, 2006). When animals are disoriented, Cheng (2008) proposes, they move so as to reduce the discrepancy between a stored representation of the two-dimensional image evoked by a scene, on one hand, and the current retinal projection of that scene, on the other; retinal salience therefore determines what environmental features affect the animal's navigation. Computer simulations have demonstrated that search in a geometrically structured environment can result from such models of navigation, and that the frequently observed failures to reorient by wall color can result from the visual similarities of the edges of different colored walls against a simulated sky or background (Cheng, 2008; Stürzl et al., 2008). Image matching theory presents a stark contrast both to the other theories, because it makes no distinction between 2D features and 3D surface layouts. In particular, the shape of the surface layout plays no role in reorientation apart from the salience of its projected image features in the retinal array. Insofar as this theory applies to human navigation, it predicts, with the adaptive combination theory, that the influence of layout shape on children's reorientation will diminish as it becomes more subtle. Contrary to both the modular geometric and the adaptive combination theories, this theory further predicts that 3D and 2D landmarks will have equivalent effects on reorientation if they project equivalent edges in retinal images of the scene.

Prior evidence bearing on the theories

The theory that surface geometry plays a privileged role in guiding navigation gains plausibility from both ecological and computational analyses of navigation. From an ecological standpoint, the extended 3D surface layouts that form the hills, valleys, and ridges in an animal's terrain are the most stable, reliable, distinctive cues in the natural environment. Surface features such as colors and 2D markings tend to change over time, whereas the global shape of the terrain tends to be invariant (Gallistel, 1990). Moreover, objects and surface markings have many look-alikes, whereas natural surface layouts rarely are symmetrical and so can specify one's position uniquely. Therefore, reorientation mechanisms attuned to the surface layout geometry may be more adaptive for the purpose of computing relative positions and heading.

Research in robotics complements and extends the ecological analysis. Two of the greatest difficulties faced by navigating robots are (a) the error caused by misrecognition of a location when the robot encounters similar or displaced objects in different parts of the environment (Thrun, 2002), and (b) the computational explosion caused by the accumulation of representations of complex, cluttered environments (Silveira, Malis, & Rives, 2008). These difficulties interact, because objects in nature, such as trees and rocks, and surface markings, such as leaf striations, can only be distinguished from one another by means of fine-grained, computationally expensive comparisons (Thrun, 2002). In contrast, extended surfaces tend to be smooth, especially when the objects that stand in front of them are eliminated from the layout representation. Thus, the shape of a surface layout that excludes objects and their features can be described more economically than that of a layout that includes them (Gee, Chekhlov, Calway, & Mayol-Cuevas, 2008). Because the extended surface layout tends to have a shape that is unique, enduring, and relatively economical to describe, a reorientation system that focuses on representing the shape of the continuous surface layout minimizes both of the central problems faced by navigating robots.

Nevertheless, these considerations do not serve to decide among the present three theories. Although a modular system for analyzing layout geometry would capture the most reliable geometric information economically, a system that learned to navigate by adaptively combining cues in accord with their reliability also should converge on the geometry of the layout, because it is experienced as most reliable. Insofar as layout geometry produces the most salient features in panoramic 2D images of navigable arrays, moreover, image-matching processes might also be devised so as to yield adaptive navigation systems in natural environments (although see Gee et al., 2008).

Several lines of behavioral evidence support the thesis that human and animal navigation depends on distinct processes for analyzing the geometry of the surrounding environmental surface layout, on one hand, and for analyzing the properties of objects within it, on the other. Following the work of Cheng (1986), many experiments on diverse animals, including recent studies with mice and ants, have shown that training influences navigating animals' use of landmarks but has little effect on animals' use of surface layout geometry, which is spontaneously encoded (Wystrach & Beugnon, 2009; for a review of the older literature, see Cheng & Newcombe, 2005). Training effects have also been observed in children (Twyman, Friedman, & Spetch, 2007). With practice using a distinctively colored wall in a triangular room, children's subsequent use of a colored wall in a small rectangular room was improved (Twyman et al., 2007). Yet, no such flexibility has been found in children's use of geometry. Consistent with the evidence from training experiments, controlled-rearing experiments with both chicks and fish, performed by two different groups of researchers, have shown that reorientation by room shape develops independently of an animal's history of experience navigating in geometrically structured spaces, supporting the view that reorientation is innately attuned to layout geometry (Brown, Spetch, & Hurd, 2007; Chiandetti & Vallortigara, 2008): animals raised in circular environments reoriented just as adeptly by the shape of a rectangular arena as animals who were raised in the very same rectangular environments. In contrast, the use of landmark features is reliably influenced by prior navigation experience (Brown et al., 2007): when geometry and features were placed in conflict, animals who were raised in a circular environment were more likely to use featural cues over geometry, while those who were raised in a rectangular environment were less likely to use the featural cues over geometry. Thus, learning appears to influence navigation by non-geometric features, whereas surface layout geometry is used even in the absence of learning.

Consistent with this evidence, behavioral studies of spatial learning by human adults provide evidence for distinct processes of encoding the geometry of the bordering surfaces of an array, on one hand, and for encoding the positions and identities of objects on the other (Doeller & Burgess, 2008). Oriented adults, navigating in a virtual environment containing both an extended bordering surface and a freestanding object, were required to encode the positions of specified targets so as to relocate the targets on a subsequent test. Patterns of performance indicated that the adults spontaneously encoded the target position relative to the border, and that their encoding was resistant to interference from other associative processes in memory. Adults also encoded the target position relative to the landmark object, but the latter encoding showed interference effects that are signatures of attention-dependent associative learning. Within a single array and task, therefore, landmark-related learning obeyed the laws of associative reinforcement, whereas boundary-related learning was automatic and incidental. If these conclusions apply to children and animals, they could explain why reorienting by layout geometry is so prevalent across tasks and environments: before animals or children are disoriented, they may encode layout geometry spontaneously. Moreover, these results could explain the emergence of featural cue use by both animals and children after training (e.g., Cheng, 1986; Twyman et al., 2007): with experience, children and animals come to associate featural cues of objects in the environment directly with target locations.

Finally, evidence for separable systems for navigating in relation to layout geometry and object features comes from studies probing the neural mechanisms of navigation in animals and in humans. Single-cell recording studies of rats' hippocampal place cells, which fire when an animal moves to a particular location in the environment (O'Keefe & Nadel, 1978), have shown that extended surfaces, such as the walls of the testing space, are crucial to the representation of location (O'Keefe & Burgess, 1996). Importantly, while changes in surface boundaries affect place cell activations, changes in texture and material do not (Lever, Wills, Cacucci, Burgess, & O'Keefe, 2002). In addition, the activation of both hippocampal place cells and parahippocampal head-direction cells, which fire when a rat is oriented a particular way with respect to the environment, are controlled by objects placed at the periphery of the testing space, where they contribute to the shape of its borders, but not by objects placed in the center of the space (Cressant, Muller, & Poucet, 1997; Zugaro, Berthoz, & Wiener, 2001).

Representation of geometric borders has recently been found in the entorhinal cortex of rats; these border cells are hypothesized to define the perimeter of the environment and serve as reference frames for encoding locations within that environment (Solstad, Boccara, Kropff, Moser, & Moser, 2008). Like cells in the nearby hippocampus, their activity is modulated by the positions of layout boundaries but not of freestanding objects. In humans, moreover, functional neuroimaging studies have shown activation of the right posterior hippocampus for processing locations with respect to environmental boundaries and activation of the right dorsal striatum for landmark-related locations (Doeller, King, & Burgess, 2008).

All these findings are consistent with Cheng and Gallistel's original theory of a modular geometric process for reorientation, and pose problems for the other theories. In particular, the snapshot (i.e., image-matching) theory fails to explain why both behavioral patterns of navigation and place cell firing in rats is unaffected by plunging the animals into darkness so that no visual matching process could guide them (e.g., Quirk, Muller, & Kubie, 1990). Moreover, the adaptive combination view predicts flexibility in cue use, but studies of the activity of hippocampal neurons in actively navigating rats show persistent reliance on the shape of the surrounding environment, despite large changes in other featural properties such as the color, texture, and composition of its surfaces (Lever et al., 2002).

Nevertheless, other findings favor the alternative views. In particular, both place cells and head-direction cells respond to cue cards of distinct brightness on one side of a circular arena: when the card is rotated to a new position in the arena, these place cells and head-direction cells undergo a corresponding rotation (Knierim, Kudrimoti, & McNaughton; Muller & Kubie, 1987; Taube, Muller, & Ranck, 1990). These findings have been widely interpreted as providing evidence for reorientation in accord with the relative lightness of the walls of the chamber. It is possible, however, that the thickness of the card introduces a subtle geometrical discontinuity into the chamber, or that it introduces a brightness gradient throughout the chamber that serves as a compass signal.

The adaptive combination and image matching theories also are supported by evidence for dramatic changes in the behavior of trained, navigating animals, tested within an environment of a single invariant shape, when the walls of the environment change in color from white to black or the reverse: although the shape remains the same, animals may cease to use this information when the brightness relations reverse (e.g., Pearce et al., 2006). Nevertheless, it is not clear whether the trained animals in these studies reorient by the lightness information or simply learn to disregard their internal sense of orientation in mastering this spatial task. In the latter case, the frequent alternations in the walls of the environment may signal to the animals that the environment is continuously changing, rendering their orientation system irrelevant for mastering the task.

Studies using a range of spatial tasks, species, and empirical approaches therefore provide mixed evidence concerning the separability of the processes by which disoriented animals analyze surface layout geometry, on one hand, and object and their features, on the other. Moreover, none of the above studies directly probes the reorientation process itself so as to test whether it depends on an encapsulated analysis of the shape of the surface layout, on adaptive combination of all environmental features, or on image-matching processes. The present research was conducted for this purpose, focusing on the navigation performance of young children.

Prior tests of the views through studies of reorientation in children

Studies over the past 20 years have resulted in a wealth of evidence concerning the types of environmental cues that support reorientation by young children. These studies reveal that large, extended 3D surfaces guide children's reorientation whether or not they form a connected enclosure (Gouteux & Spelke, 2001) or surround the child (Huttenlocher & Vasilyeva, 2003). Furthermore, children reorient according to surface layout in spaces that are asymmetric (Wang et al., 1999), triangular (Lourenco & Huttenlocher, 2006), or (by 4 years of age) rhombic (Hupbach & Nadel, 2005), providing evidence that the use of surface layout geometry to reorient is not specific to familiar, connected rectangular spaces. Other evidence suggests, however, that young children are more sensitive to the distances and directions of surfaces than to their angular relations (Lee & Spelke, in review). These findings suggest a convergence between behavioral studies of children and neurophysiological studies of animals, whose hippocampal place cells respond primarily to surface distance and direction (O'Keefe & Burgess, 1996).

As discussed above, disoriented children use landmarks in large rooms with one large distinctively colored wall (Learmonth et al., 2001; Learmonth et al., 2002; Hupbach & Nadel, 2005; Learmonth et al., 2008). In some studies testing disoriented children's capacity to use featural information in the absence of informative room shape (in a circular enclosure), children use the featural information as a direct marker of an object's location. For example, if the environment contains several identical containers and one container of a distinctive color, disoriented children will use the distinctively colored container to find the object if it is hidden directly at that container, but they do not use the container to reorient themselves and therefore they fail to correctly retrieve the object from other featurally identical locations (Gouteux & Spelke, 2001; Lee, et al., 2006). Children also fail to reorient by 2D forms in geometrically distinctive arrays. For example, disoriented children search randomly in geometric arrays of identical objects in the middle of a circular enclosure, even when they are connected by 2D lines on the floor to form a triangle or rectangle (Gouteux & Spelke, 2001; Lee & Spelke, 2008). Distinctive objects and 2D surface markings serve as beacons in these studies, but they fail to guide children's reorientation.

In contrast, children's reorientation is affected by objects that are placed at the periphery of the room. For example, children reorient by a rectangular array of large 3D objects when the objects are placed adjacent to the walls of a circular enclosure (Garrad-Cole, Lew, Bremner, & Whitaker, 2001). Similarly, while children fail to use a freestanding object on one side of a room to distinguish between geometrically identical corners (Hermer & Spelke, 1996), they succeed when there is a 3D bulge on one of the walls of the room (Wang et al., 1999). These findings are reminiscent of the findings from neurophysiological studies of rats and from neuroimaging studies of adult humans that navigation depends on an automatic encoding of information at the borders of the navigable space (e.g., Cressant et al., 1997; Doeller et al., 2008). Nevertheless, it is not clear whether these successes with objects at the walls of the enclosures indicate reorientation by the objects themselves or reorientation only by surface borders, which now incorporate the 3D peripheral objects into the representation of the overall environmental shape. In the present research, we attempt to distinguish these two possibilities.

Intriguing recent evidence concerning the mechanisms of reorientation comes from studies in which children are disoriented in a square room whose opposite walls have contrasting brightness or patterns. If the opposite walls of such a room differ in color but not brightness (i.e., the alternating walls of the room are blue and red), children search the four corner hiding places equally: they fail to confine their search to the two corners with the appropriate directional relations to the colored wall (Huttenlocher & Lourenco, 2007). In contrast, if the opposite walls differ in brightness (i.e., the alternating walls are blue and white), children successfully confine their search to the two corners with the appropriate brightness and directional properties (e.g., corner with a brighter wall on the left) (Nardini, Atkinson, & Burgess, 2008). Children also use some, but not all, kinds of pattern information to reorient in square rooms. If the opposite walls of a square room are covered by different 2D patterns (circles vs. crosses), or if one wall is patterned and the other is not (black circles on a white background vs. homogeneous grey), children search the four corners equally, failing to combine pattern and directional information. In contrast, if the opposite walls present the same pattern at different sizes and densities (small and dense circles vs. large and sparse circles), children confine their search to the two corners with appropriate pattern and directional properties (e.g., corners with the smaller circles on the left). It is unclear, however, whether differences in surface brightness and pattern size influence children's reorientation because they are processed directly by the reorientation system, contrary to the theory of a geometric module, or because they engage mechanisms of depth perception in ways that result in distortions to the perceived 3D shape of the room, consistent with the theory that only the shape of the layout influences reorientation (see Spelke, Lee & Izard, in press, for discussion).

Further evidence concerning the mechanisms of reorientation in children comes from experiments testing children's reorientation in a large room with a single distinctively colored wall. As we noted, children's search is influenced by both the room shape and the colored wall's position in this environment, when a toy is hidden at a corner of the room directly adjacent to two walls (Learmonth et al., 2001; Learmonth et al, 2002). In contrast, if children are instructed to stay within a smaller rectangular area with no distinctive coloring that is embedded within the larger rectangular room, and if the toy is hidden in a corner of this arena, away from the walls of the large room, children under 6 years of age continue to use room shape, but fail to use the distant wall color, to locate the hidden object (Learmonth et al., 2008). When the toy is hidden directly in a corner of the larger rectangular room, however, children succeed at 4 years of age; 3-year-olds also succeed in this case following four unrestricted trials, as in Learmonth et al. (2001). Interestingly, children of all ages used the geometric shape of the room equally well across all conditions of these experiments.

These findings accord with the predictions of Cheng's (1986) original account of disoriented search behavior: children may reorient by the shape of the room but then use the distinctive coloring of the walls in the large room as a beacon that guides them to the hidden object, provided that the object is close to the walls. By this account, the size or relevance of a non-geometric feature wall can modulate children's reliance on it as a beacon (Learmonth et al., 2001, 2002); likewise, relevant training or practice, as in Learmonth, et al. (2008) and Twyman et al. (2007), can also affect the degree in which children use them associatively. The adaptive combination theory could explain these findings, however, by proposing that children rarely are faced with situations in which they are restricted to the middle of the room and must use a potential landmark, especially when it is not directly related to the target location, and so they fail to process the colored wall under these unusual conditions. While such an interpretation would have difficulty explaining children's consistent use of room geometry, the adaptive combination theory could additionally posit that children do have relevant learning experience for using the walls of the room, across all situations. It is not clear how image-matching theories would account for effects of hiding location on search performance. Like Cheng's original two-process account, however, an image-matching theory of reorientation could appeal to a separate beacon-guidance process to explain these findings.

A recent study by Lee and Spelke (2008) provides a further challenge to the adaptive combination theory of reorientation. Children successfully reoriented by a rectangular arena consisting of 30-cm-high walls that they could see beyond and step over, as accurately as they did in an arena with 1-m-high walls that obstructed both their vision and locomotion. In contrast to their success with the layout of wall-like surfaces, children did not reorient using a rectangular array of four 1.8-m-high columns or a 2D rectangular outline form on the floor. Importantly, the children in the column condition and the 2D form condition never failed to look in one of the relevant hiding places (a column, or a 2D corner), showing that they both attended to and encoded these objects as direct cues to location. These findings cast doubt on the adaptive combination theory in three ways. First, the direct functional relevance of high walls that blocked both vision and navigation did not cause children to rely more heavily on them than on the lower walls that children could see and step over. Second, the large size and apparent stability of the columns nevertheless did not lead children to use their relative positions for reorientation. Finally, the 2D rectangle was not used for reorientation, despite the fact that the round room was devoid of any other competing cues such as a geometrically distinct room shape. In these experiments, therefore, reorientation by surfaces cannot likely be attributed to their functional relevance to navigation, according to their size, salience, and functions as barriers to vision or locomotion.

Nevertheless, all three views can accommodate these findings, in different ways. The modular geometric process view explains children's disoriented behavior in terms of distinct processes for reorienting by 3D surface layouts and for beacon-homing on featurally distinct objects or 2D patterns. On this account, the 3D borders of the surface layout, large and small, distal and proximal, stable and unstable, provide the only inputs to the modular computation of reorientation. At the same time, objects and featural cues are used as beacons, independently of the surface layout computation for reorientation.

The image-matching theory would seem to have particular trouble explaining children's failure to reorient by a configuration of columns, because responses to the retinal projections of configurations of columns provided the first evidence for snapshot-matching processes in insects (Cartwright & Collett, 1982). Nevertheless, the image-matching theory could attribute children's success with wall-like surfaces, as opposed to columns, objects and 2D forms, to the visual salience of the 2D retinal projections from these components of the environment. While the total surface area of the columns tested by Lee and Spelke (2008) was comparable to that of the 30-cm-high walls, the projections of the flat surfaces of the walls, at the child's eye height, produced regions of greater area than those of the cylindrical columns that were 1.8 m tall but only 10 cm wide. Thus, a snapshot matching process may have performed better on spatial localization using the continuous surface array. More conclusive evidence against an image-matching theory would come from studies that do not vary the sizes or shapes of environmental features--variations that affect 2D images as well as 3D layouts--but from studies that vary the dimensionality of those features. If reorientation depends on processes of 2D image matching, then 2D and 3D environmental features that produce the same patterns of brightness contrast should have equivalent effects on children's reorientation.

Finally, the adaptive combination theory can propose that short enclosures, large columns and 2D markings are rare in the environments that children typically experience, and so children may have failed to learn to use them to modulate their navigation strategies. More conclusive tests of the adaptive combination theory would come from studies in which children's performance with the very same objects is compared across systematic changes in the objects' geometric properties. If children's reorientation depends on learned reliability of any environmental component, as predicted by the adaptive combination theory, then children's level of success at using the objects should be modulated by properties such as their size, stability, and distance, and not by their effects on 2D projections of the array (as predicted by image-matching theory) or on the extended 3D surface layout (as predicted by the theory of a geometric module).

The three theoretical positions described above therefore make contrasting, testable predictions concerning the types of arrays and events that will influence children's reorientation. The present research aims to test these predictions by investigating the effects of an object's size, stability, distance, dimensionality, and continuity to the larger layout on the navigation patterns of disoriented children.

Materials and Methods

Overview

Each experiment presented children with two featurally identical columns, boxes, or flat surfaces (hereafter, objects) on one side of an otherwise empty circular room. The two objects were separated by a 90 degree arc and oriented perpendicular to the radius of the circular room such that they faced the center of the room (see Figure 1). In Experiments 1 and 2, the objects were two large, 3D, stationary columns, positioned so that all three theories would predict successful reorientation. Experiments 3-7 then attempted to identify the crucial characteristics of the layout that cause children to include or exclude particular entities in the disoriented spatial representation. Experiment 3 manipulated the objects' size and salience by replacing the columns with small boxes; Experiment 4 and 5 manipulated the stability and mobility of the columns by displacing them between or within trials; Experiment 6 manipulated the dimensionality of the objects by replacing the solid 3D columns with 2D strips of the same area; and Experiment 7 manipulated both the retinal size of the images projected by the columns and the continuity of the columns to the borders of the space, by moving the two columns away from the walls of the room and closer to the child so that they were freestanding.

Figure 1.

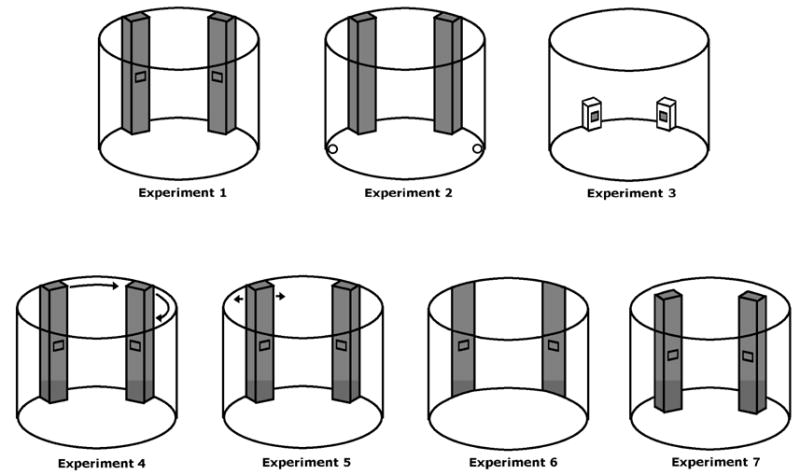
Schematic depictions of the experimental setup. In all experiments, the two objects were separated by a 90-degree arc. In Experiment 4, the columns were moved to a new location between trials but maintained the same relative positions for all trials. Arrows indicate the motion of the column containing the object in Experiment 5; for half the children, the opposite column contained the landmark and was moved.

Testing room

All experiments were conducted within a circular testing room, consisting of twelve curved wall panels, soundproof walls, a solid gray floor, and symmetrical lights mounted on the ceiling. One of the twelve wall panels functioned as the door to the room; from inside the room, the door panel was indistinguishable from the other eleven wall panels. A hidden camera, mounted at the center of the ceiling, provided a video feed to the adjacent room where parents and coders watched the experiment.

Subjects

Subjects were 3-year-old children who were recruited from the greater Boston area. Their parents received cost reimbursements for travel to the lab, as well as toys for the child to take home.

Design and Procedures

All experiments implemented a disoriented search task with a sticker hidden behind one of two flaps that were attached flush against each object (Experiments 1 and 3-7) or with a sticker hidden underneath one of two small containers (Experiment 2). Upon entering the testing space, the experimenter pointed out the two flaps or containers that served as hiding places; with the exception of Experiments 4 and 5, in which the columns were picked up and moved, no experiments involved labeling or calling attention to the objects. The experimenter then showed the child a sticker and placed it in one of the two hiding locations. Next, the child was blindfolded and turned around in place until disoriented (typically about three or four rotations). Disorientation was checked by asking the child to point to the door while blindfolded; if he/she pointed to the door correctly, the child was turned one or two more times and asked to point to the door again. After disorientation was confirmed, the experimenter stood behind the child and faced the child towards one of four predetermined directions. Finally, the blindfold was removed, the child was encouraged to find the hidden sticker, and his or her first choice was recorded. Four such search trials were administered with the facing directions varied across trials and the order counterbalanced across subjects. The hiding location was held constant across all trials for a given subject, but counterbalanced across subjects.

Tests for the children's abilities to maintain their orientation

In order to test whether children reorient themselves by the information provided within an experiment, it is important to ensure that children do not maintain their orientation during the turning procedure. In experiments using a fully symmetrical room, evidence for disorientation comes from children's pattern of search performance itself: if children fail to maintain their orientation during turning, then they will distribute their search equally between the correct location and all other equivalent locations. In the present experiments (as in most experiments in which children respond to a featural cue such as a distinctively colored wall), however, there is only one uniquely correct location. If children succeeded at identifying that location, therefore, their success could stem either from their use of the intended information to reorient themselves or from their discovery of a means to maintain their orientation during turning (for example, by tracking the direction of a subtle light or sound source [see Wang & Spelke, 2000] or attending to surfaces in the room that the occluding hand fails to obscure completely). Because only search at the correct location will yield the hidden reward, children will be motivated to seek such unintended information if it is available, as it will prevent them from becoming disoriented.

In the present study, we tested children's state of disorientation by having them point to the door before opening their eyes, and continuing the turning procedure whenever their pointing was approximately accurate. Because the door is a relatively uninteresting part of the room, however, failure to point correctly during turning provides only weak evidence for disorientation. Consequently, we tested for children's disorientation in two further ways. First, we monitored the number of turns given to children on each trial and tested for an inverse correlation of this variable with children's search performance. Correlations were very small and non-significant in all the experiments (see Table 1). Second, because efforts to maintain one's sense of orientation are apt to improve over successive disorientation trials, we compared children's performance across trials to test for improvements in search. No improvements were seen in any experiment (Table 1).

Table 1.

Effects of turning, trials, and spatial language on children's performance.

	Mean # of turns/trial (SD)	Correlation: Accuracy vs. # of turns	First vs. last trial accuracy	Mean accuracy “Left/Right” test (SD)	“Left/Right” accuracy vs. 50% chance	Correlation: Accuracy vs. “Left/Right”
Exp. 1	4.39 (1.75)	0.05, n.s.	t(15)<1, n.s.	0.56 (0.18)	t(15)=1.38, n.s.	0.09, n.s.
Exp. 2	4.02 (0.99)	0.18, n.s.	t(15)<1, n.s.	0.54 (0.12)	t(15)=1.46, n.s.	0.36, n.s.
Exp. 3	3.68 (1.17)	0.16, n.s.	t(15)<1, n.s.	0.55 (0.24)	t(15)<1, n.s.	0.10, n.s.
Exp. 4	3.81 (1.53)	0.04, n.s.	t(15)<1, n.s.	0.52 (0.20)	t(15)<1, n.s.	0.40, n.s.
Exp. 5	4.32 (1.69)	0.12, n.s.	t(15)<1, n.s.	0.54 (0.22)	t(15)<1, n.s.	0.19, n.s.
Exp. 6	3.70 (1.16)	0.07, n.s.	t(15)<1, n.s.	0.60 (0.32)	t(15)=1.29, n.s.	0.11, n.s.
Exp. 7	3.81 (1.53)	0.01, n.s.	t(15)<1, n.s.	0.58 (0.17)	t(15)=1.93, n.s.	0.26, n.s.

Tests for the use of spatial language to guide children's search

Although young children and animals show similar patterns of spatial performance when they are disoriented, older children and human adults have sometimes been found to navigate in distinctive ways, and their distinctive navigation has been associated with the use of spatial language (Hermer-Vasquez, Spelke, & Katsnelson, 1999; Hermer-Vasquez, Moffet, & Munkholm, 2001; Shusterman & Spelke, 2005). Spatial language has also been found to influence children's performance in other spatial tasks (Dessalegn & Landau, 2008). Although many children do not master productive use of the terms left and right until 6 years of age, the age of acquisition of these terms is quite variable and develops over a protracted time period (Rigal, 1994). By one measure of particular relevance to reorientation, 75% of 5-year-old children can apply the terms left and right appropriately to their own bodies. In order to ensure that young children's performance in the present tasks is not influenced by the early acquisition of spatial language that might allow for representations that extend beyond the nonlinguistic systems of navigation, we assessed the spatial language of each child in these experiments. Following the search trials, children were tested on their comprehension of the words left and right through a set of six randomly ordered questions on their left/right body parts (3 question on left and 3 on right: e.g., “Can you raise your right hand?”). In each experiment, performance on this test was compared to chance (50%) by a two-tailed t-test. Because comprehension of left/right language tends to precede production (Hermer-Vazquez et al., 2001), chance performance on this comprehension test is a conservative means to assure that the participants in the present studies did not draw on spatial language to enhance their search performance. In each experiment, moreover, we tested for the correlation between performance on the spatial language test and performance on the test of reorientation. In all the experiments to be described, children performed at chance on this language test, and their performance on the test showed no correlation with their navigation performance (see Table 1).

Experiment 1

Children were presented with a white circular room with two large, dark, featurally indistinguishable columns placed against its borders, at positions that were 90 degrees apart (Figure 1). On each trial, a sticker was placed in the pocket of one of the columns, the child was disoriented, and then the child was encouraged to find the sticker. The experiment therefore tested whether disoriented children would (a) confine their search to the correct column, consistent with their use of the column as a guide to reorientation, or (b) confine their search to the two columns but search these columns equally, consistent with their use of a column as a beacon.

Methods

Two square columns were placed against the wall on one side of the circular room. The columns were built out of thick foam boards and measured 38 cm on each side and 1.45 m in height. They were covered with blue fabric on the sides and the front, and were white on the back. A flat square pocket (10 cm on each side) was attached to the front of each column and served as hiding places for the stickers. The columns were placed directly against the curved wall, 90 degrees apart, such that they were oriented to face toward the center of the room.

Subjects were 7 boys and 9 girls, between 36 and 46 months old (M = 39.6 months). Two additional children's data were excluded from the analyses because they refused to follow instructions for the disorientation procedure and did not cover their eyes while turning.

Results

On every trial, children directly headed for and searched one of the two columns without searching any other part of the room. Children tended to search in the correct location (66% correct search, SD = 22%, compared to chance = 50%, Cohen's d = 0.71, t(15) = 2.83, p = 0.013, two-tailed). There was no difference in accuracy between the two hiding places, t(14) = 0.55, n.s. There were no sex differences in performance, t(14) = 0.77, n.s.

Discussion

Consistent with the findings of past research, the present experiment provides evidence that disoriented children navigate in accord with the distinctive layout shape produced by two large, stable columns placed against one side of a large circular room. Which of the two navigation processes--reorientation or beacon homing--accounted for their performance? Because the two columns were featurally identical, the simplest beacon homing process would specify the target location in relation to a single column, producing equal search at the two locations. Children distinguished between the two columns, however, and confined their search to the correct column, so their performance is not consistent with this simple beacon homing process. Nevertheless, children's performance could be explained either by a reorientation process that used the columns' locations to break the room's symmetry or by a more complex beacon guidance process. Because the target object was hidden directly at a column, it is possible that children treated the configuration of two columns as a single two-part pattern and then differentiated between the pattern's left and right sides. Although discrimination of the left and right sides of a two-part pattern is subject to error, children can perform this discrimination above chance under some conditions (Dessalegn & Landau, 2008). To distinguish between these two possibilities, the next experiment tested whether children would use the configuration of columns to locate a hidden sticker that was displaced from the configuration of columns, consistent with a reorientation process but not with the kind of beacon-guidance process that has been found to allow children to locate objects that are hidden at, or very close to, the beacon itself.

Experiment 2

Experiment 2 used the same testing space and columns as Experiment 1. In this experiment, however, the columns did not serve as hiding places. Instead, two white 2D disks were placed at the periphery of the round room, separated by 180 degrees such that one disc was situated 45 degrees to the left of the left column and the other was situated 45 degrees to the right of the right column. As in Experiment 1, the target objects were stickers, now hidden underneath one of the two disks. If children's successful search in Experiment 1 depended on their use of the column configuration as a beacon that directly specified the sticker's location, then disoriented children should search at random for the stickers in Experiment 2. In contrast, if children's successful search depended on a reorientation process that incorporated geometric information from the positions of the columns against the room's walls, then children should be able to reorient themselves in Experiment 2 as effectively as in Experiment 1, and locate the hidden object despite its distance from the columns themselves.

Methods

Subjects were 7 boys and 9 girls, between 35 and 46 months old (M = 41.3 months). The apparatus and procedure were identical to those of Experiment 1 except that the dark flaps that served as hiding places on the columns were removed and replaced by two white discs, 10 cm in diameter, that served as hiding places on the floor (Figure 1).

Results

On every trial, children directly headed for and searched at one of the two disks without searching any other part of the room. Children searched more often in the correct location (66% correct, SD = 22%, compared to chance = 50%, Cohen's d = 0.71, t(15) = 2.83, p = .013, two-tailed). There was no difference in accuracy between the two hiding places, t(14) = 1.20, n.s. There was no significant sex difference in performance, although there was a trend favoring females (girls: 75% correct; boys: 54% correct; t(14) = 2.14, p = .051).

Discussion

Although the goal locations were spatially separated from the columns, children used the columns to guide their search to the correct goal location. They performed as well in this experiment as in Experiment 1, in which one of the two columns served as the location at which the target object was hidden. Together, Experiments 1 and 2 provide evidence that children used the columns as information for reorientation with respect to the shape of the surrounding layout. When these large and stable columns were placed against the walls of the room, children reoriented themselves in accord with their relative positions.

Because the columns in Experiments 1 and 2 were large and stable, the findings of these experiments are consistent with all three theories of reorientation, which offer different explanations for children's performance. According to the modular reorientation theory, children reorient by the columns because they are included in the geometric representation of the borders of the room. According to the image-matching theory, children's reorientation is modulated by the columns because they give rise to large and salient image features in retinal projections of the environment. According to the adaptive combination theory, children reorient by the two columns because they have learned that large and distal landmarks are stable and reliable. The remaining experiments test these explanations by varying properties of the columns that bear on the shape of the room's borders, the image properties of the room's projections, and the size and stability of the environmental objects.

In all these experiments, we use the method of Experiment 1, in which a sticker is hidden directly at one of two columns. The experiments use this method, because they seek to specify the conditions under which disoriented children succeed and fail to reorient in accord with a pair of environmental objects, and the method of Experiment 2 yields ambiguous findings in any experiment in which the reorientation system fails. If children were to search at random between two spatially displaced locations arranged as in Experiment 2, that failure would be open to two distinct interpretations: children's reorientation system might be impervious to the information provided by the landmarks, or children might fail to detect, attend to, or remember the landmarks. The method of Experiment 1 distinguishes between these potential accounts of unsuccessful search performance. If children fail to attend to or remember the objects, then they should fail to search altogether or search at random locations within the space or around the objects. If children attend to and remember the objects and their features but fail to reorient by them, in contrast, then children should reliably confine their search to the two hiding places but choose between them equally.

Experiment 3

Experiment 3 tested the importance of the size and salience of the objects to disoriented children's ability to use their spatial arrangement, by replacing the large and dark columns of Experiment 1 with small boxes of the same color as the surrounding walls (white). The adaptive combination theory predicts lower performance in this situation than in Experiment 1, because children are expected to have learned that small objects are movable and, therefore, less reliable than large objects as cues to location (Newcombe & Ratliff, 2007). The visual snapshot account makes a weak prediction of lower performance in this case, given that the small boxes of the same color as the walls of the room may not be salient enough to contribute strongly to a coarse-grained visual image matching system. In contrast to these two theories, the modular theory predicts success even with small objects when the objects are placed at the borders of the array, as they yield 3D surface contour information for the geometric reorientation system.

Methods

The methods for Experiment 3 were identical to those of Experiment 1 except for the size and color of the objects: the large blue columns were replaced by small white boxes (30 cm by 15 cm by 15 cm) (see Figure 1). The flat pockets on one face of the boxes were the same size and color as in Experiment 1. Subjects were 8 girls and 8 boys between 36 and 45 months of age (M = 39.6 months).

Results

On every trial, children directly headed for and searched one of the two boxes without searching any other part of the room. Children tended to search in the correct location (67% correct, SD = 18%, compared to chance = 50%, Cohen's d = 0.98, t(15) = 3.91, p = 0.001, two-tailed). There was no difference in accuracy between search at the two hiding places, t(14) = 1.07, n.s. There was no effect of sex, t(14) = 0.13, n.s. Performance in this experiment did not differ from performance in Experiment 1, with columns against the wall (t(30) = 0.22, n.s.), showing that the reduction in the size of the objects did not affect children's use of them.

Discussion

Although the objects on the wall were small and subtle, disoriented children used their relative positions to differentiate the two boxes and search the correct location more often than the incorrect one. Children searched as accurately when the room's symmetry was perturbed by small boxes as when it was perturbed by large columns, even though performance in both conditions was well below ceiling. In these experiments, therefore, object size failed to influence children's navigation.

What explains disoriented children's ability to use the layout of the columns and boxes in these experiments? According to the adaptive combination theory, the stability and permanence of an environmental component affect the degree to which children use it to reorient. While the boxes in Experiment 3 were small, they were nevertheless stable – children never saw them move. It is possible, therefore, that children encoded them as attached to the wall and as permanent features of the room. Experiments 4 and 5 test the claim that experiencing objects as movable and unstable decreases children's dependence on them for navigation.

Experiment 4

Experiment 4 investigated the search behavior of disoriented children using the same array of columns as in Experiment 1. In contrast to Experiment 1, however, the stability of the columns was compromised by moving them to new locations against the wall before each trial. At the start of each reorientation trial, the two columns appeared in the center of the round room. Then the experimenter lifted each column in turn and placed it against the wall, so as to form the same geometric configuration as in Experiment 1. While moving the columns, the experimenter commented on her actions and labeled the columns with a term typically applied to movable objects: as boxes. To reduce the perceived stability of the columns still further, their absolute positions against the wall were rotated 90 degrees between successive trials, although their relative positions were invariant. Research on place learning in rats indicates that landmark stability affects oriented rats' use of an object as an indirect cue to a goal location (Biegler & Morris, 1996). Will the perceived movement of the columns diminish children's spontaneous tendency to reorient by them?

According to the adaptive combination theory, children should learn not to rely on unstable, movable objects for reorientation; thus children should use the movable objects as cues to a lesser degree than they use stable, immoveable surfaces. In contrast, the snapshot view and the modular two-process view both predict success, although for different reasons. According to the snapshot view, large, salient columns should have the same effect on the image matching process that is applied to a retinal array, regardless of their stability, because snapshots are blind to the history of an array. According to the modular view, the mechanisms by which an animal reorients have evolved to be sensitive to the surface layout because of their distinctiveness and stability (and therefore, their ecological validity) in ancestral environments. Nevertheless, the encapsulated nature of the computation should render the child incapable of strategically suspending this analysis in the face of information that a part of the layout that typically is stable has in fact proved to be movable in this particular environment.

Methods

The methods for Experiment 4 were identical to those of Experiment 1 except for the movement of the columns before each trial: the columns started out placed in the middle of the room. When the experimenter brought the child inside the circular room, she moved the columns to one side of the room and placed them against the wall in one of four possible positions before starting the first hiding event. After the disorientation and search trial, the experimenter moved the columns one by one to a new position in the room against the wall, before beginning the next hiding event. Whenever the columns were moved, the experimenter said to the child, “Now, we are going to move the boxes.” The left/right relation of the two columns was held constant (i.e., the left column was still the left column after the columns were moved to new positions), and the distance between the two columns was the same as in Experiment 1. Because the positions of the columns was varied across trials, the direction in which the child faced after disorientation was fixed, such that for each search trial the relative position of the columns to the child (at the time the child opened his/her eyes) was varied and therefore identical to the other experiments. The order of the positions was varied and counterbalanced across subjects. Subjects were 9 boys and 7 girls, between 36 and 45 months of age (M = 39.4 months).

Results

On every trial, children directly headed for and searched one of the two columns without searching any other part of the room. Children searched in the correct location reliably (69% correct, SD = 21%, compared to chance = 50%, Cohen's d = 0.88, t(15) = 3.50, p = 0.003, two-tailed). There was no difference in accuracy between search at the two hiding places, t(14) = 1.18, n.s. We found no significant effect of sex, but a trend favoring the boys (boys: 79% correct, girls: 61% correct; t(14) = 1.72, p = 0.11).

Discussion

Despite clear evidence that the columns were labeled as boxes, were movable and therefore were an unstable part of the spatial layout, children performed as accurately as they did in Experiment 1. These results suggest that children do not adjust their dependence on layout geometry in the face of evidence for their impermanence and instability. However, it is possible that the methods used in this particular experiment failed to compromise the columns' stability sufficiently. Specifically, the movement of the columns always occurred before a particular hiding-finding trial, never during the trial. It is possible that children refreshed their spatial representation of the layout between trials and represented the columns as stable within each trial of the task. Experiment 5 addressed this possibility by providing children with evidence of the columns' mobility within trials rather than between them.

Experiment 5

In Experiment 5, both the movement of a column and its labeling as a box were introduced at a more critical point in the task: after the object was hidden at the column and just before the child was disoriented. If children are capable of suspending the use of layout geometry for reorientation when layout features cease to be stable, then children should perform less reliably in this experiment than in the previous studies.

Methods

The methods were identical to Experiment 1, except for the introduction of a single “column shaking” step between the hiding and disorientation procedures. After the sticker was hidden in one of the columns, the experimenter picked up the column and while shaking it said, “Now, we are going to pick up the box and shake it. See? It can move around!” To avoid introducing long time delays or difficulties in tracking the correct column, the columns were not carried to new positions in the room and only the correct column was shaken. Following the shaking procedure, the column was placed back in its original location, and the child was brought to the center of the room to be disoriented. Subjects were 9 girls and 7 boys between 36 and 43 months of age (M = 39.9 months).

Results

On every trial, children directly headed for and searched one of the two columns without searching any other part of the room. Children searched in the correct location successfully (75% correct, SD = 20%, compared to chance = 50%, Cohen's d = 1.22, t(15) = 4.90, p < 0.001, two-tailed). There was no difference in accuracy between the two hiding places, t(14) = 1.25, n.s. We found no effect of sex, t(14) = 1.25, n.s. Performance in the two stationary conditions using this method (Experiments 1 and 3) did not differ from performance in the two movement conditions (Experiments 4 and 5) (67% vs. 72%, t(62) = 1.08, n.s., Bonferroni corrected), showing that stability did not affect children's use of the columns for reorientation.

Discussion

The results of Experiment 5, like those of Experiment 4, show that evidence of impermanence and instability does not influence whether an object is used as a part of the surface layout representation for reorientation. Children's tendency to search in relation to the columns was not diminished by evidence for their mobility, either overall or progressively over the course of the experiment. These findings suggest that reorientation performance is quite resistant to evidence that the borders of the layout are not stable, contrary to the predictions of the adaptive combination theory.

This resistance is consistent both with the modular geometric theory and with the image-matching theory. The former theory interprets these results as evidence for an automatic, encapsulated representation of surface layout whose operation cannot be adjusted strategically in the face of information that components of the large-scale layout cannot be trusted. While the stability of the environmental shape may have played a role in the evolution of sensitivity to surface layouts, observation of mobility in real time does not change the way reorientation is computed. Therefore, children relied on the movable columns as though they were a part of the surface layout in the same way they did in Experiment 1. The image-matching theory provides an equally plausible account of these findings, because snapshots of an array contain no information about its history. No matter how the columns were moved in Experiments 4 and 5, the resulting arrays always projected the same contours in panoramic 2D images. The image-matching theory therefore accounts well for performance in Experiments 1, 2, 4 & 5. Moreover, it can be stretched to account for children's performance with small objects in Experiment 3, by assuming either that snapshots of an array are fine-grained or that the grain of the snapshots is adjusted in accord with the amount of information in the images, such that projections of small objects will influence the image-matching process when images are otherwise homogeneous. Consequently, the last two experiments contrasted these two views directly.

Experiment 6

Experiment 6 tested the effect of dimensionality on reorientation by presenting children with two 2D, dark rectangular strips with the same dimensions as the columns used in the previous experiments. These strips were placed directly on the circular wall at the same angular positions as in Experiments 1-5, relative to the child's position at the room's center. According to the image-matching theory, large objects will be used for reorientation, regardless of whether they are 3D or 2D, because only their salience in the retinal projection influences the image comparison process that guides navigation. Although the experiment was not designed to test the adaptive combination theory, it also predicts success with the distal 2D strips, especially because there are no competing cues in the room. In contrast, the modular geometric theory predicts that children will use 2D surface markings as beacons by which they can localize an object, but not as part of the geometry of the surface layout by which they reorient. On this view, therefore, disoriented children should confine their search to the two strips but choose at random between them.

Methods

Subjects were 9 boys and 7 girls, between 36 and 45 months old (M = 39.9 months). One additional child participated whose data were not included in the analyses because he refused to follow the blindfolded disorientation procedures. Experiment 6 was identical to Experiment 1, except that the 3D columns were replaced by 2D strips of equal width and height as the 3D columns (38 cm by 145 cm), made of contact paper and pasted on the wall of the room (see Figure 1). The hiding pockets were also formed using contact paper and functioned in the same manner as the pockets in the previous conditions.

Results

On every trial, children directly headed for and searched one of the two strips without searching any other part of the room. Children nevertheless searched the correct strip only 47% of the time (SD = 30%, compared to chance = 50%, Cohen's d = 0.10, t(15) = 0.42, n.s.). There was no difference in accuracy between the two hiding places, t(14) = 0, n.s. There was no effect of sex, t(14) = 0.10, n.s. Comparing with the conditions in which hiding places were 3D and stationary (Experiments 1 and 3), children's performance was significantly worse using the 2D strips in the present experiment (67% vs. 49%, t(46) = 2.69, p = 0.03 0.05, two-tailed, Bonferroni corrected).

Discussion

Children searched directly and consistently at the locations of the two 2D strips, showing that they detected the strips, remembered that the object was hidden at one of them, and used one or the other strip as a beacon to guide their search. Despite this ability, children failed to reorient by the strips and confine their search to the correct location, as they did in the previous experiments. These results provide evidence that children reorient by 3D surfaces but not by 2D surface markings yielding regions of different color and brightness, contrary to image matching and adaptive combination views and in accord with the modular reorientation view. This failure to use a 2D surface marking in a large space, in which children were allowed to freely move about, provides further evidence that children use non-geometric surface features like a distinctively colored wall as a beacon to guide their search to nearby locations, but fail to reorient by this information.

The findings also provide further evidence that children's success in Experiments 1-5 does not depend on a complex beacon guidance process, in which two objects are represented as a single two-part landmark with distinctive leftward and rightward sides. If children were able to use a complex beacon guidance process to constrain their search, then they should have located the hidden object at the leftward or rightward strip even if they remained in a state of disorientation. In the absence of an effective reorientation process, however, disoriented children were not able to engage such a search process to locate the hidden object under the conditions of the present experiment.

While it seems that the physical properties of mobility and distance from the observer are not the determining factors for reorientation in this task, it is still unclear why children succeeded in Experiments 1-5, given that they failed to use arrays of freestanding columns and objects in the middle of the room in prior experiments (Gouteux & Spelke, 2001; Lee et al., 2006; Lee & Spelke, 2008). Research on navigating rodents suggest that the borders of a navigable space profoundly influence neural encoding of an animal's own position (O'Keefe & Burgess, 2006; Solstad et al., 2008), whereas freestanding objects do not (Cressant et al., 1997). Thus, objects that are continuous with the walls of the room may change the room's perceived shape (i.e., surface layout), whereas objects in its interior may not. If that is indeed the case, then the same 3D columns that successfully guided children's reorientation in the previous experiments should not serve as a basis for reorientation if they are offset from the walls of the room, such that the real and perceived shape of the room is circular and uninformative for a geometric reorientation process. In contrast, columns that are offset from the walls will be represented more prominently in the retinal projection of the room than are columns at the borders of the room, because of the greater size of the images they project in panoramic retinal arrays. The image-matching theory and the geometric module theory therefore make opposite predictions concerning children's reorientation by columns that are offset from the walls of the room.

Experiment 7

In Experiment 7, we placed the 3D columns from Experiments 1, 2, 4 and 5 in two positions that were similar to those of Experiment 1 but that were offset from the circular wall of the room. Various precautions were taken to prevent possible confusion by the children as to which side of the columns they faced following disorientation. The front and sides of each column were of a different color and texture from the back and the hiding pocket appeared only on the front side. Furthermore, the placement of the columns in the circular space was clearly asymmetrical, as they were placed perpendicular to the radius of the circular room such that they were oriented to face the center of the room. To show children that the columns were separated from the walls without giving verbal cues, children were walked once along the periphery of the room at the beginning of the session. Thereafter, all testing occurred with the child in the center of the room, viewing the wall and the columns from the same perspective as in the past experiments.

Methods

Subjects were 7 boys and 9 girls, between 36 and 47 months old (M = 42.4 months). One additional child's data were excluded from the analyses because he refused to keep his eyes covered while turning. Experiment 7 was identical to Experiment 1 except that the columns were fixed to the floor at a distance of 25 cm from the edge of the room, which was far enough from the wall so that the experimenter could walk behind them without touching them (see Figure 1). Before starting the game, the experimenter walked the child once along the edge of the room.

Results

On every trial, children directly headed for and searched one of the two columns, without searching any other part of the room and without walking to the other side of the room or approaching the columns from the opposite side. Nevertheless, children searched the two columns at random (50% correct, SD = 25%, compared to chance = 50%, Cohen's d = 0, t(15) = 0, n.s. There was no difference in accuracy between search at the two hiding places, t(14) = 1.36, n.s. There were no sex differences, t(14) = 1.05, n.s. Comparing against the two conditions in which columns or objects were stationary and against the wall (Experiments 1 and 3), we find that the present manipulation of setting the columns off from the wall resulted in significantly lower accuracy (67% vs. 50%, t(46) = 2.51, p = 0.048, two-tailed, Bonferroni corrected).

Discussion

Children successfully detected the columns and remembered that a sticker was hidden in one of them. Nevertheless, children failed to reorient in accord with the positions of these two large, stable, freestanding columns within the large circular room. These results accord with the modular account of reorientation, which proposes that only the continuity of objects with the extended surface layout allows objects to be incorporated into the geometric representation of the borders of the navigable array.

The present findings are difficult to reconcile with the image-matching theory. When the columns are moved from the walls of the display toward the center of the display, their projections on the retinal snapshot increase in size. Depending on the detailed nature of the image-comparison process, this size difference should either enhance image comparison and reorientation (if the resolution of the snapshot is extremely poor) or should fail to affect it (if resolution is sufficiently high: see Stürzl et al., 2008). In neither case, however, can a 2D image-matching process account for the present failure of children to reorient by the freestanding columns. We conclude that children's reorientation depends not on processes for matching unanalyzed retinal projections but on processes for establishing geometric congruence between the perceived and the remembered borders of the 3D spatial layout.²

General Discussion

One of the most lively debates in psychology stems from the idea that the mind consists of specialized, encapsulated parts that are functional adaptations, evolved to solve specific tasks by processing only a subset of the available environmental information (Fodor, 1983; Newcombe & Ratliff, 2007; Cheng, 2008). In the present study, we used studies of children to contrast the influential hypothesis of Cheng (1986) and Gallistel (1990) - that a modular process, sensitive to the geometry of the surrounding surface layout, underlies our sense of orientation - with two of its primary challengers: the adaptive combination theory of Newcombe & Ratliff (2007) and the image-matching theory of Cheng (2008; Stürzl et al., 2008). Through a series of seven experiments, we demonstrated that disoriented children's search is guided by surface layout geometry regardless of whether the surfaces are large or small, distal or proximal, stable or movable, salient or subtle Figure 2. Because disoriented children searched equally well when a sticker was hidden at a column (Experiment 1) or away from it (Experiment 2), the experiments provide evidence that the columns were used for reorientation, not for beacon guidance. Because disoriented children searched equally effectively with large columns (Experiments 1 and 2) or small objects (Experiment 3), and equally effectively with columns or objects that were stable (Experiments 1-3) or movable (Experiments 4 and 5), the experiments provide evidence that reorientation was not affected by a history of learning that makes distal, large, and stable objects more reliable cues than proximal, small or unstable ones. Finally, because children reoriented by columns or objects that were positioned so that they protruded from the walls of the room (Experiments 1-5) but not when they were offset from the walls (Experiment 7) or were replaced by 2D markings of similar size and contrast (Experiment 6), the experiments provide evidence that children's reorientation was guided by representations of the 3D shape of the borders of the array and not by the representation of salient objects or of contrast edges in retinal arrays. The crucial factor that determines whether children will use a potential landmark to reorient is not its size, distance, stability, or image features, but its alteration of the shape of the surrounding environment - the extended 3D surface layout. All these findings provide evidence for Cheng and Gallistel's original claims for a geometric module.

Figure 2.

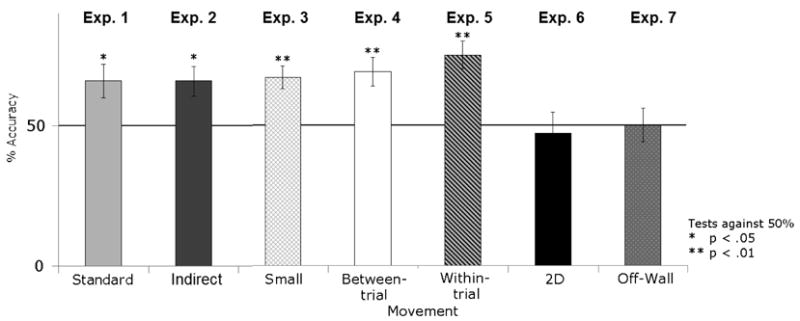
Children's accuracy in each experiment, with two-tailed t-tests against a chance level of 50%; significant comparisons are marked with asterisks. The first study (“Standard”) used large 3D stationary columns against the walls: each of the other studies changed one aspect of this display; labels indicate the nonstandard aspect.

Modularity³ has been mistakenly thought to imply that an encapsulated computation determines behavior regardless of other cognitive processes. Thus, evidence that disoriented children's search is guided by features or objects in an array is sometimes taken as evidence against a modular reorientation process (see Newcombe & Ratliff, 2007; Cheng, 2008). However, experiments in which disoriented children ignore non-geometric features of an array altogether provide only weak evidence for an encapsulated process of geometrical analysis, because they do not reveal whether children detect, attend to, remember, and appreciate the relevance of the non-geometric information. Encapsulation--the central property of a modular system--refers to the impenetrability of the computations that the system performs (see Fodor, 1983). Thus, the best evidence for a geometric module for reorientation comes from experiments in which disoriented navigators detect, remember, and use non-geometric information to guide other navigation processes, but reorient only by surface layout geometry. The present studies provide just such evidence. The children in these studies used both 2D patterns (in Experiment 6) and freestanding 3D columns (in Experiment 7) as beacons to guide their search, but they failed to reorient by these objects. Children reoriented only by the 3D shape of the extended, bordering surface layout.

The finding that children reorient by large objects placed at the walls of the enclosure (Experiments 1, 2, 4 and 5) but not by large freestanding objects (Experiment 7), is consistent with new findings by Nardini, Thomas, Knowland, Braddick, & Atkinson (2009). In a series of experiments, 4-8 year old children viewed the hiding of a toy in a symmetrical environment, while standing on one side of a centrally located, large freestanding object, with a shape that distinguished its front and back. When children subsequently were disoriented and then were presented with the array from the same position or from the opposite side of the object, children six years and older were able to locate the target from both perspectives. In contrast, younger children searched correctly only when they viewed the array from the same perspective at hiding and test. Importantly, control experiments revealed that the young children succeeded in locating the hidden target from any perspective when they remained oriented between hiding and test (Nardini et al., 2009). Although children of these ages have overcome some of the limits on younger children's disoriented searches (e.g., Hupbach & Nadel, 2005; Hermer-Vazquez et al., 2001), they evidently could not use the distinctive geometric structure of the freestanding object in order to reorient themselves and locate the hidden target. These findings provide further evidence that young children can use object features as beacons, but that they fail to use large, freestanding objects as reliable cues for reorientation.

The finding that children reorient by border features that are 3D but not 2D accords with those of Gouteux & Spelke (2001), in which children failed to reorient by a single large 2D patch on a circular enclosure, but they fail to accord with the findings of new experiments by Newcombe, Ratliff, Shallcross & Twyman (2009), in which disoriented children used a single large potential landmark (a red sheet or quilt that covered one wall) to distinguish between distant search locations. When two containers were placed in the middle of a circular room with a quilt hanging on one side, 4- to 5-year-old children who were disoriented performed above chance levels at relocating an object hidden in one of the containers. Newcombe et al. (2009) also tested three-year-old children in an octagonal room with walls of two alternating lengths, with all-white walls in one condition and with one of the walls covered by a red sheet in a second condition. In the all-white condition, children tended to search the correct corner more than other three geometrically equivalent corners (23% vs. 16%), suggesting the possibility of incomplete disorientation, but this tendency was not significant (t (16) = 1.63, p = .07, one-tailed). In the condition with one red wall, children searched the correct corner significantly more than the other three corners (35% vs. 12.5%). The authors did not report analyses comparing across these conditions, and so we cannot be sure that children's rate of correct searching was significantly enhanced by the addition of the red wall. If it was, however, then these findings also provide evidence for use of a colored wall in reorientation, contrary to the present findings and those of numerous past experiments (Gouteux et al., 2001; Wang et al., 1999; Learmonth et al. 2008).

Nevertheless, direct comparisons of the present findings to those of Newcombe et al. (2009) are complicated by a number of differences between the experiments. For the circular room experiments, Newcombe et al. tested children of a range of ages overlapping the age--5 years--at which most children have begun to learn the terms left and right (Rigal, 1994), did not check for children's individual knowledge of left/right spatial language, and did not confirm children's disorientation during the search procedure, leaving open alternative factors that may have affected performance. Moreover, the potential landmark was an object with some thickness--a quilt--that was placed on the wall and may have introduced subtle asymmetries into the shape of the 3D environment. Because the octagonal room experiments were conducted with much younger children, the first two of the above issues do not apply to them, but the lack of direct evidence for use of the red wall, and the possibility of incomplete disorientation remain. Future experiments will be needed to determine the reasons for these differing findings.

While the present experiments are suggestive of a specialized process of geometric surface layout representation, further research is needed across the many areas of cognitive science to clarify exactly how children and animals represent the shape of their environment and what information they extract about environmental shape. In particular, recent studies suggest that children's representation of layout geometry fails to incorporate information about the angles at which surfaces meet (Lee & Spelke, in review; see also Hupbach & Nadel, 2005), and that children fail to reorient by the shapes of complex surface layouts consisting of four walls of unequal lengths or 3-4 large objects at the periphery of a circular array, separated by unequal distances (Lew, Gibbon, Murphy, & Bremner, 2010). Although Lew et al. (2010) suggest that children may reorient successfully only in symmetrical arrays, children successfully reoriented by asymmetrical arrays both in the present experiments and in those of Wang et al. (1999). Children's failure to reorient by 4 walls or 3-4 object separations of unequal distances may stem from the greater demands that multiple geometrical contrasts place on children's relational processing (see Huttenlocher & Lourenco, 2007) or memory (see Feigenson & Carey, 2005). In either case, however, children's reorientation system evidently fails to capture the full richness of Euclidean geometry (Spelke, Lee, & Izard, in press).

Research also is needed to clarify the ways in which the outputs of the reorientation system combine with the outputs of other mechanisms, such as beacon-guidance, to guide the behavioral decisions of humans and animals. It is possible that navigation behavior results from a process of weighting one's own sense of orientation, and the information conveyed by beacons, according to one's experience of their past usefulness in similar situations, as envisaged by adaptive combination theories of decision-making (see Footnote 1). The findings of a new controlled-rearing experiment on mice provide suggestive support for this possibility (Twyman, Newcombe & Gould, in review). In this experiment, mice who were raised in a rectangular environment encoded and used the shapes of testing environments automatically in disoriented search tasks, providing evidence that they, like normally reared animals and animals raised in environments devoid of both geometry and features (Brown et al., 2007; Chiandetti & Vallortigara, 2008), reoriented by layout geometry and used their sense of orientation to find the hidden object. In contrast, mice raised in a circular environment, with only a colored wall to specify the location of food throughout the rearing period, searched according to the color cue in subsequent tasks in which room shape cue was also available. After a lifetime of rewarded experience with only beacons, and not room geometry, the mice may have learned to be selectively attentive to beacons and may disregard their sense of orientation by geometry in foraging tasks. Although the reorientation process is modular, decisions to use or to disregard the information that it provides may indeed result from an adaptive combination of information from diverse sources, weighted in accord with its past validity (Huttenlocher & Newcombe, 2006; Cheng et al., 2007)

Further research also is needed to specify the processes by which navigating animals and humans single out features of the environment as beacons. Image-matching processes aid in children's selection of beacons for navigation, just as they aid oriented insects in their encoding of the locations of food sources (Cartwright & Collett, 1982). The findings of Experiments 6 and 7 provide some support for this possibility, because children were equally able to use 3D freestanding columns and 2D surface patches for beacon guidance, despite their differing dimensionality and affordances. Although image-matching theories fail to account for children's reorientation, they may play a role in explaining other aspects of children's navigation.

Beyond the immediate issues that motivate the present experiments are larger suggestions concerning the place of modular cognitive systems in the development of human knowledge. The present findings provide evidence that children's reorientation system has two properties of Fodor's (1983) proposed modular systems: it is automatic (children encode the shape of the 3D layout before they are disoriented and learn of its relevance) and encapsulated (children fail to encode the shape of 2D environmental features or the positions of freestanding objects, even though they detect them, remember them, and appreciate their relevance to the search task). Research on other populations, using other methods, provides evidence that the system for encoding environmental geometry has further hallmarks of a Fodorian module: it develops in the absence of relevant experience navigating in a geometrically structured layout (Chiandetti & Vallortigara, 2008; Brown et al., 2007), it has a specific genetic basis (Lakusta, Dessalegn, & Landau, 2010), it is instantiated in specific neural mechanisms (e.g., Doeller et al., 2008; Solstad, et al., 2008), and its operation appears to be mandatory (Doeller & Burgess, 2008; Cheng & Newcombe, 2005; although see Pearce, et al., 2006; Twyman et al., in review; and Cheng, 2008, for evidence that animals can learn navigation strategies that disregard its outputs).

The system for analyzing layout geometry may have a final property of Fodorian modules: it may be largely opaque to conscious reflection. In distinguishing the geometrically appropriate from the inappropriate corners of a rectangular room, human adults reliably distinguish locations on the left of a geometrically distinctive axis from those on the right. When adults are asked to explain their choices of corners, however, they rarely appeal explicitly to these directional relations (Hermer, personal communication 1994), and many adults report that they have trouble distinguishing left and right in verbal or other symbolic contexts. Similarly, the children in past experiments and in the present ones reliably distinguish correct from incorrect hiding locations by representing their directional (left/right) relations, yet such children have not yet acquired consistent spatial language and are far below the age at which children can reliably distinguish object shapes from their mirror images on the basis of their sense relations (Izard & Spelke, 2009; see also Dessalegn & Landau, 2008). Although Fodor's modularity hypothesis was articulated in a very different context, it appears to capture many of the properties of this navigation system.

At the same time, this encapsulated reorientation mechanism has a property that connects it to abstract higher cognition: it is a system of geometric representation and computation. In different ways, image matching and adaptive combination theories both deny that children's navigation involves geometric representations and computations, in a mathematically interesting sense. On the adaptive combination view, navigation depends on whatever environmental features prove to be reliable: geometrical concepts like long and left have no special status for navigation that distinguishes them from non-geometrical concepts like red or shiny (Newcombe & Ratliff, 2007). On the image-matching view, geometric concepts and computations do not even enter into the computations that support navigation, which depend only on local analyses of image contours (Cheng, 2008). In contrast to both these views, the present findings suggest that truly geometric representations and computations have a distinct and special status for the navigating child.

Together, these two suggestions prompt a third. Abstract Euclidean geometry is one of the highest achievements of the human mind, permitting the construction of maps, measurement devices, and mathematical reasoning abilities that are unique to humans. It is possible, however, that this geometry is grounded, in part, in representations and computations so ancient that they are shared by insects, and so encapsulated that they operate without awareness in the youngest children, who cannot yet fathom the meanings of the words or symbols that describe them. Over the course of human development, the geometry in this encapsulated navigation system may give rise to representations that enter both into the maps and models of the child who uses symbols to solve spatial problems, and into the axioms and postulates of the adult who reasons about formal geometry. Studies of disoriented children's navigation within small and unusual environments, for all their idiosyncrasies, may serve as a springboard for testing these possibilities.