Connecting Biology and Mathematics: First Prepare the Teachers

Abstract

Developing the connection between biology and mathematics is one of the most important ways to shift the paradigms of both established science disciplines. However, adding some mathematic content to biology or biology content to mathematics is not enough but must be accompanied by development of suitable pedagogical models. I propose a model of pedagogical mathematical biological content knowledge as a feasible starting point for connecting biology and mathematics in schools and universities. The process of connecting these disciplines should start as early as possible in the educational process, in order to produce prepared minds that will be able to combine both disciplines at graduate and postgraduate levels of study. Because teachers are a crucial factor in introducing innovations in education, the first step toward such a goal should be the education of prospective and practicing elementary and secondary school teachers.

INTRODUCTION

Students often choose biology as a safe refuge inside the archipelago of science disciplines, one where mathematics can be in most cases tolerated or even ignored. Negative attitudes toward mathematics are not unusual. I illustrate this statement with my own case. In my career as a biology and ecology teacher at a general secondary school and higher vocational school over 23 years (1985–2008), I began with the attitude that biology and mathematics have nothing in common. Later, however, during the struggle against rote learning and a decrease of interest in science (Baram-Tsabari and Yarden, 2009), I made a personal transition from mathematics ignoramus (or even hater) to a user, if not admirer. The transition did not happen as a moment of ecstatic enlightenment but step by step over years of work in the classroom.

The transition had nothing to do with the pressing need of the research community to connect both disciplines (Elser and Hamilton, 2007; Robeva and Laubenbacher, 2009), neither was it the consequence of the tectonically top-down curricular changes to the Slovenian school system. The transition has its roots in the recognition, as a teaching practitioner, and well supported by a body of research (Michael, 2006; Metz, 2008; DiCarlo, 2009), that only active learning methods work to increase student learning. This recognition facilitated my transition from teaching based on descriptive instruction toward explanation and inquiry, and from expository toward inquiry- and problem-based labs (Domin, 1999). This transition was by no means easy. When, as a teacher, I left behind the well-traveled ways of traditional explanatory instructions, I found myself, together with my students, in a wild landscape of misconceptions, partial truths, and patches of “terra incognita”. The first thing I had to acknowledge was that not knowing something is not a matter of shame but a possible route of inquiry. Another impetus was my research work with partners from universities, for which I read primary scientific articles and learned basic multivariate statistical methods. I was lucky enough to escape the fate of the majority of secondary school teachers, who are mostly ignored as potential partners or project coworkers by the large body of researchers in the field of science or technology; it is even well known that such connections are fruitful for students as well (Krontiris-Litowitz, 2009; Seidenberg, 2009). Last and not least important was the introduction of information and communication technology (ICT) into laboratory work (Šorgo and Kocijančič, 2006; Šorgo et al., 2008), an impetus to more deeply understand mathematics (Murovec and Kocijančič, 2004; Šorgo and Kocijančič, 2004).

THE SLOVENIAN SCHOOL SYSTEM

Slovenian primary and secondary schools are predominantly public (private schools, <1%). Science is taught as an integrated subject in the first seven years of compulsory schooling. After that it is divided into biology, chemistry, and physics and taught as separate subjects in the last two years of compulsory schooling. Mathematics is compulsory in all nine years. The same division is prolonged into general high schools, called “gimnazija.” About 98% of the students continue compulsory education in upper secondary schools. The main goal of the gimnazija is preparation of the students for final examinations called “matura,” success in which is a prerequisite for entering university. Biology, chemistry, and physics are compulsory subjects in the first three years of the gimnazija and elective in the fourth year. Mathematics is a compulsory final exam for all general secondary school students. In vocational schools subject diversity is much higher (Aberšek, 2004).

Curricula for the whole school system are approved by the governmental bodies, a situation which allows only limited autonomy to individual schools and teachers in choosing elective content and subjects but which leaves teachers free to choose teaching methods (Šorgo and Šteblaj, 2007). At the upper secondary level, teaching is greatly influenced by the external matura examinations (Ivanuš Grmek and Javornik Krečič, 2004, Pšunder and Harl, 2008).

BEYOND PERCENTAGES

Mathematics syllabi demonstrate expectations for students to be able to calculate/solve limits, functions, derivatives, integrals, and infinite series, to solve a variety of equations, define extremes and plot graphs, convert one trigonometric function into another, and many other tricks from the magic hat of mathematics, just to pass the matura examination. To pass the biology examination students must know that calcium ions can function as secondary transmitters in cells, understand why in the evolution of mosses, ferns, and vascular plants there is a trend for domination of diploid over the haploid phase in their life cycles, and to be able to compare and find differences between C3 and CAM photosynthesis. Standard syllabi rarely make connections between biology and mathematics, and even those are at an elementary level. According to the biology curriculum, a very successful biology teacher only needs to know how to calculate percentages, construct graphs from the tables, and calculate probabilities in Mendelian genetics. On the other hand, the only biology an excellent mathematics teacher needs to know is that population growth is exponential and how to calculate probabilities in Mendelian genetics.

Table 1.

Science subjects and weekly hours dedicated to science subjects and mathematics in compulsory 9-yr education

Subject/grade	1	2	3	4	5	6	7	8	9	Total
Mathematics	4	4	5	5	4	4	4	4	4	1318
Environmental studies	3	3	3							315
Science and technology				3	3					210
Science						2	3			175
Biology								1.5	2	116.5
Chemistry								2	2	134
Physics								2	2	134
Technic and technology						2	1	1		140

Table 2.

Science subjects and weekly hours dedicated to science subjects and mathematics in general secondary education

Subject/grade	1	2	3	4	Total
Biology	2	2	2	4*	210 (350)
Chemistry	2	2	2	4*	210 (350)
Physics	2	2	2	4*	210 (350)
Mathematics	4	4	4	4	560

* Elective for students who chose one or two elective science subjects as part of the general matura examination.

BEYOND EUCLID: FRACTALS HELP STUDENTS SEE CONNECTIONS AND INCITE INTEREST IN BIOMATHEMATICS

“Western culture is obsessed with order, smoothness, and symmetry, to the point that we often impose on nature patterns and models derived from classical Greek geometry”(Kenkel and Walker, 1996; p. 77). In reality, many biological processes can be described with the use of mathematical models using traditional high school mathematics, but most of them go far beyond the boundaries of traditional high school curricula, as in the case of fractal structures in biology.

Even though the fractal nature of physical objects in nature is well documented and many biological structures follow fractal patterns, it comes as a surprise that this knowledge is mostly ignored by the creators of mathematics and science curricula in Slovenia. In the syllabi of biology, chemistry, physics, and geography, the word fractal is not mentioned; in the mathematic syllabus fractals are mentioned only once in the context that fractals could be a topic for students' individual research work in their spare time.

I have used fractals in regular classes to teach branching of trees and pulmonary and vascular systems, with no intention of going deeper into the underlying mathematical explanation of fractals. Students are quite interested in fractal patterns in nature. I share with them the words written by Michael F. Barnsley (1993, p. 3):

“Fractal geometry will make you see everything differently. There is danger in reading further. You risk the loss of your childhood vision of clouds, forests, galaxies, leaves, feathers, flowers, rocks, mountains, torrents of water, carpets, bricks, and much else besides. Never again will your interpretation of these things be quite the same.”

In 1998 I mentored an international group of secondary school students in a project week entitled Chaos and Order (www.websammlung.at/IAAC/gmunden/gmunden1.htm). We searched for fractal structures in nature and explored their properties (Figure 1). Later that year the students decided to prepare a youth research project for an annual contest for young researchers in 1999. Two 16-year-old students (Rene Suša and Jure Gojič) used the fractal dimension of leaves as an estimator of environmental damage caused by traffic. The fractal dimension was estimated using the box counting technique of leaf scans. The analysis of leaf shape using fractal geometry is described in greater detail in an article by Hartvigsen (2000). The following year, the same pair of students won a state award for their work entitled “Optimization of Space and Surface Filling”, using fractal structures, and exploring themes of interest not only in biology but in other fields of research and application (Batty, 2008; Bru et al., 2008; Kaligarič et al., 2008).

Figure 1.

Living fractal assembled by students and professors (photo Ernst Meralla).

The lesson I learned was that even 16-years-olds can grasp basic ideas such as nonlinearity or fractals. So, the failure to introduce such issues into science or mathematics teaching can be blamed only on the conservative nature of science and mathematics teaching practices and on the ignorance of curricular committees and teachers.

IT CANNOT BE PART OF THE CURRICULUM BECAUSE IT CAN BE OF USE OUTSIDE THE ACADEMIC DISCIPLINE

Computers are everywhere, even in school science laboratories, where computers equipped with data-loggers and a couple of sensors can be used to extend laboratory exercises. Their importance was recognized by the Ministry of Education and Sport of Slovenia, and all general secondary schools received donations of such equipment between 2001 and 2004. In subsequent years it was recognized that such equipment was only rarely being used in the classroom (Šorgo et al., 2010), so we can draw a similar conclusion to that of McFarlane and Sakellariou (2002) for England and Wales “that data loggers remain token rather than having found a place in routine science classes.” Even when they were used in the laboratory, their most important role was to facilitate the transition from cookbook labs to computer-supported cookbook labs.

When data-loggers are used on a “plug and play” basis, with preloaded data files, and the only thing a student has to do is to sink a sensor into something and plot a graph to be inserted into a ready-prepared report, then there is no need for additional mathematics. However, when a teacher uses computers to enhance understanding (Šorgo and Kocijančič, 2006; Šorgo et al., 2008), some knowledge about mathematics is inevitable.

One such case is analog–digital conversion. The question “Why do our graphs appear in stepped lines?” used to crop up often with earlier 8-bit analog digital converters. Nowadays, when most converters are 16 bits, this pattern is recognized mainly when students try to zoom in to the curve.

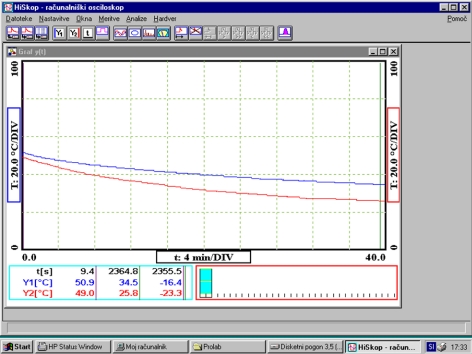
A good example is the graph (Figure 2) that was obtained as a screen capture during laboratory work on the insulation properties of hair and feathers. We can recognize the step pattern as a result of 8-bit analog-digital conversion. In such conversion, continuous measurement is transformed to (2)ⁿ discrete values. We can easily explain such a pattern to the students with conversion of our experimental plots to lower 2- and 3-bit conversion entering the mathematical domains of exponential functions and binary numbers (Šorgo and Kocijančič, 2004).

Figure 2.

Screen capture of a graph of the difference in temperature changes in a bottle insulated with feathers and a bottle without insulation, both filled with warm water.

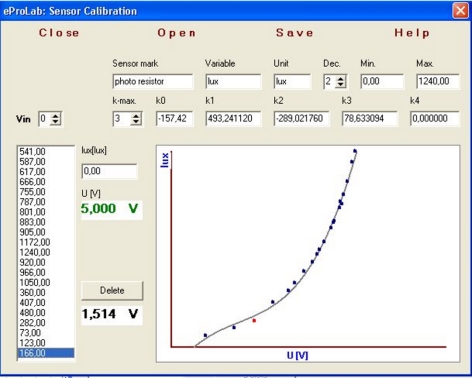
Another issue is calibration of sensors. At school students are rarely involved in calibration, especially if teachers trust automatic recognition and preloaded values. When students have to calibrate sensors, even future philosophers or kindergarten teachers must become conversant with the idea that sensors transform one or another physical or chemical quantity to voltage, and they must connect these two quantities (Figure 3). Through such work they become familiar with terms like “best-fit curve” or “outlying result” and use their mathematical knowledge about polynomials and functions (Kocijančič and Jamsek, 2004; Šorgo and Kocijančič, 2004).

Figure 3.

Calibration curve of nonlinear homemade temperature sensor using e-ProLab data acquisition system (Murovec and Kocijančič, 2004).

CONCLUSIONS AND RECOMMENDATIONS

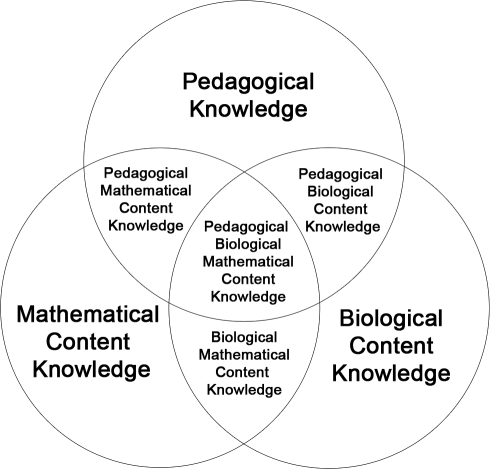
Making the first connections between biology and mathematics on the university level is an enterprise condemned to limited success or even to failure. Connecting biology and mathematics requires prepared minds to be successful. If students who take biology because they envision a science career but “don't like math,” they are preselected to ignore mathematics. Adding a mathematical subject or two taught by mathematic experts to the university biology curriculum won't work, because students will not discover the connections by themselves. Even if someone were to show them the connections, they would probably stay within the safe field of biology when discussing biological issues (Ortiz, 2006). Connecting the two sciences should therefore start as early as elementary school and continue throughout all preuniversity or precollege education. This does not mean that biology teachers should teach mathematics or mathematic teachers should teach biology. Both subjects should be taught by experts, but some overlapping zone must be found on the attractive content level and pedagogy of teaching. Mathematical–biology or biological–mathematics content knowledge for all teachers should be established. One suitable model to be reworked is the model of pedagogical content knowledge (Shulman, 1986, 1987), upgraded to technological pedagogical content knowledge by Mishra and Koehler (2006) and used in cell biology by Usak (2009). The most important part of the model to be considered is its central section named Pedagogical Biological Mathematical Content Knowledge (Figure 4).

Figure 4.

Model of pedagogical biological mathematical content knowledge.

Because teachers are the most important factor in schools (Kalin and Zuljan, 2007), introduction of any new trend should start with them. When connecting mathematics and biology, we should learn from the case of computers, where it was recognized that buying computers is the easiest part of the job (Špernjak and Šorgo, 2009). To compile a curriculum based on content from both biology and mathematics will, by analogy, be the easiest part, but the transformation of outdated teaching may require more time and effort. So the safest way to be successful in connecting these disciplines is to start thinking about professional development of pedagogical mathematical–biological content knowledge in the education of prospective elementary, biology, and mathematics teachers.