Evolution of virulence when transmission occurs before disease

Abstract

Most models of virulence evolution assume that transmission and virulence are constant during an infection. In many viral (HIV and influenza), bacterial (TB) and prion (BSE and CWD) systems, disease-induced mortality occurs long after the host becomes infectious. Therefore, we constructed a model with two infected classes that differ in transmission rate and virulence in order to understand how the evolutionarily stable strategy (ESS) depends on the relative difference in transmission and virulence between classes, on the transition rate between classes and on the recovery rate from the second class. We find that ESS virulence decreases when expressed early in the infection or when transmission occurs late in an infection. When virulence occurred relatively equally in each class and there was disease recovery, ESS virulence increased with increased transition rate. In contrast, ESS virulence first increased and then decreased with transition rate when there was little virulence early in the infection and a rapid recovery rate. This model predicts that ESS virulence is highly dependent on the timing of transmission and pathology after infection; thus, pathogen evolution may either increase or decrease virulence after emergence in a new host.

1. Introduction

The degree that parasites exploit and harm their host has been of considerable interest to theoretical biologists for many decades (Levin & Pimentel 1981; Anderson & May 1982; Bull 1994; Frank 1996; Day 2001, 2003). Most theoretical studies focus on simple but general models and postulate a ‘trade-off’ between parasite transmission rate and parasite-induced host mortality (Ebert & Bull 2003; Alizon et al. 2009). Fewer theoretical studies have considered more complex models that contain heterogeneity in the host population (Regoes et al. 2000; Gandon et al. 2003; Gandon 2004; Gandon & Day 2007) or changing ecological dynamics during the course of pathogen evolution (Lenski & May 1994; Day & Gandon 2007).

Another form of host heterogeneity that affects virulence evolution is the host's immune defence-related pathology (Day et al. 2007). Here, the heterogeneity occurs within a host during the course of an infection. After infection, the pathogen experiences a rapidly changing host environment because of the developing host immune response. Early in an infection, there may be little or no morbidity experienced by the host but there may be substantial pathogen transmission. Later in the same infection, the host immune response may begin to control pathogen density, cause pathology and potentially lower transmission. Alternatively, the pathogen may begin to destroy host tissues and compromise host function in later stages of an infection. Depending on the particular details of the pathogen transmission mechanisms, the decoupling in time between transmission and virulence may lead to selection for changes in virulence (Day et al. 2007).

In this paper, we develop a model for understanding the evolution of virulence (disease-induced mortality) when virulence and transmission change over the course of an infection. While we are motivated by a specific system where transmission occurs very quickly after infection but pathology develops more slowly, many emerging human and wildlife diseases share this pattern. For example, in house finch (Carpodacus mexicanus) infected by the bacteria Mycoplasma gallisepticum, infection causes severe conjunctivitis that peaks approximately two weeks after infection (Dhondt et al. 2008). Experimentally infected finches, however, are maximally infectious in the first few days after inoculation (Dhondt et al. 2008). Other emerging human and wildlife pathogens, such as immunodeficiency viruses (HIV), human and bovine tuberculosis (TB) and chronic wasting disease (CWD) also become infectious before severe disease symptoms are evident.

2. Methods

We incorporate changing virulence and transmission after infection by adding an additional infected host class to the typical Susceptible (S), Infected (I) and Resistant (R) model:

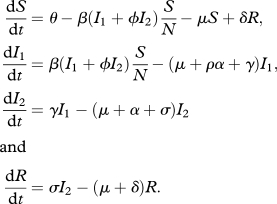I₂ is an infectious class with a later infection age as well as class-specific transmission and virulence rates that differ from those of I₁. Susceptible host inflow is at a constant rate θ, the transmission rate is β, the background mortality rate common to all host classes is μ, σ is the recovery rate and N is the total population size. The parameter γ controls the transition rate between the infected classes (host leave the initial infected class after a time period of 1/γ on average). The parameters ϕ and ρ control the relative differences between the classes in the transmission and virulence parameters, respectively, and must be greater than or equal to 0.

We conduct an evolutionary invasion analysis by first deriving the fitness of a mutant pathogen in a population of residents at endemic equilibrium (Gandon 2004). Evolutionary invasion analysis is an analytical method to understand the long-term evolutionary dynamics of a system (Otto & Day 2007). At the heart of this method is determining when a rare mutant can increase in frequency in a population of residents at equilibrium. In effect, the analysis assumes that mutations to new trait values are rare, such that the population always reaches equilibrium before the next mutation arises. Successive mutation and invasion may then lead to a point in trait space that cannot be invaded by other mutants—a ‘convergence stable’ evolutionarily stable strategy (ESS). For our epidemic model, we also assume that hosts are only infected with one strain at a time.

Following the methods of invasion analysis (Gandon 2004; Otto & Day 2007), we find that the instantaneous growth rate of a mutant (subscript m) into a population of resident (subscript r) genotypes is the dominant eigenvalue of

which is known as the invasion fitness, λ(z_m, z_r), and pathogen traits are the vector z_x = (α_x, α_x). We assume that other parameters are fixed during evolution. The equilibrium proportion of susceptible hosts for the resident population is (ignoring subscripts)

Finally, we search for the points where

These points are evolutionarily stable against invasion from mutants.

We assume that transmission and virulence are pleiotropic traits linked through pathogen exploitation in the host. Pathogen exploitation is the total or tissue-specific pathogen load of a host, and for simplicity we equate pathogen exploitation to virulence α. Next, we assume that β is made up of two components: the contact rate between individuals (π) and the probability of infection per contact (τ), which is a monotonically increasing function of pathogen virulence, τ = 1 − exp(−ψα). Here ψ is the infection hazard for each unit of pathogen exploitation (Osnas & Lively 2004; Ben-Ami et al. 2008). Therefore, the relationship between transmission and virulence becomes β(α) = π [1 − exp(−ψα)].

3. Results

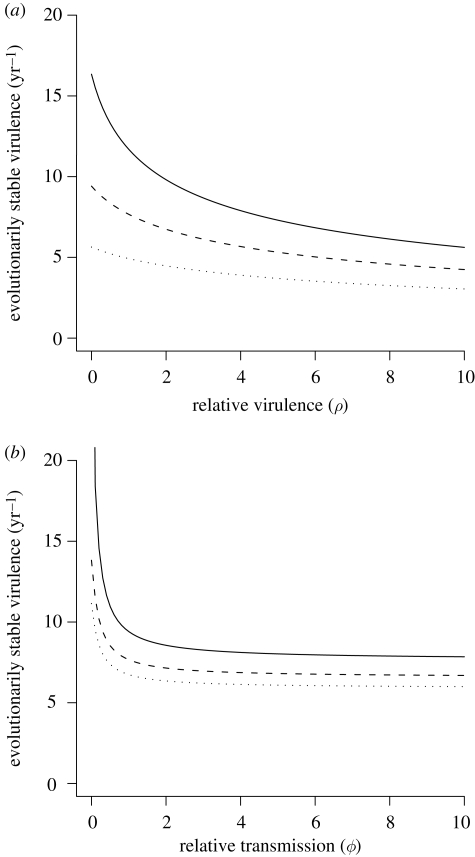
We first examine the effect of disease mortality in the I₁ class (parameter ρ). As relative disease mortality in I₁ increases, the ESS virulence decreases. The degree of decrease in ESS virulence depends on the parameter scaling virulence relative to transmission (ψ, figure 1a). As the amount of transmission increases per unit of virulence, the ESS virulence decreases. The decrease in ESS virulence is greatest when there is no disease mortality in I₁ (ρ = 0; figure 1a).

Figure 1.

(a) The relationship between evolutionarily stable virulence (α) and the relative virulence in the first infected class (ρ) for three levels of infection hazard (solid line, ψ = 0.1; dashed line, ψ = 0.2; dotted line, ψ = 0.4). Parameters are μ = 0.5, γ = 50, σ = 10 and ϕ = 1. (b) The relationship between evolutionarily stable virulence (α) and the relative transmission in the second class (ϕ) for three levels of ρ (solid line, ρ = 0.001; dashed line, ρ = 1; dotted line, ρ = 2). Here, ψ = 0.2.

The relative amount of transmission in I₂ (ϕ) also has a large effect on ESS virulence (figure 1b). As the amount of transmission in I₂ increases, the ESS virulence decreases, and the rate of decrease depends on the level of mortality that occurs in the I₁ class. As the level of transmission in I₂ and the disease mortality rate in the I₁ class (ρ) approach zero, the ESS virulence goes to infinity (figure 1b). These results can be understood by realizing that for any fixed level of virulence (α), decreases in the transmission parameter ϕ reduce the fitness benefit of reaching the second class (I₂), while increases in ρ both decrease the probability of reaching the second class and decreases the infectious period in the first class. Therefore, as both parameters reach zero, there is no benefit in reaching the second class and no cost to virulence in the first class. Thus, ESS virulence is very high and virulence will have a greater tendency to increase after introduction.

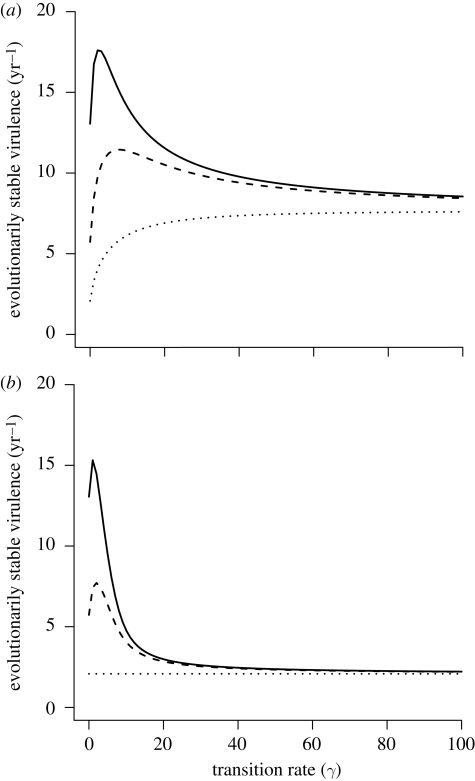
The effect of the transition rate (γ) between the classes on ESS virulence depends strongly on the relative amount of disease mortality in I₁ (ρ) and on the recovery rate (σ; figure 2). When disease mortality in the classes is similar (ρ = 1) and there is recovery from the second class (σ = 10), ESS virulence increases with increases in transition rate between the classes (figure 2a). When there is no recovery, however, there is no change in ESS virulence with transition rate (figure 2b). This result is because of the pathogen, on average, spending relatively more time in the I₁ class as γ decreases. In the limit as γ goes to zero, the pathogen spends its whole life in I₁ and has an infectious period of 1/(μ + α). Thus, pathogen virulence adapts to I₁, and reductions in virulence translate directly to a longer infectious period. As γ increases, however, the pathogen spends little time in I₁ and, in the limit, experiences an infectious period of 1/(μ + α+ σ). Thus, the pathogen adapts to the conditions of the I₂ class and to a higher virulence as long as σ > 0.

Figure 2.

The relationship between evolutionarily stable virulence (α) and the transition rate between the infected classes (γ) for three levels of relative virulence (ρ) with and without recovery (σ) from the second infected class. In (a) there is recovery (σ = 10) and in (b) there is no recovery (σ = 0). Solid line, ρ = 0.01; dashed line, ρ = 0.1; dotted line, ρ = 1. For both, ϕ = 1 and other parameters are as in figure 1.

With less disease mortality in I₁ (ρ < 1), ESS virulence first increases and then decreases as transition rate (γ) increases from zero (figure 2a,b). The degree of increase, and the inflection point where ESS virulence begins to decrease, depends on the amount of disease mortality in I₁ (ρ, figure 2). At the extremes of γ (0, ∞), the ESS virulence converges to that of a model with one infectious stage, just as described above, but here the ESS virulence is always higher for low values of ρ and γ (figure 2).

4. Discussion

When a pathogen invades a new host population, the initial virulence is a complex interaction between host and pathogen properties and may be far from an ESS. Thus, virulence may increase or decrease after pathogen emergence in the new host population. ESS virulence will be much larger, however, in systems with a delay between transmission and disease symptoms that affect mortality (Day 2003). We have also shown that host properties controlling patterns of transmission and virulence during the course of an infection can greatly affect ESS virulence. Such factors could be immune-related pathology, which we have assumed is because of a host immune response that increases with pathogen exploitation and uncouples transmission from virulence because of a delay. If the uncoupling is great enough, then there is effectively no upper limit to the ESS virulence (figure 1). When this delay is large and little disease mortality occurs early in the infection, high virulence is to be expected at the ESS virulence (figure 2). Thus it is reasonable to expect virulence to increase after the initial disease outbreak in systems where these conditions are met.

In order to fully understand our results, we derive the basic reproductive ratio of an infection according to the ‘next generation’ method (Diekmann et al. 1990), which gives the expected number of secondary infections from an initial infection,

This shows that R₀ is the sum of the R₀ for each stage separately with the second stage weighted by the fraction of infections reaching that stage. Thus, the second stage rapidly becomes more important for pathogen transmission when compared with the first stage as γ increases. That is, the R₀ of the first stage goes to 0, while the fraction that reaches the second stage goes to 1 as γ increases. For intermediate values of γ, the relative importance of each stage to R₀ will depend on the parameters ϕ, ρ and σ. All else being equal, ρ < 1 will decrease the cost of virulence in the first stage and allow for higher ESS virulence.

These predictions are relevant for many emerging wildlife and human diseases. For example, experimental infections with the bacteria Mycoplasma gallisepticum suggest that the infectiousness of house finches is high before peak eye swelling is obtained and infectiousness declines before eye swelling (Dhondt et al. 2008). Such a situation corresponds to small values of the parameters ρ and ϕ; thus, our model predicts that these are the conditions that could easily lead to a high ESS virulence (figure 1). If the delay in producing the immunopathological response is also significant (γ is small), higher levels of ESS virulence are predicted.