Abstract
How often will elections end in landslides? What is the probability for a head-to-head race? Analyzing ballot results from several large countries rather anomalous and yet unexplained distributions have been observed. We identify tactical voting as the driving ingredient for the anomalies and introduce a model to study its effect on plurality elections, characterized by the relative strength of the feedback from polls and the pairwise interaction between individuals in the society. With this model it becomes possible to explain the polarization of votes between two candidates, understand the small margin of victories frequently observed for different elections, and analyze the polls' impact in American, Canadian, and Brazilian ballots. Moreover, the model reproduces, quantitatively, the distribution of votes obtained in the Brazilian mayor elections with two, three, and four candidates.
Introduction
The outcome of elections is one of the most stunning phenomena of democracy. Sometimes one candidate wins in a landslide victory and many times two candidates compete head-to-head leaving much suspense. What is the probability of finding a certain situation? This question can be assessed through the distribution of the percentages that each candidate obtains and has in fact been monitored in several large countries with reasonably good statistics. Instead of being Gaussian as one would expect in a simple-minded application of the central limit theorem, an anomalous distribution has been observed. Different mechanisms can affect the electors' opinion formation during an electoral process [1]–[7]. Understanding the effect of such mechanisms poses interesting challenges to both political sciences [4], [8], [9] and statistical physics [10], [11]. One distinguishes two different types of elections [12]: proportional elections, where candidates become elected in proportion to their party's voting fraction [13]–[16], and plurality elections, where only the most voted candidate gets a position [17]. Besides, the latter type can occur on one single ballot or be “run-off majority” voting, with two rounds. For each type of election different mechanisms were identified [2], [12]. We focus on plurality elections with one single ballot. To describe the tactical strategies applied by electors when 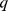 candidates compete against each other, we introduce a model where the competition between pairwise interaction with peers and polls feedback is considered.
candidates compete against each other, we introduce a model where the competition between pairwise interaction with peers and polls feedback is considered.
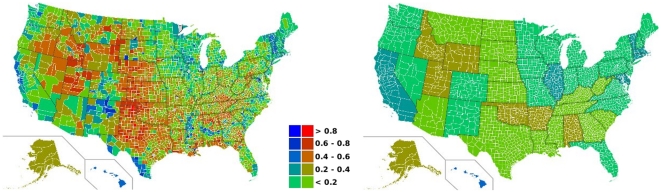
For plurality elections tactical voting as a response to information from polls is a dominant effect. Specifically, since only the most voted candidate will win, electors can change their vote to an “unwanted” candidate just to decrease the margin of victory (also known as “useful vote”). Maurice Duverger, a French sociologist, recognized this effect as responsible for the emergence of two-party systems in a statement known, today, as Duverger's law [12]. Empirical results from different electoral processes also reveal that, typically, victories occur by small margins. As an example, let us consider the 2008 American presidential elections. The maps of Fig. 1 show the difference in the fraction of votes (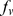 ) between Barack Obama and John McCain per county (left) and per state (right), defined as
) between Barack Obama and John McCain per county (left) and per state (right), defined as
| (1) |
where 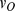 and
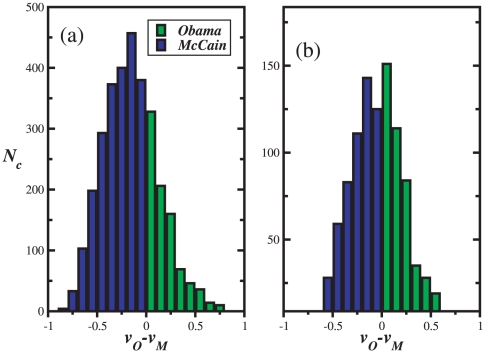
and  are, respectively, the fraction of votes for the Democratic and Republican candidates. For a more quantitative analysis, Fig. 2 contains the histograms of the differences in the fraction of votes per county, considering the entire set, Fig. 2(a), or only for the ones with more than 20 thousand electors, Fig. 2(b). We observe from these maps and histograms typical head-to-head runs. In fact, only in a few counties where the difference in the fraction of votes is slightly above
are, respectively, the fraction of votes for the Democratic and Republican candidates. For a more quantitative analysis, Fig. 2 contains the histograms of the differences in the fraction of votes per county, considering the entire set, Fig. 2(a), or only for the ones with more than 20 thousand electors, Fig. 2(b). We observe from these maps and histograms typical head-to-head runs. In fact, only in a few counties where the difference in the fraction of votes is slightly above 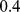 , and, at the scale of the state, only margins of victory (
, and, at the scale of the state, only margins of victory (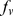 ) below
) below 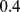 appear. From both histograms we conclude that landslides mostly occur for counties with a small number of electors.
appear. From both histograms we conclude that landslides mostly occur for counties with a small number of electors.
Figure 1. Empirical results for the 2008 American presidential elections.
Maps of the relative difference of votes, 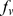 , in each county (left) and each state (right) showing typical head-to-head runs. Each color represents an interval of
, in each county (left) and each state (right) showing typical head-to-head runs. Each color represents an interval of 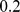 . Blue refers to a landslide victory of Barack Obama and red to a victory of John McCain. Green corresponds to a head-to-head run.
. Blue refers to a landslide victory of Barack Obama and red to a victory of John McCain. Green corresponds to a head-to-head run.
Figure 2. Histogram of the relative difference of votes for the 2008 American presidential elections in each county.
Landslides mostly occur for counties with small number of electors. For histogram (a) all counties have been considered and for (b) we have only taken counties with more than 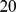 thousand electors.
thousand electors.
Recently, Restrepo et al.
[18] proposed a population dynamics model to study the effect of polls released during the electoral process. They considered two different scenarios: head-to-head and landslide voting. In the former, the margin between the first and second candidate is so tight that individuals tend to stick their vote to their favorite candidate. In the latter, the leading candidate has a large margin with respect to all the others, which increases the tendency towards tactical voting. To study the effect of the “useful vote” on the ballots, we propose a model where a vote results from the competition between two mechanisms: the tendency to align with peers (herding) and the “useful vote” [19]. The first one, corresponds to an attractive interaction between somewhat linked electors and solely depends on their opinion. The second mechanism, however, depends on the polls and should be proportional to the difference between the fraction of votes of the leading candidate (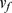 ) and the second one (
) and the second one (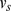 ). The larger the difference, the stronger the tendency towards tactical voting. Let us consider
). The larger the difference, the stronger the tendency towards tactical voting. Let us consider 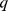 candidates identified by an index
candidates identified by an index 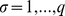 . We propose a balance function
. We propose a balance function 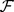 to quantify the degree of indecision in the society due to the coexistence of different opinions, defined as
to quantify the degree of indecision in the society due to the coexistence of different opinions, defined as
| (2) |
where 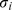 is the candidate for which citizen
is the candidate for which citizen  votes and
votes and 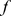 is the index of the leading candidate (Note that,
is the index of the leading candidate (Note that, 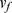 can only assume values ranging between
can only assume values ranging between 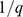 and unity.). The delta function
and unity.). The delta function 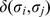 is unity when
is unity when  and
and 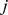 chose the same candidate and zero otherwise. The herding coefficient
chose the same candidate and zero otherwise. The herding coefficient 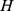 measures the strength of interaction between connected individuals and
measures the strength of interaction between connected individuals and  the polls impact. The natural tendency in society is to minimize its degree of indecision. Together with the principle of maximum entropy, this leads to a probability distribution of each state
the polls impact. The natural tendency in society is to minimize its degree of indecision. Together with the principle of maximum entropy, this leads to a probability distribution of each state 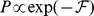 . Curiously, in the absence of polls (
. Curiously, in the absence of polls (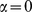 ), our model boils down to the so-called
), our model boils down to the so-called 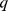 -state “Potts model” used in magnetism [20]. For
-state “Potts model” used in magnetism [20]. For 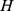 above
above 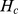 a stable majority opinion can arise, while below it, the interaction between individuals is insufficient to lead to a fixed majority. The system is then controlled by the competition between the relative strength of the polls impact,
a stable majority opinion can arise, while below it, the interaction between individuals is insufficient to lead to a fixed majority. The system is then controlled by the competition between the relative strength of the polls impact, 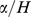 , and its degree of subcriticality,
, and its degree of subcriticality, 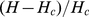 , given by the ratio
, given by the ratio 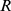 ,
,
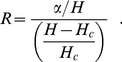 |
(3) |
The numerator measures the tendency toward tactical voting in the system, the greater 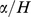 , the stronger the response to polls. This mechanism competes with the emergence of consensus due to pairwise relationships. The strength of the psychological coupling between individuals within a society is represented in the model by the social degree of subcriticality. Therefore, in our model, for
, the stronger the response to polls. This mechanism competes with the emergence of consensus due to pairwise relationships. The strength of the psychological coupling between individuals within a society is represented in the model by the social degree of subcriticality. Therefore, in our model, for 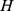 above
above 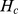 , in the limit of
, in the limit of  , when electors decide regardless of the global opinion and only are affected by their peers, rapidly a clear majority appears. With increasing
, when electors decide regardless of the global opinion and only are affected by their peers, rapidly a clear majority appears. With increasing 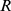 , the tendency towards tactical voting becomes more relevant and the margins of victory diminish. In the limit of large
, the tendency towards tactical voting becomes more relevant and the margins of victory diminish. In the limit of large 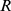 , the winners' fraction of votes is slightly above
, the winners' fraction of votes is slightly above 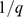 , i.e., no clear majority evinces. For simplicity we consider a square lattice where each node is an elector and solely interacts with its nearest neighbors. In fact, as we discuss later, societies are typically better described by small-world networks where long-range connections are also present [11].
, i.e., no clear majority evinces. For simplicity we consider a square lattice where each node is an elector and solely interacts with its nearest neighbors. In fact, as we discuss later, societies are typically better described by small-world networks where long-range connections are also present [11].
Results and Discussion
To verify the model, let us first consider Brazilian mayor elections since, for such elections detailed data is available [21] for the number of candidates for each election as well as their percentage of votes. Besides, ballot is compulsory and the number of cities and electors large (more than 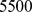 cities and
cities and 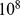 electors). Since results are resilient over the three elections (2000, 2004, and 2008), we consider, unless otherwise stated, the average over them.
electors). Since results are resilient over the three elections (2000, 2004, and 2008), we consider, unless otherwise stated, the average over them.
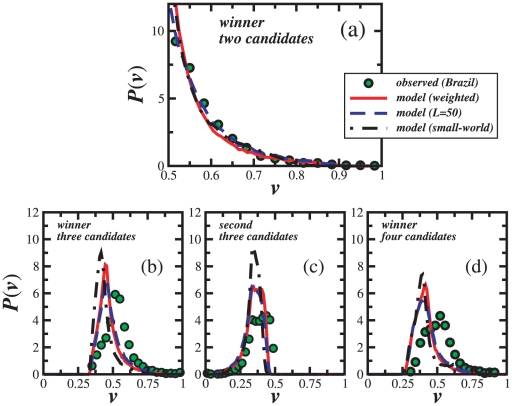
Figure 3(a) shows the winners' distribution of votes in the mayor elections with two candidates. We use these results to characterize the society in terms of the ratio 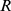 , of Eq. (3). Fitting the simulational results (
, of Eq. (3). Fitting the simulational results (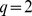 ) to the empirical ones, we obtain
) to the empirical ones, we obtain 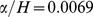 and
and 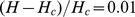 , corresponding to a ratio
, corresponding to a ratio 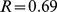 . Since the competition involves only two candidates, the winner's percentage of votes is always above
. Since the competition involves only two candidates, the winner's percentage of votes is always above 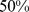 . In fact, most of the winners have a fraction of votes below
. In fact, most of the winners have a fraction of votes below 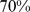 , in agreement with the predicted small margins of victory. The real distribution is characterized by an exponential tail [17] which is also obtained with our model. Additionally the model shows that the asymmetry of the distribution increases with
, in agreement with the predicted small margins of victory. The real distribution is characterized by an exponential tail [17] which is also obtained with our model. Additionally the model shows that the asymmetry of the distribution increases with  .
.
Figure 3. Model comparison with empirical results for Brazilian mayor elections.
Winners' distribution of votes (green) for elections with: a) two, b) three, and d) four candidates. Due to the resilience of the 2000, 2004, and 2008 results, we average over these three elections to improve statistics. The red-solid lines are the same distributions obtained with the model for 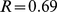 , by considering a weighted average over three system sizes, namely,
, by considering a weighted average over three system sizes, namely, 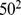 ,
, 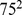 , and
, and 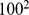 electors. The blue-dashed lines correspond to the square lattice with
electors. The blue-dashed lines correspond to the square lattice with 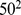 electors. The black-dashed-dotted lines are obtained on the small-world topology with
electors. The black-dashed-dotted lines are obtained on the small-world topology with 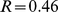 . The second candidate's distributions for the elections with three candidates are included as well (c). All results are averages over
. The second candidate's distributions for the elections with three candidates are included as well (c). All results are averages over 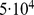 samples.
samples.
Let us consider now the limit of large 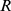 , i.e., when the strength of the feedback field is much larger than the interaction with peers. In this limit the function from Eq. (2) can be simplified as,
, i.e., when the strength of the feedback field is much larger than the interaction with peers. In this limit the function from Eq. (2) can be simplified as,
| (4) |
using the equality 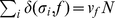 , where
, where 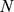 is the number of individuals in the system. The distribution of the winner's fraction of votes in this limit is then
is the number of individuals in the system. The distribution of the winner's fraction of votes in this limit is then
| (5) |
For the special case of two candidates (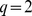 ), since
), since 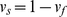 , Eq. (5) simplifies as
, Eq. (5) simplifies as 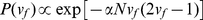 . The resulting dominance of the exponential tail is a trademark of all distributions which has also been pointed out in empirical investigations [17].
. The resulting dominance of the exponential tail is a trademark of all distributions which has also been pointed out in empirical investigations [17].
Since both the degree of subcriticality and tactical voting impact characterize the society, they should be independent on the number of candidates. In Fig. 3(b) we also see the winners' distribution of the fraction of votes for elections with three candidates. Results for the candidates ranked as second are included as well, Fig. 3(c). Since three candidates are considered, the first candidate has always more than 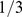 of the total number of votes and the second and third less than
of the total number of votes and the second and third less than 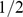 and
and 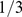 , respectively. For the winner, a maximum close to
, respectively. For the winner, a maximum close to 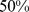 is obtained and low margins of victory are observed. As for elections with two candidates, more than
is obtained and low margins of victory are observed. As for elections with two candidates, more than 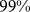 of the winners obtain less than
of the winners obtain less than 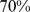 of the total votes. The red-solid lines represent the simulations for
of the total votes. The red-solid lines represent the simulations for 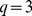 using the parameters obtained from elections with two candidates. A good quantitative agreement between simulational and empirical results is obtained for both candidates (first and second). Following Duverger's law, a polarization between two candidates is observed. Also in Fig. 3(d), we show results for elections with four candidates. Once again, with the same parameters of the two-candidates elections, our model is able to reproduce the empirical results.
using the parameters obtained from elections with two candidates. A good quantitative agreement between simulational and empirical results is obtained for both candidates (first and second). Following Duverger's law, a polarization between two candidates is observed. Also in Fig. 3(d), we show results for elections with four candidates. Once again, with the same parameters of the two-candidates elections, our model is able to reproduce the empirical results.
As referred before a square lattice is not very realistic due to the lack of long-range connections. In real systems, individuals can contact each other beyond their neighborhood (e.g., by phone, e-mail, virtual-social networks…). To account for such a type of connections we also consider a small-world network [22]. To generate the graph we start with a square lattice and randomly add long-range links until 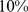 of the individuals have five bonds. As seen from the black-dashed-dotted line in Fig. 3, it is again possible to quantitatively reproduce the empirical distributions for all considered numbers of candidates with a single value of
of the individuals have five bonds. As seen from the black-dashed-dotted line in Fig. 3, it is again possible to quantitatively reproduce the empirical distributions for all considered numbers of candidates with a single value of 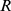 , namely,
, namely, 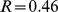 . Different values of
. Different values of 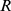 were obtained for the small-world and regular networks. Yet, for each type of topology, when the same value is considered for elections with different number of candidates, the main features of the distribution of votes are recovered. The real topologies of interest for opinion dynamics (for example, friendship) are typically small world without scale-free properties [23]. For scale-free networks, with an exponent of the degree distribution
were obtained for the small-world and regular networks. Yet, for each type of topology, when the same value is considered for elections with different number of candidates, the main features of the distribution of votes are recovered. The real topologies of interest for opinion dynamics (for example, friendship) are typically small world without scale-free properties [23]. For scale-free networks, with an exponent of the degree distribution 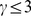 , models of opinion dynamics, as well as magnetic ones, are characterized by a clear dominance of an opinion over the other, due to the presence of highly connected nodes [11], [24]–[26]. This is not observed in the empirical results which is consistent with the assumption that topologies underlying opinion interactions are not scale free.
, models of opinion dynamics, as well as magnetic ones, are characterized by a clear dominance of an opinion over the other, due to the presence of highly connected nodes [11], [24]–[26]. This is not observed in the empirical results which is consistent with the assumption that topologies underlying opinion interactions are not scale free.
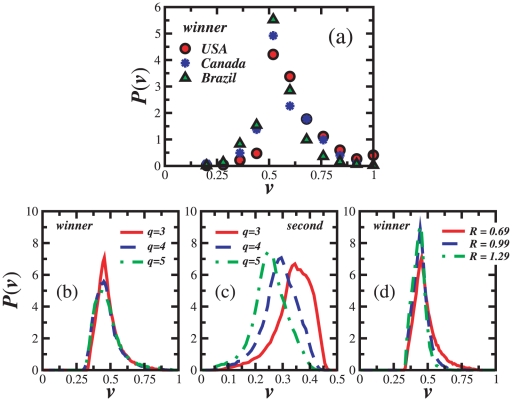
Let us now consider the results from mayor elections in the United States (2008) [27] and Canada (2008) [28]. In Fig. 4(a) we show the winners' distribution of votes for these elections together with the ones from Brazil [17], [21]. Regardless of the number of candidates, a maximum is clearly observed for a fraction of votes around 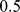 , with small margins of victory. For most countries the available results are not organized by the number of candidates, therefore, to compare the impact of the feedback field in different countries, it is useful to analyze how the distribution changes with the number of candidates in our model. In Fig. 4(b) we show this dependence by considering elections with three, four, and five candidates. We use always the same value of the relative strength of the feedback field,
, with small margins of victory. For most countries the available results are not organized by the number of candidates, therefore, to compare the impact of the feedback field in different countries, it is useful to analyze how the distribution changes with the number of candidates in our model. In Fig. 4(b) we show this dependence by considering elections with three, four, and five candidates. We use always the same value of the relative strength of the feedback field, 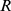 . With increasing
. With increasing 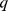 a shift of the peak to lower values occurs, which is also observed in the empirical results for three and four candidates in Ref. [17]. To compare the tails of the distributions we remove this shift (
a shift of the peak to lower values occurs, which is also observed in the empirical results for three and four candidates in Ref. [17]. To compare the tails of the distributions we remove this shift (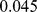 and
and 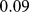 for four and five candidates, respectively). We observe that the right-hand side tail of all distributions are the same. Besides, a polarization between the first two candidates is observed for all values of
for four and five candidates, respectively). We observe that the right-hand side tail of all distributions are the same. Besides, a polarization between the first two candidates is observed for all values of 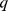 in the inset of Fig. 4(c).
in the inset of Fig. 4(c).
Figure 4. Comparison between different systems.
(a) Distribution of the winners' fraction of votes for mayor elections in different countries, namely, United States 2008 (red circles) [27], Canada 2008 (blue stars) [28], and Brazil (green triangles) [17], [21] where, for the latter one, results have been averaged over three different years 2000, 2004, and 2008, to improve statistics. (b) Distribution of the winners' fraction of votes for different number of candidates, namely, three (red-solid line), four (blue-dashed line), and five (green-dotted line). Observed shift with increasing number of candidates has been removed for four and five candidates. (c) Distributions for the candidate ranked second. (d) Distribution of the winners' fraction of votes for different values of relative strength of the feedback field, for 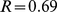 (red-solid line),
(red-solid line), 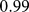 (blue-dashed line), and
(blue-dashed line), and 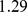 (green-dashed-dotted line). All simulational results have been averaged over
(green-dashed-dotted line). All simulational results have been averaged over 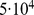 samples on a square lattice with
samples on a square lattice with 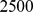 electors.
electors.
To understand how the right-hand side tail is affected by the ratio 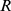 , we show in Fig. 4(d) the distributions for different values of
, we show in Fig. 4(d) the distributions for different values of 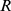 (
(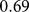 ,
, 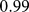 , and
, and 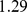 ). The larger the strength of the feedback field,
). The larger the strength of the feedback field, 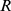 , the steeper the right-hand side of the distribution. A larger
, the steeper the right-hand side of the distribution. A larger 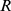 can be achieved either through a decrease in the degree of subcriticality or an increase in the impact of the feedback field. Therefore we can still draw conclusions about the relative strength of the feedback field, through the right-hand side of the distributions for different countries, regardless of the number of candidates. From Fig. 4(a) we observe that the impact of the polls in United States and Canada are very similar. Yet, in Brazil the impact reveals to be even stronger.
can be achieved either through a decrease in the degree of subcriticality or an increase in the impact of the feedback field. Therefore we can still draw conclusions about the relative strength of the feedback field, through the right-hand side of the distributions for different countries, regardless of the number of candidates. From Fig. 4(a) we observe that the impact of the polls in United States and Canada are very similar. Yet, in Brazil the impact reveals to be even stronger.
Summarizing, to understand plurality elections, we put forward a model where the relevant parameter corresponds to the ratio between the strength of polls impact and society's degree of subcriticality. With the model we have been able to compare the distributions from three large countries, namely, United States, Canada, and Brazil. The analysis reveals a similar impact of the polls on the elections in United States and Canada and an even stronger one in Brazil. Using available results for mayor elections with two candidates in Brazil, we have been able to parametrize the set of electors. With the obtained parameters we reproduce the distribution of the fraction of votes in elections with three and four candidates. The model has been implemented on a square lattice and a small-world network and, despite the differences discussed previously, the main features of the model do not depend on these topologies, e.g., for both systems, the small margins of victories as well as the polarization between the first two candidates (Duverger's law) are quantitatively reproduced. At this stage, our model does not account for blank or null votes, notwithstanding, that such votes shall not affect the candidates' relative fraction of votes. Our approach opens up the possibility to make statistical predictions about the outcome of elections by determining the new characteristic parameter 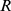 defined as the ratio between polls feedback and herding. The next challenge would be to determine this factor
defined as the ratio between polls feedback and herding. The next challenge would be to determine this factor 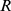 from independent controlled experiments as, for instance, through Internet surveys or questionnaires in schools or neighborhoods.
from independent controlled experiments as, for instance, through Internet surveys or questionnaires in schools or neighborhoods.
Methods
We have performed Monte Carlo simulations on the square lattice, for three different system sizes, namely, 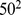 ,
, 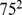 , and
, and 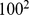 electors, and on the small-world network with
electors, and on the small-world network with 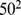 electors. All results have been averaged over
electors. All results have been averaged over 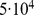 samples.
samples.
Footnotes
Competing Interests: The authors have declared that no competing interests exist.
Funding: Funding was received from Competence Center for Coping with Crises in Complex Socio-Economic Systems, ETH (Swiss Federal Institute of Technology) Grant 01-08-2, and Swiss National Science Foundation Grant 200021_126853. Additional funding was provided by Brazilian agencies CNPq (Conselho Nacional de Desenvolvimento Científico e Tecnológico), CAPES (Coordenação de Aperfeiçoamento de Pessoal de Nível Superior) and FUNCAP (Fundação Cearense de Apoio ao Desenvolvimento Científico), and the National Institute of Science and Technology for Complex Systems. The funders had no role in study design, data collection and analysis, decision to publish, or preparation of the manuscript.
References
- 1.Mobilia M. Does a single zealot affect an infinite group of voters? Phys Rev Lett. 2003;91:028701. doi: 10.1103/PhysRevLett.91.028701. [DOI] [PubMed] [Google Scholar]
- 2.Galam S. Contrarian deterministic effects on opinion dynamics: “the Hung elections scenario”. Physica A. 2004;333:453–460. [Google Scholar]
- 3.Todorov A, Mandisodza AN, Goren A, Hall CC. Inferences of competence from faces predict election outcomes. Science. 2005;308:1623–1626. doi: 10.1126/science.1110589. [DOI] [PubMed] [Google Scholar]
- 4.Geys B. Explaining voter turnout: a review of aggregate-level research. Elect Stud. 2006;25:637–663. [Google Scholar]
- 5.Castellano C, Muñoz MA, Pastor-Satorras R. Nonlinear q-voter model. Phys Rev E. 2009;80:041129. doi: 10.1103/PhysRevE.80.041129. [DOI] [PubMed] [Google Scholar]
- 6.Cipra B. Can mathematics map the way toward less-bizarre elections? Science. 2009;323:874. doi: 10.1126/science.323.5916.874a. [DOI] [PubMed] [Google Scholar]
- 7.Antonakis J, Dalgas O. Predicting elections: child's play! Science. 2009;323:1183. doi: 10.1126/science.1167748. [DOI] [PubMed] [Google Scholar]
- 8.Brams SJ, Herschbach DR. The science of elections. Science. 2001;292:1449. doi: 10.1126/science.292.5521.1449. [DOI] [PubMed] [Google Scholar]
- 9.Buchanan M. How strategists design the perfect candidate? Science. 2004;306:799–800. doi: 10.1126/science.306.5697.799. [DOI] [PubMed] [Google Scholar]
- 10.Sznajd-Weron K, Sznajd J. Opinion evolution in closed community. Int J Mod Phys C. 2000;11:1157–1165. [Google Scholar]
- 11.Castellano C, Fortunato S, Loreto V. Statistical physics of social dynamics. Rev Mod Phys. 2009;81:591–646. [Google Scholar]
- 12.Riker WH. The two-party system and Duverger's law: an essay on the history of political science. The American Political Science Review. 1982;76:753–766. [Google Scholar]
- 13.Costa Filho RN, Almeida MP, Andrade JS, Moreira JE. Scaling behavior in a proportional voting process. Phys Rev E. 1999;60:1067–1068. doi: 10.1103/physreve.60.1067. [DOI] [PubMed] [Google Scholar]
- 14.Costa Filho RN, Almeida MP, Moreira JE, Andrade JS. Brazilian elections: voting for a scaling democracy. Physica A. 2003;322:698–700. [Google Scholar]
- 15.Bernardes AT, Stauffer D, Kertész J. Election results and the Sznajd model on Barabasi network. Eur Phys J B. 2002;25:123–127. [Google Scholar]
- 16.Fortunato S, Castellano C. Scaling and universality in proportional elections. Phys Rev Lett. 2007;99:138701. doi: 10.1103/PhysRevLett.99.138701. [DOI] [PubMed] [Google Scholar]
- 17.Araripe LE, Costa Filho RN, Herrmann HJ, Andrade JS. Plurality voting: the statistical laws of democracy in Brazil. Int J Mod Phys C. 2006;17:1809–1813. [Google Scholar]
- 18.Restrepo JM, Rael RC, Hyman JM. Modeling the influence of polls on elections: a population dynamics approach. Public Choice. 2009;140:395–420. [Google Scholar]
- 19.Pattie C, Johnston R. People who talk together vote together. Ann Assoc Am Geogr. 2000;90:41–66. [Google Scholar]
- 20.Wu FY. The Potts model. Rev Mod Phys. 1982;54:235–268. [Google Scholar]
- 21.Electoral results for Brazilian mayor elections. Available: http://www.tse.gov.br/. Accessed 2010.
- 22.Sánchez AD, López JM, Rodríguez MA. Nonequilibrium phase transitions in directed small-world networks. Phys Rev Lett. 2002;88:048701. doi: 10.1103/PhysRevLett.88.048701. [DOI] [PubMed] [Google Scholar]
- 23.González MC, Herrmann HJ, Kertész J, Vicsek T. Community structure and ethnic preferences in school friendship networks. Physica A. 2007;379:307–316. [Google Scholar]
- 24.Andrade JS, Herrmann HJ, Andrade RFS, da Silva LR. Apollonian networks: simultaneously scale-free, small world, euclidean, space filling, and with matching graphs. Phys Rev Lett. 2005;94:018702. doi: 10.1103/PhysRevLett.94.018702. [DOI] [PubMed] [Google Scholar]
- 25.Andrade RFS, Herrmann HJ. Magnetic models on Apollonian networks. Phys Rev E. 2005;71:056131. doi: 10.1103/PhysRevE.71.056131. [DOI] [PubMed] [Google Scholar]
- 26.Andrade RFS, Andrade JS, Herrmann HJ. Ising model on the Apollonian network with node-dependent interactions. Phys Rev E. 2009;79:036105. doi: 10.1103/PhysRevE.79.036105. [DOI] [PubMed] [Google Scholar]
- 27. Electoral results for American mayor elections (accessed 2010). http://usmayors.org/elections/
- 28. Electoral results for Canadian mayor elections (accessed 2010). http://www.civicinfo.bc.ca/