Skip to main content
PMC home page
Annals of Botany logoLink to Annals of Botany
. 2010 Jul 19;106(4):647–652. doi: 10.1093/aob/mcq145

Effects of positive interactions, size symmetry of competition and abiotic stress on self-thinning in simulated plant populations

Cheng-Jin Chu 1, Jacob Weiner 2, Fernando T Maestre 3, You-Shi Wang 4, Charles Morris 5, Sa Xiao 1, Jian-Li Yuan 1, Guo-Zhen Du 1, Gang Wang 1,*
PMCID: PMC2944970  PMID: 20643802

Abstract

Background and Aims

Competition drives self-thinning (density-dependent mortality) in crowded plant populations. Facilitative interactions have been shown to affect many processes in plant populations and communities, but their effects on self-thinning trajectories have not been investigated.

Methods

Using an individual-based ‘zone-of-influence’ model, we studied the potential effects of the size symmetry of competition, abiotic stress and facilitation on self-thinning trajectories in plant monocultures. In the model, abiotic stress reduced the growth of all individuals and facilitation ameliorated the effects of stress on interacting individuals.

Key Results

Abiotic stress made the log biomass – log density relationship during self-thinning steeper, but this effect was reduced by positive interactions among individuals. Size-asymmetric competition also influenced the self-thinning slope.

Conclusions

Although competition drives self-thinning, its course can be affected by abiotic stress, facilitation and competitive symmetry.

Keywords: Density-dependent mortality, positive interactions, self-thinning, size symmetry competition, abiotic stress, zone of influence model, ZOI

INTRODUCTION

Self-thinning (density-dependent mortality) in plant populations has been a widely investigated phenomenon. It is usually modelled as w = kρβ, an allometric relationship between mean surviving plant biomass (w) and plant density (ρ), where k and β are the self-thinning coefficient and exponent, respectively. Although there is broad consensus concerning this general pattern, there has been much debate about the values and universality of the parameters, especially β (White et al., 2007). Self-thinning is driven by resource limitation: because the resources within a given area are finite, some individuals must die if others are to grow as a crowded plant stand develops when all resources are being consumed (Morris, 2002, 2003).

However, not all plant–plant interactions are competitive. Studies over the last 20 years have shown that positive interactions are common in plant communities in physically harsh environments, through ameliorating locally stressful conditions such as increasing temperature (Callaway, 2007; Pakeman et al., 2009; Pugnaire, 2010). In these ecosystems, competition and facilitation interact and influence the structure of populations and communities (Michalet et al., 2006; Wang et al., 2008; Chu et al., 2009a, b). Recent research has demonstrated that abiotic stress associated with positive interactions can delay the onset of extensive density-dependent mortality and increase the size inequality of populations undergoing density-dependent mortality (Chu et al., 2009a). However, the potential effects of facilitation on self-thinning trajectories have not, to our knowledge, been studied. Many different methods have been implemented to explore the effects of various factors on self-thinning process, either experimentally or theoretically (e.g. Weller, 1987; Morris, 2002, 2003; Larjavaara, 2010). In the present paper, we use a spatially explicit model to investigate the role of facilitation, competition and abiotic stress on self-thinning in simulated plant populations.

MATERIALS AND METHODS

The model

The model employed here has been described previously (Chu et al., 2008, 2009a). It is based on Weiner et al.'s (2001) ‘zone-of-influence’ (ZOI) model. In the ZOI model, the potential resources available for an individual without neighbours are reflected by the area of a circular zone, A, related to the plant's biomass, B, as A = cB2/3 (we set c = 1·0 here), and neighbouring plants compete for the resources when their areas overlap. The realized growth rate of the plant considering competition is described by the equation:

graphic file with name mcq145eqnU1.jpg 1

where Bmax is the maximum (asymptotic) plant mass, r is the initial (maximum) growth rate (in units of mass area−1 time−1), and Ac is the effective area of a plant (Schwinning and Weiner, 1998; Weiner et al., 2001; Weiner and Damgaard, 2006).

Abiotic stress can be included in the model with a parameter s ranging from 0 (no stress) to 1 (maximum stress). It decreases the growth rate of all plants in a simple linear fashion (Molofsky and Bever, 2002). Based on our current understanding of facilitation (Callaway, 2007), we assume that the linear relationship between facilitation and abiotic stress only occurs when the stress factor is not itself a resource such as temperature (Maestre et al., 2009). We assume that facilitation experienced by a plant is a function of the total of all areas of overlap with neighbouring plants, Af, such as increasing the local temperature in an alpine region (Callaway, 2007). The realized growth rate of the plant growing under stress and with facilitation is modelled as

graphic file with name mcq145eqnU2.jpg 2

Here we consider three stress levels: s = 0·0, s = 0·4 and s = 0·8. The above formula reflects the fact that competition and facilitation usually act simultaneously in nature (Callaway, 2007).

To study self-thinning, we assume that individuals die if their actual growth rate over a time interval falls below 1 % of their current biomass (Weiner et al., 2001; Stoll et al., 2002). This threshold is necessary if self-thinning is to occur under size-symmetric competition; without such a threshold all plants stop growing and there is no mortality. We simulated a relatively high initial population density (7225 individuals m−2). We also implemented simulations for other density levels, and the results were similar to those presented here.

To explicitly explore the potential effects of facilitation on self-thinning lines, we set Af = 0·0 in eqn (2), giving the trajectory of populations in harsh conditions without positive interactions between neighbours. In this case, the realized growth rate becomes

graphic file with name mcq145eqnU3.jpg 3

To explore the effect of the size symmetry of competition on the behaviour of the model, we consider four types of size symmetry, which are defined by the parameter p. These represent different ways of dividing areas of overlap among competing individuals in determining a plant's effective area (Ac): p = 0·0 for complete symmetry (overlapping areas divided equally among all overlapping individuals), p = 0·5 for partial size symmetry (uptake of the overlapped area increases with size, but less than proportionally), p = 1·0 for perfect size symmetry (overlapping areas are divided in proportion to the sizes of the overlapping individuals) and p = 10·0 for a high degree of size asymmetry (the largest individuals obtain almost all the overlapped area; Weiner et al., 2001). To explicitly incorporate the effect of p, we formulate an equation for n individuals dividing the overlapping resource with area Ao:

graphic file with name mcq145eqnU4.jpg 4

where Aoi refers to the amount of resource taken by plant i in the neighbourhood of j (Schwinning and Weiner, 1998; Weiner and Damgaard, 2006). Thus for plant i, the effective area Ac is equal to Ano + 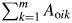Inline graphic where Ano denotes the area not overlapping with other plants and m the number of overlapping regions (for an example see Supplementary Data, available online).

The simulations were stochastic, and the initial parameter settings were the same as in Chu et al. (2009a). We took a ‘wraparound’ (torus) approach to avoid edge effects (Grimm and Railsback, 2005). Individuals were distributed randomly in space. All simulations were conducted and replicated ten times using landscapes with a size of 100 × 100 grids in NetLogo (Wilensky, 1999).

Statistical analyses

To fit the self-thinning equation, the simulated data points of populations undergoing extensive mortality were selected a posteriori (Weller, 1987). Due to the dependence between population density and mean individual mass, we used the bootstrapping method to explore the relationships between them. After obtaining the slopes for the log mean mass vs. log density relationships for ten replicates given a parameter setting, we calculated the means (n = 10) and 95 % confidence intervals. Regression and bootstrapping (with replacement sampling and a total of 2000 replications) analyses were conducted using R 2·9·1 (R Development Core Team, 2009).

RESULTS

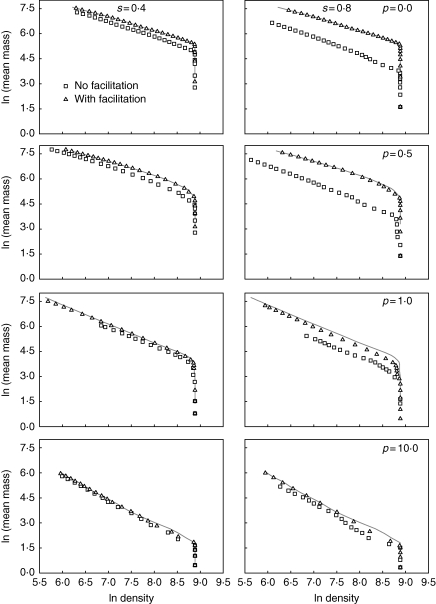
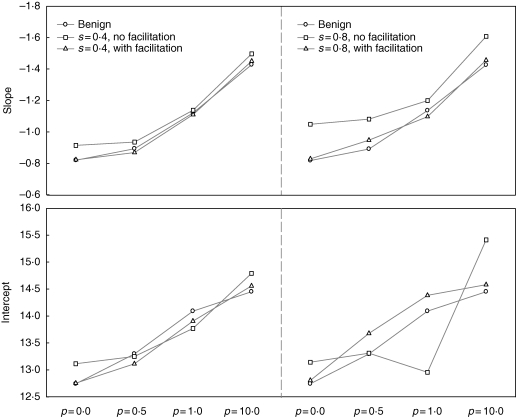
Abiotic stress itself lowered the position of the thinning trajectory: there was less biomass at a given density under conditions of abiotic stress (Fig. 1). Both abiotic stress and size-asymmetric competition made the self-thinning slopes steeper (Table 1, Fig. 2). The inclusion of positive interactions ameliorated the effects of stress on the allometric slopes and significantly affected the thinning intercepts (Table 1, Figs 1 and 2). Ninety-five per cent confidence intervals of slopes and intercepts for populations in harsh conditions with facilitation did not overlap with those of the corresponding populations without facilitation.

Fig. 1.

Fig. 1.

Open in a new tab

Self-thinning trajectories for simulated populations (7225 individuals) with and without facilitation under harsh conditions. s defines the level of stress (0–1), and p defines the size symmetry of competition (0: completely symmetric; 0·5: partially size symmetric; 1·0: perfectly size-symmetric; 10·0: highly size-asymmetric). For comparison, results for benign conditions (s = 0·0) are shown as grey lines on each figure.

Table 1.

Bootstrapping means and 95 % confidence intervals of self-thinning slopes of the relationship between survivor densities and mean individual mass (both log-transformed; n = 10)

Slope
 Intercept
p s F? Mean 95 % CI Mean 95 % CI
0 0·0 n –0·8204 –0·8212 to –0·8196 12·743 12·738–12·749
0·5 0·0 n –0·8934 –0·8989 to –0·8879 13·296 13·258–13·335
1·0 0·0 n –1·1381 –1·1428 to –1·1333 14·090 14·059–14·122
10·0 0·0 n –1·4278 –1·4305 to –1·4251 14·451 14·433–14·469
0 0·4 n –0·9148 –0·9158 to –0·9138 13·115 13·108–13·122
0 0·4 y –0·8228 –0·8232 to –0·8225 12·754 12·751–12·757
0 0·8 n –1·0514 –1·0526 to –1·0502 13·143 13·134–13·151
0 0·8 y –0·8309 –0·8315 to –0·8304 12·806 12·802–12·810
0·5 0·4 n –0·9354 –0·9384 to –0·9323 13·249 13·229–13·268
0·5 0·4 y –0·8685 –0·8721 to –0·8648 13·113 13·087–13·139
0·5 0·8 n –1·0838 –1·0844 to –1·0832 13·311 13·640–13·716
0·5 0·8 y –0·9497 –0·9550 to –0·9443 13·678 13·307–13·315
1·0 0·4 n –1·1216 –1·1264 to –1·1167 13·769 13·880–13·929
1·0 0·4 y –1·1106 –1·1136 to –1·1077 13·905 13·731–13·870
1·0 0·8 n –1·2019 –1·2037 to –1·2001 12·958 13·368–13·397
1·0 0·8 y –1·0996 –1·1013 to –1·0978 14·383 12·943–12·972
10·0 0·4 n –1·4970 –1·4984 to –1·4955 14·790 14·779–14·801
10·0 0·4 y –1·4503 –1·4517 to –1·4489 14·558 14·548–14·568
10·0 0·8 n –1·6095 –1·6117 to –1·6074 15·412 15·395–15·428
10·0 0·8 y –1·4585 –1·4602 to –1·4567 14·580 14·567–14·593
Open in a new tab

s defines the level of stress (0–1), and p defines the size symmetry of competition (0: completely symmetric; 0·5: partially size-symmetric; 1·0: perfectly size-symmetric; 10·0: highly size-asymmetric). F? indicates whether the case includes facilitation.

Fig. 2.

Fig. 2.

Open in a new tab

Comparisons of self-thinning slopes (upper panels) and intercepts (lower panels) of the relationships between survivor densities and mean individual mass in simulated populations. s defines the level of stress (0–1), and p defines the size symmetry of competition (0: completely symmetric; 0·5: partially size-symmetric; 1·0: perfectly size-symmetric; 10·0: highly size-asymmetric).

DISCUSSION

In our model, abiotic stress by itself resulted in steeper (more negative) and lower self-thinning trajectories. When competition was highly size-asymmetric, its effects overwhelmed those of abiotic stress. Stress is modelled in eqn (2) as a factor reducing the growth of all plants, which should give the common self-thinning line result. A common self-thinning line has been observed in some experimental cases where the reduction in growth has been imposed by resource limitation (reviewed in Morris, 2003). However, there have been other numerous cases where growth reduction achieved by resource limitation has resulted in different self-thinning lines, for example for shade (Westoby and Howell, 1981; Lonsdale and Watkinson, 1982), nutrients (Morris, 2002, 2003) and water (Deng et al., 2006). In some of these cases, resource limitation was shown to alter the way plants occupied canopy space (Lonsdale and Watkinson, 1982; Morris, 2003); in eqn (2), this would mean that Ac would vary with resource level, possibly leading to different results from those shown here.

The inclusion of facilitation reduced or eliminated the effect of harsh conditions on the allometric slope. Abiotic stress reduces the growth rate of individuals in our model, while facilitation from neighbours could keep an individual's growth rate above the threshold for mortality (1 % here) and postpone the onset of death. The self-thinning slope for spring wheat Triticum aestivum in water-stressed conditions was flatter than in well-watered conditions (−1·35 vs. −1·49; Liu et al., 2006), but the difference was not statistically significant. The self-thinning slope (based on tree stem diameter and density) under semi-arid conditions was higher than expected from existing models of allometric plant growth (−0·25 vs. −0·33). In our model, the allometric slopes for populations in harsh environments without facilitation were steeper than those under benign conditions (Figs 1 and 2, Table 1). Water limitation may alter the size-symmetry of competition as well as the level of stress.

The degree of size asymmetry of competition also has pronounced effects on the self-thinning lines; a steeper slope occurs under more size-asymmetric competition (Figs 1 and 2, Table 1). Stoll et al. (2002) found a flatter self-thinning slope with more asymmetric competition, the opposite of the result obtained here. The main difference is that we excluded dead individuals from our analyses (Weiner et al., 2001), whereas Stoll et al. (2002) included them in the total population biomass.

While the differences in thinning slopes we found for populations under benign conditions and those under harsh conditions with facilitation are statistically significant, they are relatively small. It will be difficult to identify and disentangle the effects of positive interactions in empirical studies because some patterns generated by interactions between competition and facilitation can be very similar to those generated by competition alone (Michalet et al., 2006). Explicit tests are needed to ask whether the patterns predicted by our model are of biological relevance under real conditions. To explore whether abiotic stress and facilitation make self-thinning slopes steeper, experiments can be conducted to compare the patterns of populations with similar plant density along natural or imposed (e.g. through warming) abiotic stress gradients. There have also been attempts to experimentally change the degree of size asymmetry in competition without affecting other aspects of competition. There is evidence that genetically modified plants that do not show ‘shade avoidance’ behaviour compete more size asymmetrically than normal plants (Ballaré et al., 1994; Stoll et al., 2002). Increased soil heterogeneity appears to make competition more size asymmetric in some (e.g. Maestre and Reynolds, 2006) but not other (Casper and Cahill, 1998) cases. The degree of size asymmetry can also be measured (Schwinning and Weiner, 1998), offering the possibility of correlative tests of some of the predictions of our model.

Many researchers have questioned the existence of a universal thinning exponent (White et al., 2007; Isaac and Carbone, 2010). There is evidence that many factors, such as the mode of competition and nutrient limitation, can influence the thinning slope (e.g. Stoll et al., 2002; Deng et al., 2006). Our results show that facilitation among individuals could be another factor that influences the thinning exponents and suggest that positive interactions, size symmetry competition and abiotic conditions interact to influence the self-thinning trajectory of plant populations.

SUPPLEMENTARY DATA

Supplementary data are available online at www.aob.oxfordjournals.org and provide an example of the generic presentation of the way to divide the areas of overlap among competing individuals in determining a plant's effective area (eqn 4 in the text).

Supplementary Material

Supplementary Data
supp_106_4_647__index.html (954B, html)

ACKNOWLEDGEMENTS

We thank two anonymous referees and Professor Bill Shipley for valuable comments on a previous version of this manuscript. The study was supported by the National Natural Science Foundation of China (30770360, 40721061), the Fundamental Research Funds for the Central Universities (lzujbky-2009-88) and the Youth Innovation Research Fund for interdisciplinary studies of Lanzhou University (LZUJC200915). F.T.M. acknowledges support from the European Research Council under the European Community's Seventh Framework Programme (FP7/2007-2013)/ERC Grant agreement no. 242658.

LITERATURE CITED

  1. Ballaré CL, Scopel AL, Jordan ET, Vierstra RD. Signaling among neighboring plants and the development of size inequalities in plant populations. Proceedings of the National Academy of Science USA. 1994;91:10094–10098. doi: 10.1073/pnas.91.21.10094. [DOI] [PMC free article] [PubMed] [Google Scholar]
  2. Callaway RW. Positive interactions and interdependence in plant communities. Dordrecht: Springer; 2007. [Google Scholar]
  3. Casper BB, Cahill JF., Jr. Population-level responses to nutrient heterogeneity and density by Abutilon theophrasti (Malvaceae): an experimental neighborhood approach. American Journal of Botany. 1998;85:1680–1687. [PubMed] [Google Scholar]
  4. Chu CJ, Maestre FT, Xiao S, et al. Balance between facilitation and resource competition determines biomass–density relationships in plant populations. Ecology Letters. 2008;11:1189–1197. doi: 10.1111/j.1461-0248.2008.01228.x. [DOI] [PubMed] [Google Scholar]
  5. Chu CJ, Weiner J, Maestre FT, et al. Positive interactions can increase size inequality in plant populations. Journal of Ecology. 2009a;97:1401–1407. [Google Scholar]
  6. Chu CJ, Wang YS, Li Q, et al. Effects of traits, species identity and local environmental conditions on the assessment of interactions: insights from an alpine meadow community. Journal of Plant Ecology. 2009b;2:135–141. [Google Scholar]
  7. Deng JM, Wang GX, Morris EC, et al. Plant mass–density relationship along a moisture gradient in north-west China. Journal of Ecology. 2006;94:953–958. [Google Scholar]
  8. Grimm V, Railsback SF. Individual-based modeling and ecology. Princeton, NJ: Princeton University Press; 2005. [Google Scholar]
  9. Isaac NJB, Carbone C. Why are metabolic scaling exponents so controversial? Quantifying variance and testing hypotheses. Ecology Letters. 2010;13:728–735. doi: 10.1111/j.1461-0248.2010.01461.x. [DOI] [PubMed] [Google Scholar]
  10. Larjavaara M. Maintenance cost, toppling risk and size of trees in a self-thinning stand. Journal of Theoretical Biology. 2010;265:63–67. doi: 10.1016/j.jtbi.2010.04.021. [DOI] [PubMed] [Google Scholar]
  11. Liu J, Wei L, Wang CM, Wang GX, Wei XP. Effect of water deficit on self-thinning line in spring wheat (Triticum aestivum L.) populations. Journal of Integrative Plant Biology. 2006;48:415–419. [Google Scholar]
  12. Lonsdale WM, Watkinson AR. Light and self-thinning. New Phytologist. 1982;90:431–445. [Google Scholar]
  13. Maestre FT, Reynolds JF. Nutrient availability and atmospheric CO2 partial pressure modulate the effects of nutrient heterogeneity on the size structure of populations in grassland species. Annals of Botany. 2006;98:227–235. doi: 10.1093/aob/mcl093. [DOI] [PMC free article] [PubMed] [Google Scholar]
  14. Maestre FT, Callaway RM, Valladares F, Lortie CJ. Refining the stress-gradient hypothesis for competition and facilitation in plant communities. Journal of Ecology. 2009;97:199–205. [Google Scholar]
  15. Michalet R, Brooker RW, Cavieres LA, et al. Do biotic interactions shape both sides of the humped-back model of species richness in plant communities? Ecology Letters. 2006;9:767–773. doi: 10.1111/j.1461-0248.2006.00935.x. [DOI] [PubMed] [Google Scholar]
  16. Molofsky J, Bever JD. A novel theory to explain species diversity in landscapes: positive frequency dependence and habitat suitability. Proceedings of the Royal Society of London – Biological Sciences. 2002;269:2389–2393. doi: 10.1098/rspb.2002.2164. [DOI] [PMC free article] [PubMed] [Google Scholar]
  17. Morris EC. Self-thinning lines differ with fertility level. Ecological Research. 2002;17:17–28. [Google Scholar]
  18. Morris EC. How does fertility of the substrate affect intraspecific competition? Evidence and synthesis from self-thinning. Ecological Research. 2003;18:287–305. [Google Scholar]
  19. Pakeman RJ, Pugnaire FI, Michalet R, et al. Is the cask of facilitation ready for bottling? A symposium on the connectivity and future directions of positive plant interactions. Biology Letters. 2009;5:577–579. doi: 10.1098/rsbl.2009.0384. [DOI] [PMC free article] [PubMed] [Google Scholar]
  20. Pugnaire FI, editor. Positive plant interactions and community dynamics. Boca Raton, FL: CRC Press; 2010. [Google Scholar]
  21. R Development Core Team. R: A language and environment for statistical computing. Vienna: R Foundation for Statistical Computing; 2009. http://www.R-project.org . [Google Scholar]
  22. Schwinning S, Weiner J. Mechanisms determining the degree of size asymmetry in competition among plants. Oecologia. 1998;113:447–455. doi: 10.1007/s004420050397. [DOI] [PubMed] [Google Scholar]
  23. Stoll P, Weiner J, Muller-Landau H, Müller E, Hara T. Size symmetry of competition alters biomass–density relationships. Proceedings of the Royal Society of London – Biological Sciences. 2002;269:2191–2195. doi: 10.1098/rspb.2002.2137. [DOI] [PMC free article] [PubMed] [Google Scholar]
  24. Sumanta B. Relationship between size hierarchy and density of trees in a tropical dry forest of western India. Journal of Vegetation Science. 2007;18:389–394. [Google Scholar]
  25. Wang YS, Chu CJ, Maestre FT, Wang G. On the relevance of facilitation in alpine meadow communities: an experimental assessment with multiple species differing in their ecological optimum. Acta Oecologica. 2008;33:108–113. [Google Scholar]
  26. Weiner J. How competition for light and nutrients affects size variability in Ipomoea tricolor populations. Ecology. 1986;67:1425–1427. [Google Scholar]
  27. Weiner J, Damgaard C. Size-asymmetric competition and size-asymmetric growth in a spatially explicit zone-of-influence model of plant competition. Ecological Research. 2006;21:707–712. [Google Scholar]
  28. Weiner J, Stoll P, Muller-Landau H, Jasentuliyana A. The effects of density, spatial pattern and competitive symmetry on size variation in simulated plant population. American Naturalist. 2001;158:438–450. doi: 10.1086/321988. [DOI] [PubMed] [Google Scholar]
  29. Weller DE. A reevaluation of the –3/2 power rule of plant self-thinning. Ecological Monographs. 1987;57:23–43. [Google Scholar]
  30. Westoby M, Howell J. Self-thinning: the effect of shading on glasshouse populations of silver beet (Beta vulgaris) Journal of Ecology. 1981;69:359–365. [Google Scholar]
  31. White CR, Cassey P, Blackburn TM. Allometric exponents do not support a universal metabolic allometry. Ecology. 2007;88:315–323. doi: 10.1890/05-1883. [DOI] [PubMed] [Google Scholar]
  32. Wilensky U. NetLogo. 1999 http://ccl.northwestern.edu/netlogo/ . Center for Connected Learning and Computer-Based Modeling, Northwestern University. [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Data
supp_106_4_647__index.html (954B, html)
supp_mcq145_mcq145supp.pdf (42.1KB, pdf)

Articles from Annals of Botany are provided here courtesy of Oxford University Press

RESOURCES