Abstract
Most of the high-order aberrations of the eye are not circularly symmetric. Hence, while it is well known that human vision is subject to cortically based orientation preference in cell tuning, the optics of the eye might also introduce some orientational anisotropy. We tested this idea by measuring contrast sensitivity at different orientations of sine-wave gratings when viewing through a closed-loop adaptive optics phoropter. Under aberration-corrected conditions, mean contrast sensitivity improved for all observers by a factor of 1.8× to 5×. The detectability of some orientations improved more than others. As expected, this orientation-specific effect varied between individuals. The sensitivity benefits were accurately predicted from MTF model simulations, demonstrating that the observed effects reflected the individual's pattern of high-order aberrations. In one observer, the orientation-specific effects were substantial: an improvement of 8× at one orientation and 2× in another orientation. The experiments confirm that, for conditions that are not diffraction limited, the optics of the eye introduce rotational asymmetry to the luminance distribution on the retina and that this impacts vision, inducing orientational anisotropy. These results suggest that the traditional view of meridional anisotropy having an entirely neural origin may be true for diffraction-limited pupils but that viewing through larger pupils introduces an additional orientation-specific optical component to this phenomenon.
Keywords: contrast sensitivity, spatial vision, higher order aberrations, adaptive optics
Introduction
Increasing the pupil size of the eye reduces the effect of diffraction but increases the ocular aberrations arising from the small decentrations, tilts, and other irregularities that are present in any biological optical system. In humans, diffraction and high-order (HO) aberrations are balanced for optimum optical performance for a pupil diameter of slightly less than 3.0 mm (Campbell & Gubisch, 1966). While second-order defocus and astigmatism are readily corrected with ophthalmic lenses, correcting HO aberrations is more complex. The relatively recent development of techniques to measure and correct the HO aberrations (Liang, Williams, & Miller, 1997; Smirnov, 1962) has raised the possibility of exploring the true spatial limits of human vision by increasing pupil size but at the same time retaining a high-quality retinal image. While this is feasible using interference fringes that can eliminate distortion associated with both diffraction and aberrations, images composed of incoherent light are more representative of everyday vision. Controlling HO aberrations allows the possibility of exploring their effects on vision by simulating the appearance of objects with and without aberrations (e.g., Applegate, Ballentine, Gross, Sarver, & Sarver, 2003), but questions regarding the benefits of totally eliminating aberrations persist (Nio et al., 2002). Indeed, it would be surprising if the presence of chromatic and monochromatic aberrations was not utilized in some way or another by the visual system, as has been shown to be the case for accommodation (Chen, Kruger, Hofer, Singer, & Williams, 2006; Chin, Hampson, & Mallen, 2009; Kruger, Mathews, Aggarwala, & Sanchez, 1993).
For theoretical reasons (Artal, Santamaria, & Bescos, 1988; Hopkins, 1955), the starting point for assessing the benefits of correcting HO aberrations should be the effect on the visibility of sinusoidal gratings. The expected improvement in the quality of the retinal image can be calculated from the Optical Transfer Function, D(R, ψ):
| (1) |
where R is the spatial frequency, ψ is the grating orientation, T(R, ψ) is the modulation transfer function (MTF) that shows the spatial frequency-dependent attenuation between an object and its image, and θ(R, ψ) is the phase transfer function (PTF). It indicates the lateral shift of an image and in the case of a single frequency stimulus such as a grating has minimal effect on visibility (but see Walsh & Charman, 1989). It follows from Equation 1 that if the bars of a grating have a particular orientation ψ, then the MTF can be expected to vary with grating orientation if the eye does not possess rotational symmetry. Though there are some exceptions (Artal, 1990; Walsh & Charman, 1992), the influence of orientation on the MTF and the PTF has been largely neglected. In fact, it can be shown theoretically that the MTF for an eye that suffers from moderate degrees of HO aberrations may be markedly different at different orientations for a particular spatial frequency. Of course, this applies particularly when a relatively high proportion of non-symmetrical aberrations such as coma are present, and studies of large populations have shown the presence of varying amounts of such aberrations in human eyes (Porter, Guirao, Cox, & Williams, 2001; Thibos, Hong, Bradley, & Cheng, 2002).
Liang et al. (1997) and Yoon and Williams (2002) have reported experiments illustrating the improvement in contrast thresholds when using adaptive optics (AO) to correct the aberrations of the eye. Comparisons made between AO-corrected and non-corrected conditions showed a benefit of a factor of approximately 3× in contrast sensitivity averaged across two observers and spatial frequencies of 16 and 24 c/deg. Elliott et al. (2009) used an AO system to measure the effects of minimizing ocular aberrations in older and younger eyes. They reported data from a larger number of observers (n = 20) and found an improvement in sensitivity of similar magnitude with AO correction, but the improvement was dependent on pupil size. Most studies used 6-mm diameter or larger pupils, and as shown by Dalimier, Dainty, and Barbur (2008), pupil size is important when assessing the functional benefits of correcting HO aberrations.
In the present paper, we describe experiments that extend the observations of previous work by assessing the effects of grating orientation when compensating the HO aberrations in a contrast sensitivity experiment. Others, such as Yoon and Williams (2002), avoided the issue of orientation by measuring horizontal gratings and basing their calculations on the corresponding MTF. There were, however, substantial variations in the extent to which contrast sensitivity was improved for their observers when aberrations were corrected. It is therefore possible that these effects may be due to the different composition of aberrations in different eyes.
There is little doubt that grating orientation is theoretically important when measuring contrast sensitivity. The question addressed here is whether the effects are large enough to be of practical importance. From model eye simulations, Tahir et al. (2008) showed that contrast sensitivity at different orientations should change when the pupil is increased due to the presence of the non-circularly symmetric aberrations. Their corresponding measurements, comparing contrast sensitivity at different orientations through small (3 mm) and large (>6 mm) pupils, were consistent with the theory. This is evidence that ocular aberrations can selectively affect the visibility of different orientations. Murray et al. (2008) made similar observations in patients who had undergone refractive surgery. This procedure induces substantial changes in the HO aberrations. They recommended that orientation be taken into account when using gratings to assess contrast sensitivity following refractive surgery because of the confounding effects of orientation.
It is important to recognize that even with a diffraction-limited system, sensitivity, determined psychophysically, is not independent of orientation. Many classical psychophysical studies have shown a strong preference for vertical and horizontal lines or gratings, compared with those at oblique axes. This phenomenon was described by Appelle (1972) as the oblique effect. Performance on a wide variety of visual tasks was described as superior in the cardinal compared with the oblique axes in humans and in behavioral measures in many species. In his comprehensive review, Appelle reports many experiments supporting the role of ocular optics in the oblique effect. This idea was tested by Campbell, Kulikowski, and Levinson (1966) who bypassed the optics of the eye using interference fringes and compared measurements when viewing through relatively small pupils (2.8 mm). The fact that strong meridional effects remained under these conditions and that stimuli aligned with the cardinal axes were most easily detected was taken as strong evidence that orientational anisotropy should be attributed to anatomical mechanisms, probably in visual cortex. As reported in the famous physiological experiments of Hubel and Wiesel (1959), analysis of orientation is first seen in the primary visual cortex, and Furmanski and Engel (2000) and Kamitani and Tong (2005) have published more contemporary descriptions of the phenomenon, based on functional Magnetic Resonance Imaging (fMRI). These studies showed the cardinal axes are overrepresented in visual cortex. Psychophysical experiments suggest that the diminished neural representation of oblique stimuli arises in the human cortex, rather than from impairments of sensitivity or resolution in the initial geniculo-cortical projection (Heeley, Buchanan-Smith, Cromwell, & Wright, 1997; McMahon & MacLeod, 2003). All these studies point to a neural basis for the oblique effect.
It is crucial to point out, however, that in using small pupils Campbell et al. (1966) did not eliminate the possibility that optical factors may contribute to orientation selectivity in addition to the neural factors. As shown by Tahir et al. (2008), optical modeling indicates strong orientation anisotropies in the MTF in the presence of moderate levels of ocular aberrations. As stated above, it has also been reported in a series of papers (Tahir, Parry, Brahma, Ikram, & Murray, 2009; Tahir, Parry, Pallikaris, & Murray, 2009) that the ocular optics can selectively influence the detectability of different orientations.
In this paper, we describe experiments designed to test the idea that optical effects introduce meridional-specific changes in contrast sensitivity. Thresholds were measured at different orientations using an AO setup, comparing normal with AO-corrected conditions. Thus we are able to measure the effects of the aberrations on the detectability of different orientations directly rather than implicitly as in Tahir et al. (2008). The objectives of the experiments were given as follows; first, we wished to confirm the expected theoretical effects of correcting the HO aberrations. The comparison between theoretical and empirical observations is important because it provides insight as to whether the substantial predicted effects might be reflected in the psychophysical measurements or whether the visual system may mask them in some way, such as perhaps by long-term adaptation (Artal et al., 2004; Mon-Williams, Treslian, Strang, Kochkar, & Wann, 1998). Second, previous studies have found substantial differences in the extent to which different observers benefit from having their HO aberrations corrected. This may be linked to the measurements being confined to only a single orientation, or it may be that different observers' mix of aberrations influences how their performance is improved when HO aberrations are corrected. If this is the case, we should expect the detectability of some orientations to benefit more than others from compensation of the HO aberrations, compelling evidence that the ocular optics contribute to the well-known meridional anisotropy called the oblique effect.
Methods
Stimuli
Sinusoidal gratings were generated on a gamma-corrected, custom-built 25-cm monochrome CRT display (Moraine Displays). The background had CIE 1931 coordinates x = 0.358, y = 0.546 and was broadband with λmax = 550 nm. It was driven by a Macintosh G4 with 10-bit color resolution. Mean screen luminance was 50 cd/m2 (at the plane of the eye pupil). Gratings were sinusoidally reversing at 1 Hz and presented as Gabor patches of diameter 1.5° to confine the stimulus to the isoplanatic patch of retina.
Wavefront sensing and correction
The AO setup has been described in detail by Choi et al. (2006) and its use in psychophysical testing was described by Elliott et al. (2009). Briefly, wavefront aberrations were measured using a Shack–Hartmann wavefront sensor (WFS) with a 20 × 20 lenslet array, across a 6-mm pupil. A superluminescent diode (SLD) operating at 835 ± 20 nm was used to form a wavefront sensor beacon on the retina. High-order aberrations were corrected with a 68-mm, 109 actuator, continuous surface deformable mirror (DM, Litton ITEK) that has an approximate mirror stroke of ±2 μm. Direct slope control was used for the individual DM actuators. The wavefront was sampled at 20 Hz, yielding a closed-loop bandwidth of ~0.9 Hz and a gain of 30%. A −0.75 D trial lens was placed at a pupil plane in the non-common path to correct for wavelength difference of focus between the SLD used for wavefront sensing and the CRT. This calculation was based on the new reduced-eye model (Thibos, Ye, Zhang, & Bradley, 1992). All aberrations are specified according to the OSA convention (Thibos, Applegate, Schwiegerling, Webb, & VSIA Standards Taskforce Members, 2000).
Optical modeling
The method used for optical modeling is given in detail in Tahir, Parry, Pallikaris et al. (2009). In brief, the MTF was derived using a Matlab (Version 7.4, The Mathworks) routine. The model assumed a reduced schematic eye with refracting power of 60 D and refractive index of 1.32. A set of Zernike coefficients for a particular wavefront aberration was calculated to determine a pupil function. The squared modulus of the pupil function was used to construct the point spread function (PSF). The Fourier transform of this gave the optical transfer function (OTF) and the modulus of this, the MTF for a particular orientation and spatial frequency of a grating. The MTF when expressed in polar coordinates as r and θ gives the particular spatial frequency and orientation, respectively, as in Equation 1.
The Stiles–Crawford effect (Stiles & Crawford, 1933) is incorporated within the modeling as an apodizing neutral density filter. For this, a standard ρ factor value of 0.12 was used (Applegate & Lakshminarayanan, 1993).
The aberrations used for each subject were taken as the mean of 25 samples (frames) recorded during the contrast sensitivity testing for each particular condition (with and without AO for each orientation of grating) with a 6-mm pupil. All aberration data shown or used for simulations are based on a 6-mm pupil.
Psychophysical methods
Monocular contrast thresholds were obtained with the 12 c/deg grating presented at either eight orientations at 22.5° intervals (1 observer) or at four orientations (45, 90, 135, and 180 deg, 3 observers). For one additional observer, 16 c/deg gratings were tested at eight orientations. Pupils were dilated using a combination of 1% Tropicamide and 2.5% Phenylephrine instilled twice, 30 min and 20 min before the experiments started. Orientations were randomly presented and each was measured once with and once without AO compensation. Refractive correction was carefully obtained to within ±0.12 DS and ±0.25 DC. Lenses were located in a trial lens holder close to the observer's eye. A bite bar was used to stabilize head movements. A version of Quest (Watson & Pelli, 1983), a Bayesian adaptive two-alternative forced-choice technique, was used to determine contrast thresholds. Experimental software was written in MATLAB v. 5.2.1 using the psychophysical toolbox extensions (Brainard, 1997; Pelli, 1997).
In order to ensure optimal subjective refraction, the visibility of a Landolt C generated on the display was optimized using spherical lenses located in a pupil plane in the non-common path. Fixation was centered using the wavefront sensor camera, and this was monitored and adjusted as required throughout the experiment. All the subjects obtained better than 6/6 visual acuity. The AO correction was over 6.8 mm of the pupil and the stimulus was viewed through a 6-mm artificial pupil. All pupils were dilated above 7 mm.
Note that we have used a dB scale for sensitivity
| (2) |
where S = sensitivity expressed in dB and C = threshold contrast. To calculate the improvement in contrast thresholds or “visual benefit” as in Elliott et al. (2009) and Yoon and Williams (2002), the following expression was used:
| (3) |
where S2 = sensitivity with AO and S1 = sensitivity without AO.
For the optical modeling, the theoretical visual benefit (TS′) was calculated using the following expression:
| (4) |
where MTF2 = MTF calculated with residual aberrations measured when aberrations were corrected with AO and MTF1 = MTF calculated with habitual HO aberrations present.
Following the correction of the lower order aberrations with refractive lenses, some residual second-order aberrations still remained. These were included in our analysis in both the modeling and in calculations of the equivalent defocus. Similar to Thibos et al. (2002), equivalent defocus was calculated as the sum of the residual second-order aberration present and the equivalent defocus of the HO aberration terms (third to sixth order) calculated using
| (5) |
where R = pupil radius and RMSE is the root mean square error of the second- to sixth-order aberrations.
Observers
Five observers were tested. Observer ages ranged between 18 and 35 years old (mean age 24 ± 6.6 years, 2 males). None of the subjects had any ocular pathology as established by an optometrist and/or ophthalmologist, and all were either emmetropic or were slightly myopic (<−1.25D) with normal VA (6/6 or better). All observers had prior experience in psychophysical testing. Two were measured at eight orientations and three at four orientations.
Written informed consent was obtained following the Tenets of Helsinki and with approval of the Institutional Review Board of the University of California, Davis, School of Medicine.
Results
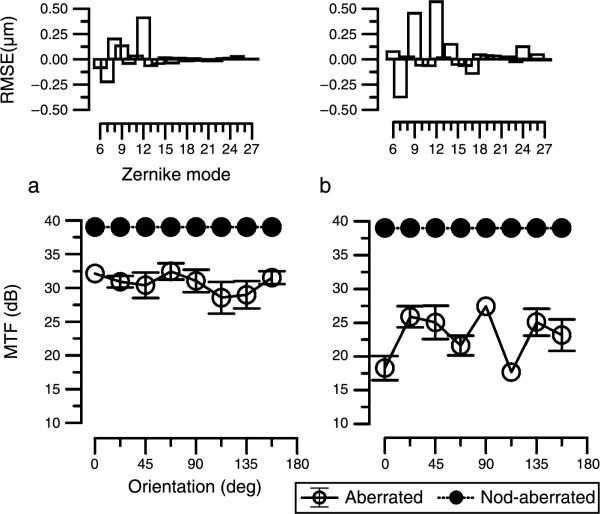
In Figures 1a and 1b, we illustrate how individual variations in wavefront aberrations influence the calculated MTF at different orientations for a pupil size of 6 mm and a 12 c/deg sinusoidal grating. For the aberration-free condition (closed circles), the MTF is independent of orientation. However, when aberrations are introduced (in the example illustrated, the HO aberrations of two of the authors are used and their respective Zernike aberrations are shown in the panels above the MTF data in Figure 1), the MTF exhibits considerable asymmetry with orientation and the effects are evidently different for the two sets of aberrations. For one observer (Figure 1a, IJM, right eye), the MTF has small undulations as a function of orientation whereas in the other (Figure 1b, HJT left eye), the HO aberrations induce substantial differences between orientations. As these data are based on the optics alone, the effects are due to the differences in the mix of aberrations between the two observers. Note for example that in Figure 1b there is a difference in sensitivity of around 9.5 dB (a factor of ~3) between 0° and 90° for this observer's aberrations.
Figure 1.
Two examples of calculated modulation transfer functions (MTF) for a 6-mm pupil, either with no aberrations (filled circles) or calculated using the aberrations of a 6-mm pupil of two of the authors (open circles, (a) and (b)). The aberration data for each subject are shown in the top panel for the third- to sixth-order Zernike modes (in μm). The MTF was calculated for 4 separate measurements of aberrations and error bars indicate the standard deviation. Note that the MTF for subject HJT is based on the aberrations of the right eye.
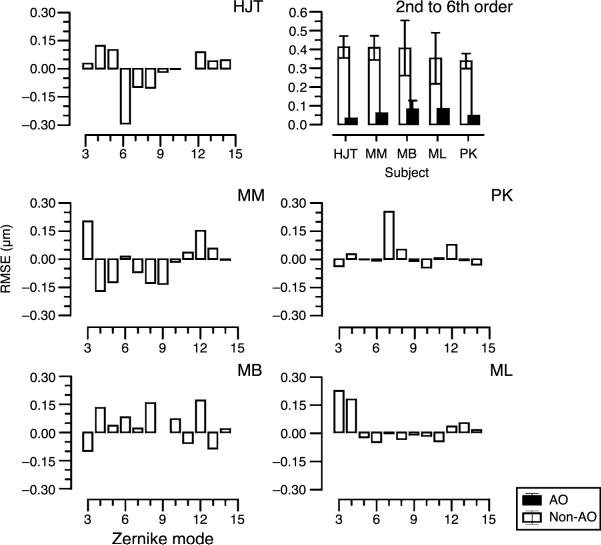
In Figure 2, we present the non-AO-corrected aberrations for second- to fourth-order Zernike aberrations for five observers. In each case, the horizontal axis depicts the Zernike mode from 3 to 15. Note that modes 3, 4, and 5 are the low-order aberrations, astigmatism, and sphere blur, 6 and 9 are trefoil, and 7 and 8 are coma. Zernike mode 12 is primary spherical aberration. Most errors occur in modes 3, 4, and 5. The top right panel shows the AO-corrected (solid bars) versus the uncorrected RMSE for each observer (second- to sixth-order Zernike aberrations). In these calculations, the second-order Zernike aberrations (lower order astigmatism and spherical defocus) are the residual error remaining after the best refractive correction used in the contrast sensitivity measurements. This shows that high-order aberrations were reduced to less than 0.1 μm during AO correction as for previous reports. Uncorrected RMSE for all subjects are similar (between 0.34 and 0.41 μm), but as seen in the individual panels, the distribution of the aberrations is quite different for each subject.
Figure 2.
Aberrations (μm) for each subject (top left, middle, and bottom panels) for the second to fourth Zernike orders (6-mm pupil). Second-order aberrations are shown as the residual error remaining after best refractive correction. Top right panel shows total root mean square error (RMSE) of the second- to sixth-order aberrations for each subject without AO correction (open bars) and with AO correction (closed bars). Error bars indicate ±1 standard deviation.
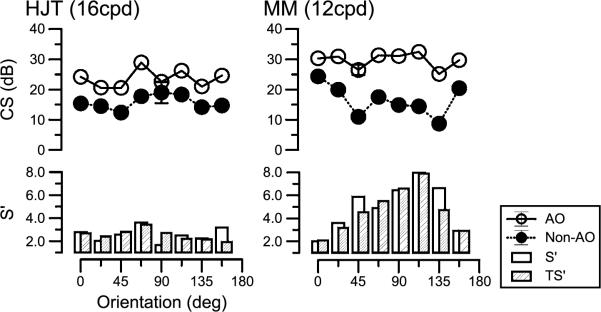
Figure 3 illustrates the link between thresholds at different grating orientations and the calculated theoretical improvement in two subjects at eight orientations. Here we present data for two spatial frequencies, 16 c/deg for HJT (left eye) and 12 c/deg for MM (right eye). Contrast sensitivity is plotted for each of the 8 orientations, with open symbols depicting AO-corrected measurements and closed symbols, without AO-corrected measurements. It is clear that there are improvements in sensitivity across all orientations when aberrations are corrected. It is also clear that some orientations are improved more than others, as shown previously (Tahir et al., 2008). For example, for subject MM at 112.5° the improvement in performance is of the order of 8× whereas at 180° around the horizontal meridian, improvement is around 2×. In the two lower panels, we illustrate S′, the measured improvement in contrast sensitivity (open bars) compared with the theoretical (hatched bars) improvement, TS′. Mean improvement (S′) across orientations for HJT was 2.56×, and for MM, it was 4.7×. With the exception of 157° for HJT and 135° for MM, the actual improvement in sensitivity closely matched those calculated according to theory.
Figure 3.
Orientation-selective contrast sensitivity (dB) for two subjects measured at eight orientations for both AO-corrected measurements (open symbols) and non-corrected measurements (closed symbols). Measured and calculated theoretical benefits, S′ and TS′, shown in bar graph below. Error bars indicate ±standard deviation. Note that the psychophysical measurements for subject HJT are for the left eye.
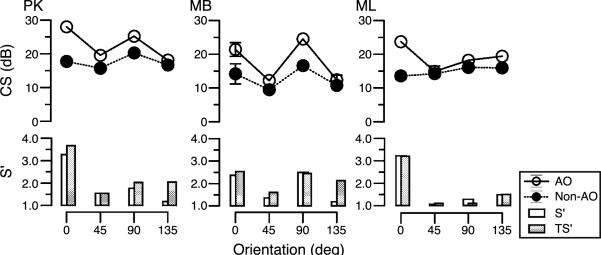
Figure 4 illustrates similar data at 12 c/deg for three other observers, but with four orientations rather than eight. Again there is an improvement in sensitivity when the gratings are viewed with the AO correction. Sensitivity to some orientations benefits more than others from AO correction. In nearly all cases, the predicted improvement at individual orientations corresponds to that seen in the contrast sensitivity measurements.
Figure 4.
Orientation-selective contrast sensitivity (dB) for three subjects measured at four orientations for both AO-corrected measurements (open symbols) and non-corrected measurements (closed symbols). Measured and calculated theoretical benefits, S′ and TS′, shown in bar graph below. Error bars indicate ±standard deviation.
Details of the relations between the aberrations and the effects of their compensation on contrast thresholds are seen in Table 1. Note that the data for HJT are based on 16 c/deg and those for the other observers are based on 12 c/deg.
Table 1.
Details of the relations between the aberrations (converted to equivalent defocus (DS)) and the effects of their compensation on contrast thresholds (S′). Note that the data for HJT are based on 16 c/deg and those for the other observers are based on 12 c/deg. Values in parentheses indicate ±standard deviation.
| Subject | Equivalent defocus (DS) | Average S′ | Max-min orientation specific S′ |
|---|---|---|---|
| MM | 0.32 | 5.0 (±2.2) | 8.0-2.0 |
| HJT | 0.21 | 2.6 (±0.6) | 3.6-1.6 |
| PK | 0.21 | 1.9 (±0.91) | 3.3-1.2 |
| MB | 0.15 | 1.9 (±0.7) | 2.5-1.2 |
| ML | 0.12 | 1.8 (±1.0) | 3.2-1.1 |
From Table 1, it can be seen that those subjects with the largest equivalent defocus (as defined in Methods section) without AO are inclined to show the greatest values of S′, as might be expected. For example, MM had the largest equivalent defocus (−0.32 DS) and the largest average value of S′ (5.0 ± 2.2) while ML had the smallest equivalent defocus (0.12 DS) and the lowest average S′ value (1.8 ± 1.0). The calculated cylindrical error for MM was 0.22 DC × 75 (equivalent to 0.1 DS) and ML had a 0.21 DC × 86 (equivalent to 0.1 DS). Most subjects displayed very similar maximum increase in CS for any one orientation, with maximum S′ between 2.5× and 3.6×. The one exception was MM, who displayed ×much higher values, with a maximum increase of 8× when AO correction was used. This may be related to the high equivalent defocus and the fact that most of the RMSE lies within the third, fourth, and fifth Zernike modes that relate to lower order astigmatism and spherical defocus (as can be seen from Figure 2). In addition, the largest HO aberration terms for this subject are in the middle of the Zernike pyramid. Note that it has been shown that the Zernike modes in the center of the pyramid have more impact on vision than those at the edge (Applegate, Marsack, Ramos, & Sarver, 2003). Both the measured and theoretical data for these observers are entirely consistent with these observations.
Overall, the values for S′achieved as a result of removing the aberrations are very similar to previously reported visual benefit. Yoon and Williams (2002), for example, found a factor of 5× improvement (averaged between two spatial frequencies) for one observer and a factor of 3.2× improvement for the other observer. Elliott et al. (2009) compared contrast sensitivity in young and older groups of subjects. They found a 2.5× improvement after AO compensation at 18 c/deg for young subjects and a 3× improvement at 9 c/deg for older subjects. What is interesting about our data is that 3 observers had at least one orientation where there was very little improvement at all with the AO correction (see minimum values of orientation specific S′ in Table 1). These minima were closely matched in both orientation and size to the theoretical predictions. The orientation for this minimum varied between observers but highlights the asymmetrical nature of the HO aberrations and their impact on contrast thresholds.
In order to examine the relation between theory and data, Figure 5 presents the predicted against the measured sensitivity improvement S′ for all observers and all conditions. It is evident that there is a close association between the two measures (r2 = 0.86, p < 0.001). There are, however, some exceptions and these are considered in the Discussion section.
Figure 5.
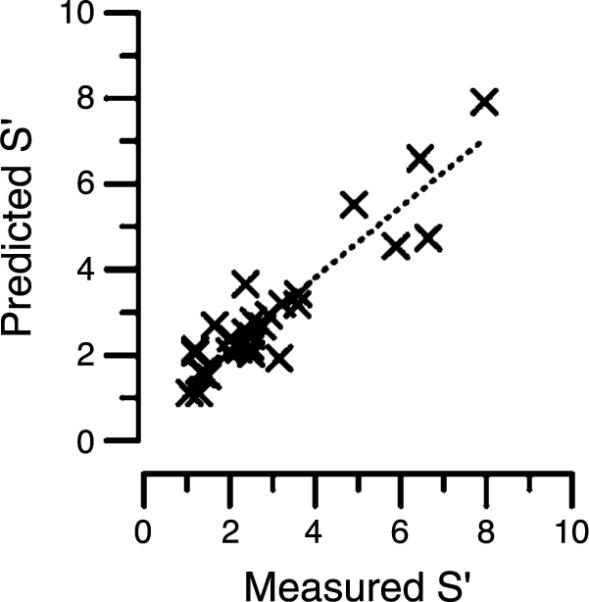
Correlation between measured and predicted theoretical benefit, S′ for all subjects in the study. R2 = 0.86, p < 0.001.
Discussion
We have shown that correcting the high-order aberrations of the eye leads to between 1.1× and 8× increase in sensitivity to a sinusoidal grating. This finding in itself is not an original observation; there are other reports showing similar levels of improvement in sensitivity. The novel feature of the present paper is that we have measured sensitivity at different orientations. We find that, in line with theory, when pupils are large (6 mm in this study), there are strong orientation-specific effects in all observers, with sensitivity to some orientations benefiting more than others from viewing through an AO system. Walsh and Charman (1992) emphasized that rotational asymmetries in the MTF and PTF vary markedly between individuals. The data presented here confirm this in that, to a large extent, there was a close correlation between theory and measurement. The effect of orientation can be seen by comparing the maximum and minimum values of S′ in Table 1. In all subjects, the orientation-specific difference in S′was always greater than 1 implying that the maximum benefit seen for the AO correction was highly orientation specific. The variation of these values between subjects illustrates that this effect depends on the mix of aberrations of a particular subject. For example, in subject PK, the dominant aberration term was vertical coma, and correspondingly, the greatest benefit seen for the correction was for the horizontal grating. Where observers' MTF was predicted to be strongly orientation dependent, this was reflected in the data. This is illustrated in Figure 5, where the correlation between the theoretical and actual measurements was significant (R2 = 0.86, p < 0.001).
It is clear, however, that the theory cannot account for all cases. While we modeled the effects based on the optical quality of the eye as measured during the experiment, other factors will inevitably be at work in a biological optical system. With broad band sources, transverse chromatic aberration (Thibos, 1987; van Meeteren, 1974) associated with individual differences in angle α can affect rotational asymmetry. Other uncontrolled factors include the small inter-individual differences in pupil centration and the fact that pupil centration may vary with pupil size (Walsh, 1988). A further possibility of course is that due to the idiosyncrasies of post-retinal processing, the visual system is not capable of capitalizing on improved optical quality of the retinal image. This eventuality is discussed with respect to myopia and emmetropia by Rossi, Weiser, Tarrant, and Roorda (2007). They used a visual acuity-based task and found that myopic observers did not benefit from AO correction as much as did emmetropic observers. They speculate that retinal factors, cortical factors, and the previous visual experience of the myopes may impede their ability to improve visual acuity when the quality of their ocular optics was improved with an AO system. Similar conclusions were reached by Sabesan and Yoon (2009) when testing keratoconic patients.
One variable that is important to consider is spatial frequency. As pointed out by Walsh and Charman (1992), in the presence of aberrations, the variation in MTF with orientation is small at low spatial frequencies and increases progressively with spatial frequency. This effect was also illustrated in Tahir, Parry, Pallikaris et al. (2009). In order to optimize theory and practice in these experiments, 12 c/deg and 16 c/deg were selected as a satisfactory compromise between spatial frequency and sensitivity; lower spatial frequencies have higher sensitivity but the meridional effects are minimal, whereas sensitivity for higher spatial frequencies is poor but the meridional effects can be expected to be greater. Montes-Mico and Charman (2001) used 12 c/deg for similar reasons. One intriguing issue here is that the gradual increase in orientational anisotropy with spatial frequency in the MTF matches the sensitivity measurements made at different spatial frequencies by Campbell et al. (1966) extremely well. This observation invites the possibility that in the first months of life, sensitivity at different orientations and the quality of the ocular optics develop in tandem. These issues are discussed in the next section.
Optical effects and early development
In everyday vision, as illustrated by Dalimier et al. (2008), it is likely that HO aberrations are of minimal importance in the adult eye. However, it has recently been argued that in addition to lower order aberrations, HO aberrations may influence the development of the visual system. It has been estimated that aberrations in neonate eyes are of the order of 20% greater than in adult eyes (Wang & Candy, 2005). They considered that the particular mix of HO aberrations may provide a signal for eye growth. This notion is supported by Williams and Boothe (1981) who found that the optical quality of the newborn monkey was superior to the resolution limit of the overall visual system. The highly aberrated neonate eye would almost certainly stimulate some meridians more than others, suggesting that the fine tuning of the orientation columns might be strongly influenced by the small irregularities in optics in the first few months of life. As shown in the data above, the HO aberrations influence sensitivity to contrast differently at different orientations and this raises the possibility that the resultant distortion of the retinal image may affect the development of the meridional anisotropy present in all individuals. Note that, as is evident in many papers (e.g., Tahir, Parry, Brahma et al., 2009; Timney & Muir, 1976) and the data presented here, sensitivity at the cardinal meridians is not superior to obliques in all observers. Some cases exhibit preferences for oblique axes or for axes slightly away from the vertical and horizontal. Another puzzling aspect of meridional asymmetries is that they are not always the same in an observer's two eyes. If the so-called oblique effect arose entirely from cortical factors, symmetry between the two eyes might be expected. Both these observations suggest that optical factors intervene in the early years of life to shape the overall orientation sensitivity of the visual system.
At present, there are two main lines of thought regarding orientation preference. The carpentered environment theory hypothesizes that a relative deficit in sensitivity could arise as a result of development in an environment dominated by vertical and horizontal contours (Coppola, Purves, McCoy, & Purves, 1998). However, Charman and Voisin (1993) argued against this hypothesis in light of the observed spatial frequency spectrum of typical rural and urban scenes (Mayer, Fulton, & Sossen, 1983) and the possibility that head tilt is likely to mitigate this effect (Banks, Aslin, & Letson, 1975). Instead, they cite the possibility that optical factors may contribute in a subtle way to orientation preference. It is known that the accommodation system of infants is highly active and Charman and Voisin (1993) show that in a with or against the rule astigmatic eye, when accommodation is optimized for minimal detail in the line spread function at different orientations, the worst performance will be along the oblique axes. This means that in these cases the retinal image is rarely exposed to any obliquely orientated high spatial frequencies.
The presence of with or against the rule astigmatism of even 0.25 to 0.50 DC during the critical period of visual development will result in the oblique Fourier components of a multiple frequency stimulus being significantly blurred and this may lead to the development of a permanent neural anisotropy. Mitchell, Freeman, Millodot, and Haegerstrom (1973) demonstrated that neural orientation selectivity can be influenced by uncorrected optical blur in early life in the human visual system by measuring orientation selectivity in subjects with astigmatism that had remained uncorrected until late in life. These observers were found to have reduced resolution limits to gratings corresponding to the axis of astigmatism, which remained when the optics of the eye were bypassed. Freeman and Thibos (1975) showed a similar effect for a range of spatial frequencies using visual-evoked potentials. These studies point to an optical influence of astigmatism on neural orientation selectivity development, but the influence of the HO aberrations has not previously been considered. As the data in this study have shown, aberrations can induce significant effects in orientation-selective contrast sensitivity. The presence of such blur in the critical period of visual development may also influence the neural development in early life.
In this paper, we report that the theoretical calculations showing the dependence of the MTF on orientation are substantiated by the aberration-corrected sensitivity measurements. In a previous paper (Tahir, Parry, Brahma et al., 2009), we showed that within a population of 34 individuals, orientation-specific sensitivity is dependent on whether they are viewing through normal or dilated pupils. With normal pupils some, but by no means all, observers show a grating sensitivity preference for cardinal (90° and 180°) orientations. There are subtle differences individually; many subjects have their peak sensitivity at orientations other than 90 and 180° and these exceptions to conventional orientational preferences are exaggerated when the pupil is dilated. We have speculated that these observations reveal a significant contribution of the ocular optics to orientational anisotropy. The data presented here support this idea, providing direct evidence of the role of ocular optics in orientational preference.
Acknowledgments
We thank Nathan Doble and Steve Jones for technical support. HJT was supported by a stipend from the College of Optometrists (UK). This research was supported by the National Institute on Aging (Grant AG04058). We also thank the referees for constructive comments.
Footnotes
Commercial relationships: none.
References
- Appelle S. Perception and discrimination as a function of stimulus orientation: The “oblique effect” in man and animals. Psychological Bulletin. 1972;78:266–278. doi: 10.1037/h0033117. [DOI] [PubMed] [Google Scholar]
- Applegate RA, Ballentine C, Gross H, Sarver E, Sarver C. Visual acuity as a function of Zernike mode and level of root mean square error. Optometry and Vision Science. 2003;80:97. doi: 10.1097/00006324-200302000-00005. [DOI] [PubMed] [Google Scholar]
- Applegate RA, Lakshminarayanan V. Parametric representation of Stiles–Crawford functions: Normal variation of peak location and directionality. Journal of the Optical Society of America A. 1993;10:1611–1623. doi: 10.1364/josaa.10.001611. [DOI] [PubMed] [Google Scholar]
- Applegate RA, Marsack JD, Ramos R, Sarver EJ. Interaction between aberrations to improve or reduce visual performance. Journal of Cataract and Refractive Surgery. 2003;29:1487–1495. doi: 10.1016/s0886-3350(03)00334-1. [DOI] [PubMed] [Google Scholar]
- Artal P. Calculations of two-dimensional foveal retinal images in real eyes. Journal of the Optical Society of America A. 1990;7:1374–1381. doi: 10.1364/josaa.7.001374. [DOI] [PubMed] [Google Scholar]
- Artal P, Chen L, Fernandez EJ, Singer B, Manzanera S, Williams DR. Neural compensation for the eye's optical aberrations. Journal of Vision. 2004;4(4):4, 281–287. doi: 10.1167/4.4.4. http://www.journalofvision.org/content/4/4/4, doi:10.1167/4.4.4. [DOI] [PubMed] [Google Scholar]
- Artal P, Santamaria J, Bescos J. Phase-transfer function of the human eye and its influence on point-spread function and wave aberration. Journal of the Optical Society of America A. 1988;5:1791–1795. doi: 10.1364/josaa.5.001791. [DOI] [PubMed] [Google Scholar]
- Banks MS, Aslin RN, Letson RD. Sensitive period for the development of human binocular vision. Science. 1975;190:675–677. doi: 10.1126/science.1188363. [DOI] [PubMed] [Google Scholar]
- Brainard DH. The psychophysics toolbox. Spatial Vision. 1997;10:433–446. [PubMed] [Google Scholar]
- Campbell FW, Gubisch R. Optical quality of the human eye. The Journal of Physiology. 1966;186:558–578. doi: 10.1113/jphysiol.1966.sp008056. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Campbell FW, Kulikowski JJ, Levinson J. The effect of orientation on the visual resolution of gratings. The Journal of Physiology. 1966;187:427–436. doi: 10.1113/jphysiol.1966.sp008100. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Charman WN, Voisin L. Astigmatism, accommodation, the oblique effect and meridional amblyopia. Ophthalmic & Physiological Optics. 1993;13:73–81. doi: 10.1111/j.1475-1313.1993.tb00429.x. [DOI] [PubMed] [Google Scholar]
- Chen L, Kruger PB, Hofer H, Singer B, Williams DR. Accommodation with higher-order monochromatic aberrations corrected with adaptive optics. Journal of the Optical Society of America A. 2006;23:1–8. doi: 10.1364/josaa.23.000001. [DOI] [PubMed] [Google Scholar]
- Chin SS, Hampson KM, Mallen EA. Role of ocular aberrations in dynamic accommodation control. Clinical & Experimental Optometry. 2009;92:227–237. doi: 10.1111/j.1444-0938.2009.00361.x. [DOI] [PubMed] [Google Scholar]
- Choi SS, Doble N, Hardy JL, Jones SM, Keltner JL, Olivier SS, et al. In vivo imaging of the photoreceptor mosaic in retinal dystrophies and correlations with visual function. Investigative Ophthalmology & Vision Science. 2006;47:2080–2092. doi: 10.1167/iovs.05-0997. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Coppola D, Purves H, McCoy A, Purves D. The distribution of oriented contours in the real world. Proceedings of the National Academy of Sciences. 1998;95:4002–4006. doi: 10.1073/pnas.95.7.4002. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Dalimier E. n., Dainty C, Barbur JL. Effects of higher-order aberrations on contrast acuity as a function of light level. Journal of Modern Optics. 2008;55:791–803. [Google Scholar]
- Elliott SL, Choi SS, Doble N, Hardy JL, Evans JW, Werner JS. Role of high-order aberrations in senescent changes in spatial vision. Journal of Vision. 2009;9(2):24, 1–16. doi: 10.1167/9.2.24. http://www.journalofvision.org/content/9/2/24, doi:10.1167/9.2.24. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Freeman RD, Thibos LN. Visual evoked responses in humans with abnormal visual experience. The Journal of Physiology. 1975;247:711–724. doi: 10.1113/jphysiol.1975.sp010953. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Furmanski CS, Engel SA. An oblique effect in human primary visual cortex. Nature Neuroscience. 2000;3:535–536. doi: 10.1038/75702. [DOI] [PubMed] [Google Scholar]
- Heeley DW, Buchanan-Smith HM, Cromwell JA, Wright JS. The oblique effect in orientation acuity. Vision Research. 1997;37:235–242. doi: 10.1016/s0042-6989(96)00097-1. [DOI] [PubMed] [Google Scholar]
- Hopkins HH. The frequency response of a defocused optical system. Proceedings of the Royal Society of London A. 1955;231:91–103. [Google Scholar]
- Hubel DH, Wiesel TN. Receptive fields of single neurones in the cat's striate cortex. The Journal of Physiology. 1959;148:574–591. doi: 10.1113/jphysiol.1959.sp006308. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kamitani Y, Tong F. Decoding the visual and subjective contents of the human brain. Nature Neuroscience. 2005;8:679–685. doi: 10.1038/nn1444. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kruger PB, Mathews S, Aggarwala KR, Sanchez N. Chromatic aberration and ocular focus: Fincham revisited. Vision Research. 1993;33:1397–1411. doi: 10.1016/0042-6989(93)90046-y. [DOI] [PubMed] [Google Scholar]
- Liang J, Williams DR, Miller DT. Supernormal vision and high-resolution retinal imaging through adaptive optics. Journal of the Optical Society of America A. 1997;14:2884–2892. doi: 10.1364/josaa.14.002884. [DOI] [PubMed] [Google Scholar]
- Mayer DL, Fulton AB, Sossen PL. Preferential looking acuity of pediatric patients with developmental disabilities. Behavioural Brain Research. 1983;10:189–197. doi: 10.1016/0166-4328(83)90164-x. [DOI] [PubMed] [Google Scholar]
- McMahon MJ, MacLeod DI. The origin of the oblique effect examined with pattern adaptation and masking. Journal of Vision. 2003;3(3):4, 230–239. doi: 10.1167/3.3.4. http://www.journalofvision.org/content/3/3/4, doi:10.1167/3.3.4. [DOI] [PubMed] [Google Scholar]
- Mitchell DE, Freeman RD, Millodot M, Haegerstrom G. Meridional amblyopia: Evidence for modification of the human visual system by early visual experience. Vision Research. 1973;13:535–558. doi: 10.1016/0042-6989(73)90023-0. [DOI] [PubMed] [Google Scholar]
- Montes-Mico R, Charman WN. Choice of spatial frequency for contrast sensitivity evaluation after corneal refractive surgery. Journal of Refractive Surgery. 2001;17:646–651. doi: 10.3928/1081-597X-20011101-03. [DOI] [PubMed] [Google Scholar]
- Mon-Williams M, Treslian JR, Strang NC, Kochkar P, Wann JP. Improving vision: Neural compensation for optical defocus. Proceedings of the Royal Society. 1998;265:71–77. doi: 10.1098/rspb.1998.0266. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Murray I, Parry N, Ritchie S, Bremner R, Brahma A, Ikram K, et al. Importance of grating orientation when monitoring contrast sensitivity before and after refractive surgery. Journal of Cataract & Refractive Surgery. 2008;34:551–556. doi: 10.1016/j.jcrs.2007.11.043. [DOI] [PubMed] [Google Scholar]
- Nio YK, Jansonius NM, Fidler V, Geraghty E, Norrby S, Kooijman AC. Spherical and irregular aberrations are important for the optimal performance of the human eye. Ophthalmic & Physiological Optics. 2002;22:103–112. doi: 10.1046/j.1475-1313.2002.00019.x. [DOI] [PubMed] [Google Scholar]
- Pelli DG. The videotoolbox software for visual psychophysics: Transforming numbers into movies. Spatial Vision. 1997;10:437–442. [PubMed] [Google Scholar]
- Porter J, Guirao A, Cox IG, Williams DR. Monochromatic aberrations of the human eye in a large population. Journal of the Optical Society of America A. 2001;18:1793–1803. doi: 10.1364/josaa.18.001793. [DOI] [PubMed] [Google Scholar]
- Rossi EA, Weiser P, Tarrant J, Roorda A. Visual performance in emmetropia and low myopia after correction of high-order aberrations. Journal of Vision. 2007;7(8):14, 1–14. doi: 10.1167/7.8.14. http://www.journalofvision.org/content/7/8/14, doi:10.1167/7.8.14. [DOI] [PubMed] [Google Scholar]
- Sabesan R, Yoon G. Visual performance after correcting higher order aberrations in keratoconic eyes. Journal of Vision. 2009;9(5):6, 1–10. doi: 10.1167/9.5.6. http://www.journalofvision.org/content/9/5/6, doi:10.1167/9.5.6. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Smirnov MS. Measurement of the wave aberration of the human eye. Biophysics. 1962;7:766–795. [Google Scholar]
- Stiles WS, Crawford BH. The luminous efficiency of rays entering the eye pupil at different points. Proceedings of the Royal Society of London B. 1933;112:428–450. [Google Scholar]
- Tahir H, Parry N, Pallikaris A, Ritchie S, Bremner R, Murray I. Orientation selectivity for sinusoidal gratings; evidence for an optical component. Journal of Modern Optics. 2008;55:839–847. [Google Scholar]
- Tahir HJ, Parry NR, Brahma A, Ikram K, Murray IJ. The importance of grating orientation in contrast sensitivity following refractive surgery. Ophthalmic & Physiological Optics. 2009;29:518–525. doi: 10.1111/j.1475-1313.2009.00676.x. [DOI] [PubMed] [Google Scholar]
- Tahir HJ, Parry NR, Pallikaris A, Murray IJ. Higher-order aberrations produce orientation-specific notches in the defocused contrast sensitivity function. Journal of Vision. 2009;9(7):11, 1–12. doi: 10.1167/9.7.11. http://www.journalofvision.org/content/9/7/11, doi:10.1167/9.7.11. [DOI] [PubMed] [Google Scholar]
- Thibos LN. Calculation of the influence of lateral chromatic aberration on image quality across the visual field. Journal of the Optical Society of America A. 1987;4:1673–1680. doi: 10.1364/josaa.4.001673. [DOI] [PubMed] [Google Scholar]
- Thibos LN, Applegate RA, Schwiegerling JT, Webb R, VSIA Standards Taskforce Members . Standards for reporting the optical aberrations of eyes. OSA trends in optics and photonics. In: Lakshminarayanan V, editor. Vision science and its applications. vol. 35. Optical Society of America; Washington, DC: 2000. pp. 232–244. [Google Scholar]
- Thibos LN, Hong X, Bradley A, Cheng X. Statistical variation of aberration structure and image quality in a normal population of healthy eyes. Journal of the Optical Society of America A. 2002;19:2329–2348. doi: 10.1364/josaa.19.002329. [DOI] [PubMed] [Google Scholar]
- Thibos LN, Ye M, Zhang X, Bradley A. The chromatic eye: A new reduced-eye model of ocular chromatic aberration in humans. Applied Optics. 1992;31:3594–3600. doi: 10.1364/AO.31.003594. [DOI] [PubMed] [Google Scholar]
- Timney BN, Muir DW. Orientation anisotropy: Incidence and magnitude in Caucasian and Chinese subjects. Science. 1976;20:699–701. doi: 10.1126/science.948748. [DOI] [PubMed] [Google Scholar]
- van Meeteren A. Calculations on the optical modulation transfer function of the human eye for white light. Optica Acta. 1974;21:395–412. [Google Scholar]
- Walsh G. The effect of mydriasis on the pupillary centration of the human eye. Ophthalmic & Physiological Optics. 1988;8:178–182. doi: 10.1111/j.1475-1313.1988.tb01034.x. [DOI] [PubMed] [Google Scholar]
- Walsh G, Charman W. The effect of defocus on the contrast and phase of the retinal image of a sinusoidal grating. Ophthalmic & Physiological Optics. 1989;9:398–404. [PubMed] [Google Scholar]
- Walsh G, Charman WN. Variation in ocular modulation and phase transfer functions with grating orientation. Ophthalmic & Physiological Optics. 1992;12:365–369. [PubMed] [Google Scholar]
- Wang J, Candy TR. Behavioral sensitivity to defocus in human infants. Investigative Ophthalmology and Visual Science. 2005;46 E-Abstract. [Google Scholar]
- Watson AB, Pelli DG. QUEST: A Bayesian adaptive psychometric method. Perception & Psychophysics. 1983;33:113–120. doi: 10.3758/bf03202828. [DOI] [PubMed] [Google Scholar]
- Williams RA, Booth RG. Development of optical quality in the infant monkey (Macaca nemestrina) eye. Investigative Ophthalmology and Visual Science. 1981;21:728–736. [PubMed] [Google Scholar]
- Yoon GY, Williams DR. Visual performance after correcting the monochromatic and chromatic aberrations of the eye. Journal of the Optical Society of America A. 2002;19:266–275. doi: 10.1364/josaa.19.000266. [DOI] [PubMed] [Google Scholar]