Applicability of non-destructive substitutes for leaf area in different stands of Norway spruce (Picea abies L. Karst.) focusing on traditional forest crown measures

Research highlights

▶ Determination of individual tree leaf area usually is only possible destructively. ▶ Surrogates which can be assessed non-destructively are investigated. ▶ From about 150 trees leaf area is estimated by 3P-branch sampling. ▶ These estimates are best correlated with crown surface area. ▶ Equations to determine individual tree leaf area non-destructively are presented.

Abstract

Since individual tree leaf area is an important measure for productivity as well as for site occupancy, it is of high interest in many studies about forest growth. The exact determination of leaf area is nearly impossible. Thus, a common way to get information about leaf area is to use substitutes. These substitutes are often variables which are collected in a destructive way which is not feasible for long term studies. Therefore, this study aimed at testing the applicability of using substitutes for leaf area which could be collected in a non-destructive way, namely crown surface area and crown projection area. In 8 stands of Norway spruce (Picea abies L. Karst.), divided into three age classes and two thinning treatments, a total of 156 trees were felled in order to test the relationship between leaf area and crown surface area and crown projection area, respectively. Individual tree leaf area of the felled sample trees was estimated by 3P-branch sampling with an accuracy of ±10%. Crown projection area and crown surface area were compared with other, more commonly used, but destructive predictors of leaf area, namely sapwood area at different heights on the bole. Our investigations confirmed findings of several studies that sapwood area is the most precise measure for leaf area because of the high correlation between sapwood area and the leaf area. But behind sapwood area at crown base and sapwood area at three tenth of the tree height the predictive ability of crown surface area was ranked third and even better than that of sapwood area at breast height (R² = 0.656 compared with 0.600). Within the stands leaf area is proportional to crown surface area. Using the pooled data of all stands a mixed model approach showed that additionally to crown surface area dominant height and diameter at breast height (dbh) improved the leaf area estimates. Thus, taking dominant height and dbh into account, crown surface area can be recommended for estimating the leaf area of individual trees. The resulting model was in line with many other findings on the leaf area and leaf mass relationships with crown size. From the additional influence of dominant height and dbh in the leaf area model we conclude that the used crown model could be improved by estimating the position of the maximum crown width and the crown width at the base of the crown depending on these two variables.

1. Introduction

Leaf area as photosynthetically active area is one of the main drivers for tree growth and thus an important tree characteristic for tree growth studies. For silvicultural purposes trees have to be considered as parts of stands, and individual tree growth has to be investigated in relation to stand structure. Thus, O’Hara (1988) used the area for individual trees as a measure of site occupancy. Leaf area in relation to stand parameters, e.g., ground area potentially available (APA), which could be named as individual tree leaf area index, but also leaf area in relation to stemwood increment which is described as growth efficiency (Waring, 1983) are important research issues. However, leaf area is hard to determine precisely and non-destructively. For leaf area index determination of stands various optical instruments like LAI-2000 (Li-Cor) or SunScan (Delta-T) are available. But these instruments are limited by the complexity of the canopy structure and improvement in accuracy is still needed (Moser et al., 1995; Chen et al., 1997; Pokorny and Marek, 2000; Pokorny et al., 2004). Another way to determine stand leaf area index is to use the individual tree leaf area. Hence, different approaches to estimate individual tree leaf area in an indirect way were and are investigated. Such investigations aim at strong relations of leaf area to other tree characteristics. Based on the pipe model of Shinozaki et al. (1964), which supposed that a given leaf area is supplied with water from a respective quantity of conducting pipes, mainly sapwood area (e.g., Waring et al., 1982; Bancalari et al., 1987; Meadows and Hodges, 2002), early sapwood area (Eckmüllner and Sterba, 2000), and diameter at breast height (e.g., Gholz et al., 1979; Baldwin, 1989) are used as estimators for leaf area or leaf biomass. A few studies deal with estimating leaf area with allometric functions based on different other tree characteristics (e.g., Pereira et al., 1997; Kenefic and Seymore, 1999).

The majority of studies dealing with indirect leaf area estimation describe sapwood area as the most accurate estimator for leaf area (e.g., Long et al., 1981; O’Hara and Valappil, 1995; Meadows and Hodges, 2002). But to get continuously information about leaf area and related characteristics, e.g., growth efficiency, and their development over time, the determination via sapwood area is not feasible, because from the same trees cores cannot be taken every 5 or 10 years over a long term. Additionally, it is well known that the relationship between leaf area and sapwood area, even within species, is not constant. Differences could be shown between sites, crown classes, stand density, and age (Long et al., 1981; Keane and Weetman, 1987; Coyea and Margolis, 1992; Shelburne et al., 1993; Gilmore et al., 1996). Furthermore, recently conducted studies show that climate can also influences the hydraulic architecture and therefore the ratio of leaf area to sapwood area (Poyatos et al., 2007; Martínez-Vilalta et al., 2009). And even within trees the relationship between leaf area and sapwood area can vary with the position within the tree (Mencuccini and Bonosi, 2001). Thus, for studies regarding the development of leaf area over time, other indirect methods for estimating leaf area should be found, preferably ones which are based on tree characteristics which can be collected easily and in a non-destructive way.

The use of other crown characteristics to estimate leaf area, such as crown ratio, crown length, crown projection area, and crown surface area is rarely investigated (Pereira et al., 1997; Kenefic and Seymore, 1999). Badoux (1945) and Assmann (1970) used crown surface area as substitute for leaf area with the evident assumption that most of the growth influencing photosynthetically active leaves are at the crown surface. Assmann (1970) also described that therefore differences in efficiency (there: growth per crown projection area) should lead to differences in the ratio of crown surface area to crown projection area and further, that trees with large crowns are less efficient than trees with smaller crowns due to their large inner crown volume (cubic content) bearing no leaves or needles. Therefore, this study aimed at the question if traditional forest crown measures, particularly crown surface area (CSA) and crown projection area (CPA) are good measures for leaf area (LA), and if not, whether they can be improved by corrections through additional tree measures or stand measures.

2. Materials and methods

2.1. Study area and selected stands

The study area was located near Bärnkopf, Lower Austria (15°00′20″ E, 48°23′24″ N) in the Bohemian Massif. On similar sites 8 even-aged Norway spruce (Picea abies L. Karst.) stands were investigated. The stands represented three different age classes and two thinning variants. We selected four pole stage stands, two premature, and two mature stands (ages of about 40, 80, and 125 years); two of the pole stage stands, and one of the premature and mature stands, respectively, were thinned 5 years ago (subsequently named “thinned”) and the other ones were not thinned for more than 10 years (subsequently named “un-thinned”). No other management, e.g., pruning or fertilization was performed in any of the investigated stands. Because of the relatively small size of the pole stage stands, for each thinning treatment two stands were selected. The fieldwork was conducted between April and September 2008.

2.2. Sample tree selection

At first in each stand, the diameter at breast height (dbh), the tree height, the height to the crown base, and the coordinates of each tree were assessed. Additionally, all trees were cored at breast height (one core), and their sapwood area at breast height was estimated from the measured dbh and the sapwood border of the increment core. The sapwood border was visually determined and marked in the field, immediately after core extraction, where the border between sapwood and heartwood can easily be recognized by differences in light transmittance. Since we intended to select sample trees covering the whole range of individual leaf area index (LAI = LA/APA) in the stand, a first approximation of both, individual tree leaf area (LA) and the ground area potentially available (APA), were needed. For the first approximation of leaf area we assumed a strong relationship between sapwood area at breast height and leaf area (Eckmüllner and Sterba, 2000), and thus used sapwood area as a proxy for leaf area. While Assmann (1970) defined APA by the crown projection area of a tree plus a proportional part of the surrounding gaps (or minus the proportional overlaps with other trees), we used leaf area instead of crown projection area for defining APA, because leaf area is supposed to reflect the respective growing space more accurately (Assmann, 1970). Thus, we allotted the stand area to each tree proportionally to its leaf area. For the actual calculation of APA we used the procedure of Römisch (1995) with the square root of leaf area as a weight: the procedure starts with dividing the stand area into little squares of 1 dm², and each of these squares is then attributed to that tree for which $D\text{/}\sqrt{LA}$ is minimum, with D, the distance between the centre of the square and the position of the tree, and LA, the leaf area estimated from the sapwood area. Then, in order to select sample trees, the trees of each stand were split into 3 equally frequent classes of dbh, and each of the dbh-classes was further split into 3 classes (equal size) of leaf area index. In each of these 9 classes 3 trees were selected randomly, however, avoiding trees on the edge of the stand, trees with any kind of abnormal crown growth (e.g., signs of defoliation, broken tops), and those whose neighbours were one of the few broadleaf trees in some of the stands. Thus, the sample size resulted in 27 sampled trees per stand. Since the two thinned and un-thinned pole stage stands, respectively were pooled for the selection of sample tress we finally had 162 sample trees.

2.3. Leaf area estimation of sample trees

To estimate the leaf area of each sampled tree we calculated in a first step the dry needle mass of each sampled tree. In a second step, we used the strong relationship between dry weight of 100 needles and specific leaf area (SLA) according to Hager and Sterba (1985) to get the SLA of each tree. SLA refers to projected leaf area. The leaf area of each sampled tree could then be easily calculated by multiplying the SLA and the dry needle mass. The detailed procedure is described subsequently.

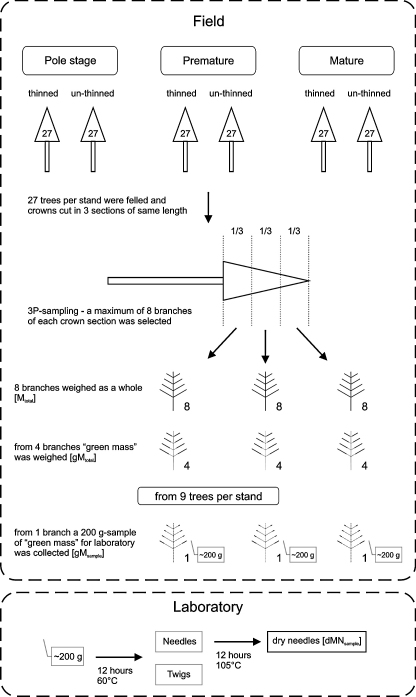
Each of the 27 sample trees of a stand was felled and its crown was cut into three sections of equal length (where crown base was defined as the first live branch where no whorl with only dead braches was above) (Fig. 1). Within each crown section we selected a maximum of 8 sample branches with a probability proportional to the square of their base diameter (3P-sampling, i.e., probability (of being selected in the sample) proportional to prediction according to Grosenbaugh, 1965). 3P-sampling is a well established and efficient sampling method, resulting in unbiased and thus reliable estimates (e.g., Schreuder et al., 1993). Although mainly used for estimating stand volume by selecting sample trees with a probability proportional to their estimated volume, it has also been used for estimating sparse species (Ringvall and Kruys, 2005) and needle mass (Eckmüllner and Sterba, 2000). Branches with a branch base diameter between 5 and 10 mm were not included in the 3P sample. All 24 selected branches per tree were weighed as a whole for determining the total fresh mass of the branch (M_total). From 12 branches (4 per crown section) the parts bearing no needles were discarded and the remaining fresh mass (green mass) was weighed again (gM_total). For 9 trees one branch per crown section was selected randomly, and for each of these branches a random sample of approx. 200 g from the gM_total was weighed accurately (gM_sample), filled into paper bags, and brought to the laboratory for further measurements to get the dry needle mass. There, these samples were dried for 12 h at 60 °C. After this, the needles were separated from the branches and twigs, and dried again for 12 h at 105 °C. After cooling to room temperature the needles were weighed to get the dry needle mass for the sample (dMN_sample). To determine the total dry needle mass of each sample tree (dMN_tree) we used the following steps:

Fig. 1.

Workflow to determine the dry needle mass of an individual tree.

First we calculated the ratio of the green mass, gM_total, to M_total, the total mass for 12 branches (4 of each crown section) of each of the 27 sample trees where we had determined gM_total.

$$q_{\text{gMM}}=\frac{g{M}_{\text{total}}}{M_{\text{total}}}$$ (1)

q_gMM was then modelled for each tree separately, depending on the branch base diameter (bbd) and the respective crown third.

$$q_{\text{gMM}}=a+b\cdot bbd+c\cdot c{s}_{\text{l}}+d\cdot c{s}_{\text{m}}+e\cdot (bbd\cdot c{s}_{\text{l}})+f\cdot (bbd\cdot c{s}_{\text{m}})$$ (2)

where cs_l and cs_m are dummy variables for the lower crown section and the middle crown section, respectively.

Furthermore, to determine the total dry needle mass of the selected branches (dMN_total) we needed the ratio of dry needle mass and green mass which we got from the samples in the laboratory with the following equation:

$$q_{\text{dg}}=\frac{dM{N}_{\text{sample}}}{g{M}_{\text{sample}}}$$ (3)

q_dg was not modelled for each tree separately, but as one common model for each stand, i.e., from 27 branches per stand – one branch from each crown third of the 9 sub-sample trees per stand.

$$q_{\text{dg}}=a+b\cdot \text{ln}\mathrm{dbh}+c\cdot bbd+d\cdot c{s}_{\text{l}}+e\cdot c{s}_{\text{m}}+f\cdot (c{s}_{\text{l}}\cdot \text{ln}\mathrm{dbh})+g\cdot (c{s}_{\text{m}}\cdot \text{ln}\mathrm{dbh})+h\cdot (c{s}_{\text{l}}\cdot bbd)+i\cdot (c{s}_{\text{m}}\cdot bbd)$$ (4)

where q_dg is the ratio according to Eq. (3), dbh, the breast height diameter of the tree, bbd, the branch base diameter, and cs_m and cs_l the dummy variables for the respective crown third.

Using these estimated ratios, the dry needle mass, $dM{N}_{{\text{total}}_{ij}}$, of each ith sampled branch of the jth sample tree in the respective stand, i.e., 27 trees with a maximum of 24 sample branches each, was estimated.

$$dM{N}_{{\text{total}}_{ij}}=M_{{\text{total}}_{ij}}\cdot q_{{\text{gMM}}_{ij}}\cdot q_{\text{dg}}$$ (5)

In the last step we had to determine the dry needle mass for all branches of each sample tree. Therefore we built the ratio between dry needle mass and branch basal area (bba), since the latter one we had for all branches.

$$q_{\text{nmbb}}=\frac{dM{N}_{\text{total}}}{bba}$$ (6)

Eq. (6) was calculated separately for each sampled branch of each of the 27 trees in each stand and then modelled depending on the crown section.

$$q_{nmbb}=a+b\cdot c{s}_{\text{l}}+c\cdot c{s}_{\text{m}}$$ (7)

Eq. (7) was then used to estimate the dry needle mass of all branches of all 27 sample trees in each stand.

$$dM{N}_{\text{total}\text{All}}=q_{\text{nmbb}}\cdot bba$$ (8)

Finally, the branches with a base diameter < 10 mm, which were not part of the 3P-sample, had to be added. We counted all these branches and then assumed an average branch base diameter of 8 mm and with this, calculated their dMN_{total All} according to Eq. (8).

Since we calculated the specific leaf area for each crown section separately (see below) we also had to calculate the total dry needle masses (dMN_jk) of each jth crown section of each kth sample tree. We therefore summed the dry needle masses (dMN_{total All}) of all n branches (indicated by i) of each crown section of each sampled tree.

$$dM{N}_{jk}=\sum _{i=1}^{n}dM{N}_{{\text{total\hspace{0.17em}All}}_{ijk}}$$ (9)

Applying the law of error propagation, and thus calculating the standard error of the needle mass of an individual tree (dMN_tree) from the standard errors of the ratios q in Eqs. (5)–(7), we achieved an average standard error of ±10.5%. This is just slightly above the result of a similar approach done by Eckmüllner and Sterba (2000) who had a CV of ±8.8%.

In a second step we calculated the specific leaf area from the dry mass of 100 needles. Out of the dMN_sample the mass of 50 needles was measured with an accuracy of 0.001 g and doubled to get the dry mass of 100 needles. With the relationship between specific leaf area and dry mass of 100 needles (Hager and Sterba, 1985) we calculated the specific leaf area for the respective branch. The polynomial model describing this strong relationship is only plausible up to 600 g dry mass of 100 needles, i.e., higher needle weights result in an implausibly increasing specific leaf area. Hence, for all branches with a dry mass of 100 needles higher than 600 g, the specific leaf area was set to the specific leaf area of a branch with 600 g dry mass of 100 needles. The specific leaf area was now available for one sampled branch per crown section and for 9 trees per stand (for the pole stands, the two thinned and the 2 un-thinned stands were pooled). This branch is assumed to be representative for its respective crown section and therefore its specific leaf area could also be taken as the representative specific leaf area for all branches in this crown section. Furthermore the selected 9 trees were taken as representatives of the 3 trees of their respective “dbh-LAI-class” (see Section 2.2). For example, the specific leaf area of the branch in the lowest crown section of sampled Tree 1 was taken for the entire lowest crown section of Tree 1, 2 and 3, since all these trees were in the same dbh-LAI-class. The leaf area of the kth sampled tree (LA_k) was finally calculated by multiplying its specific leaf areas of the jth crown sections (SLA_jk) and the according dry needle masses (dMN_jk) and summing these products.

$${\text{LA}}_k=\sum _{j=1}^3{\text{SLA}}_{jk}\cdot dM{N}_{jk}$$ (10)

It is this estimate, to which we later on refer as “individual tree leaf area”.

In the course of 3P-sampling, for three trees in one third of the crown no branch fell into the 3P sample. Hence, for these trees the leaf area could not be calculated correctly in one crown third. For one pre-selected sample tree no sample of needles was collected. Therefore, for all three trees of the respective “dbh-LAI-class” no needle mass and leaf area could be calculated. Thus, finally there were 156 sample trees left for further analyses (Table 1).

Table 1.

Sample tree characteristics: diameter at breast height (dbh) and standard deviation (SD).

	Stands
	Mature thinned	Mature un-thinned	Premature thinned	Premature un-thinned	Pole stage thinned A	Pole stage un-thinned A	Pole stage thinned B	Pole stage un-thinned B
Number of trees	27	25	26	27	14	10	13	14
dbh (cm)
Min	34.00	29.50	28.90	24.90	15.00	15.20	17.30	8.90
Mean	50.89	45.66	38.03	37.34	24.89	24.33	25.28	14.50
Max	61.30	65.40	51.90	48.90	31.50	32.50	34.00	25.40
SD	8.62	11.26	6.59	7.60	5.79	5.28	5.07	4.58
Height (m)
Min	27.30	24.70	24.40	22.20	15.80	15.50	18.60	8.60
Mean	35.96	33.68	29.55	28.74	21.74	19.75	22.60	14.61
Max	41.20	42.00	37.20	34.50	24.30	24.70	31.70	18.40
SD	3.73	4.98	3.02	2.93	2.48	2.69	3.46	2.92
Crown length (m)
Min	6.60	7.90	9.00	8.30	5.80	7.00	5.70	2.00
Mean	17.63	16.08	14.50	14.27	9.81	11.54	10.72	5.46
Max	27.70	24.10	19.30	18.80	13.30	17.20	15.90	8.70
SD	5.30	5.35	2.53	3.32	2.25	2.86	2.87	2.27
Crown width (m)
Min	3.85	3.48	4.79	3.70	2.18	2.20	2.69	1.18
Mean	7.06	6.52	5.96	5.35	3.58	3.79	3.93	2.14
Max	10.15	9.41	7.10	7.16	4.84	4.99	6.20	3.31
SD	1.54	1.64	0.72	1.04	0.89	0.88	1.11	0.59
Crown projection area (m2)
Min	11.62	9.53	17.83	11.14	3.74	3.97	5.68	1.08
Mean	40.87	35.26	28.36	23.18	10.54	11.21	13.11	3.84
Max	82.17	69.71	40.39	39.81	18.18	20.05	30.23	8.47
SD	17.77	17.11	6.84	8.71	4.85	4.67	7.89	2.06
Crown surface area (m2)
Min	56.12	53.27	101.10	65.83	29.67	35.62	40.11	6.96
Mean	247.60	212.74	168.04	150.12	69.23	83.54	83.72	23.99
Max	459.18	429.71	262.75	244.36	109.08	163.53	180.02	54.56
SD	104.93	108.99	41.59	56.94	28.91	33.51	42.10	14.69
Leaf area (m2)
Min	55.94	45.70	45.87	36.13	17.46	32.88	9.09	2.80
Mean	196.26	162.85	114.24	106.98	76.86	81.69	70.50	25.16
Max	435.23	446.05	209.50	292.99	140.98	156.52	164.10	64.99
SD	87.51	116.66	39.36	56.13	36.18	34.49	44.51	20.39

2.4. Determination of other tree measures

The dbh was measured with a diameter tape and the height with a Vertex IV (Haglöf, Sweden AB). The exact assessment of crown base, total height and crown length was performed on the felled trees with a measuring tape. To be able to calculate the crown projection area (CPA) we used Field-Map^® Version 8 (IFER, 2008) – a laser based tool for computer aided field data collection – to get coordinates of the tree positions and coordinates of 6–8 points (depending on the crown shape) of the crown border of each tree. While Field-Map^® also requires a person to visually determine the crown border, and therefore cannot help to increase the accuracy for the position of crown border points, it improves the overall accuracy for calculating the crown projection area. It allows recording more border points in the same time than conventional methods and therefore increasing the number of crown radii per tree which is much more essential for a precise calculation of the crown projection area than measuring a few radii with a high precision (Röhle and Huber, 1985). After collecting the data in the field we calculated the crown projection area using the quadratic mean of the recorded crown radii. For the crown surface area (CSA) we used the crown model described by Pretzsch (2001). This model assumes that the crown of Norway spruce consist of a cone above the maximum crown width, and a truncated cone between this maximum crown width and the base of the crown. The maximum crown width is assumed to occur at 33% of the crown length from below, and the crown width at the base of the crown is assumed to be half of the maximum crown width.

From each of the felled sample trees, three disks were taken: one at breast height, one at three tenth of the tree height, and one at the base of the crown. Immediately after taking the disks, the sapwood border was marked and then the disks were brought into the laboratory. There, along 4 radii, the sapwood border was recorded in order to calculate the sapwood area.

2.5. Statistical analyses

In a first step we compared the predictive power of crown surface area (CSA), crown projection area (CPA), and basal area (BA) with that of other often used substitutes for leaf area, e.g., sapwood area at crown base (SAP_cb), at breast height (SAP_dbh), and at three tenth of the tree height (SAP₀₃), for each stand separately by using log-linear regression models of the following form:

$$\text{ln}\text{LA}=a+b\cdot \text{ln}X$$ (11)

with LA the leaf area, a the intercept and b the coefficient for the respective substitute variable X. The coefficients were estimated by log-linear regression in order to avoid heteroscedasticity. Further on, analysis of covariance was used to test if (i) the assumption of a common slope for all stands was justified, (ii) the relation between LA and X was proportional (b = 1), and (iii) the intercepts did not differ between the stands.

Here should be mentioned that, if b = 1 the intercept a represents the proportionality factor of LA to X in the delogarithmized form of Eq. (11). In a next step the same procedures were used to test if the estimation of leaf area within the stands can be improved by including more variables into the above equation (11). Finally, we investigated if the leaf area models can be generalized by using tree and stand variables in the mixed model equation (12).

$$\text{ln}(\text{LA})=a+b\cdot \text{ln}(X)+c^{\text{T}}\cdot STANDVAR+u+e$$ (12)

Additionally to the variables and coefficients of Eq. (11) following variables were included: c^T a vector of the coefficients of STANDVAR which is a vector of the stand variables (Table 2) and a dummy variable for the thinning treatment. In the models the natural logarithm of each variable in Table 2 has been used. Finally, u, and e are the random effects of the stands and the trees, respectively. All statistical analysis were performed with Microsoft^® Office Excel 2003 (2003) and the statistical software package SPSS for Windows – Rel. 13.0 (2004). The mixed models were analysed and parameterized with the procedure “MIXED” of SPSS for Windows. In all models only variables with significant coefficients (p ≤ 0.05) were included. For comparing the models and finding the final ones, following goodness of fit criteria were used: R² for log-linear regression models with the same number of predictor variables, adjusted R² for log-linear regression models with a different number of predictor variables, and the Akaike Information Criterion (AIC) for mixed models according to Demidenko (2004).

Table 2.

Stand variables in each stand: basal area per hectare (G/ha), diameter corresponding to mean basal area of a stand (dg), stand density index (SDI) and crown competition factor (CCF).

	Stands
	Mature thinned	Mature un-thinned	Premature thinned	Premature un-thinned	Pole stage thinned A	Pole stage un-thinned A	Pole stage thinned B	Pole stage un-thinned B
Area (ha)	3.05	2.93	1.72	1.24	0.48	0.15	0.30	0.13
Trees/ha
Norway spruce	163	226	315	466	617	1888	774	2181
Broadleaves	8	17	0	6	0	0	0	0
Total	170	244	315	472	617	1888	774	2181
Dominant height (m)	37.2	37.3	31.0	30.4	24.0	21.1	23.6	17.8
G/ha (m2 ha−1)	33.86	43.94	37.14	47.54	30.20	47.45	32.41	37.63
dg (cm)	50.31	47.92	38.74	35.80	24.97	17.89	23.09	14.82
Age (years)	123	128	78	77	43	38	57	41
Site classa (m3 ha−1 a−1)	12.30	12.19	11.05	11.00	15.00	15.00	9.36	9.50
SDIb	573.93	754.37	674.29	881.26	615.50	1055.50	674.13	879.47
CCFc	114.62	146.55	146.32	201.69	164.41	316.14	183.61	302.46

^aMean annual increment at age 100 according to Marschall (1975).

^bSDI = N·(25/dg)^−1.73 according to Sterba (1987).

^cThe sum of the crown projection areas of equally large open grown trees in percent of the stand area according to Krajicek et al. (1961) with open grown tree crown widths of Hasenauer (1997).

3. Results

Judged from the average R² and the standard error of estimate of the natural logarithm of leaf area, the sapwood areas at crown base and at three tenth of the tree height are the best predictors for leaf area (Table 3). The accuracy of the equations with sapwood area as independent variable increases from breast height towards the base of the crown. Among the other substitute variables crown surface area seems to be the best, even better than sapwood area at breast height. Basal area and crown projection area are the poorest proxy for leaf area. However, it has to be noted that the figures in Table 3 concern regressions with different intercepts and slopes in each stand, and thus cannot be generalized.

Table 3.

Coefficient of determination (R²) and standard error of estimate (se) for the equation: ln LA = a + b ln X, with leaf area (LA), as dependent and different substitute variables (X): sapwood area at crown base (SAP_cb), sapwood area at three tenth of the tree height (SAP₀₃), sapwood area at breast height (SAP_dbh), crown surface area (CSA), basal area (BA) and crown projection area (CPA). $\text{Average}{R}^2=1-\left(\sum \text{sum of squares residuals}/\sum \text{sum of squares total}\right)$. $\text{Average se}={\left(\sum \text{sum of squares residuals}/\sum \text{degrees of freedom}\right)}^{0.5}$.

Stands		SAPcb	SAP03	CSA	SAPdbh	BA	CPA
Mature-thinned	R2	0.841	0.734	0.676	0.434	0.701	0.649
	se	0.194	0.251	0.276	0.366	0.266	0.288
	sig	***	***	***	***	***	***
Mature un-thinned	R2	0.824	0.805	0.522	0.631	0.758	0.444
	se	0.295	0.310	0.485	0.426	0.345	0.523
	sig	***	***	***	***	***	***
Premature thinned	R2	0.608	0.444	0.410	0.440	0.392	0.152
	se	0.219	0.260	0.268	0.261	0.272	0.322
	sig	***	***	***	***	**	*
Premature un-thinned	R2	0.728	0.707	0.639	0.663	0.620	0.559
	se	0.287	0.298	0.331	0.320	0.339	0.365
	sig	***	***	***	***	***	***
Pole stage thinned, A	R2	0.823	0.669	0.700	0.721	0.724	0.464
	se	0.255	0.350	0.333	0.321	0.319	0.445
	sig	***	***	***	***	***	**
Pole stage un-thinned, A	R2	0.735	0.740	0.721	0.836	0.691	0.803
	se	0.237	0.213	0.243	0.187	0.256	0.204
	sig	**	*	**	***	**	***
Pole stage thinned, B	R2	0.901	0.884	0.755	0.848	0.864	0.342
	se	0.246	0.266	0.387	0.305	0.288	0.634
	sig	***	***	***	***	***	*
Pole stage un-thinned, B	R2	0.725	0.600	0.773	0.422	0.365	0.663
	se	0.502	0.606	0.457	0.729	0.764	0.557
	sig	***	**	***	*	*	***
Average	R2	0.785	0.714	0.656	0.600	0.635	0.515
	se	0.281	0.325	0.356	0.384	0.367	0.423

^*<0.05.

^**<0.01.

^***<0.001.

Next, the relationships according to Eq. (11) were investigated for common slopes (Table 4). For all sapwood areas the hypothesis that the slopes do not differ between the stands had to be rejected. The same is true for the basal area as a proxy for the leaf area. Only for crown projection area and for crown surface area, a common slope could be assumed. Among those, the adjusted R² indicates that the estimations from the crown surface area are better than those from the crown projection area. Interestingly the crown surface area with a common slope seems to be a better estimator for leaf area than the sapwood area at breast height. Furthermore, the test for the hypothesis that the slope does not deviate from 1, indicates that leaf area can be assumed proportional to all substitute variables, except for the sapwood area at breast height.

Table 4.

Models of the form ln LA = a + b ln X, with common slopes (b), but different intercepts (a) by stand: t_slope = 1 is the t-statistic for the hypothesis that b = 1, i.e., that leaf (LA) area is proportional to the substitute variable (X). The null hypothesis that the slopes do not differ between the stands is tested by an F-test with 7 and 140 degrees of freedom (F_{equal slopes}). Sapwood area at crown base (SAP_cb), sapwood area at three tenth of the tree height (SAP₀₃), sapwood area at breast height (SAP_dbh) and crown surface area (CSA), basal area (BA) and crown projection area (CPA).

Substitute variables	Adj. R2	Slope	tslope = 1	p(>t)	Fequal slopes	p(>F)
SAPcb	0.878	0.943	1.281	0.202	2.874	0.008
SAP03	0.826	0.991	0.145	0.884	3.673	0.001
CSA	0.816	1.006	0.099	0.921	1.526	0.163
SAPdbh	0.790	0.859	2.333	0.021	5.175	<0.001
BA	0.799	1.026	0.376	0.707	2.144	0.043
CPA	0.747	0.914	1.128	0.261	0.944	0.475

The test, if the intercepts differ is only applicable if the slopes do not differ between stands, thus only for the crown projection area and for the crown surface area. This test is the same as the test for differences of the adjusted means. These adjusted means differed significantly by stands for both independent variables ln CPA and ln CSA, with F = 3.227 and 4.086 and p > F of 0.0033 and 0.0004 respectively. Hence, LA/CSA and LA/CPA are proportional in all stands but the ratios differ significantly between the stands. This is, why later on we will investigate the relationship between the intercepts and stand variables (Eq. (12)).

Deciding that among those substitute variables, which can be assessed in a non-destructive way, crown surface area is the best choice to predict leaf area, we furthermore investigated if these estimations can be improved by adding additional variables. Since crown length and crown width are both parameters from which the crown surface area is calculated, the main additional information for leaf area has been expected to come from the dbh, which is not part of Pretzsch's (2001) crown model. However, the analysis of covariance for the model:

$$\text{ln}\text{LA}=a+b\cdot \text{ln}\text{CSA}+c\cdot \text{ln}\text{dbh}$$ (13)

exhibited first that in no stand both variables, crown surface area and dbh, were significant. Only in one stand, crown surface area was significant, and in three stands, dbh was significant. Second, assuming common coefficients b and c for all stands, both coefficients were significant. However, the hypothesis for equal coefficients had to be rejected (p = 0.00012).

Finally, the best general equation (lowest AIC) for estimating leaf area, estimated as mixed model with the stands as random effects, was

$$\text{ln}\text{LA}=a+b\cdot \text{ln}\text{CSA}+c\cdot \text{ln}\text{dbh}+d\cdot \text{ln}{h}_{\text{dom}}$$ (14)

with LA, the leaf area in m², CSA, the crown surface area in m², dbh, the diameter at breast height in cm, and h_dom the dominant height in m, defined as the mean height of the 100 largest (by dbh) trees per hectare, and the coefficients given in Table 5.

Table 5.

Estimated coefficients of Eq. (14) to predict leaf area (LA).

Dependent variable	a	b	c	d
ln LA	1.024	0.631***	0.944***	−0.840**

^**p ≤ 0.01.

^***p ≤ 0.001.

None of the other variables, age, site index, the dummy variable for thinning, and the measures of stand density were significant. The variances of the random effects were 0.012347 for the stand and 0.118556 for the tree respectively. The random effect of the stand was not significant (p > Wald_z = 0.278).

The only stand variable, affecting leaf area turned out to be the dominant height, which can be understood as a compensatory measure for age and site class, indicating the stage of development of the stand. Thus, we conclude that the stand effect is sufficiently described by the dominant height of the stands.

In order to describe and for a better understanding of the relationship between leaf area and crown surface area the final model can be rearranged as:

$$\frac{\text{LA}}{\text{CSA}}=e^{1.024}\cdot {\text{CSA}}^{-0.365}\cdot {\text{dbh}}^{0.944}\cdot h_{\text{dom}}^{-0.840}$$ (15)

Furthermore, at a given dominant height, i.e., within a stand, the dbh can be understood as a measure for the social position (crown class) of a tree within the stand, which can be described as h_dom/dbh.

Inserting the ratio, h_dom/dbh, into Eq. (15) results in:

$$\frac{\text{LA}}{\text{CSA}}=2.784\cdot {\text{CSA}}^{-0.369}\cdot h_{\text{dom}}^{0\text{.104}}\cdot {\left(\frac{h_{\text{dom}}}{\text{dbh}}\right)}^{-0.944}$$ (16)

now describing the leaf area per crown surface area as a function of crown surface area, dominant height as a compensatory measure for age and site class, and the h_dom/dbh, the social position of the tree within the stand. From this equation the sensitivity of the LA/CSA ratio to the independent variables can be easily studied. An increase of dominant height by 10% leads to an only 1% higher leaf area per crown surface area; an increase of 10% in crown surface area results in a decrease of this ratio by 3.5% and increasing the h_dom/dbh ratio by 10% decreases the leaf area per crown surface area by 8.6%.

4. Discussion

Our findings confirm what many other authors stated, that sapwood area is a very precise measure for leaf area (e.g., Waring et al., 1982; Bancalari et al., 1987; Meadows and Hodges, 2002). Within stands, the sapwood area was a better indicator for leaf area, the nearer to the base of the crown it was determined (Table 3). However, the coefficients of the log-linear relationship between leaf area and sapwood area differed significantly between the investigated stands (Table 4). The sapwood area at breast height, which can be more easily determined than those higher up on the bole, exhibited the largest differences of the coefficients between the stands. This result is in line with several other studies where the stand was identified as a driver causing differences in the ratio leaf area to sapwood (Binkley and Reid, 1984; Long and Dean, 1986; Coyea and Margolis, 1992). Among the other tree variables the crown surface area calculated according to Pretzsch (2001) was by far the best predictor for leaf area. At least within the crown measures this is not surprising, since, in contrary to the 2-dimensional crown projection area in the crown surface area the crown length, as additional information of the third dimension, is included. Obviously, crown surface area shows a more realistic model of the actual crown shape. Furthermore, the coefficients of the log-linear relationship with leaf area did not differ significantly between the stands, and the common coefficient of this relationship was nearest to one. Thus, within stands, crown surface area can be assumed to be proportional to leaf area.

Some other authors who also worked on non-destructive methods for estimating leaf area found their models also improved by adding crown parameters. But, in contrary to our study, they used crown length (Pereira et al., 1997; Kenefic and Seymore, 1999) or crown ratio (Valentine et al., 1994). Like crown surface area, their influential crown parameters also contained information about the third dimension of the crown. Hence, the importance to consider crown variables describing the length of the crown to find models of high quality for the estimation of leaf area seems to be crucial. Our test to improve the leaf area estimation through additional variables showed that for all stands together, the common relationship with crown surface area and dbh was better than the one with crown surface area alone. However, this relationship with both variables had significantly different coefficients between the stands, and therefore it would have to be parameterized separately in every stand. Thus, the advantages of crown surface area as a measure for leaf area within stands are (i) its high correlation with leaf area, even better than that for sapwood area at breast height (see Tables 3 and 4), (ii) its property of having a relationship with leaf area with a coefficient not different between stands, and (iii) a coefficient very near 1, so that it can be assumed being proportional to leaf area. All together makes the crown surface area an applicable measure for the leaf area within stands. Because of this strong relationship the crown surface area could also be used to distribute a given stand's leaf area appropriately to individual trees within this stand. In some studies regarding crown damage and tree growth the crown surface area was used as a kind of substitute for dry needle mass without testing the relationship between these two parameters (Kramer, 1986; Halmschlager et al., 2007). Given that the leaf area is highly correlated with the dry needle mass (Hager and Sterba, 1985) – in our study leaf area is actually calculated out of the dry needle mass – the results of these studies are justified retrospectively by our results.

So far, only the within-stand relationships between leaf area and its surrogates have been discussed. The general model over all investigated stands showed the preceding results (i) the best relationship of leaf area was found for crown surface area and (ii) the dbh played an additional role in this relationship. However, the dbh was not significant in any of the individual stands if the crown surface area was in the model. Finally, (iii) significantly different intercepts of the stand's common relationship between leaf area and crown surface area were found. The latter fact was accounted for, by inserting the dominant height as stand variable into the final general model (Eq. (14)). Furthermore, the model was rearranged and the social position of trees was also included (Eqs. (15) and (16)).

The fact that at a given dominant height, the ratio h_dom/dbh describes the social position of the tree in the stand, with high ratios for poor social positions (crown classes) and vice versa, may be the reason, why also a few other authors (Valentine et al., 1994; Kenefic and Seymore, 1999) also published models of high qualities with both, dbh or basal area and crown variables, as independent variables.

Eq. (16) is used to depict this relationship for the lowest and the highest dominant height of the investigated stands (Fig. 2).

Fig. 2.

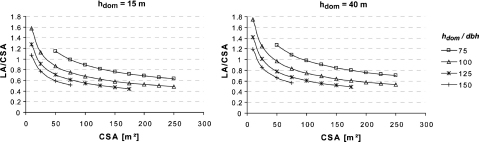
Comparison of the relationship between leaf area (LA) and crown surface area (CSA) of the lowest and highest dominant height (h_dom) concerning different ratios of dominant height to diameter in breast height (dbh).

Clearly, at a given dominant height, i.e., in a given stand, and at a given social position (h_dom/dbh) the leaf area per crown surface area decreases with increasing crown surface area, i.e., crown size. This is very much in line with Assmann's (1970) expectation that within a crown class, the larger crowns assimilate less efficient, because of their higher “proportion of strongly respiring shoots”, i.e., the ratio crown surface area to cubic crown content decreases. That, on the other hand, a tree with a given crown surface area has the more leaf area the better its crown class (lower h_dom/dbh ratio) is, was expected.

Unfortunately, the early investigations of Burger (1939a,b) on needle mass and crown size do not consider crown class as an influential variable. However, using his results, and assuming a specific leaf area of 4 m² per kg needle mass (from Hager and Sterba, 1985 for dominant trees), comparable results can be shown, namely a LA/CSA ratio of about 0.8 and only minor differences in this ratio between the two investigated stands, which differed clearly in age (98 and 132 years respectively), in site quality, and in density. These differences resulted clearly in different average crown surface areas, but not so in the average LA/CSA ratio.

5. Conclusion

As an estimator for individual tree leaf area within stands, crown surface area calculated from Pretzsch's (2001) crown model for Norway spruce was even slightly better than sapwood area at breast height (R² = 0.656 compared with 0.600). The main advantage of crown surface area as compared to sapwood area is that it can be estimated in a non-destructive way without coring. In the investigated stands, crown surface area turned out to be proportional to leaf area, and thus, crown surface area can be used as a weight in calculating the stand area potentially available for individual trees and – as the ratio between these two measures – as an individual tree leaf area index in order to repeatedly monitor this figure in long term experiments.

In the pooled leaf area model for all investigated stands together, additionally to crown surface area, dominant height and breast height diameter significantly improved the leaf area estimates. Thus, the crown model of Pretzsch (2001) probably could be improved by relating the position of the maximum crown width to these variables. For such an improved crown model however, a larger data base, including more stands with a larger variation in site quality would be necessary. Meanwhile, Eq. (16) turned out to be in line with many older findings on the relationship of leaf area or leaf mass and crown size, and thus can be recommended for estimating the leaf area of individual Norway spruce trees, when coring the trees should be avoided.