Proton radiotherapy: the biological effect of treating alternating subsets of fields for different treatment fractions

Abstract

Purpose

Common practice in proton radiotherapy is to deliver a subset of all fields in the treatment plan on any given treatment day. We investigate using biological modeling if the resulting variation in daily dose to normal tissues has a relevant detrimental biological effect.

Methods and Materials

For four patient groups, the cumulative normalized total dose (NTD) was determined for normal tissues (OARs) of each patient using the clinically delivered fractionation schedule (FS_clin), and for hypothetical fractionation schedules delivering all fields every day (FS_all) or only a single field each day (FS_single). Cumulative 3D NTD distributions were summarized using the generalized equivalent uniform dose (gEUD) model.

Results

For the skull base/cervical spine chordoma group, the largest effect is a 4 Gy increase in gEUD of the chiasm when treating only a subset of fields on any day. For lung cancer and pancreatic cancer patients the variation in the gEUD of normal tissues is less than 0.2 Gy. For the prostate group, FS_clin increases the gEUD of the femoral heads by 9 Gy compared to FS_all. Use of FS_single resulted in the highest NTD to normal tissues for any patient. FS_all resulted in an integral NTD to the patient that is on average 5% lower than FS_clin and 10% lower than FS_single.

Conclusion

The effects of field set of the day treatment delivery depend on the tumor site and number of fields treated each day. Modeling these effects may be important for accurate risk assessment.

Introduction

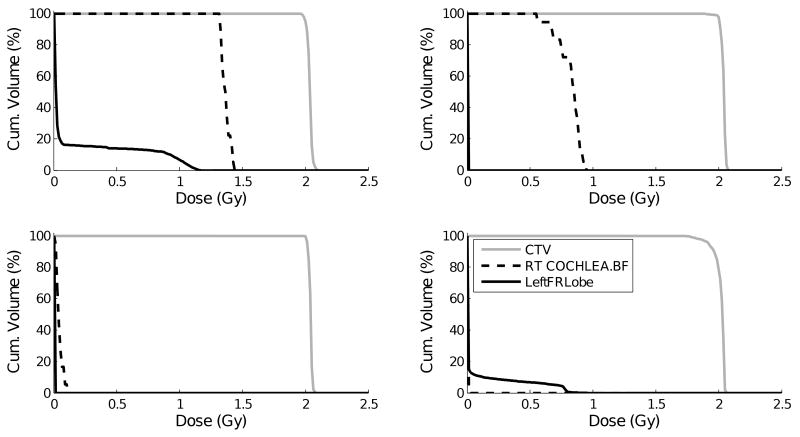
Worldwide more than 40.000 cancer patients have received proton radiotherapy. The vast majority of these patients have been treated with passively-scattered proton radiotherapy in which each field, or each patch combination (1), delivers a homogeneous dose to the target volume. Unlike photon radiotherapy, where daily delivery of all fields is required to achieve the desired homogeneous dose to the target, in proton radiotherapy one can choose to use a varying subset of fields each day while maintaining target dose homogeneity. This methodology of only delivering a field set of the day is often applied in clinical proton radiotherapy practice. The drawback is that, on a daily basis, the integral dose to each of these patients is more concentrated in the irradiated normal tissues. Correspondingly, each (voxel of) normal tissue is subject to a varying daily physical dose, which has a negative biological effect (2, 3). As an example, Figure 1 shows dose-volume histograms (DVH) for four unique treatment fractions of a single skull base/cervical spine chordoma cancer patient. Although the clinical target volume (CTV) receives full dose for each fraction, the dose to normal tissues can vary substantially on a treatment day-to-day basis.

1).

Dose volume histograms for the tumor and two normal tissues for four different proton radiotherapy treatment fractions of the same chordoma cancer patient.

The cumulative DVHs and isodose distributions within the treatment planning system, upon which physicians make decisions regarding a treatment plan, fail to evaluate the impact of this variation in normal tissue dose. Additionally, the lack of acknowledgement of delivering a field set of the day may confound late effect reporting from cooperative groups, such as the Children's Oncology Group (COG) or Radiation Therapy Oncology Group (RTOG).

In this manuscript we investigate the effect of field set of the day treatment delivery on the biologically relevant dose to normal tissues for a variety of tumor sites currently being treated with proton radiotherapy.

Methods and Materials

Throughout this article when we use the term “dose” or “physical dose” this denotes the physical dose corrected for the radiobiological effectiveness of the radiation used. For protons we assume the widely used RBE = 1.1. The abbreviation NTD (4) is used after conversion of physical dose into the normalized total dose taking into account dose-per-fraction effects by means of the linear-quadratic model (2).

Patient selection

At our proton therapy center, we treat a large variety of patients. In this study we limited ourselves to only a few representative tumor sites, as presented in Table 1. We will briefly discuss the characteristic treatment plans for each group of patients.

Table 1.

Treatment plan characteristics.

Patient Group	Patients (#)	Prescribed dose (Gy)	Fraction dose (Gy)	Fractions (#)	Fields in plan (#)	Fields per day (#)	UFC‡ (#)
Prostate	8	78	2	39	4†	1	2
Chordoma	5	76	2	38	9 – 11	2	6 or 7
Pancreas	4	25	5	5	3	2	3
Lung	5	70	2	35	3	2	3

^†Effectively two fields only, see text.

^‡UFC = unique field combinations

The base of skull/cervical spine chordomas in this study were in close proximity to the brainstem or spinal cord. The prescribed dose was 76 Gy in 38 fractions. No explicit PTV is designed in the planning process. Rather, pProximal and distal range uncertainties are directly taken into account in the choice of range and modulation of the spread-out Bragg peak (SOBP) and uncertainties in patient positioning are directly translated into increased aperture margin and range compensator smearing. For the clinically applied treatment plan, all patients received 20 Gy to the CTV via a three photon field treatment. This was followed by 4-5 more or less equally weighted proton fields raising the CTV dose to 60 Gy. The final 16 Gy was delivered to the GTV using one or two patch combinations (i.e. two or four fields). Each 38 fraction treatment plan included 6 or 7 unique field combinations (UFC). The lung cancer patients analyzed in this study were in clinical practice treated with photons. New treatment plans were designed each consisting of proton fields only. Each plan consisted of 3 fields, typically not equally weighed, to deliver a total dose of 70 Gy in 35 fractions. No PTV was used in treatment plan design. Each field was first designed to cover the CTV tightly with the prescribed isodose level (95%). Next, the aperture margin and smearing were increased by 8 mm to account for setup uncertainties and patient breathing (5). Furthermore, the range and modulation of the SOBP were increased to take into account range uncertainties of 3.5% plus 1 mm. As only two fields were assumed to be treated every day, the total dose was delivered using 3 UFC.

The pancreatic cancer patients received proton radiotherapy only. Each treatment plan consisted of three fields. Safety margins in treatment planning were applied as described earlier for the chordoma group. Each treatment fraction consisted of a combination of two treatment fields and the total dose was delivered using 3 UFC. Typical beam directions are a posterior field, a right lateral or right anterior oblique field, and a left inferior oblique (to undercut the stomach). The field depositing the least dose in the stomach delivered 40% of the total dose. The other two fields were equally weighed at 30%.

A typical prostate plan consists of a left lateral and a right lateral field treating the CTV (GTV + seminal vesicles) to 50 Gy, followed by coned-down left and right lateral fields treating the GTV only to 78 Gy. A PTV with a 1 cm uniform margin is used for treatment planning and an additional range uncertainty of 3.5% plus 1 mm is applied. For the cone-down a reduced safety margin is used posteriorly towards the rectum. In clinical practice only a single field is delivered each day. The large fields and coned-down fields use exactly the same beam directions and have almost the same shape and size. Therefore a prostate plan can be thought of as consisting of only a left lateral and a right lateral field, while there are only 2 unique field combinations (UFC) consisting of a single beam each. We randomly selected treatment plans of 8 patients to be included in this study.

Fractionation schedules

For each patient in each group, we analyzed three different fractionation schedules (FS).

FSphys	The cumulative physical dose distribution, i.e. corrected for RBE only and not for the biological effect of varying dose per fraction.
FSall	This fractionation schedule assumes that all fields in the entire treatment plan are delivered every day. It therefore consists of only a single unique treatment fraction. Cumulative dose distributions are corrected for RBE and the dose-per-fraction.
FSclin	The exact clinical representation of the delivered treatment plan as obtained from our record and verify system, i.e. using a subset of treatment fields each day. Cumulative dose distributions are corrected for RBE and the dose-per-fraction.
FSsingle	This fractionation schedule assumed that only a single proton field, or a single patch combination, was used each treatment day. The number of unique treatment fractions therefore equaled the number of unique fields (and patch combinations). Cumulative dose distributions are corrected for RBE and the dose-per-fraction.

Please note that the fractionation schedules do not vary the dose per fraction as prescribed to the target, but only vary the combination of fields used to deliver the prescribed fraction dose on different treatment days.

Data analysis

The 3D dose distribution for each separate field in a treatment plan was exported from the treatment planning system to the Matlab¹ programming environment. The 3D dose distribution for each UFC was constructed by adding the appropriate dose cubes, and subsequently converted into normalized total dose by means of equation 1

$${\mathit{\text{NTD}}}_{i,f}=D_{i,f}\frac{(\stackrel{\alpha }{}/\underset{\beta }{}+D_{i,f})}{(\stackrel{\alpha }{}/\underset{\beta }{}+D_{\mathit{\text{ref}}})}$$ (1)

with D_i,f the RBE-corrected dose to voxel i in fraction f, D_ref the reference fraction dose, and parameter α/β as in the linear-quadratic model for cell survival. The cumulative NTD for each voxel i was a summation over all fractions n_f

$${\mathit{\text{NTD}}}_i={\sum }_{f=1}^{n_f}{\mathit{\text{NTD}}}_{i,f}$$ (2)

For each patient and fractionation schedule the cumulative 3D distribution was summarized into a DVH for each of the relevant delineated tissues. The cumulative 3D NTD distribution was then summarized into the generalized equivalent uniform dose (gEUD) using equation (3)

$$\mathit{\text{gEUD}}={\left(\frac{{\sum }_i{({\mathit{\text{NTD}}}_i)}^a}{i}\right)}^{\stackrel{1}{}/\underset{a}{}}$$ (3)

with a the volume component that depends on the structure of interest (6). For FS_phys the gEUD is calculated over the 3D physical dose distribution, that was corrected for the RBE only and not for dose per fraction effects.

Results

In the data we present the reference fraction dose, D_ref, is always 2 Gy unless specifically mentioned otherwise. In Table 2 we summarize the gEUD data. The gEUD for FS_phys expresses the mean gEUD for a certain normal tissue as averaged over all patients in a group. Subsequent columns express the difference in mean gEUD with respect to the previous column. For example, the gEUD of the chiasm in the chordoma group is 51.8 Gy for FS_phys. When taking into account dose per fraction effects only (i.e. still treating all fields every day, FS_all) the gEUD of the chiasm is lower by 4.6 Gy. Using the clinically applied treatment delivery schedule (FS_clin), increases the gEUD by 3.8 Gy compared to FS_all.

Table 2.

Biological modelling parameters used, and gEUD of normal tissues as a function of the frationation schedule. The gEUD values express the mean gEUD, averaged over all patients within a patient group. Data in the last three columns express the difference in mean gEUD compared to the fractionation schedule of the preceding column. Data in bold satisfies p < 0.05 (Student's t-test, one-sided, paired) and a difference in mean gEUD of at least 1 Gy.

Patient Group	Normal Tissue	α/β/ Dref /‘a’	FSphys gEUD (Gy)	FSall ΔgEUD (Gy)	FSclin ΔgEUD (Gy)	FSsingle ΔgEUD (Gy)
Chordoma	Brainstem	2 / 2 / 7	44.2	-5.3	3.4	0.3
	Chiasm	3.5 / 2 / 6	51.8	-4.6	3.8	0.3
	Cochlea (left)	3.5 / 2 / 10	30.2	-6.3	2.8	1.2
	Cochlea (right)	3.5 / 2 / 10	33.0	-6.4	2.6	1.2
	Frontal lobe (left)	2 / 2 / 5	21.1	-4.1	1.9	0.6
	Frontal lobe (right)	2 / 2 / 5	21.4	-4.2	1.9	0.7
	Temporal lobe (left)	2 / 2 / 5	26.8	-3.7	1.8	0.4
	Temporal lobe (right)	2 / 2 / 5	27.4	-3.7	1.6	0.3
Lung	Esophagus	3.5 / 2 / 8	41.7	-2.2	0.1	0.5
	Heart	2 / 2 / 4	56.6	-0.5	0.0	0.2
	Lung minus GTV	3.5 / 2 / 1	11.8	-1.1	0.1	0.5
Pancreas	Bowel (small)	3.5 / 5 / 4	14.7	-0.5	0.0	0.5
	Duodenum	3.5 / 5 / 4	24.7	0.4	0.0	0.1
	Kidney (left)	3.5 / 5 / 1	5.7	-1.2	0.2	0.8
	Kidney (right)	3.5 / 5 / 1	6.8	-1.2	0.1	0.5
	Stomach	10 / 5 / 4	14.6	-0.5	0.0	0.3
Prostate	Femoral head (left)	1 / 2 / 4	30.8	-11.9	8.8	0.0
	Femoral head (right)	1 / 2 / 4	31.5	-12.1	8.9	0.0
	Rectum (anterior)	5 / 2 / 5	61	-0.8	0.1	0.0
	Rectum (posterior)	5 / 2 / 5	25.8	-2.4	0.1	0.0

Finally, using only a single field each day increases the gEUD of the chiasm by another 0.3 Gy. We performed Student's t-test (one-sided, paired) to assess significant differences. Data in bold-face in Table 2 satisfies the following two constraints; p < 0.05 and at least 1 Gy difference with the previous column.

Chordoma group

Figure 2a shows the typical dose distribution for a cervical spine based chordoma tumor. Figure 2b shows DVHs of the left frontal lobe for a typical skull base chordoma patient. The main differences are in the 10-20 Gy region. For all normal tissues in the chordoma group the gEUD is increased by at least 1.0 Gy, and up to 3.8 Gy, when using field-set of the day treatment delivery rather than treating all fields every day. Treating only a single field each day for these patients, which is not clinical practice at our institute or any other institute that we know of, increases the gEUD of normal tissues by 0.3-1.2 Gy.

2).

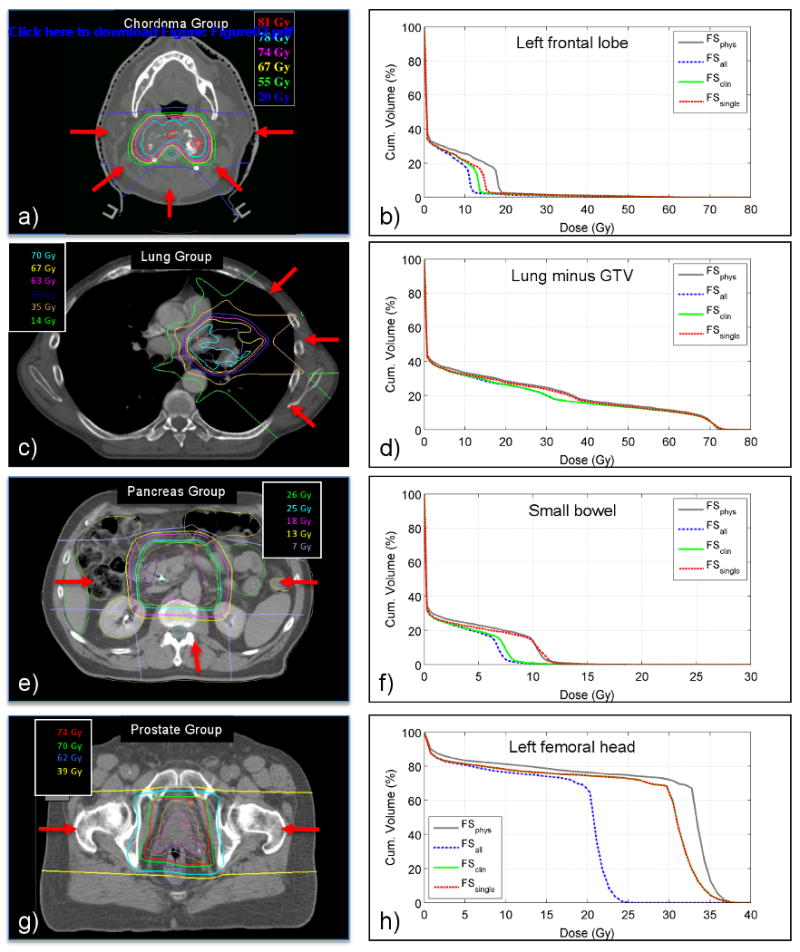
The left column shows typical dose distributions, not corrected for dose per fraction, for the Chordoma Group, Lung Group, Pancreas group and Prostate Group, respectively. Arrows indicate some of the beam directions used in the treatment plan. The right column shows DVHs for a single normal tissue of a typical patient of the patient group in the left column. These DVHs are shown as a function of the fractionation schedule.

Lung group

Figure 2c shows a typical proton radiotherapy dose distribution for a lung cancer patient showing that very limited dose is deposited in the contra-lateral lung. Figure 2d shows the DVHs of the lungs excluding the GTV. Only a minimal increase of 0.1 Gy in gEUD is observed between FS_all and FS_clin, see Table 2. Treating a single field each day, out of a total of three treatment fields, increases the gEUD of the esophagus and lung by 0.5 Gy, and of the heart by 0.2 Gy.

Pancreas group

Figure 2e shows the typical dose distribution for a pancreatic tumor. Figure 2f shows DVHs for the small bowel for a typical patient, for a reference fraction dose of D_ref = 5 Gy. The small difference in DVHs between FS_all and FS_clin does not result in an increase in gEUD (see Table 2). Delivering only a single field each day, on the other hand, results in a higher NTD for those parts of the normal tissue receiving a dose between about 5 and 15 Gy, expressed in an increase in gEUD of 0.5 Gy. DVHs for the small bowel, the kidneys, the stomach and the liver show similar variations as a function of fractionation schedule. The celiac axis and porta hepatis are typically entirely within the high dose region. They therefore receive full dose every day regardless the fractionation schedule and no difference in gEUD is noticed regardless the number of fields treated each day.

Prostate group

Figure 2g shows the typical dose distribution for proton radiotherapy of the prostate. For the left lateral and right lateral field treating the CTV, and also for the two fields treating the GTV, the high dose region is basically symmetric in the central sagittal plane. Being centered at the patient midline as well, the rectum receives an identical dose regardless the use of a left lateral or a right lateral field. Between patients the DVH of the anterior rectum varies but for each patient the DVH is similar regardless the fractionation schedule. Figure 2h shows the DVHs for the left femoral head for a typical patient. As the left femoral head is beyond the end of range for the right lateral fields, it will only be irradiated when a left lateral field treats the target. Typically the relative physical dose in the left femoral head for this left lateral field is about 85% (33 Gy out of 39 Gy delivered with this field). The relative volume of the femoral head receiving this dose varies from patient to patient depending on the location of the prostate with respect to the femoral head. There is a large difference in gEUD of the femoral head of almost 9 Gy between treating a single field each day or both lateral fields each day.

Integral NTD

Table 3 shows the integral NTD for each of the four fractionation schedules. For convenience we assumed α/β = 2 Gy and a = 1 for the entire patient while calculating the integral NTD over the available CT-data only. For the lung and pancreas group there is only a marginal difference between FS_all and FS_clin, while the difference between FS_clin and FS_single is almost 10%. For the chordoma and prostate group the first difference is almost 10% while the latter difference is marginal. For all groups the difference in integral NTD between treating a single field every day, and all fields every day, is roughly 10%.

Table 3.

Integral NTD, in percent, for each patient group and fractionation schedule. The integral NTD is determined over the extent of the CT-scan using α/β = 2 Gy and D_ref = 2 Gy. Values are averaged over all patients within a group, and subsequently normalized relative to the integral NTD for the physical dose distribution.

	Chordoma	Lung	Pancreas	Prostate
FSphys	100	100	100	100
FSall	75	85	83	84
FSclin	83	87	86	93
FSsingle	87	94	96	93

Sensitivity to model parameters

The NTD calculation depends on the α/β ratio, while the gEUD calculation depends on the model parameter ‘a’ (volume component). Because of the inherent uncertainty in both parameters we performed a sensitivity analysis, see Table 4. From equation 1 it follows that the difference between NTD and physical dose diminishes for increasing α/β ratio. For both the frontal lobe and the femoral head the difference in gEUD between FS_all and FS_clin, varies by almost a factor two as a when varying the α/β ratio (between 1.5 - 2.4 Gy and 4.8 – 8.8 Gy, respectively). Sensitivity of the gEUD to variation in parameter ‘a’ is larger for the frontal lobe in the chordoma group (between 1.0 – 2.7 Gy) than for the femoral head in the prostate group (between 8.2 – 8.9 Gy). This can be explained by the fact that the physical dose distribution in the femoral head is more homogeneous than for the frontal lobe.

Table 4.

Difference between gEUD for FS_all and FS_clin as a function of α/β-ratio and gEUD volume parameter ‘a’, both in absolute dose and as a percentage. The underlined values for each normal tissue corresponds with the α/β ratio as used in this work.

	α/β	a	FSall	FSclin	Diff. (Gy)	Diff. (%)
Chordoma group Frontal lobe (left)	1.0	5.0	16.1	18.5	2.4	13
	2.0	5.0	17.0	18.9	1.9	10
	3.5	5.0	17.7	19.2	1.5	8
	2.0	2.0	9.1	10.1	1.0	10
	2.0	5.0	17.0	18.9	1.9	10
	2.0	10.0	24.2	26.9	2.7	10
Prostate group Femoral head (left)	1.0	4.0	18.9	27.7	8.8	32
	2.0	4.0	21.9	28.5	6.6	23
	3.5	4.0	24.3	29.1	4.8	16
	1.0	2.0	18.0	26.2	8.2	31
	1.0	4.0	18.9	27.7	8.8	32
	1.0	6.0	19.4	28.3	8.9	31

Discussion

For our analysis we use two radiobiological models, the NTD model and the gEUD model. Not only do our results depend on the accuracy of the parameters used in each model, in a more general sense neither model has been sufficiently validated in clinical trials. Our results therefore should only be taken as an indication of what the biological effect may be of treating a field set of the day in proton radiotherapy. Our biological modelling furthermore does not employ a time component. The overal treatment time is unaffected by the number of fields treated each day.

The largest effect observed of field set of the day treatments is for the femoral head in the case of prostate cancer irradiation. Use of both lateral fields each day would result in approximately a 9 Gy reduction in gEUD of the femoral heads. As all dose levels for the femoral head (gEUD, FS_phys ≈ 33 Gy, FS_all ≈ 20 Gy, FS_clin ≈ 29 Gy) are substantially lower than the tolerance level of TD_5/5 = 52 Gy (7), this may not be a clinically relevant reduction. For the chordoma group the we observed an increase in gEUD of normal tissues between 1.6-3.8 Gy. Our in-house tolerances, in RBE-corrected physical dose for this specific patient group, are 67 Gy, 55 Gy and 62 Gy for the brainstem surface, the brainstem center and the chiasm, respectively. During clinical treatment planning the location of the CTV with respect to the brainstem and chiasm results in cumulative doses that are typically equal to or very close to their tolerance doses and taking into account dose per fraction effect may be important. The TD_5/5 for normal brain tissue 60, 50 or 45 Gy for irradiating 1/3, 2/3 or 3/3 of the brain (7). As the gEUD of the frontal and temporal lobes varies between 20 and 30 Gy depending on the fractionation schedule, an increase in gEUD of 1 to 2 Gy due to field set of the day delivery may not be clinically relevant. A study of cochlear dose at the University of Michigan shows a dose-response relationship even below 20 Gy (8). This relationship is steeper for the high frequency audible range.

For both the pancreatic cancer and lung cancer group the increase in dose to normal tissues due to field set of the day delivery is negligible, even when treating only a single field each day instead of two out of three fields.

The dose-per-fraction corrected integral NTD is between 2% and 9% lower when all fields are delivered every day (FS_all) compared to the clinically applied fractionation schedule (FS_clin). This may be beneficial in reducing the probability of secondary cancer induction (9). The reduction in integral NTD is, however, very small compared to the factor 2 or 3 decrease in integral dose of proton therapy compared to photon therapy, see e.g. (10). Please keep in mind that the integral NTD is calculated over the CT-scan only and that we applied a uniform value of α/β = 2 and a = 1 for the entire CT-scan.

The effect of variations in dose from fraction to fraction on the cumulative NTD incorporating radiobiologic considerations has been discussed by other authors. Van Herk et al. (11) modeled the effect of random and systematic errors on the biologically corrected cumulative dose distribution to the target, by means of a convolution method. Song et al. (12) discussed the accuracy of such a convolution method in determining the biologically corrected cumulative dose, both for the target and for normal tissue. Bortfeld et al. (3) used simple equations to show that dose variations up to 15% (1 standard deviation) result in a less than 1% variation in the NTD for the relevant spectrum of α/β parameters (i.e. α/β > 2 Gy). They conclude that another source of dose variation, “interplay effects” between leaf motion and target motion in IMRT, has negligible biologic consequences as the expected dose variation is less than 10% (1 standard deviation). Our study differs in that we investigate the biological consequence of the use of daily alternating sub-sets of fields in proton radiotherapy. Dose variation in our study is not an accidental by-product of the radiotherapy treatment delivery process but rather the result of a conscious decision on the part of the physician.

Random setup errors lead to a substantial increase in dose variation, and therefore to biological effects, for those points located in the penumbral region of any field. Field set of the day treatment delivery, on the other hand, affects those points in the penumbra as well as any other point inside the patient that receives dose. Only those points that are in the high dose region are not affected as they receive full dose for every fraction regardless the combination of treatment fields used.

The results and contemplations in our paper are mainly relevant to passive-scattered proton therapy, and other types of particle therapy, in which it is customary to treat only a (varying) subset of treatment fields of a (sub-)plan each day. “Blindly” converting the histogram of the cumulative 3D dose distribution or the entire treatment plan to NTD, gEUD or any other dose metric, may lead to inaccuracies. In our analysis FS_clin represents the actual gEUD, taking into account the clinically applied fractionation schedule. FS_phys, which overestimates the NTD to normal tissues, represents what the physicians see “on the screen” and what may be the basis for their clinical decision. FS_all, on the other hand, underestimates the NTD to normal tissues. When sending the cumulative DVH (physical dose) to a reporting center (such as RTOG) this may be what they determine to be the relevant dose metric. Knowledge and modeling of the exact treatment delivery schedule may be of importance in devising the best possible radiotherapy treatment for each individual patient, and may be important in accurately determining parameters for models of normal tissue complication probability (NTCP) based on patient group statistics.

Conclusions

The effects of field set of the day treatment delivery for the dose to normal tissues depend on the tumor site and number of fields treated each day. It may be necessary to take the exact treatment delivery schedule into account for designing the optimal patient treatment plan, and for maximum accuracy in correlating normal tissue complication rates with delivered doses.