Abstract
Background and objectives: Estimation of GFR in children from serum creatinine measures in regional databases is limited by a lack of height data. Furthermore, the ability of GFR estimating equations to quantify changes in GFR over time is unknown. Two methods of estimating GFR when height is unknown and the ability of several GFR equations to quantify GFR changes over time were evaluated.
Design, setting, participants & measurements: This retrospective study included 195 children (mean age 11.9 ± 4.6 years, GFR = 78.8 ± 34.5) who underwent iothalamate GFR, serum creatinine, and height measurements; 93 children underwent a second GFR measurement and 47 a third GFR measurement. Four equations were evaluated for bias and precision and for quantifying GFR change over time: (1) Schwartz, using measured height; (2) Schwartz, using estimated height (based on previous height percentile); (3) a locally derived modification of a previously derived height-independent equation.
Results: The Schwartz (measured height) displayed the least bias (−2 to +7%), followed by the modified height-independent equation and Schwartz (estimated height). All equations were imprecise. All equations performed similarly at capturing change in measured GFR over time, with no significant difference between estimated and measured GFR percentage change over time. The height-estimated Schwartz formula performed similarly to the height-measured Schwartz in all aspects of equation performance.
Conclusions: Pediatric GFR follow-up studies may be possible using height-independent equations. Estimating height from prior height measurements enhances GFR estimation when height is unknown. These findings will hopefully help advance future pediatric renal function database studies.
Research on long-term renal function monitoring in children has been hindered by difficulties in measuring changes in estimated GFR over time. Because serum creatinine (SCr) increases with growth and development, changes in SCr alone do not necessarily reflect changes in GFR. Therefore, in children, efforts must be made to measure GFR, or at least to estimate it, using GFR equations. Hospital and regional databases containing SCr data represent a potentially valuable source of data for assessment of long-term renal function outcomes (1,2). However, there are two obstacles for the use of such databases in children. First, most pediatric GFR equations require a height measurement concurrent with SCr; although height may often be recorded at an initial visit (hospital clinic or hospital admission), follow-up outpatient heights are generally unavailable in such databases. Second, although several studies have validated the ability of GFR equations to estimate GFR at a single time point, there is little known regarding the ability of GFR equations to accurately represent changes in GFR over time.
In this study, we evaluate the accuracy with which a commonly used height-dependent equation, the Schwartz formula (3), can quantify changes in GFR over time, and propose two options to facilitate the assessment of GFR change over time in children for whom complete height data are lacking. The first involves estimating height at follow-up time points from a measured height at the first assessment. We hypothesized that the accuracy of changes in GFR identified using the Schwartz formula with estimated follow-up heights would be comparable with those identified using the Schwartz formula with measured follow-up heights. The second option proposed involves the use of height-independent GFR equations, which we hypothesized would quantify GFR changes over time with similar accuracy to height-based equations. We also sought to validate the use of the recently derived updated simple Schwartz formula in our population of children with chronic kidney disease (CKD).
Materials and Methods
Design and Patients
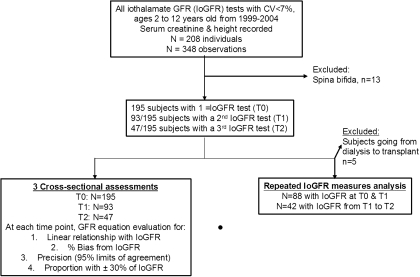
This was a retrospective cohort study from a previously constructed database (4) at the Montreal Children's Hospital, Canada. Figure 1 displays the study flow chart for subject selection. All children aged 2 to 18 years who had an iothalamate GFR (IoGFR) measurement performed at our center between 1999 and 2004 were eligible for inclusion. Children with spina bifida (because of the altered association between SCr and GFR) and those with a coefficient of variation for iothalamate clearance of >7% (poor IoGFR accuracy) were excluded. Children at our center underwent IoGFR measurement for either diagnosis or clinical follow-up of renal disease. There were 195 children in whom IoGFR was measured at least once; 93 subsequently underwent a second IoGFR measurement (median [interquartile range] time from first IoGFR = 13.2 [9.6] months, 10th and 90th percentile = 6.0 and 27.6 months, respectively), and 47 underwent a third IoGFR measurement (median [interquartile range] time from first IoGFR = 13.1 [9.6] months, 10th and 90th percentile = 6.0 and 24.0 months, respectively) during that time period. Repeated IoGFR testing was performed at the discretion of the attending nephrologist for follow-up of CKD progression. The time of the first or only GFR measure will be referred to as time 0 (T0), the second as time 1 (T1), and the third as time 2 (T2). The study was approved by the institutional Research Ethics Board.
Figure 1.
Study flow chart. Chart of the subject selection and exclusion strategy. T0, first IoGFR test; T1, second IoGFR test; T2, third IoGFR test.
GFR Measurement and Estimation
The methods used to determine IoGFR have been described elsewhere (5). Briefly, the IoGFR measurement method was performed by subcutaneous loading and constant infusion of iothalamate meglumine, followed by blood draw for iothalamate measurement (by HPLC) to calculate plasma disappearance. Height, weight, and SCr were measured on the same day as each IoGFR measurement. Height and weight were measured using a single stadiometer and scale (respectively) present in the clinical investigation unit, where IoGFR testing was performed. SCr was measured enzymatically, using the Vitros machine (Ortho-Clinical Diagnostics Inc., Mississauga, Ontario, Canada).
For every IoGFR measurement episode, GFR was estimated using equations. Estimated GFR (eGFR) by the recently updated Schwartz formula (shown in Table 1) was calculated, using height and SCr. Two additional methods to estimate GFR when height is not available were evaluated, as described below. The first method is based on equations previously derived by others, which are height-independent. The second method is based on simulating the scenario wherein height is known at an initial visit (from which GFR can be estimated using the standard Schwartz formula), but not at follow-up assessments (for example, using a database, where only SCr may be available).
Table 1.
GFR equations evaluated in this study
| Schwartz | 36.5 × (height [cm]/SCr [μmol/L]) |
| Modified BCCH | Inverse ln of: 8.067 + (1.034 × ln[1/SCr (μmol/L)]) + (0.305 × ln[age (years)]) + 0.064 if male |
| Height-estimated Schwartz | (1) Estimate height based on height percentile known from previous measurements |
| (2) Apply the Schwartz formula as above |
Schwartz, updated Schwartz formula using measured height; modified BCCH, height-independent BCCH formula re-derived from our sample population; height-estimated Schwartz, updated Schwartz formula using estimated heights at T1 or T2.
New Estimated GFR Method #1: Height-Independent GFR Equation
On the basis of a height-independent formula developed at the British Colombia Children's Hospital (the BCCH formula) (6), we derived a center-specific height-independent equation. The original BCCH formula includes SCr, age, and gender—but not height—and was derived from a sample of children with CKD using technetium-99m-diethylenetriaminepentaacetic acid (99mTc-DTPA) nuclear scan as the gold standard. We derived a modified version of the BCCH formula (modified BCCH) using data from the 195 patients with a T0 IoGFR measurement. The variables entered into the modified equation were the same as those included in the BCCH formula: 1/SCr, age, and gender (6). However, all variables were natural logarithm (ln)-transformed to improve the fit (3,4,7). Basic validation of this equation was verified by deriving an equation using a subgroup of 120 randomly selected patients and then evaluating the accuracy and precision of this equation in the remaining 75 patients. The subgroup validation equation was virtually identical to that derived using the entire group of 195 patients (not shown).
New Estimated GFR Method #2: Height Estimation Based on Previously Measured Height with Application of the Schwartz Formula
We used the first measured height (T0) to estimate height at the subsequent two time points to simulate the situation in which height is known at initial assessment, but not at times of subsequent SCr measurement. We first determined the gender-specific height-for-age percentile of each subject at T0 using the National Center for Health Statistics data (8). With use of the age and gender of each subject, and the assumption that each remained at the same height percentile at subsequent assessments, the heights at T1 and T2 were estimated. Agreement between estimated and measured heights at T1 and T2 was assessed using the Bland-Altman method. GFR was then estimated at T1 and T2 time points by the Schwartz formula, but with use of these estimated heights (referred to as height-estimated Schwartz).
Statistical Analyses
Continuous variables (which were mostly non–normally distributed) were described using median [interquartile range or 25th and 75th percentile]. Categorical variables were described using percentages (%). All analyses were conducted using Intercooled STATA version 10 (College Station, TX).
Cross-sectional Assessments of GFR Equation Performance
Agreement between each GFR equation and IoGFR was assessed in the following way. Using linear regression, we evaluated the linear relation between IoGFR and GFR estimated with each of the equations. The Bland-Altman method was used to investigate for bias (expressed as percentage of difference from IoGFR,) and precision of estimation (95% limits of agreement [LOA]). In addition, we determined the proportion of estimated GFRs to fall within ±30% and within ±10% of IoGFR. This assessment of GFR equation performance was performed for all three time points (T0, T1, and T2).
Evaluation of GFR Equations To Capture GFR Change over Time
We calculated the percentage of change in IoGFR and in eGFR by each formula from T0 to T1 and from T1 to T2 by dividing GFR change by GFR at initial assessment during the given time period. For example, percentage of change in GFR between T0 and T1 was calculated as [GFRT1 − GFRT0]/GFRT0 × 100. For these analyses, we excluded patients who underwent renal transplant between time periods (in whom GFR increase would be evident). The percentage of change in estimated GFR for each equation was compared with the percentage of change in IoGFR using paired t tests. Percentage of change in IoGFR was plotted against percentage of eGFR change to evaluate the correlation (r) between the two values. Mixed linear model analysis (including all observations and accounting for the fact that repeated values within patients were not independent, with patient identification number as the random effects variable) was used to determine whether there was a significant difference in percentage of GFR change between any of the equations and IoGFR at any of the time intervals.
Results
Subject characteristics at T0 to T2 are summarized in Table 2. Of 195 patients, 122 at T0 (62.6%) had CKD of various etiologies (including glomerular disease, cystic and dysplastic disease, obstruction, chemotherapy-induced toxicity, and cystinosis), 49 (25.1%) had kidney transplants, 16 (8.2%) had other organ transplantation, and 8 (4.1%) had other conditions.
Table 2.
Patients characteristics at time 0 (first GFR measurement), time 1, and time 2
| Time 0 (n = 195), Median (25th, 75th Percentiles) or n (%) | Time 1 (n = 93), Median (25th, 75th Percentiles) or n (%) | Time 2 (n = 47), Median (25th, 75th Percentiles) or n (%) | |
|---|---|---|---|
| Age (years) | 12.1 (8.2, 15.7) | 12.9 (8.3, 15.8) | 13 (9.1, 15.4) |
| Height (cm) | 140.4 (121.6, 159.4) | 140.6 (124.5, 155.7) | 140.0 (131.2, 160.1) |
| Height percentile | 15.5 (2.5, 50.3) | 15.7 (1.3, 39.2) | 15.0 (2.4, 40.5) |
| IoGFR ml/min per 1.73 m2 | 82 (54, 99) | 74 (46, 91) | 75 (42, 87) |
| Years since prior IoGFR | NA | 1.1 (0.8, 1.6) | 1.1 (0.9, 1.7) |
| Male | 119 (61.0) | 61 (65.6) | 34 (72.3) |
| Renal transplant | 49 (25.1) | 36 (38.7) | 17 (36.2) |
| Other transplant | 16 (8.2) | 36 (38.7) | 15 (31.9) |
| CKD evaluation or follow-up | 122 (62.6) | 16 (17.2) | 15 (31.9) |
| Other | 8 (4.1) | 5 (5.4) | 0 |
NA, not applicable.
Accuracy of Height Estimation
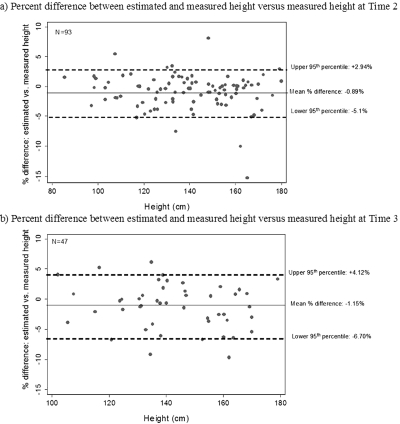
Figure 2 illustrates the mean percentage of difference between estimated and measured height at T1 and T2. Differences were very small: −0.89% (95% LOA + 2.9% to −5.1%) at T1 and −1.15% (95% LOA + 4.1% to −6.7%) at T2, confirming that our height estimation method was accurate and fairly precise in this cohort.
Figure 2.
Plots of percentage difference between estimated and measured height versus measured height, at time 1 (a) and time 2 (b). Center line: mean difference (negative indicates estimated height underestimates measured height). Upper and lower dotted lines: upper and lower 95% confidence interval of the percentage of difference.
Evaluation of GFR Estimating Equations at Three Cross-sectional Time Periods
Table 3 summarizes the performance characteristics of the height-measured Schwartz, the height-estimated Schwartz, and the modified BCCH equations at T0, T1, and T2. Median bias differed little between equations, although the modified BCCH tended to overestimate IoGFR and the height-measured and height-estimated Schwartz tended to underestimate IoGFR (median percentage of bias shown in Table 3). The R2 values describing the relationship between eGFR and IoGFR were 6 to 9% higher for both Schwartz equations compared with the modified BCCH equation (all P values <0.05).
Table 3.
Comparison of performance of the different GFR equations at initial (T0) and follow-up (T1, T2) visits
| GFR (ml/min per 1.73 m2), Median (25th, 75th Percentiles) | Relation to IoGFR (R2) | Median Percentage Bias (Range) (%) | 95% LOA (%) | Within 30% of IoGFR (%) | Within 10% of IoGFR (%) | |
|---|---|---|---|---|---|---|
| Time 0 (n = 195) | ||||||
| IoGFR | 82.0 (54.0, 99.0) | NA | NA | NA | NA | NA |
| height-measured Schwartz | 68.9 (52.6, 85.5) | 0.66 | −8.9 (−53.1, +61.0) | −52.2 to 38.4 | 76.4 | 29.7 |
| height-estimated Schwartz | NA | NA | NA | NA | NA | NA |
| modified BCCH | 79.2 (58.4, 93.7) | 0.61 | +3.8 (−50.6, +168.2) | −53.7 to 61.3 | 75.4 | 36.4 |
| Time 1 (n = 93) | ||||||
| IoGFR | 74 (46, 91) | NA | NA | NA | NA | NA |
| height-measured Schwartz | 65.0 (48.0, 84.0) | 0.71 | −3.2 (−55.1, +146.1) | −57.1 to 52.9 | 82.8 | 29.0 |
| height-estimated Schwartz | 65.0 (47.6, 82.5) | 0.71 | −3.9 (−56.8, +150.0) | −58.5 to 52.5 | 82.8 | 31.2 |
| modified BCCH | 72.8 (53.9, 96.4) | 0.67 | +7.0 (−51.0, +216.5) | −59.6 to 78.5 | 74.2 | 24.7 |
| Time 2 (n = 47) | ||||||
| IoGFR | 75.0 (42.0, 87.0) | NA | NA | NA | NA | NA |
| height-measured Schwartz | 65.0 (49.4, 83.2) | 0.85 | −9.5 (−49.7, +41.7) | −43.0 to 33.0 | 85.1 | 31.9 |
| height-estimated Schwartz | 64.6 (46.4, 81.9) | 0.83 | −10.5 (−48.8, 42.9) | −44.3 to 32.2% | 83.0 | 27.7 |
| modified BCCH | 68.3 (56.0, 91.7) | 0.77 | +2.1 (−44.2, +68.5) | −40.5 to 51.0% | 80.9 | 40.4 |
Height-measured Schwartz, updated Schwartz formula using measured height; BCCH, height-independent BCCH formula; modified BCCH, height-independent formula derived from our sample population; height-estimated Schwartz, updated Schwartz formula using estimated heights at T1 or T2. NA, not applicable.
Precision of estimation as assessed by the 95% LOA was best when using the height-measured Schwartz equation at all three time points, and extremely similar when using the height-estimated Schwartz equation at T1 and T2 (Table 3), being 17 to 21% narrower than the 95% LOA for the modified BCCH. The height-measured Schwartz equation estimated IoGFR within ±30% in 76% of patients at T0 and 85% at T2. The modified BCCH formula showed similar but slightly less accurate results (Table 3). All equations performed similarly at estimating GFR within ±10% of IoGFR.
Evaluating Percentage of Change in GFR over Time
Among 88 patients with IoGFR measured at T0 and T1 (excluding 5 patients who underwent renal transplantation), IoGFR decreased by a median of 7.5% between T0 and T1 (Table 4). Among the 42 patients with IoGFR measured at T1 and T2 (excluding those who underwent renal transplantation), IoGFR decreased by a median of 3.3% in the interval. Percentage of change in eGFR from T0 to T1 and from T1 to T2 for each equation is shown in Table 4. Percentage of change in eGFR did not statistically significantly differ from percentage of IoGFR change for any of the equations between any of the time points (paired t tests, all P values >0.05). In the mixed linear model analysis, including all equations at both time periods and accounting for time between GFR measurements, there was no significant difference in percentage of change over time for any of the eGFR equations, relative to IoGFR change over time.
Table 4.
Agreement between percentage of change in eGFR and percentage of change in IoGFR
| Percentage of GFR Change, Median (25th, 75th Percentiles)a | Paired t Test P Value for Difference between Percentage of eGFR Change and Percentage of IoGFR Change | |
|---|---|---|
| Time 0 to 1, n = 88 | ||
| IoGFR | −7.5 (−27.1, +10.4) | NA |
| height-measured Schwartz | −4.6 (−18.8, +8.4) | 0.73 |
| modified BCCH | −7.7 (−20.2, +7.4) | 0.99 |
| height-estimated Schwartz | −7.4 (−19.5, +8.6) | 0.78 |
| Time 1 to 2, n = 42 | ||
| IoGFR | −3.3 (−17.5, +13.6) | NA |
| height-measured Schwartz | −5.8 (−15.6, +13.1) | 0.54 |
| modified BCCH | −9.1 (−20.2, +11.1) | 0.56 |
| height-estimated Schwartz | −7.7 (−17.6, +12.2) | 0.43 |
Percentage of change between the two time periods: negative percentage of change indicates decrease in GFR over time.
Height-measured Schwartz, updated Schwartz formula using measured height; BCCH, BCCH formula; modified BCCH, height-independent formula derived from our sample population; height-estimated Schwartz, updated Schwartz formula using estimated heights at T1 or T2. NA, not applicable.
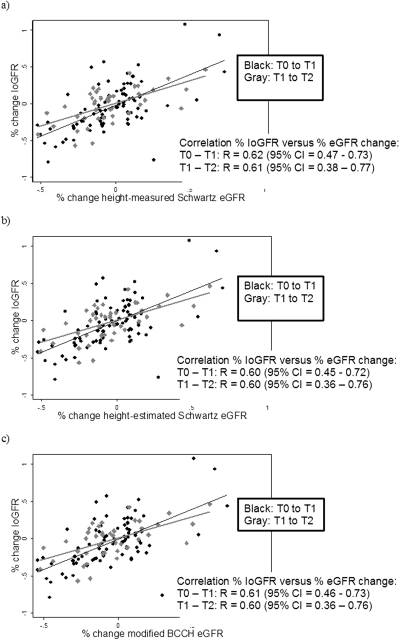
To further explore the relationship between percentage of change in IoGFR and percentage of change in eGFR between time periods, the scatter plots shown in Figure 3 were constructed. Percentage of change in eGFR by all three equations correlated well with percentage of change in IoGFR for both intervals (Pearson R values ranging from 0.60 to 0.62, with 95% confidence intervals shown in Figure 3, all P values <0.0001). There appeared to be a reasonable linear relationship between percentage of IoGFR change over time and percentage of eGFR change over time.
Figure 3.
Percentage of IoGFR change versus percentage of change in eGFR for each formula between each time period. Each graph contains the scatter plot and best fit line of the percentage of change in IoGFR versus percentage of change in eGFR (a) for the height-measured Schwartz, (b) for the height-estimated Schwartz, and (c) for the modified BCCH between two time periods. Time period T0 to T1 is plotted in black and time period T1 to T2 is plotted in gray. Pearson correlation coefficient “R” is displayed, with associated 95% confidence intervals.
Discussion
Our ability to perform database studies (using institutional or regional laboratory databases) on long-term renal function in children has been hampered by an inability to accurately estimate GFR from SCr measures because of the lack of available height measures. We proposed and validated two methods of quantifying change in renal function over time in children when measured heights are not available at every time point: height estimation from baseline height percentile, and a height-independent GFR equation. We have also validated the use of the recently updated Schwartz formula for estimating GFR in an external cohort of children with CKD.
An important finding of this study was that estimated heights agreed very closely with measured heights. As a result, height-estimated Schwartz GFR agreed well with height-measured Schwartz GFR, and changes in renal function over time were captured equally well when height was estimated compared with when height was measured at follow-up time points. This suggests that it is acceptable to use estimated height in GFR equations when measured height is unavailable. However, caution is advised when applying this method, which assumes that a child's height percentile does not change substantially over time. In the case of our cohort, height percentile did not change significantly over time. Accuracy maybe compromised in children with unusual growth patterns. This “height estimation” method should be validated in other populations and used with caution in groups of children at risk for growth retardation or in children using therapeutic growth hormone (who may cross up height percentiles).
Mattman et al. attempted to address the problem of missing height data by deriving a GFR equation (BCCH) including only age, gender, and SCr (6). This equation was never validated externally. Furthermore, a different gold standard GFR method was used in that study compared with this study. To validate the approach used by Mattman et al., we re-derived an equation with our own population, including the same prediction terms, with IoGFR as the gold standard. Because the relationships between GFR and each of age and 1/SCr are not linear, we ln-transformed these variables to improve the model fit (3,4,7). The modified BCCH equation performed with accuracy and precision similar to that achieved with the height-measured Schwartz formula. However, the modified BCCH equation should be validated in an external population before widespread application.
This study validates the recently updated Schwartz formula (3) in an external population of children. The updated Schwartz formula was derived from a large group of children with CKD (mean iohexol GFR = 41 ml/min per 1.73 m2) in a multicenter observational study in the United States and Canada (the CKiD study) (3). We have shown that this equation performs reasonably well at estimating GFR, at a population level, in children with earlier stages of CKD (mean IoGFR = 78.8 ml/min per 1.73 m2).
None of the equations estimated GFR with good precision. Even the best performing equation (updated Schwartz) had very wide 95% LOA, with estimated GFR as much as 57% lower to 53% higher than measured GFR. Caution is advised when using these equations for individual patient care.
An area of GFR estimation which has been largely ignored in the literature is the ability of equations to quantify changes in renal function over time. Abraham et al. (using the CKiD population) recently proposed a method whereby clinical parameters and knowledge of prior GFR can be used to improve estimation of GFR at follow-up time points (9). We have shown that, on a population level, the studied estimating equations quantify changes in renal function over time with reasonable accuracy and good correlation. This suggests that utilizing institutional or state laboratory databases (which contain SCr measurements and not height) to follow renal function over time may be possible, as is performed in adults. An area of research which has not been explored at all is the use of cystatin C–based equations for evaluating change in renal function over time, in comparison to SCr-based equations. Given that previous literature strongly suggests that cystatin C–based equations provide more accurate estimates of GFR when studied in a cross-sectional fashion (7,10), it is reasonable to hypothesize that they will also predict change over time with more accuracy, which would obviate the need for repeated height measurements. To prove this, a study performing prospective, repeated measurements over time of gold standard GFR, SCr, cystatin C, and height would be required, as is performed by the CKiD study. Hopefully, the CKiD study will elucidate upon this aspect of pediatric renal function monitoring. If cystatin C does in fact reflect change in GFR better than SCr does, this would support the more widespread use of cystatin C for renal function measurement in tertiary pediatric institutions.
This study has some limitations. First, although the cohort did contain patients with IoGFR >90 ml/min per 1.73 m2, most patients had some degree of renal dysfunction at baseline. It may be difficult to apply our findings to children with normal or near-normal renal function. Sample sizes at follow-up time points were small, resulting in poorer precision of the estimated parameters. Finally, data on growth hormone use were not available; a substantial portion of our population was likely receiving growth hormone. Introduction of growth hormone therapy between time points may have led to a crossing up of height percentiles, reducing the precision in height estimation at follow-up time points.
Conclusions
We have demonstrated that the updated Schwartz formula provides a valid estimate of GFR in groups of children with CKD, and is able to quantify changes in renal function over time with reasonable accuracy, at the population level. Furthermore, we have proposed two methods of estimating GFR when height is unknown at all time points which appear to be approximately as good as the height-measured Schwartz formula: the modified BCCH formula and estimation of height at follow-up time points from baseline height.
Disclosures
None.
Acknowledgments
Drs. Zappitelli and Foster, members of the McGill University Health Centre Research Institute (supported in part by the Fonds de la recherche en santé du Québec [FRSQ]), received salary support from the FRSQ. Dr. Zappitelli also holds a grant from the FRSQ and additional salary support from the Kidney Research Scientist Core Education and National Training Program and the McGill University Health Centre Research Institute.
Footnotes
Published online ahead of print. Publication date available at www.cjasn.org.
Access to UpToDate on-line is available for additional clinical information at http://www.cjasn.org/
References
- 1.Hemmelgarn BR, Clement F, Manns BJ, Klarenbach S, James MT, Ravani P, Pannu N, Ahmed SB, MacRae J, Scott-Douglas N, Jindal K, Quinn R, Culleton BF, Wiebe N, Krause R, Thorlacius L, Tonelli M: Overview of the Alberta Kidney Disease Network. BMC Nephrol 10: 30, 2009 [DOI] [PMC free article] [PubMed] [Google Scholar]
- 2.Philipneri MD, Rocca Rey LA, Schnitzler MA, Abbott KC, Brennan DC, Takemoto SK, Buchanan PM, Burroughs TE, Willoughby LM, Lentine KL: Delivery patterns of recommended chronic kidney disease care in clinical practice: Administrative claims-based analysis and systematic literature review. Clin Exp Nephrol 12: 41–52, 2008 [DOI] [PubMed] [Google Scholar]
- 3.Schwartz GJ, Munoz A, Schneider MF, Mak RH, Kaskel F, Warady BA, Furth SL: New equations to estimate GFR in children with CKD. J Am Soc Nephrol 20: 629–637, 2009 [DOI] [PMC free article] [PubMed] [Google Scholar]
- 4.Zappitelli M, Joseph L, Gupta IR, Bell L, Paradis G: Validation of child serum creatinine-based prediction equations for glomerular filtration rate. Pediatr Nephrol 22: 272–281, 2007 [DOI] [PubMed] [Google Scholar]
- 5.Sharma AK, Mills MS, Grey VL, Drummond KN: Infusion clearance of subcutaneous iothalamate versus standard renal clearance. Pediatr Nephrol 11: 711–713, 1997 [DOI] [PubMed] [Google Scholar]
- 6.Mattman A, Eintracht S, Mock T, Schick G, Seccombe DW, Hurley RM, White CT: Estimating pediatric glomerular filtration rates in the era of chronic kidney disease staging. J Am Soc Nephrol 17: 487–496, 2006 [DOI] [PubMed] [Google Scholar]
- 7.Zappitelli M, Parvex P, Joseph L, Paradis G, Grey V, Lau S, Bell L: Derivation and validation of cystatin C-based prediction equations for GFR in children. Am J Kidney Dis 48: 221–230, 2006 [DOI] [PubMed] [Google Scholar]
- 8.Kuczmarski RJ, Ogden CL, Grummer-Strawn LM, Flegal KM, Guo SS, Wei R, Mei Z, Curtin LR, Roche AF, Johnson CL: CDC growth charts: United States. Adv Data June8(314): 1–27, 2000 [PubMed] [Google Scholar]
- 9.Abraham AG, Schwartz GJ, Furth S, Warady BA, Munoz A: Longitudinal formulas to estimate GFR in children with CKD. Clin J Am Soc Nephrol 4: 1724–1730, 2009 [DOI] [PMC free article] [PubMed] [Google Scholar]
- 10.Filler G, Lepage N: Should the Schwartz formula for estimation of GFR be replaced by cystatin C formula? Pediatr Nephrol 18: 981–985, 2003 [DOI] [PubMed] [Google Scholar]