Skip to main content
PMC home page
NIHPA Author Manuscripts logoLink to NIHPA Author Manuscripts
. Author manuscript; available in PMC: 2010 Nov 13.
Published in final edited form as: J Am Soc Echocardiogr. 2008 Jul 14;21(9):1006–1009. doi: 10.1016/j.echo.2008.05.010

Assessing Mitral Valve Area and Orifice Geometry in Calcific Mitral Stenosis: A New Solution by Real-Time Three-Dimensional Echocardiography

John W Chu 1, Robert A Levine 1, Sarah Chua 1, Kian-Keong Poh 1, Eleanor Morris 1, Lanqi Hua 1, Thanh-Thao Ton-Nu 1, Judy Hung 1
PMCID: PMC2980787  NIHMSID: NIHMS250344  PMID: 18620839

Abstract

Background

Planimetry of mitral valve area (MVA) is difficult in calcific mitral stenosis (CaMS) in which limiting orifice is near the annulus, and unlike rheumatic mitral stenosis (RhMS), does not present an area for planimetry at the leaflet tips. Moreover, pressure half time (PHT)-derived MVA (MVAPHT) has limitations in patients with CaMS in whom there are coexisting conditions that affect LV chamber compliance. We tested the hypothesis that real-time 3-dimensional echocardiography (RT3D) can guide measurement at the narrowest orifice in CaMS.

Methods

In 34 patients with CaMS, MVA by RT3D (MVART3D) was obtained using a color-defined planimetry technique performed “en face” at the smallest annular orifice cross-section (diastolic maximum). MVART3D and MVAPHT were compared with an independent standard: MVA by continuity equation (MVACEQ). In a subgroup of 10 patients with CaMS or RhMS, the 3-dimensional shape of the stenotic mitral valve was examined, guided by color flow mapping.

Results

MVAPHT overestimated the mitral orifice area compared with MVACEQ (2.01 ± 0.52 cm2 vs 1.75 ± 0.46 cm2; P = .037), whereas there was no significant difference in MVART3D and MVACEQ (1.83 ± 0.52 cm2 vs 1.75 ± 0.46 cm2, respectively, P = .61). MVART3D had a greater correlation with MVA CEQ than MVAPHT (R = 0.86 vs 0.59 MVART3D vs MVAPHT, respectively). There was better agreement between MVA by RT3D and MVA by continuity equation than MVA by PHT and MVA by continuity equation (difference in MVA: 0.23 ± 0.15 cm2 vs 0.43 ± 0.29 cm2; P < .0001, MVART3D − MVACEQ vs MVAPHT − MVACEQ, respectively). In CaMS, there was a tubular geometry to the valve shape. In contrast, RhMS had a doming funnel-shaped geometry.

Conclusion

RT3D provides an accurate measurement of MVA in CaMS. In contrast with the doming valve shape present in RhMS, the limiting anatomic orifice area occurs at the annulus in CaMS as measured by RT3D and reflects the effective orifice area as present in a tubular valve geometry.

Keywords: Mitral stenosis, 3D echocardiography, Valvular disease

The reliable assessment of mitral valve area (MVA) is essential in the management of patients with mitral stenosis. Mitral annular calcification is a common condition that can result in calcific mitral stenosis (CaMS).1–3 CaMS occurs when there is extension of the annular calcification onto the mitral leaflets resulting in narrowing of the diastolic flow area.4,5 An accurate measurement of mitral orifice in CaMS remains problematic. In CaMS, which is associated with advanced age and coexisting left ventricular hypertrophy, coronary artery disease, and diabetes mellitus, the measurement of MVA by the pressure half-time (PHT) method is limited and often inaccurate because of frequently associated abnormal left ventricular compliance.6–8 Furthermore, direct planimetry of MVA, which is commonly applied in rheumatic mitral stenosis (RhMS), is difficult in CaMS where the limiting orifice is near the annulus and does not present an area for planimetry at the leaflet tips as in RhMS. The development of transthoracic real-time 3-dimensional echocardiography (RT3D) has the potential to display a unique “en-face” view of the complete mitral valve and its neighboring structures.9,10 Recent studies have shown that RT3D is a feasible, accurate, and highly reproducible technique for assessing MVA in RhMS, correlating well with invasive methods. 11,12 We aimed to investigate the usefulness of RT3D in measuring MVA in patients with CaMS at the limiting annular orifice by its ability to display an “en face” view of the mitral valve orifice as dictated by the color planimetry of the flow stream. MVA by RT3D (MVART3D) was compared with MVA by PHT (MVAPHT), and MVA derived from the continuity equation (MVACEQ) was used as an independent standard.

MATERIALS AND METHODS

Patients referred for an echocardiogram at Massachusetts General Hospital and identified to have CaMS were recruited in a prospective, consecutive manner. CaMS was defined echocardiographically as the presence of a bright echo-producing structure (calcification) located at the mitral annulus and posterior mitral valve leaflet on the parasternal long-axis and apical 4-chamber that extended into the base of the mitral leaflets, creating diastolic inflow obstruction.4,5 Patients with a minimum mean transmitral gradient of 4 mm Hg by Doppler and technically adequate image quality for RT3D processing were included in the study. Patients with significant (more than mild) mitral regurgitation or aortic regurgitation were excluded so that the continuity equation could be applied for obtaining the effective MVA as an independent standard. All patients had standard transthoracic 2-dimensional echocardiographic images using a Sonos 5500 or 7500 ultrasound machine (Philips Medical Systems, Bothell, WA) and an S3 probe (Philips Medical Systems) in parasternal and apical views. The transmitral gradient and the time velocity integral (TVIMV) were obtained by continuous-wave Doppler tracings of the maximal diastolic velocity across the mitral valve from the apical 4-chamber view. The PHT was obtained by dividing the peak velocity by √2 and measuring the time (in milliseconds) from the peak velocity to the point where this decrease was found.13,14 If there was a short early diastolic high velocity peak (ie, the early velocity descent was curvilinear rather than linear), this was omitted, and the PHT was derived by extrapolating the mid-diastolic linear descent backward.15 Three cardiac cycles for patients in sinus rhythm and 5 representative cycles for patients in atrial fibrillation were recorded, and their results were averaged for every patient.

RT3D was performed immediately or within 24 hours after the 2-dimensional study using a Sonos 7500 or an IE-33 ultrasound machine (Philips Medical Systems) and an X4 or X3 probe (Philips Medical Systems). By adjusting the mitral valve to the center of the screen, sector settings were optimized for image and color resolution. 3D pictures were acquired in real time using the “full-volume” mode, which forms a “pyramid” of data (90 × 85 degrees) from 4 to 7 sequential cardiac cycles. During acquisition, respiration was suspended to minimize motion artifact. All images were stored on a compact disk and transferred for offline analysis using QLAB Advanced Quantification Software, version 4.2 (Philips Medical Systems).

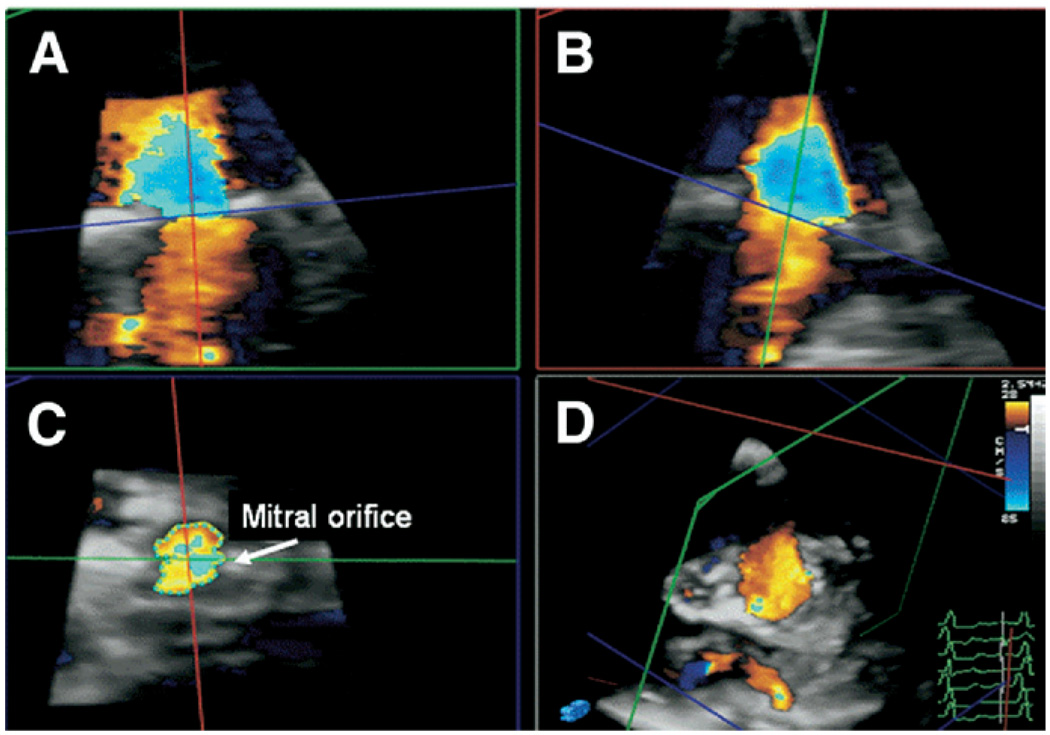
MVART3D was obtained by measuring the narrowest annular orifice viewed “en face” in the short-axis view. This was identified using 3 planes displayed simultaneously from the 3D color pyramid database (Qlab, Philips Medical Systems). Parameters were standardized with color gain set at the minimum (1%) and brightness set at 50%; color baseline was adjusted to observe the location of maximal flow acceleration at the annulus where color-defined orifice area was measured (Figure 1). In case of significant color signal dropout at the annulus because of heavy annular calcification, planimetry guided by color Doppler flow was then performed proximal to the annulus where significant change of flow velocity was detected. MVA derived from the pressure half-time method (MVAPHT) was calculated as:16 MVAPHT = 220/PHT.

Figure 1.

Figure 1

Open in a new tab

RT3D-guided color-defined planimetry of the MVA in CaMS. The RT3D data set (D) is cropped using simultaneous display of 3 imaging planes (A, B, C) to obtain MVA. Color figure online.

For an independent MVA standard, MVA derived from the continuity equation (MVACEQ) was calculated:17 MVACEQ = left ventricular outflow tract (LVOT) diameter2 × 0.785 × TVILVOT/TVIMV.17 Measurements of the LVOT diameter were obtained in the parasternal long-axis view. Measurements using the continuity equation were performed blinded to the MVART3D or MVAPHT data.

In a subgroup of 10 patients with CaMS and 10 patients with RhMS, the 3-dimensional shape of the stenotic mitral valve was determined using color Doppler flow mapping. This was performed to determine whether the valve geometry was consistent with a tubular flow stream in CaMS, which might be expected given the mechanism of narrowing occurring at the base of the leaflets in contrast with a funnel-shaped (doming) geometry such as occurs in RhMS. The 3D shape of the mitral valve was determined by assessing the valve in 3 views displayed simultaneously. A tubular shape was defined as having a similar area at the mitral annulus and leaflet tips, whereas a doming funnel shape was defined as a narrowing of the valve area at the leaflets tips compared with the base. RhMS was defined as thickening and calcification of leaflets and chordae, and fusion of the mitral commissures with decreased leaflet tip motion and opening.18,19

Measurements of MVART3D, MVAPHT, and MVACEQ were performed independently and blinded to the results of the other MVA measurements. For interobserver and intraobserver variability: RT3D images of 15 different patients were analyzed at different times by 3 independent observers. The same images were also analyzed on a different day by one of these same observers. Variability was evaluated as the standard deviation of the differences in MVART3D expressed as the percent of mean MVART3D.

Statistical Analysis

Continuous variables were expressed as the mean (± standard deviation) and compared by the Student t test. Statistical analysis of the association of variables was performed with the Pearson correlation coefficient. Agreement between RTMVART3D and MVAPHT was compared with MVA by continuity equation as assessed by Bland-Altman Plots.20 Comparisons were considered significant with a P value < .05.

RESULTS

Fifty patients were screened, with 16 patients excluded because of poor image quality (n = 4), greater than mild mitral regurgitation (n = 8), or a mean gradient of less than 4 mm Hg (n = 4). Thirty-four patients with CaMS were examined for this study. The mean age was 72 ± 13 years, and 82% were female. The majority of patients (80%) had a history of hypertension. Approximately one third of patients had a history of coronary artery disease (36%), aortic stenosis (36%) (aortic stenosis was defined as a valve area < 1.5 cm2), or diabetes mellitus (32%). Only 4% of patients had none of these diagnoses in their medical history. The peak and mean transmitral gradients were 16.4 ± 7.4 mm Hg and 7.6 ± 3.8 mm Hg, respectively.

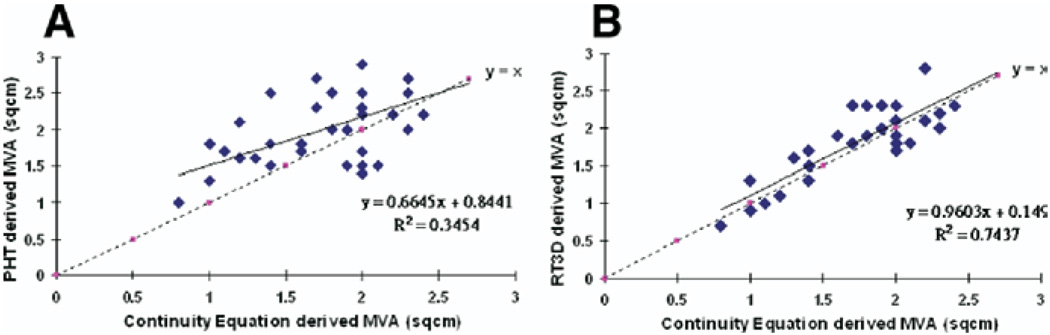
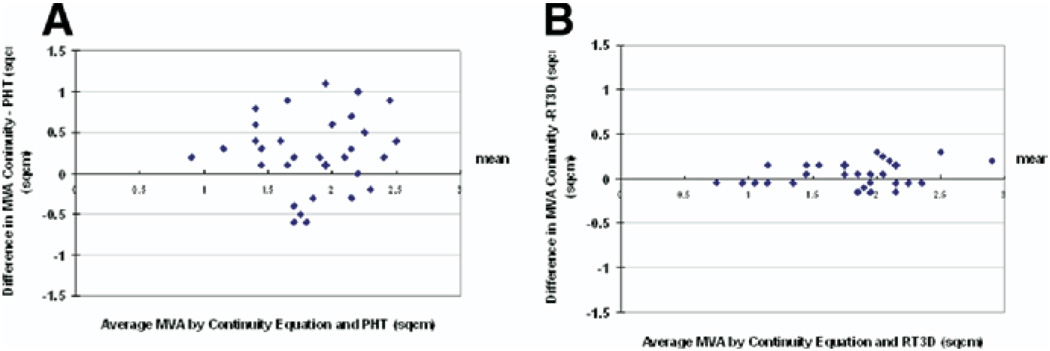
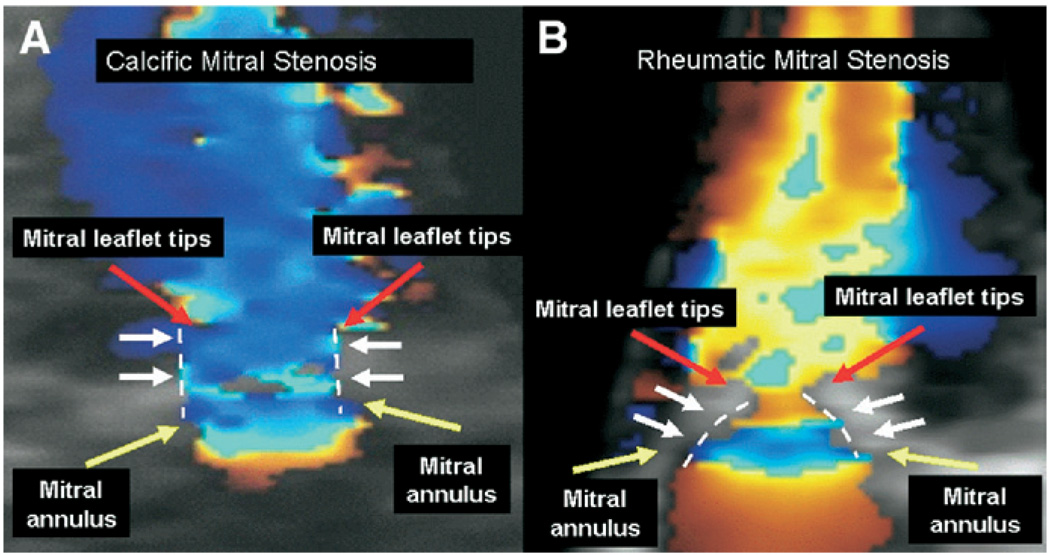
In CaMS, MVAPHT overestimated the MVA compared with MVACEQ (2.01 ± 0.52 cm2 vs 1.75 ± 0.46 cm2; P = .037), whereas there was no significant difference in MVART3D and MVACEQ (1.83 ± 0.52 cm2 vs 1.75 ± 0.46 cm2, respectively, P = .61). MVART3D had a greater correlation correlated with MVACEQ than MVAPHT (R = 0.86 vs 0.58; MVART3D vs MVA PHT) (Figure 2). In addition, there was better agreement between MVART3D and MVACEQ, with a mean difference in MVA of 0.23 ± 0.15 cm2 between MVART3D and MVACEQ compared with a mean difference of 0.43 ± 0.29 cm2 for MVAPHT and MVACEQ; P = .0007 (Figure 3). The shape of the mitral valve at the annulus and leaflet tips was similar for CaMS, corresponding to a tubular geometry of the stenotic orifice. In contrast, in RhMS, there was a funnel-shaped geometry to the valve (Figure 4). Intraobserver and interobserver variability of RT3D MVA measurements was 5% and 8%, respectively.

Figure 2.

Figure 2

Open in a new tab

Correlation of MVA by PHT method (A) and real-time 3D (B) to that of continuity equation. Color figure online.

Figure 3.

Figure 3

Open in a new tab

Bland-Altman plots of MVA by PHT method (A) and real-time 3D (B) compared with continuity equation. Color figure online.

Figure 4.

Figure 4

Open in a new tab

Geometry of the mitral valve in CaMS and RhMS. CaMS displays a tubular geometry (A) compared with the doming pattern seen in RhMS (B). Yellow and red arrows outline the mitral annulus and leaflet tips; white arrows and dashed lines outline the geometry of the valve. Color figure online.

DISCUSSION

We found that direct assessment of MVA by real-time 3-dimensional color Doppler echocardiography is able to accurately measure the limiting mitral annular orifice area in CaMS and correlated well with the MVA obtained by the continuity equation method, which is an independent standard for measuring the effective MVA. MVA by color-guided planimetry using RT3D had a greater correlation and agreement to MVA by continuity equation than MVA by PHT.

The Doppler PHT method for calculating MVA has limitations in patients with CaMS because these patients are usually elderly with a significant percentage having a history of systemic hypertension, coronary artery disease, aortic valve stenosis, or left ventricular hypertrophy. Increased left ventricular stiffness has been reported in patients with aortic stenosis and coronary artery disease;21 therefore, patients with CaMS likely have impaired left ventricular compliance and thus altered left atrial to left ventricular pressure difference. This in turn has effects on the accuracy of the PHT measurement.22,23 The PHT would be shorter as a result of a more rapid equilibration of left atrial and ventricular pressure with impaired left ventricular compliance, resulting in an overestimation of the derived MVA, which was our experience in this study. Although 2-dimensional planimetry is widely accepted as an accurate method for measuring the valvular orifice in RhMS, this method has limitations particularly in regard to positioning the correct image plane orientation. This is even more pronounced in CaMS because the limiting orifice is near the annulus and practically does not present an area for planimetry at the leaflet tips as in RhMS. RT3D overcomes these limitations and improves the ability of the operators to perform an appropriately orientated and well-defined planimetry of the mitral orifice area in CaMS by its ability to manipulate the cropping plane to measure the narrowest “en face” area in short axis. This 3D perspective of intersecting views is necessary to locate and standardize the appropriate view and is unavailable in standard transthoracic 2-dimensional imaging. In addition, 3D color flow Doppler imaging offers better delineation of the annular flow area and thus more accurate assessment of the orifice area. In clinical practice, patients with moderate or severe CaMS often have a significant degree of mitral regurgitation, probably because of increased rigidity of the mitral annulus, which renders MVA calculation by the continuity equation not applicable. The findings of the present study therefore show that RT3D is a useful noninvasive tool for measuring MVA. RT3D color-planimetry measurements were highly reproducible with excellent interobserver and intraobserver variability.

Three-dimensional Geometry of the Calcific Mitral Valve

The principle of fluid mechanics states that flow, directed to converge toward a narrow orifice, will continue to converge beyond the orifice until its convergence is blunted by interaction with surrounding fluid. The contraction coefficient of the orifice of the valve is defined as the effective orifice area at the vena contracta (the smallest cross-sectional area encountered by flow) divided by the anatomic area.24,25 Previous work has demonstrated that the contraction coefficient and the related net pressure loss are affected by the variation in leaflet geometry in patients with mitral stenosis.19 In the CaMS group, RT3D demonstrated no apparent contraction of the flow stream distal to the limiting mitral orifice by color flow Doppler images compatible with a collimated flow stream reflecting the tubular geometry of the limiting mitral orifice in CaMS, whereas in the RhMS group, there was a doming funnel leaflet geometry. The finding of a tubular geometry in CaMS has several important clinical implications. Gilon et al,26 using 3D echocardiographic laser stereolithography, demonstrated that changes in pressure gradient across a stenotic valve depend not only on its limiting orifice area but also on its 3D geometry proximal to the orifice. For the same anatomic area and flow rate, the pressure gradient was higher for a flat valve shape (smaller contraction coefficient of 0.6) compared with that of a dome funnel shape (larger contraction coefficient of 0.8) by up to 40%. By extrapolating these concepts, the pressure gradient across the stenotic orifice would be even lower in CaMS for the same anatomic orifice area because with a tubular geometry the contraction coefficient is approximately 1. This finding suggests that CaMS, which is a common finding in clinical practice, is not generally associated with clinically important flow obstruction. Moreover, because the anatomic and effective orifice area is essentially equal, the Gorlin’s equation derived MVA (a measure of effective orifice area) practically represents the anatomic limiting orifice area and vice versa. Thus, RT3D color planimetry effectively can supplant invasive evaluation.

Study Limitations

Accurate delineation of the MVA by RT3D is dependent on the quality of the images. The current size of matrix phase-array probes may limit adequate acoustic windows for optimal 3D images even to experienced operators. However, the development of newer generation matrix-phase array probes should improve frame rate and thus image quality.

The patient population examined was a selected one because 16 of 50 patients screened were excluded for various reasons based on predetermined criteria, and the results presented may not apply for all patients with CaMS.

The independent standard used in this study did not involve an invasive method such as the MVA calculated by the Gorlin equation used in catheterization laboratories. However, the Gorlin equation is essentially derived from the continuity principle; this principle was also applied using noninvasive Doppler quantification methods as an independent comparison in this study. Using a noninvasive independent standard also limited comparison variability inherent when comparing a method obtained invasively in the catheterization laboratory under sedated conditions with methods obtained noninvasively without sedation.

CONCLUSIONS

RT3D provides an accurate measurement of MVA in CaMS. In contrast with doming RhMS, the limiting anatomic orifice area occurs at the annulus in CaMS, as measured by RT3D, and reflects the effective orifice area as present in a tubular valve geometry.

Acknowledgments

Dr John W. Chu was supported in part by a grant from the National Heart Foundation of New Zealand, Auckland, New Zealand. This work was also supported in part by K23HL004504 and R21EB005294 (J.H.) and R01HL072265 (R.A.L.).

Footnotes

There are no conflicts to declare.

REFERENCES

  • 1.Geill T. Calcification of the left annulus fibrosus (230) cases. Acta Med Scand Suppl. 1950;239:153. [PubMed] [Google Scholar]
  • 2.Simon MA, Liu SF. Calcification of the mitral valve annulus and its relation to functional valvular disturbance. Am Heart J. 1954;48:497. doi: 10.1016/0002-8703(54)90116-7. [DOI] [PubMed] [Google Scholar]
  • 3.Kirk RS, Russell JGB. Subvalvular calcification of mitral valve. Br Heart J. 1969;31:684. doi: 10.1136/hrt.31.6.684. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 4.Otto C. The practice of clinical echocardiography. 3rd ed. Philadelphia: WB Saunders; 2004. [Google Scholar]
  • 5.Weyman AE. Principles and practices of echocardiography. 2nd ed. Boston: Lea & Febiger; 1994. [Google Scholar]
  • 6.Fulkerson PK, Beaver BM, Auseon JC, et al. Calcification of the mitral annulus: etiology, clinical associations, complications and therapy. Am J Med. 1979;66:967–977. doi: 10.1016/0002-9343(79)90452-2. [DOI] [PubMed] [Google Scholar]
  • 7.Waller BF. The old-age heart: normal aging changes which can produce or mimic cardiac disease. Clin Cardiol. 1988;11:513–517. doi: 10.1002/clc.4960110802. [DOI] [PubMed] [Google Scholar]
  • 8.Chu J, Hung J, Levine RA. Pressure half-time for calculating mitral valve area: Is it valid in patients with calcific mitral stenosis? J Am Soc Echocardiogr. 2005;18:550. [Google Scholar]
  • 9.Ota T, Kisslo J, von Ramm OT, et al. Real-time, volumetric echocardiography: usefulness of volumetric scanning for the assessment of cardiac volume and function. J Cardiol. 2001;37 Suppl I:93–101. [PubMed] [Google Scholar]
  • 10.Ahmad M. Real-time three-dimensional echocardiography in assessment of heart disease. Echocardiography. 2001;18:73–77. doi: 10.1046/j.1540-8175.2001.00073.x. [DOI] [PubMed] [Google Scholar]
  • 11.Zamorano J, Cordeiro P, Sugeng L, et al. Real-time three-dimensional echocardiography for rheumatic mitral valve stenosis evaluation: an accurate and novel approach. J Am Coll Cardiol. 2004;43:2091–2096. doi: 10.1016/j.jacc.2004.01.046. [DOI] [PubMed] [Google Scholar]
  • 12.Sebag IA, Morgan JG, Handschumacher MD, et al. Usefulness of three-dimensionally guided assessment of mitral stenosis using matrix-array ultrasound. Am J Cardiol. 2005;96:1151–1156. doi: 10.1016/j.amjcard.2005.06.046. [DOI] [PubMed] [Google Scholar]
  • 13.Thuillez C, Theroux P, Bourassa MG, et al. Pulsed Doppler echocardiographic study of mitral stenosis. Circulation. 1980;61:381–387. doi: 10.1161/01.cir.61.2.381. [DOI] [PubMed] [Google Scholar]
  • 14.Hatle L, Angelsen B, Tromsdal A. Noninvasive assessment of atrioventricular pressure half time by Doppler ultrasound. Circulation. 1979;60:1096–1104. doi: 10.1161/01.cir.60.5.1096. [DOI] [PubMed] [Google Scholar]
  • 15.Quinones MA, Otto CM, Stoddard M, et al. Recommendations for quantification of Doppler echocardiography: a report from the Doppler Quantification Task Force of the Nomenclature and Standards Committee of the American society of Echocardiography. J Am Soc Echocardiogr. 2002;15:167–184. doi: 10.1067/mje.2002.120202. [DOI] [PubMed] [Google Scholar]
  • 16.Hatle L, Angelsen B. Doppler ultrasound in cardiology: physical principles and clinical applications. 1985:115–122. [Google Scholar]
  • 17.Oh J, Seward JB, Tajik AJ. The echo manual. 2nd ed. Philadelphia: Lippincott-Raven; 1999. [Google Scholar]
  • 18.Nichol PM, Gilbert BW, Kissio JA. Two-dimensional echocardiographic assessment of mitral stenosis. Circulation. 1977;55:120–128. doi: 10.1161/01.cir.55.1.120. [DOI] [PubMed] [Google Scholar]
  • 19.Martin RP, Rakowski H, Kleiman JH, et al. Reliability and reproducibility of two dimensional echocardiograph measurement of the stenotic mitral valve orifice area. Am J Cardiol. 1979;43:560–568. doi: 10.1016/0002-9149(79)90014-6. [DOI] [PubMed] [Google Scholar]
  • 20.Bland JA, Altman DG. Statistical methods for assessing agreement between two methods of clinical measurement. Lancet. 1986;1:307–310. [PubMed] [Google Scholar]
  • 21.Peterson KL, Tsuji J, Johnson A, et al. Diastolic left ventricular pressure-volume and stress-strain relations in patients with valvular aortic stenosis and left ventricular hypertrophy. Circulation. 1978;58:77–89. doi: 10.1161/01.cir.58.1.77. [DOI] [PubMed] [Google Scholar]
  • 22.Flachskampf F, Weyman AE, Guererro JL, et al. Influence of orifice geometry and flow rate on effective valve area: an in vitro study. J Am Coll Cardiol. 1990;15:1173–1180. doi: 10.1016/0735-1097(90)90260-v. [DOI] [PubMed] [Google Scholar]
  • 23.Thomas J, Weyman AE. Doppler mitral pressure half-time: a clinical tool in search of theoretical justification. J Am Coll Cardiol. 1987;10:923–929. doi: 10.1016/s0735-1097(87)80290-5. [DOI] [PubMed] [Google Scholar]
  • 24.Fox R, McDonald TA. Introduction to fluid mechanics. 2nd ed. New York, NY: John Wiley & Sons; 1978. [Google Scholar]
  • 25.Daughtery R, Franzini JB, Finnemore EJ. Fluid mechanics with engineering applications. New York, NY: McGraw-Hill; 1985. [Google Scholar]
  • 26.Gilon D, Cape EG, Handschumacher MD, et al. Insights from three-dimensional echocardiographic laser stereolithography. Effect of leaflet funnel geometry on the coefficient of orifice contraction, pressure loss and the Gorlin formula in mitral stenosis. Circulation. 1996;94:452–459. doi: 10.1161/01.cir.94.3.452. [DOI] [PubMed] [Google Scholar]

RESOURCES