Kin competition, natal dispersal and the moulding of senescence by natural selection

Abstract

Most theoretical models for the evolution of senescence have assumed a very large, well mixed population. Here, we investigate how limited dispersal and kin competition might influence the evolution of ageing by deriving indicators of the force of selection, similar to Hamilton (Hamilton 1966 J. Theor. Biol. 12, 12–45). Our analytical model describes how the strength of selection on survival and fecundity changes with age in a patchy population, where adults are territorial and a fraction of juveniles disperse between territories. Both parent–offspring competition and sib competition then affect selection on age-specific life-history traits. Kin competition reduces the strength of selection on survival. Mutations increasing mortality in some age classes can even be favoured by selection, but only when fecundity deteriorates rapidly with age. Population structure arising from limited dispersal however selects for a broader distribution of reproduction over the lifetime, potentially slowing down reproductive senescence. The antagonistic effects of limited dispersal on age schedules of fecundity and mortality cast doubts on the generality of conditions allowing the evolution of ‘suicide genes’ that increase mortality rates without other direct pleiotropic effects. More generally, our model illustrates how limited dispersal and social interactions can indirectly produce patterns of antagonistic pleiotropy affecting vital rates at different ages.

1. Introduction

Hamilton (1966) laid the formal foundations for mathematical theory of the evolution of ageing (Rose et al. 2007). His insight was (i) to use the Malthusian population growth rate as an adequate measure of fitness; (ii) to consider mutations with variable effects on different age classes; and (iii) to measure the force of selection acting on such mutations by computing sensitivities of population growth rate to changes in age-specific mortality or fecundity, using standard models for the dynamics of age-structured populations. Hamilton (1966) showed that such indicators of the force of selection would decline monotonically with age, thus lending mathematical rigour to earlier ideas that the vanishing force of selection could provide a major evolutionary explanation for ageing (Fisher 1930; Haldane 1941; Medawar 1946, 1952; Williams 1957; see also Rose 1991; Charlesworth 2000 for historical perspectives).

Much of the mathematical theory developed after the seminal work of Hamilton (1966) incorporated his scaling of the forces of natural selection in more explicit population genetics models, examining, for instance, patterns of genetic variation and covariation among life-history traits with age, or the effect of non-random mating and sex differences in vital statistics (see Charlesworth 1994; and a review in Rose et al. 2007). In parallel, some authors set out to incorporate more ecological realism in Hamilton's theoretical framework, relaxing the assumptions of density and condition independence of vital rates, and examining the effect of competition within and between age classes (Abrams 1993; Williams & Day 2003; Seymour & Doncaster 2007; Bonsall & Mangel 2009). This more derived eco-evolutionary theory of senescence helped in particular to better explain the diversity of ageing patterns observed in nature (Reznick et al. 2004; Williams et al. 2006).

Despite increasing ecological realism, most theory for the evolution of senescence has assumed that populations are large and well-mixed, with no preferential interactions among kin (but see Libertini 1988). Ironically, it was Hamilton's work on the very problem of kin interactions (Hamilton 1964) that led to fundamental changes in our understanding of the role of dispersal and kin interaction in shaping the evolution of behaviour in viscous, spatially structured populations (Lehmann & Keller 2006). Hamilton himself invoked kin selection arguments in his 1966 contribution to explain discrepancies between life tables in human populations and his measure of the strength of selection, but he did not formalize such arguments (see also Hamilton 1996). Kin selection should, in principle, affect the evolution of ageing in viscous populations because an individual's longevity and fecundity is likely to affect the vital rates of her relatives (Bourke 2007).

There has recently been increasing recognition that kin selection may affect the evolution of ageing (reviewed by Bourke 2007). As proposed by Bourke (2007), we can distinguish two classes of models among formal studies on the effect of kin selection on ageing. The first class of models studies situations where increased longevity of a focal individual provides fitness benefits for its relatives (see e.g. the model of intergenerational transfers by Lee 2003; or models with parental care, Pavard et al. 2007), with a strong focus on ageing patterns in our own species. The second situation is when, on the contrary, increased longevity or fecundity of a focal individual reduces the fitness of its relatives. The present study belongs to this second class. With both of Hamilton's early contributions in mind, here we set out to further our understanding of how limited dispersal and kin competition might affect the evolution of ageing.

Previous studies have already made some headway on this problem. Assuming strong parent–offspring competition, Ronce et al. (1998) found that actuarial senescence favoured the evolution of reduced rates of dispersal with increasing maternal age. Drawing the causal arrow in the other direction, Pen (2000) showed that limited juvenile dispersal should favour individuals who shift their allocation from survival to reproduction (but see Lion 2010). Using individual-based simulations, Travis and collaborators (Travis 2004; Dytham & Travis 2006) have found that shorter dispersal distances favour the evolution of shorter lifespan even in the absence of a trade-off between survival and fecundity (see also Mitteldorf 2006). Kirchner & Roy (1999) similarly found that reduced longevity evolved in highly genetically structured metapopulations because of the epidemiological consequences of different life histories. Cant & Johnstone (2008) found that sex-biased dispersal and competition among females within families could lead to reproductive cessation in older females. Here, we aim to put these results in a broader framework, deriving analytical indicators of the force of selection, similar to Hamilton (1966), but in the presence of limited dispersal and kin competition. More precisely, we examine the effect of parent–offspring and sib competition on such indicators using a simple model of a spatially structured population similar to Hamilton & May (1977) and Ronce et al. (1998).

2. Material and Methods

(a). An indicator of the force of selection

Consider a mutation that affects some phenotypic trait, with pleiotropic effects on age-specific survival rates and fecundities. The phenotypic trait could be, for instance, the concentration of some metabolic enzyme. Wild-type individuals (A) express the enzyme at a concentration z, while carriers of the mutant allele a express the enzyme at a concentration z + δ. Following Hamilton (1966), we derive an indicator of the force of selection acting on the mutant allele a as the derivative of the growth rate of the mutant population, λ, with respect to the phenotypic effect of the mutation, δ. Here, we focus on density-regulated populations where the vital rates of individuals vary in response to the phenotypes of their competitors: λ denotes the initial growth rate of a mutant allele when confronted with the wild-type allele. We first derive general results without making any assumption about the specific effects of δ on the age schedules of birth and death. We will consider mutations of weak effects (small δ) and will concentrate on first-order effects of selection (Rousset & Billiard 2000). In an age-structured population (see also Taylor & Frank 1996; Caswell 2001), the intensity of selection can then be decomposed as:

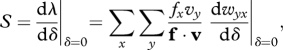2.1

where f and v are, respectively, the vector of asymptotic frequencies and the vector of reproductive values for the different age classes in the wild-type population, and w_yx is the contribution of an individual of age x with the mutant allele to the production of individuals of age y with the mutant allele. The sign of S predicts whether selection favours the mutant allele over the wild-type allele. When S < 0, then the mutant allele has a lower fixation probability than the wild-type (conversely, the mutant has a higher fixation probability than the wild-type when S > 0). In addition to its sign, the magnitude of S conveys important information for the evolution of senescence since it measures the strength of selection opposing the accumulation of deleterious mutations (i.e. those mutations with S < 0).

(b). The life cycle

We are interested in the effects of kin competition on the moulding of senescence by natural selection. We therefore focus on a simple life cycle where kin competition effects are very strong, as in Hamilton & May (1977) or Ronce et al. (1998). Consider a very large population of clonal organisms with seasonal reproduction. We distinguish two main stages in the life cycle—a mobile (or dispersing) stage, which lasts from birth to the next breeding season, and a sessile (or territorial) stage thereafter. The life cycle could thus describe that of plants, of sessile animals with a mobile larval phase or of strongly territorial animals with natal dispersal. There are an infinite number of sites or territories, each occupied by a single sessile individual. Each adult produces a very large number of clonal, mobile juveniles, a proportion d of which disperse, and a proportion 1−d that stay in their natal site. A fraction c of the dispersing juveniles dies. The surviving dispersers are distributed evenly among all sites (infinite island model of dispersal). The mobile juveniles compete to establish in sites freed by the death of their previous sessile occupants, with a fair lottery model of competition among all mobile juveniles present in a site after dispersal. Juveniles that fail to establish during their first season die. The probability of surviving to the next breeding season, p(x), and the fecundity, b(x), of sessile individuals vary with their age x. Mutations may affect age-specific survival rates p(x) and fecundities b(x) at any age but do not affect the dispersal rate of juveniles.

Our model assumes strong and asymmetric competition between mobile juveniles and sessile adults, such that juveniles can never displace a resident adult. As a result, the expected number of recruited offspring for an individual with age x is not only limited by its own offspring production, but also by the mortality of adults in the population. More precisely, we define the age-specific effective fecundity of an adult as the expected number of juveniles born to an individual at age x that will survive until the end of the season:

2.2

Here, g(x) is the probability that a mobile juvenile establishes itself after dispersal in a site previously occupied by a sessile individual of age x, and 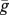is the mean probability of establishment for mobile juveniles. Effective fertility 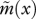is the product of the number of offspring produced (b(x)) and the probability that these offspring settle into a site. This latter probability is composed of two terms: the first term is the probability that a non-dispersing offspring settles in its natal site, and the second term is the probability that an offspring disperses, survives the dispersal event and settles successfully in another site. The probability of establishment in a site previously occupied by a sessile individual with age x is:

2.3

where 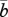is the mean fecundity of sessile individuals in the population (see electronic supplementary material, appendix). This probability decreases with increasing fecundity (stronger competition among juveniles) and increasing survival (stronger competition with adults) of the resident adult. Equations (2.2 and 2.3) thus show that, with limited juvenile dispersal, the effective fecundity of an adult at age x trades off against its survival probability. As the dispersal rate of her offspring decreases, the effective fecundity of a given adult depends more on its own mortality probability and less on that of other adults in the population.

For reference, we define m(x) as the effective fecundity in a population with complete dispersal of juveniles and the same age structure as our focal population:

2.4

where 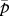is the mean survival probability of sessile individuals. Note that, in the case of complete dispersal, the effective fecundity does not depend on the dispersal cost. To facilitate the analysis of the effects of limited dispersal, we re-express the effective fecundity in the general case as:

2.5

where

2.6

is the ratio of the probability of establishment for a mobile juvenile present after dispersal in a site occupied by a sessile individual with age x over the mean probability of establishment in a population with the same age structure but with complete juvenile dispersal (see appendix). The mean value of this ratio over all age classes is .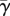

Kin competition occurs, both among adults and their own offspring, and among philopatric juveniles born to the same parent. The intensity of both kinds of kin competition decreases when the dispersal rate of juveniles increases. Given that a population has dispersal rate d and dispersal cost c, then for a given site, we can define the proportion of juveniles after dispersal that were born to an adult at age x at that site as:

2.7

At the limit corresponding to complete dispersal (d = 1 and h = 0), one should recover classical results for a large, density-regulated population with asymmetric competition between age classes and no kin interactions. We refer to this case as a well-mixed population. This model will allow us to investigate the effect of limited dispersal on the strength of selection acting on age-specific mutations that influence the pattern of ageing.

3. Results

(a). Analytical results

For an organism with the life cycle described above, a general expression for the strength of selection on a mutation affecting the age schedules of birth and death (see electronic supplementary material, appendix) is given by:

3.1

where S_p(x) is the component of the force of selection due to the effect of the mutation on survival rate between age x and x + 1 and S_m(x) is the component of the force of selection due to the effect of the mutation on fecundity at age x.

(i). Predictions in the absence of kin interactions

When dispersal is complete (d = 1), we recover Hamilton's (1966) classical result for the strength of selection on a mutation that affects survival or fecundity, respectively, acting at age x in a stationary population:

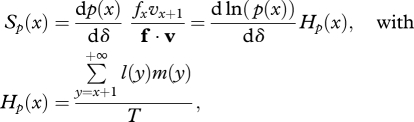3.2

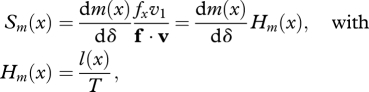3.3

where is the mean generation time (i.e. the mean age of parents of offspring produced in a population with that age structure).

Since H_p(x) and H_m(x) are strictly positive, the sign of the components of the selection gradient S_p(x) and S_m(x) depends simply on the sign of dp(x)/dδ and dm(x)/dδ, respectively. A mutation decreasing the survival rate at age x (or similarly decreasing the fecundity at age x) with no other pleiotropic effect is therefore always selected against. The force of selection acting against such a mutant however changes with age x. The quantities H_p(x) and H_m(x) always decrease with increasing age in a stable population (Hamilton 1966). Variation in the strength of selection with age measured by S_p(x) and S_m(x) however depends on the scale on which mutations act (e.g. whether mutations have additive effects on survival p(x), mortality [=−ln(p(x))], or the log of mortality, see Baudisch 2005).

(ii). Effect of restricted dispersal on selection on age-specific survival

With limited dispersal, the component of selection due to the effect of the mutation on survival at age x can be more generally expressed as (see electronic supplementary material, appendix):

3.4

Expressions for the asymptotic frequencies and reproductive values of different age classes with limited dispersal are given in the appendix. Using such expressions, the strength of selection acting on survival rate at age x can be written as:

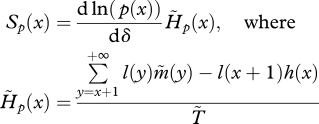3.5

and 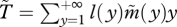is the mean generation time with limited dispersal.

Comparing equation (3.2) with equations (3.4) and (3.5) shows that limited dispersal has two main effects on the strength of selection on age-specific survival. First, juvenile dispersal changes the way in which effective fecundity 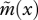varies with age (see illustrations in figures 1 and 2), and thereby changes generation time 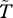in particular (figure 3). Second, limited dispersal leads to weaker selection against mutations that decrease survival at age x (and that have no other pleiotropic effects), as shown by the new negative term on the right hand-side of equations (3.4) and (3.5). The strength of selection on survival at age x now depends not only on the future reproductive value of the individual if it survives, but also on the effect of its survival on the establishment of related juveniles. The reproductive value of the older individual, v_x+1, is balanced against the reproductive value of a newly established juvenile, v₁, weighted by the probability h(x) that this juvenile was born locally and is genetically identical to its parent (equation (3.4)).

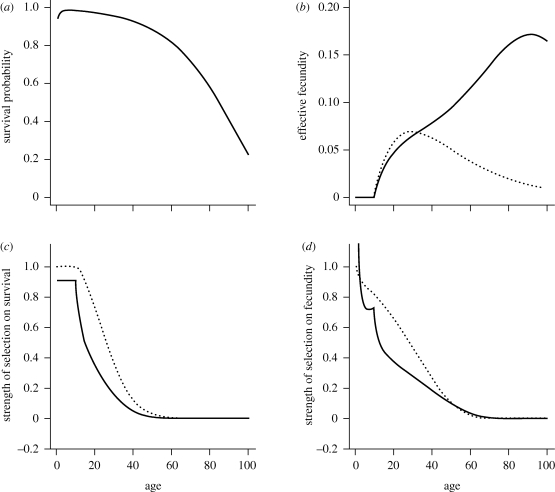
Figure 1.

Age-specific vital rates and the strength of selection acting on them for two different juvenile dispersal rates and a life cycle with an extended period of fecundity. Continuous line: partial dispersal (d = 0.5). Dashed line: complete dispersal (d = 1). (a) Age-specific survival probability p(x) as given by the Siler equation (equation (3.11)) with parameters α₁ = 0.1, α₂ = 0.01, β₁ = 0.8 and β₂ = 0.05. Note that this is unaffected by the dispersal rate of juveniles. (b) Effective fecundity 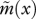as defined by equation (2.2) with intrinsic fecundity b(x) given by equation (3.12) with parameters ɛ = 10 and φ = 0.05. (c) Strength of selection on age-specific log-survival measured by (see equation (3.5)). (d) Strength of selection on age-specific fecundity measured by 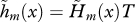(see equation (3.10)). With limited dispersal (d = 0.5), the strength of selection on the fecundity of the first age class (not shown) is here about twice as large as the same measure in the case of complete dispersal (d = 1). For clarity of presentation, the indicators of the force of selection have all been standardized by the mean generation time in the case of complete dispersal (T) as given by equation (3.3). The cost of dispersal is null (c = 0).

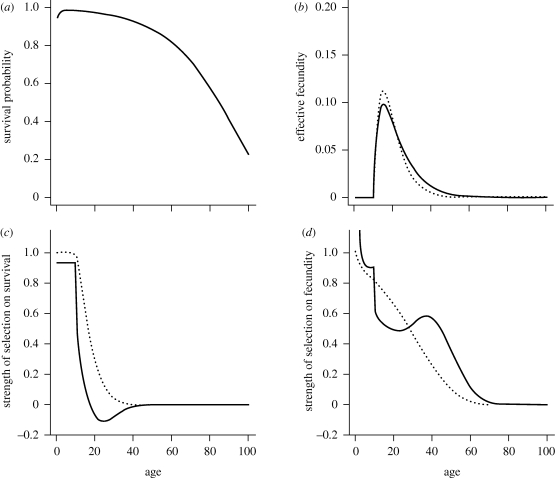
Figure 2.

Age-specific vital rates and the strength of selection acting on them for two different juvenile dispersal rates and a life cycle with a narrow period of fecundity. Continuous line: partial dispersal (d = 0.5). Dashed line: complete dispersal (d = 1). (a) Age-specific survival probability p(x) as given by the Siler equation (equation (3.11)) with parameters α₁ = 0.1, α₂ = 0.01, β₁ = 0.8 and β₂ = 0.05 (same as in figure 1). Note that this is unaffected by the dispersal rate of juveniles. (b) Effective fecundity 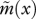as defined by equation (2.2) with intrinsic fecundity b(x) given by equation (3.12) with parameters ɛ = 10 and φ = 0.2. (c) Strength of selection on age-specific log-survival measured by 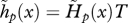(see equation (3.5)). (d) Strength of selection on age-specific fecundity measured by 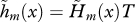(see equation (3.10)). With limited dispersal (d = 0.5), the strength of selection on the fecundity of the first age class (not shown) is here about twice as large as the same measure in the case of complete dispersal (d = 1). For clarity of presentation, the indicators of the force of selection have all been standardized by the mean generation time in the case of complete dispersal (T) as given by equation (3.3). The cost of dispersal is null (c = 0).

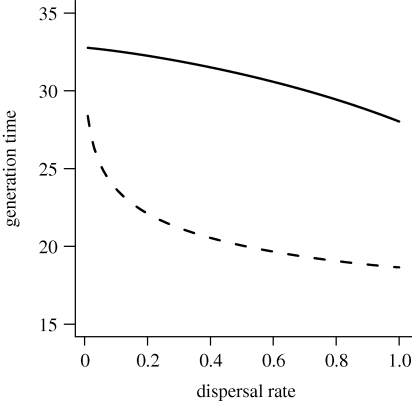
Figure 3.

Effective generation time (as given in equation (3.5)) as a function of juvenile dispersal rate. Age-specific survival rates p(x) and fecundities b(x) are as in figure 1 (wide fecundity period, continuous line) and in figure 2 (narrow fecundity period, dashed line), respectively.

Consider a mutation with effects on a single age class and with no pleiotropic consequences. Equation (3.4) predicts that for some age x, selection may favour mutations that increase mortality (i.e. S_p(x) > 0 with dp(x)/dδ < 0), as long as:

3.6

which can alternatively be written as:

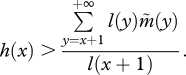3.7

Thus, selection will actually favour genes that increase mortality at age x if the number of successfully established juveniles expected to be produced during the residual reproductive lifespan is smaller than the proportion of philopatric juveniles currently present in the site (see figure 2 for an example).

In the absence of senescence on fecundity (i.e. b(x) = b for all age x), using the definition of h(x) in equation (2.7), condition (3.7) becomes:

3.8

which is obviously never true. This leads to the critical conclusion that selection favouring mutations that increase mortality requires pre-existing senescence on fecundity. For equation (3.7) to hold, fecundity at age x must be high and must drop quickly at subsequent ages. That is, selection favouring deleterious mutants may lead to increased mortality, but at least in this model, they cannot account for the evolution of senescence from a non-senescent life cycle.

(iii). Effect of restricted dispersal on selection on age-specific fecundity

With limited juvenile dispersal, the force of selection owing to the effect of the mutation on fecundity at age x becomes:

3.9

Using expressions for reproductive values given in the electronic supplementary material, appendix, the strength of selection on age-specific fecundity at age x can be expressed as:

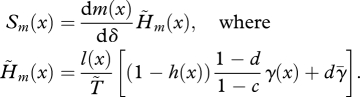3.10

While increased mortality may sometimes be favoured when juvenile dispersal is limited, selection always opposes the decline in fecundity (since the sign of S_m(x) depends only on that of dm(x)/dδ, regardless of the dispersal rate). Nonetheless, the strength of selection on fecundity varies with the dispersal rate. In particular, the force of selection on fecundity vanishes as the dispersal rate tends toward 0 (since h tends towards 1). The pattern of variation with age of the strength of selection is also affected by limited dispersal. In particular, 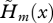does not necessarily decrease monotonically with age (figures 1 and 2) as it does in a well-mixed population (compare with equation (3.3)). Indeed, with limited dispersal, the first term in the brackets of equation (3.10) is larger for those age classes with low survival and/or low fecundity than for age classes with high fecundity and survival.

(b). Numerical exploration

Here, we illustrate the effects of limited juvenile dispersal and kin competition on the strength of selection on age-specific mortality and fecundity, using hypothetical iteroparous populations in which ageing occurs in both survival and fecundity (figures 1–3). More precisely, we assume that μ(x), the risk of mortality at age x, changes with age according to the Siler model (Siler 1983):

3.11

with p(x) = e^−μ(x). This function allows mortality to decrease with age before sexual maturity and to increase again with senescence (see example in figures 1a and 2a). We assume that fecundity increases after sexual maturity to reach a maximum at some intermediate age, then decreases with age (figures 1b and 2b):

3.12

We varied the shape of those functions (including cases of constant survival with age and/or constant fecundity). The effect of limited dispersal on the strength of selection on survival and fecundity was mostly affected by the rate of decline in fecundity with age. To contrast extreme situations, figure 1 shows the case of a species with an extended period of fecundity throughout old ages, while figure 2 shows the case of a species in which the period of fecundity is short with respect of its lifetime.

We compared Hamilton's indicators of the force of selection on age-specific survival and fecundity, with complete dispersal (d = 1, figures 1 and 2, dashed line), and with only partial dispersal of offspring (d = 0.5, figures 1 and 2, continuous line). In the presence of partial dispersal, parent–offspring competition modifies patterns of age-specific fecundity, lowering the effective fecundity of young adults with high survival prospects and increasing the effective fecundity of older adults with lower survival (figures 1b and 2b). Variation of juvenile dispersal thus has antagonistic pleiotropic effects on effective fecundities at different ages. As a result, effective generation time here increases as the juvenile dispersal rate decreases (figure 3).

The longer generation time explains, in part, why the strength of selection acting on the survival of immature age classes, measured by 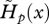, is lower with partial juvenile dispersal than with complete dispersal (figures 1c and 2c). The decline in the strength of selection on survival, which predicts the onset of senescence, occurs just after the age of first reproduction with partial dispersal as in classical theory with complete dispersal (see also equation (3.5)). Such decline is however faster with partial dispersal. Selection on age-specific survival can even become negative with partial dispersal, favouring increasing mortality, as predicted by equations (3.6–3.7), but this occurs only when the period of fecundity is particularly narrow with respect to the average lifetime (compare figures 2c and 1c). Negative selection on survival then affects those age classes where the fecundity peaks (compare figure 2b,c). The strength of selection on survival vanishes in older ages, at about the same time for both partial and complete dispersal (figures 1c and 2c) because of the overall low abundance of very old individuals.

While Hamilton's indicator of the force of selection on age-specific fecundity declines monotonically with age from birth in the case of complete dispersal, the same indicator 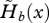shows more complex patterns of variation with age when dispersal is limited (figures 1d and 2d). When the survival rate of immature individuals is lower than the average survival rate of sessile individuals, the force of selection to improve fecundity in young age classes is much higher with partial dispersal than in a well-mixed population. In the former case, the force of selection however drops as the survival rate of immatures improves (figures 1d and 2d). During periods of increasing fecundity immediately following maturity, limited dispersal can lead to an even faster decline in the force of selection on fecundity (figures 1d and 2d). This is due to strong kin competition among the numerous sibs produced by the most fecund adults in their period of peak fecundity, which diminishes the benefits of increased fecundity. Conversely, selection to increase fecundity in older adults with low fecundity and low survival is higher with partial dispersal than with complete dispersal (see in particular figure 2d), because offspring born to such individuals have establishment prospects higher than average. When the period of fecundity is narrow with respect to the average lifetime (e.g. figure 2), limited juvenile dispersal thus selects more strongly for both earlier onset and later arrest of reproduction. Kin competition favours a more even distribution of reproductive events throughout a longer reproductive lifespan. Finally, the force of selection on fecundity vanishes with further increase in age, for both partial and full dispersal, as it does for survival.

4. Discussion

Most theoretical models of the evolution of senescence have assumed a very large, well-mixed population (Hamilton 1966; and following studies reviewed in Rose et al. 2007). A few recent models, however, have shown that spatial structure, metapopulation dynamics and limited dispersal can significantly affect the evolutionary trajectories of various life-history traits (see review in Ronce & Olivieri 2004), including reproductive effort (Ronce & Olivieri 1997; Pen 2000, but see Lion 2010), age at maturity (de Jong et al. 2000) and lifespan (Kirchner & Roy 1999; Travis 2004; Dytham & Travis 2006; Mitteldorf 2006). At the same time, patterns of senescence can, in turn, affect the evolution of natal dispersal (Ronce et al. 1998). Here, we investigate how limited dispersal might influence the evolution of ageing. The assumptions of our simple model bear strong similarity to some of those previous models (e.g. Ronce et al. 1998; Pen 2000; Travis 2004), including natal dispersal, territorial or sessile adults, absence of parental care and strong asymmetric competition between juveniles and adults. The principal merit of the present analysis is to formalize the effect of kin competition and limited dispersal on indicators of the strength of selection, similar to those derived by Hamilton (1966). Our analysis thus helps to connect recent results on kin selection effects with classic theory for the evolution of senescence.

In particular, with complete dispersal, our expressions for the force of selection on age-specific survival and fecundity simplify to those proposed by Hamilton (1966). In the presence of kin competition and limited dispersal, we found that the indicator of the strength of selection on age-specific survival comprises two terms (equation (3.5)): the first term is formally equivalent to that derived by Hamilton (1966) and is proportional to the remaining fecundity. The second term is negative and measures the deleterious effects of the focal individual's survival on its relatives (in our model, its own offspring). It is interesting to note the formal parallel with similar attempts to incorporate the effect of parental care or intergenerational transfers on life-history evolution (Lee 2003; Pavard et al. 2007). Modelling maternal care effects on offspring survival, Pavard et al. (2007) show that the strength of selection on age-specific survival is also made up of two terms, one corresponding to Hamilton (1966)'s prediction and a second term, positive this time, measuring the positive effects of a mother's survival on her children (see also Lee 2003 for similar formal results, discussed in Bourke 2007). This structural resemblance suggests that kin selection effects on life-history evolution, corresponding to either beneficial (Lee 2003; Pavard et al. 2007) or deleterious (the present study) effects of increased longevity on relatives fitness, could potentially be formalized within a single unified framework.

We found that reduced juvenile dispersal decreases the strength of selection on adult survival, consistent with previous studies (e.g. Pen 2000; Travis 2004), which found that limited dispersal favoured the evolution of shorter lifespan. Equations in the model of optimal reproductive effort by Pen (2000) can easily be recovered using our equations (3.5) and (3.10) and assuming that vital rates do not vary with age (results not shown). A shift of resources from maintenance to production of new offspring is favoured in genetically structured populations because only juveniles can disperse to alleviate kin competition. Even in the absence of a direct trade-off between reproduction and survival, parent–offspring competition under limited dispersal further generates an indirect negative relationship between the effective fecundity (measured by the number of surviving offspring) of an individual and its survival prospects. Assuming a positive effect of maternal presence on its offspring's survival, Pavard et al. (2007) found conversely that maternal care increases the strength of selection on adult survival, even beyond the last age of reproduction.

Our model also predicts that kin competition and dispersal could shape patterns of age-specific fecundity, a topic which has received less interest in the theoretical literature than lifespan variation. In particular, we found that intense sib competition reduces the strength of selection on fecundity in ages corresponding to the peak fecundity. Conversely, selection to increase the fecundity of age classes producing few offspring, including younger and older individuals, is relatively stronger. With limited dispersal, sib competition would then favour a larger spread of reproductive events throughout life. Interestingly, the evolution of other life-history traits spreading offspring in space or time, such as dispersal, diapause and dormancy, have been explained as strategies to reduce kin competition (Hamilton & May 1977; Olivieri 2001). Our model also sheds a different light on terminal investment in reproduction. A burst of reproduction in individuals close to death may be explained, in part, by the relaxed parental competition that their offspring will experience after the parent dies. Parent–offspring competition would then also contribute to selection for longer reproductive period. Conversely, parental care results in narrowing the period of fecundity (Pavard et al. 2007).

The contrasting effects of kin competition on selection affecting age-specific fecundity and survival raise questions about the joint evolution of vital rates in spatially structured populations. Limited dispersal reduces selection on survival of those age classes with peak fecundity, but favours at the same time a more even distribution of reproduction throughout life. In other words, the effect of limited dispersal on the evolution of survival rates is strong in those species with a narrow fecundity period, but we do not expect such reproduction patterns to be selected for in the same ecological context. Further modelling is required to elucidate how evolution might reconcile selection for iteroparity and shorter lifespan in spatially structured populations. A further complication is that natal dispersal itself could evolve to alleviate kin competition (see Dytham & Travis (2006) for a model of joint evolution of lifespan and dispersal).

Some researchers have argued that, at least in some cases, senescence has evolved because it has been favoured by natural selection. That is, genes have spread whose ‘purpose’ is to kill off individuals that carry these genes. Both empirical (Longo et al. 2005) and theoretical (Travis 2004; Mitteldorf 2006) results have been offered up in support of this notion of ‘programmed ageing’. This notion of senescence being favoured by natural selection has been controversial and may be in part purely semantic (see an interesting discussion in Bourke 2007). While our model shows that selection can favour increased mortality at some ages, we argue that this does not mean that senescence has arisen because it was favoured by natural selection. Using individual-based simulations with limited dispersal and strong kin competition, Travis (2004) concluded that programmed death at some age could be selected for in the absence of any direct trade-off between survival and fecundity. Note that although there is no direct trade-off between fecundity and survival in his model (nor in ours), kin competition creates an indirect trade-off between the survival of sessile adults and the establishment of their philopatric progeny. Travis (2004) argued that a prerequisite for the selection of suicide genes in his spatially structured simulations was that of a decline in fecundity with age, a result confirmed by our analytical model. Thus, selection for suicide genes may only reduce lifespan in an already senescent life cycle. Our numerical analysis further suggests that the decline in fecundity must be fast and the period of fecundity short with respect to lifetime to observe selection for increasing mortality in some age classes. As discussed above, it is unclear whether such life cycles may evolve frequently in the context of strong kin competition because of the joint selection on fecundity patterns.

The simplicity of our model allowed us to derive relatively condensed analytical predictions, with good illustrative value, but this simplicity also has several drawbacks. The first limitation is that the strength of selection acting on survival and fecundity informs us only partly about the evolution of life histories. Knowledge about patterns of genetic variation and covariation among traits is necessary to go further. As have other authors (Pen 2000; Travis 2004; but see Cant & Johnstone 2008), we assumed asexual reproduction, such that offspring are genetically identical to their mother. With sexual reproduction, relatedness asymmetries may result in conflicts over optimal lifespan or fecundity schedules between offspring and their parent (see Bourke 2007), a topic worthy of further investigation. Patterns of kin competition within groups sharing the same resources can be more complex in nature, potentially involving competition with older sibs (Ronce et al. 2000) or varying with the demographic context (Lion 2010). Finally, it would be interesting to incorporate both kin competition and helping in the same model of life-history evolution as the two phenomena may not be exclusive.

There has been recurrent discussion in the literature of whether sudden death after reproduction observed in a number of taxa represents adaptive suicide, benefiting relatives (see review in Bourke 2007; Longo et al. 2005). Bourke (2007) cites several instances of harassment of reproductive individuals in social species, which could be interpreted as cases of conflict over the timing of resource handover among relatives. The same author proposes examining patterns of age-specific senescence in species with strong sex-bias in dispersal, expecting the most philopatric sex to age more rapidly if kin competition has an important role in the evolution of senescence. A more straightforward test of the previous theory would rely on experimental evolution manipulating the degree of spatial mixing of the population. For instance, does the frequency of apoptosis described by Longo et al. (2005) increases when yeasts have been cultured in solid medium for many generations rather than in well-shaken medium?

Hamilton's (1966) model gave us the mathematical framework to understand theories for the evolution of senescence. Building on the foundation of his work, we have shown that limited dispersal does affect how selection shapes patterns of senescence, but that the intensity of these effects varies depending on the exact life cycle. Our model illustrates how limited dispersal and social interactions may generate patterns of antagonistic pleiotropy, which are relevant for the evolution of life histories, but may be undetectable under laboratory conditions where different age classes rarely compete with one another. A genetic modifier of dispersal would for instance have antagonistic effects on the effective fecundity of adults of different ages, which considerably increases the scope for genes potentially involved in shaping trade-offs affecting vital rates. These findings encourage us to broaden the range of ecological conditions in which the evolution of ageing is studied both from a theoretical and experimental point of view.