Abstract
Fertility decline, driven by the one-child policy, and son preference have contributed to an alarming difference in the number of live male and female births in China. We present a quantitative model where people choose to sex-select because they perceive that married sons are more valuable than married daughters. Due to the predominant patrilocal kinship system in China, daughters-in-law provide valuable emotional and financial support, enhancing the perceived present value of married sons. We argue that inter-generational transfer data will help ascertain the extent to which economic schemes (such as pension plans for families with no sons) can curtail the increasing sex ratio at birth.
1 Introduction
China is currently experiencing rapid demographic changes. A rapid decline in fertility, driven by the one-child policy, has produced a rapidly aging population and been followed by a large increase in the sex ratio at birth (SRB). The latter is primarily due to son preference (Coale 1991, Johansson and Nygren 1991, Li et al 2000a), which is widespread in China and derives in part from Confucian principles and a rigid patrilineal culture. Fertility decline, a strong parental desire to have at least one son, and the increasing availability of sex-selection technology have led to a far greater number of male live births than female live births as compared to the numbers expected in most human populations (Coale 1991, Sen 1990). Continued growth in the SRB will likely have serious implications for China’s marriage market and its future stability (Tuljapurkar, Li and Feldman 1995).
In this paper we focus on understanding how policy interventions by the Chinese government, particularly economic programs, could affect the sex ratio at birth. Previous research has shown that the kinship system and nature of marriage strongly affect son preference in China, Northern India and South Korea (Das Gupta et al. 2003). In the long run there is likely to be feedback from the marriage squeeze created by a large number of excess males, which may force people to rethink sex-selection, at least to some extent (Tuljapurkar, Li and Feldman 1995). To capture the relationship between son preference, marriage and the value of children, we introduce the notion of perceived present values of married and unmarried sons and daughters (Appendix A contains a glossary of terms).
We present a simple economic model of son preference using the terminology of Li, Feldman and Tuljapurkar (2000). We show that under our model assumptions, the decisions made by couples acting in their own interests do not maximize the total expected present value for all couples in society. Although sex-selection is not socially efficient, it is the equilibrium solution for couples acting independently. There is an incentive for people to sex-select because they perceive that a married son is more valuable than a married daughter. We apply the model to data from China in 1989, to estimate the relationship between the perceived present values of sons and daughters assuming that the observed demographic rates were close to the equilibrium values. Finally, we demonstrate how to analyze policies involving economic benefits (such as pension plans for families with no sons) in terms of our model. We compare policies based on their effectiveness in decreasing the difference between the perceived present value of sons and daughters, thereby reducing the sex ratio at birth.
2 Sex ratio at birth, son preference and the one-child policy
The sex ratio at birth (SRB) is the ratio of number of boys to girls born in a certain time period. The SRB is usually stated as the number of boys born for every hundred girls born. An SRB of 105 is generally accepted to be naturally occurring, and does not change much with parity, i.e. the birth order of the child (Johansson and Nygren 1991). The increasing SRB in China (figure 1) and in other Asian countries like India, South Korea and Taiwan indicate a growing deviation from this normal value. Increases in the SRB are attributed to an increase in the rate of sex-selection, using such methods as abortion, infanticide or abandonment, to ensure having at least one son. Thus, son-preference, the cultural trait of desiring sons, is prevalent in these countries. Note that there is great regional variation in the SRB in China. Appendix B lists the SRB along with certain socio-economic factors by province in year 2000.
Figure 1.
Sex ratio at birth (SRB) in China, 1970–2005.
Source: For 1982, 1990 and 2000, tabulation on the population census in 1982, 1990 and 2000; for other years, 1% Population Sampling Survey, National Bureau of Statistics of China.
Why has the SRB increased in recent years even though son preference has always existed in these countries? The main driving force is that couples are having fewer children, either voluntarily (India, South Korea) or due to regulations (China). In an earlier high-fertility era couples could have several children until they had a son. Also, sex-selective technology was not available before the 1980s, although infanticide and high female infant mortality were not uncommon (Coale and Banister 1996). Now that fewer children are being born, couples have a much lower chance of having a son and people are motivated to intervene to select the sex of their children. Ultrasound technology enabling the pre-natal determination of sex has been widely available since the 1980s, leading to large-scale selective abortion of females (Zeng et al. 1993). Even though sex-selection is illegal in China, as Graham, Larsen and Xu (1998) write, “many couples find ways through the ‘back door’ to learn the sex of their unborn child, and abortion is an accepted form of birth control in China.”
Fertility has declined dramatically in China ever since the implementation of the one-child policy in the late 1970s. Technically, the policy is not really a ‘one-child’ policy. The Chinese government recognised that ethnic differences across regions had to be accommodated, and although the policy is a national one headed by the State Population and Family Planning Commission, administration and monitoring are decentralized to the provincial governments. Local administrators are responsive to regional needs and there are significant regional differences among the specific family planning regulations. The one-child policy includes rewards for people who agree to follow the policy, exceptions allowing some parents (e.g., in rural farming communities) to have a second (or higher order) child, and punishments for those who sign an agreement but break the rules. Conditions that may allow parents to have a second child include: 1) the first child is a girl; 2) the first child is disabled; 3) the parents are only children; 4) the parents have certain special occupations (Hung 2004).
However, while the one-child policy would seem to make sex-selection more attractive, it is not necessarily the only cause of China’s sex ratio imbalance. Comparisons are often made with other countries where the SRB has increased despite the lack of coercive family planning regulations (Banister 2004, Wang 2003, Li et al 2000b). These discussions suggest that the SRB may not be significantly different in China even if the one-child policy were revoked or modified.
3 Causes of son preference
Son preference is deeply rooted in the culture of several Asian countries. China has a Confucian patriarchal tradition and sons are preferred for several reasons, including passing on the family name, providing old-age support for parents, ancestor worship, maintaining higher status in society and assisting parents in agricultural or production activities (Poston et al. 1997, Banister 2004, Zeng et al. 1993). Many of these factors also apply in other countries where son preference is observed. In India, the practice of dowry provides economic incentives for parents to prefer sons. It is difficult to disentangle the financial and cultural aspects of these issues. For instance, dowry is both a cultural and a financial phenomenon.
Even though socio-economic factors are very different in India, China and Korea, the common factor driving son preference in all three countries is believed to be a rigid patrilineal kinship system (Das Gupta et al. 2003, Feldman et al 2006) in which the lineage of the family is continued solely by males, and productive assets are typically inherited only by males. Patrilocality, or cohabitation of a married couple with the husband’s parents, is typical of such a kinship system. Patrilocal marriage weakens ties between a daughter and her parents, and she may be neither obligated nor encouraged to support her parents after her marriage. Even though there has been a surge in economic opportunities for women in recent times, the value of a daughter is believed to remain low because she provides support (in money, time, and care) only to her in-laws.
4 Son preference, marriage and old-age support
The nature of marriage is a central theme in our discussion of son preference and the sex ratio at birth. Most traditional marriages in China are virilocal, with the married couple residing with the husband’s parents. Uxorilocal marriages are the opposite kind in which a married couple resides with the wife’s parents. The fraction of marriages that is uxorilocal has slowly increased in recent times due to the increase in the number of no-son families following sustained low fertility. Li et al. (2006) consider this a good sign because uxorilocal marriage helps to stabilize low fertility, alleviate son preference, decrease male bias in the sex ratio at birth, and improve female child survival and women’s social status. However uxorilocal marriage is still infrequent and has had little effect on the trend towards increasing the SRB in China.
In China, as in India, there is little or no pension support for the elderly in rural areas and more than half of the rural elderly rely on transfers from children. The prevalence of virilocal marriage in China means that sons are most likely to live with their parents, and to be the principal source of emotional, instrumental (household work, etc.) and financial support. Two surveys were performed in three different counties in Shaanxi and Hubei provinces in 1997 and 2000 respectively, to understand the effect of marriage form and other factors on the SRB (Li et al. 2006). The studies took place in three rural counties in China, namely Sanyuan (a low-prevalence uxorilocal marriage area), Lueyang (a high-prevalence uxorilocal marriage area) and Songzi (a medium-prevalence uxorilocal marriage area). A strict patrilineal family system is observed in Sanyuan, which results in strong son preference reflected in the SRB of 117.2 during the years from 1990 to 1996. The relaxed patrilineal family system in Lueyang results in relatively weak son preference as the overall SRB in Lueyang was 105.0 during the same time. In Songzi, both virilocal and uxorilocal marriages have been prevalent for a long time and the diversity of marriage form directly results in a normal overall SRB of 105.8 from 1990 to 1996. The survey results in Songzi also showed no significant difference between the amounts of financial support received by parents in their old age from sons and daughters (Li, Feldman and Jin 2004).
Jin, Li and Feldman (2006) conducted a survey in Shenzhen of rural-urban migrants. One of the purposes of their study was to explore the relationship between gender of married migrants and the financial support provided to their non-co-residing parents before and after migration. We use results from their survey to compare the mean annual financial support provided by sons and daughters to their parents before migration, i.e. in the rural area. Table 1 shows the mean net financial transfers from sons, daughters and their respective spouses to parents. The numbers suggest that the key driver of value for the parents is the daughter-in-law! There is no significant difference between the financial support provided by sons and daughters to their biological parents, but the daughter-in-law provides more support than any of the other children. For a country where virilocal marriages are common we also expect that the daughter-in-law will provide a fair amount of emotional and instrumental support.
Table 1.
Net financial support from children and their spouses to parents; survey results from Shenzhen, before migration to urban areas.
Source: Jin, Li and Feldman (2006).
| Mean Annual Financial Support (Yuan) | |
|---|---|
| Son | 459 |
| Daughter-in-law | 879 |
| Daughter | 453 |
| Son-in-law | 720 |
These results show that marriage and son preference have a more complicated relationship than has been argued previously. The analysis of transfers suggests that parents who opt for sex-selection in China are expecting their son to marry and bring home a daughter-in-law who will provide them support in their old age. The irony is that as more people decide to sex-select, their sons may not find spouses to marry and a key source of intergenerational transfer will be lost. Other benefits of a son, such as carrying on the family line, will also not be realized if the son fails to marry. Current data show little evidence of a feedback from the marriage market to son preference in China, but past and ongoing growth of the SRB means that in the future a marriage squeeze is likely to affect the decision to sex-select.
5 Implications of the increasing sex ratio at birth
The fertility and mortality declines in China and the growth of the SRB increase the uncertainty about China’s demographic future. Several researchers have shown that there will be a significant number of excess males born in the future, at the expense of ‘missing women’, and these excess males will be unable to find brides. These males are known as the ‘guang gun’, meaning ‘bare branches’ or ‘bare sticks’. The first-marriage market will be highly imbalanced in the future (Tuljapurkar, Li and Feldman 1995) – it is estimated that there were more than 23 million unmarried boys born between 1980 and 2001 in China, and a similar number of excess males is expected in the next 20 years (Poston and Glover 2005).
What will this population of unmarried males do? Only time will answer to this question, but several gloomy scenarios have been proposed. There may be an increase in violence as there is extensive sociological evidence that if men do not marry, they are more likely to spread disease and commit crimes (Poston and Glover 2005). There has already been a steady rise in the number of kidnappings and forced marriages reported around China. The surplus men are also likely to have a profound impact on the future of HIV spread in China. They may become a significant new HIV risk group, and therefore future interventions to curb the HIV epidemic in China will need to consider their potential effects (Tucker et al 2005). The Chinese government has recognised the need to address the problem of sex-selection both for the long term and also to counter the unethical and illegal behaviour of sex-selection in China. As Poston and Morrison (2005) point out, “the surplus of boys and shortage of girls ‘made in China’ could soon become not just a concern for China, but for the world.”
6 Sex ratio at birth, fertility decline and sex-selective potency
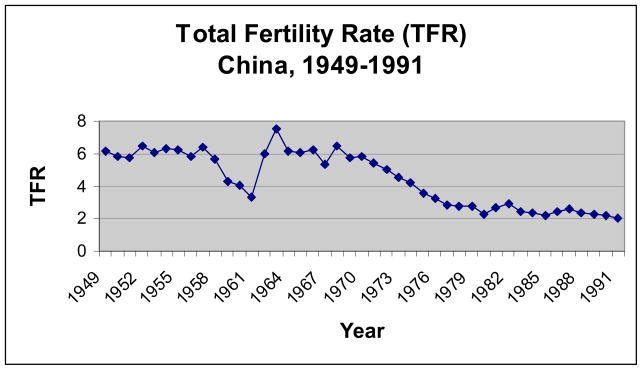
China’s SRB has been increasing steadily as the total fertility rate (TFR, or the average number of children borne to a woman) has declined from over 6 in the 1950s to close to 2 in the 1990s (figure 2). The one-child policy has played a role in this decrease as fertility has declined much quicker than under a voluntary family planning programme. However, there is reason to believe that the one-child policy has aggravated the trend to increasing SRBs and not created it. Fertility decline plus inherent cultural son preference may lead some couples to take action to ensure that they have a son. When technology to detect the sex of unborn children is readily available as it is in most places in China, some of these couples resort to abortion, abandonment or infanticide of daughters and these actions result in a higher than normal SRB.
Figure 2.
Total fertility rate (TFR) in China, 1949–1991.
Source: National Bureau of Statistics, P.R. China, China Statistical Yearbook 2000. Available at: http://www.cpirc.org.cn/en/year.htm
The SRB in China is significantly higher at higher parities, especially for the last-born child and even more so when the previous children are daughters (Graham, Larsen and Xu 1998, Zeng et al. 1993). There is some evidence that indicates that almost all sex-selection is by couples who have no previous sons and are able to have at most one more child. Keeping this notion in mind, it is useful to decompose the effects of fertility decline and son preference on the increasing SRB. Li, Feldman and Tuljapurkar (2000) introduce the following terminology to define and measure separately the impact of the demographic issue of fertility decline and that of the cultural/behavioural issue of son preference on SRB. They define the phrase ‘a woman being in the sex-selection situation’ as the random event Y in the life of a woman such that this woman is able to have only one more child and that she has no sons borne to her previously. The sex-selection pressure, y, is the probability that she is in this situation. The sex-selective potency (which we just call potency for brevity) is the probability that a woman will have a son through sex-selection given that she is in the sex-selective situation. The term sex-selection refers to any activity that ensures that the last child is a boy, including infanticide, sex-determination followed by abortion, etc. Now we summarize some mathematical relationships derived in Li, Feldman and Tuljapurkar (2000) that will be useful for subsequent sections:
Let T be the total fertility rate (TFR), or the expected number of children a woman has during her life, and s0 be the normal sex ratio at birth, i.e. sex ratio without sex-selection. If there are n women and each woman has a probability y of being in the sex-selective situation and a sex-selective potency of p, then the expected number of sex-selective boys is npy. The total expected number of children is nT, therefore the expected number of children born without sex-selection is n(T − py). These children are born without sex-selection and thus we expect their numbers to have the normal sex ratio at birth. There are n(T−py)s0/(1+s0) boys who are born without sex-selection and n(T−py)/(1+s0) girls, on average. The total number of boys is the sum of the boys who are born as a result of sex-selection and those who are not.
| (1) |
The prevalent sex ratio at birth after sex-selection, s, is the ratio of the total expected number of boys divided by the total expected number of girls,
| (2) |
Rearranging the terms, we can also express the potency in terms of T, s and y.
| (3) |
The advantage of using potency p (as opposed to s) as an indicator of the level of son preference in a community is that p expresses a decision variable, the probability that a woman will undertake sex-selection. In current modern-day populations and particularly in China, the TFR and the sex-selection pressure y have stabilized or is expected to stabilize soon, so changes in the realized sex ratio s are driven by changes in the potency p.. Potency is a useful index for comparing populations that have similar fertility rates but different realized sex ratios (like provinces across China). In subsequent sections, we use potency as the measure of son preference, i.e. a population with a higher potency is one where son preference is more prevalent.
7 A quantitative model
7.1 Perceived present values and son preference
We have suggested that a daughter-in-law may be the main driver of added value for people in their old-age, assuming their sons have virilocal marriages, as is typical throughout China. An increase in the rate of sex-selection will decrease the marriage rate because there are too few women in the marriage market. This is precisely what is being observed in China today – the ‘guang gun’ are the excess males that may not be able to find mates in China.
How do people in the sex-selective situation behave if they are trying to do the best they can for themselves? To answer this question we introduce the notion of perceived present value (PPV). We define the perceived present value of a child to be the worth of a child to its parents throughout their lives, discounted back to the child’s birthday. We argue that people in China and other countries who practice sex-selection perceive that a son has a higher PPV than a daughter. The PPV is the sum of the present value (PV) of the financial aid that a child might provide for old-age support, plus the value of cultural benefits that are more difficult to quantify but which we assume can be expressed in money equivalents. For instance, sons may have a higher PPV because they help in ancestor-related rituals, continue the family lineage, or improve parents’ social status. We label the PPV as ‘perceived’ to include cultural values of the latter sort that are not necessarily financial and ‘present’ values to sum over the long term benefits over the life of a newborn child.
We now present a simple theoretical model that relates the potency, as discussed in the previous section, and the perceived present values. Let the expected PPV for a married son, an unmarried son and a daughter in a population be vm, vu and vd respectively. We assume for simplicity that all women marry once, and that if there are excess males born each year, they will never marry. Also, we presume that vm > vd > vu > 0, i.e. the married son has the highest expected PPV and the unmarried son has the lowest expected PPV.
7.2 The efficient optimal potency
How would people in the sex-selective situation behave if they wanted to maximize society’s value, i.e. the sum total of expected values for every person? The efficient (socially optimal) potency in a particular year is achieved when the total PPV of all children is maximized. When the potency is p, sex-selection pressure is y, number of women is n and TFR is T, the expected number of boys and girls can be evaluated (see equation (1) and the corresponding section). We assume that people who sex-select are successful in having sons. The expected number of excess boys is the expected total number of boys minus the expected total number of girls; these excess males will not be married when they are of marriageable age. From equation (1) and its preceding paragraph,
| (4) |
We know the expected number of married boys, married girls, unmarried boys and also the PPV for each. Hence the total expected value Vs for all people is:
| (5) |
Recall that vm > vd > vu, and the values are assumed to be positive. Thus, vm + vd > 2vu. The total expected value is a decreasing linear function in p, and therefore the maximum value of Vs is attained when p = 0. We can see this intuitively as well: if there are 10 children to be born, it is socially optimal if there are 5 boys and 5 girls so that they can pair up. If exactly one of the women giving birth to a girl had sex-selected, there would be 4 pairs of married boys and girls and 2 unmarried boys, resulting in a lower value overall. According to this simple model, it is efficient for society if people do not sex-select.
7.3 The equilibrium optimal potency
In most Chinese provinces, the efficient optimal potency value of 0 is not observed in practice, suggesting that there is an incentive for people to sex-select to try to increase their individual expected PPV. A woman in the sex-selective situation may believe that the chance that her son will find a mate is high enough for her to prefer the sex-selection option. There is a trade-off involved – when the potency is high, there are fewer girls born and the chance for boys to find a partner decreases. We suppose that the ith couple chooses whether to sex-select knowing what the population’s potency will be. In other words, the couple chooses their own potency pi, their probability of bearing a son, in order to maximize their own expected PPV. Since we assume that everybody has the same expected PPV values, by symmetry, everybody has the same optimal potency p* at the Nash equilibrium. In this sub-section we use a simple game theoretic model to understand the behaviour of couples regarding sex-selection, under equilibrium conditions. To obtain the equilibrium potency p* we analyze the perspective of a particular couple in the sex-selective situation. We suppose that the other n−1 couples have chosen this equilibrium potency, and ask whether the couple can maximize its PPV by choosing an individual potency pi > 0.
For the n−1 other couples, and using s0 = 1 as an approximation, the number of excess boys is given by replacing n by n−1 and s0 by 1 in equation (4), which equals (n−1) p*y. For any boy in the population, the probability q* that he will marry, is the number of girls (which is the number of married boys) divided by the total number of boys. We assume that mortality rates are the same for both girls and boys from birth till marriageable age, hence mortality can be ignored. Also, assuming n is a large number, the ith couple’s decision does not affect q*. Note that in the symmetric equilibrium, the other n−1 women all choose the (identical) optimal potency p*. Thus, q* is given as,
| (6) |
Notice that q* is a decreasing function of p*. The couple under consideration will have a son if they sex-select with probability pi, or if they don’t sex-select and happen to have a boy. As mentioned earlier, we use s0 = 1 as a simplifying approximation, so it is equally likely that a couple will have either a son or a daughter, if they do not sex-select.
| (7) |
They will either have a son who marries, a son who doesn’t marry, or a daughter. If they have a son, the son will marry with a probability q*, or will remain unmarried with probability 1−q*. The mathematical expression for q* was obtained in equation (6). Therefore, the expected PPV for the couple, Ve, is given by:
| (8) |
The couple chooses a value of pi that maximizes Ve, given that the other couples choose p*. Now Ve in the equation above is either an increasing or decreasing linear function of pi, unless the coefficient of pi equals 0. The coefficient of pi in this expression is q*(νm−νu)− (νd−νu) where both terms in parentheses are positive. But q* is adecreasing function of p*, so there may be a value of p* between 0 and 1 at which this coefficient is zero, and hence at which the couple’s PPV is maximized. This value yields the only possible symmetric equilibrium solution, where the ith woman’s optimal pi equals p*. Using (6) and setting the coefficient of pi to 0 yields the unique value for p*:
| (9) |
Note that the equilibrium potency is 0 when the PPV for a married son is the same as that of a married daughter. This simple model can easily be extended to incorporate mortality rates of children and marriage among people born in different years. A demographic model similar to the cultural transmission model of Li, Feldman and Li (2000) can be used to project the actual SRB as a function of the PPVs. This will provide a forecast for the SRB and one can perform a sensitivity analysis on the parameters to see the effects of different potency or perceived value trajectories on the SRB.
8 Perceived present value ratios: An application in China
It may be difficult to quantify the PPVs for a population, but if we assume that people behave optimally and the system is in equilibrium, then we can estimate some relationships between the PPVs: vm, vu and vd. Let the ratios vm/vu and vd/vu be m and d respectively (where m > d > 1). We will refer to these ratios as the value ratios. In 1989 the TFR in China was 2.25 (close to the replacement level) and the SRB was 1.14 (which is above the normal SRB of 1.05). Li, Feldman and Li (2000) estimated the sex-selective pressure y to be 0.58 and potency p to be 0.17 using the quantitative model they developed. Assuming that the equilibrium potency was attained in 1989, we can use equation (9) to find the relationship between value ratios m and d in China in 1989. Rearranging terms in equation (9), we obtain:
| (10) |
We see that m is a linear function of d. Replacing the 1989 values in equation (10),
| (11) |
Equation (11) shows the relationship between m and d according to the model, assuming that the potency was at equilibrium in 1989. For instance, if we assume that married daughters were perceived to be twice as valuable as unmarried sons, then married sons were perceived as 2.1 times as valuable as unmarried sons in 1989. We discuss these issues in more detail in the following section.
9 The implications of policy interventions
The ultimate goal of any policy intervention is to reduce the SRB by eliminating the gap in the perceived values of sons and daughters (Banister 2004, Das Gupta et al. 2003, Wang 2003). Laws and regulations can help but may not change son preference in the population. The gap between perceived values of sons and daughters may be reduced by socially relevant messages from the mass media, efforts by the government to introduce flexibility into the kinship system, educating women and involving men in family planning.
The lack of adequate social security for the rural population appears to be an important factor in people’s use of sex-selective abortion. Can economic support help in controlling son preference? Policies such as the provision of adequate pensions to families with no sons can bridge the gap between the PPVs by increasing the value of a daughter for people in a sex-selective situation. How much financial support in the form of pensions should the government provide to families with no sons? We can answer this question using our model. Suppose that the government wishes to decrease the equilibrium potency p to another equilibrium at a lower value p′ by spending an amount x to increase the PPV of a daughter from vd to vd′.
Equation (10) can be written as:
m= K1(p)d+ K2(p), where K1 and K2 are functions of p,
| (12) |
Since both p and p′ are equilibrium values, equation (10) must hold for both. Therefore,
| (13) |
The required pension for each family with no sons can be calculated by manipulating equation (13):
| (14) |
To find x, we would require estimates of vm and vu, i.e. the perceived present values of married and unmarried sons. It may be difficult to quantify these values, but we can base estimates around intergenerational transfers from children and their spouses. Thus our model provides a framework to quantify interventions that can reduce the SRB.
10 Conclusion and discussion
The skewed sex ratio at birth in China deserves more attention from scholars and policy-makers. Policy interventions are necessary to ensure that sex-selection does not continue to be a chosen option for couples. In this paper we present a model that attempts to quantify the relationship between son preference and marriage. We introduce the notion of perceived present values to explain observed sex-selective behavior based on the differences in perceived values for sons and daughters. The equilibrium potency model estimates how people might behave in the equilibrium situation, given their perceived present values for children. The model is applicable not only to China, but in all countries where the SRB has been rising. Several papers have previously discussed the issues regarding policy and son preference, but there has been little effort to quantify the role of policy interventions. Son preference is related to several complicated factors, and this paper is an attempt to quantify its relationship with marriage. Future research should estimate the perceived value of children through survey information, so that the Chinese government can develop appropriate incentives to control the sex ratio at birth.
Acknowledgments
We gratefully acknowledge the help of several scholars who provided us data and feedback relevant to the project, notably Prof. Thomas Weber, Prof. Dudley Poston, Prof. Shuzhuo Li, Xiaoyi Jin and Lulu Song. We also thank the Morrison Institute for Population and Resource Studies at Stanford University for support.
Appendix A
Glossary of terms
- Parity
The birth order of a child. For example, the first born child has parity 1, the second child has parity 2, and so on
- Perceived present value (PPV)
the worth of a child to a couple, throughout their lives and discounted back to the child’s birthday. It includes not only the financial support that a child might provide for old-age support, but also cultural benefits that are more difficult to quantify
- Potency (same as sex-selective potency)
The probability that a woman will give birth to a son through sex-selection, given that she is in the sex-selective situation
- Sex ratio at birth (SRB)
The ratio of live male births to live female births in a certain time period, usually expressed in terms of live male births per hundred live female births. The natural SRB has been observed to be around 105
- Sex-selective situation
A woman is in the sex-selective situation if she has no previous sons and if she is able or willing to have only one more child
- Son preference
A general phrase indicating a preference for sons over daughters in a population
- Total fertility rate (TFR)
The average number of children borne to a woman in a population, calculated as the ratio of number of live births to number of women in a certain time period
- Uxorilocal marriage
A marital living arrangement where the married couple resides with the wife’s parents after marriage
- Virilocal marriage
A marital living arrangement where the married couple resides with the husband’s parents after marriage
Appendix B
SRB and socio-economic factors by province in China, 2000. Source: Hung (2004).
| Province Name | SRB | % Urbanization | GDP per capita (yuan) | % Agriculture | % Women in High School | % Women in College |
|---|---|---|---|---|---|---|
| Beijing | 114.58 | 77.54 | 22000 | 31.49 | 24.12 | 17.55 |
| Tianjin | 112.97 | 71.99 | 17940 | 41.69 | 21.85 | 9.44 |
| Hebei | 118.46 | 26.08 | 7663 | 80.4 | 11.34 | 2.85 |
| Shanxi | 112.75 | 34.91 | 5137 | 73.04 | 12.62 | 3.74 |
| Inner Mongolia | 108.48 | 42.68 | 5872 | 64.72 | 14.69 | 4.06 |
| Liaoning | 112.17 | 54.24 | 11226 | 54 | 13.93 | 6.52 |
| Jilin | 109.87 | 49.68 | 6842 | 56.49 | 15.86 | 5.18 |
| Heilongjiang | 107.52 | 51.54 | 8562 | 54.25 | 14.63 | 5.06 |
| Shanghai | 115.51 | 88.31 | 34426 | 25.38 | 23.87 | 11.34 |
| Jiangsu | 120.19 | 41.49 | 11773 | 67.86 | 13.76 | 4.13 |
| Zhejiang | 113.11 | 48.67 | 13461 | 77.89 | 11.46 | 3.4 |
| Anhui | 130.76 | 27.81 | 4867 | 80.4 | 8.21 | 2.47 |
| Fujian | 120.26 | 41.57 | 11601 | 79.39 | 11.27 | 3.17 |
| Jiangxi | 138.01 | 27.67 | 4838 | 77.4 | 10.71 | 2.81 |
| Shandong | 113.49 | 38 | 9555 | 73.15 | 11.75 | 3.55 |
| Henan | 130.3 | 23.2 | 5444 | 81.8 | 10.79 | 2.88 |
| Hubei | 128.02 | 40.22 | 7188 | 72.03 | 13.3 | 4.12 |
| Hunan | 126.92 | 29.75 | 5639 | 79.9 | 11.84 | 3.12 |
| Guangdong | 137.76 | 55 | 12885 | 68.82 | 14.03 | 3.88 |
| Guangxi | 128.8 | 28.15 | 4319 | 82.52 | 10.37 | 2.59 |
| Hainan | 135.04 | 40.11 | 6849 | 74.13 | 13.69 | 3.47 |
| Chongqing | 115.8 | 33.09 | 5200 | 78.62 | 9.28 | 3.03 |
| Sichuan | 116.37 | 26.69 | 4805 | 81.39 | 8.2 | 2.67 |
| Guizhou | 105.37 | 23.87 | 2662 | 85.54 | 6.34 | 2.14 |
| Yunnan | 110.57 | 23.36 | 4559 | 84.52 | 7.27 | 2.23 |
| Xizang | 97.43 | 18.93 | 4559 | 85.95 | 3.85 | 1.41 |
| Shaanxi | 125.15 | 32.26 | 4607 | 77.16 | 13.1 | 4.43 |
| Gansu | 119.35 | 24.01 | 3838 | 80.78 | 10.76 | 2.91 |
| Qinghai | 103.52 | 34.76 | 5068 | 71.67 | 11.44 | 3.63 |
| Ningxia | 107.99 | 32.43 | 4839 | 71.28 | 12.14 | 4.11 |
| Xinjiang | 106.65 | 33.82 | 7470 | 64.79 | 13.27 | 5.64 |
Contributor Information
Anant Sudarshan, Email: anants@stanford.edu.
Shripad Tuljapurkar, Email: tulja@stanford.edu.
Ross Shachter, Email: shachter@stanford.edu.
Marcus Feldman, Email: marc@charles.stanford.edu.
References
- Banister J. Shortage of girls in China today. Journal of Population Research. 2004;21(1):19–45. [Google Scholar]
- Coale AJ. Excess female mortality and the balance of the sexes in the population: An estimate of the number of ‘missing females’. Population and Development Review. 1991;17(3):517–523. [Google Scholar]
- Coale AJ, Banister J. Five decades of missing females in China. Proceedings of the American Philosophical Society. 1996 Dec;140(4):421–450. [Google Scholar]
- Das Gupta M, Zhenghua J, Bohua L, Zhenming X, Chung W, Bae H. Why is son preference so persistent in East and South Asia? A cross-country study of China, India and the Republic of Korea. The Journal of Development Studies. 2003 December;40(2):153–187. [Google Scholar]
- Feldman MW, Tuljapurkar S, Li S, Jin X, Li N. Son preference, marriage, and intergenerational transfer in rural China. In: Gauthier A, Chu CY, Tuljapurkar S, editors. Allocating public and private resources across generations: Riding the age waves. Vol. 2. Springer; Netherlands: 2006. pp. 139–162. [Google Scholar]
- Graham MJ, Larsen U, Xu X. Son preference in Anhui province, China. International Family Planning Perspectives. 1998 June;24(2):72–77. [Google Scholar]
- Hung Y. Bachelor’s Honors Thesis. Stanford University; 2004. China’s one-child policy: regional regulation variation and the sex ratio at birth. [Google Scholar]
- Jin X, Li S, Feldman MW. The effects of rural-urban migration on intergenerational financial transfer in China: A gender-based perspective. Population Association of America, 2006 Annual Meeting; March 30–April 1; Los Angeles. 2006. [Google Scholar]
- Johansson S, Nygren O. The missing girls of China: A new demographic account. Population and Development Review. 1991;17(1):35–51. [Google Scholar]
- Li N, Feldman MW, Li S. Cultural transmission in a demographic study of sex ratio at birth in China’s future. Theoretical Population Biology. 2000a;58:161–172. doi: 10.1006/tpbi.2000.1478. [DOI] [PubMed] [Google Scholar]
- Li N, Feldman MW, Tuljapurkar S. Sex ratio at birth and son preference. Mathematical Population Studies. 2000b;8(1):91–107. [Google Scholar]
- Li S, Feldman MW, Jin X. Children, marriage form and family support for the elderly in contemporary rural China. Research on Aging. 2004;26(3):352–384. [Google Scholar]
- Li S, Jin X, Feldman MW, Li N, Zhu C. Uxorilocal marriage in contemporary rural China. Social Sciences Academic Press (SSAP); China: 2006. Uxorilocal marriage in contemporary rural China: A research summary. 2006. [Google Scholar]
- Poston DL, Glover KS. Too many males: Marriage market implications of gender imbalances in China. Genus, LXI. 2005;(2):119–140. [Google Scholar]
- Poston DL, Gu B, Liu PP, McDaniel S. Son preference and the sex ratio at birth in China: A provincial level analysis. Social Biology. 1997 Spring–Summer;44(1–2):55–76. doi: 10.1080/19485565.1997.9988934. [DOI] [PubMed] [Google Scholar]
- Poston DL, Morrison PA. China: Bachelor bomb. International Herald Tribune; Wednesday, September 14.2005. [Google Scholar]
- Sen A. More than 100 million women are missing. New York Review of Books; 20 December; 1990. pp. 61–66. [Google Scholar]
- Tucker J, Henderson G, Wang T, Huang Y, Parish W, Pan S, Chen X, Cohen M. Surplus men, sex work, and the spread of HIV in China. AIDS. 2005;19:539–547. doi: 10.1097/01.aids.0000163929.84154.87. [DOI] [PubMed] [Google Scholar]
- Tuljapurkar S, Li N, Feldman MW. High sex ratios in China’s future. Science. 1995;267:874–876. doi: 10.1126/science.7846529. [DOI] [PubMed] [Google Scholar]
- Wang Y. What should China do about the gender imbalance problem? EMerge: A Graduate Journal of International Affairs. 2003 May;4 Available at SSRN: http://ssrn.com/abstract=547982.
- Zeng Y, Ping T, Baochang G, Yi X, Bohua L, Yongping L. Causes and implications of the increase in China’s reported sex ratio at birth. Population and Development Review. 1993;19(2):283–302. [Google Scholar]