Mechanisms of mechanical signaling in development and disease

Abstract

The responses of cells to chemical signals are relatively well characterized and understood. Cells also respond to mechanical signals in the form of externally applied force and forces generated by cell–matrix and cell–cell contacts. Many features of cell function that are generally considered to be under the control of chemical stimuli, such as motility, proliferation, differentiation and survival, can also be altered by changes in the stiffness of the substrate to which the cells are adhered, even when their chemical environment remains unchanged. Many examples from clinical and whole animal studies have shown that changes in tissue stiffness are related to specific disease characteristics and that efforts to restore normal tissue mechanics have the potential to reverse or prevent cell dysfunction and disease. How cells detect stiffness is largely unknown, but the cellular structures that measure stiffness and the general principles by which they work are beginning to be revealed. This Commentary highlights selected recent reports of mechanical signaling during disease development, discusses open questions regarding the physical mechanisms by which cells sense stiffness, and examines the relationship between studies in vitro on flat substrates and the more complex three-dimensional setting in vivo.

Introduction

If we probed our environment using only those senses that rely on biochemical signaling, that is, smell and taste, we would be highly limited in the amount and type of information we could process, and would therefore probably make decisions with undesirable and ultimately devastating consequences. Such a limited repertoire of stimuli and information is imposed on the cell if biology is defined solely by the signals a cell receives from molecules that bind in specific ways to its receptors, by the intracellular biochemical reactions that are triggered by these binding events and by the direct biochemical consequences of genetically encoded information.

Specificity and high affinity of molecular interactions are sometimes perceived as hallmarks of biological relevance, as though evolution has devoted specific efforts to create structures and interactions that overwhelm ‘non-specific’ physical effects, such as electrostatic and mechanical forces, rather than exploit these forces to direct biological function within different cells and organisms. However, with the continuing growth of available genomic and proteomic information, increasing evidence shows that genetics and biochemistry alone are not sufficient to explain important biological phenomena. For example, the observations that different cell types have different shapes and that different organs have different stiffness, two factors of fundamental importance for diagnosing disease or evaluating wound healing and embryonic development, cannot be explained in purely biochemical terms. Furthermore, the mechanical environment of a cell determines not only its mechanical properties, such as stiffness and contractility, but also its phenotype. Despite remarkable progress in genetics, biochemistry and cell biology, clinical diagnoses are still routinely based on how a tissue feels, how it blocks radiation and how it yields to a knife. The properties of cells and tissues that prove useful in diagnosis further demonstrate the fundamental importance of physical factors in biology. Among the features that differentiate normal from diseased tissues and cells is often stiffness, which is generally quantified as an elastic modulus; see Box 1 and Buxboim et al. (Buxboim et al., 2010), Chen (Chen, 2008), and Janmey and Schliwa (Janmey and Schliwa, 2008) for summaries of how soft-matter mechanics and terminology apply to cell biology. It remains to be proven whether a change in tissue stiffness is merely a consequence of disease or also a contributing or even initiating factor in its development (Georges et al., 2007; Levental et al., 2007; Levental et al., 2009). However, several lines of evidence suggest that matrix and cell mechanics can act as powerful signals for control of the cell cycle (Klein et al., 2009; Winer et al., 2009a), initiation of specific transcription programs (Engler et al., 2007; Li et al., 2007) and development of organ dysfunction (Georges et al., 2007).

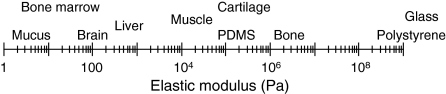
Box 1. Terminology of cell and tissue mechanics

Stress. The force exerted on an object normalized by the area over which the force is acting. The SI unit of stress is the pascal, Pa, or N/m². 1 Pa = 1 pN/μm². Force exerted perpendicular to the surface of a material results in compressional or extension stress, and force exerted parallel to the surface results in shear stress.

Tension. The magnitude of a pulling force. Forces in the opposite direction generate compression. For example, activation of myosin within a sarcomere generates tension at cell–cell or cell–tendon junctions. The force of gravity generates compression on cartilage and joints. Tension is a force and not a stress, and these terms are not interchangeable.

Strain. A dimensionless number that is the formal definition of deformation; it reports the geometric change in shape of a material under stress. Very approximately, it is the distance a material is stretched or compressed relative to its resting length. Cells typically undergo strains of 10–100% during lung expansion or muscle contraction, for example.

Elasticity. The property of a material to deform to a defined extent in response to a force and then return to its original state when the force is removed.

Elastic modulus. A constant describing the resistance of a material to deformation, defined as the ratio of stress to strain. Young's modulus (E) is commonly used to quantify elastic resistance to elongation or compression, and the shear modulus (G) describes the resistance of a material to deformation in shear. A summary of approximate elastic moduli for different materials is shown in the figure below. Precise values depend on the magnitude and duration of the deformation, and on whether the deformation is in shear or extension.

Linear elasticity. A linear relationship between stress and strain; equivalently, the elastic modulus is constant over a range of strains.

Nonlinear elasticity. For an ideal elastic material, stress is proportional to strain. Because the elastic modulus is the slope of the linear plot of stress versus strain, it is a constant. Many complex materials exhibit non-linear elasticity, that is, their elastic modulus changes with increasing strain. Such material can be either strain softening or strain stiffening, as is the case for cross-linked cytoskeletal and extracellular filament networks.

Viscosity. The ratio of stress to the rate of strain (or flow rate) for liquids. The SI unit is Pa•s = 10 Poise. The viscosity of water, for example, is approximately 1 mPa•s.

Viscoelasticity. The combination of viscosity and elasticity in a material. Viscoelastic materials have some ability to recover their initial shape after a deforming stress is applied (the hallmark of elasticity), but they also continue to increase strain the longer a stress is applied, until they either reach a limiting plateau (viscoelastic solid) or slowly flow or creep without limit (viscoelastic liquid or viscoplastic material), and only partly recover their initial shape after the stress is removed.

Several studies in the past decade showed that application of force either to the cell membrane or within the cell, as well as variations in the resistance to forces generated by the cell itself, can alter fundamental cellular processes, such as mitosis, cytokinesis, differentiation and response to soluble stimuli. For example, when collagen-coated magnetic beads are bound to the surface of a fibroblast, a protein complex assembles at the site where the bead is attached and forms an integrin-based adhesion that is linked to the actin cytoskeleton. If a magnetic field is then applied to pull on the attachment, the size of this protein complex increases and its composition changes, resulting in activation of myosin II and recruitment of filamin A (Glogauer et al., 1997). Moreover, the cell also rapidly recruits and activates myosin motors to pull back on the bead. Another important early study showed that, even without the application of external force, the adhesion strength of a cell attached to a fibronectin-coated bead, but not a bead non-specifically bound to the membrane, is greater when the bead is held in an optical trap that has a large spring constant, as determined by the intensity of the focused light, compared with a bead held in a more compliant trap (Choquet et al., 1997). For both collagen- and fibronectin-coated beads, the changes at the cell–bead interface are controlled by cytoplasmic signals, such as changes in intracellular Ca²⁺ concentration and tyrosine phosphorylation of multiple targets. However, the specific signals elicited in each case that are essential for the response to force are likely to differ depending on which adhesion receptors are engaged and are only beginning to be cataloged.

The concept that cells not only respond to external forces but also generate their own forces, which they exert on their substrate, was shown by the wrinkling of soft rubber sheets by cultured cells in studies designed to define the mechanisms of cell locomotion (Harris et al., 1980). Further demonstration of the role of mechanical sensing came from experiments that used collagen-coated polyacrylamide gels as substrates for cell attachment that had identical surface topography and protein coating, but different rigidities. These studies showed that the morphology and motility of fibroblasts undergo dramatic changes in response to substrate stiffness. This was particularly evident as increased spread area and more intense staining by anti-phosphotyrosine antibodies as stiffness increased and a faster random motility on softer substrates. The size of focal adhesions also increased with increasing stiffness, with concomitant changes in the dynamics of focal adhesion proteins, such as vinculin, which becomes more stably anchored at the attachment sites (Pelham and Wang, 1997). These studies confirmed earlier evidence that found phenotypic differences between cells that had been cultured on substrates with different stiffnesses (Keese and Giaever, 1991; Weiss and Garber, 1952), and showed that the response of cells to these physical cues engaged the same intracellular signaling pathways as biochemical signals that are initiated at transmembrane receptors.

The impact of forces and mechanical environment on the structure and function of cells and tissues has been increasingly documented in many recent studies and summarized in recent reviews (Mammoto and Ingber, 2010; Tenney and Discher, 2009; Vogel and Sheetz, 2009). Many of these research efforts have been directed at demonstrating that physical features such as stiffness are direct stimuli for specific cellular responses, rather than attributable to biochemical differences in the substrates. In this Commentary, we discuss the differences between physical and chemical stimuli that can influence cell and tissue function, and consider the fundamental mechanisms that might enable cells to measure the stiffness of the extracellular matrix and of neighboring cells. Candidate proteins and signals that are involved in sensing or responding to forces and might also be relevant to stiffness sensing have been well discussed in other recent reviews (Koivusalo et al., 2009; Kumar and Weaver, 2009; Mammoto and Ingber, 2010; Tenney and Discher, 2009; Vogel and Sheetz, 2009).

How does mechanical signaling differ from chemical signaling?

In vivo, the behavior of cells and tissues is determined by a combination of biochemical and mechanical signals and, in some cases, the same molecules can serve in both signaling modes. For example, integrins (Friedland et al., 2009; Paszek et al., 2009) and T-cell receptors (Ma and Finkel, 2010) bind to chemical ligands in a highly specific manner, but their full activation appears to also require the application of force or resistance from a stiff matrix. The manner in which soluble ligands and forces impinge on cell receptors suggests interesting differences in their potential to transmit signals.

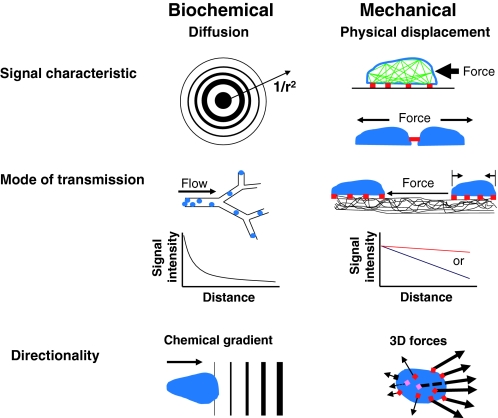
Chemical messengers either diffuse from their site of production to their target or are carried to their target by fluid flow. These processes confer limits on the length and time scales by which chemical signaling can operate. For example, on the plane of a flat surface, the diffusion of molecules from a point source produces a signal whose intensity decays rapidly – more than linearly – with the distance from the source (approximately as 1/r²); in 3D, the decay is even more rapid. The precise rate of signal decay over time depends on the rate of production and removal of the messenger molecule, but generally speaking, most autocrine or paracrine signals are effective only over distances of less than several tens of micrometers. Mechanical signals, which are quantified as the strain generated by a cell pulling on a simple elastic matrix with linear elasticity, decay according to 1/r. However, real tissues are not simple linear elastomers, but rather can be considered as networks of elongated polymers with a large separation between filaments, defined as the mesh size, and a highly non-linear viscoelasticity (see Box 1). In such networks, the application of force is distributed non-uniformly (Kang et al., 2009; Liu et al., 2007), with the consequence that the decay of the strain field, or distance over which significant deformation occurs, is much more gradual in some directions and that strains can be generated hundreds of micrometers away from the point of force application (Winer et al., 2009b) (see Fig. 1).

Fig. 1.

Comparison of biochemical and mechanical signaling. Biochemical signals can diffuse from a point source, such as a cell or a gland, or can be released into the circulation (top two illustrations on the left). In both cases, the strength of the signal decreases with distance from its origin at a rate of 1/r² (in a 2D system) or becomes diluted in the blood flow in circulation, as shown in the graph on the left. By contrast, mechanical signals are transmitted through the effects of the force on the target cell, typically causing its deformation. The mechanical signal can be applied directly to a single cell (top image on the right). Here, the cell is anchored to a matrix through focal adhesions (red squares) and its mechanical properties are determined by its cytoskeleton (actin fibers in green) and protein interactions with its plasma membrane (cell membrane in blue surrounding cell). Force could also be applied indirectly, from one cell to another, as shown in the second illustration on the right (cells in blue, cell–cell adhesion in red), or, alternatively, at a distance and transmitted through another structure such as the fibers in a matrix. This is shown in the third illustration on the right, whereby the cell on the right contracts, thus pulling the matrix through focal adhesions (red squares). The matrix fibers are displaced, immediately exerting force on the left cell through its focal adhesions (red squares). In principle, signals of this type do not significantly lose their intensity with distance (shown schematically in the graph below, red line). However, in reality, signal strength might be altered by the elastic properties of the transmitting substance. If the transmitting substance (here, the basement membrane with collagen fibers) is not rigid but elastic, the full force of the initial deformation might not be transmitted. Nevertheless, the strength of the signal does not decrease over distance or time to the same extent as chemical signals (blue line in the graph). The directionality of biochemical and mechanical signaling also differs. Cells respond to, and migrate in response to, chemical gradients by chemotaxis, as shown in the bottom image on the left, in which the intensity of the gradient is represented by the thickness of the vertical lines. Generally speaking, chemotaxis represents a simple 2D response. In a tissue, a cell responds to spatially complex mechanical stimuli (bottom illustration on the right). The arrows shown represent the direction and magnitude of forces impinging on a cell through its focal adhesions (red squares), resulting in a net force vector that is directed to the right.

Another difference between chemical and mechanical signaling is directional specificity. Chemical signals can exert directional control through chemotaxis but, at best, any directional information is only two dimensional and limited to the distance over which it can be perceived. However, mechanical stresses that are generated by fibers within the cell and transmitted through fibrous extracellular matrices (ECMs) can produce highly directionally specific signals and therefore convey complex spatial information. For example, the open fibrous meshworks of semiflexible polymers, such as those that form the cytoskeleton and the ECM, align their fibers along the direction of the strain as these networks deform and thereby transfer stress from filament bending to stretching as the strain increases (Didonna and Lubensky, 2005; Onck et al., 2005). As a result, the more cells strain their cytoskeleton and their ECM, the farther the stress can be transmitted along the fibers in the preferred direction. Eventually, this can lead to the formation of force dipoles in cells, in which the mechanical communication between the cell and the matrix and between different cells is maximized. This is because the forces are concentrated in the direction from one cell to another, rather than radiating equally in all directions as they would if an isotropic contraction was exerted on a uniform continuous elastic material (Schwarz and Safran, 2002; Zemel et al., 2010). Such effects might contribute to pattern formation during tissue development, as suggested by the concept of attraction fields (Weiss and Katzberg, 1952). This term is used to describe the phenomenon of directed growth and movement of distant cells from the ends of tissues, such as nerves that are embedded in plasma clots or other soft solids in which the growth and migration of cells between the separated tissues is highly oriented to form a bridge between the distant cell centers. By contrast, such outgrowth has a uniform radial pattern when only a single cell source is embedded in the same matrix. Whether this directed movement and growth result from mechanical stresses (tension), the orientation of fibrin strands caused by forces generated by the tissues or some other mechanism is as highly controversial now as it was 60 years ago, when quantitative measures, such as the distance dependence of the preferential growth, were first analyzed (Katzberg, 1951).

Another difference between chemical and mechanical signaling is the rate at which these types of signals can be generated or halted. Chemical signaling can be very rapid, as in synaptic signaling, but is slower in most other cases because the generation, transport and removal of diffusible messengers requires timescales of seconds to minutes, or even greater when de novo synthesis is required, such as in the production of cytokines. Diffusion and directed transport in flowing fluids also considerably constrain signal transmission distance, direction and duration. Mechanical signals, by contrast, can be generated rapidly, as already present motor proteins apply tension to the cytoskeleton that is then transmitted through the fibers of collagen or other ECM components. Once these fibers are in a tensed state, the mechanical signal is transmitted so fast that distance is no longer an important variable. The lifetime of a mechanical signal can be controlled by the time over which the force is maintained, or the decay of the stresses and deformations owing to the viscoelasticity of the biomaterials involved. It has also been proposed that mechanical stimuli are transmitted directly to their ultimate targets, rather than being translated first into chemical signals that subsequently initiate the eventual response (Wang et al., 1993). This concept of ‘hard-wired’ signaling is supported by studies that showed that the activation of intracellular and even nuclear proteins (Wang et al., 2009) occurred faster than would be expected if mechanical signals acting at the plasma membrane needed to be transduced first into biochemical messengers (Na et al., 2008).

The effect of stiffness on tissue dysfunction

Several examples, at either the level of organ function or the cellular and molecular level, have demonstrated that the mechanics of cells and tissues play a fundamental role in cell growth and differentiation, as well as in the development of disease. As discussed below, in each of these cases, mechanical factors are primary signals for specific cell or tissue behaviors, although they usually act in the context of additional biochemical signals.

Differentiation

Numerous recent studies in vitro have shown how the stiffness of the underlying substrate helps determine the form and function of cells in a highly cell-type-specific manner that is likely to have relevance to their function in vivo. When brain cortical cells are plated on soft substrates (0.15–0.30 kPa) that resemble the stiffness of normal brain, neurons grow selectively, but when they are plated on more rigid substrates (2 kPa), glial cells such as astrocytes are activated and proliferate. On the stiffer substrate, neurons are found less frequently and then adhere to astrocytes, which are soft (Lu et al., 2006), rather than adhering directly to the stiff gel (Georges et al., 2006). Mesenchymal stem cells (MSCs) differentiate along three lineages depending on the rigidity of their substrate, as determined by analysis of differentiation markers. When cells are grown on substrates in the range 0.1–1.0 kPa, which mimics the stiffness of brain, MSCs differentiate into neuronal cells, whereas the use of substrates with a similar stiffness to muscle (8–17 kPa) gives rise to myocyte-like cells and the stiffest substrates (25–40 kPa) result in osteoblast-like cells (Engler et al., 2007).

The stiffness (elastic modulus) of many soft tissues, including breast, lung, liver, kidney and some blood vessels, is in the range 0.2–4.0 kPa, (Levental et al., 2007) and the variance in stiffness for individual tissues types is often small, within 10–15% of the mean value (Georges et al., 2007). Under normal conditions, the controlled stiffness helps to maintain a specific differentiated cell phenotype and limits cell-cycle progression to maintain homeostasis. Increased stiffness in the range 12 kPa or greater can lead to aberrant cell-cycle progression and potentially abnormal tissue (Assoian and Klein, 2008; Klein et al., 2009; Kumar and Weaver, 2009; Levental et al., 2009). An example of this effect applied to stem cell biology is a recent study demonstrating that the mechanical environment of muscle stem cells is essential to their ability to proliferate and then differentiate into myocytes (Gilbert et al., 2010). Skeletal muscle has an elastic modulus of approximately 12 kPa and contains stem cells that can replace myocytes following injury. When mouse muscle stem cells are isolated from muscle and immediately transplanted into mouse tibialis muscles, they differentiate into mature myocytes; by contrast, if the stem cells are grown on plastic for seven days (elastic modulus >10⁹ Pa), they do not form myocytes. However, if the stem cells are isolated and grown on a matrix with identical chemical composition to the cells grown on plastic, but with an elastic modulus of 12 kPa, they form myocytes when transplanted into tibialis muscle. These results demonstrate that the mechanical environment of stem cells might be essential to their ability to maintain stem cell characteristics.

Cancer

Normal breast tissue is soft (approximately 0.2 kPa), whereas breast tumors are much stiffer (on the order of 4 kPa) (Baker et al., 2009; Levental et al., 2007). Dense and probably stiff breast tissue increases the risk of a patient developing breast cancer. Experiments using substrates with varying degrees of stiffness provide clues to the underlying molecular effects of increased matrix stiffness on breast tissue. For example, normal breast epithelial cells that have been plated on substrates with increasing stiffness within the range 0.17–1.2 kPa acquire progressively more abnormal characteristics, including loss of normal acinar structure and increased extracellular-signal-regulated kinase (ERK) and Rho activity (Paszek et al., 2005). It has been suggested that increased matrix stiffness is a first step in promoting an invasive epithelial cell phenotype through increased integrin-induced signaling. This is presumably achieved by increasing the resistance to internally generated tension, thereby enhancing myosin activity (Provenzano et al., 2009). Furthermore, studies of animal tumor models showed that increased collagen cross-linking and its stiffening through oxidation by lysyl oxidase induced invasiveness of oncogene-activated epithelial cells, generated larger focal adhesions and promoted phosphoinositide 3-kinase activity (Levental et al., 2009).

Cardiovascular disease

Cardiac myocytes require a specific mechanical environment for their optimal development and function. Normal cardiac muscle tissue has a stiffness of 10–15 kPa and, hence, embryonic cardiac myocytes that are grown under ‘normal’ matrix conditions, that is to say on matrices with a stiffness of ~11 kPa, maintain their differentiated state and beat. However, if myocytes are grown on a substrate with a stiffness comparable to that of a myocardial infarct scar (35–70 kPa), they lose their striations, their beating frequency decreases, and the fraction of cells that beat decreases from the typically greater than 30% to less than 10% (Engler et al., 2008).

Cardiac muscle must relax to allow filling of ventricles. In pressure-overloaded heart disease, the ventricle is stiffer than normal, resulting in diastolic heart failure. It was shown that muscle strips from these hearts are significantly stiffer than either normal or volume-overloaded muscle (Chaturvedi et al., 2010). The increased stiffness could be attributed to muscle hypertrophy, which is characterized by an increased number of elastic units arranged in a parallel manner. However, it was found that the muscle strips from pressure-overloaded hearts retain their increased stiffness after myofibrils and titin, proteins responsible for the elastic properties of muscle fibers, have been extracted, thus suggesting that the increased stiffness of diseased tissue is due to changes in the matrix, rather than in the cardiac muscle cells. The amount of collagen was similar to that in normal cardiac muscle, indicating that the increased matrix stiffness could not be explained by increased matrix collagen content. Consequently, the difference in matrix stiffness is most probably attributable to modifications of the matrix, such as increased cross-linking. However, in failing hearts that have dilated ventricles and a reduced ability to relax or to generate force, muscle strips were found to be softer than normal.

Arterial stiffness, which is measured clinically as pulse wave velocity (PWV), is another independent risk factor for, and cause of, adverse cardiovascular outcomes, such as myocardial infarcts, heart failure and strokes (Cecelja and Chowienczyk, 2009; Mitchell et al., 2010). Its development is governed by a number of factors, such as aging, blood pressure, genetic factors and systemic diseases (DeLoach and Townsend, 2008; Lacolley et al., 2009). Increased aortic stiffness causes increased cardiac work and impaired coronary artery perfusion by altering the timing of pressure pulse-wave reflection in the aorta (DeLoach and Townsend, 2008). The combination of cardiac-generated pressure waves and abnormal mechanical properties of the arterial system contributes to cardiac hypertrophy, that is, thicker heart muscle, leading to a stiffer heart, impaired cardiac performance and difficulty in supplying blood to the increased cardiac muscle mass.

Liver disease

The elastic modulus of normal liver is 0.4–0.6 Pa, but can increase to as much 15 kPa following injury and fibrosis (Georges et al., 2007; Wells, 2008). Like many other cell types that maintain a differentiated state and rarely divide under normal conditions, liver cells, including hepatocytes, stellate cells and portal fibroblasts, lose their differentiated characteristics and begin to divide more rapidly in response to increasing matrix stiffness (Li et al., 2007). Following deliberate injury in experimental studies, liver tissue becomes significantly stiffer, based on shear modulus measurements before increased amounts of collagen are detectable, a surprising finding because increased stiffness is commonly associated with increased fibrous tissue (collagen). This increase in stiffness can be prevented by inhibitors of lysyl oxidase, an enzyme that cross-links collagen (Georges et al., 2007). These results indicate that increased matrix stiffness is an early response to injury in this model and is associated with increased collagen cross-linking before changes in the synthesis of matrix components occur.

Renal glomerular disease

Reduced stiffness of renal glomerular podocytes, the cells that support the glomerular capillaries, might contribute to renal disease. For example, it was shown that conditionally immortalized glomerular podocytes from a mouse model of HIV-associated nephropathy (HIVAN) are substantially softer than normal podocytes (by 25%), as assessed by atomic force microscopy (AFM) and microaspiration (Tandon et al., 2006). At a stage of the disease that showed no detectable pathological changes, the glomeruli from these mice were 30% softer than normal glomeruli (1.5 kPa compared with 2.5 kPa, as measured by AFM) (Wyss et al., 2011). Glomeruli and podocytes in other disease models are also significantly softer than normal cells, indicating that the increased mechanical deformability of podocytes and glomeruli might be a common feature of a number of renal diseases. This could make these structures more susceptible to hemodynamic injury or could represent a mechanical environment that is inhospitable to normal glomerular cells, resulting in apoptosis or dedifferentiation.

Open questions in mechanosensing – dimensionality, distance and timescales

Several fundamental properties of cellular mechanosensors are unknown. Most mechanosensing studies of purified cells have been performed on the surfaces of gels or elastomers, rather than in 3D matrices, largely for the same reasons that nearly all studies of chemical sensing in vitro are done on transparent glass or plastic surfaces. This geometry might be appropriate for endothelial or epithelial cells, but any obtained results are risky to extrapolate to the 3D setting, in which fibrosis or tumor formation occur. Other important unknown factors are the time it takes a cell to detect substrate stiffness and the distance over which the cell makes its rheological measurement, that is, determining any deformation as a result of applied force. Understanding these parameters can help define the molecular mechanisms by which stiffness sensing occurs. For example, if micron-scale deformations are needed to detect stiffness, then single nanometer-scale proteins are probably not sufficient by themselves to accomplish this function and larger complexes, such as focal adhesions or actin bundles, need to be assembled for this purpose.

Three dimensions and two-dimensional substrates

In many cases, the trends observed with increasing stiffness in 2D systems are also observed in 3D systems of the same elastic modulus. For example, the elastic moduli of polyacrylamide gels that best support neurite extension in cortical neurons and that inhibit the activation of astrocytes are similar to the elastic moduli of fibrin gels that support the differential growth of either neurons or astrocytes grown in fibrin networks (Georges et al., 2006; Ju et al., 2007). Another example is the finding that extracellular substrate stiffness regulates endothelial cell stiffness, both within 3D collagen gels and on the surface of collagen-coated polyacrylamide gels (Byfield et al., 2009a). In other cases, the response of a cell to changes in the stiffness of simple linear elastic gels, such as polyacrylamide gels that are coated with adhesion proteins, differs from that of the same cell type bound to or within a 3D network made of the same protein. This is, for example, evident in the different morphologies of mesenchymal stem cells that are cultured on fibrin gels or fibrin-coated polyacrylamide gels with the same low-strain elastic modulus (Winer et al., 2009b). Differences in the morphology of cells bound in 2D or 3D are also strongly affected by formation of dorsal cell–ECM adhesions. A rapid, substrate-stiffness-dependent transition from a well-spread, flat morphology to an elongated bipolar or stellate morphology, which more closely resembles the in vivo structure, occurs when a second ECM surface is placed on top of fibroblasts initially cultured in 2D (Beningo et al., 2004; Beningo and Wang, 2007).

Distance over which mechanosensing occurs

One hypothesis for the distance over which stiffness is sensed considers a role for single proteins at cell–substrate adhesion sites, where, when stressed by molecular motors, individual proteins partially unfold to either relieve autoinhibition of their enzymatic activity or expose new substrate-binding sites (Brown and Discher, 2009). Examples of proteins implicated in force or substrate stiffness responses are talin (del Rio et al., 2009), vinculin (Ezzell et al., 1997; Grashoff et al., 2010) and filamin (Byfield et al., 2009b; Gehler et al., 2009; Kasza et al., 2009), which all are flexible proteins that help link actin filaments to membrane adhesion sites. The size of individual proteins is of the order of a few nanometers and the complexes required to link the cytoskeleton to the membrane are smaller than a micrometer, suggesting that mechanosensing requires only very small movements and that substrate stiffness can be probed with submicrometer resolution. Other models of mechanosensing suggest that the sensor involves transmembrane ion channels that are linked mechanically to the adhesion sites (Glogauer et al., 1997; Koivusalo et al., 2009) and, therefore, the size of the integrated sensor is likely to be considerably lager than nanometer scale. Alternative models suggest a delocalized distribution of stresses throughout the cytoskeleton or the presence of sites within the actin stress fiber, possibly distant from the membrane adhesion complexes, that can act as mechanosensors.

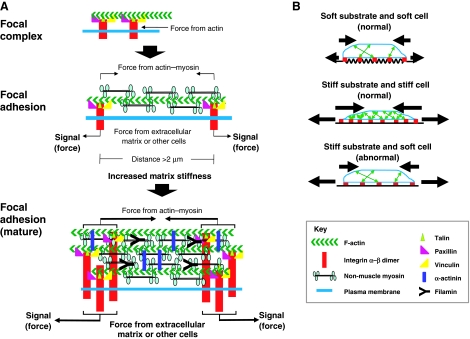
Recent studies of cells grown on thin flexible pillars have made it possible to estimate the distance over which cellular sensors measure stiffness. Arrays of either micrometer-sized polydimethylsiloxane (PDMS) posts or nanoscale silicon posts have been extensively used to measure the magnitude of force that cells apply locally to different regions of their adherent surface based on how they deflect these posts (Ghibaudo et al., 2008; le Digabel et al., 2010; Saez et al., 2007; Tan et al., 2003). In most initial studies, the posts have been sufficiently flexible so that their movement can be detected by high-resolution microscopy. Nevertheless, given the material properties of PDMS and the manufacturing constraints, the posts are so stiff that the cell cannot move them by more than approximately a micrometer. However, because the bending stiffness of a pillar varies inversely with the cube of its length, it is possible to produce much softer (more compliant) arrays of microposts by only modest changes in post length (Fu et al., 2010; le Digabel et al., 2010). For example, when the length of PDMS posts of 2 μm diameter is varied from 1 to 10 micrometers, which changes their bending stiffness by a factor of 1000, cells will deflect them more extensively and the overall cell morphology will resemble that of a cell that has been cultured on a soft gel rather than on a rigid surface. The integrated stiffness of a microarray of posts with diameters greater than a micrometer can be calculated from the properties of the individual posts and their density in the array. It has been shown that the morphology of cells grown on arrays of flexible posts is highly similar to that of cells grown on continuous gels of the same effective stiffness. This correlation has made it possible to determine a clear lower limit of the length scale of the stiffness-sensing apparatus. Independent of the diameter or length of a PDMS post, the elastic modulus of PDMS itself is constant and is very high (100,000 Pa) compared with the elastic modulus of soft gels (typically 100–10,000 Pa). On microarrays of posts, the only substrate integrins and other adhesion proteins can bind to is PDMS with its relatively high stiffness. However, the cell morphology appears to depend on the stiffness of the pillars when they are sufficiently flexible rather than on the stiffness of PDMS itself. Because the ‘soft gel’ morphology of the cells can be induced on arrays of pillars that are as large as 2 μm in diameter (Ghibaudo et al., 2008), as long as they are tall enough to be flexible, the cell appears to not measure stiffness over a distance of less than 2 μm. If it did, all the above arrays would appear stiff to the cell (i.e. the stiffness of PDMS), leading to a cell morphology resembling that grown on a rigid substrate. The greater than micrometer scale of stiffness sensing inferred from micropost experiments suggests that large complexes, such as stress fibers or focal adhesions (Heil and Spatz, 2010; Nicolas et al., 2008; Riveline et al., 2001), rather than single proteins linked to integrins, are potential stiffness sensors (Fig. 2).

Fig. 2.

Sensation of, and responses to, matrix-generated mechanical signals. (A) The basic molecular machinery that senses and responds to matrix-generated mechanical signals. When a cell encounters a matrix, integrins bind molecules in the matrix and additional proteins aggregate, forming a focal complex (top image). A focal complex contains integrins that connect the ECM to actin fibers, as well as additional essential proteins that participate in the activation and aggregation of integrins to link them to actin fibers. These proteins include talin, paxillin and vinculin. Other factors, such as kinases and phosphatases, which are also important for these processes, are not shown for simplicity. In the presence of force, probably generated by actin polymerization, additional integrins and other proteins aggregate and bind to F-actin fibers and non-muscle myosins, resulting in formation of a focal adhesion (middle image). The cell surveys its mechanical environment with periodic contraction of actin and non-muscle myosin stress fibers, which are attached to the integrins that pull against the matrix. Focal complexes and focal adhesions differ significantly in the content and phosphorylation state of their proteins, as well as their stability over time, focal contacts being transient unless they mature into focal adhesions. An important difference between the two structures is the presence of non-muscle myosin in stress fibers that join focal adhesions. The presence of non-muscle myosin permits generation of significantly more force than can occur with actin polymerization alone; cells without non-muscle myosins cannot sense matrix stiffness. Over time, and as a result of mechanical force acting on the integrins from the actin fibers and non-smooth muscle myosins, the aggregation of these additional proteins results in development of mature focal adhesions (bottom image). Additionally, proteins including α-actinin, filamin and cortactin cross-link actin fibers, thereby adding mechanical strength to the actin cytoskeleton and, consequently, the cell overall. Filamin and α-actinin also participate in linking actin fibers to integrin β subunits. If the cell finds itself on a matrix with increased stiffness (as indicated by thicker and longer force arrows at the bottom of the integrins in the bottom illustration), the cell senses the increased stiffness through reduced ability of the non-muscle myosin–actin fibers to contract against the focal adhesions attached to the matrix and displace it. This process leads to an increase in integrin aggregation and thus enlarged focal adhesions through further aggregation of proteins and additional actin fibers with more contraction force. The cellular cytoskeletal and contractile elements increase their force of contraction to match the new increased stiffness of the matrix. The cell spreads on the matrix by pulling against it and sending out lamellipodia that establish new focal complexes that mature into focal adhesions under stress (transition from the middle to the bottom image; see also transition between top and middle illustration in B). At some point, which is characteristic of each cell type, the cell reaches its maximum stiffness value; this might be less than that of the matrix. As the stiffness of the cell approaches its maximum value, thick bundles of actin, often called stress fibers, form. Stress fibers bridge focal adhesions and result in a stiffer cell. Their formation is illustrated by the transition from the middle to the bottom image here, and also in the transition between the top and the middle illustration in B. (B) The responses of normal cells to soft and stiff matrices (top two illustrations) and of an abnormal cell (bottom image) that is unable to sense matrix stiffness. In the top illustration, a cell is shown on a soft matrix, represented by a wavy black line, indicating that the cell can contract against the matrix and deform it. This cell has only a few focal adhesions (red squares) and actin fibers (green arrows). When the same cell is placed on a stiffer matrix, which the contractile apparatus of the cell cannot deform (middle), the number of focal adhesions increases. The number of actin–non-muscle myosin stress fibers and their thickness also increase, leading to cell spreading and stiffening. In disease states such as cancer and scarring, cells might encounter abnormally stiff matrix and therefore take on abnormal mechanical and cell biological characteristics. The illustration at the bottom of the panel shows a cell that cannot sense or respond to matrix-generated mechanical signals on a stiff matrix. The cell remains soft with only a few focal adhesions and actin fibers. Cells with these characteristics are found, for example, in filamin-null M2 melanoma cells, in cells that lack integrins, in glomerular podocytes from a mouse model of HIV-associated nephropathy, in α-actinin-4-null mice and in cells without functional non-muscle myosins. These cells all have defects in adhesion and migration, and show increased susceptibility to injury by mechanical force.

Time dependence of mechanosensing

Resolving the timescale of mechanosensing is complicated by the fact that the observable properties of cells – for example, shape, stiffness, cytoskeleton or focal adhesion assembly, protein phosphorylation – are the result of mechanosensing, followed by transduction of the mechanical signal to an intracellular signal and finally a response that causes a detectable change in the cell properties. The observed time needed to respond to a change in the mechanical properties of a substrate, for example, when a cell crosses the boundary between hard and soft substrates, or a change in the strength of a laser trap that the cell is held in, determines the upper limit for the time needed to detect substrate stiffness. The fastest of these observable changes tend to be on the order of tens of seconds to minutes. For example, when fibroblasts in suspension are plated on gels that have different stiffnesses but are equally adhesive, the earliest time point at which they are measurably different by commonly used parameters (adhesion, morphology, spreading) is 2 minutes (Yeung et al., 2005). A lower limit for the timescale of mechanosensing is likely to be the fastest active movement, such as the cycling time of a molecular motor, which is in the range of milliseconds. A more accurate estimate of the time needed for mechanosensing can be inferred from comparing the phenotypes of cells grown on substrates with a constant, time-independent stiffness and those of cells in a material such as a tissue whose stiffness varies over time, as discussed below.

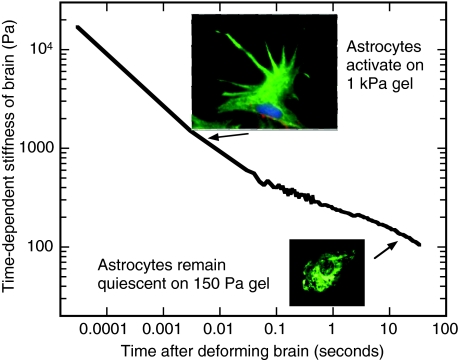
Measurements of the rheology of intact tissues over time show that soft tissues, for example, brain and liver, are not simple elastomers such as polyacrylamide gels, but exhibit a high degree of stress relaxation that can be quantified in the form of a time-dependent elastic modulus (see Box 1) (Georges et al., 2006). Consequently, the resistance to forces applied by the cell will diminish the longer the force is applied, and the magnitude of mechanical resistance to which the cell responds can be used to calculate the time constant at which its mechanosensor responds. For example, the stress relaxation of freshly excised normal rat brain deformed by simple shear is shown in Fig. 3. On the timescale from 0.03 to 60 seconds, the elastic modulus of the brain decreases from 600 Pa to 100 Pa. At the higher frequencies used in magnetic resonance imaging elastometry (equivalent to 0.003 seconds), the shear modulus for brain is reported as 1500 Pa (Sack et al., 2008); at the even shorter timescale relevant to its deformation during trauma, brain stiffness approached 20 kPa (Nicolle et al., 2004).

Fig. 3.

Time-dependent stiffness of rat brain at 10% shear strain. The shear modulus measured after imposition of a 10% strain decays over time as the tissue rearranges to relieve stress. The images show the structure of rat astrocytes, visualized by staining for the intermediate filament glial fibrillary acidic protein. The astrocytes were cultured on polyacrylamide gels of constant time-independent stiffnesses that mimic either the rapid elastic response (1.1 kPa, top) or the long-time elastic response (150 Pa, bottom) of the intact brain.

Studies of purified rat astrocytes that have been cultured on polyacrylamide gels, which have a constant time-independent elastic modulus, show that stiffnesses of 500 Pa are sufficient to trigger astrocyte activation, as is evident from their multi-armed star-shaped morphology (Georges et al., 2006) (Fig. 3 inset), whereas shear moduli of 50 Pa maintain astrocytes in the round, resting state that is dominant in the normal brain. Similar studies showed that the branching efficiency of cultured rat neurons is optimal below 300 Pa, but decreases to low levels at 600 Pa (Flanagan et al., 2002). Assuming that astrocytes are mainly quiescent in the normal brain, these data suggest that the time constant for mechanosensing by these cells is in the range near or above 0.1 seconds. If astrocytes deformed their surroundings at a rapid rate and measured stiffness on a timescale of milliseconds, the resulting elastic modulus would be sufficiently large to trigger their activation, whereas slower deformations and perhaps integration of the stiffness response over longer timescales would allow the surrounding tissue to relax and produce an effective elastic response equivalent to 100 Pa, therefore preventing spontaneous activation. A time constant on the order of 0.1 seconds means that mechanical signals are sensed and integrated, and responses are observable at approximately 10-second intervals. Potential mechanisms that take place and can be detected over this time frame include protein folding or unfolding (e.g. of talin, vinculin or filamin), Ca²⁺ oscillations, phosphorylation-dephosphorylation reactions (non-receptor kinases such as FAK), limited protein degradation (filamin) and movement of proteins in the cell, such as aggregation of integrins and accumulation of proteins at focal complexes or adhesions (Fig. 2). Faster processes, such as molecular motor duty cycles or molecular motor slippage in response to force (Chan and Odde, 2008), which take place on much shorter timescales, probably also contribute to mechanical signaling, but alone might not be sufficient to elicit the full response.

Conclusions

Mechanical and chemical signals complement each other in biology. A number of phenomena, such as cell shape and differentiated phenotype, and elastic properties cannot be explained by chemical signals alone. Mechanical and chemical signaling have distinct characteristics, despite the fact that they share many intracellular molecules and processes. Chemical signals, with which we are most familiar, decay rapidly in strength with distance from their source and so are usually meaningful over relatively short distances. Because they rely on diffusion or need to be carried in fluids or gases, chemical signals generally travel slowly. By contrast, mechanical signals, transmitted by tensed networks of fibers or other substances, travel rapidly over long distances, and might be terminated equally fast. Finally, mechanical signals can contain complex spatial information from multiple sources, whereas chemical information is usually restricted to relatively simple chemical gradients.

Disruption of the normal mechanical environment can perturb cell function to the same extent as chemical stimuli, and new methods to either measure or impose small, biologically relevant forces acting on cells have demonstrated the potential of mechanosensing and mechanotransduction to collaborate with chemical stimuli to control cell and tissue function. Cells and tissues have tightly controlled elastic properties (Levental et al., 2007) that are specific to their cell type and functions, and that are determined by their intrinsic mechanical properties and interactions with their mechanical environment. Abnormalities in cell mechanical properties or mechanical environment can result in altered cell function and disease, including malignancy, loss of stem cell potential and cardiac hypertrophy.

A number of questions regarding the mechanisms by which cells sense force and measure stiffness remain unresolved and should provide many opportunities for discovery. The mechanisms involved in mechanical signaling are only beginning to be revealed. Although integrins, the cytoskeleton, the proteins that connect them, non-muscle myosins, kinases and phosphatases are all involved, precisely how cells measure the stiffness of their environment is not fully understood. Based on studies with microfabricated pillars, cells appear to require distances greater than 2 μm to measure the stiffness of their environment, but the precise spatial requirements are not defined. Similarly, the time required for mechanosensing is not well defined, in part because the efferent limbs of the generally accepted cellular responses to changes in mechanical environment – cell spreading, adhesion or shape change – require substantial amounts of time to observe, thus overestimating the time frame for receiving and processing mechanical signals. A lower estimate of the time required for mechanical signaling can be derived from studies of the time dependence of stiffness in the brain, but even these estimates suggest a time frame of approximately 10 seconds. This estimate is probably too high, again because of the slow observation time for cellular responses. Finally, although it is clear that cells can sense and respond to complex 3D mechanical information, how they process this information to result in an integrated cell response and why the response can differ significantly between cells in chemically similar 2D and 3D environments is not known.

The ability of cells to differentiate appropriately, maintain their differentiated state and function normally is likely to depend on their biochemical and mechanical environments, but how the mechanical environment can be controlled in vivo is not clear. Although tissue stiffness appears to be important in many types of tumors, it remains to be determined how this factor can be defined and exploited in a range of tumors for therapeutic benefit. Finally, the role of tissue mechanics in response to injury and aging, and in diseases of organs including the kidney, liver, brain and cardiovascular system, as well as approaches to modify it, are a promising area for future discovery and applications.