Interactions among thermal parameters determine offspring sex under temperature-dependent sex determination

Abstract

In many animals, temperatures experienced by developing embryos determine offspring sex (e.g. temperature-dependent sex determination, TSD), but most studies focus strictly on the effects of mean temperature, with little emphasis on the importance of thermal fluctuations. In the jacky dragon (Amphibolurus muricatus), an Australian lizard with TSD, data from nests in the field demonstrate that offspring sex ratios are predictable from thermal fluctuations but not from mean nest temperatures. To clarify this paradox, we incubated eggs in a factorial experiment with two levels of mean temperature and three levels of diel fluctuation. We show that offspring sex is determined by an interaction between these critical thermal parameters. Intriguingly, because these two thermal descriptors shift in opposing directions throughout the incubation season, this interactive effect inhibits seasonal shifts in sex ratio. Hence, our results suggest that TSD can yield offspring sex ratios that resemble those produced under genotypic sex-determining systems. These findings raise important considerations for understanding the diversity of TSD reaction norms, for designing experiments that evaluate the evolutionary significance of TSD, and for predicting sex ratios under past and future climate change scenarios.

1. Introduction

Most species exhibit some form of genotypic sex determination (GSD), whereby offspring sex is determined by genetic factors (e.g. sex chromosomes) inherited at conception. However, numerous organisms exhibit environmental sex determination (ESD), in which offspring sex is determined by environmental conditions experienced during development [1]. A critical difference between genotypic and environmentally based systems (aside from the mechanisms involved) is that the primary sex ratios produced will often differ. Genotypic systems are expected to yield evolutionarily stable sex ratios, with sons and daughters often produced in equal numbers [2]. Environmental systems, however, often lead to biased primary sex ratios, which could have negative consequences on demographics of populations [3,4]. Because extreme biases in primary sex ratios have been observed in natural populations (e.g. [5]), understanding these patterns has been a major focus in evolutionary biology.

A common form of ESD is temperature-dependent sex determination (TSD), whereby temperatures experienced during embryogenesis determine offspring sex. TSD occurs in a diversity of organisms [6], and includes a diversity of reaction norms (e.g. which sex is produced at which temperature). Classifying a species as having TSD tells us only that temperature somehow affects the relative numbers of sons versus daughters emerging from eggs at the end of incubation; it does not tell us how temperature influences the genetic and physiological pathways which direct sexual differentiation. Additionally, classifying a species as TSD conveys no information about which attributes of nest thermal regimes influence sex determination. Most published studies have not incorporated the complexity of environmental stimuli experienced by embryos within a natural nest. Indeed, almost all laboratory-based studies that describe sex-determination reaction norms are based upon constant-temperature incubation (e.g. [7]), yet we know that temperatures in natural nests fluctuate daily and seasonally [8,9]. Importantly, thermal fluctuations experienced by embryos can have significant effects on offspring phenotypes, including sex ratios [10–14].

How can we best incorporate this kind of real-world complexity into our understanding of the thermal parameters that affect offspring sex? In general terms, the two most important attributes of any dataset involve a measure of central tendency (mean or mode) and a measure of dispersion (variance or standard deviation). Minimally, then, we should explore the relative significance of mean values and diel ranges as thermal cues for sex determination. Even if we restrict analysis to these two parameters, however, an important complication arises: embryogenesis typically takes weeks or months, raising the possibility of complex interactions between thermal means and variances over that period. For example, if the primary cause for thermal variation from one day to the next involves sunny versus cloudy weather (and hence, variation in the degree to which a nest is heated by solar radiation), then nightly minimum temperatures inside a nest probably will be fairly constant, and the days with high mean nest temperatures will be those with high maximum nest temperatures (and thus, a high diel range). Over longer periods, seasonal shifts may create the opposite correlation between daily means and diel variances: for example, the level of disparity between day and night temperatures will shift from early spring into mid-summer. Because night-time temperatures drop relatively low during early spring, we might expect high thermal fluctuations around a low mean temperature within nests at this time. Later in the summer, when days are warm, night temperatures do not drop as low as those during early spring, resulting in more stable thermal conditions and an overall greater mean temperature. Additionally, mean ground temperatures (important for species that construct subterranean nests) may rise in summer at the same time that increasing vegetative growth insulates the soil surface from radiant heating. Overall, we might expect the correlation between thermal means and variances inside a nest to range from positive in some cases, and over some timescales to negative under other conditions.

How can we best clarify the relative effects of thermal means and variances on sex determination? Two kinds of data are needed. First, to ensure relevance of the results to natural conditions, we can monitor thermal regimes (both means and fluctuations) within natural nests, and document the sex ratios of offspring emerging from those nests (e.g. [5]). Second, we can incubate eggs under standardized conditions in the laboratory, manipulating both means and fluctuations in thermal regimes to characterize the sex-ratio responses to each combination of thermal means and ranges. Here, we conducted such a study with a lizard from southeastern Australia. The jacky dragon (Amphibolurus muricatus) exhibits a pattern of TSD whereby laboratory incubation at extreme constant temperatures (less than 26°C and more than 30°C) produces female-biased sex ratios, and intermediate constant temperatures (26–30°C) produce nearly balanced sex ratios [15]. Field data, however, suggested a more complex picture: mean nest temperature did not predict offspring sex ratios [16]. To clarify this paradox, we examined data on thermal regimes in natural nests in more detail to explore relationships among thermal means, thermal fluctuations and offspring sex ratios. Additionally, we designed an experiment to deconfound the impacts of thermal means and diel fluctuations on phenotypic traits (including sex) of the offspring. By mimicking a range of thermal environments that eggs experience naturally, such controlled laboratory experiments will provide critical insights into the degree of primary sex-ratio skews that occur under TSD in nature.

2. Material and methods

(a). Field nests

Data for nest temperatures in the field were obtained during previous studies [16,17], but our methodology is described briefly below. To locate field nests during the Austral spring and summer (in 2005/2006), gravid A. muricatus were fitted with radio-transmitters and then released at Royal National Park, 32 km south of Sydney, Australia. Females were relocated every 1–2 h and observed for signs of nesting behaviour. Immediately after nesting occurred, we carefully excavated each nest to count and weigh the eggs. After egg removal, we measured the depth of the nest and then dug a small cavity on the side of the main nest cavity (at mid-depth), enabling us to place a thermochron iButton (model DS1921: Dallas Semiconductors, Texas; programmed to record hourly temperatures) among the eggs. Eggs were then placed back inside each nest in the same order as they had been removed. Prior to covering the eggs with substrate, we measured the depth to the top egg.

We made multiple trips back to the study site to collect the unhatched eggs and iButtons. Eggs were incubated in the field for an average of 62 days (s.d. = 5.5, range = 53–70 days), resulting in an average of 86 per cent of the total incubation period in the field, which is after the critical period when offspring sex is determined [18]. Eggs were placed in individual glass jars (covered with plastic wrap and sealed with a rubberband) with moist vermiculite (−200 kPa). All eggs experienced a constant 28°C for the remainder of incubation (mean ± s.d. days in the laboratory before hatching = 10.2 ± 6.7, range 0–24 days). Jars were checked twice daily for hatchlings. We recorded hatching date and hatchling sex [19].

(b). Laboratory incubation experiment

To explain relationships (or lack thereof) observed in the field, we conducted a laboratory incubation experiment during the following spring/summer (in 2006/2007) to understand how thermal means and diel fluctuations during embryonic development directly impact offspring phenotypes and sex ratios. Thus, the laboratory incubation experiment used ecologically relevant temperature regimes that mimicked the variation observed in the field nests from the previous year.

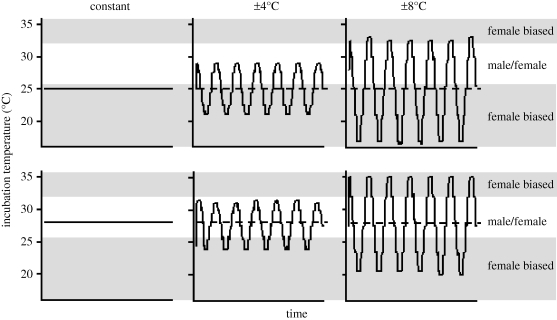
During 2 October to 12 November 2006, clutches of jacky dragon eggs were collected from 22 females in our wild-caught breeding colony [20] within 24 h of oviposition. All eggs were weighed, and then placed in individual glass jars (125 ml) containing moist vermiculite (−200 kPa). Glass jars were covered with plastic wrap (sealed with a rubberband) to prevent moisture evaporation, and then placed in one of six programmable incubators. Accordingly, eggs from each clutch were allocated among six experimental treatments in a 2 × 3 factorial design (two levels of thermal means and three levels of thermal fluctuation; figure 1). Eggs allocated to two control treatments were kept at constant mean temperatures of 25°C and 28°C. We chose a mean 25°C incubation temperature because this was the overall average nest temperature in the field. The 28°C mean incubation temperature was chosen because this was the upper-most extreme mean nest temperature in the field. Additionally, these two mean temperatures produce significantly different offspring sex ratios under laboratory incubation [15]. Eggs allocated to the remaining four treatments were placed in incubators that fluctuated either ±4° or ±8° around mean temperatures of 25°C and 28°C. These diel temperature fluctuations were chosen because they encompass typical levels seen in natural nests (figure 2). Eggs were thus exposed to variable periods each day within temperature ranges that are known to produce female-biased and balanced sex ratios under constant temperature incubation (figure 1). Egg jars were rotated within each incubator three times per week to minimize potential effects of spatial thermal variation within incubators. Two iButtons in each incubator were checked on a weekly basis to ensure proper thermal cycling.

Figure 1.

Experimental design illustrating three levels of daily temperature fluctuations around two mean temperatures (25°C or 28°C). Eggs were exposed to six temperature regimes using programmable incubators. Dashed lines represent the mean incubation temperature in the fluctuating treatments. Shaded areas of the graph illustrate ranges of incubation temperature that produced female-biased sex ratios under constant temperature incubation. Unshaded areas represent constant incubation temperatures that produce about 1 : 1 sex ratios [15]. The x-axis represents only about 6 days of the total incubation period.

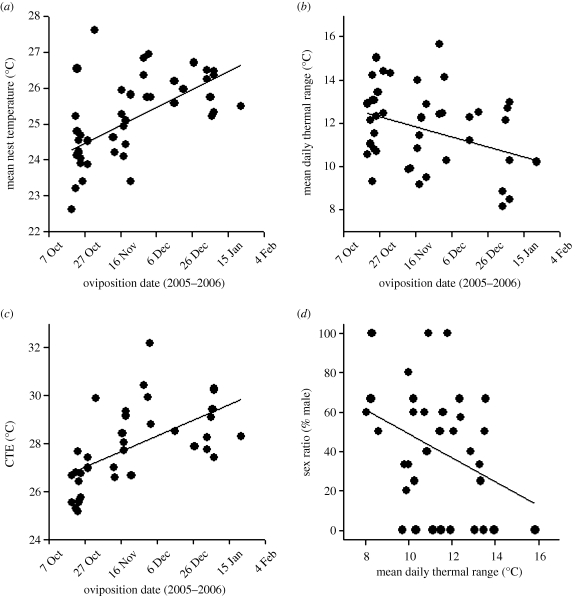
Figure 2.

Relationships among oviposition date and sex ratio with thermal parameters in natural nests of the jacky dragon (Amphibolurus muricatus). (a) Oviposition date versus mean nest temperature, (b) oviposition date versus mean daily thermal fluctuation, (c) oviposition date versus constant temperature equivalent (CTE) and (d) mean daily thermal fluctuation versus sex ratio. Statistics are reported in the text.

After eggs hatched, we recorded hatching date and measured hatchling phenotypes (snout-vent length (SVL), tail length (TL), body mass, and sex). All hatchlings were uniquely marked by toe-clipping. At approximately two weeks of age, locomotor performance of each hatchling was measured on a 1 m long electronically timed race track (see methodology in Warner & Shine [21]). All trials were conducted at 32°C, which is near the preferred body temperature of A. muricatus [22]. Hatchlings were housed in outdoor enclosures (1.3 m long × 0.75 m wide × 0.55 m tall), and remained in these enclosures until 7 March 2007, at which time the young lizards were remeasured (SVL, TL and mass) and released at the field site.

(c). Statistical analyses

All dependent variables were inspected for normality and log-transformed when necessary. Data analyses were performed with SAS software (v. 9.1 [23]). For the field data, we used a series of linear regressions to evaluate relationships between oviposition date (expressed as Julian date), nest depth (to nest bottom and to top egg) and nest temperature. Nest temperature was characterized by mean temperature and mean daily thermal fluctuation (thermal range divided by 2). The effects of these nest variables on clutch sex ratio were evaluated with the Genmod procedure in SAS [24]. The model contained a binomial error structure with a logit link function, and sex ratio (proportion of males in each clutch) was the dependent variable, and oviposition date, nest depth, mean nest temperature and mean daily thermal fluctuation were independent variables. Nest temperature was restricted to data collected during the first 60 per cent of incubation because this period reflects the critical time when embryonic sex determination is responsive to external cues [18]. Because about a third of development occurs prior to oviposition in squamates, this first 60 per cent of incubation probably corresponds to the thermo-sensitive period (TSP; typically, the middle third of development) identified in other reptiles with TSD (e.g. [25]).

To further analyse the relationship between natural thermal regimes and sex ratio, we converted thermal profiles of nests to constant temperature equivalents (CTE). Details of the CTE approach can be found elsewhere [26,27], but briefly, this approach transforms a fluctuating thermal regime into a value that is equivalent to a constant temperature incubation regime. More specifically, the CTE is a temperature value above and below which half of the development occurs; this statistic is calculated on a daily basis and incorporates thermal means and variance, as well as extreme fluctuations beyond temperatures that inhibit development (e.g. the developmental zero; see the electronic supplementary material, figure S1). Using the models described by Georges et al. [9,27], we calculated CTEs from thermal profiles from each nest, focusing only on data during the TSP (i.e. first 60 per cent of the incubation period). After CTE values were calculated for each nest, we used linear regression to evaluate relationships among oviposition date, nest depth and CTE. The relationship between CTE and clutch sex ratio was evaluated with logistic regression using the Genmod procedure in SAS.

For the laboratory data, because all eggs were from first clutches of the season, our experiment removed potentially confounding factors owing to differences among successive clutches [20]. In addition, because the average clutch size is six eggs for jacky dragons [15], more than one egg per clutch was rarely (on only 11 instances out of 120 eggs) allocated to the same treatment, thereby minimizing problems associated with pseudoreplication at the clutch level. We used logistic regressions (Logistic procedure in SAS) to analyse egg hatching success and hatchling sex ratios. For analysis of egg survival, we included initial egg mass, thermal mean, thermal fluctuation and their interactions as independent variables, and hatching success (whether eggs hatched or not) was the binomial response variable. For hatchling sex ratio, the two treatment effects (temperature mean and thermal fluctuation), their interaction and maternal identity were used as independent variables, and offspring sex (male versus female) was the binomial response variable. To evaluate the use of CTE models for A. muricatus when incubating eggs under controlled fluctuating temperatures, we converted the experimental incubation regimes into CTEs and made qualitative comparisons of the resultant sex ratios from the present study with those of a previously published constant temperature incubation experiment [15].

Most of our analyses of offspring phenotypes employed three-way mixed model analyses of variance or covariance, using thermal mean, thermal fluctuation, hatchling sex and their interactions as fixed effects, with maternal identity as a random effect. Because ‘thermal mean × sex’ and ‘thermal fluctuation × sex’ interactions never approached significance for any trait, these interaction terms were dropped from the final models; final analyses included the three main effects, as well as the ‘thermal mean × thermal fluctuation’ and the ‘mean × fluctuation × sex’ interactions. As dependent variables, incubation duration was defined as the number of days between oviposition and hatching; initial egg mass was used as a covariate in analyses of SVL and body mass; SVL was used as a covariate for analyses of TL and body condition (i.e. body mass adjusted for SVL). Growth rate was measured as the difference between hatchling size at release and at hatching divided by the number of days between measurements. Maximal running performance was evaluated as the fastest 25 cm distance, and analyses used hatchling SVL (measured immediately prior to racing trials) as a covariate. We also evaluated treatment and sex effects on the number of stops that hatchlings made over the entire 1 m distance.

3. Results

(a). Nest thermal regimes in the field

Mean temperatures and daily thermal fluctuations inside nests (n = 44) varied considerably and shifted across the season (figure 2). Mean nest temperature ranged from 23°C to 28°C, but owing to daily fluctuations, temperature extremes ranged up to 44.5°C and down to 11.5°C for brief periods of time on some days (for nests with at least one surviving egg). During the TSP for sex determination, mean daily thermal fluctuations inside nests generally varied from about ±4°C to ±8°C (i.e. thermal ranges of 8–16°C, respectively). Eggs laid later in the season experienced higher mean temperatures (r = 0.59, p < 0.001; figure 2a), but smaller daily thermal fluctuations than those laid early in the season (r = −0.34, p = 0.023; figure 2b). When we accounted for thermal means and fluctuations using CTEs, eggs laid earlier in the season experienced temperatures equivalent to relatively low constant temperature incubation, whereas eggs that were laid later in the season experienced higher CTEs (r = 0.59, p < 0.001; figure 2c). Depth to the top egg in a nest (range: 1.2–8.0 cm) and to the bottom of a nest (range: 4.1–9.8 cm) was not related to mean nest temperature (p-values more than 0.469) or mean daily thermal fluctuation (p-values more than 0.05).

Clutch sex ratio was neither related to mean nest temperature during the TSP (χ² = 1.2, p = 0.276) nor to nest depth (to top egg: χ² = 1.6, p = 0.214; to nest bottom: χ² = 1.0, p = 0.325). Despite the seasonal increase in mean nest temperature and CTE, sex ratios did not exhibit seasonal shifts (χ² = 0.25, p = 0.617). Accordingly, sex ratio was not related to CTE (χ² = 0.93, p = 0.334; electronic supplementary material, figure S2). However, clutch sex ratio was negatively related to the mean daily thermal fluctuation during the TSP (χ² = 5.0, p = 0.026; figure 2d).

(b). Laboratory incubation experiment

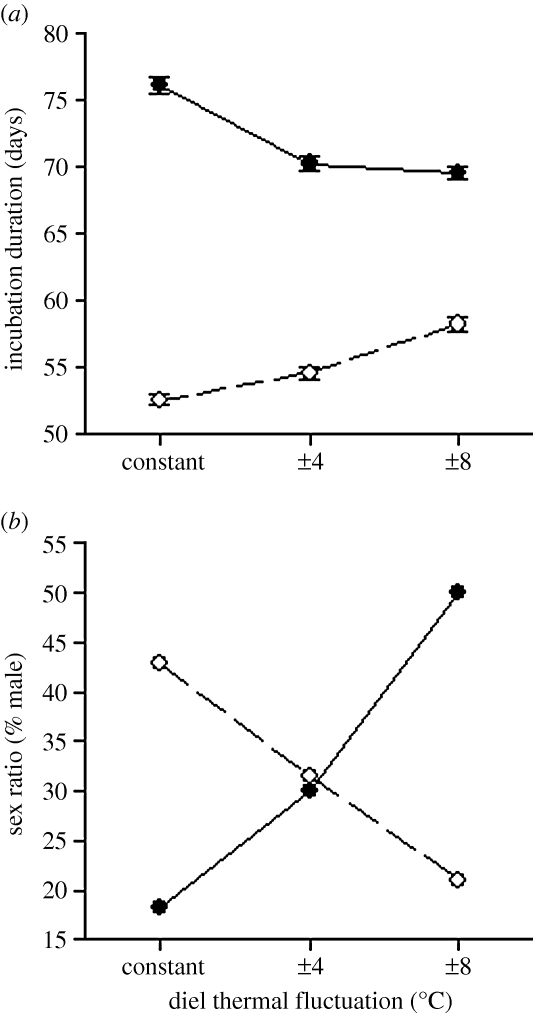
Thermal means and fluctuations did not affect egg hatching success (93% overall egg survival), but had substantial effects on other aspects of development (electronic supplementary material, table S1). Incubation duration was affected not only by thermal means, but also by thermal fluctuations and the interaction between these two temperature descriptors (thermal mean × fluctuation interaction: F_2,86 = 99.8, p < 0.001; figure 3a). Overall, embryonic development was faster when eggs were incubated at a higher mean temperature (28°C versus 25°C), but the influence of thermal fluctuations on incubation duration depended on mean temperature. At a mean of 28°C, thermal fluctuations delayed hatching; whereas at a mean of 25°C, greater diel fluctuations accelerated hatching.

Figure 3.

Effects of thermal means and diel fluctuations during laboratory egg incubation on (a) incubation duration and (b) hatchling sex ratios. Error bars represent 1 s.e. (filled circles, 25°C mean; open circles, 28°C mean).

Thermal fluctuations affected hatchling sex ratio, but the direction of this effect depended upon mean incubation temperature (thermal mean × fluctuation interaction: χ² = 6.7, p = 0.035; figure 3b; see the electronic supplementary material, table S1). A mean constant incubation temperature of 25°C produced predominately female offspring, but as daily temperature fluctuations increased, sex ratios shifted towards 1 : 1. The reverse pattern was observed when eggs were incubated at a mean temperature of 28°C. A constant 28°C incubation temperature produced a nearly balanced sex ratio, but increasing fluctuations around this mean generated increasingly female-biased sex ratios. After converting fluctuating incubation regimes into CTE values, our resultant sex ratios did not agree closely with those from a previous constant temperature incubation experiment (electronic supplementary material, table S2).

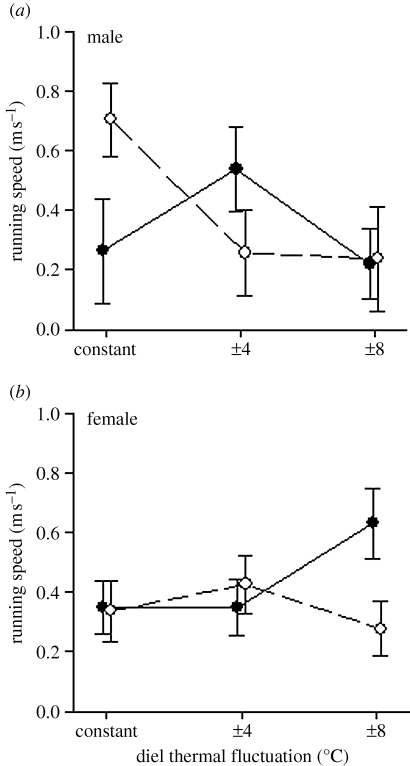
Incubation temperature had little effect on other hatchling phenotypes (table S1). Hatchling morphology was affected by diel thermal fluctuations but not by mean incubation temperature. Eggs exposed to fluctuating temperature regimes produced larger offspring (in SVL) with longer tails (relative to SVL) than did eggs from constant-temperature incubation (electronic supplementary material, figure S3). The interactive effect of these two thermal parameters on running speed differed between male and female offspring (three-way interaction: F_5,80 = 2.8, p = 0.024; figure 4). Locomotor performance of male offspring was enhanced when eggs were incubated at a constant 28°C, a temperature that yields a 1 : 1 sex ratio. The opposite pattern was observed for female offspring, whereby locomotor performance was enhanced when temperatures fluctuated ±8° around a mean of 25°C (also a thermal regime that produces a 1 : 1 sex ratio).

Figure 4.

Effects of thermal means and diel fluctuations during laboratory egg incubation on running speed (over 25 cm) for (a) male and (b) female hatchlings. Least-squares means are reported. Error bars represent 1 s.e. (filled circles, 25°C mean; open circles, 28°C mean).

4. Discussion

(a). Incorporating multiple thermal parameters into TSD reaction norms

Although TSD researchers have long acknowledged the importance of thermal fluctuations in sex determination (e.g. [8,28]), reaction norms that describe TSD are still based upon constant temperature incubation. For example, patterns of TSD are invariably classified under three main types that describe different directions and shapes of the relationship between sex ratio and constant incubation temperature (i.e. type Ia = males produced at cool temperatures and females at warm temperatures; type Ib = females at cool temperatures and males at warm temperatures; type II = females at both extremes and males at intermediate temperatures). These reaction norms have been the basis of standard classification schemes ever since the early descriptions of TSD [6,29]. Based on our findings and those of other studies [8,11,14,28], we suggest that the traditionally used reaction norms that describe TSD are oversimplified, because both means and fluctuations interactively affect sex determination. Here, we provide further evidence that we need to evaluate multiple thermal parameters to fully understand reaction norms in nature. Fortunately, controlled experiments that mimic fluctuating natural regimes are becoming increasingly common, and we urge researchers to use more natural thermal regimes in future studies.

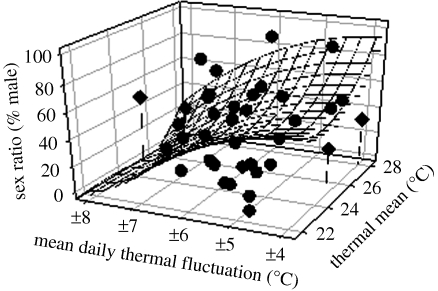
Studies that take a multi-dimensional approach by incorporating both thermal means and diel variation may more accurately depict reaction norms in nature. A similar approach has previously been used to predict sex ratios from natural nests in turtles [28], suggesting that three-dimensional reaction norms may be more useful in visualizing patterns of TSD (figure 5) than are the traditionally used two-dimensional representations. This approach will undoubtedly increase the complexity and diversity of TSD patterns. For example, a three-dimensional reaction norm will reveal many thermal conditions that produce 1 : 1 sex ratios, rather than just one or two pivotal temperatures that define the traditionally used TSD patterns [28]. Additionally, laboratory-based experiments that manipulate both thermal means and variances can be used to characterize a three-dimensional surface, which can then be used to predict clutch sex ratios by plotting nest thermal parameters measured during the TSP of sex determination. Figure 5 illustrates this approach based on observational field data (which reports only secondary sex ratios), but the reaction norm surface could potentially be improved by using an experimental approach. A caveat, however, is that experiments designed to describe multi-dimensional TSD reaction norms will be complex and require a large number of treatments. Our laboratory experiment used only a fraction of the possible combinations of thermal means and diel fluctuations that occur in nature. Nevertheless, it is clear that multiple thermal parameters are needed to describe TSD reaction norms, and this approach will enhance our understanding of TSD under natural conditions. Although we advocate this multi-dimensional approach for understanding the complexities of TSD reaction norms, studies that use constant temperature incubation are still useful in identifying the presence of TSD. Importantly, however, studies that use constant temperature incubation should at least acknowledge that shapes of reaction norms might be quite different under natural thermal environments, rather than categorizing TSD patterns as either type I or II.

Figure 5.

Three-dimensional reaction norm describing the relationship between egg incubation thermal regimes and offspring sex ratios of jacky dragons (Amphibolurus muricatus). Data points represent single nests in the field and thermal parameters were calculated only from temperature data during the first 60% of incubation (i.e. during the thermo-sensitive period for sex determination; [18]). The plane (i.e. reaction norm) represents a parabaloid equation. The fluctuating thermal treatments from the laboratory experiment are represented by the diamond symbols (with drop lines). Note that the sex ratio produced under the 25 ± 8°C fluctuating treatment was substantially less female-biased than that predicted from the field data. This inconsistency may be explained by the relatively high egg mortality in nests with relatively high levels of thermal fluctuation [16]. Because of egg mortality in natural nests, field-based data are based on secondary sex ratios and laboratory data are based on primary sex ratios.

Why do thermal means and diel variation interactively affect sex ratios? Theoretical and empirical studies indicate that daily fluctuations can slow (or inhibit) development during times when high or low temperatures are reached, thereby resulting in a nonlinear relationship between temperature and developmental rate at thermal extremes [9]. This nonlinear relationship probably explains the interactive effects of thermal mean and thermal fluctuation on incubation duration, and consequently, will impact the proportion of development spent at male- versus female-producing temperatures ([27]; see figure 1). As expected from past studies of jacky dragon TSD [15], constant incubation at 25°C produced female-biased sex ratios, and a constant 28°C produced nearly equal numbers of males and females. Under a mean of 25°C, increasingly greater thermal fluctuations increase the amount of development within a male-producing range of temperatures, as well as the time spent near the developmental zero (the temperature at which development is arrested, about 17.2°C in A. muricatus; see the electronic supplementary material, figure S1). Thus, excessively low temperatures (close to the lower female-producing range) have little effect on sex determination because development is essentially arrested. As a result, we would expect sex ratios to shift away from a female bias with increased fluctuations. Intriguingly, by shifting the mean temperature up to 28°C, the same levels of thermal fluctuations increasingly expose embryos to female-producing temperatures (near both thermal extremes). Hence, we see the opposite shift in sex ratios, towards a female bias at a mean of 28°C.

The patterns described above are broadly consistent with predictions of the CTE modelling approach [8]. The CTE approach has been critical in evaluating the influence of natural nest incubation regimes on sex ratios in TSD species [26,27], but there are some limitations. For example, CTE models were developed to predict sex ratios only for species with a single pivotal temperature (unlike A. muricatus), and even then are not always successful at predicting sex ratios (e.g. [30]). In our study, CTE was not related to sex ratios from field nests (see the electronic supplementary material, figure S2) and CTEs calculated for our experimental treatments produced sex ratios that did not closely correspond with those from constant temperature incubation (table S2). Although ours, to our knowledge, is the first study to apply the CTE approach to a species with multiple pivotal temperatures, our results reinforce the notion that current CTE models are not applicable to these complex TSD systems [8]. In cases where CTE models cannot be applied, multi-dimensional reaction norms will better describe TSD patterns and facilitate predictions of sex ratios from natural nests.

Although we argue that multi-dimensional reaction norms will be useful, we also note several caveats. First, complex thermal variation is not the only variable that may complicate comparisons between field data and those obtained from controlled experiments. For example, nest moisture conditions may interact with temperature to influence developmental rate in some reptiles [31], which in turn could influence sex ratios from natural nests. Second, thermal gradients within nests should be considered. For example, eggs near the soil surface may experience warmer temperatures and greater diel thermal fluctuations than those near the bottom of the nest [32]. Hence, temperatures recorded at the core of the nest may not accurately represent the range of thermal conditions experienced by each egg, thereby reducing the efficacy of sex ratio predictions. This issue is of minor concern in the current study, because of the small size of A. muricatus nests; the thermal conditions experienced by top eggs probably differed very little from those of bottom eggs (the average distance from the top egg to bottom of the nest was only 1.7 cm; range = 0.2–4.4 cm). Moreover, neither mean nor fluctuation in nest temperature was related to the depth of the top egg or the bottom of the nest. A third caveat is that the multi-dimensional approach accounts only for thermal means and variances, and does not incorporate the shape of the daily thermal cycle in a nest; this would add another dimension to the reaction norm surface that could be accounted for in a more complex equation.

(b). A mechanism for reducing sex ratio biases under TSD

Our results highlight how multiple thermal parameters can interactively affect sex ratios under TSD. These experimental results coupled with nest thermal patterns in the field identify a plausible mechanism for how TSD can reduce skews in offspring sex ratios. For example, the seasonal increase in mean nest temperatures with the concomitant decrease in diel fluctuations (figure 2), would prevent seasonal shifts in sex ratios owing to the interactive effect of these two critical parameters. Hence, offspring sex ratios would remain close to 1 : 1 throughout the season despite TSD and seasonal shifts in nest thermal environments. Additionally, previous work has highlighted the importance of maternal effects in generating variation in primary sex ratios [33], which reinforces the notion that TSD systems may yield stable 1 : 1 primary sex ratios even when the average nest thermal environment deviates from the pivotal temperature. Overall, although our study organism exhibits TSD, the complex interactions among thermal parameters (and maternal effects; [20]) can reduce sex-ratio biases, and hence produce sex ratios similar to those expected under GSD. Indeed, sex ratios from our nests in the field varied widely, but did not shift seasonally despite seasonal changes in thermal parameters.

Previous studies of the adaptive significance of TSD in short-lived organisms (such as A. muricatus) posit that this sex-determining mechanism enables each sex to hatch at their own optimal time of the season [34,35]. Despite evidence for sex-specific optima for the timing of hatching in A. muricatus [35], our current findings challenge this hypothesis because interactions among thermal parameters will constrain an adaptive shift in sex ratio across the breeding season. Importantly, we do not claim that sex differences in the optimal timing of hatching were not important for the evolution of TSD in short-lived species, but instead our results only suggest that this hypothesis may not explain the current maintenance of TSD.

(c). Implications for tests of adaptive significance

The most compelling hypothesis for the adaptive significance of TSD states that parental fitness is enhanced because TSD enables sons and daughters to develop under the thermal environment that is optimal for them (i.e. Charnov–Bull [36] model). Our recent work has provided strong support for this model [37], but many critical issues remain unanswered. For example, because multiple thermal parameters can affect fitness-relevant phenotypes and sex ratios [8,10,11,13], experiments need to evaluate how these complex interactions affect sex-specific fitness. Experiments designed to address this issue will provide novel insights into the ecological relevance of TSD and enhance our understanding of selection on TSD reaction norms.

Our experimental design enabled us to address this issue, but our results did not support theoretical predictions [36]. Although we found a significant three-way interactive effect (thermal mean × thermal fluctuation × sex) on locomotor performance, the patterns were not consistent with the Charnov–Bull model. For example, performance was significantly enhanced in males produced at a constant 28°C, which is a thermal regime unlikely to occur in nature. Additionally, female performance was enhanced only when produced by a thermal regime that yields 1 : 1 sex ratios, rather than a female-biased sex ratio. Although other fitness-relevant traits (e.g. body size) were affected by thermal fluctuations, this effect did not differ between male and female offspring; similar effects have been observed in other reptiles [12,38]. Thus, our findings are inconclusive in this respect, and thermal regimes within nests may influence sex-specific fitness through many other pathways [39–41]. Moreover, to fully understand how different developmental thermal regimes impact sex-specific fitness, studies need to move beyond measurements of performance and evaluate long-term survival and reproductive success (e.g. [37]). Future studies that evaluate interactive effects of nest thermal parameters on reproductive fitness will bring experimental tests of the Charnov–Bull model to a new level, and will provide novels insights into the evolution of ESD.

(d). Conclusions

The importance of thermal fluctuations during development has long been recognized in studies of TSD, but this complexity is rarely considered in reaction norms that describe TSD patterns. Future experiments that incorporate multiple thermal parameters will undoubtedly reveal that the patterns of TSD are more diverse and complex than embodied in the traditionally used two-dimensional reaction norms. Our results also indicate that interactions among thermal means and variances will probably reduce sex ratio skews and yield patterns similar to that expected under GSD. Recognizing this complexity, and incorporating it into experimental tests of adaptive significance, will enhance our understanding of the ecology and evolution of ESD.

Fluctuations in thermal conditions have critical impacts on many facets of organismal biology [42]. How organisms have adapted to such conditions has been the focus of many empirical and theoretical investigations [43]. More recently, how sex ratios in organisms with TSD might have dealt with past climate change or will respond to predicted shifts in thermal environments (owing to climate change) has become an increasingly common issue in the literature [5,44–46]. Current predictions indicate that global and regional temperatures will not only shift directionally, but will also fluctuate more dramatically owing to human-induced climate change [47,48]. Our results suggest that future shifts in both of these thermal parameters will have important impacts on sex ratios under TSD. Given that complex interactions among multiple thermal parameters may result in several conditions that yield 1 : 1 sex ratios under TSD, the outlook for some of these species may be more promising than previously expected [44–46]. To better understand these effects, models that predict offspring sex ratios under future climate change scenarios must incorporate thermal fluctuations, as well as sex-determining reaction norms involving multiple thermal parameters.