Invariant scaling relationships for interspecific plant biomass production rates and body size

Abstract

The allometric relationships for plant annualized biomass production (“growth”) rates, different measures of body size (dry weight and length), and photosynthetic biomass (or pigment concentration) per plant (or cell) are reported for multicellular and unicellular plants representing three algal phyla; aquatic ferns; aquatic and terrestrial herbaceous dicots; and arborescent monocots, dicots, and conifers. Annualized rates of growth G scale as the 3/4-power of body mass M over 20 orders of magnitude of M (i.e., G ∝ M^3/4); plant body length L (i.e., cell length or plant height) scales, on average, as the 1/4-power of M over 22 orders of magnitude of M (i.e., L ∝ M^1/4); and photosynthetic biomass M_p scales as the 3/4-power of nonphotosynthetic biomass M_n (i.e., M_p ∝ M_n^3/4). Because these scaling relationships are indifferent to phylogenetic affiliation and habitat, they have far-reaching ecological and evolutionary implications (e.g., net primary productivity is predicted to be largely insensitive to community species composition or geological age).

It has long been noted that metabolic rates B scale nonlinearly with respect to adult body mass M (1) and that this allometric relationship is best described by a 3/4 scaling exponent (i.e., B ∝ M^3/4) (2, 3). Broad interspecific comparisons for mature unicellular and multicellular animals by numerous researchers have experimentally verified this exponent (4–11). Many other physiological, anatomical, and life-history characteristics also scale as “quarter-power” exponents of animal body mass or length, e.g., annualized rates of biomass production G scale to the 3/4-power (9–11). Nevertheless, the origin of the 3/4 and other quarter-power exponents has long been the subject of considerable theoretical speculation (7–17).

Allometric relationships have played a central role in the development of zoology. Unfortunately, with few exceptions, botanical studies have not traditionally considered the role of body size (11–13), and thus the extent to which the scaling relationships observed for animals hold true for photoautotrophs is unclear. Recently, however, there has been both empirical and theoretical justification to expect the scaling relationships observed for animals to apply equally well for plants (12–18). Theoretical models based on the evolution of fractal-like networks predict that many attributes of organic structure and function should scale with quarter-powers of body mass (14, 17, 18). These models specifically predict numerous allometric scaling relationships, including that B ∝ M^3/4, that L ∝ M^1/4, and that the total effective tissue exchange area (e.g., leaf or root surface area) with the environment will scale as M^3/4. Because rates of biomass production are intimately dictated by metabolic rates B, annualized rates of gross metabolic or biomass production G are expected to be directly proportional to B (G ∝ B) such that G ∝ M^3/4 (2–11, 17, 18), although this may not hold true over ontogenetic time scales.

This theoretical expectation has been verified to a limited extent based on interspecific comparisons among unicellular algal species that show rates of biomass production scale as the 3/4-power of cell mass over eight orders of magnitude of body size (19, 20). Likewise, rates of biomass production in tropical trees appear to scale as M^3/4 (13). However, 11 orders of magnitude in body size separate the largest known unicellular algae and the largest multicellular terrestrial plants. It is thus unclear whether a single or multiple scaling relationships best describe the relationship between G and M across all or most major plant clades and grades. Likewise, the relationship between plant body length and mass is not well established, although a scaling exponent statistically similar to 1/4 has been reported for a limited number of unicellular and multicellular plant species (21).

Here, we report the relationship between annualized biomass production rates and body size for representative species of unicellular algae, aquatic ferns, and a broad spectrum of dicot, monocot, and conifer species. Our analyses of these data, which span 20 orders of magnitude of body mass, unequivocally demonstrate that plant allometric relationships (among rates of biomass production, photosynthetic mass, and body length and mass) conform both to those previously described for animals and with the predictions made by recent allometric and biomechanical theoretical models (12–18).

Materials and Methods

Unicellular Algae.

All of the data used in our analyses were collected from the primary literature (refs. 21–31, compiled in ref. 12). Values for cell (body) mass M (pg of carbon per cell), length L (μm), biomass production (“growth”) rates G (pg of carbon per cell per h), and total cell pigment content (pg of carbon) C reported in the primary literature were converted to their corresponding units reported for metaphytes (i.e., kg of carbon per cell, m, kg of carbon per cell per year, and kg of carbon per cell, respectively; see below). In the majority of cases, the values published for G were based on monocultures grown under optimal or near optimal conditions (12, 19). Three phyla are represented by these data.

Aquatic Metaphytes.

Two fern and one dicot species are represented in this data set (Azolla caroliniana, Azollaceae; Salvina natans, Salviniaceae; and Lemna minor, Lemnaceae; respectively). Stock cultures of these species (from Carolina Biological Supply) were grown in shallow glass dishes (containing sterilized pond water) placed in a growth chamber at a temperature of 25° F (with a day/night photoperiod of 15/9 h and an average light intensity of 354 μmol photons/m²/s measured at the water-air interface). Growth in M and L were monitored every 48 h by culling ≈80% of each population and counting the number of culled plants, which were then air-dried and weighed. Body mass M was computed from the quotient of total kg of dry matter and culled plant number; annualized G was computed based on total kg of dry matter per day and the number of culled plants.

Terrestrial Metaphytes.

These species include herbaceous and tree-size monocots (grass and palm species), and herbaceous and arborescent dicots and conifers. With the exception of the data reported for Pearl Millet (Pennisetum glaucum, Poaceae) provided by Steven Mason (Department of Agronomy, University of Nebraska), the data are primarily those compiled by Cannell (32) based on the primary literature published up to mid-1981 for ≈1,200 forest stands from 46 countries worldwide. In most cases, these data are for even-age monospecific stands grown under horticulturally controlled field conditions (n = 600 of 880 data sets for which authors reported the data required to compute G and M) such that the variance in tree G and M is likely low and thus roughly comparable to that of the unicellular algae and aquatic metaphyte data (see above).

The Cannell compendium provides the number of plants per hectare (“plant density”), average plant height, total basal stem cross-sectional area, and biomass and net production (in units of metric tons of dry matter subsequently converted into units of kg of dry matter) of stem wood, bark, branches, fruits, foliage, and roots whenever reported by an author. Body mass M (kg of dry matter per plant) was computed from the quotient of total standing biomass and plant density per 1.0 ha of forest sample. Annualized biomass production rates (kg of dry matter per plant per year, accounting for annual losses of dry matter attributable to mortality, litter-fall, decay, or consumption) were computed as the quotient of total net production (of stem wood, bark, foliage, roots, and reproductive organs) and plant density. Values of foliage (photosynthetic) M_p and stem and root (nonphotosynthetic) M_n biomass (kg of dry matter per plant) were computed from the quotients of total biomass allocated to each body part and plant density. Body length L is plant height, and thus neglects root length (which was not reported in the Cannell data sets). Values for L were unavailable for Pearl Millet. Additional data for metaphyte M and L (taken from ref. 21) were pooled with the Cannell data in our analyses. These data include those from the General Sherman tree (Sequoia) and the Giant Pacific Kelp (Macrocystis).

Statistical Analyses.

All computations and analyses used the software package jmp Version 3 (SAS Institute, Cary, NC). Model Type II (reduced major axis, denoted as RMA) regression analysis was used to compute scaling exponents (slopes of curves designed as α_RMA) because the error variance resulting from measurement error and real biological variation was equivalent among all variables (12). The 95% confidence intervals of α_RMA were used to assess whether an empirically determined scaling exponent complied with that predicted by theory. The 95% confidence intervals of Y-intercepts were also used to determine whether data sets sharing the same α_RMA could be pooled for additional regression analyses (12).

Results

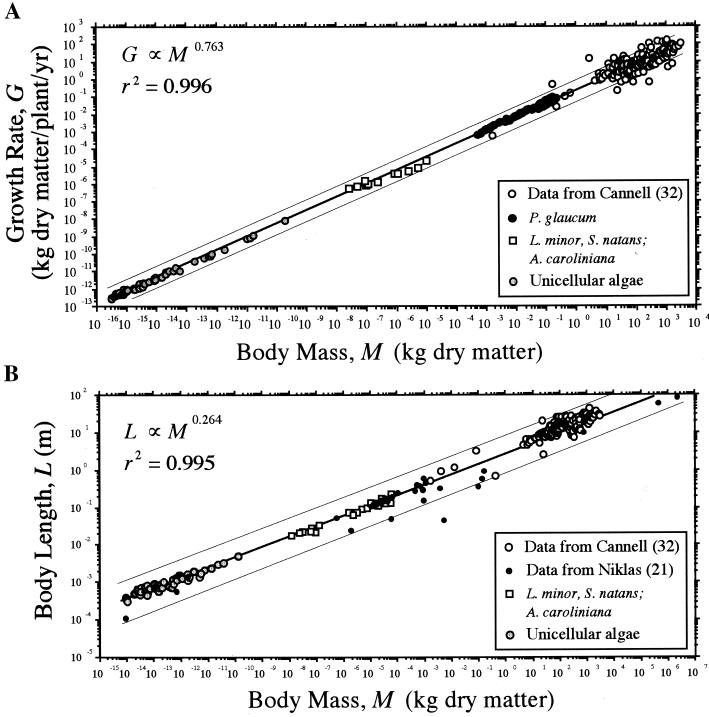
A 3/4 scaling exponent was statistically verified for the relationship between annualized rates of biomass production G and body mass M for the species representing individual higher taxa or evolutionary grades (e.g., dicots and unicellular algae, respectively; Fig. 1A). Specifically, among all metaphyte species, α_RMA = 0.749 ± 0.007 and Y-intercept = 0.214 ± 0.016 (n = 334, r² = 0.972, F = 11,695, P < 0.0001); across tree species, α_RMA = 0.791 ± 0.03 and Y-intercept = 0.301 ± 0.16 (n = 178, r² = 0.705, F = 421, P < 0.0001). Similarly, unicellular algae G scaled as the 3/4-power of body mass (α_RMA = 0.749 ± 0.008 and Y-intercept = 0.119 ± 0.107; n = 66, r² = 0.995, F = 9745, P < 0.0001). When the data were pooled and regressed for all plant species, a single allometric scaling (regression) formula with a 3/4 exponent described the relationship between G and M across 20 orders of magnitude of M (α_RMA = 0.763 ± 0.003 and Y-intercept = 0.208 ± 0.016; n = 387, r² = 0.996, F = 84933, P < 0.00001). However, one decade of body size was unoccupied by these data (i.e., 10⁻⁹ ≤ M ≤ 10⁻⁸ kg of dry matter).

Figure 1.

Annualized biomass production (growth) rates G (A) and body length L (B) of unicellular and multicellular plants plotted against body mass M. Solid lines are reduced major axis (Model Type II) regression curves (hair lines indicate 95% confidence intervals); scaling exponents (upper left) based on log₁₀-transformed data.

A 1/4 scaling exponent was statistically verified for the relationship between L (maximum cell linear dimension; metaphyte body length or height) and M across all species. Specifically, α_RMA = 0.264 ± 0.019 and Y-intercept = 2.58 ± 0.012 (n = 398, r² = 0.995,, F = 345.1, P < 0.0001; Fig. 1B). Likewise, algal cell length scaled as the 1/4-power of M (n = 66, r² = 0.879, α_RMA = 0.264 ± 0.025, Y-intercept = 2.16 ± 0.026, F = 489.3, P < 0.0001). However, for tree species, L scaled approximately as the 1/3-power of M (n = 271, r² = 0.663, α_RMA = 0.345 ± 0.012, Y-intercept = 3.71 ± 0.026, F = 529.5, P < 0.0001). This deviation from the 1/4 exponent was not attributable to neglecting root length; the scaling exponent for the relationship between plant (stem) height and above-ground body mass (= stem and leaf mass) was higher than that predicted by a 1/3 scaling relationship (i.e., α_RMA = 0.367 ± 0.009). Rather, we attribute the deviation in the exponent to the log–log nonlinear relationship between L and M for tree species (i.e., the slope for the regression of L against M is statistically ambiguous). This nonlinearity is likely attributable to variation in the ability of above-ground branching structures to efficiently occupy space (16).

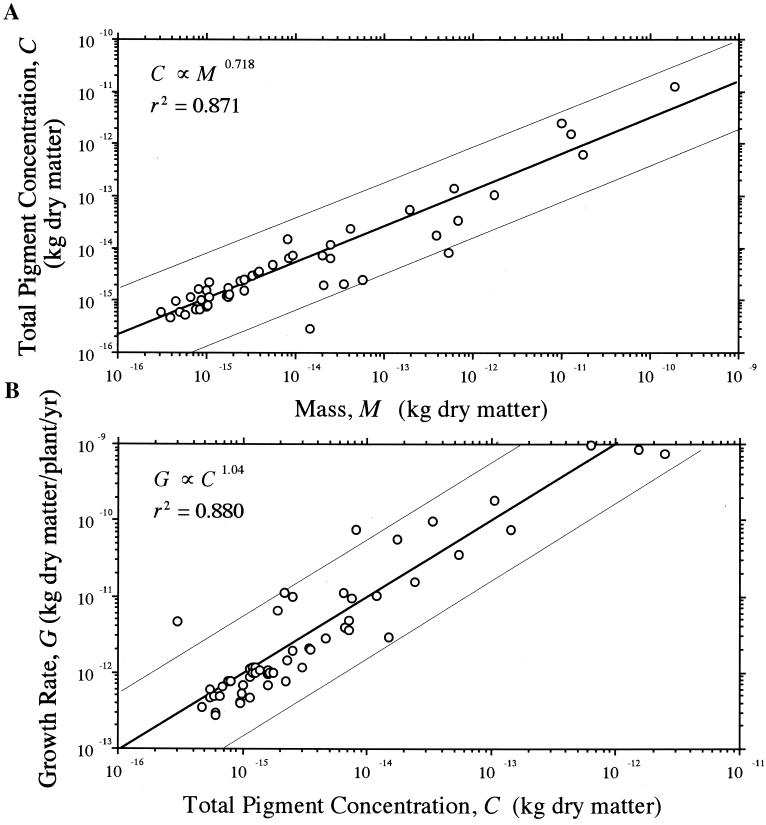
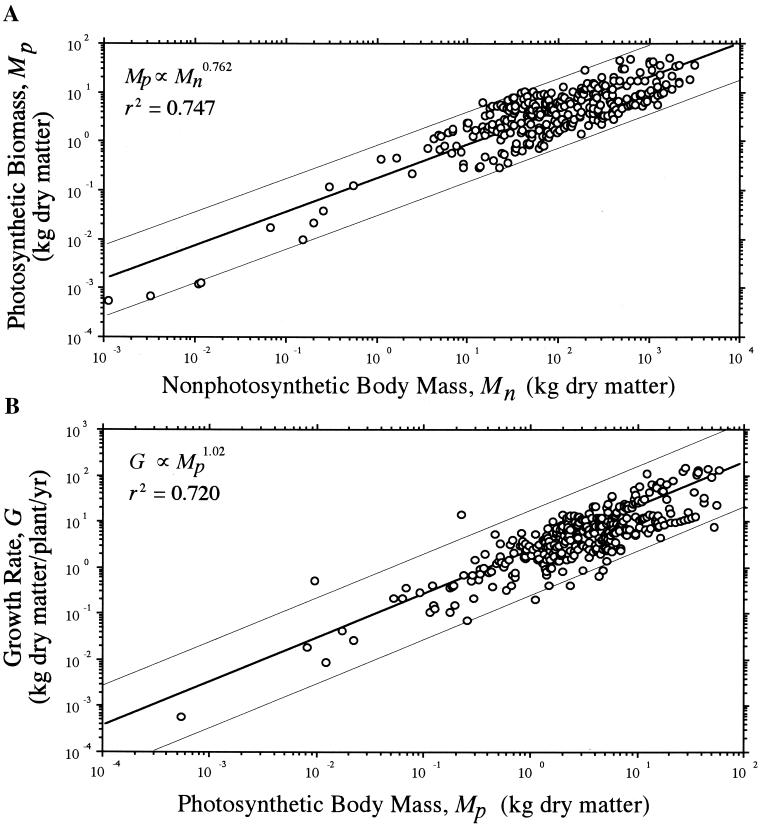
The “light-harvesting capability” of plants H (measured here as either total algal cell pigment content or total foliage biomass) scaled as the 3/4-power of M and as the 1.0-power of G. These scaling relationships are allometrically internally consistent; because H ∝ M^3/4 and G ∝ M^3/4, it follows that G ∝ H. Thus, because total cell pigment content C scaled as the 3/4-power of cell mass M (α_RMA = 0.718 ± 0.035 and Y-intercept = 1.12 × 10⁻⁵ ± 0.497; n = 56, r² = 0.871, F = 363.1, P < 0.0001; Fig. 2A), it follows that algal G will scale isometrically with respect to C (α_RMA = 1.04 ± 0.049 and Y-intercept = 438 ± 0.709; n = 56, r² = 0.880, F = 396.8, P < 0.0001; Fig. 2B). Likewise, total foliage biomass M_p scaled as the 3/4-power of total nonphotosynthetic biomass M_n (α_RMA = 0.762 ± 0.023 and Y-intercept = 0.192 ± 0.047; n = 292, r² = 0.747, F = 856.2, P < 0.0001; Fig. 3A). Thus, G scaled isometrically with respect to M_p (α_RMA = 1.02 ± 0.049 and Y-intercept = 1.81 ± 0.024; n = 337, r² = 0.720, F = 861.5, P < 0.0001; Fig. 3B). Noting that total metaphyte body mass M and M_p are autocorrelated, M_p nonetheless scaled approximately as the 3/4-power of M (α_RMA = 0.779 ± 0.022 and Y-intercept = 0.166 ± 0.047; n = 292, r² = 0.765, F = 856.2, P < 0.0001), such that the isometric relationship between G and M_p holds across species.

Figure 2.

Total cell pigment content C plotted against body mass M (A) and annualized biomass production (growth) rates G plotted against total cell pigment content for unicellular plants (B). Solid lines are reduced major axis (Model Type II) regression curves (hair lines indicate their 95% confidence intervals); scaling exponents (upper left) based on log₁₀-transformed data.

Figure 3.

Photosynthetic (foliage) biomass M_p plotted against nonphotosynthetic (stem and root) body mass M_n (A) and annualized biomass production (growth) rates G plotted against photosynthetic body mass M_p for metaphytes (data taken from ref. 32) (B). Solid lines are reduced major axis (Model Type II) regression curves (hair lines indicate their 95% confidence intervals); scaling exponents (upper left) based on log₁₀-transformed data.

We note in passing that, because G ∝ M_p ∝ M_n^3/4, short-term (ontogenetic) growth rates for woody species can vary as a function of shifts in the relative amounts of photosynthetic and nonphotosynthetic biomass. Juveniles will have higher growth rates in comparison to more mature conspecifics provided they produce disproportionately large foliage biomass. However, for tree species, analyses indicate that M_n/M_p scales nearly isometrically with respect to plant age and that, on average, G ∝ M^3/4 within as well as across species.

Discussion

Regardless of clade, grade, or habitat, annualized rates of plant biomass production, G, on average, scale with respect to body mass or length in the same manner previously described for unicellular and multicellular animals (i.e., Kleiber's 3/4-power “rule”). However, unlike animal clades, which have similar allometric exponents but different intercepts (i.e., normalization constants), all plants comply with a single allometric formula that spans 20 orders of magnitude in body mass. We attribute this invariance to the fact that, unlike heterotrophs, all photoautotrophs share the same basic physiological machinery and requirements. It is also evident that the relationship between plant G and M is intimately related to light-harvesting capacity H (pigment content per algal cell; foliage biomass per metaphyte), because H also scales as the 3/4-power of M such that G ∝ H. These scaling relationships reflect the ability of unicellular and multicellular plants and animals to use available energy for growth. Thus, it is likely that a common explanation for quarter-power allometric relationships rests on general principles applicable to all manner of living things.

One global explanation, advanced on theoretical grounds (13, 16–18), argues that all unicellular and multicellular organisms have either virtual or real fractal-like distribution networks for the internal transport of metabolites, thereby endowing them with a “fourth spatial dimension” (17). These networks are purported to maximize metabolic capacity and efficiency by maximizing the available exchange surface area for absorption of limiting resources from the environment but yet minimize transport distance and time (17). This “zeroth order” model predicts that scaling (allometric) exponents for G, M, and L (as well as other biological properties) must be either 1/4 or multiples of 1/4. Accordingly, the fractal body length, which accounts for the internal spatial dimension of an organism, is predicted to increase as the 1/4-power of body mass (L ∝ M^1/4), whereas growth (and metabolic) rates are predicted to scale as the 3/4-power of body mass (G ∝ B ∝ M^3/4), regardless of an organism's phyletic affiliation or habitat.

Our data are consistent with this model in virtually all respects. In addition to showing that G ∝ M^3/4 and that L ∝ M^1/4 hold true across 20 decades of plant body mass, we demonstrate that the scaling relationship between photosynthetic to nonphotosynthetic biomass for metaphytes and the relationship between total cell pigment content and cell size for unicellular algae abide by a 3/4-power “rule” such that G ∝ H. We interpret this isometric relationship to indicate that an approximate constant fraction of short-term biomass production is allocated to the construction of photosynthetic and nonphotosynthetic body parts. Such invariance suggests that, over the evolutionary diversification of plant clades, there has been no major change in the basic physiological basis for biomass production and in the allocation pattern of biomass to energy-harvesting relative to the biomass supporting other body functions. This proportionality helps to explain phenomenologically why plant annualized growth rates scale in the same manner regardless of clade or grade. More significantly, it is precisely what is expected if natural selection maximized metabolic capacity by maximizing effective surface area while simultaneously minimizing metabolite transport distance and time across or within cells (14–17).

The implications of this theory, which are supported here for plants, are ecologically and evolutionarily far reaching. For example, allometric scaling critically influences many attributes of ecological organization. In particular, extension of allometric theory predicts that the number of individuals N within any large sample of a plant community will be proportional to the 3/4-power of body mass (i.e., N ∝ M^−3/4), whereas annualized production rates will be proportional to the 3/4-power of body mass (i.e., G ∝ M^3/4) (33). Assuming that G is a reasonable surrogate measure of the rate of resource use per individual Q, we note that the rate of total (community) metabolic production Q_Tot (which is proportional to the rate of community biomass production G_Tot) is the product of the number of individuals N and Q. Thus, total (community) resource use R_Tot is predicted to be proportional to N_TotQ and, because Q ∝ G ∝ M^3/4, it follows that R_Tot ∝ G_Tot ∝ QN ∝ M^3/4 M^−3/4 ∝ M⁰ (33). This relationship implies that, within communities, the intrinsic capacities for annualized rates of resource use (as well as biomass production) will be invariant with respect plant size or species composition.

Allometric theory in tandem with the data presented here generates similarly important hypotheses when juxtaposed with biomechanical theory. For example, engineering first principles indicate that the critical load that can be supported by a vertical columnar support member P_cr is proportional to the quotient of flexural rigidity EI and the square of column L (i.e., P_cr ∝ EI/L²) (34). This proportionality indicates that stem biomass will scale as the 8/3-power of stem diameter (M_s ∝ D^8/3) and that additional lateral loads, such as photosynthetic (foliage) biomass, will scale as the 2-power of stem diameter (M_p ∝ D²) (34). Noting that our data for metaphytes show that photosynthetic (foliage) biomass scales as the 3/4-power of stem biomass (M_p ∝ M_s^3/4) and that annualized metaphyte growth rates scale as the 3/4-power of foliage biomass (G ∝ M_p^3/4), it follows that annualized growth rates are predicted to scale as the 3/2-power of stem diameter (G ∝ D^3/2). If this hypothesis is true, then size-frequency distributions of stem area could be used as surrogate measures for the annualized growth rates of plant communities (33).

These and other arguably provocative allometric hypotheses must be tested based on additional broad interspecific and intercommunity comparisons. However, all of the available data for unicellular and multicellular plants demonstrate scaling relationships that thus far comply in every respect with allometric and biomechanical theory (33, 34). Because present-day plants and animals appear to abide by the same or very similar scaling “rules,” there is good reason to expect these rules extend into deep geological (evolutionary) time. As such, allometric and biomechanical theory provides a potentially powerful tool for predicting many important properties for past as well as present-day organisms and the communities in which they live.

Abbreviations

G

annualized biomass production (“growth”) rate

M

body mass

L

body length

C

total (algal) cell pigment content

M_p

photosynthetic (foliage) biomass

M_n

nonphotosynthetic (stem and root) biomass

α_RMA

scaling exponent (slope) of reduced major axis regression curve