Patterns and properties of polarized light in air and water

Abstract

Natural sources of light are at best weakly polarized, but polarization of light is common in natural scenes in the atmosphere, on the surface of the Earth, and underwater. We review the current state of knowledge concerning how polarization and polarization patterns are formed in nature, emphasizing linearly polarized light. Scattering of sunlight or moonlight in the sky often forms a strongly polarized, stable and predictable pattern used by many animals for orientation and navigation throughout the day, at twilight, and on moonlit nights. By contrast, polarization of light in water, while visible in most directions of view, is generally much weaker. In air, the surfaces of natural objects often reflect partially polarized light, but such reflections are rarer underwater, and multiple-path scattering degrades such polarization within metres. Because polarization in both air and water is produced by scattering, visibility through such media can be enhanced using straightforward polarization-based methods of image recovery, and some living visual systems may use similar methods to improve vision in haze or underwater. Although circularly polarized light is rare in nature, it is produced by the surfaces of some animals, where it may be used in specialized systems of communication.

1. Introduction

Light arriving on Earth from natural sources, either directly from the Sun or reflected from the Moon, is unpolarized or, at most, weakly polarized. Nevertheless, there is an abundance of polarized light in natural scenes, whether in the air, on the surface of the Earth, or underwater. This seemingly paradoxical situation arises because polarized light is readily produced by the interactions of unpolarized light with materials, particles and surfaces. Here, we consider some features of light's polarization in nature, focusing on its formation and distribution as well as some of its properties useful to animals and to humans.

In a depolarized non-diverging beam of light, photons travel in parallel but have randomized axes of electric field vectors (or e-vectors). From such a beam, fully or partially linearly polarized light can be produced by differential absorption, differential reflection or differential scattering of subsets of these photons (figure 1). Thus, when light impinges on a dielectric surface that can reflect specularly, the reflected beam is commonly partially linearly polarized (in other words, it contains a non-random mix of e-vectors, producing an average e-vector orientation in a single plane—therefore, linearly polarized light is also called plane-polarized light). Also, if this surface is transparent and permits refraction, the refracted beam entering the surface is itself polarized. Similarly, partially polarized light results from scattering from dispersed or suspended particles. In nature, reflection from dielectric surfaces such as calm water, wet features, leaves or other shiny non-metallic objects is often polarized. These reflections produce patterns of polarized light that are specific to a given scene, depending on the types and orientations of surfaces in the scene. The patterns can change rapidly as these surfaces move and change in orientation, and can vary as the source of light (e.g. Sun or Moon) changes position. In contrast, scattering in bulk media, such as the atmosphere or underwater, produces a stable polarization field that varies only slowly with time and is largely predictable, depending on the types of scattering particles and on the orientation of the illuminant. Thus, in nature, both the Sun and the Moon can launch predictable polarization fields that are useful for orientation or for enhancing object visibility. In this review, we will discuss such patterns, considering primarily patterns of linearly (or plane) polarized light. However, circularly polarized light, while rare in nature, is sometimes present and will be discussed briefly as well.

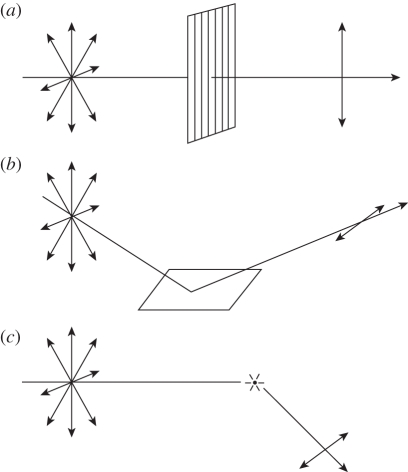
Figure 1.

The most common ways in which polarized light is generated in nature. (a) Polarization by transmission. Materials that preferentially transmit (or absorb) one plane of polarization will convert depolarized light to linearly polarized light. This is relatively uncommon in natural scenes, but some animals display signals produced by dichroic biological materials. (b) Polarization generation by reflection. At smooth, dielectric surfaces, reflection produces plane-polarized light. At a particular angle, known as Brewster's angle (which depends on the refractive indices of the two materials at the interface), the reflected light is fully polarized parallel to the surface. Polarization by reflection in nature occurs at water surfaces, at the surfaces of wet objects, or from shiny dielectric surfaces such as leaves or insect cuticle. (c) Production of polarized light by scattering. The plane of polarization of scattered light is perpendicular to the plane containing the incident and the scattered ray. When the angle of scattering is orthogonal to the axis of the ray being scattered, fully polarized light is generated.

2. Polarized-light patterns in the sky

It has been known that polarized light exists in the sky almost since the beginnings of the study of light's polarization [1–3]. In fact, as noted by Horváth et al. [4] Viking mariners may have used the polarization of skylight for navigation even earlier than this. The polarization is produced by Rayleigh scattering, resulting in a band of maximum polarization at angles near 90° to the Sun (see figure 1c for a schematic of polarization produced by scattering). At sunrise or sunset, this band is particularly prominent, as it passes directly overhead from north to south (figure 2). In a very clear sky, the degree of linear polarization in this band can reach at least 90 per cent, but it typically is quite a bit lower [1]. Looking away from the 90° arc of maximum polarization, the degree of polarization decreases smoothly, reaching 0 per cent near the Sun or the position of the anti-Sun. Interestingly, several polarization singularities also exist in the sky, near the Sun and anti-Sun, that result from multiple-path scattering (see [5] for a discussion), but these are unlikely to play any role in biological processes. The overall polarization pattern, however, is used by many species of animals for orientation and navigation (see [6] and [7] for reviews, as well as many papers in this Special Issue).

Figure 2.

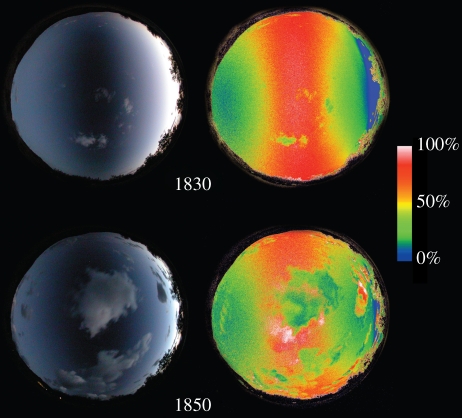
Polarization visible in the sky at sunset. These images were obtained using a calibrated digital camera fitted with a 180° ‘fisheye’ lens, aimed at the zenith, and with a linear polarizer placed behind the lens. In each image, north is to the top and east is to the left. Two examples are illustrated, with the raw image captured with the polarizer oriented east/west shown on the left. Since the polarization band passing through the zenith is polarized perpendicular to the Sun's position below the horizon in the west, the dark band shows polarization with the e-vector oriented north/south. Analysed images for the degree of polarization are illustrated on the right (the key for the analysed, false-colour image is provided on the far right). Actual sunset was at 18.14 h at this location on this day (15 September 2004; Lizard Island, Queensland, Australia). Note that the polarization pattern remained stable until well after sunset, and that the presence of clouds locally depolarizes the skylight.

Skylight polarization produced by moonlight has been much less studied than that originated by the Sun, but its distribution and properties generally mimic those of the daytime sky, being different primarily in intensity [8]. Some nocturnal animals, in fact, are known to orient to polarization produced by the Moon ([9]; see also [10]). Because moonlight competes with other nocturnal sources of light in the sky, even in nature, the degree of polarization is expected to be less than in a similarly clear daytime sky, especially when the Moon is not full. Also, artificial light sources will severely corrupt the natural nocturnal pattern of celestial polarization, but these have essentially no effect on the pattern during the day. Celestial polarization survives poorly in hazy or cloudy conditions, as the presence of suspended particles or water droplets rapidly depolarizes light by multiple-path scattering. Nevertheless, if the sky is not entirely covered in cloud, the patches of open atmosphere that are visible from the Earth's surface retain the polarization characteristic of their relationship to the Sun [11]. Thus, animals that rely on celestial polarization can continue their activities as long as the sky is not completely occluded by haze or clouds (figure 2).

Since the upper atmosphere continues to be illuminated by sunlight even when the Sun itself is not visible below the horizon, skylight polarization is still present and may be used by animals for orientation during the period of twilight [12,13]. Patterns of skylight polarization throughout the entire twilight period have been examined using whole-sky imaging polarimetry and polarization spectrometry [14], demonstrating that the simple overhead pattern of skylight polarization exists for nearly an hour before sunrise or after sunset (figure 3). Thus, when the sky is clear or even moderately cloudy, skylight polarization from the Sun is visible and potentially usable by animals throughout most of the day, and exists nearly around the clock at times when the Moon is visible and bright at night.

Figure 3.

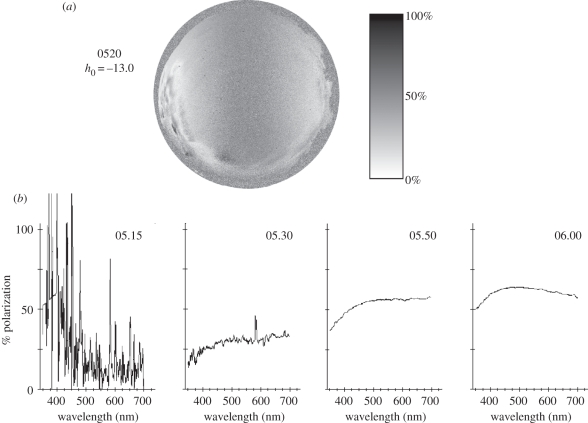
Skylight polarization during morning twilight. These data were collected at the same location as those of figure 2. (a) The polarization pattern at 5.20 h, when the Sun was 13° below the horizon (h₀). The key for the degree of polarization is at the right, with the analysis carried out as in figure 2. Predicted sunrise was at 06.10 h on this day, so the characteristic skylight polarization pattern is visible here almost 1 h before actual sunrise. (b) The spectrum of polarization measured at the zenith using a calibrated Ocean Optics QE65 000 spectrometer fitted with a 15° radiance-collecting head, 16 September 2007. Predicted sunrise was at 06.03 h, and measurements began at 04.15 h. Note that the characteristic skylight polarization spectrum was not measurable at 05.15 h, but that it is fully developed (though less than at sunrise) by 05.30 h. Polarization in the sky decreases at short wavelengths owing to multiple-path scattering.

3. Polarized-light patterns in water

How polarized light is distributed throughout the overall underwater light field is highly variable, even at a single geographical location at a single time of day. Unlike the situation in air, where the celestial polarization field is reasonably constant with small changes in height or altitude, underwater, the polarization fields at depths separated by only a few metres or tens of metres can be extremely different. In part, this is a consequence of vertical variations in the amount and the quality of suspended material. A much more significant effect, however, is produced at shallow depths by variations in the relative contributions of the aerial polarization field, transmitted into water but refracted at the water's surface, and the internal submarine light field produced by scattering within the water itself. The refractive index change between air and water restricts the view of the sky to a conical viewport (called Snell's window in deference to Snell's law of refraction) about 96° in diameter, within which the aerial polarization field may be visualized [7]. Outside Snell's window, polarized light is produced by scattering of downwelling sunlight. Near the surface, especially when the water is calm and clear, and there are few waves or ripples, polarization in the sky is readily observed (figure 4). As depth increases, multiple-path scattering destroys the remnants of the celestial pattern, and only in-water scattering, produced relatively near the observer, creates the polarization field.

Figure 4.

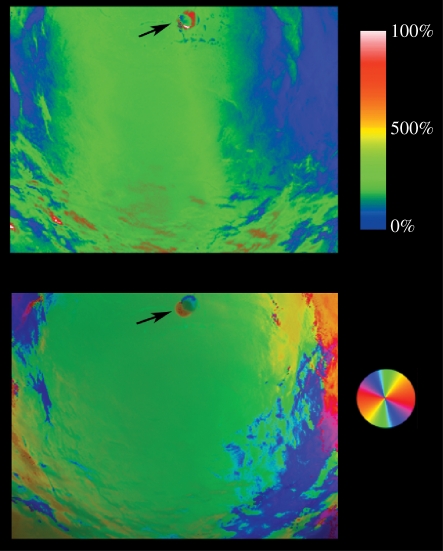
Polarization visible through the air–water interface at a depth of approximately 5 m in a coral-reef habitat at Horseshoe Reef, Lizard Island, Australia at sunset on 10 September 2003. Images were collected using a digital camera in a submersible housing fitted with a rotatable linear polarizer, and series of three sets of images were analysed for degree and angle of polarization. The camera was angled upward so as to image the zenith (indicated by a tethered float at the position of the arrow in each image); south is to the bottom of the image and west is to the right. The edges of Snell's window (where the image of the sky terminates) lie near the arc of rippled water near the bottom of each panel. The top panel shows the degree of polarization in false colour, while the bottom panel shows the angle of polarization (e-vector angle); colour keys are provided to the right. Note that the skylight pattern of polarization is visible underwater at this depth to the edge of Snell's window, although the degree of polarization is reduced somewhat from what would be visible in air (e.g. figure 2). Below Snell's window, polarization was oriented horizontally (not shown), but the band of overhead polarization is vertically polarized in the image, being oriented north/south. The small patches of random false colour near the margins of Snell's window are owing to differences in the three photographic frames used for the analysis (mostly caused by ripples at the surface); the float also drifted slightly between photographs and thus is multi-coloured in these images.

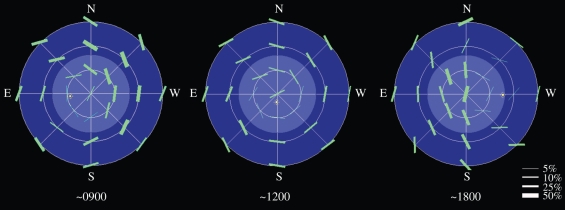
The first detailed examination of the overall pattern of polarized light in natural water was initiated by Waterman [15], who used a visual polarization analyzer (an axis-finder) to estimate the polarization distribution in shallow marine water (approx. 2–3 m depth) at different times of the day. At this relatively shallow location, the features of the aerial polarized-light distribution were obvious. The development of a submersible spectropolarimeter permitted Cronin & Shashar [16] to continue from this pioneering work, making spectral measurements of polarization at 25 points throughout the upper hemisphere at a depth of 15 m in marine waters. This second set of measurements differed from Waterman's [15] set in that the greater distance between the instrument and the water's surface was sufficient to obliterate the aerial pattern (figure 5). Shashar et al. [17] showed that even in clear natural water, polarization is reduced by 50 per cent owing to multiple-path scattering as light passes through each 2–4 m of water, so at 15 m depth there would be little to no trace remaining of celestial polarization. Instead, scattering of incident sunlight, arriving at its refracted angle after transiting the water's surface, is entirely responsible for the observed polarization at this depth. Consequently, the distribution of polarization is centred on the refracted position of the Sun, which is easily observed in the panels of figure 5 as the Sun moves from east to west in the refracted sky.

Figure 5.

Polarization underwater at a wavelength of 500 nm throughout the day. Data were collected at the Aquarius underwater laboratory in Key Largo, FL, USA on 15 and 16 August 1999 (see also [16]). Times given are Eastern Standard Time. Each part of the figure shows the polarization pattern looking upwards (this is why east and west are reversed from their positions on a compass card). The margin of each panel represents horizontal, and the concentric circles show elevations at 30° intervals. The lighter inner circle indicates the region within Snell's window, where skylight is visible through the sea's surface. At each location of measurement, the e-vector angle is plotted as the angle between a tangent to the elevation circle, and the degree of polarization is coded by the thickness of the plotted line (see the key at the lower right). The estimated position of the Sun at the midpoint of each series of measurements is indicated by the yellow symbol within Snell's window.

While the Sun's position in the sky continues to strongly influence the underwater polarization pattern at moderate depths, sunlight is increasingly diffused at greater depths owing to continuous scattering. In addition, absorption of sunlight oriented away from the zenith, owing to the large paths through water at depth, gradually creates a stable, or asymptotic, light field centred on the zenith that varies little if at all in its radiance distribution throughout the day [18,19]. Thus, throughout most of the ocean's depths where sunlight is able to penetrate (to approx. 1000 m in the clearest water), the polarization pattern is stable, with the e-vector oriented within a few degrees of horizontal (see [20]). Even near the surface, refraction confines the apparent position of the Sun to the bounds of Snell's window, limiting angular variation in e-vector orientation (figure 5).

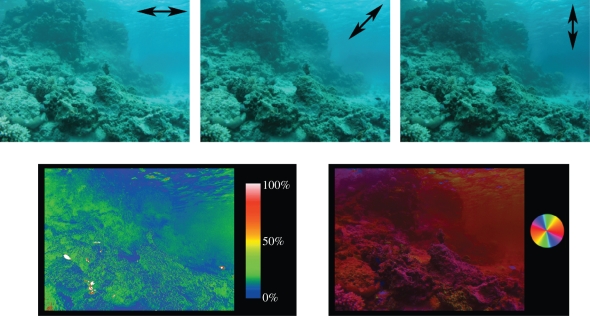
Because underwater polarization originates locally by scattering, the observed degree of polarization in a scene varies strongly with the brightness of the background. Reflection of light by background objects is rarely strongly polarized (as it can be in air) because of the relatively greater refractive index of water, but even if it were polarized, the rapid attenuation of polarization state with distance removes the original signal at viewing distances of only a few metres. Thus, polarization tends to be relatively stronger against dark parts of an underwater scene (or from the water background) than bright regions, because most of the light reaching the viewer from these regions has been scattered by water itself. This is obvious in the example illustrated in figure 6, showing the appearance of an underwater reef scene photographed through horizontal, 45°, and vertical linear polarizers. From these images, the degree and angle of linear polarization can be computed and displayed for each pixel (figure 6, bottom panels). Note that in this scene, which is typical, polarization is close to horizontal throughout much of the field of view, although there are variations related to surface orientation of nearby objects (most of the blue and green spots are artefacts caused by wave ripple or fish motion between frames). Note that the degree of polarization correlates well with the background darkness and also that the open water region, on the right margin of the image, is evenly polarized with a uniformly horizontal axis.

Figure 6.

Underwater images of polarization, taken at a site on the Great Barrier Reef, Australia, near the Lizard Island Research Station using a calibrated digital camera in a submersible housing fitted with a rotating linear polarizer. The top set of three images shows the raw images captured with the polarizer rotated to three positions, transmitting the e-vector indicated by the double-headed arrow in the upper right corner of each image. Note that the image with the polarizer horizontal is distinctly hazier than the image taken with the polarizer oriented vertically. The bottom panels show the data analysed for degree of polarization and angle of polarization (e-vector angle), plotted in false colour with the appropriate colour key to the right of each image. Note that polarization is generally greater against darker regions of the background, as well as in the open water view on the right margin of the image. The angle of polarization is near horizontal throughout the image, with some small variations visible on the substrate owing to reflection (although the reflected polarization is very weak). Small patches of random colour in the analysed images are owing to motions of the water's surface or to movements of fishes swimming over the reef.

4. Polarization and visibility underwater

Close inspection of the images in the top row of figure 6 reveals subtle differences in contrast and clarity. In particular, since most underwater scatter creates polarization that is near horizontal in orientation, images taken using a horizontal polarizer are notably hazier than images using a vertically oriented filter. This simple means of image enhancement can extend visual range and object detection in water by at least 20 per cent and up to 80 per cent [21,22], a fact that has been discovered by some midwater predators that use polarization vision to detect prey [23,24]. The significant, if marginal, gains available simply by using polarization filters have encouraged more complex instrumental or computational approaches to enhancing underwater vision and image quality ([25–28]; see also [29]). Several techniques have taken advantage of the natural polarization properties of underwater light, following the observation that the polarization originates in water, and that submerged objects reflect relatively little polarized light, especially when viewed from some distance away.

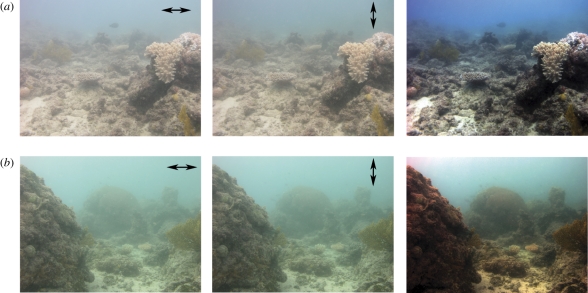
Schechner & Karpel [27] described a particularly effective approach that relies on subtle differences between image pairs acquired through polarizers oriented orthogonally. Their idea is based on the recognition that generation of veiling light in water is closely related to polarization, since both processes result from scattering of naturally incident light. Furthermore, loss of object visibility also results in part from scattering, as light originally reflected from objects becomes scattered away and mixed with haze produced between the object and the viewer. If the haze can be removed, and the original intensity and colour of the object restored by estimating its original reflectance, compensating for scattering and absorption of light by the intervening water, the scene can be restored to the view that would be available in a clear, non-scattering medium. Schechner & Karpel's [27] approach uses the relationship between polarization and the quantity of intervening haze, which they call ‘backscatter’. Lythgoe [30] termed this light, when it appears in front of viewed objects, ‘veiling light’. If the backscatter is polarized, but light coming from the object is not, it is possible to estimate the backscatter by comparing two polarization images. An additional benefit of this approach is that the distance to an object can be estimated by the quantity of backscatter between it and the observer. The quantity of backscatter at effective infinity, and the degree of polarization created by scattering, can both be estimated from portions of the image that include only the background water. The method is very sensitive to small variations in these estimates, which might arise from inhomogeneity in lighting or from different amounts of scattering along different visual axes (particularly away from the horizontal), but it can be extremely effective in both removing haze and restoring object radiance. If the correction is done independently for different colour channels in an image, the resulting image is largely colour-corrected as well. See examples in figure 7, where the Schechner & Karpel approach produces very satisfying colour and haze correction.

Figure 7.

Image enhancement via polarization processing using the approach of Schechner & Karpel [27] on two sets of underwater images. The original input images are illustrated at the left, taken as pairs with polarizing filters horizontal or vertical as shown by the double-headed arrows. None of the images, either original or analysed, has been altered in any way. Note that the input images differ only subtly, and neither has much contrast nor much colour saturation. Also, the colour tends towards hazy blue with increasing distance into the scene. After analysis for backscatter and degree of polarization at each pixel, the analysed image recaptures much of the inherent contrast in the scene and restores colour. Note how the objects deeper into the scene in particular recover their contrast and their inherent colour.

5. Circularly polarized light in nature

Thus far, the discussion has included only linear polarization (partial polarization). Light can also be circularly polarized; in fact, a complete description of light's polarization requires knowing intensity, orientation and degree of linear polarization and handedness and degree of circular polarization. Circular polarization refers to the phenomenon whereby the e-vector of light rotates in a plane, where typically the surface normal indicates the direction of propagation. It is typically produced by circular dichroism, whereby objects or molecules preferentially absorb one handedness of circular polarization (common in organic molecules) or by passing linearly polarized light through a retarder, delaying one axis of the linear polarization by 1/4 wavelength and resulting in an output e-vector that rotates. Particular, and uncommon, scattering or reflective events also produce circular polarization. At retardations other than 1/4 wavelength, the resulting beam has elliptical polarization, containing a mix of linear and circular polarization.

Circular polarization is relatively rare in nature, and almost never appears as a major component in natural scenes. It has been observed in light internally reflected from the underside of the sea's surface [18], where it arises from light originally linearly polarized by in-water scattering. Surprisingly, however, circular polarization is produced by several groups of animals. Larval fireflies emit circularly polarized light, by an unknown mechanism, from their bioluminescent lanterns [31]. Many species of scarab beetles reflect circularly polarized light, produced by a helical arrangement of chitin lamellae forming a chiral structure in their exoskeleton [32,33]. Stomatopod crustaceans of various species also reflect circularly polarized light, which they use for visual signalling [34]. Perhaps, the occasional use of circular polarization by animals is useful because it is rare in scenes, and thus prominent when used, but at this point research on biological aspects of circular polarization is still in its infancy.

6. Conclusions

Polarized light is abundant in natural scenes, and has the special attribute in such scenes of having been produced locally, almost always either by scattering or reflection. Thus, the patterns of polarized light visible to animals with polarization vision or to artificial instruments sensitive to light's polarization provide abundant information about objects in the scene, location of the Sun and time of day. Furthermore, natural polarization distributions can be used to enhance information indirectly, either simply by minimizing the contribution of scattered polarized light, or possibly more elaborately by processing polarization signals for visual enhancement. Technological approaches to polarization analysis are also attractive, for communication, object discrimination, object recognition and image enhancement.

In addition to the natural features of polarized-light distributions in scenes, animals themselves produce polarization signals (in some cases, circularly polarized ones), the study of which is still in its early phases. It is also possible that animals may manipulate polarized light in ways that decrease their visibility, creating ‘polarization camouflage’. While the importance of light's polarization for animals has been recognized for over half a century, recent research emphasizes how this property of light, of which humans are only dimly aware, plays many roles in biology. As is revealed by the papers throughout this special issue, our understanding of the roles that polarized light plays in the lives of animals continually evolves, and many questions concerning polarization vision, polarization signalling and the mechanisms by which living photoreceptors detect and analyse light's polarization remain unanswered—and sometimes, even unasked!.