Hyperfiltration Affects Accuracy of Creatinine eGFR Measurement

Summary

Background and objectives

Surrogate markers such as creatinine, cystatin C (CysC), and beta trace protein (BTP) have been used to estimate GFR (eGFR). The accuracy of eGFR may be altered with hyperfiltration and differences in filtration fraction (FF). It is hypothesized that the accuracy of creatinine for eGFR may be affected by hyperfiltration and different effective renal plasma flow (ERPF).

Design, setting, participants, & measurements

A total of 127 pediatric patients with various renal diseases underwent simultaneous measurements of GFR using ⁵¹Cr-EDTA renal scan and ERPF (¹³¹I-hippurate clearance) to calculate the FF (FF = GFR/ERPF). The eGFRs were calculated using the commonly used Schwartz (creatinine), Filler (CysC), and Benlamri (BTP) formulas. Agreement of the eGFRs with the measured isotope GFRs was assessed by Bland–Altman plots. Correlation analysis was performed using nonparametric tests to compare FF with eGFR − GFR.

Results

The 127 children at a median age (with 25th percentile, 75th percentile) of 11.9 (8.5, 14.9) years had a mean ⁵¹Cr EDTA-GFR of 100.6 ± 32.1 ml/min per 1.73 m² and a median ¹³¹I-hippurate clearance (ERPF) of 588 (398,739) ml/min per 1.73 m². Mean FF was 17.7 ± 4.5% with no correlation between the FF and the error (eGFR − GFR) for CysC and BTP eGFR, whereas there was a significant negative correlation between the error for Schwartz eGFR and FF.

Conclusions

There is a significant negative correlation between the error for the Schwartz eGFR and the FF. CysC and BTP are not affected by differences in FF.

Introduction

Renal function measurement is often focused on the GFR and, to a lesser extent, on renal blood flow (1). The gold standard for measuring GFR is inulin clearance (2). However, nuclear medicine studies have replaced inulin clearance because of convenience and absence of urine collection. In Europe, ⁵¹Cr-EDTA is the most widely used method for the determination of GFR (3), whereas in North America, the ⁹⁹Tc-diethylenetriamine pentaacetic acid renal scan enjoys the most widespread utilization (3–5). Although less demanding than inulin clearance studies, nuclear GFR studies are still cumbersome, invasive, and involve radiation. Endogenous markers for estimated GFR (eGFR), such as serum creatinine and more recently cystatin C (CysC), are hampered by diagnostic imprecision (6). Recently beta trace protein (BTP) has been introduced as a surrogate marker for GFR measurement (7).

Creatinine (molecular weight = 113 D, neutrally charged) is the metabolic product of creatine and phosphocreatine found in muscle; therefore, it reflects muscle mass (8,9). Given the large variability of muscle mass, there is substantial interpatient variability of serum creatinine concentration (10) because of its high water solubility (11).

Serum CysC has been shown to be an excellent marker for GFR (6,12,13). It is a small-molecular-weight protein (molecular weight = 13 kD, positive charge with isoelectric point of 9.3) that was initially known as γ-trace protein and its amino acid sequence was determined in 1981 (14,15). This protein is produced at a very constant rate and is affected by only a few conditions, such as uncontrolled hyperthyroidism (16).

BTP (molecular weight = 23 to 29 kD, mildly negatively charged with isoelectric point of 5.8 to 6.7, also known as prostaglandin D synthase) has been traditionally used as a marker of cerebrospinal fluid leakage (17,18). It is expressed in all tissues except the ovaries (19). Preliminary studies have confirmed a good correlation between serum BTP levels and GFR measurement by inulin clearance and nuclear medicine techniques (5,20).

Hyperfiltration is considered an abnormal increase in the GFR (21,22). However, this definition ignores the fact that hyperfiltration can take place in a single nephron even with globally decreased GFR. Other sources have defined hyperfiltration as the result of an increase in the glomerular capillary pressure (23,24). The filtration fraction (FF) is the ratio of GFR and effective renal plasma flow (ERPF) (24). A normal FF is 18.7 ± 3.2% in healthy young adults between the ages of 20 and 30 years (25). Hyperfiltration should be considered if the FF is above the reference interval.

We were interested in whether hyperfiltration affects the diagnostic accuracy of commonly used eGFR measurements using creatinine, CysC, and BTP in a pediatric population. The precision between the surrogate markers and the eGFR is reduced at higher GFR. One possible explanation for this phenomenon may be that some patients hyperfilter and others do not.

Patients and Methods

Patients

The study received full approval of the local ethics boards and was in accordance with the ethical standards of the Helsinki declaration of 1975 (revised in 1983) (5). Written consent was obtained in each case from the parents and, in the case of a consenting minor, from the patients as well. One hundred twenty-seven of the patients had a ⁵¹Cr-EDTA renal scan with concomitant determination of the ¹³¹I-hippurate clearance (ERPF), thus allowing for the calculation of the FF. Venous blood samples were obtained from 127 children with various renal pathologies, referred for determination of nuclear medicine GFR study. Patients were recruited consecutively and their ages ranged from 1.0 to 18.0 years with a mean of 11.5 ± 4.2 years. Thirty-five percent of patients were girls. Mean height was 136.7 ± 28.4 cm (range 62.3 to 189.1 cm), mean weight was 40.2 ± 20.0 kg (range 6.5 to 104.0 kg), and mean body surface area was 1.22 ± 0.42 m² (range 0.33 to 2.20 m²). The main indications for GFR measurements were various forms of GN (44.7%), obstructive uropathy (19.9%), reflux nephropathy (13.6%), postrenal transplantation (5.4%), and others (16.4%, including posthemolytic uremic syndrome, steroid-sensitive nephrotic syndrome, cystinosis, orthostatic proteinuria, etc.).

Materials and Methods

The methods for the simultaneous measurement of GFR and ERPF using ⁵¹Cr-EDTA renal scan with concomitant determination of the ¹³¹I-hippurate clearance have been described elsewhere (25). GFR and ERPF were corrected to a standard body surface area of ml/min per 1.73 m². For consistency, by GFR and ERPF we mean the corrected values per 1.73 m² of body surface area throughout the manuscript. FF was calculated as the ratio between ⁵¹Cr-EDTA GFR and ¹³¹I-hippurate ERPF and was expressed in percent. Serum creatinine was measured with an enzymatic assay (Ortho Clinical Diagnostics). Because enzymatic assays measure approximately 20% lower than the Jaffé method that was used in the original formula by George Schwartz (26), we used 20% higher constants (38 for children >1 year of age, 48 for adolescent boys) to calculate the GFR estimate according to Schwartz. We validated these revised constants for the Schwartz formula in our patient cohort. For adolescent boys, the estimated constant was 49.4 ± 10.5, not significantly different from 48 (P = 0.3271, one-sample t test). For the nonadolescent male patients, the constant was 40.3 ± 7.7, again not significantly different from 38. We therefore used the constants of 38 and 48. The formula reads

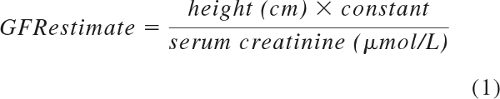The methods for the determination of CysC (Siemens Diagnostics GmbH) and BTP (Siemens Diagnostics) were described in the previous study (5). CysC eGFR was calculated using the Filler formula (27). For the BTP eGFR, we used a recently published and validated formula by Benlamri et al. (28).

We calculated the error between the measured GFR and the eGFR for creatinine using Schwartz, CysC, and BTP using (eGFR_Parameter − measured GFR)/measured GFR.

Statistical Analyses

All statistical analyses were performed using GraphPad Prism Software for Science Version 4.0c (San Diego, CA). Standard regression and correlation analyses were applied. Normal distribution was assessed using the Shapiro–Wilk test.

Agreement between methods was tested using the Bland–Altman plot method (29). The Bland–Altman plot is a statistical method used to compare two measurement techniques. In this graphical method, the differences (or alternatively the ratios) between the two techniques are plotted against the averages of the two techniques. Horizontal lines are drawn at the mean difference, and at the mean difference ± 1.96 times the SD of the differences. If the differences within the mean ± 1.96 SD are not clinically important, the two methods may be used interchangeably. SD of the differences between the two assay methods is used to calculate the limits of agreement, computed as the mean bias ± 1.96 times its SD. The bias is computed as the value determined by one method minus the value determined by the other method. If one method is sometimes higher, and sometimes the other method is higher, the average of the differences will be close to zero. If it is not close to zero, this indicates that the two assay methods are producing different results. Correlation analysis was performed using appropriate parametric (in case of normal distribution) or nonparametric tests (Spearman rank). In the case of non-normal distribution, data are given as a median (25th percentile, 75th percentile). The percentiles in brackets are also known as the interquartile range (IQR). P < 0.05 was considered statistically significant.

Results

The 127 children had a median age of 11.9 (IQR 8.5, 14.9) years, weighed 39.9 (28.8, 54.3) kg, and had a height of 146.0 (131.0, 163.8) cm. Mean body surface area was 1.30 ± 0.39 m². The mean measured ⁵¹Cr-EDTA GFR was 100.6 ± 32.1 ml/min per 1.73 m². The median ¹³¹I-hippurate clearance (ERPF) was 588 (398, 739) ml/min per 1.73 m². The mean FF was 17.7 ± 4.5%. Median serum creatinine was 56 (52, 74) μmol/L, whereas median CysC was 0.98 (0.83, 1.21) mg/L and median BTP was 0.76 (0.62, 0.98) mg/L. The results of the most important parameters are summarized in Table 1.

Table 1.

Statistics of the most important measured and calculated parameters

	51Cr-EDTA (ml/min per 1.73 m2)	123I-Hippurate (ml/min per 1.73 m2)	FF (%)	Creatinine (μmol/L)	Schwartz eGFR (ml/min per 1.73 m2)	CysC (mg/L)	CysC eGFR (ml/min per 1.73 m2)	BTP (mg/L)	BTP eGFR (ml/min per 1.73 m2)
Number	127	127	127	127	127	127	127	127	127
25th Percentile	77	398	14.6	41.55	84.5	0.83	73.97	0.62	81.67
Median	97	588	17.4	55.69	108.7	0.98	93.72	0.76	103.4
75th Percentile	121	739	20.3	74.26	134.6	1.21	113	0.98	126
Mean	100.6	614	17.7	58.76	112.4	1.105	91.27	0.8935	102.4
SD	32.08	296.5	4.5	23.26	37.86	0.376	27.8	0.4774	31.35
Shapiro–Wilk normality test
Wilk constant	0.9843	0.9104	0.9857	0.9567	0.9466	0.8616	0.9806	0.6735	0.988
P	0.1501	P < 0.0001	0.2032	0.0005	P < 0.0001	P < 0.0001	0.0648	P < 0.0001	0.332

Bland–Altman analysis revealed a bias of 10.8% ± 21.2%, with a 95% limit of agreement from −0.8% to 52.4% between the Schwartz formula eGFR and the measured GFR. For CysC, the bias was −9.6% ± 21.6% with a 95% limit of agreement from −52.0% to 32.7%, and for BTP the bias was 1.4 ± 28.3 with a 95% limit of agreement from −54.0% to 56.8% (Table 2).

Table 2.

Bland–Altman results summarized for agreement of various eGFR formulas with the measured isotope GFR

Formula	Schwartz eGFR	Cystatin C eGFR	BTP eGFR
Bias	10.8	9.6	1.4
SD %	21.2	21.6	28.3
95% Limit of agreement	−0.80 to 52.4	−52.0 to 32.7	−54.0 to 56.8

The median (IQR) relative error (eGFR − GFR/GFR) for the Schwartz formula was +12 (IQR −4, +24)%, whereas the median error for CysC eGFR was −9 (IQR −21, +6)%, and for BTP eGFR was +5 (IQR −16, +25)% (Table 3).

Table 3.

Error by level of eGFR (eGFR − GFR/GFR) for various eGFR formulas

Formula	Schwartz eGFR	Cystatin C eGFR	BTP eGFR
Median error	+12.0	−9.0	+5.0
25th percentile error	−4.0	−21.0	−16.0
75th percentile error	+24.0	+6.0	+25.0

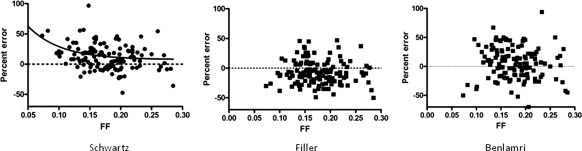
There was no correlation between the FF and the error for CysC eGFR and BTP eGFR, whereas there was a significant negative correlation between the error for the Schwartz eGFR and the FF (Figure 1). Furthermore, a significant negative correlation existed between FF and GFR, Schwartz GFR, CysC eGFR, and BTP eGFR. Clinically, this suggests that most patients with a lower GFR hyperfilter, whereas only some hyperfilter with normal GFR.

Figure 1.

The relationship between the percentage error of the Schwartz formula eGFR and the measured GFR plotted against the FF. There was a significant negative correlation (Spearman r = −0.2365, P = 0.0074). For the nonlinear regression model, we used a one-phase exponential decay model with the constants SPAN = 2295, K = 0.0001440, PLATEAU = −2295, and HalfLife = 4815.

Table 4 summarizes the correlation analysis (Spearman rank). There was no significant correlation between the error for the CysC eGFR and BTP eGFR and the FF. On the other hand, a significant negative correlation existed between FF and GFR, Schwartz GFR, CysC eGFR, and BTP eGFR.

Table 4.

Spearman rank correlations between the error of the GFR estimate models (BTP, CysC, Schwartz) with FF

Parameter	Schwartz Percent Error	CysC Percent Error	BTP Percent Error
Number of xy pairs	127	127	127
Spearman r	−0.2365	−0.08541	−0.1089
95% Confidence interval	−0.3988 to −0.05968	−0.2607 to 0.09535	−0.2826 to 0.07185
P value (two-tailed)	0.0074	0.3397	0.2232
P value summary	Significant	NS	NS

NS, not significant.

Discussion

The main finding of the study was that creatinine-based eGFR was influenced by the FF, whereas the accuracy of the eGFR from the Filler equation using serum CysC and the Belamri equation using serum BTP was unaffected. In fact, there was a significant negative correlation between error of eGFR calculated from the Schwartz formula and the measured GFR and the FF. To the best of our knowledge, this is the first study that demonstrates that the error between Schwartz formula eGFR and measured GFR is altered by hyperfiltration. By contrast, eGFR based on low-molecular-weight proteins was not altered by hyperfiltration.

This finding is novel and has significant implications. Previous studies have focused on the errors in eGFR from various surrogate markers and their respective formulas to the nuclear GFR studies (30–32). Better agreement was consistently found in the low GFR range, whereas the precision between measured GFR and surrogate marker eGFR worsened with normal and high GFR values (24,30). It was therefore logical to assess the effect of hyperfiltration on the diagnostic performance of surrogate eGFR markers. Previous studies did not include ERPF or FF.

What does this mean? It appears that a small-molecular-weight soluble substance can be affected by hyperfiltration, which weakens its diagnostic performance as a GFR marker, whereas low-molecular-weight proteins are unaffected. This would render serum creatinine a less accurate marker for eGFR in the presence of hyperfiltration. Because GFR may remain constant in the early stages of chronic kidney disease whereas the nephron endowment deteriorates secondary to a renal disease, patients with a normal GFR may or may not be hyperfiltrating. In advanced chronic kidney disease, all remaining nephrons hyperfilter (33). It is therefore conceivable that the degree of hyperfiltration may serve as the main explanation for the reduced precision of any surrogate GFR marker in the normal and high GFR range. Admittedly, the correlation was only 0.24, which was significant, but not very impressive. The study was not designed to discover a strong correlation between the error of a creatinine-based eGFR formula and the FF; rather, it was designed to test the hypothesis whether some of the variance of the scatter can be explained by the degree of hyperfiltration. The clinical significance of our findings lies in the fact that hyperfiltration can indeed explain some of the imprecision of creatinine-based eGFR, and patients early in the course of diabetic nephropathy and IgA nephropathy may especially have significant hyperfiltration. In the low GFR range, the phenomenon becomes less important, but our data suggest that creatinine handling may be altered by the FF to a degree that it renders the marker less favorable when compared with the low-molecular-weight eGFR markers.

Of course, the question arises as to why the small molecule creatinine is handled differently than the small-molecular-weight proteins CysC and BTP. All surrogate eGFR markers have different charges and isoelectric points. Because CysC and BTP are handled identically, electric charge is unlikely to explain the negative correlation between the error in eGFR for creatinine in the measurement of the FF. One possible explanation for this increase in error in creatinine-based eGFR using the Schwartz formula is that creatinine is also secreted by the renal tubule along with the excretion from glomerular filtration, whereas there is minimal or no tubular reabsorption of creatinine (34). CysC and BTP are exclusively eliminated through glomerular filtration. Therefore, with low FF, there is more blood flow in the efferent arteriole and subsequently more creatinine available in the peritubular capillaries for tubular secretion. This may lead to an increase of tubular secretion at lower FF, thereby creating an overestimation in the eGFR. It should be noted that the difference between the 25th and 75th percentile (i.e., IQR, a measure of precision) was inferior for BTP, suggesting that of the two low-molecular-weight proteins, CysC should be preferred.

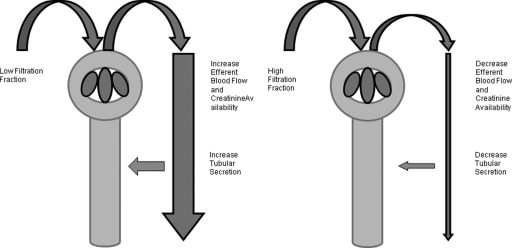
When the FF is increased, there is a decrease in efferent blood flow with a subsequent decrease of creatinine available for tubular secretion. Therefore, the eGFR from the creatinine-based formula correlates better with measured GFR at higher FF. The proposed differential handling of creatinine with lower and higher FF is demonstrated in Figure 2. Because tubular secretion does not modify CysC and BTP concentrations, the FF is unaffected by the error between the eGFR errors for CysC and the BTP-based formulas.

Figure 2.

The relationship between the FF and efferent blood flow. When the FF decreases, there is an increase in efferent blood flow and creatinine availability. This will lead to an increase in tubular secretion.

Our study has limitations. The first limitation is related to the nuclear medicine methods chosen to determine GFR and ERPF. No separate gold standard such as inulin clearance and para-aminohippuric acid (PAH) clearance were used to evaluate the accuracy of the nuclear medicine methods. Nuclear medicine methods are known to be imperfect measures of GFR and ERPF. Inulin and PAH clearance studies are no longer performed in most tertiary centers. However, the methods were validated and performed as described in reference 25 as the standard of care. Although earlier studies comparing inulin clearance and ⁵¹Cr-EDTA clearance mostly reported correlations upon introduction of the nuclear medicine methods, a recent study from Medeiros et al. used appropriate testing for agreement with Bland–Altman analysis for an identical method to ours and revealed narrow limits of agreement and a difference (bias) of 2.8 and 2.7 ml/min, respectively. They concluded that ⁵¹Cr-EDTA clearance was a reliable method to measure GFR compared with inulin clearance (35). We are unaware of any modern studies using Bland–Altman analysis to study agreement between ¹³¹I-hippurate clearance with PAH clearance; however, a study from 1980 demonstrated identical results with PAH clearance and slightly better performance of the ¹³¹I-hippurate clearance that we used in our study when compared with PAH clearance (36). Furthermore, this study was conducted in a pediatric population and it is unclear whether these findings can be generalized to all ages. In children, eGFR is calculated using the Schwartz formula that is based on creatinine and patient height. Adult eGFR calculations based on serum creatinine, such as the Modification of Diet in Renal Disease study equation and the Cockcroft–Gault equation, require additional information such as age, weight, gender, and ethnicity. In children, these formulas cannot be used (37). Before the findings of this study can be generalized, the increased eGFR errors that occur at lower FF will need to be confirmed in adult populations using adult eGFR equations that are based on serum creatinine.

The eGFR errors using the Schwartz formula change with the state of FF. It is impractical to measure FF in every patient. FF can only be measured if GFR and ERPF are determined simultaneously. In Canada, ¹³¹I or ¹²¹I-PAH are not commercially available. Furthermore, for a simultaneous nuclear medicine method, two different isotopes with γ and β radiation are required, which is impractical in North America because ⁵¹Cr-EDTA is not commercially available (38). Although we have a general rule of thumb that the tubular secretion for creatinine is approximately 10% of the total excretion (34), this may not be applicable for different degrees of hyperfiltration. The creatinine-based eGFR formulas may be unpredictable in terms of the eGFR errors. Other limitations include a relatively low sample size of 127 patients and a small proportion of patients with low GFR. Our study casts significant doubts on the accuracy of serum creatinine in patients with a variable degree of hyperfiltration. Early in the course of disease, hyperfiltration may or may not be operant. In the case of a GFR >150 ml/min per 1.73 m², hyperfiltration can be assumed, but in the case of normal GFR, hyperfiltration may or may not occur. Our study suggests that a surrogate marker for eGFR should be based on a low-molecular-weight protein rather than on serum creatinine.

The question of the clinical applicability of these findings remains to be established. Short of performing a proper inulin and PAH clearance study, FF is not easily measurable. Clinically, we assume hyperfiltration whenever the GFR is high. Our study also suggests that all patients with a low GFR hyperfilter. The importance of this study lies less in the clinical applicability of the effect of hyperfiltration on creatinine excretion, but more points to an important factor that explains some of the scatter when using surrogate markers for the estimation of eGFR. The study also suggests that CysC is less affected by hyperfiltration than creatinine.

In conclusion, this study showed that the creatinine-based Schwartz formula is influenced by FF. The errors of eGFR negatively correlate with FF. Only the eGFRs based on low-molecular-weight proteins (Filler equation using CysC and the Belarmi equation using BTP) are unaffected at different levels of FF. Further studies are required to test the result in adult populations with other creatinine-based formulas.

Disclosures

None.