Abstract
Recent work has demonstrated that sensitivity to interaural time differences (ITD) carried by high-rate cochlear implant pulse trains or analogous acoustic signals can be enhanced by imposing random temporal variation on the stimulus rate [see Goupellet al. (2009). J. Acoust. Soc. Am. 126, 2511–2521]. The present study characterized the effect of such “temporal jitter” on normal-hearing listeners’ weighting of ITD and interaural level differences (ILD) applied to brief trains of Gabor clicks (4 kHz center frequency) presented at nominal interclick intervals (ICI) of 1.25 and 2.5 ms. Lateral discrimination judgments were evaluated on the basis of the ITD or ILD carried by individual clicks in each train. Random perturbation of the ICI significantly reduced listeners’ weighting of onset cues for both ITD and ILD discrimination compared to corresponding isochronous conditions, consistent with enhanced sensitivity to post-onset binaural cues in jittered stimuli, although the reduction of onset weighting was not statistically significant at 1.25 ms ICI. An additional analysis suggested greater weighting of ITD or ILD presented following lengthened versus shortened ICI, although weights for such “gaps” and “squeezes” were comparable to other post-onset weights. Results are discussed in terms of binaural information available in jittered versus isochronous stimuli.
INTRODUCTION
It is well established that psychophysical sensitivity to envelope interaural time differences (ITD) carried by amplitude-modulated (AM) stimuli is limited by modulation rate: At modulation rates near 100 Hz, normal-hearing listeners are comparably sensitive to ITD carried by high-frequency AM signals and ITD carried by low-frequency pure tones, while for modulation rates beyond 200–300 Hz ITD sensitivity is generally poor (Henning, 1974; Hafter and Dye, 1983; Buell et al., 2008; Majdak and Laback, 2009). A number of explanations have been offered to account for this rate limitation, with one of the most persistent being “binaural adaptation,” a loss of sensitivity to post-onset binaural information evidenced by suboptimal improvement of discrimination thresholds with increasing stimulus duration for high-rate AM signals (Hafter and Dye, 1983; Hafter et al., 1983; Hafter et al., 1990). An unexpected feature of binaural adaptation described by Hafter and Buell (1990) and later Freyman et al. (1997) is the so-called “restarting” phenomenon, wherein a temporally irregular modulation in a high-rate AM signal (e.g., a lengthening or shortening of the interval between successive click pairs) improves ITD discrimination performance. More recent studies have shown that ITD sensitivity at high rates may be similarly enhanced by introducing random temporal perturbations (binaurally synchronous “temporal jitter”) in the timing of electrical stimulation (Laback and Majdak, 2008) or in the temporal envelopes of acoustic signals (Goupell et al., 2009), leading the authors of those studies to suggest that the benefit of temporal jitter might be attributable to a restarting of the adapted binaural system (after Hafter and Buell, 1990).
An alternative explanation for the results of Laback and Madjak (2008), first offered by van Hoesel (2008a,b), is that jitter improves ITD sensitivity simply by slowing the pulse rate over discrete portions of the stimulus and reducing the interpulse ambiguity of post-onset ITD (cf. Freyman et al., 1997). Goupell et al. (2009) evaluated this possibility but found that normal-hearing listeners were better able to discriminate ITD carried by jittered 1200 pulse-per-second (pps) stimuli than isochronous 600 pps stimuli, even though the 1200 pps stimuli contained interpulse intervals (IPI) at most equal in duration to the IPI of the isochronous 600 pps stimuli.
Although the possible application of these findings for the improvement of ITD sensitivity in bilateral cochlear implant (CI) users is intriguing, a number of critical questions concerning the effect of jitter remain. For example, to our knowledge, the effect of jitter on the discriminability of interaural level differences (ILD) carried by high-rate pulse trains has not been measured. The relationship between jitter and ILD sensitivity is of particular interest given the restarting hypothesis adopted by Laback and Majdak (2008) and Goupell et al. (2009), as binaural adaptation has been reported for both ITD (Hafter and Dye, 1983) and ILD (Hafter et al., 1983), and even when the cues have been presented alternately in interleaved pulse pairs—a result taken to suggest that binaural adaptation for both cues arises from a common mechanism (Hafter et al., 1990). Thus, although bilateral CI users generally show better baseline sensitivity to ILD than ITD, perhaps attributable in part to basic neurodevelopmental factors (Hancock et al., 2010; Litovsky et al., 2010), the same high-rate electrical stimulation thought to induce binaural adaptation for ITD (Laback and Majdak, 2008) should be expected to induce binaural adaptation for ILD. It follows that if the findings of Laback and Majdak (2008) and Goupell et al. (2009) are explained by a restarting of the adapted binaural system, imposing jitter on high-rate pulse trains should simultaneously improve listeners’ sensitivity to both ITD and ILD (i.e., temporal jitter should have no deleterious effect on ILD sensitivity). If, alternatively, the improvement in ITD sensitivity with jitter is not directly relatable to binaural restarting (cf. van Hoesel, 2008a,b), the effect of jitter on ILD sensitivity is not readily predictable. Given that free-field sound localization by bilateral CI users is thought to depend primarily on sensitivity to ILD, examining the effect of jitter on ILD sensitivity is essential for further consideration of its clinical implementation (see van Hoesel and Tyler, 2003; Grantham et al., 2008; Litovsky et al., 2010). Real-world sounds carry both ITD and ILD, and unintended disruption of ILD sensitivity by jitter could at least negate any gains in ITD sensitivity.
In a recent investigation, we characterized normal-hearing listeners’ sensitivity to ITD and ILD by measuring temporal weighting functions (TWFs) for discrimination of ITD or ILD carried by brief trains of clicks (Brown and Stecker, 2010). Rather than measuring whole-stimulus discrimination thresholds (e.g., Hafter and Dye, 1983; Hafter et al., 1983), TWFs measure the influence of discrete temporal portions of a stimulus on a subject’s perception, with portions of the stimulus that exert greater influence on perception receiving higher “weights” (e.g., Saberi, 1996; Stecker and Hafter, 2002; van Hoesel, 2008a,b; Brown and Stecker, 2010). A fundamental assumption of binaural adaptation is that lateralization is dominated by onset ITD and ILD at high modulation rates, while post-onset information contributes little to subjects’ perception. Correspondingly, TWFs measured in normal-hearing listeners for ITD and ILD carried by high-rate acoustic click trains (e.g., 800 Hz, Brown and Stecker, 2010) or in bilateral CI users for high-rate electrical pulse trains (e.g., 600 Hz, van Hoesel, 2008a) generally show high weights at onset and uniformly low weights for individual post-onset clicks, although these and other recent studies of temporal weighting of ITD and ILD (e.g., Saberi and Antonio, 2003; Saberi et al., 2004; Stecker and Brown, 2010) also suggested reduced onset dominance for ILD relative to ITD—a finding in conflict with the notion of equal binaural adaptation for ITD and ILD (Hafter et al., 1990). The nature of differences in the time courses of ITD versus ILD sensitivity, including the common finding of significant individual differences in sensitivity across listeners, remains an area of great interest to us and others at present (cf. McFadden et al., 1973 ; Krumbholz and Nobbe, 2002; Saberi and Antonio, 2003; Saberi et al., 2004; van Hoesel, 2008a,b; Litovsky et al., 2010; Stecker and Brown, 2010; Brown and Stecker, 2010; see Sec. 4B).
Nonetheless, since improved sensitivity to post-onset portions of the stimulus (i.e., reduced onset dominance) is the main consequence of binaural restarting, the restarting hypothesis adopted by Laback and Majdak (2008) and Goupell et al. (2009) clearly predicts that TWFs measured for jittered pulse trains should have more comparable weights across the duration of the stimulus than isochronous pulse trains. That is, to the degree that onset weights are elevated relative to post-onset weights given isochronous stimulation, jitter-induced restarting should “flatten” TWFs for both ITD and ILD. Baseline differences in ITD and ILD sensitivity (e.g., McFadden et al., 1973; Stecker and Brown, 2010; Litovsky et al., 2010) could affect the magnitude of differences for the two cues, but a reduction of onset weighting should be expected in both cases (cf. Hafter et al., 1990).
In the present study we measured TWFs to evaluate the effect of binaurally synchronous temporal jitter on normal-hearing listeners’ sensitivity to ITD and ILD carried by high-rate click trains. TWFs were measured for jittered click trains and compared to TWFs measured in a previous study using isochronous click trains (Brown and Stecker, 2010). In an additional analysis, we evaluated the weights of ITD and ILD presented after the longest and shortest gaps in the jittered click trains. Although binaural restarting has not previously been evaluated at the highest rate tested in the present investigation (800 Hz; see Hafter and Buell, 1990; cf. Freyman et al., 1997), van Hoesel’s (2008a,b) interpretation of the results of Laback and Majdak (2008) suggests that the effect of jitter might be directly observed as increased weighting of ITD presented following the longest interclick intervals (ICIs) in the jittered stimuli. Alternatively, the hypothesis of restarting of binaural adaptation adopted by Laback and Majdak (2008) and Goupell et al. (2009) suggests, by comparison to the data of Hafter and colleagues (Hafter and Buell, 1990; Hafter et al., 1990), that both the longest ICI (“gaps”) and shortest ICI (“squeezes”) should produce increased weights, with a similar effect for both ITD and ILD.
METHODS
The procedural methods employed in the current study were identical, except for differences in the stimuli presented, to those described previously by Brown and Stecker (2010). All procedures, including recruitment, consenting, and testing of human subjects followed the guidelines of the University of Washington Human Subjects Division and were reviewed and approved by the cognizant Institutional Review Board.
Subjects
Six normal-hearing subjects (0501, 0502, 0601, 0804, 0805, and 0815) aged 21–36 yr participated in this experiment. The same six subjects had participated in a previous experiment, from which the “isochronous” data in the present report are taken (Brown and Stecker, 2010). Subjects 0601, 0804, 0805, and 0815 were naive to the purposes of the experiment and were compensated for their participation. Subjects 0501 and 0502 were the two authors. All subjects reported normal hearing and demonstrated pure-tone detection thresholds <20 dB hearing level (HL) over the range 250–8000 Hz.
Stimuli and procedure
Stimuli were trains of 16 Gabor clicks (Gaussian-windowed tone bursts). Each click consisted of a 4 kHz cosine multiplied by a Gaussian temporal envelope with σ = 221 μs (367 μs duration at 3 dB below peak). The resulting spectrum was also Gaussian, with σ = 750 Hz (−3 dB bandwidth = 1250 Hz). Clicks were synthesized at 48.848 kHz (Tucker-Davis Technologies RP2.1, Alachua, FL) and presented using STAX model 4070 closed-back electrostatic headphones (STAX, Ltd., Saitama, Japan). Click trains were presented at approximately 70 dB sound pressure level (SPL) with mean peak-to-peak ICIs of 1.25 or 2.5 ms. The timing of individual clicks was determined using the temporal jitter procedure described by Goupell et al. (2009). For each trial, the ICI between successive clicks was drawn randomly from a uniform distribution centered at the nominal ICI (1.25 or 2.5 ms) with a width equal to 2k times nominal ICI. The parameter k thus defined the degree of temporal jitter, with k = 0 corresponding to temporal isochrony (no jitter) and k = 1 to maximal jitter (individual ICI ranging from 0 to 2*ICI). In the current experiment, “jittered” stimuli were generated with k = 0.9, and isochronous stimuli with k = 0.
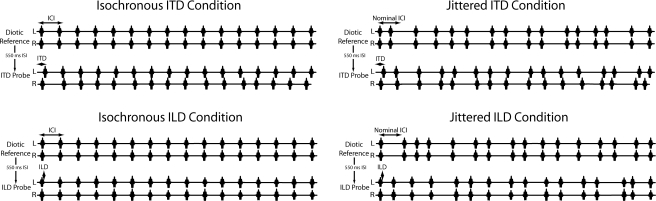
A button box (TDT RBOX) recorded subject responses. Testing was completed in a double-walled sound booth (IAC, Bronx, NY). Each trial consisted of two equal-duration click trains separated by a 550 ms silent interval. Click train duration was dependent on the ICI. The first train, presented as a reference, was always diotic; the probe train followed, presented with one of the three different “base” interaural values. Additional ITD or ILD variation was imposed on each click to facilitate the computation of TWFs (see Sec. 2C). Note that such variation was independent of the binaurally synchronous temporal jitter imposed on the ICI. Two separate conditions employed ITD and ILD cues (see Fig. 1).
Figure 1.
Schematic illustration of stimuli (not to scale). Each trial consisted of a diotic reference stimulus followed by a probe stimulus. In isochronous (left) conditions (tested in a previous investigation), the reference stimulus was comprised of 16 equal-amplitude Gabor clicks presented synchronously to the left and right earphones. Following a 550 ms silent interval, an ITD or ILD probe stimulus was presented, comprised of 16 Gabor click pairs with random ITD or ILD imposed on each. In the ITD probe, each click pair carried an ITD drawn from a uniform distribution of ±100 μs about a base ITD of −100, 0, or 100 μs (0 in the above illustration); clicks were presented at equal amplitude to the two earphones in this condition. In the ILD probe, each click pair carried an ILD drawn from a uniform distribution of ±2 dB about a base ILD of −1, 0, or 1 dB (0 in the above illustration); clicks were presented synchronously to the two earphones in this condition. The ICI, which corresponded to the rate of click presentation, was held constant within and between trials of a single run. The jittered conditions (right) were identical to the isochronous conditions with the exception that the ICI in both the reference and probe stimuli were varied randomly about the nominal ICI according to the parameter k (see text).
The base interaural value was varied pseudorandomly from trial to trial. In the ITD conditions, the base values were −100, 0, or 100 μs; in the ILD conditions, the base values were −1, 0, or 1 dB.1 By convention, negative ITD and ILD values led to or favored the left ear; positive ITD and ILD values led to or favored the right ear. Additional random ITD or ILD variation, drawn from a uniform distribution of ±100 μs or ±2 dB, was added independently to each click in a train. These ranges were selected to correspond roughly to the ±11° azimuthal variation employed by Stecker and Hafter (2002), based on the physical correspondence between azimuth, ITD, and ILD described by Gulick et al. (1989) and an ITD∕ILD trading ratio of approximately 50 μs∕dB (Hafter and Jeffress, 1968). Due to the per-click variation in ITD or ILD, trials with a base value of 0 carried nonzero interaural differences on each click, and thus a nonzero average ITD or ILD per trial.
Subjects were instructed to indicate via button press whether the probe stimulus was presented to the left or right of the reference stimulus. All subjects received training with visual feedback until performance stabilized. Feedback was then turned off and testing began. One hundred trials comprised a single run; in each run, 60 trials had 0 base interaural difference, 20 were left-leading, and 20 were right-leading. Base values were presented in random order over the course of each run; rate and cue type were randomized and counterbalanced between runs. For the jittered condition (k = 0.9), each subject completed four runs at each combination of ICI (1.25 or 2.5 ms) and cue type (ITD or ILD), giving 1600 trials in total (400 trials × 2 ICI × 2 cues). Only 0 base trials were included in the TWF analysis1 (960 trials in total; 240 trials × 2 ICI × 2 cues). An additional and equivalent set of runs was completed without temporal jitter (k = 0). Those data, previously reported by Brown and Stecker (2010), comprise the isochronous data included in the present report.
Statistical methods
TWFs were computed following the logic of previous observer-weighting approaches (e.g., Berg, 1989; Saberi, 1996; Stecker and Hafter, 2002). In the present investigation, weights were computed using a receiver operating characteristic (ROC) analysis to quantify the accuracy with which listener responses could be predicted on the basis of interaural differences applied on each trial. (For a detailed discussion of the application of ROC analysis for the computation of TWFs, see Brown and Stecker, 2010.) Classification performance was quantified by the area under the ROC curve (AUC) obtained for classification of subjects’ responses by several independently assessed classification variables, including the ITD or ILD applied to individual clicks (termed “AUC1” for click No. 1, “AUC2” for click No. 2, etc., plotted against click number to form the TWF itself), the mean ITD or ILD across clicks in a train (“AUCmean,” assessing the degree to which listener responses followed the mean ITD or ILD across clicks in the trains), and the ITD or ILD carried by the click pairs following the longest and shortest ICI of each trial (“AUCmaxICI” and “AUCminICI,” respectively). Since AUC values corresponded directly to the proportion of the subject’s responses correctly classified by each classification variable, ranging from chance (AUC = 0.5) (zero weight) to perfect classification (AUC = 1), obtained AUC values can be construed as perceptual weights, quantifying the influence of interaural differences carried by each classification variable on listeners’ performance. Comparing AUC1 against AUCmean (i.e., AUC1 − AUCmean) thus provides a measure of onset dominance, since onset dominance entails weighting of onset cues over long-term interaural information (cf. Saberi and Perrott, 1995), where optimal performance in this task is obtained by a cue-averaging strategy that minimizes decision variance (Saberi, 1996). Values of AUC1 − AUCmean and other AUC measures were compared in several null hypothesis tests described in Secs. 3 and 4. Statistical confidence intervals on weight estimates were generated using 1000-repeat permutation tests to define the range of chance classification about AUC = 0.5 (Figs. 2 and 3; see Brown and Stecker, 2010).
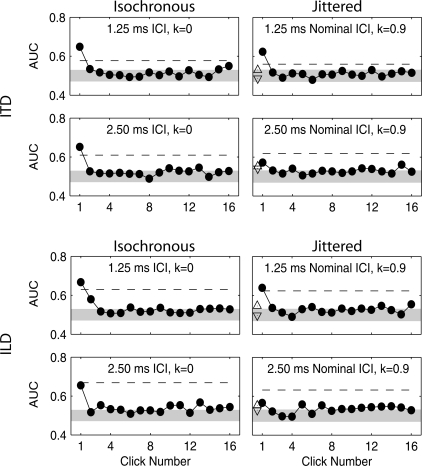
Figure 2.
TWFs averaged across subjects for isochronous (left column) and jittered (right column) conditions across ICI (rows) for both ITD (upper panels) and ILD (lower panels). Within each panel, per-click ITD or ILD weights (AUC1…AUC16, filled circles) are plotted against click number to form the TWF; the weight of mean ITD or ILD (AUCmean, dashed line) and the weight of ITD or ILD following the longest (AUCmaxICI, upward triangle) and shortest (AUCminICI, downward triangle) ICI per click train are plotted for comparison (see text). The shaded region plots the range of chance classification (see text).
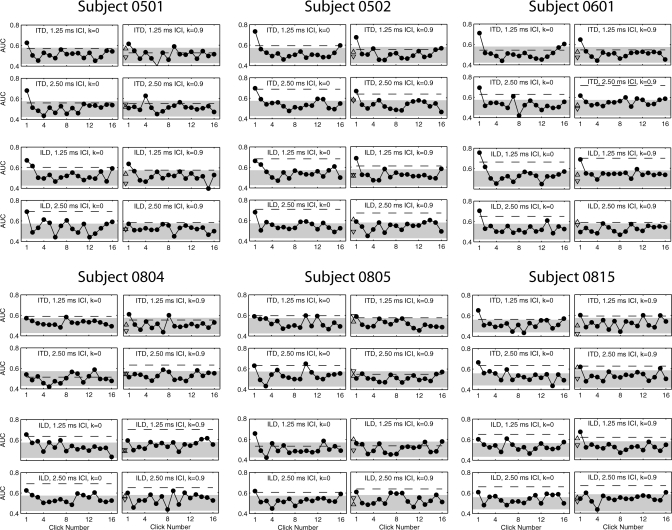
Figure 3.
Individual TWFs for isochronous and jittered conditions. Legend as in Fig. 2.
RESULTS
TWFs for ITD and ILD
Figure 2 displays TWFs for the four jittered conditions tested in the present investigation and four corresponding isochronous conditions tested in a previous investigation (Brown and Stecker, 2010). Filled circles in each panel plot the AUC obtained using each click ITD or ILD (AUC1…AUC16), the dashed line plots the AUC obtained using average ITD or ILD (AUCmean), and triangles plot the AUC obtained using the ITD or ILD of clicks following the maximum and minimum ICI within each click train (AUCmaxICI, upward triangle; AUCminICI, downward triangle; see Secs. 3B and 4C). The shaded region plots the range of chance classification (95% CI of AUC = 0.5) according to the distribution of AUC values obtained by classification of randomized responses (1000-repeat permutation test). Visually evident across both isochronous (left column) and jittered (right column) conditions is the decrease in AUC1 relative to AUC2…AUC16 and increase in AUCmean (dashed line) at 2.5 ms ICI versus 1.25 ms ICI. Correspondingly, for both ITD (upper panels) and ILD (lower panels), the value of AUC1 − AUCmean (i.e., the degree of onset dominance) is uniformly lower at 2.5 ms ICI than at 1.25 ms ICI. The trend is more pronounced in the jittered conditions; the value of AUC1 falls below AUCmean for both ITD and ILD at 2.5 ms ICI. Individual TWFs (Fig. 3) support these observations, although individual variability is clear (for an extended discussion of individual differences in TWFs, see Brown and Stecker, 2010).
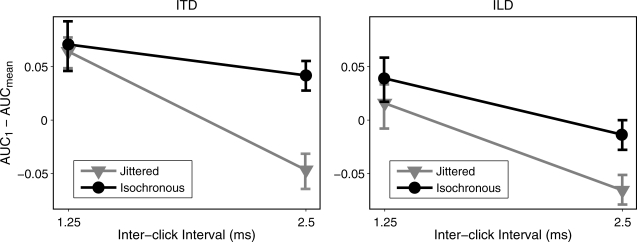
Individual subject values of AUC1 − AUCmean were submitted to a 2 × 2 × 2 repeated-measures analysis of variance (ANOVA) with factors of rate (1.25 and 2.5 ms ICI), jitter (isochronous and jittered), and cue (ITD and ILD). The main effect of rate was significant [F(1,5) = 87.87, p < 0.05], as was the rate × jitter interaction [F(1,5) = 7.04, p < 0.05], while neither the main effect of jitter [F(1,5) = 5.56, p = 0.065] nor the main effect of cue [F(1,5) = 6.43, p = 0.052] were significant. Taken together, these results suggest a general reduction of onset dominance with increasing ICI, augmented by the effect of jitter at 2.5 ms ICI in particular. Follow-up pairwise t-tests verified that AUC1 − AUCmean was significantly reduced by jitter for both ITD [t(5) = 3.21, p < 0.025] and ILD [t(5) = 5.29, p < 0.025] at 2.5 ms ICI but for neither cue at 1.25 ms ICI [ITD: t(5) = 0.20, p = 0.85; ILD: t(5) = 0.46, p = 0.66]. These results are summarized in Fig. 4.
Figure 4.
Cross-subject averages of AUC1 − AUCmean against ICI for isochronous (black) and jittered (gray) conditions for both ITD (left panel) and ILD (right panel). Error bars indicate one standard error of the mean. Data evidenced a significant reduction of onset dominance with jitter at 2.5 ms ICI.
Longest versus shortest gaps
Under a strict interpretation of the restarting hypothesis adopted by Laback and Majdak (2008) and Goupell et al. (2009), both lengthened ICI (gaps) and shortened ICI (squeezes) should contribute comparably to ITD discrimination, with the result expected to extend to both ITD and ILD discrimination on the basis of the work of Hafter et al. (cf. Hafter and Buell, 1990; Hafter et al., 1990). Thus, to test the effectiveness of ITD and ILD carried by clicks following gaps and squeezes, we computed AUC weights for ITD and ILD presented after the longest (AUCmaxICI) and shortest (AUCminICI) ICI in each train (jittered conditions only). The obtained values of AUCmaxICI and AUCminICI are plotted in the right panels of Figs. 2 and 3 as upward and downward triangles, respectively. Both AUCmaxICI and AUCminICI appear generally comparable to other individual post-onset weights (i.e., AUC2…AUC16), while AUCmaxICI appears marginally higher than AUCminICI. To test this difference statistically, the values of AUCmaxICI and AUCminICI were compared in a 2 × 2 × 2 repeated-measures ANOVA with factors of measure (AUCmaxICI and AUCminICI), rate (1.25 and 2.5 ms ICI), and cue type (ITD and ILD). The analysis yielded a significant main effect of measure [F(1,5) = 7.29, p < 0.05] but no significant main effect of cue [F(1,5) = 0.11, p = 0.75] or measure by cue interaction [F(1,5) = 0.34, p = 0.58]. The main effect of rate was measured at F(1,5) = 5.47, p = 0.066. Taken together, these results indicate that both ITD and ILD following the longest ICI exerted more influence on subjects’ perception than ITD and ILD following the shortest ICI, although both AUCminICI and AUCmaxICI were comparable to other post-onset weights and less than AUC1 and AUCmean across conditions. Further, the average value of both AUCmaxICI and AUCminICI fell above the range of chance classification in the 2.5 ms ICI ITD condition—the condition of the present investigation most similar to restarting conditions measured by Hafter and Buell (1990) and Freyman et al. (1997) (see Fig. 2).
DISCUSSION
The effect of jitter on TWFs for ITD and ILD
The present results indicate that imposing binaurally synchronous temporal jitter on high-rate (2.5 ms ICI) click trains reduces listeners’ dependence on onset ITD and ILD cues for lateral discrimination judgments. The effect was not apparent, however, at the highest rate tested (1.25 ms ICI), for which onset dominance was only marginally lower in jittered than in isochronous conditions (see Fig. 4). A reduction in onset dominance for jittered stimuli, attributable to improved sensitivity to post-onset binaural information (i.e., reduced binaural adaptation), is consistent with the overall improvements in ITD discrimination described by Laback and Majdak (2008) and Goupell et al. (2009). The finding of greater onset dominance at 1.25 ms ICI than 2.5 ms ICI with or without jitter is further consistent with results of previous TWF investigations using filtered clicks (e.g., Saberi, 1996; Stecker and Hafter, 2002), noise bursts (Dizon et al., 1998), and electrical impulses (van Hoesel, 2008a). It is interesting that the present investigation did not reveal an effect of jitter at 1.25 ms ICI (800 Hz), as Laback and Majdak (2008) and Goupell et al. (2009) demonstrated improved ITD discrimination with jittered stimuli at still higher rates. This discrepancy may be attributable to differences in the stimuli or performance measures employed. For example, the two previous studies employed trains of microsecond biphasic or monophasic pulses carrying fixed interaural cues, while the present investigation employed trains of millisecond Gabor clicks carrying variable interaural cues. Further, while the investigations of Laback and Majdak (2008) and Goupell et al. (2009) employed stimuli with gradual onsets so that subjects were forced to rely exclusively on post-onset information for discrimination judgments, the present study employed stimuli with strong onset cues. Finally, the present investigation did not explicitly measure listeners’ ITD or ILD discrimination performance but rather estimated relative sensitivity to ITD or ILD over discrete portions of the stimuli.
Greater cue-averaging for ILD than ITD
Although binaural adaptation occurs for both ITD and ILD (Hafter and Dye, 1983; Hafter et al., 1983; Hafter et al., 1990) and has been attributed to common pre-binaural processing (Hafter et al., 1990; cf. Goupell et al., 2009), there are strong indications in the literature that the time course of processing of ITD and ILD diverges at one or more levels of the auditory system. For example, discrimination of ILD presented at signal offset appears to be relatively better than discrimination of ITD at signal offset (Stecker and Brown, 2010; see also Saberi et al., 2004). Additionally, TWFs for lateralization suggest that onset dominance is reduced for ILD relative to ITD in both bilateral CI users (van Hoesel, 2008a,b) and normal-hearing listeners (Brown and Stecker, 2010). Suggestive of further differences in the time courses of ITD and ILD processing, Krumbholz and Nobbe (2002) demonstrated a striking disparity between ITD- and ILD-based “echo thresholds” in the buildup and breakdown of the precedence effect, with buildup more robust for click pairs carrying ITD than ILD and breakdown much more robust for click pairs carrying ILD than ITD.
In the present investigation (and the previous, Brown and Stecker, 2010) we have operationally defined onset dominance as the salience of onset information over long-term average information (AUC1 − AUCmean) after Saberi and Perrott (1995). As described above, jitter systematically lowered the value of AUC1 for both ITD and ILD, with no significant difference in the effect between ITD and ILD conditions. However, cue-averaging alone, as measured by the value of AUCmean—an explicit measure of the perceptual salience of mean ITD or ILD of all clicks in each train without respect to the weighting of individual clicks—appeared to be greater for ILD than ITD in all conditions (dashed lines, Fig. 2). To test this difference statistically, individual subject values of AUCmean were submitted to a 2 × 2 × 2 repeated-measures ANOVA with factors of jitter (isochronous and jittered), rate (1.25 and 2.5 ms ICI), and cue type (ITD and ILD). The main effects of both rate [F(1,5) = 25.76, p < 0.05] and cue [F(1,5) = 33.38, p < 0.05] were significant, suggesting that listeners not only relied on cue-averaging to a greater degree at 2.5 ms than at 1.25 ms ICI (cf. Hafter and Dye, 1983; Hafter et al., 1983) but also to a greater degree in ILD than ITD conditions (cf. Brown and Stecker, 2010; Stecker and Brown, 2010). Importantly, jitter neither affected the value of AUCmean [main effect of jitter, F(1,5) = 0.58, p = 0.48] nor differentially affected AUCmean for ITD versus ILD [jitter by cue interaction, F(1,5) = 0.36, p = 0.58]. This result suggests that the mechanism of jitter-reduced onset weighting may be separate from the mechanism facilitating greater cue-averaging for ILD, an interpretation consistent with similar time courses of ITD and ILD sensitivity evidenced in some paradigms (e.g., studies of binaural adaptation, Hafter and Dye, 1983; Hafter et al., 1983) and different time courses of sensitivity evidenced in others (e.g., studies of the precedence effect, Krumbholz and Nobbe, 2002; Saberi et al., 2004; cf. Stecker and Brown, 2010).
Restarting versus listening after the longest gaps
Hafter and Buell’s (1990) original account of binaural restarting indicated that the effect could be induced by either lengthening or shortening the ICI (i.e., by insertion of both gaps and squeezes). This result was tentatively verified by Freyman et al. (1997), who demonstrated using two subjects that either lengthening or shortening the IPI in an ongoing pulse train induced a shift in the perceived laterality of the stimulus to the side cued by the ongoing ITD, while perception was dominated by the opposing ITD cued at stimulus onset without such manipulations. Under the restarting hypothesis adopted by Laback and Majdak (2008) and Goupell et al. (2009), cues following both gaps and squeezes introduced by jitter should thus contribute to subjects’ discrimination judgments. This prediction, however, is not perfectly clear for the highest rates tested in this and the previous investigations (Laback and Majdak, 2008; Goupell et al., 2009). Hafter and Buell (1990) originally reported the restarting effect for 5 ms gaps inserted in 2.5 ms ICI click trains and for 2.5 ms squeezes inserted in 5 ms ICI Hz click trains. For the jittered 800 Hz (1.25 ms ICI) stimuli tested in the present investigation, the minimum possible ICI was 125 μs (k = 0.9), while in the 1200 pps condition of Goupell et al. (2009), the minimum possible ICI was 0 μs (k = 1.0). Thus, at very high rates, jittered stimuli are likely to include clicks which overlap partially or completely, such that, for acoustic stimulation, squeezes should be less effective or completely ineffective compared to gaps. Note, however, that this consideration does not necessarily apply to electrical stimulation (Laback and Majdak, 2008) and that benefits of jitter for acoustic stimulation have also been demonstrated at relatively low values of k (e.g., k = 1∕3, Goupell et al., 2009).
Our test of AUCmaxICI versus AUCminICI demonstrated that ITD and ILD following gaps were in fact more salient than ITD and ILD following squeezes. This result is consistent with TWFs measured for free-field sound localization by Stecker (2000; also see Stecker and Hafter, 2002), who reported restarting (as evidenced by increased weight following altered ICI) following 2 ms gaps but not 1 ms squeezes applied to click trains with 3 ms ICI. Although the finding is also roughly consistent with the suggestion of van Hoesel (2008a,b) that jitter enhances sensitivity to ITD following long intervals specifically, we note that the difference between AUCmaxICI and AUCminICI was statistically identical for stimuli carrying ITD and stimuli carrying ILD across all conditions. The result is therefore difficult to understand in terms of reduced temporal ambiguity of ITD (cf. Freyman et al., 1997 and van Hoesel, 2008a,b), because temporal ambiguity per se (i.e., multiple ITD cued by “slipped cycles” in a periodic click train with short ICI) is not expected to influence sensitivity to ILD (cf. Hartmann and Constan, 2002). Rather, it may be more appropriate to consider the effect of temporal jitter on peripheral filtering (Stecker and Brown, 2009) or neural refractoriness (see Goupell et al., 2009; van Hoesel, 2008b). Although the physiological bases of binaural adaptation and the restarting effect remain uncertain, Goupell et al. (2009) characterized the effects of temporal jitter on the firing synchrony of a model auditory nerve and showed that cross-correlating such responses led to better ITD sensitivity than did cross-correlation of responses to isochronous stimuli. While this account does not explicitly address the complementary question of ILD coding and is otherwise rather tentative, the fact that it does not require an active restarting mechanism suggests that at least some aspects of the effect of temporal jitter (and restarting itself) may reflect fairly low-level mechanisms of adaptation in primary auditory neurons. In future studies of observer weighting, classifiers which consider the combinatorial effect of multiple stimulus features (e.g., multiple long and short ICI) might thus be employed to better account for subjects’ behavior. Finally, future work aiming to assess the clinical feasibility of binaurally synchronous temporal jitter for the improvement of bilateral CI users’ binaural sensitivity should further examine the effects of temporal jitter on both ITD and ILD sensitivity.
SUMMARY AND CONCLUSIONS
-
(1)
TWFs for discrimination of ITD and ILD carried by isochronous high-rate click trains demonstrated greater weighting of cues applied to onset clicks than to individual post-onset clicks, consistent with previous studies demonstrating dominance of onset cues over ongoing cues for the localization of sounds with regular, rapid modulation.
-
(2)
The introduction of binaurally synchronous random temporal jitter in the envelopes of high-rate modulated stimuli reduced the dependence of listeners’ discrimination judgments on onset ITD and ILD. This finding is consistent with the view that improved ITD discrimination performance evidenced in previous investigations using jittered stimuli might be attributed to improved sensitivity to post-onset information (reduced binaural adaptation). Importantly, this finding suggests that binaural jitter has no deleterious effect on ILD sensitivity.
-
(3)
Cue-averaging (quantified by weight applied to the mean ITD or ILD of the complete click train) was found to be greater for ILD than for ITD stimuli, consistent with a greater role for temporal integration in ILD than ITD processing. This difference was not affected by the introduction of temporal jitter.
-
(4)
Thus, improved discrimination with jitter is not likely attributable to better cue-averaging over the duration of the stimulus or to reduced stimulus “ambiguity”; rather, the combinatorial effect of multiple long and short ICI may enhance the neural representation of the stimulus envelope (e.g., by increasing the effective modulation depth) to facilitate the encoding of interaural differences by central mechanisms (Goupell et al., 2009; Stecker and Brown, 2009).
ACKNOWLEDGMENTS
The authors thank Associate Editor Ruth Litovsky and two anonymous reviewers for comments on an earlier version of this manuscript, and Shiboney Dumo for help with data collection. This work was supported by National Institute on Deafness and Other Communicative Disorders (NIDCD), Grant Nos. R03-DC009482, T32-DC000033, and F31-DC010543.
Footnotes
AUC values (and thus TWFs) were computed and compared only on trials where the base ITD or ILD was 0. Trials presented with nonzero base ITD and ILD were included in the experiment to mediate the perceived task difficulty and to provide a basis for feedback during training. Although per-click variation in ITD or ILD was likely effective in biasing the degree of lateralization perceived, our discrimination task was inherently insensitive to gradations of lateralization; nonzero base trials, being in general strongly lateralized according to the sidedness of the base, were instead useful in sustaining vigilance between 0 base trials (on which biasing across the midline could be measured), and in ascertaining that subjects could perform the task at the outset of the experiment. Mean values for nonzero base conditions (±1 dB ILD, ±100 μs ITD) were selected to produce approximately equivalent lateralization in both conditions.
References
- Berg, B. G. (1989). “Analysis of weights in multiple observation tasks,” J. Acoust. Soc. Am. 98, 1909–1920. [DOI] [PubMed] [Google Scholar]
- Brown, A. D., and Stecker, G. C. (2010). “Temporal weighting of interaural time and level differences in high-rate click trains,” J. Acoust. Soc. Am. 128, 332–341. 10.1121/1.3436540 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Buell, T. N., Griffin, S. J., and Bernstein, L. R. (2008). “Listeners’ sensitivity to ‘onset/offset’ and ‘ongoing’ interaural delays in high-frequency, sinusoidally amplitude-modulated tones,” J. Acoust. Soc. Am. 123, 279–294 . 10.1121/1.2816399 [DOI] [PubMed] [Google Scholar]
- Dizon, R. M., Culling, J. F., Litovsky, R. L., Shinn-Cunningham, B. G., and Colburn, H. S. (1998). “On the development of a post-onset temporal weighting function,” Assoc. Res. Otolaryngol. Abstr. 21, 42. [Google Scholar]
- Freyman, R. L., Zurek, P. M., Balakrishnan, U., and Chiang, Y. C. (1997). “Onset dominance in lateralization,” J. Acoust. Soc. Am. 101, 1649–1659. 10.1121/1.418149 [DOI] [PubMed] [Google Scholar]
- Goupell, M. J., Laback, B., and Majdak, P. (2009). “Enhancing sensitivity to interaural time differences at high modulation rates by introducing temporal jitter,” J. Acoust. Soc. Am. 126, 2511–2521. 10.1121/1.3206584 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Grantham, W. D., Ashmead, D. H., Ricketts, T. A., Haynes, D. S., and Labadie, R. F. (2008). “Interaural time and level difference thresholds for acoustically presented signals in post-lingually deafened adults fitted with bilateral cochlear implants using CIS+ processing,” Ear Hear. 29, 33–44. [DOI] [PubMed] [Google Scholar]
- Gulick, W. L., Gescheider, G. A., and Frisina, R. D. (1989). Hearing: Physiological Acoustics, Neural Coding, and Psychoacoustics (Oxford University Press, New York: ), pp. 321–329. [Google Scholar]
- Hafter, E. R., and Buell, T. N. (1990). “Restarting the adapted binaural system,” J. Acoust. Soc. Am. 88, 806–812. 10.1121/1.399730 [DOI] [PubMed] [Google Scholar]
- Hafter, E. R., and Dye, R. H., Jr. (1983). “Detection of interaural differences of time in trains of high-frequency clicks as a function of interclick interval and number,” J. Acoust. Soc. Am. 73, 644–651. 10.1121/1.388956 [DOI] [PubMed] [Google Scholar]
- Hafter, E. R., Dye, R. H., Jr., and Wenzel, E. W. (1983). “Detection of interaural differences of intensity in trains of high-frequency clicks as a function of interclick interval and number,” J. Acoust. Soc. Am. 73, 1708–1713. 10.1121/1.389394 [DOI] [PubMed] [Google Scholar]
- Hafter, E. R., Dye, R. H., Jr., Wenzel, E. W., and Knecht, K. (1990). “The combination of interaural time and intensity in the lateralization of high-frequency complex signals,” J. Acoust. Soc. Am. 87, 1702–1708. 10.1121/1.399418 [DOI] [PubMed] [Google Scholar]
- Hafter, E. R., and Jeffress, L. A. (1968). “Two-image lateralization of tones and clicks,” J. Acoust. Soc. Am. 44, 563–569. 10.1121/1.1911121 [DOI] [PubMed] [Google Scholar]
- Hancock, K. E., Noel, V., and Delgutte, B. (2010). “Neural coding of ITD with bilateral cochlear implants: Effect of auditory experience,” Assoc. Res. Otolaryngol. Abstr. 33, 795. [Google Scholar]
- Hartmann, W. A., and Constan, Z. A. (2002). “Interaural level differences and the level-meter model,” J. Acoust. Soc. Am. 112, 1037–1045. 10.1121/1.1500759 [DOI] [PubMed] [Google Scholar]
- Henning, G. B. (1974). “Detectability of interaural delay in high-frequency complex waveforms,” J. Acoust. Soc. Am. 55, 84–90. 10.1121/1.1928135 [DOI] [PubMed] [Google Scholar]
- Krumbholz, K., and Nobbe, A. (2002). “Buildup and breakdown on echo suppression for stimuli presented over headphones—The effects of interaural time and level differences,” J. Acoust. Soc. Am. 112, 654–663. 10.1121/1.1490594 [DOI] [PubMed] [Google Scholar]
- Laback, B., and Majdak, P. (2008). “Binaural jitter improves interaural time-difference sensitivity of cochlear implantees at high pulse rates,” Proc. Natl. Acad. Sci. U.S.A. 105, 814–817. 10.1073/pnas.0709199105 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Litovsky, R. Y., Jones, G. L., Agrawal, S., and van Hoesel, R. J. M. (2010). “Effect of age at onset of deafness on binaural sensitivity in electric hearing in humans,” J. Acoust. Soc. Am. 127, 400–414. 10.1121/1.3257546 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Majdak, P., and Laback, B. (2009). “Effects of center frequency and rate on the sensitivity to interaural delay in high-frequency click trains,” J. Acoust. Soc. Am. 125, 3903–3913. 10.1121/1.3120413 [DOI] [PMC free article] [PubMed] [Google Scholar]
- McFadden, D., Jeffress, L. A., and Russel, W. E. (1973). “Individual differences in sensitivity to inter aural differences in time and level,” Percept. Mot. Skills 37, 755–761. [DOI] [PubMed] [Google Scholar]
- Saberi, K. (1996). “Observer weighting of interaural delays in filtered impulses,” Percept. Psychophys. 58, 1037–1046. [DOI] [PubMed] [Google Scholar]
- Saberi, K., and Antonio, J. V. (2003). “Precedence-effect thresholds for a population of untrained listeners as a function of stimulus intensity and interclick interval,” J. Acoust. Soc. Am. 114, 420–429. 10.1121/1.1578079 [DOI] [PubMed] [Google Scholar]
- Saberi, K., Antonio, J., and Petrosyan, A. (2004). “A population study of the precedence effect,” Hear. Res. 191, 1–13. 10.1016/j.heares.2004.01.003 [DOI] [PubMed] [Google Scholar]
- Saberi, K., and Perrott, D. R. (1995). “Lateralization of click trains with opposing onset and ongoing delays,” Acustica 81, 272–275. [Google Scholar]
- Stecker, G. C. (2000). “Observer weighting in sound localization,” Ph.D. thesis, University of California, Berkeley. [Google Scholar]
- Stecker, G. C., and Brown, A. D. (2009). “Modeling temporal weighting of interaural time and level differences in high-rate click trains,” J. Acoust. Soc. Am. 125, 2523. 10.1121/1.3124776 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Stecker, G. C., and Brown, A. D. (2010). “Temporal weighting of binaural cues revealed by detection of dynamic interaural differences in high-rate Gabor click trains,” J. Acoust. Soc. Am. 127, 3092–3103. 10.1121/1.3377088 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Stecker, G. C., and Hafter, E. R. (2002). “Temporal weighting in sound localization,” J. Acoust. Soc. Am. 112, 1046–1057. 10.1121/1.1497366 [DOI] [PMC free article] [PubMed] [Google Scholar]
- van Hoesel, R. J. M. (2008a). “Observer weighting of level and timing cues in bilateral cochlear implant users,” J. Acoust. Soc. Am. 124, 3861–3872. 10.1121/1.2998974 [DOI] [PubMed] [Google Scholar]
- van Hoesel, R. J. M. (2008b). “Binaural jitter with cochlear implants, improved interaural time-delay sensitivity, and normal hearing,” Proc. Natl. Acad. Sci. U.S.A. 105, E51. 10.1073/pnas.0801746105 [DOI] [PMC free article] [PubMed] [Google Scholar]
- van Hoesel, R. J. M., and Tyler, R. S. (2003). “Observer weighting of level and timing cues in bilateral cochlear implant users,” J. Acoust. Soc. Am. 113, 1617–1630. 10.1121/1.1539520 [DOI] [PubMed] [Google Scholar]