Abstract
Tumor vasculature and tissue oxygen pressure can influence tumor growth, metastases and patient survival. Elevated levels of lactate may be observed during the process of aggressive tumor development accompanied by angiogenesis (the evolution of the microenvironment). The non-invasive MR detection of lactate in tumor tissues as a potential biomarker is difficult due to the presence of co-resonating lipids that are present at high concentrations. Methods were previously reported for lactate editing using the SELective Multiple Quantum Coherence (SelMQC) method. Here we report a sequence ‘SS-SelMQC’, Spectral-Selective SelMQC, which is a modified version of SelMQC using binomial pulses. Binomial pulses were employed in this editing sequence for frequency excitation or inversion of selective lactate resonances. Lactate detection has been demonstrated using SS-SelMQC, both in-vitro (30 mM lactate/H2O doped with 25 μM Gd-DTPA) and in-vivo (Dunning R3337-AT prostate tumors), and compared to similar measurements made with SelMQC. Lactate areas were measured from non-localized spectra, one-dimensional (1D) localized spectra and two-dimensional chemical shift images (CSI) of the localized slice. In data from whole phantoms, the modified pulse sequence yielded enhancement of the lactate signal of 2.4 ± 0.40 times compared to SelMQC. Similar in-vivo lactate signal enhancement of 2.3 ± 0.24 times was observed in 1D slice-localized experiment.
Keywords: Lactate editing, single-scan lipid suppression, spectral-selective binomial pulses, 2D chemical shift imaging
INTRODUCTION
In vivo proton magnetic resonance spectroscopy (MRS) provides a noninvasive, biochemical measure of metabolism which can be used to differentiate healthy tissues from tumor tissues based on the metabolic abnormalities (1–3). Metabolites may be a priori markers of prognosis or metabolic changes can be very sensitive early indicators of treatment response. As such, metabolic approaches show promise for monitoring, and perhaps even ultimately, selecting treatments. As tumors are heterogeneous, multi-voxel magnetic resonance spectroscopic imaging (MRSI) provides a method to investigate therapy response and optimization of individual treatment regime based on various metabolite levels or concentrations within each voxel, which might signify aggressive (or indolent) disease (4–8) in local regions of the tumor. Among the many detectable metabolites, lactate (Lac) is an important compound which reflects elevated tumor glycolysis and/or poor tissue perfusion which may result in accelerated tumor growth, malignant transformation and metastases (9). Lac is the end product of glycolysis and the methyl resonance peak is typically a doublet situated at 1.3 ppm. Different levels of tumor lactate in the magnetic resonance (MR) spectrum were reported in the literature (10–15). High Lac was observed in extracts from biopsy specimens of breast tumors (10,16) and prostate tumors (11). Low extracellular pH and high Lac levels were shown to be indicators of metastatic risk in breast cancer xenografts (12,13). Elevated Lac content in biopsy samples was shown to correlate with increased risk of metastasis and poor patient survival in head and neck cancer (14) and cervical cancer (15), while a decrease in steady-state tumor Lac levels related to tumor response to radiation (17,18) and chemotherapy (19,20). It has also been shown by Pavel et al. (21) that Lac is likely to be found in malignant breast tumors which may be a marker for tumor diagnosis. Therefore, non-invasively measured Lac may be an additional characteristic metabolic marker for cancer studies and it may improve diagnostic specificity, serve as an early marker of tumor response, and provide functional information about prognosis.
Reliable measurement and interpretation of MRS-derived metabolites requires knowledge of the factors affecting Lac resonance detection and quantitation. Detection of relatively low Lac levels in tumor tissues using conventional MRS techniques such as PRESS (22) and STEAM (23), is a challenging task due to the presence of the intense co-resonant lipid (Lip) signals. To differentiate Lac from the co-resonating Lip resonances, spectral editing techniques, such as spin-echo (SE) J-difference spectroscopy (24) and multiple-quantum (MQ) coherence filters (25–28), have frequently been employed. Although the J-difference approach detects lactate with 100% signal sensitivity, the subtraction of sequential scans with different echo times (TE) for lipid suppression can cause artifacts resulting from subject motion and instrumental instabilities. MQ filters alleviate the problem of motion artifacts by the technique of a single-shot acquisition. However, these sequences generally exhibit signal contamination from residual lipid MQ coherences (25,26). In contrast, the selective MQ-coherence (SelMQC) transfer sequence avoids excitation of lipid MQ coherences, and thus offers complete lipid and water suppression in a single scan (28). Like most other MQ filters, the SelMQC sequence has the disadvantage of recovering only 50% of the lactate signal, due to the selection of a single coherence-transfer pathway. Although full Lac signal recovery is possible, a 2-step phase cycling procedure is required to refocus the lipid single quantum coherences and makes this method susceptible to motion artifacts. The conversion of this edited signal into accurate tissue concentrations of Lac requires corrections for signal loss arising from various additional sources. These include molecular diffusion, scalar coupling (J) effects, RF pulse imperfections, spin-lattice (T1), and spin-spin (T2) relaxation times.
In previous work (28), the SelMQC technique has been developed for selective detection of Lac CH3 protons without any interference from water and co-resonating lipid resonances. This technique has been successfully applied for lactate detection in breast cancer (21), the detection of an anti-neoplastic agent Iproplatin in murine RIF-1 tumors (27), and for detecting early response to cyclophosphamide treatment of RIF-1 tumors (17). Although this sequence’s efficiency for lactate editing with lipid suppression depends on selective excitation with RF pulses, it was shown that substituting a slice selective pulse for the first frequency selective RF pulse results in tolerable residual lipid resonances (28). To ensure complete lipid suppression, two step phase cycling of the first CH pulse with respect to the receiver is needed, which again may have motional instabilities.
Here we report a novel modification of the SelMQC, spectral-selective SelMQC (SS-SelMQC) sequence (Fig. 1) using composite binomial pulses (29) for Lac detection in non-localized and slice-localization versions, with complete removal of lipid and water resonances. Lactate spectra from non-localized, one-dimensional (1D) localized sagittal slice and two-dimensional (2D) CSI-lactate images were obtained from 30 mM Lac/Lip phantoms and in-vivo Dunning R3327 prostate animal tumors using our sequence and compared with the SelMQC sequence. Theoretical simulations were generated to understand the performance of the new sequence for different parameters employed in our experiments.
Fig. 1.
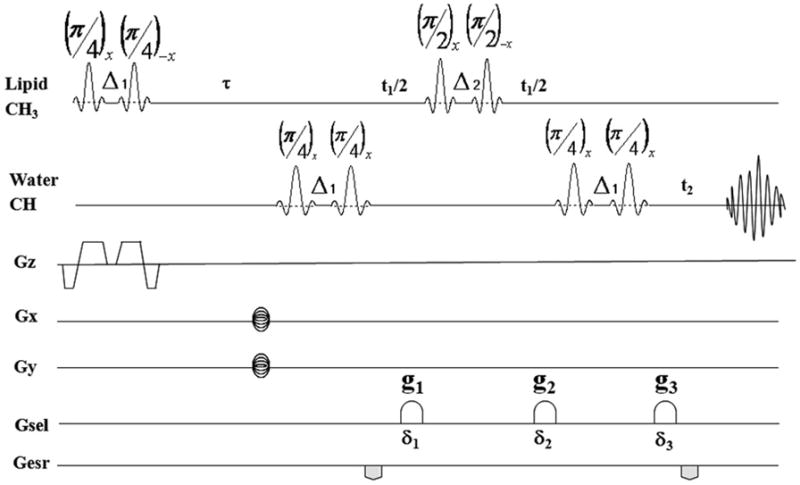
The SS-SelMQC pulse sequence. The ZQ→DQ coherence transfer pathway is selected with the Gsel gradients in a ratio of g1: g2: g3 = 0: −1: 2 (0: −14 G/cm: 28 G/cm) with duration δ1 =δ2 = δ3 = 2 ms. All other parameters were given in methods section.
METHODS
Theoretical
In the literature (30), composite binomial pulses with increasing order were described as {1-1}, {1-2-1}, {1-3-3-1}, etc. Initially binomial pulses were designed for spectral-selective excitation or inversion of spin resonances with water suppression in NMR spectroscopy (30). In recent years, the behavior of these pulses was explored for application to spatial selection in addition to spectral selection, particularly for water-fat MR imaging (31–33). RF pulses and slice select gradients for composite spatial-spectral pulses were applied in different ways (31).
In our present study, we used a {1-1} composite pulse consisting of two RF pulses of equal amplitude with spacing (Δ) between them. Frequency selective excitation was achieved by using a binomial pulse block and adjusting the phases of RF pulses to selectively excite either off-resonant Lac CH3 (spin I) resonances (φ1, φ2 = x, −x) or on-resonant CH (spin S) resonances (φ1, φ2 = x, x). Frequency selective inversion was achieved using [(π/2)x − Δ − (π/2)−x] for the Lac CH3 resonances without perturbing CH chemical shift. The time difference between the pulses, ‘Δ’, is equal to the reciprocal of twice the difference of the center frequencies of maximum (υI) and null (υS) excitation bands of I and S resonances.
where δυIS is the frequency difference I and S resonances.
When RF pulses are considered as delta pulses, Δ is the time delay between two elements of the {1-1} composite pulse. Under our experimental conditions where the RF pulses are shaped pulses with finite pulse widths, Δ needs to be corrected for finite pulse width effects. If we assume pw(π/4) and pw(π/2) are pulse widths of (π/4) and (π/2) RF pulses within binomial 90° and 180° composite pulses, then Δ1 (Δ − pw(π/4)) and Δ2 (Δ − pw(π/2)) are the effective interpulse delays (pulse width corrected) for 90° and 180° binomial blocks respectively.
Numerical computer simulations were generated to calculate the selectivity of these pulses for the Lac ‘IS’ spin system using spin density matrix calculations assuming the experimental field strength of 4.7T. The response of a nuclear spin ensemble to multiple RF irradiation pulses often deviates from the expected or the desired effect, primarily due to imperfections such as the spatial inhomogeneity of the radio frequency field and off-resonance effects. Due to the long durations of the shaped RF pulses, evolution due to scalar couplings and chemical shifts of both spins needs to be considered. The Hamiltonian of an IS system during the application of a RF pulse and free evolution (no RF effect) in the doubly rotating frame are given by
| [1] |
and
| [2] |
where ΔωI, ΔωS, JIS are chemical shifts of I, S spins and scalar coupling.ω1I(t),ω1S (t) and φ(t) are the RF field strengths in 3-lobe sinc shape with step duration ‘t’ on I and S spins, and their phase, respectively. We have examined the transient response of this spin system following the SS-SelMQC pulse sequence in the absence of the slice gradient which can be described as
| [3] |
where ρ(0) represents the equilibrium density matrix and, the RF pulse effect was calculated from the Hamiltonian using Eqn. [1] by considering scalar evolution term in addition to RF terms to account for coupling evolution during finite pulse durations. RF pulses within binomial pulse blocks individually are non-selective pulses and achieve spectral selectivity as an effect of composite blocks.
If we consider R(θ, φ) as a rotation propagator representing shaped RF pulse of flip angle θ and phase φ and U(t) as a free evolution propagator during time period ‘t’, Utotal can be written as
| [4] |
where
| [5] |
Although the propagator only deals with the first two lines of the pulse sequence, the effect of MQ gradients were considered in the simulation, by manually selecting the MQ coherence elements in the density matrix to choose the ZQ → DQ pathway.
Hence from Eqn. [3], the final transverse magnetization is calculated as
| [6] |
where the normalized in-phase <Mx (t)> and out-of phase<My (t)> components of the induced signal are given as
| [7] |
| [8] |
This time-domain complex signal is Fourier transformed to obtain the lactate spectra to test the performance of resonance offsets, coupling effects and RF inhomogeneity. We have employed the MATLAB software (MatWorks, Natick, MA) to develop a home-built pulse program for simulation of spectral-selective behavior, slice profiles, to study the influence of scalar coupling effects, RF finite pulse width effects, RF inhomogeneity effects and molecular diffusion effects.
Experimental
All MR imaging and spectroscopy experiments were performed on a 4.7T Bruker Biospin spectrometer (40 cm horizontal bore). Animal studies were conducted in compliance with protocols approved by the animal care protocols in Memorial Sloan-Kettering Cancer Center.
Phantom Preparation
A two-compartment cylindrical phantom (25 mm diameter) was prepared with 30 mM lactate/H2O doped with 25 μ Gd-DPTA and vegetable shortening (Crisco) side by side. This phantom was used to demonstrate the non-localized and localized Lac editing with water and lipid suppression (Fig. 3). A second phantom of 10 mM Lac/D2O was prepared for generation of slice profile (Fig. 4) and checking the experimental signal loss.
Fig. 3.
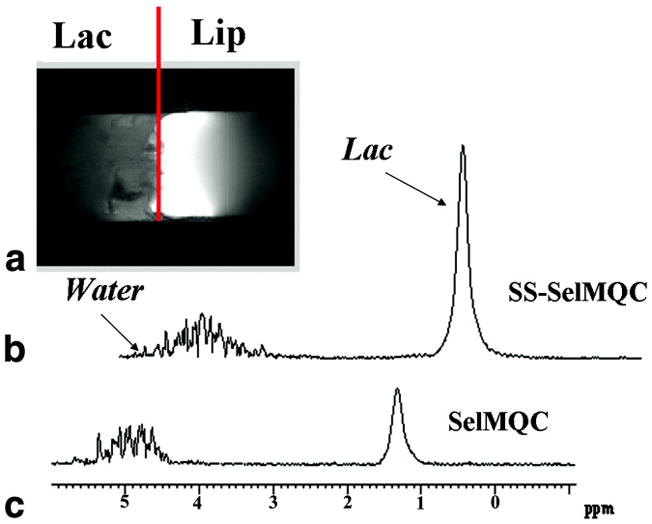
(A) Experimental coronal MR image obtained from lactate/lipid two-compartment phantom. Un-localized proton spectra of lactate obtained from this phantom were compared using SS-SelMQC (B) and SelMQC (C). Enhancement of 2.8 times was observed in SS-SelMQC compared with SelMQC. Water suppression with new sequence is similar to that obtained with SelMQC. Data was acquired with 16 number of scans with TR = 2 s. spectral width = 2500 Hz and total acquisition time is less than a 2 minutes. Measured linewidths of Lac signal were 34 Hz, 29 Hz in (B) and (C) respectively.
Fig. 4.
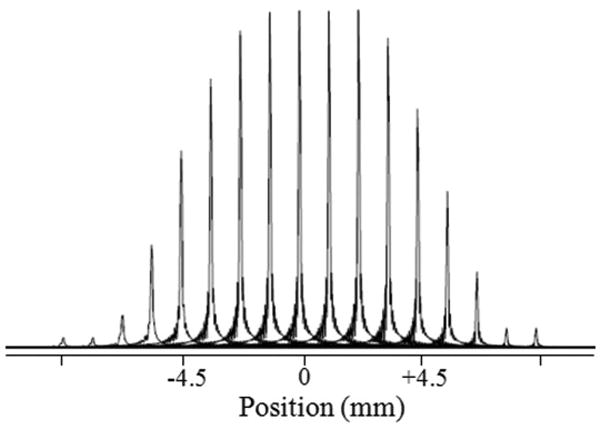
Experimental spatial-profile of lactate following the slice selective RF pulse block. TR=3 s, number of scans = 1, number of data points = 512, total acquisition time is less than 5 minutes. We used 10 mM Lac/D2O phantom for this experiment.
Animal Preparation and Tumor Volume Measurements
Frozen Dunning R3327-AT prostate cancer cells syngeneic to rats were thawed and seeded in 75 cm2 flasks. Copenhagen rats, supplied by Charles River Laboratories, were implanted with five million cells subcutaneously in the thigh. Tumor growth was evident visually about 10 days after implantation on the thigh region and volume increased to 800 mm3 – 2600 mm3 (not corrected for skin thickness) in an additional 5 to 8 days. Tumor volume was calculated as V = (π/6) * x * y * z; where x, y and z are the length, breadth and depth of the tumor.
MR experiments were performed at the tumor volume of 857 mm3. Each rat was anesthetized using a mixture of isoflurane (1.5 – 2.5%) and air and placed in the animal holder. The tumor was adjusted to be placed inside a 2 turn home built coil (25 mm diameter) tuned to 200 MHz and matched to 50 ohms prior to initiating MR experiments. The animal was placed inside a custom-designed MR platform with a 315 mm long section of 120 mm diameter plastic tube (the center of this platform matches with isocenter of the magnet). The animal was positioned in the center of this plastic cradle containing a RF coil, and was held in place with tape. Temperature was maintained at 37°C. The magnet was shimmed and resulted in a full width at half maximum peak height (FWHM) of less than 50 Hz for the water resonance in vivo.
MR Imaging
The Bruker ParaVision Tripilot pulse sequence was used to create three perpendicular images (FOV = 40 mm) as scout images for positioning of the animal tumor within the coil to ensure that the tumor was in the center of the magnet. Using these Tripilot scout images, T2-weighted MR sagittal images with a 5 mm thickness, using a Multi Slice Multi Echo (MSME) sequence (repetition time (TR) = 3734 ms; echo time (TE) = 30 ms; 512 × 128 matrix, number of excitations (NEX) = 2; field of view (FOV) = 40 mm) were obtained from the tumor located at isocenter. Total MR image acquisition including shimming took about 10 minutes.
MR Spectroscopy
Non-localized Lac MR spectra from whole phantom/tumors, 1D localized 5 mm sagittal slice as well as 2D CSI images from a sagittal slice were collected using SS-SelMQC sequence and compared with the previously reported SelMQC sequence (28). In vitro Lac/Lip phantom and in vivo R3327-AT prostate tumors were studied.
The MR spectroscopy acquisition parameters for SelMQC (28) included a 1 ms three-lobe sinc shaped slice-selective pulse and a frequency selective 15 ms single-lobe Sinc RF pulses for frequency selective excitation and inversion of CH and CH3 resonances. In SS-SelMQC, we used a high power three-lobe sinc shaped RF pulses for both (π/4) (200 μs duration) and (π/2) (400 μs duration) flip angles, a pulse repetition time (TR) of 2 s and spectral width of 2500 Hz; t1 = 2 ms; Δ1 = 693 μs; Δ2 = 493 μs (see Fig. 1); Due to RF finite pulse widths within the binomial pulse blocks, Δ1 and Δ2 values were adjusted to include the chemical shift evolution starting from the center of the first pulse to the center of the second pulse. In the binomial spectral-selective pulses, we chose to use broad band sinc shaped RF pulses. A slice gradient (trapezoidal shape) was applied to choose a 5 mm or 10 mm slice thickness. Gradient rise time and ramp down times were typically 100–150 μs. A phase cycling gradient scheme with g1: g2: g3 = 0: −1: 2 (0: −14 G/cm: 28 G/cm) with duration δ1 =δ2 =δ3 = 2 ms was employed in both sequences for the selection of ZQ→ DQ coherence selection pathway. The transmitter was set at the CH frequency. The Lac CH resonance frequency is at 4.1 ppm compared to the water signal (4.8 ppm). Whole tumor and 1D slice localized Lac spectra were obtained with 16 transients, respectively, without and with the application of slice-selection, and no phase encoding gradients were applied. The 2D 1H CSI data were collected by applying 16 × 16 phase encoding steps and field of view (FOV) = 40 mm, which results in a voxel size of 2.5 × 2.5 × 5 mm3. The total time for the 2D CSI experiment was ~1 hr 15 minutes (Number of excitations = 8). The anatomical sagittal T2-1H reference images were obtained with the same setup as the 2D CSI before starting CSI scan, so the MR images can be co-registered with 2D CSI data. The slice thickness in the T2-weighted image was matched with the slice thickness in the 2D-CSI for co-registration.
Data Processing
Non-localized and 1D slice-localized Lac spectral data were processed using Bruker Xwinnmr software and lactate signals were integrated using the area under the peak. To calculate the Lac signal enhancement for the SS-SelMQC sequence, we calculated the ratio of the lactate peak area in the SS-SelMQC to the Lac peak area in the SelMQC. Although quantification of lactate can be performed by the “substitution” method (34) which was used previously (35), since these studies were done sequentially without moving the sample (phantom/tumor), there was identical coil loading and similar rf inhomogeneity and therefore these imperfections will not affect the Lac signal enhancement ratio. Signal enhancement factors are represented in terms of mean ± standard deviation. 2D 1H-CSI images were reconstructed and overlaid with corresponding T2-weighted MR image using 3DiCSI processing software (Provided by Dr. Truman Brown, Columbia University). In 2D CSI data, voxels fully filled with lactate/lipid solution (phantom) were identified and the lactate peak areas within these voxels were calculated using iterative nonlinear least squares method using XSOS package (provided by Dr. Dikoma Shungu, Weill Medical College). The exact same voxels were analyzed from the data obtained using both sequences without considering B1 inhomogeneity factors and coil loading factor.
RESULTS
Excitation and inversion profiles following binomial pulses were generated using an in-house computer program representing the experimental conditions. The selective excitation profile for the binomial spectral-selective pulse is shown for excitation of −2.8 ppm resonance without disturbing 0 ppm resonance (Fig. 2A) and vice versa (Fig. 2B). Similarly, the selective inversion profile of the binomial spectral-selective pulse excites the ‘−2.8’ ppm resonance without disturbing ‘0’ ppm resonance (Fig. 2C). In generating this figure, the Lac CH peak was referenced to ‘0’ ppm and hence Lac CH3 will appear at ‘2.8’ ppm on the chemical shift. Fig. 3A shows the MR image of a 5 mm thick coronal slice across the phantom showing the two compartments with Lac and Lip respectively. Fig. 3B shows the non-localized Lac spectra obtained using SS-SelMQC and compared with SelMQC (Fig. 3C), from the whole phantom with excellent lipid suppression. Lipid suppression was verified by choosing a slice, using SS-SelMQC and SelMQC from the lipid portion of the cylindrical phantom, and no signal was detected at the Lac/Lip frequency (data not shown as voxels spectra contain no signal). Lac signal enhancement was observed in SS-SelMQC over SelMQC with no significant difference in performance with respect to water and Lip suppression levels. We repeated this experiment at three different times (each time point corresponds to a new experiment starting with positioning, tuning and matching) to test the consistency of signal enhancement in lactate signal from the whole Lac/Lip phantom. The integrated lactate signal area in SS-SelMQC is 2 to 3 times the lactate signal observed in SelMQC with similar suppression of lipid and water signals. Using paired t-test from EXCEL software, the differences in the Lac signal were statistically significant (p < 0.05) between different experiments with the signal enhancement factor being 2.4 ± 0.40 in non-localized SS-SelMQC, compared to SelMQC. Similarly we tested localized in vitro 1D Lac signal which yielded similar signal enhancement factors (data not shown). Localization was achieved by applying slice-select gradient on the first spectral-selective CH3 binomial block and its spatial profile was shown in Fig. 4.
Fig. 2.
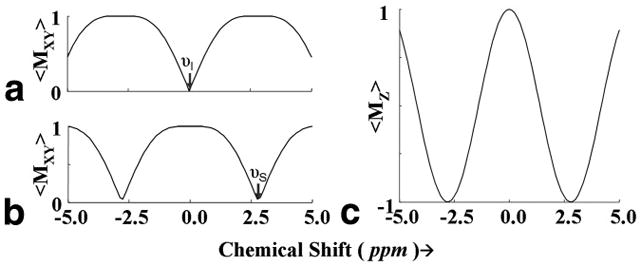
Theoretical excitation following ⌊(π/4)φ1 − Δ1 − π/4) φ2⌋ pulse sequence for spectral selection with (φ1, φ2=x, −x) (A) and (φ1, φ2=x, x) (B), as a function of resonance offset. Inversion profile of ⌊ (π/2)x − Δ2 −(π/2)−x⌋ for inversion of −2.8 ppm resonances shown in (C). In these simulations, the Lac CH peak was referenced to ‘0’ ppm and hence Lac CH3 will appear at ‘2.8’ ppm on the chemical shift. RF pulse parameters and interpulse durations were chosen to match the experimental conditions given in the text.
The efficacy of this pulse sequence was also analyzed by generating lactate signals derived from localized slices. 2D chemical shift imaging data was obtained from a 10 mm sagittal slice across the phantom located at the center (covering both Lip and Lac) using both methods (data not shown). With similar water and lipid suppression, lactate signal from different voxels shows statistically significant (p < 0.05) signal enhancement factor of 2.4 ± 0.29 times in SS-SelMQC, compared with SelMQC sequence. The result is similar to the enhancement observed in the non-localized spectra obtained using both sequences.
The same effect was demonstrated using R3337 tumors. Fig. 5A shows the stacked spectrum of lactate signal obtained from a 5 mm sagittal slice from a tumor (volume = 857 mm3) using both sequences. From peak area integrals, the lactate signal to noise with SS-SelMQC is more than 200% that obtained with the SelMQC. We also obtained 16 × 16 2D-CSI lactate maps (Fig. 5B) co-registered with a T2-weighted image corresponding to the slice shown in Fig. 5A. For comparison of both sequences, we did not move the sample between the two experiments.
Fig. 5.
(A) Stacked plot of experimental 1D localized 1H lactate spectrum obtained from in vivo R3327-AT tumor (volume = 857 mm3) using SS-SelMQC and SelMQC. Experimental parameters are described in text. (B) 2D Lactate CSI from sagittal 5 mm thick slice using SS-SelMQC. CSI data is overlaid on T2-contrast MR image. Chemical shift scale displayed over the tumor is between 2.5–0.5 ppm. Measured linewidths of Lac signal were 27 Hz, 25 Hz using SelMQC and SS-SelMQC sequences. Number of scans = 8, TR = 2s and total acquisition time is 1 hr 15 mins.
DISCUSSION
Enhanced signal to noise ratios of metabolites are important since it will lead to decreased scan time which is important both in clinical studies to minimize motion and patient discomfort, and in preclinical studies to minimize anesthesia, or higher spatial resolution.
Comparison of SS-SelMQC and SelMQC Pulse sequences
Following a series of preparation RF pulses and J-evolution delays in both sequences, lipid protons in addition to lactate protons can generate MQ coherences. By applying Lac CH-and CH3-frequency selective excitation pulses and CH3-inversion pulse, the fraction of lipid MQ coherences that can be converted to observable magnetization was minimized. In SelMQC, frequency selective sinc pulses were applied whereas in SS-SelMQC these pulses were replaced by binomial {1-1} pulses. We chose the ZQ→ DQ pathway in both sequences resulting in observation of only 50% Lac signal. Although full Lac signal recovery is possible by adding ZQ→DQ and DQ→ZQ coherence transfer pathways using a two step phase cycling of Lac CH pulse (28), this modification would make the method susceptible to subject motion and instrumental instabilities.
Single-slice localization in the SelMQC sequence by replacing the first frequency selective CH3-excitation pulse with a broad band excitation 3-lobe sinc pulse may introduce residual MQ coherences, although it is noted by He et al. (28) that this degradation in lactate editing efficiency is not noticeable. This effect was avoided in SS-SelMQC by replacement of the slice selective RF pulse of SelMQC with binomial pulse that removes the generation of residual lipid single quantum coherences that interferes with lactate signal and therefore reduces the lactate signal contamination. The theoretical profile for spectral selection (based on Fig. 2) has sharp transitions with approximately 50 Hz range is within the non-excitation band. For our study, prostate tumors were implanted on the thigh region and respiration implied frequency shift is likely to be negligible. But we propose to use higher order binomial pulses to have broader non-excitation region to alleviate the spectral selectivity issues due to the frequency shifts occur due to respiration. In SS-SelMQC, by manipulating the slice gradient profile, this binomial pulse acts as a spectral-spatial pulse although higher order binomial pulses are desirable for improved spectral-spatial behavior.
Reasons for Signal enhancement in SS-SelMQC
Reduced effects of J-scalar coupling evolution, molecular diffusion as well as T2 relaxation loss in SS-SelMQC are the likely causes of the signal enhancement observed experimentally, compared to SelMQC. These effects were simulated using computer simulations of un-localized lactate signals assuming an IS spin system. Lac editing efficiency in SelMQC sequence depends on frequency selective excitation or inversion profiles which necessitates the use of long pulses which increases the MQ evolution period and affects the lactate editing efficiency (28). In SS-SelMQC, we replaced all the long frequency-selective pulses with short binomial composite RF pulses for frequency selection resulted in shorter MQ evolution delays. This effect increased the Lac editing efficiency by reducing J-coupling evolution as well as molecular diffusion effects as described below.
Scalar coupling effects
Approximately 24% of the increase in the lactate signal observed in SS-SelMQC (Fig. 6A), in comparison with SelMQC (Fig. 6B) (assuming no RF pulse imperfections), can be explained from increased scalar coupling evolution during RF pulse finite widths. We denoted RF pulse imperfection (W1/W10), where W1 and W10 are actual and ideal RF field strength experienced by samples within RF coils. Hence (W1/W10) = 1 corresponds to no RF pulse imperfections and (W1/W10) = 0.9, 0.8 corresponds to the presence of 10% and 20% RF pulse imperfections respectively. In Fig. 6A and Fig. 6B, the X-axis is the chemical shift scale and the lactate doublet is centered on the reference chemical shift ‘1.32’ ppm which is the experimental frequency of CH3. The peak intensity is plotted with (J≠0) and without (J=0) scalar coupling evolution considered during RF finite pulse widths in the presence of RF pulse imperfections. The Y-axis denotes the Fourier transform of total transverse magnetization ‘Mxy’ following the SS-SelMQC and SelMQC sequences. Lac signal intensity was normalized as 100% when there were no scalar coupling effects and RF imperfections. Introducing scalar evolution during finite pulse widths, causes a decrease in Lac signal intensity to 99% in SS-SelMQC, with further reductions to 87% with 10% and 59% with 20% RF pulse imperfections. Similarly, in SelMQC, Lac signal intensity decreases to 75%, further reduces to 62% with 10% and 40% with 20% RF pulse imperfections. These simulations yielded a 24% signal gain for SS-SelMQC compared with SS-SelMQC. It should be noted that for patient studies at the clinical magnetic field strengths of 1.5T or 3.0T, the signal loss due to scalar coupling effects will be more severe using SelMQC compared to SS-SelMQC. The reason for this is that frequency selective pulses used in SelMQC need to be very long as chemical shift dispersion is very low and results in longer t1 MQ evolution delays leading to higher signal loss. Relatively much shorter t1 values can be maintained by binomial selective pulses in SS-SelMQC sequence resulting in minimum scalar coupling losses increases Lac signal sensitivity. We can further minimize signal loss observed in simulations due to RF imperfections by customized RF coils generating homogeneous RF fields.
Fig. 6.
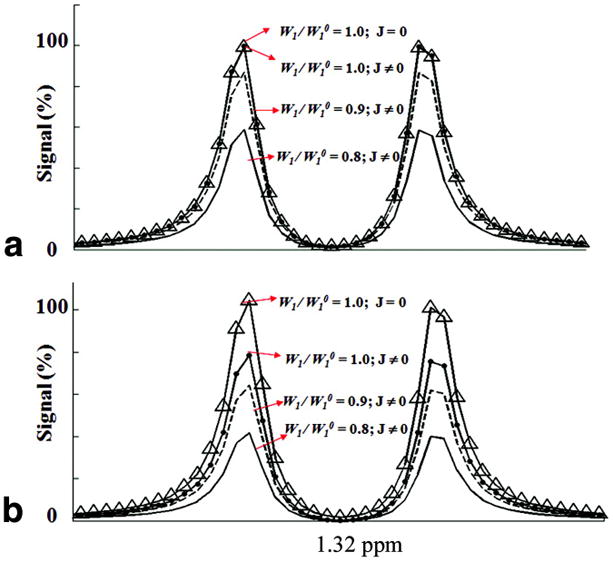
Computer simulated lactate spectra generated as a function of RF pulse imperfections and scalar coupling evolutions (J≠0) during RF pulses using A) SS-SelMQC and B) SelMQC sequence. Parameters used for generating these line plots were marked on figures. Y-axis represents percentage lactate signal normalized to 100% when there were no scalar coupling effects (J=0) and RF imperfections. These graphs show simulated spectra of lactate CH3 resonance corresponding to the 4.7T experimental conditions and assuming IS spin system for lactate (CH = I and CH3 = S) with scalar coupling JIS = 7 Hz.
T2 Relaxation Losses
Relatively short T2 values of lactate have been reported in in-vivo, for eg., rat C6-glioma with 200 ms (36) and mice tumors (37) with MCa (68 ms) and Colon-38 tumors (117 ms), which would lead to lower Lac detection efficiency. Since the in vivo T2 relaxation times are short, an optimal sequence minimizing T2 losses is critical for accurate measurements and for absolute quantification of tissue lactate concentrations. This effect may be particularly important in studies with small tumor volumes, where lactate concentrations may be low (35), or if used for early cancer diagnosis. Since the total duration of RF pulses in the modified sequence are shorter and approximately 45 ms less than SelMQC sequence, this enhances the lactate signal by 20% (assuming T2 = 200 ms) in the SS-SelMQC method by further reducing T2 relaxation losses.
Molecular Diffusion Effects
When two gradients are applied during the MQ evolution period to choose ZQ→ DQ pathways, Lac signal attenuation occurs due to molecular diffusion of molecules during the period (28) between these gradients of duration δ ms, following the Stejskal-Tanner equation (38). We assumed phantom conditions in our calculations using the diffusion coefficient D = 1 × 10−5 cm2 s−1 at room temperature. Using the equation reported earlier (28), the signal loss due to molecular diffusion is as follows,
| [9] |
Where Δ = t1+δ+ de, p is the coherence order, de is gradient dead time, γ is gyromagnetic ratio = 2.675e8 s−1T−1; for our present SelMQC parameters, the lactate signal loss will be 30% and signal loss in SS-SelMQC will be only 6%. Hence a signal gain of 24% is obtained in SS-SelMQC. The molecular diffusion losses will be severe in choosing the DQ→ZQ pathway to obtain the full signal intensity of lactate, or at low magnetic field strengths where long pulses result in higher signal loss.
Although the signal loss was calculated theoretically for individual imperfections by assuming other imperfections as zero, much higher signal loss obtained in our experiments may be due to these combined imperfection effects simultaneously in addition to magnetic field inhomogeneity effects. Signal enhancement was also confirmed from the Lac signal areas from SS-SelMQC, a single hard pulse, and SelMQC scans. We prepared 10 mM Lac/D2O solution as a phantom to test the non-localized lactate signal obtained by one pulse (rectangular shape), SelMQC, and SS-SelMQC sequences. If we normalize lactate signal from single pulse to 100%, approximately 50% lactate signal is obtained from SS-SelMQC compared to 24% signal obtained from SelMQC using identical experimental parameters for latter sequences (unpublished data). The possible in vivo lactate detection limit of SS-SelMQC sequence is in the range of 2–4 mM.
CONCLUSIONS
In summary, the demonstrated ability of the SS-SelMQC sequence to measure tumor lactate in the presence of subcutaneous lipids facilitate quantitative mapping of tumor Lac distributions in in vivo tumors. With lipid and water completely suppressed in a single scan, the lactate signal can be detected by SS-SelMQC from a desired sample or slice of interest in tumor tissues that contain lipid. These results demonstrate that the SS-SelMQC sequence can detect lactate with higher sensitivity without compromising the editing efficiency of SelMQC with similar lipid and water suppression in a single scan. Lactate signal obtained from whole tumors detected by SS-SelMQC has a statistically significant (p < 0.05) signal enhancement of 2.4 ± 0.40 times compared to the SelMQC sequence. 1D localized and 2D CSI maps were obtained from 5 mm thick sagittal slice using SS-SelMQC and showed similar sensitivity enhancement. These preliminary studies indicate efficient selection of a slice element and clearly point out the possibilities for obtaining, via the methodology outlined here, volume selection such that the desired metabolite region of interest is excited without exciting the unwanted lipid and water resonances. We are in the process of applying higher order binomial pulse such as {1-3-3-1} for uniform spectral-spatial behavior for slice and volume excitations. This method can be useful for carrying out studies for detecting increased glycolysis in exercised muscles and human breast cancer, as well as for lactate detection in the internal organs of transgenic animal tumor models.
Acknowledgments
NIH support from grants P50 CA86438, R24CA83084, P50-CA92629 and P01 CA115675.
Footnotes
Parts of this work was presented at the annual meeting of the International Society for Magnetic Resonance in Medicine, Toronto, ON, Canada; 3–9 May 2008.
References
- 1.Smith IC, Princz EJ, Saunders JK. Magnetic resonance spectroscopy in cancer research. Can Assoc Radiol J. 1990;41(1):32–38. [PubMed] [Google Scholar]
- 2.Fountas KN, Kapsalaki EZ, Gotsis SD, Kapsalakis JZ, Smisson HF, 3rd, Johnston KW, Robinson JS, Jr, Papadakis N. In vivo proton magnetic resonance spectroscopy of brain tumors. Stereotact Funct Neurosurg. 2000;74(2):83–94. doi: 10.1159/000056467. [DOI] [PubMed] [Google Scholar]
- 3.Tosi MR, Fini G, Tinti A, Reggiani A, Tugnoli V. Molecular characterization of human healthy and neoplastic cerebral and renal tissues by in vitro 1H NMR spectroscopy (review) Int J Mol Med. 2002;9(3):299–310. [PubMed] [Google Scholar]
- 4.Kurhanewicz J, Vigneron DB, Nelson SJ. Three-dimensional magnetic resonance spectroscopic imaging of brain and prostate cancer. Neoplasia. 2000;2(1–2):166–189. doi: 10.1038/sj.neo.7900081. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 5.Zaider M, Zelefsky MJ, Lee EK, Zakian KL, Amols HI, Dyke J, Cohen G, Hu Y, Endi AK, Chui C, Koutcher JA. Treatment planning for prostate implants using magnetic-resonance spectroscopy imaging. Int J Radiat Oncol Biol Phys. 2000;47(4):1085–1096. doi: 10.1016/s0360-3016(00)00557-5. [DOI] [PubMed] [Google Scholar]
- 6.Pirzkall A, McKnight TR, Graves EE, Carol MP, Sneed PK, Wara WW, Nelson SJ, Verhey LJ, Larson DA. MR-spectroscopy guided target delineation for high-grade gliomas. Int J Radiat Oncol Biol Phys. 2001;50(4):915–928. doi: 10.1016/s0360-3016(01)01548-6. [DOI] [PubMed] [Google Scholar]
- 7.Zakian KL, Sircar K, Hricak H, Chen H-N, Shukla-Dave A, Eberhardt S, Muruganandham M, Ebora L, Kattan MW, Reuter VE, Scardino PT, Koutcher JA. Correlation of Proton MR Spectroscopic Imaging with Gleason Score Based on Step-Section Pathologic Analysis after Radical Prostatectomy. Radiology. 2005;234(3):804–814. doi: 10.1148/radiol.2343040363. [DOI] [PubMed] [Google Scholar]
- 8.Chang J, Thakur S, Perera G, Kowalski A, Huang W, Karimi S, Hunt M, Koutcher J, Fuks Z, Amols H, Narayana A. Image-fusion of MR spectroscopic images for treatment planning of gliomas. Med Phys. 2006;33(1):32–40. doi: 10.1118/1.2128497. [DOI] [PubMed] [Google Scholar]
- 9.Rofstad EK. Microenvironment-induced cancer metastasis. Int J Radiat Biol. 2000;76(5):589–605. doi: 10.1080/095530000138259. [DOI] [PubMed] [Google Scholar]
- 10.Gribbestad IS, Petersen SB, Fjosne HE, Kvinnsland S, Krane J. 1H NMR spectroscopic characterization of perchloric acid extracts from breast carcinomas and non-involved breast tissue. NMR Biomed. 1994;7(4):181–194. doi: 10.1002/nbm.1940070405. [DOI] [PubMed] [Google Scholar]
- 11.Swanson MG, Zektzer AS, Tabatabai ZL, Simko J, Jarso S, Keshari KR, Schmitt L, Carroll PR, Shinohara K, Vigneron DB, Kurhanewicz J. Quantitative analysis of prostate metabolites using 1H HR-MAS spectroscopy. Magn Reson Med. 2006;55(6):1257–1264. doi: 10.1002/mrm.20909. [DOI] [PubMed] [Google Scholar]
- 12.Aboagye EO, Mori N, Bhujwalla ZM. Effect of malignant transformation on lactate levels of human mammary epithelial cells. Adv Enzyme Regul. 2001;41:251–260. doi: 10.1016/s0065-2571(00)00019-4. [DOI] [PubMed] [Google Scholar]
- 13.Bhujwalla ZM, Artemov D, Ballesteros P, Cerdan S, Gillies RJ, Solaiyappan M. Combined vascular and extracellular pH imaging of solid tumors. NMR Biomed. 2002;15(2):114–119. doi: 10.1002/nbm.743. [DOI] [PubMed] [Google Scholar]
- 14.Walenta S, Salameh A, Lyng H, Evensen JF, Mitze M, Rofstad EK, Mueller-Klieser W. Correlation of high lactate levels in head and neck tumors with incidence of metastasis. Am J Pathol. 1997;150(2):409–415. [PMC free article] [PubMed] [Google Scholar]
- 15.Walenta S, Wetterling M, Lehrke M, Schwickert G, Sundfor K, Rofstad EK, Mueller-Klieser W. High lactate levels predict likelihood of metastases, tumor recurrence, and restricted patient survival in human cervical cancers. Cancer Res. 2000;60(4):916–921. [PubMed] [Google Scholar]
- 16.Gribbestad IS, Fjosne HE, Haugen OA, Nilsen G, Krane J, Petersen SB, Kvinnsland S. In vitro proton NMR spectroscopy of extracts from human breast tumours and non-involved breast tissue. Anticancer Res. 1993;13(6A):1973–1980. [PubMed] [Google Scholar]
- 17.Poptani H, Bansal N, Graham RA, Mancuso A, Nelson DS, Glickson JD. Detecting early response to cyclophosphamide treatment of RIF-1 tumors using selective multiple quantum spectroscopy (SelMQC) and dynamic contrast enhanced imaging. NMR Biomed. 2003;16(2):102–111. doi: 10.1002/nbm.816. [DOI] [PubMed] [Google Scholar]
- 18.Quennet V, Yaromina A, Zips D, Rosner A, Walenta S, Baumann M, Mueller-Klieser W. Tumor lactate content predicts for response to fractionated irradiation of human squamous cell carcinomas in nude mice. Radiother Oncol. 2006;81(2):130–135. doi: 10.1016/j.radonc.2006.08.012. [DOI] [PubMed] [Google Scholar]
- 19.Aboagye EO, Bhujwalla ZM, Shungu DC, Glickson JD. Detection of tumor response to chemotherapy by 1H nuclear magnetic resonance spectroscopy: effect of 5-fluorouracil on lactate levels in radiation-induced fibrosarcoma 1 tumors. Cancer Res. 1998;58(5):1063–1067. [PubMed] [Google Scholar]
- 20.Lee SC, Huang MQ, Nelson DS, Pickup S, Wehrli S, Adegbola O, Poptani H, Delikatny EJ, Glickson JD. In vivo MRS markers of response to CHOP chemotherapy in the WSU-DLCL2 human diffuse large B-cell lymphoma xenograft. NMR Biomed. 2008;21(7):723–733. doi: 10.1002/nbm.1250. [DOI] [PubMed] [Google Scholar]
- 21.Shkarin P, He Q. Lactate Detection in Human Breast Cancer on 2.1T MR System. Proceedings of the 9th Annual Meeting of ISMRM; Glasgow, Scotland, UK. 2001. p. 2327. [Google Scholar]
- 22.Bottomley P. Spatial Localization in NMR Spectroscopy in Vivo. Annals of the New York Academy of Sciences. 1987;508 doi: 10.1111/j.1749-6632.1987.tb32915.x. [DOI] [PubMed] [Google Scholar]; Physiological NMR Spectroscopy From Isolated Cells to Man. :333–348. [PubMed] [Google Scholar]
- 23.Frahm J, Bruhn H, Gyngell ML, Merboldt KD, Hanicke W, Sauter R. Localized high-resolution proton NMR spectroscopy using stimulated echoes: initial applications to human brain in vivo. Magn Reson Med. 1989;9(1):79–93. doi: 10.1002/mrm.1910090110. [DOI] [PubMed] [Google Scholar]
- 24.Rothman DL, Behar KL, Hetherington HP, Shulman RG. Homonuclear 1H double-resonance difference spectroscopy of the rat brain in vivo. Proc Natl Acad Sci U S A. 1984;81(20):6330–6334. doi: 10.1073/pnas.81.20.6330. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 25.Freeman DM, Sotak CH, Muller HH, Young SW, Hurd RE. A double quantum coherence transfer proton NMR spectroscopy technique for monitoring steady-state tumor lactic acid levels in vivo. Magn Reson Med. 1990;14(2):321–329. doi: 10.1002/mrm.1910140217. [DOI] [PubMed] [Google Scholar]
- 26.Trimble LA, Shen JF, Wilman AH, Allen PS. Lactate editing by means of selective-pulse filtering of both zero- and double-quantum coherence signals. J Magn Reson. 1990;86:191–198. [Google Scholar]
- 27.He Q, Bhujwalla ZM, Maxwell RJ, Griffiths JR, Glickson JD. Proton NMR observation of the antineoplastic agent Iproplatin in vivo by selective multiple quantum coherence transfer (Sel-MQC) Magn Reson Med. 1995;33(3):414–416. doi: 10.1002/mrm.1910330315. [DOI] [PubMed] [Google Scholar]
- 28.He Q, Shungu DC, van Zijl PC, Bhujwalla ZM, Glickson JD. Single-scan in vivo lactate editing with complete lipid and water suppression by selective multiple-quantum-coherence transfer (Sel-MQC) with application to tumors. J Magn Reson B. 1995;106(3):203–211. doi: 10.1006/jmrb.1995.1035. [DOI] [PubMed] [Google Scholar]
- 29.Hore PJ. Solvent suppression in Fourier transform nuclear magnetic resonance. J Magn Reson. 1983;55:283–301. [Google Scholar]
- 30.Hore PJ. Nuclear magnetic resonance. Solvent suppression. Methods Enzymol. 1989;176:64–77. [PubMed] [Google Scholar]
- 31.Hardy PA, Recht MP, Piraino DW. Fat suppressed MRI of articular cartilage with a spatial-spectral excitation pulse. J Magn Reson Imaging. 1998;8(6):1279–1287. doi: 10.1002/jmri.1880080615. [DOI] [PubMed] [Google Scholar]
- 32.Kwok WE, Totterman SM, Zhong J. 3D interleaved water and fat image acquisition with chemical-shift correction. Magn Reson Med. 2000;44(2):322–330. doi: 10.1002/1522-2594(200008)44:2<322::aid-mrm21>3.0.co;2-q. [DOI] [PubMed] [Google Scholar]
- 33.Peng Q, McColl RW, Wang J, Weatherall PT. Novel rapid fat suppression strategy with spectrally selective pulses. Magn Reson Med. 2005;54(6):1569–1574. doi: 10.1002/mrm.20694. [DOI] [PubMed] [Google Scholar]
- 34.Hennig J, Pfister H, Ernst T, Ott D. Direct absolute quantification of metabolites in the human brain with in vivo localized proton spectroscopy. NMR Biomed. 1992;5(4):193–199. doi: 10.1002/nbm.1940050406. [DOI] [PubMed] [Google Scholar]
- 35.Yaligar J, Thakur S, Lupu M, Wang Y, Matei C, Zakian K, Koutcher J. Comparative study of tumor lactate and tumor vasculature in aggressive and indolent prostate cancer animal models by 2D MR Spectroscopic Imaging and DCE-MRI. Proceedings of the 16th Annual Meeting of ISMRM; Toronto, Ontario, Canada. 2008. p. 369. [Google Scholar]
- 36.Terpstra M, High WB, Luo Y, de Graaf RA, Merkle H, Garwood M. Relationships among lactate concentration, blood flow and histopathologic profiles in rat C6 glioma. NMR Biomedicine. 1996;9:185–194. doi: 10.1002/(SICI)1099-1492(199608)9:5<185::AID-NBM414>3.0.CO;2-X. [DOI] [PubMed] [Google Scholar]
- 37.Muruganandham M, Koutcher JA, Pizzorno G, He Q. In vivo tumor lactate relaxation measurements by selective multiple-quantum-coherence (Sel-MQC) transfer. Magn Reson Med. 2004;52(4):902–906. doi: 10.1002/mrm.20206. [DOI] [PubMed] [Google Scholar]
- 38.Saarinen TR, Johnson CSJ. High-Resolution Electrophoretic NMR. J Am Chem Soc. 1988;110:3332–3333. [Google Scholar]



