Lung tissue mechanics as an emergent phenomenon

Abstract

The mechanical properties of lung parenchymal tissue are both elastic and dissipative, as well as being highly nonlinear. These properties cannot be fully understood, however, in terms of the individual constituents of the tissue. Rather, the mechanical behavior of lung tissue emerges as a macroscopic phenomenon from the interactions of its microscopic components in a way that is neither intuitive nor easily understood. In this review, we first consider the quasi-static mechanical behavior of lung tissue and discuss computational models that show how smooth nonlinear stress-strain behavior can arise through a percolation-like process in which the sequential recruitment of collagen fibers with increasing strain causes them to progressively take over the load-bearing role from elastin. We also show how the concept of percolation can be used to link the pathologic progression of parenchymal disease at the micro scale to physiological symptoms at the macro scale. We then examine the dynamic mechanical behavior of lung tissue, which invokes the notion of tissue resistance. Although usually modeled phenomenologically in terms of collections of springs and dashpots, lung tissue viscoelasticity again can be seen to reflect various types of complex dynamic interactions at the molecular level. Finally, we discuss the inevitability of why lung tissue mechanics need to be complex.

the mechanical properties of lung tissue have been a subject of ongoing scientific interest ever since it was first realized that lung elastic recoil plays a crucial role in breathing. Furthermore, a variety of important diseases involve significant alterations in lung tissue mechanics. For example, elastic recoil is markedly elevated in pulmonary fibrosis (69) and surfactant deficiency (41) and can be greatly reduced in emphysema (21). In fact, virtually all pulmonary diseases of either the obstructive or restrictive classification involve some abnormality of lung tissue mechanics (8, 63). This has led to a substantial effort to try and understand how the macroscopic mechanical properties of lung tissue arise from its microscopic components (57, 64). The fact that our knowledge of the link between lung tissue structure and function is still incomplete attests to the complexity of the problem. This complexity arises because the mechanical behavior of lung tissue is not simply a reflection of the properties of its constituents, and in fact bears little resemblance to the behavior of the individual proteins, fibers, cells, and fluids of which it is composed. Instead, gross tissue mechanics arise principally from the way in which the components are arranged with respect to each other and how they interact. Lung tissue mechanical properties are thus emergent in the sense that they cannot be understood simply on the basis of the properties of the components in isolation (6).

The reductionist approach alone is thus not sufficient to yield a complete understanding of lung tissue mechanics. An integrative, or systems, approach is also required to elucidate how the macroscopic behavior of lung tissue emerges from the ensemble behavior of its constituents. This generally involves mathematical and/or computational modeling because the relationships involved are typically numerous and highly nonlinear. In this review, we describe our current understanding of how some of the most important mechanical aspects of lung tissue arise from the ensemble behavior of its constituents and how this leads to a better understanding of the nature of certain parenchymal diseases.

QUASI-STATIC ELASTIC BEHAVIOR OF LUNG TISSUE

The bulk elastic behavior of lung tissue is reflected in the relationship between inflation pressure and volume inhaled into the lungs (7). These measurements are easily made in an isolated lung inflated and deflated either in a slow continuous manner or step-wise with positive pressure applied at the airway opening. Measurements can also be readily obtained in a living human with the use of an esophageal balloon to determine transpulmonary pressure (P) as the difference between esophageal and airway opening pressures, while volume (V) changes are measured at the mouth. However, lung tissue is also viscoelastic, which means that its apparent elastic properties are a function of how rapidly volume is cycled. It is impossible to measure the purely static elastic properties because this would require changing V at an infinitesimal rate, so one has to settle for obtaining the quasi-static properties that manifest during cycling rates that are slow compared with the rates of normal breathing. The ratio of the changes in V to those in P obtained under these conditions defines the quasi-static compliance of the lung (C), which has a straightforward phenomenological interpretation; it describes how easy it is to inflate the lung, and it scales inversely with lung size (i.e., with the amount of tissue of which the lung is composed). On the other hand, relating C to the biophysical properties of its cells, elastin, collagen, and proteoglycan constituents is far from simple (64). Indeed, these constituents are interwoven into a complex network, so C does not reflect the properties of any of the constituents directly but instead is somehow an emergent property of the entire system.

The full P-V curve typically exhibits a sigmoidal shape. At high V, the tissue undergoes strain stiffening, defined as a progressive increase in stiffness (decrease in C) with increasing strain. As V decreases toward low values, C also decreases as a result of progressive closure of lung units. C is highest at intermediate values of V corresponding to the range typical of normal breathing.

The P-V curve of the lung is markedly affected by surface tension in the air-liquid interface that lines the alveoli and airways. This is amply demonstrated by the drastic increases in lung stiffness that occur following lavage, which removes the pulmonary surfactant, and by the corresponding decreases in stiffness seen in the saline-filled lung in which surface tension no longer exists (2). Surface tension also contributes substantially to the quasi-static hysteresis of the P-V loop (i.e., the width of the loop persists as cycling frequency tends to zero) (2, 54, 59). The hysteresis reflects the complex dynamical behavior of surfactant molecules that are recruited to the air-liquid interface from sub-surface stores during inspiration and then participate in energy dissipation through the collapse of surface molecular structures during expiration (32). Much of the bulk elastic recoil behavior of the intact lung in vivo, at least at volumes near functional residual capacity, is thus a consequence of the ensemble behavior of the surfactant lipids and proteins forced to interact with each other and various other molecules within the geometric constraints of the alveolus (32, 54, 58, 59).

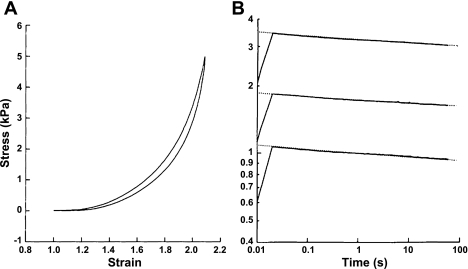
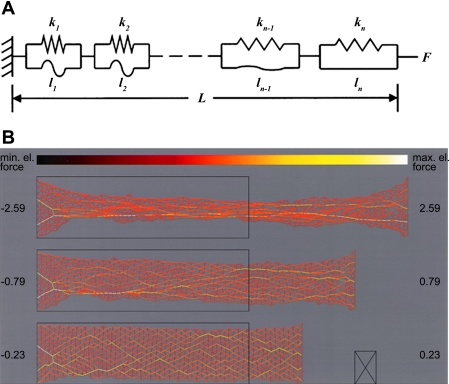
Even in isolation, however, without the complications introduced by surface tension and a tortuous surface geometry, lung parenchymal tissue alone exhibits strain stiffening as shown in Fig. 1A (6, 26) of the kind invariably seen in biological soft tissues of any origin (34). The principle reason for this has long been postulated to be due to the interplay between fibers of collagen and elastin (42, 53), the two main structural proteins found in lung parenchyma (52). Fibers of collagen and elastin are thought of as being woven randomly into a tissue network that comprises the alveolar wall, which itself exhibits a highly nonlinear length-tension relationship (18). At low strain, the stress in the network is borne predominately by the elastic fibers that are easily stretched and capable of changing length by at least a factor of two (20). Collagen, by contrast, is much stiffer and can only bear a strain of a few percent before rupturing (23), so at low strain the collagen fibers are crimped and flaccid. As strain increases, progressively more of the collagen fibers reach their uncrimped length and begin to take up the stress. This causes the overall stress in the tissue to also increase progressively, giving rise to the familiar asymptotic shape of the lung P-V curve. Notice that it is a network effect; indeed, without a distribution of crimped lengths of the collagen in a network, it is not possible to obtain a smooth stiffening curve for the overall network. The bulk P-V behavior of the tissue thus does not resemble the stress-strain behavior of either elastin or collagen at all, but rather reflects the way in which collagen gradually takes over the stress bearing role from elastin as V increases. Of course, there is much more to the micro-level architecture of lung tissue than a random array of collagen and elastin fibers. While cell contractility (47) and proteoglycans (10) can modulate stiffness, a major contributing role is played by alveolar geometry and surface forces (68), with concomitant effects on fiber orientation (43). Nevertheless, the essential idea of collagen fiber recruitment can be modeled quantitatively in terms of either a sequence of identical springs associated with parallel strings of different lengths or as a sequence of identical strings with parallel springs having a distribution of stiffnesses (37) (Fig. 2A). In the latter case, the predicted distribution of spring constants is reminiscent of the distribution of widths of elastin fiber bundles in the lung (56).

Fig. 1.

A: quasi-static stress-strain curve from a strip of degassed canine lung parenchymal tissue stretched uni-axially between an actuator and a force transducer. B: stress relaxation profiles from the same tissue strip following sudden 10% increases in strain from three different starting lengths. Note that the slopes are all essentially identical in the log-log plot despite the highly nonlinear stress-strain behavior. [Reproduced with kind permission from Springer Science+Business Media; Ref. 6.]

Fig. 2.

A: schematic representation of a series of spring-string pairs representing a strip of lung parenchymal tissue. The extensible springs (springs constants k₁, k₂, etc.) represent elastin fibers, while the inextensible strings (lengths l₁, l₂, etc.) represent collagen fibers. [Reproduced with permission from Ref. 37.] B: 2-dimensional network model of lung parenchymal tissue at various stages of stretch. Each line element represents a spring with a highly nonlinear stress-strain relationship. The black rectangles show the resting tissue shape. The numbers in the margin are total force. Note how a percolating series of contiguous links with high stress appears as strain increases. [Reproduced with permission from Ref. 38.]

The same mechanism can be modeled numerically in two dimensions using a network of interconnected elements having piecewise linear stress-strain properties (38). The addition of a second dimension, however, allows another interesting type of emergent behavior to arise through a process known as percolation. Specifically, as network strain increases, increasing numbers of network elements move up onto the stiff portions of their respective stress-strain curves. To begin with, these stiffened elements appear randomly throughout the network, but eventually there comes a point at which a contiguous pathway of connected stiffened elements spans, or percolates, across the network from one end to the other (Fig. 2B). At this point, the so-called percolation threshold, the bulk stiffness of the network suddenly starts to accelerate with further strain. In other words, percolation provides a general mechanism by which changes at the microscopic level are able to eventually have a large impact at the macro scale (60). It is not the only such mechanism, however. Others include geometric effects whereby stretch in a particular direction causes realignment of microstructural components in the direction of strain so that they contribute more efficiently to the maintenance of stress (3, 10, 33).

ALTERED STRUCTURE AND FUNCTION IN DISEASE

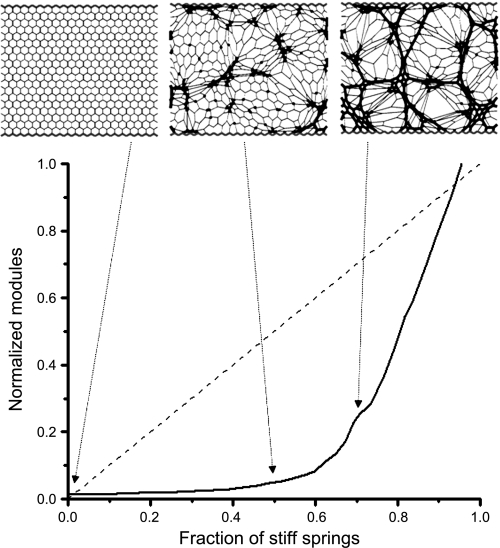
Percolation can also be invoked to explain a possible link between gross symptoms and underlying disease progression in certain parenchymal pathologies. This is best illustrated with respect to the development of pulmonary fibrosis, again in terms of a network model (5). Suppose, for example, that normal parenchymal tissue can be represented as a uniform network of interconnected springs that together have a certain bulk modulus corresponding to lung elastance. Also suppose that pulmonary fibrosis can be represented by the random stiffening of individual springs in the network, representing the development of focal fibrotic lesions. As more springs become stiffened, the bulk modulus of the entire network increases. Again, however, a percolation transition occurs when a continuous chain of stiffened springs spans the network, causing a sudden upturn in the rate of increase in bulk modulus (Fig. 3). Thus the sudden increase in the sensitivity of the network to adding one more stiff element is a collective emergent phenomenon that occurs only after percolation in the network has been achieved. Pulmonary fibrosis is, of course, a complex pathology that remains poorly understood (48), so it is by no means certain that percolation of this nature plays a dominant role in all cases of the disease. Nevertheless, this simple mechanism may explain why some patients appear to take a sudden turn for the worse after an extended period of apparently modest disease progression (40).

Fig. 3.

Simulation of the progression of pulmonary fibrosis using a 2-dimensional network of Hookean springs fixed at its borders (each line element is a spring). The solid curve shows the bulk modulus of the elastic network versus the fraction of springs randomly stiffened by a factor of 100 (normalized to the modulus of the network when all springs are stiffened). The network configurations obtained when 0%, 50%, and 67% of the springs have been stiffened are linked to their respective positions on the modulus plot by the dotted arrows and show that the percolation threshold occurs between 50% and 67%. If all of the spring constants are uniformly stiffened in a gradual manner from the baseline value of 1 to 100, the modulus follows the dashed diagonal line. [Reproduced with permission of the American Thoracic Society. Copyright American Thoracic Society (5).]

In the simple example considered above we assumed that fibrotic lesions (stiffened springs) appear at random locations within the lung tissue. It is quite plausible that inter-cell communication may cause these lesions to become spatially correlated, which would affect the average number of lesions required to reach the percolation threshold. Thus if the processes that give rise to one lesion are somehow involved in creating other lesions nearby, then the spatial pattern of pathology and the rate of progression of functional deficit would be affected. In other words, the pathologic processes themselves may provide a kind of feedback that influences further disease progression.

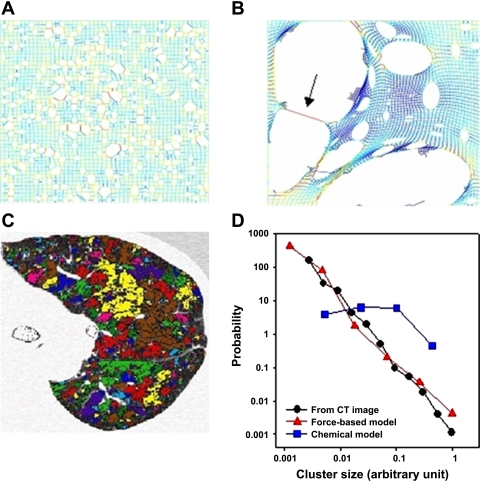
Emphysema is a parenchymal disease in which such feedback is likely to pertain. Emphysema involves the progressive destruction of the parenchymal microstructure, resulting in the characteristic morphological features reminiscent of Swiss cheese (55). However, numerical modeling of elastic networks shows that the size distribution of parenchymal holes is highly dependent on the degree of spatial correlation in the tissue destruction process (65). Random elimination of network links mimicking pure chemical dissolution of tissue produces a parenchymal pattern that looks rather different than CT images of emphysematous lung. On the other hand, if network links are preferentially eliminated on the basis of the mechanical forces they carry, the resulting pattern of holes is highly reminiscent of that seen in real diseased tissue. The patterns generated by the two approaches are shown in Fig. 4, A and B. While in both cases only ∼13% of the springs were eliminated, the resulting structures of the two networks are different. The random or chemical breakdown model shows a modestly heterogeneous structure, while the force-based approach leads to a very heterogeneous structure with a few giant defect clusters surrounded by many smaller ones. To compare these networks to the destruction patterns in a patient with advanced emphysema (Fig. 4C), we can measure the areas of the clusters in the network models and calculate the distribution of low tissue density clusters. In Fig. 4D, we compare these distributions, on a double logarithmic plot, to the size distribution of contiguous regions of low attenuation areas from the CT image. The random model provides a nearly uniform distribution of defect sizes, whereas the force-based model gives a distribution that is in quantitative agreement with the distribution obtained from the CT image. Moreover, the latter two distributions become almost perfectly straight when plotted on log-log axes. This indicates that the distributions are described by power laws, a feature that frequently arises in some form or other with regard to complex systems exhibiting emergent properties (61).

Fig. 4.

A: network simulation of random breakdown of alveolar walls (each line element is a spring). B: force-based breakdown. Note that high forces (red) occur around the perimeter of the clusters, especially in regions where a thin wall separates 2 clusters (arrow). C: color-coded CT image of an emphysema patient showing contiguous Low Attenuation Areas (LAAs) corresponding to regions of low tissue density. D: size distributions of defect clusters from A and B as well as the size distribution of LAA clusters from C plotted on log-log axes. [Reproduced with permission of the American Thoracic Society. Copyright American Thoracic Society (65).]

Thus the morphological patterns seen in diseased tissue are complex emergent expressions of how the disease develops at the cellular level in both time and space. Percolation is one example of a mechanistic concept that appears to be useful for understanding how pathologic alterations in micro-level and macro-level tissue properties may be linked. We have yet to see if percolation has any importance clinically, but one possibility is that histologic or radiologic analysis of lung parenchymal structure may help clinicians decide when a sudden downturn in functional status is imminent, allowing for an appropriate change in therapy or palliation. In particular, percolation highlights the importance of early detection of lung disease, since pathologic processes may already be well underway and in need of treatment before discernible clinical symptoms or abnormal tests of lung function become apparent. This indicates an increasingly important role for lung imaging, which has the potential to detect the early stages of parenchymal abnormality. More speculatively, percolation has been suggested as the basis for a targeted approach to tissue repair, the idea being that preferentially replacing percolating networks of diseased tissue with normal tissue might provide the fastest return toward normal function (66), although this awaits the development of the required reparative methodologies.

DYNAMIC BEHAVIOR OF LUNG TISSUE

So far we have considered lung tissue as a purely elastic material, one characterized by a single-valued relationship between stress and strain, albeit a somewhat complicated one. However, the lung is constantly changing its volume in life, so a complete understanding of lung tissue mechanics must include its dynamic behavior. The field of lung tissue dynamics received a major boost in the 1980s with the development of the alveolar capsule method for measuring alveolar pressure in living animals (15, 16). This technique made it possible to measure directly the pressures associated with the rate of change of strain of the lung parenchyma and firmly established the importance of lung tissue resistance as a key component of overall lung mechanics in both health and disease (35, 36). This built on the seminal work of Hildebrandt and colleagues (25–30) in the 1970s on the dynamic pressure-volume behavior of whole isolated lungs that showed lung tissue to exhibit a complex rheology describable by both viscoelastic and plastoelastic components. Subsequent measurements of the uniaxial dynamic stress-strain behavior of strips of degassed lung parenchyma confirmed that lung tissue alone, independent of the effects of surface tension, also exhibits complex nonlinear dynamic mechanical behavior (1, 9–12, 14, 31, 39, 44–46, 49–51, 67, 70–72).

As with the static behavior of lung tissue, understanding its dynamic behavior relies on the development of mathematical models that attempt to embody key underlying mechanisms. The first models were essentially phenomenologic, consisting of collections of idealized Hookean springs and Newtonian resistors (dashpots), each such pair being known as a Maxwell body (62). Although the springs and dashpots in these models cannot be identified with any particular structures within the tissue, they nevertheless embody the notion that energy is dissipated internally by various components sliding past each other and generating friction, while elastic energy is temporarily stored in the extension of elastic elements such as elastin fibers. Even a single linear Maxwell body gives a reasonably convincing overall rendition of the relaxation in tension that is seen when a strip of lung tissue is suddenly stretched and held at a new length or the relaxation in pressure that occurs when a lung is suddenly inflated to a new volume. Collections of Maxwell bodies with different time constants operating in parallel can model more complicated monotonically decreasing stress functions, but such a representation remains empirical.

In fact, the stress (s) in a parenchymal strip decays according to a simple power law of time (Fig. 1B). That is (6),

$$\text{s}\left(t\right)=\text{A}{t}^{-k}$$ (1)

where A is a constant and k is a positive exponent typically much less than 1. An equivalent phenomenon has been frequently observed in whole lungs in the form of a constant-phase mechanical input impedance (22), something that was preempted by earlier observations in rubber balloons (24). That is, if V̇(f) is the Fourier transform of a multi-frequency flow waveform applied to the lung and P_A(f) is the alveolar pressure relative to pleural pressure that is generated in the process, then the mechanical input impedance of the lung tissue, Z_t(f), is invariably found to be accurately described by

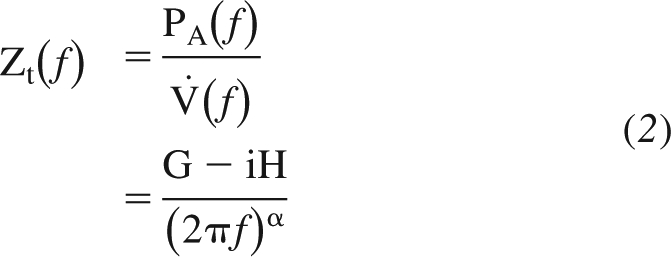where G and H characterize the dissipative and elastic properties of the tissue, respectively, and α is a constant (typically slightly less than, but close to, 1) that is a function of the ratio of G to H. From this equation it can be seen that the ratio of tissue viscance to tissue elastance (G/H) is a constant independent of the frequency of flow oscillation. The constancy of G/H (also known as hysteresivity) was first noted with respect to lung tissue in the so-called structural damping paradigm and taken to indicate that those elements that dissipate energy within the tissue are somehow intimately linked to those elements that store energy elastically (17).

The power-law behavior of lung tissue both in time and with frequency are intriguing. In fact, power-law rheological behavior has been identified in numerous biological soft tissues (19, 61), including cells (13). These observations compel us to search for some underlying physical explanation for why things should be this way. Indeed, power law processes abound in nature and a mechanism with the generality to match the phenomenon's ubiquity has yet to be identified. Nevertheless, it is clear that power law behavior cannot be attributed to a single element such as a specific molecule or fiber type, but rather must reflect some kind of complex emergent behavior. A number of specific mechanisms have been proposed to account for power law relaxation in soft tissue rheology, including a series of micro-breaks occurring at random locations throughout the tissue (4) and the tortuous movement of long polymer chains slowly untangling themselves (62). An interesting additional consideration is whether active processes within tissues (such as in actively contracting airway smooth muscle cells) also reflect qualitatively similar dynamics. In any case, while the genesis of power-law rheology remains controversial, it seems clear that a complete understanding of power-law rheology cannot be based on conventional lumped-parameter (i.e., spring-and-dashpot) models described by ordinary differential equations. Partial differential equations describing continuous parameter models are much more appropriate, and it has even been shown that the fractional calculus lends itself more naturally to the description of these phenomena (62).

The complexities of lung tissue do not stop there, however, because they are not only dynamic (having both elastic and dissipative components), they are also strongly nonlinear. Furthermore, of all the appallingly numerous forms that nonlinear dynamical behavior might take, nature seems to have chosen a rather special kind for lung tissue (and, indeed, for a number of other biological soft tissues). It turns out that the nonlinear and dynamic properties are, to a good approximation, separable, a phenomenon known as quasi-linear viscoelasticity (19). This means that the relaxation of stress in lung tissue following sudden steps in strain, x, is of the form

$$\text{s}\left(t\right)=\text{A}\left(x\right)t^{-k}$$ (3)

regardless of where along the highly nonlinear static stress-strain curve the strain steps are taken (Fig. 1B). In other words, all the nonlinear behavior in eq. 3 is wrapped up in the function A(x), while the time-dependent part, t^−k, remains linear. It is unclear as to why this curious state of affairs should pertain. One possible implication is that the physical processes giving rise to the static stress-strain behavior must be fundamentally different to those producing the stress relaxation behavior (3), although a model based on sequential micro-breaks throughout the tissue that has been shown to exhibit power-law stress relaxation also exhibits quasi-linear viscoelasticity (4). In any case, it is clear that the very particular dynamic mechanical behavior exhibited by lung tissue is some kind of emergent property that begs a rational explanation based on the ensemble behavior of its constituents.

We also note that tissue viscoelastic properties change in disease. For example, hysteresivity has been thought to reflect elementary dissipative properties of the tissue that are preserved across many conditions and species (17). However, remodeling of the tissue increases hysteresivity in both emphysema (9) and fibrosis in a way that correlates with the volume proportion of collagen (11). The physical basis of this phenomenon is not well understood.

WHY LUNG TISSUE IS COMPLEX

Lung tissue is clearly not a simple material, so we might ask if this is inevitable. Presumably a lung made of a network of pure elastin would suffice for the purposes of being able to recoil elastically during expiration, and this would certainly simplify its rheology. On the other hand, such an organ would probably be overly compliant from the perspective of the other tissues with which it interacts, especially the chest wall. An associated network of collagen is thus useful for matching the elastic recoil of the lung to its size. Collagen also provides a large measure of tensile strength as well as causing lung stiffness to increase substantially at high volumes, thereby protecting the delicate cells and blood vessels from rupture due to accidental overdistension. The bulk mechanical behavior exhibited by such an interwoven network of two mechanically different fiber types is some emergent combination of the individual networks and the way they interact. And, of course, we cannot stop there. The lung has to be home to the many cells required for tissue maintenance and gas exchange, but they require an environment more conducive than can be provided by elastin and collagen alone, so we must include proteoglycans and the water they bind that make an environment in which cells can thrive. The corresponding mechanical behavior of the system becomes affected by the extrusion of this aqueous ground substance through the protein fiber mesh as the tissue is stretched, a process that is dissipative and likely contributes significantly to the viscoelastic properties of lung tissue. Right away, however, we need surfactant molecules to mitigate the surface tension in the air-liquid interface that arises, with its concomitant effects on the P-V relationship of the lung.

The above line of reasoning can continue virtually indefinitely, with each step adding another player to the cast of characters and another layer of complexity due to the relationships that must be formed between the new player and all those already on the stage. In other words, there is no choice in the matter—lung tissue has to be a highly complex material because it has to fulfill so many disparate mechanical and biological requirements. Nevertheless, cutting through all this complexity are certain general mechanisms, such as sequential fiber recruitment and percolation, giving rise to emergent behavior irrespective of the precise details of the individual tissue components. Understanding how these mechanisms of emergence are operative constitutes true insight into the nature of lung tissue mechanics.

GRANTS

The authors acknowledge the financial support of the National Institutes of Health through NCRR-COBRE RR-15557, HL-76273, HL-75593, HL-87788, HL-90757, and HL-98976.