Nature of the Amyloid-β Monomer and the Monomer-Oligomer Equilibrium

Abstract

The monomer to oligomer transition initiates the aggregation and pathogenic transformation of Alzheimer amyloid-β (Aβ) peptide. However, the monomeric state of this aggregation-prone peptide has remained beyond the reach of most experimental techniques, and a quantitative understanding of this transition is yet to emerge. Here, we employ single-molecule level fluorescence tools to characterize the monomeric state and the monomer-oligomer transition at physiological concentrations in buffers mimicking the cerebrospinal fluid (CSF). Our measurements show that the monomer has a hydrodynamic radius of 0.9 ± 0.1 nm, which confirms the prediction made by some of the in silico studies. Surprisingly, at equilibrium, both Aβ₄₀ and Aβ₄₂ remain predominantly monomeric up to 3 μm, above which it forms large aggregates. This concentration is much higher than the estimated concentrations in the CSF of either normal or diseased brains. If Aβ oligomers are present in the CSF and are the key agents in Alzheimer pathology, as is generally believed, then these must be released in the CSF as preformed entities. Although the oligomers are thermodynamically unstable, we find that a large kinetic barrier, which is mostly entropic in origin, strongly impedes their dissociation. Thermodynamic principles therefore allow the development of a pharmacological agent that can catalytically convert metastable oligomers into nontoxic monomers.

Introduction

Alzheimer disease (AD)² is a degenerative brain disorder that is associated with the presence of extracellular aggregates of amyloid-β (Aβ) (1), which is an ∼4.5-kDa peptide containing 39–42 residues. Recent studies indicate that small soluble oligomers are key to Aβ toxicity (2–4). In the AD brain, both Aβ monomers and dimers have been isolated, and the dimers have been shown to impair synaptic plasticity in mouse hippocampal slices (5). In contrast, Aβ monomers have been shown to be devoid of neurotoxicity (5) and have in fact been suggested to be neuroprotective (6, 7). The monomer to oligomer transition is therefore not only the obligatory first event of aggregation, it is also the key event determining the transformation of a benign protein to a neurotoxic one.

We address this transition from a thermodynamic viewpoint: an aggregation-capable molecule should have a defined equilibrium between monomers and dimers (or oligomers), such that it is primarily monomeric below a certain concentration. Any oligomer-enriched solution prepared below such a concentration must be thermodynamically unstable and must dissociate to monomers at a given rate. To understand AD in terms of Aβ aggregation, we need to understand how this concentration compares with the in vivo concentrations of Aβ (which is estimated to be ≪1 μm) (8–11) and what the kinetics of Aβ oligomer dissociation is.

However, experiments probing the monomer to oligomer transition have been difficult to perform due to the low concentration at which this transition most likely occurs, and they have yielded rather confusing results. Some studies have found that Aβ is oligomeric and toxic even at 100 pm concentrations (12), whereas experiments focusing on larger aggregates found that they form only at much higher concentrations (13). Fluorescence resonance energy transfer experiments in dimethyl sulfoxide-water solution suggest that low molecular mass Aβ₄₀ exists as a dimer at least up to 10 μm (14), whereas NMR diffusion measurements suggest that it is primarily monomeric (15). It has been reported that Aβ₄₂ forms noncovalent, SDS-resistant species with apparent molecular masses of dimer, trimer, and/or tetramer (at a concentration of 200 μm in Tris buffer of pH 7.4) (16). Gel filtration analysis in physiological buffers indicates that the smallest Aβ₄₀ species co-elute at a position corresponding to an apparent molecular mass expected for a dimer (16). Photochemical oxidative cross-linking studies provide one of the most direct ways to probe the monomer-oligomer distribution in solution and suggest that monomer, dimer, trimer, and tetramers of Aβ₄₀ exist in a rapid equilibrium in a 20 μm solution (17). In contrast, the same method applied to Aβ₄₂ suggests that pentameric or hexameric aggregates are preferentially formed at a concentration of 1 μm (18). These inconsistencies among experiments may have resulted from the difference between the initial conditions, differences in buffer media, and inadequate time given to the system to reach equilibrium. Therefore, the nature of the monomer-oligomer equilibrium is far from clear and remains to be explored at physiologically relevant conditions.

Another important unknown is the conformation of the monomer itself. Because Aβ produces aggregates at the typical experimental concentrations (many micromolar) in physiological buffers, the structure of the monomer has remained speculative. It is thought that the monomer is mostly unstructured and folds into a hairpin shape when it aggregates (19). In silico studies have been helpful in probing the monomer, but they have suggested many different conformations, containing different fractions of secondary structural elements (20, 21). Ion mobility mass spectrometry is a promising new technique that has provided measures of monomer and oligomer sizes and their distributions in the vapor phase. It has shown the presence of monomers and oligomers in a high concentration (∼30 μm) Aβ solution (22) and has suggested that the monomer is a compact object (21, 23).

Because Aβ precipitates at a few μm concentration (13), investigation of the monomeric state requires tools that are sensitive at much lower concentrations. We have recently shown that fluorescence correlation spectroscopy (FCS) and time-correlated single-photon counting (TCSPC), operating at single-molecule sensitivity levels, can effectively interrogate Aβ aggregation at such low concentrations (24). Although a complete determination of the solution monomer structure may not be possible at present, structural parameters such as the hydrodynamic radius are accessible to measurements. These can help us verify the in silico predictions and can provide a basis for understanding the critical transition of the peptide to the toxic state. Here, we use three different approaches to measure independently the size evolution of the Aβ particles in solution as a function of concentration and time. FCS measures the translational diffusion of single-fluorescent particles and yields their hydrodynamic radii. TCSPC-based time-resolved anisotropy measurement probes the rotational diffusion of the particles and yields an independent measure of the hydrodynamic volume. Finally, size-exclusion-based filtration and dynamic light scattering (DLS) provides a gross but simple verification of these results.

EXPERIMENTAL PROCEDURES

Materials

Purified rhodamine-labeled Aβ₄₀ and Aβ₄₂ (labeled at the N terminus through a lysine) were purchased from rPeptide Inc. (San Jose, CA). The EDANS-labeled Aβ₄₀ was synthesized by using an automated solid phase peptide synthesizer (PS3; Protein Technologies, Tucson, AZ). The purity of the peptides was verified by HPLC (model UFLC; Shimadzu, Columbia, MD) with a C4 analytical column (Kromasil, Bohus, Sweden) and by MALDI-TOF mass spectrometry (model TOF SPEC 2E; Micromass, Manchester, England). Buffer salts were purchased from Sigma. All peptides were made in ACSF buffer (146 mm NaCl, 5.4 mm KCl, 1.8 mm CaCl₂, 0.8 mm MgSO₄, 0.4 mm KH₂PO₄, 5 mm dextrose, and 20 mm Na₂HPO₄, pH adjusted to 7.35).The 5-kDa cut-off filter was purchased from Millipore Amicon (Billerica, MA). Rhodamine-labeled Aβ₄₀ peptide concentrations were estimated by weighing and verified separately by the absorbance (molar extinction coefficient of free rhodamine is 61,500 m⁻¹cm⁻¹ at 550 nm) and fluorescence measurements with respect to a standard rhodamine B dye solution. The quantum efficiency of the free dye was found to be similar to the peptide-bound dye. For unlabeled peptides the concentrations were estimated using Tyr¹⁰ fluorescence.

Preparation of Filtrates

Aβ₄₀ and Aβ₄₂ solutions were prepared at 150 nm concentration and were passed through a 5-kDa filter. Each solution was filtered for 20 min at 2000 × g. This filtrate was collected and used for further FCS measurements.

FCS

FCS measurements were performed with a FCS instrument constructed in-house as described elsewhere (25). Briefly, the laser beam was focused in the sample volume using an apochromatic 60× water immersion objective with NA of 1.2 (Olympus; America Inc., Center Valley, PA). The emitted fluorescence was collected using the same objective and separated from the excitation beam by a dichroic mirror. A 25-μm core diameter optical fiber was used as a confocal pinhole to reject the out-of-focus fluorescence. The fluorescence was filtered by a suitable emission filter before being detected by a single-photon avalanche photodiode (APD) (PerkinElmer Life Sciences). The data were analyzed using a model with discrete diffusion components in Origin 7.5 software (OriginLab, Northampton, MA).

DLS

DLS experiments were performed on a DynaPro-MS800 instrument (Protein Solutions Inc.) that monitors the scattered light at 90° to the excitation. At least 50 measurements each of 5-s duration were performed for each sample. The solutions were filtered through 220-nm filters (Millex filter units; Millipore). Raw DLS data were analyzed with the software provided by the manufacturer. This yielded the distribution of hydrodynamic radii of particles in the solution.

Time-resolved Rotational Anisotropy

Anisotropy measurements for EDANS-labeled Aβ were carried out in a TCSPC apparatus described elsewhere (26). Briefly, the excitation wavelength of 300 nm was obtained by generating the third harmonic of the output of a mode-locked titanium: sapphire laser (model 375B; Spectra Physics, Mountain View, CA) which is pulse-picked at 4 MHz. The emission was passed through a rotatable polarizer and filtered by a colored glass filter (WG450) and by a monochromator set to 495 nm. A dilute scattering solution (a solution of milk powder) was prepared to measure the instrument response function of the entire detection system.

Anisotropy measurements for rhodamine-labeled Aβ were carried out using 130-fs pulses at 740 nm from a mode-locked titanium:sapphire laser (MIRA 900, Coherent, CA). The sample was excited by focusing a laser beam using a 20 ×, 0.75 NA Nikon objective lens mounted on a Nikon Eclipse microscope, TE300 (Japan). The epifluorescence signal was focused on a rotatable polarizer placed in front of a multichannel plate photo multiplier tube from Hamamatsu, (model R 3809U-50, Iwata City, Japan), which served as the detector for time-resolved measurements. Excitation light was blocked by a copper sulfate solution filter. All experiments were performed at room temperature. All anisotropy data were analyzed using multiexponential fitting routines using software developed by N. Periasamy.

RESULTS

Size of Aβ₄₀ and Aβ₄₂ at Low Concentrations (FCS Measurements)

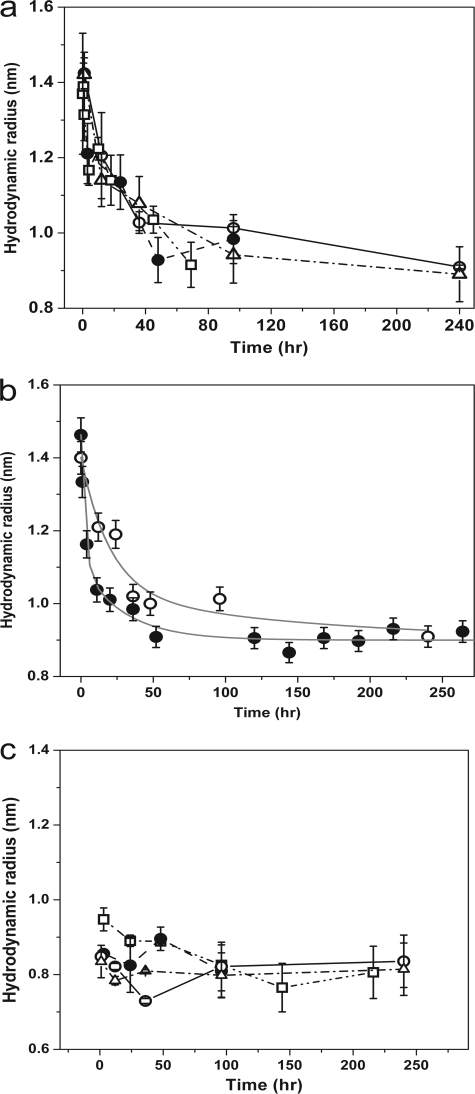
We prepared solutions of Aβ₄₀ and Aβ₄₂ (both 150 nm) in ACSF buffer at pH 7.4 (25 °C), diluting from a stock solution of 230 μm prepared in water at pH 10.5. The high solubility of Aβ in alkaline pH ensured that no fibrillar aggregates were formed at such high concentrations (27). Moreover, a 1500 × dilution from 230 μm to 150 nm ensured that the pH of the ACSF buffer remained close to pH 7.4. This particular concentration of 150 nm was chosen because previous shorter duration experiments have shown that Aβ₄₀ does not produce large multimers at such concentrations (24), and longer term experiments have shown that Aβ does not produce fibrils at submicromolar concentrations (28). We also note that this value was within the range of in vivo Aβ concentration (8–11). FCS measurements were carried out on these solutions at different times after dilution. The time of this dilution (and pH change) was taken as the initial time point (or “zero time”) of the measurement. The correlation data of these solutions were converted into hydrodynamic radii (R_h) using rhodamine B (R_h = 0.57 nm) (29) as a calibrant. A separate calibration using fluorescently labeled barstar (a 10-kDa protein of known structure) (30) resulted in a value within 10% of this measurement (data not shown). The calibrated size distribution (R_h) obtained is plotted as a function of time in Fig. 1a (Aβ₄₀ is shown as open circles, and Aβ₄₂ is shown as triangles). We see that the size distribution for both Aβ₄₀ and Aβ₄₂ started from about 1.4 nm and steadily decreased with time until they reached a steady value of about 0.9 ± 0.05 nm. The initial drop in the size was rapid, but it slowed down with time. This is presumably a signature of the approach to equilibrium and possibly signifies the dissociation of oligomers into monomers. We note that in principle, folding from an unfolded or extended state can also lead to a lowering of the average R_h. However unlike folding, dissociation rates can be expected to be concentration-dependent. To verify this, we followed this kinetics for a lower concentration (15 nm Aβ₄₀ at 25 °C; Fig. 1b, filled circles). We see that the size distribution started at 1.5 ± 0.1 nm and reached the same steady-state value of about 0.9 ± 0.05 nm. However, the observed rate at 15 nm was faster than that at 150 nm (Fig. 1b, open circles), indicating that the slow decrease of R_h is due to the dissociation of oligomers.

FIGURE 1.

Hydrodynamic radius measurements of Aβ at different conditions. a, hydrodynamic radii of rhodamine-labeled Aβ particles as a function of time after dilution to 150 nm: Aβ₄₀ at 25 °C (open circles), at 37 °C (filled circles), at 45 °C (squares); and Aβ₄₂ at 25 °C (triangles). b, hydrodynamic radii of rhodamine-labeled Aβ particles as a function of time at different concentrations: 150 nm (open circles), and 15 nm (filled circles). The gray lines are multiexponential fits, only meant for guiding the eye. c, hydrodynamic radii measured for the 5-kDa filtrate are shown for Aβ₄₀ at 25 °C (open circles), at 37 °C (filled circles), at 45 °C (squares); and for Aβ₄₂ at 25 °C (triangles).

We hypothesize that this equilibrium state with a 0.9-nm hydrodynamic radius is the Aβ monomer. To test this hypothesis, we used a membrane with a 5-kDa size cut-off to filter the solution at zero time. The concentration of the filtrate was about 12% of the initial concentration, and the pH of this solution remained within 0.1 pH unit of 7.4. The filtrate obtained at any time after preparation is expected to be mostly monomeric (because Aβ has a molecular mass of ∼4.5 kDa) and should have a size close to 0.9 nm, if our hypothesis is correct. The size determined from the FCS experiments of the filtrate obtained at different time is plotted in Fig. 1c (Aβ₄₀ is shown as open circles, and Aβ₄₂ is shown as triangles). The size is 0.85 ± 0.05 nm and is thus similar to the value obtained earlier. This supports our hypothesis that the oligomeric solution approaches a monomeric state after 10 days of incubation.

Size of Aβ₄₀ (Time-resolved Anisotropy Experiments)

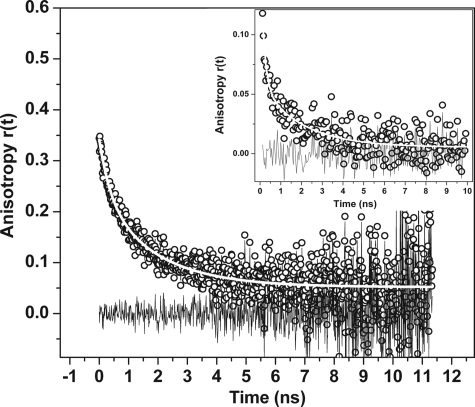
We then measured the size of the equilibrium state of Aβ₄₀ by using an independent technique, namely, time-resolved fluorescence anisotropy. Fluorescence anisotropy decay measures the rotational correlation time, which is a measure of the volume of the particle. Fig. 2 shows the anisotropy data collected from rhodamine-labeled Aβ₄₀. The data fit to a biexponential decay model. The longer component of the rotational correlation time was 1.9 ± 0.2 ns, and the relative fraction of this component was 65 ± 10%. The shorter component was 0.4 ± 0.2 ns. The short component likely resulted from the local motion of the dye, whereas the longer one was due to the global rotation of the protein molecule. Lifetime of rhodamine-labeled Aβ₄₀ in aqueous solution is about 1–2 ns (31). This is very close to the longer rotational correlation time, as a result of which the anisotropy data become noisy at this time scale. To avoid this problem we separately labeled Aβ₄₀ with the dye EDANS. This fluorophore has a longer lifetime (average lifetime ∼13 ± 0.5 ns; data not shown). The anisotropy experiment was repeated with this specimen to verify the results obtained earlier. Rotational anisotropy values obtained from a 1 μm EDANS-labeled Aβ₄₀ sample is plotted as a function of time in Fig. 2 (inset). The signal to noise ratio for the data at long times (>1 ns) was better than that of the rhodamine-labeled sample. These data were fitted to a biexponential decay model as before. The shorter component was 0.2 ± 0.08 ns (83 ± 10%) which is likely due to the local motion of the dye. The longer component was 1.7 ± 0.3 ns (17 ± 10%) and was consistent with the rotational correlation data obtained earlier from rhodamine labeled Aβ₄₀.

FIGURE 2.

Time-resolved rotational anisotropy of 1 μm rhodamine-labeled Aβ₄₀: data (black circles), biexponential decay fit (white line), and residuals (black line). Inset, rotational anisotropy measurement of EDANS-labeled Aβ₄₀: data (black circles), biexponential decay fit (white line), and residuals (black line).

Equilibrium Size of Aβ₄₀ Solution as a Function of Concentration

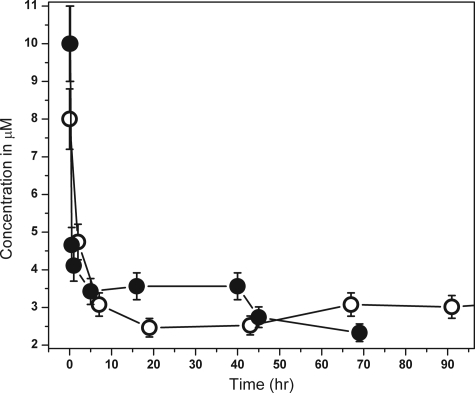
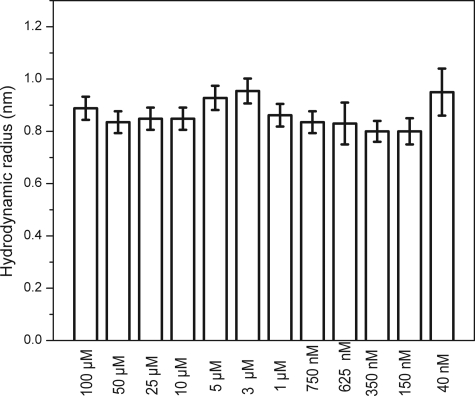
We then ascertained the maximum concentration up to which Aβ₄₀ remains monomeric. We determined the size of Aβ₄₀ at 12 different concentrations, viz. 100 μm, 50 μm, 25 μm, 10 μm, 5 μm, 3 μm, 1 μm, 750 nm, 625 nm, 350 nm, 150 nm, and 40 nm. The specimens were incubated for 7 days at 25 °C. The solutions were then centrifuged at 2000 × g for 20 min, and the supernatant was probed with FCS. The saturation concentration of Aβ₄₀ was measured to be about 3 μm (see Fig. 4 later). This implies that the supernatant at long times in all supersaturated solutions approached 3 μm. Therefore, the experiments at 100 μm, 50 μm, 25 μm, 10 μm, 5 μm, and 3 μm were essentially repeats of the same experiment. For the larger concentrations (>3 μm) the solution was a mixture of labeled and unlabeled Aβ at a ratio of 1:1000. The size distributions obtained from these solutions are shown in Fig. 3. The size reported by FCS at long time (∼7 days) remained close to 0.9 nm for all concentration. At all concentrations above the saturation concentration (3 μm), larger aggregates did form, but they grew and precipitated out well within the time scale of our observation. The soluble particles that remained in equilibrium with the larger precipitates were predominantly monomeric. We separately verified that the saturation concentration of the unlabeled protein was very similar to that of the labeled protein and was about 3 μm (Fig. 4, filled and open circles, respectively).

FIGURE 4.

Saturation concentration determination for Aβ₄₀ and rhodamine-labeled Aβ₄₀. Concentration of Aβ₄₀ remaining in solution is plotted as a function of time for: labeled Aβ₄₀ (open circles) and unlabeled Aβ₄₀ (filled circles).

FIGURE 3.

Hydrodynamic radii of the soluble fraction of Aβ₄₀ as a function of concentration after 7 days of incubation.

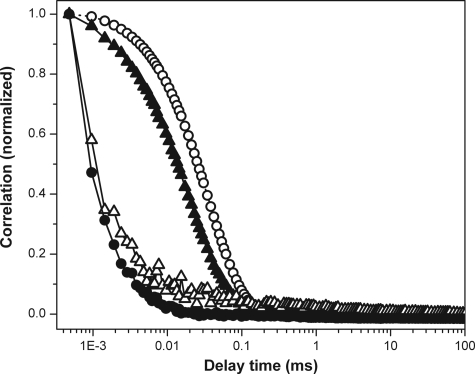
To confirm these observations further, we performed DLS measurements of the supernatants at two different concentrations (100 μm and 10 μm) of Aβ. The correlation curve obtained from the supernatant of the 100 μm Aβ solution is shown in Fig. 5 (open triangles). The correlation obtained from this solution is similar to that from the buffer solution alone (Fig. 5, filled circles). The 10 μm Aβ data are also similar (data not shown). Two standard proteins, barstar and bovine serum albumin (BSA) (both of them diluted to obtain the same scattering strengths as that of Aβ), were also measured for comparison (Fig. 5, filled triangles and open circles, respectively). The correlation traces obtained for barstar and BSA are distinct from the buffer, and they fit to the expected R_h values of 2.1 ± 0.35 and 3.9 ± 0.1 nm, respectively.

FIGURE 5.

DLS correlation functions. Aβ supernatant (open triangles), barstar (filled triangles), bovine serum albumin (open circles) and buffer (filled circles).

Kinetics of the Oligomer to Monomer Transition

The evolution of the size of Aβ₄₀ oligomers as a function of time showed that there was a slow transition from the oligomeric state to the monomeric state at 150 nm concentration (Fig. 1a). The slow kinetics indicated an activation barrier for the dissociation process. To probe this further, we carried out the size determination experiment for Aβ₄₀ at two additional temperatures, 37 °C and 45 °C. This is shown in Fig. 1a (filled circles for 37 °C and squares for 45 °C). For each of these cases the size distribution started from an oligomeric state with a size ranging from 1.2 to 1.4 nm, and it gradually came down to 0.9 ± 0.05 nm. This is very close to the size obtained for the monomers (using cut-off filters) at all the three different temperatures (Fig. 1c, open circles for 25 °C, filled circles for 37 °C and squares for 45 °C). Within the experimental errors, there was no significant difference in the rate of approach to the monomeric state between these different temperatures.

DISCUSSION

Size of the Aβ Monomer

We found that the size of preformed rhodamine-labeled Aβ oligomers at physiological concentrations gradually decreased with time and attained a constant value of 0.9 ± 0.05 nm (Fig. 1a, open circles for Aβ₄₀ and triangles for Aβ₄₂). This can happen either due to the dissociation of oligomers into smaller structures or due to a change of the peptide conformation. The rate is expected to be concentration-dependent for a dissociative process, and we indeed observed it to be faster for a lower concentration (15 nm) solution (Fig. 1b, filled circles). However, the size of Aβ particles at both the concentrations reached a constant value of 0.9 ± 0.05 nm. This value of R_h did not change further with time, indicating the attainment of an equilibrium state for Aβ.

Because the molecular shape is unknown, FCS by itself cannot establish the identity of the 0.9-nm sized particle. We hypothesize this species to be the monomer, and we tested this hypothesis by employing a 5-kDa cut-off filter. Although the pore size of such filters has a distribution, the expectation is that the filtrate would be mostly rich in monomers (because Aβ is an ∼4.5-kDa molecule). FCS measurements of the filtrate fit well to a single-component diffusion model. The hydrodynamic size of this filtrate was also found to be 0.85 ± 0.05 nm (Fig. 1c, open circles for Aβ₄₀ and triangles for Aβ₄₂). This value remained unchanged for at least 10 days. We note that in a minority of experimental repeats a two-component diffusion model analysis of the same data showed the presence of larger particles with particle size ranging from 2 to 8 nm, which possibly represented the population that leaked through the nominal 5-kDa pore size. However, the relative amplitude of this larger species did not exceed 12% in any of the measurements. Because the relative FCS amplitude of particles depended on the square of their respective brightness, this small amplitude for the larger particles showed that the filtrate solution was composed almost exclusively of stable monomers which have an R_h value of 0.85 ± 0.05 nm. This is similar to the equilibrium value that the unfiltered oligomers eventually reach after several days.

To have an independent measurement of the size of this equilibrium particle, we probed the system with fluorescence anisotropy decay measurements. This measures the rotational correlation time of a molecule and is proportional to its volume (if the particle is spherical). Rotational anisotropy data of rhodamine-labeled Aβ₄₀ fit well to a two-component decay model (Fig. 2). The shorter component was 0.4 ± 0.2 ns. This short time scale contribution is likely to be the result of the local rotation of the dye. The longer rotational correlation time fit to a value of 1.9 ± 0.3 ns with an amplitude of 65 ± 10% and was likely due to the global motion of the molecule. However, because the rotational correlation time (1.9 ns) is very close to the lifetime of rhodamine (1–2 ns), fitting of the data in the longer time window became somewhat unreliable. To overcome this problem, we investigated Aβ molecules labeled with EDANS. Rotational anisotropy data from this sample again fit to a biexponential decay. The shorter component was 0.2 ± 0.1 ns with an amplitude of 83 ± 10%. This is likely to be due to the local rotation of EDANS which is placed at the C terminus of the Aβ molecule. In fact, the rotational correlation time for the free dye IAEDANS is reported to be ∼ 0.2–0.3 ns (32). The short component and its amplitude were slightly different from those obtained earlier for the rhodamine-labeled peptide, but we note that the two dyes were different and were placed at different termini of the peptide. The longer component was determined by the overall rotation of the peptide and was expected to be independent of the identity of the dye or its placement in the peptide. Indeed, the longer rotational component of the EDANS-labeled peptide was 1.7 ± 0.3 ns and was similar to the value obtained from the rhodamine-labeled Aβ. This helped us to determine the size of the peptide. Rotational anisotropy measurements of the native monomeric state of a model protein barstar (a compact quasispherical protein with a molecular mass of 10 kDa) labeled with EDANS at the C terminus yielded a rotational correlation time of around 3.5 ns (33). We used this as a calibrant. Under a spherical shape assumption, the barstar measurement would predict a rotational correlation time of 1.6 ns for an Aβ monomer of 4.5-kDa size. This is close to the rotational correlation time obtained from both rhodamine-labeled and EDANS-labeled Aβ, indicating that it is in a compact monomeric state.

We note that it is in principle possible that the aggregation properties of the rhodamine-labeled peptide are different from those of the naturally occurring peptide. We have investigated this potential difference by measuring the saturation concentration of both the labeled and the unlabeled peptides (Fig. 4, open circles and filled circles, respectively). The saturation concentrations are similar for both, suggesting that the aggregation properties are not grossly affected by fluorescent labeling. Also, it can be asked whether the ACSF used by us is an adequate mimic for the actual CSF. We note that a short duration experiment using 10 nm Aβ showed that initial ∼1.5-nm sized Aβ particles introduced in mouse CSF evolved toward smaller-sized values.³ This is consistent with the results shown here.

Recently, several in silico studies have been performed with Aβ₄₀ and its related fragments, and our size determination helped us benchmark these in silico studies. According to Raffa et al., the radius of gyration of Aβ₄₀ and Aβ₄₂ are both ∼0.9 nm (34), whereas Baumketner et al. has suggested that the radius of gyration is ∼1 nm (21). Several simulation studies of different fragments of Aβ have reported radius of gyration between 0.8 and 1.2 nm for Aβ₄₂ (35, 36). However, there are also studies that find the radius to be larger (20). Ion mobility mass spectrometry typically yields a mixture of sizes for the monomer, but it is interesting to note that the smallest of the sizes reported is about 1 nm (21), which is consistent with the results obtained here. We note that studies of Aβ in HFIP, which is believed to keep Aβ monomeric even at high concentration, have suggested a more compact conformation for it (37, 38).

Monomer-Oligomer Transition

Next, we investigated the concentration at which chemical equilibrium starts favoring oligomers to monomers. Solubility for Aβ₄₀ in ACSF buffer at pH 7.4 and 25 °C is 3 μm (Fig. 4). Above this concentration, large aggregates form spontaneously, and they gradually precipitate out of the solution, eventually forming amyloid fibrils (28). Monomers, oligomers, and larger particles can in principle co-exist at this limiting concentration. It is interesting to note that the species that remains in the solution after a long time is nearly monomeric at all concentrations. At concentrations larger than the saturation concentration (∼3 μm in this buffer) larger aggregates do form, but they eventually precipitate out. This is consistent with our earlier observations of Aβ nucleation (28, 39). However, when saturated Aβ solutions are observed within ∼1 day of preparation, higher sized oligomers are observed (28). This indicates that the oligomers are at best quasistable species even in the presence of Aβ precipitates. This suggests that the energy of nucleation is high, and the free energy is a relatively steep function of the aggregate size for subnuclear particles (28, 40). We note that a similar absence of small oligomers, despite the presence of monomers and large aggregates (∼100 nm), has also been observed for another amyloidogenic protein, IAPP (41).

These observations were further confirmed by the DLS measurements. DLS is a robust technique for measuring solute hydrodynamic radii in equilibrium solutions. In our experiments barstar and BSA were used as standards at a dilution level where the scattering signal intensity becomes similar to that obtained from the Aβ solution. It is clear that the resolution of the instrument does not permit a measurement of R_h of Aβ, although it is adequate for measuring the R_h of BSA (3.9 nm) and even barstar (2.1 nm). This implies that the R_h of Aβ peptide in the solution is <2.1 nm. This provides a measure of the upper boundary of the Aβ particle sizes and supports the conclusion derived from the FCS measurements.

Interestingly, the concentration of 3 μm is at least an order of magnitude higher than the estimated in vivo concentrations (8–11), suggesting that Aβ should be monomeric in vivo. However, the dominant hypothesis regarding the etiology of AD hinges on the existence of oligomers in vivo (2, 42, 43). Indeed, several experiments have found oligomers in the CSF of AD patients (44, 45). These oligomers must originate under special conditions and/or in localized compartments. The cell membrane is a possible location for the formation of these amyloid oligomers. In fact, recently we have shown that at physiological concentrations, large aggregates of Aβ form spontaneously in the membrane, but not in the extracellular solution (24). Although this may explain the production of Aβ oligomers at low in vivo concentrations, such oligomers, once released in the CSF, should be unstable. This is where our finding regarding the exceptional stability of the oligomers becomes significant. Our experiments show that the preformed oligomers at such low concentrations do convert back to the monomers, but they take days to do so (Fig. 1a). Surprisingly, this rate remains about the same at the physiological temperature of 37 °C, and at an even higher temperature of 45 °C (Fig. 1a). This indicates the presence of a relatively large entropic barrier with a relatively low enthalpic barrier separating the monomeric and the oligomeric states of Aβ₄₀. The monomers are possibly entangled in the initial oligomeric state, with multiple intra- and intermolecular hydrophobic interactions aiding the entanglement. If the change of enthalpy is small, this free energy change will be dominated by the entropic part. Hence, the rates of dissociation will be slow, yet largely independent of temperature, as is observed here. If this mechanism remains true in vivo, then any Aβ oligomer that gets produced in a specialized environment, such as in the cell membrane, would be stable enough in CSF to cause damage to neurons at a distal location (assuming that the soluble oligomers are indeed the key to toxicity, as is now commonly believed) (2).

There is considerable effort toward developing agents that can reduce the oligomer concentration. Significantly, our results show that the oligomers at physiological concentrations are in a metastable state. Therefore, in principle, it is possible to develop a potent pharmaceutical agent that can catalytically convert these oligomers into benign monomers and provide effective therapy at nonstoichiometric doses.