Elastic Modulus Determination of Normal and Glaucomatous Human Trabecular Meshwork

This study determined that the mean elastic modulus was substantially increased in glaucomatous human trabecular meshwork (HTM) when compared with normal HTM. This finding is the first demonstration of a biophysical change in the trabecular meshwork in association with glaucoma.

Abstract

Purpose.

Elevated intraocular pressure (IOP) is a risk factor for glaucoma. The principal outflow pathway for aqueous humor in the human eye is through the trabecular meshwork (HTM) and Schlemm's canal (SC). The junction between the HTM and SC is thought to have a significant role in the regulation of IOP. A possible mechanism for the increased resistance to flow in glaucomatous eyes is an increase in stiffness (increased elastic modulus) of the HTM. In this study, the stiffness of the HTM in normal and glaucomatous tissue was compared, and a mathematical model was developed to predict the impact of changes in stiffness of the juxtacanalicular layer of HTM on flow dynamics through this region.

Methods.

Atomic force microscopy (AFM) was used to measure the elastic modulus of normal and glaucomatous HTM. According to these results, a model was developed that simulated the juxtacanalicular layer of the HTM as a flexible membrane with embedded pores.

Results.

The mean elastic modulus increased substantially in the glaucomatous HTM (mean = 80.8 kPa) compared with that in the normal HTM (mean = 4.0 kPa). Regional variation was identified across the glaucomatous HTM, possibly corresponding to the disease state. Mathematical modeling suggested an increased flow resistance with increasing HTM modulus.

Conclusions.

The data indicate that the stiffness of glaucomatous HTM is significantly increased compared with that of normal HTM. Modeling exercises support substantial impairment in outflow facility with increased HTM stiffness. Alterations in the biophysical attributes of the HTM may participate directly in the onset and progression of glaucoma.

Glaucoma is the leading cause of irreversible blindness, affecting nearly 70 million people worldwide. The only known treatable risk factor for glaucoma is the elevated intraocular pressure (IOP) caused by an increased resistance in aqueous humor outflow. Elevated IOP causes the trabecular meshwork (HTM) to dilate through expansion of the spaces in the inner meshwork,¹ implicating the juxtacanalicular region (JCT) of the HTM at Schlemm's canal (SC) as the principal site of outflow resistance. Analysis of the JCT/SC reveals a region rich in extracellular matrix (ECM) components.^2,3 Excess synthesis of laminin, collagen IV, cross-linked fibronectin, tissue transglutaminase (a cross-linking enzyme), and protein adducts caused by lipid oxidation have been identified in glaucomatous meshworks, suggesting that these changes contribute to outflow resistance.⁴ Studies have also correlated an increase in outflow resistance with elevated glycosaminoglycan (GAG) expression in HTM cells, which results in a shift in the ratio of chondroitin sulfate to hyaluronic acid.⁵ We have identified multiple splice variants of chondroitin sulfate-substituted versican in HTM cells, which is thought to play a role in regulating IOP and have suggested that changes in the ratios of these spliced forms are related to reduced outflow. Thus, while the causes of glaucoma are not known or understood, changes in the HTM ECM and basement membrane are consistently reported with disease. Biophysical cues related to substrate topography have been shown to affect HTM cellular behavior.⁶ Nanoscale to submicrometer topographic features influence HTM cell alignment, migration, and gene and protein expression. In addition to sensing substrate topography, cells sense and respond to the stiffness (typically expressed in terms of elastic modulus) of the underlying substrate.^7–9 Fibroblasts and osteoblasts change their stiffness by cytoskeletal reorganization to adapt to changes in substrate modulus.^10,11 Substrate modulus also affects cell alignment, migration, proliferation, and differentiation.^9,12–20 A change in substrate modulus is thought to have a role in disease development and has been implicated in vascular and muscle disease,^8,13,21–24 osteoarthritis,²⁵ liver fibrosis,^26,27 and tumor cell migration.²⁸

Atomic force microscopy (AFM) has been useful in imaging and characterizing soft biological materials.²⁹ The force sensitivity and the spatial resolution available with AFM nanoindentation allow measurement of the local elastic modulus of soft biological materials and investigation of sample heterogeneities across small size scales. AFM has been successfully used for determining the mechanical properties of many tissues and cells.^29–41 We hypothesized that changes in the ECM observed in glaucoma results in an increase in local stiffness (increase in modulus) of the tissue and that this increase, in turn, decreases outflow facility from the eye. Using AFM, we found the modulus of the HTM to be substantially increased in glaucomatous globes, and modeling exercises suggest that this increase in modulus directly results in a decrease in aqueous outflow facility.

Methods

Sample Preparation

Human donor eyes or corneal buttons determined unsuitable for transplantation were obtained from several eye banks. The donor eyes were managed in compliance with the tenets of the Declaration of Helsinki for research involving human tissue. In general, the glaucomatous samples were obtained without detailed information on the type of glaucoma, the extent of disease, or the medications used for treatment. Information with regard to the type of glaucoma was provided with only 3 of the 10 glaucoma samples, each with a diagnosis of primary open-angle glaucoma. The ages of the donors ranged from 32 to 92 years. The tissues were maintained in corneal storage medium (Optisol; Chiron Ophthalmics Irvine, CA) at 4°C before they were dissected to remove the HTM. The samples were prepared by dissection of the iris and uveal tissue with an ophthalmic knife (Alcon Surgical, Fort Worth, TX). The HTM was sectioned with a razor blade to provide samples less than 1 cm in length, and the sections were removed from the angle with forceps. The tissue was oriented so that the SC side of the JCT was probed and was affixed in the trabecular region with cyanoacrylate glue in the center of a stainless-steel AFM disc. AFM analysis was performed in 1× phosphate-buffered saline (PBS). The average time from donation to analysis was 17.5 ± 10.7 days for normal and 7.4 ± 4.2 days for glaucomatous samples (Table 1).

Table 1.

Donor Age and Elastic Modulus for Normal and Glaucomatous Samples

Donor Age (y)	Days from Donation to Measurement	E ± SD Normal (kPa)	Range of E (kPa)	n	Donor Age (y)	Days from Donation to Measurement	E ± SD Glaucomatous (kPa)	Range of E (kPa)	n
32	NA	4.8 ± 2.4	1.3–7.6	5	72	6	102.6 ± 111.4	1.4–329.7	19
39	27	3.4 ± 4.7	0.6–16	10	76*	13	122.4 ± 111.5	36.4–382.8	8
56	30	1.9 ± 1.8	0.7–6.7	10	76*	13	138.4 ± 148.0	1.7–565.3	34
61	NA	1.7 ± 0.9	0.5–3.4	7	79	5	76.8 ± 124.4	0.8–552.0	22
69	17	2.1 ± 1.9	0.5–9.3	24	82	7	65.7 ± 33.5	23.2–126.6	14
70	30	3.2 ± 1.9	1.0–7.0	21	85	16	29.6 ± 52.0	0.5–206.9	24
77	13	4.0 ± 2.2	1.8–6.1	3	87	4	70.2 ± 121.3	2.0–243.0	25
78	15	6.5 ± 2.9	1.6–10.0	7	87*	11	78.5 ± 80.5	1.3–315.8	28
83	4	8.8 ± 2.4	4.4–8.8	13	92	4	73.2 ± 71.8	5.3–178.5	5
83	4	3.5 ± 1.3	1.6–5.4	13	92	4	50.4 ± 53.0	1.5–142.5	9

The time from donation to measurement indicates the length of time the samples were stored in cornea preservative. n, the number of measurements taken on each donor sample.

^*Donors with primary open-angle glaucoma.

To characterize the region of tissue investigated with the AFM, we processed multiple normal corneal buttons for histopathology at various stages of preparation. Donor tissues were processed for routine histology as wedges of intact limbus, wedges of limbus after sharp dissection of uveal tissue, isolated HTM (longitudinal and cross section), and wedges of limbus after HTM isolation. All samples were fixed for 24 to 48 hours and processed routinely, and sections of isolated HTM were oriented for either longitudinal or cross-sectional sampling. After they were embedded, the samples were sectioned at 4 μm and stained with hematoxylin and eosin.

Instrumentation

Force curves were acquired with a scanning probe microscope (Nanoscope IIIa Multimode; Veeco Instruments, Inc., Santa Barbara, CA). The samples were transferred to the AFM without drying and placed in a commercially available liquid cell (Veeco Instruments, Inc.). Silicon nitride cantilevers with a borosilicate sphere as the tip (1 μm radius; Novascan Technologies, Inc., Ames, IA) were used to sample a large area of the HTM. The nominal spring constant of the cantilevers was 0.06 N/m. Force curves were obtained on at least 10 different locations, at either random locations on the sample or in a line with each measurement location separated by approximately 50 μm. Data exhibiting nonlinear behavior or a large adhesion with the surface were not included in the analysis, and a minimum of three locations were used to calculate the mean elastic modulus. In addition, when data were acquired at random locations, a minimum of three force curves were obtained at each location. Each force curve was taken at a rate of 2 μm/s.

Data Analysis

The force curves were analyzed with the Hertz model for a sphere in contact with a flat surface, by force curve analysis software (PUNIAS; Protein Unfolding and Nano-Indentation Analysis Software, http://punias.voila.net/). To obtain an accurate modulus, the optical sensitivity and the spring constant of each cantilever was determined. Optical sensitivity was measured as the slope of the force curve, taken in PBS, when the tip was in contact with a rigid surface. The optical sensitivity was used to convert cantilever deflection in volts to deflection in nanometers (x). Spring constants (k) were measured using Sader's method.⁴² The force was determined by F = kx. The Hertz model provides a relationship between the loading force and the indentation, which for a spherical indenter is

where F is the loading force in Newtons, ν is Poisson's ratio (assumed to be 0.5), δ is the indentation depth, E is the elastic modulus in Pascals, and R is the radius of the tip. The values obtained from the force curve are z, z₀, d, and d_0, where z is the piezo displacement, d is the cantilever deflection, and z₀ and d₀ are the values at initial contact of the tip with the sample. These values can be used to calculate the indentation, which is given by

Using these equations and knowing that F = k(d − d₀), where k is the cantilever spring constant, gives an equation for E

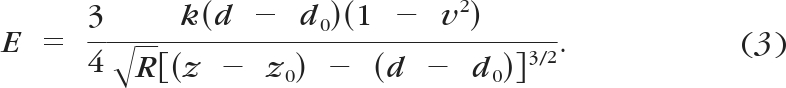Models to Describe Flow

In the combined biomechanical and biofluidic model, the HTM is considered to be an elastic, porous membrane (36 mm in length, 0.3 mm in width, and 50 μm in thickness) with parallel cylindrical micropores embedded (with the original porous diameter of 1 μm and the porosity of 350/mm² chosen, to be within the range reported in the literature⁴³). The IOP causes the HTM to bow outward, which leads to the enlargement of the pore size. The size of micropore is a function of the elastic modulus of HTM (E) and aqueous outflow rate (Q), which can be derived from the following equations. Taking the HTM as a thin, elastic, porous membrane, the assumption of a thin plate with small deflections is applied, in which the normal stresses transverse to the plate (HTM) are disregarded.⁴⁴ Under the external pressure difference, the HTM (of length l, width w, and thickness t) deforms into a spherical shape with a central angle of 2α. The deflection of the HTM membrane can be related to the IOP (ΔP) acting on the HTM and the elastic modulus. According to Laplace's equation, the relationship among IOP, geometrical parameters, and material properties of the HTM can be expressed as

where T represents internal tension parallel to the HTM and the length of the HTM is much greater than the width. Furthermore, the internal tension can be derived from the strain–stress relationship, which leads to the governing equation

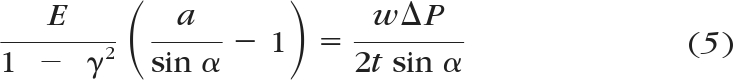where γ is Poisson's ratio. As can be seen, the central angle is a function of the IOP and the elastic modulus of the HTM. Eventually, the overall dynamic fluidic resistance of the HTM membrane can be calculated as

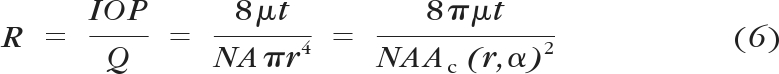where the aqueous outflow rate (Q) ranges from 2 to 3 μL/min and μ is the aqueous viscosity. Other structural parameters include the overall area and thickness of JCT (A and t), the porous density (N), as well as the area of an individual micropore (A_c), which can be calculated from the original pore size and the central angle of the HTM. Thus, the “effective” flow pathway leads to t/NA, or 0.013 μm, as modeled in this article.

Results

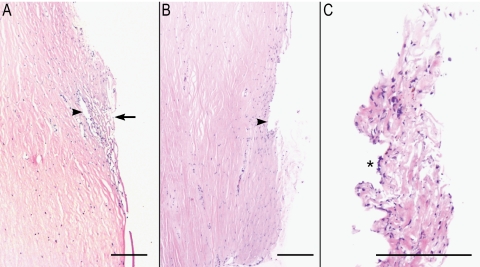
Histologic examination of donor corneal buttons confirmed the presence of HTM and SC tissue in all the buttons examined (Fig. 1A). Tissues removed via sharp dissection were largely ciliary body muscle and pigmented uvea. Isolated HTM was difficult to orient precisely for histology, but all samples were confirmed to be composed predominantly of HTM beams and cells, with variable, but typically small, amounts of scleral collagen, rare melanocytes and, in one case, remnants of Descemet's membrane. The inner wall of SC was multifocally/segmentally absent from the postisolation corneal buttons (Fig. 1B). HTM tissue was identified in well-oriented regions of isolated HTM tissue, and the inner wall of SC was identified in some sections (Fig. 1C).

Figure 1.

Histology of HTM throughout the isolation process. (A) Section of a donor cornea with HTM in situ. Arrow: the inner aspect of the HTM. Arrowhead: the outer wall of SC. (B) Donor cornea after removal of the HTM. Arrowhead: the outer wall of SC. (C) Isolated HTM after removal. The tissue is somewhat compressed (artifact). () The inner wall of SC, the surface at which elastic modulus measurements were made. H&E; Magnification (A, B) ×4; (C) ×10; scale bar, 200 μm.

AFM force curves, a plot of cantilever deflection versus z piezo movement, were obtained on HTM from normal and glaucomatous donors. The force curves obtained on these tissues, taken from the internal surface of SC, had the same characteristics as those obtained on other soft, elastic materials.^45,46 In general, the force curves consisted of a straight-line approach when the tip was still away from the surface. As the tip came into contact with the surface, there was a gradual increase in the deflection of the cantilever, as expected for soft samples. Large pull-off forces were not typically observed as the tip was retracted from the surface, indicating negligible adhesion of the tip with the HTM. The approach and retract curves overlapped, indicating an absence of viscoelastic effects at the indentation rate used (1 Hz).

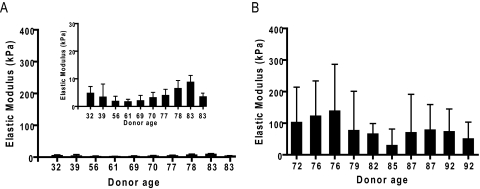
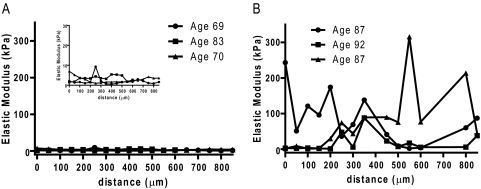
Measurement of the elastic modulus in normal HTM (n = 10; mean age, 65 years; range, 32–83 years) ranged from 1.7 to 8.8 kPa (mean, 4.0 ± 2.2 kPa; Table 1, Fig. 2). In comparison, glaucomatous HTM (n = 10; mean age, 84 years; range, 72–92 years) had a significant increase in elastic modulus ranging from 29.6 to 138.4 kPa (mean, 80.8 ± 32.5 kPa; P < 0.0001; Fig. 2). Analysis of 50-μm intervals along the glaucomatous HTM revealed large variations ranging from less than 10 kPa to greater than 200 kPa. In contrast, minimal variations in elastic modulus were identified in normal HTM, with all values less than 15 kPa (Fig. 3).

Figure 2.

HTM elastic modulus versus donor age. AFM nanoindentation was used to determine the mean elastic modulus for (A) normal (inset: data plotted on a smaller y scale) and (B) glaucomatous HTM samples.

Figure 3.

Force curve measurements taken every 50 μm on (A) normal (inset: data plotted on smaller y scale) and (B) glaucomatous HTM. Elastic modulus of the HTM every 50 μm along the tissue show little variation in normal HTM but a wide variation in glaucomatous HTM.

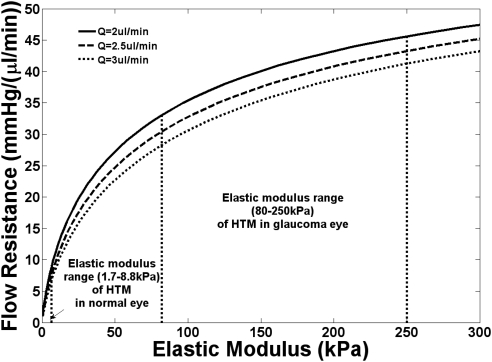
We used a mathematical model to determine whether the change in modulus between normal and glaucomatous HTM might influence the facility of aqueous outflow. A mathematical model has been established to evaluate the impact of modulating intrinsic stiffness on outflow resistance. In this model, we consider the HTM as an elastic porous membrane with conjugated biomechanical and biofluidic responses. Figure 4 shows that the increase in HTM stiffness increased the flow resistance of the JCT. This increase was due to a pressure elevation across the JCT/SC layer that enlarged the pore size, which was inversely proportional to the fourth power of the outflow resistance. As a consequence, the pathologically increased stiffness of the HTM in glaucomatous eyes led to considerably higher outflow resistance than that in the normal control group. Our model showed a similar trend of the outflow facility's dependence on IOP/flow rate variation, as predicted by previous biomechanical models.⁴⁷ While this model is one of several that could be applied, in all conventional models, a change in facility would be substantially influenced by the changes in elastic modulus measured between the normal and diseased HTM.

Figure 4.

Results from a mathematical modeling of a membrane with 1-μm pores showing the change in flow resistance with increasing elastic modulus. The curves were plotted for three different flows of liquid through the pores. These flow rates are similar to those reported for aqueous humor outflow. The model demonstrates that there is an increase in flow resistance with increasing elastic modulus.

Discussion

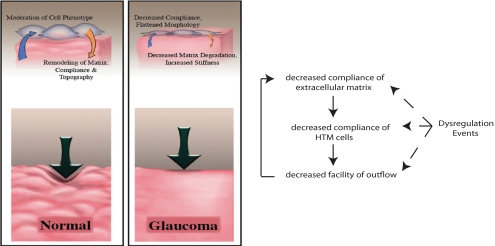
This study reveals for the first time a significant increase in HTM stiffness related to glaucoma. It is important to note that stiffness in this case refers to an intrinsic material property that is independent of the extrinsic forces generated by alterations in fluid dynamics. This finding is consistent with the reported changes that have been observed in the glaucomatous HTM, by either microscopy or gene and protein expression changes. In other work, we have demonstrated that a change in substratum stiffness profoundly alters the modulus of the overlying HTM cells (data not shown). Our data support a model for disease onset and progression in glaucoma wherein dysregulation of the ECM observed with glaucoma is associated with alterations in the stiffness of the HTM and associated cells which, in turn, cause an increase in resistance to aqueous humor outflow (Fig. 5). These interactions set up a vicious cycle that, without therapeutic intervention, would result in a progressive increase in IOP and associated visual field loss. We note that in this scenario there are two distinct contributing factors to the stiffness of the HTM, an intrinsic stiffness of the ECM (i.e., an intrinsic attribute at rest, determined by the constituents of the ECM as well as their three-dimensional nanoscale organization, and a dynamic component [stretch] induced by increasing the pressure gradient across the HTM with decreasing outflow).

Figure 5.

Interplay between the biophysical properties of the ECM, HTM cells, and outflow facility. Normal ECM of the meshwork is compliant and contains topographic features with which the HTM cells can interact. In glaucoma, the matrix is stiff causing increased stiffness of the HTM cells and reduced matrix degradation. The central concept of this proposal is that with initiation or progression of glaucoma, dysregulation events can initiate a feedback loop that increases stiffness of the matrix thereby increasing stiffness of the HTM cells and lowering outflow facility.

The variation of the modulus when taken in 50-μm intervals along the glaucomatous HTM was unexpected. The presence of an elastic modulus less than 10 kPa indicates that the disease process does not uniformly affect the HTM and suggests that relatively unaffected regions persist within the glaucomatous HTM. This finding is consistent with the concept of segmental flow of aqueous humor out of the eye.^48,49 The concept of segmental flow suggests that there is a nonuniform outflow through the meshwork into SC that results in a spatial variation in outflow facility around the circumference of the drainage pathway. Our results suggest that segmental flow is profoundly altered by glaucoma. While information on the severity of the disease for each donor is unknown, we believe that fewer and/or smaller regions having a normal (lower) modulus would be observed with disease progression.

While there are multiple mathematical models that have been and can be used to establish a correlation of modulus and outflow facility, the changes observed in local stiffness of the HTM with disease are in concert with a decreased facility of outflow. There are obvious limitations in our mathematical model as there are with all models. First, the thin plate assumption oversimplifies the real situation in the eye, in which normal stresses may play a significant role. Also, the deformation of the HTM may not be perfectly spherical. We consider the HTM to be purely elastic, and we do not include any viscosity parameters that would more accurately represent real biomaterials. The interesting aspect of the model is that basic physical phenomena are used to predict outflow facility in the HTM. Our model consists of Laplace's law, an elastic strain–stress relationship, and the thin membrane model with a small deflection. We do not consider any biological effects in the model.

These findings strongly suggest that the change in elastic modulus of the HTM is a factor that contributes to the increased IOP that is often observed in POAG. These results point to the development of novel approaches for therapeutic intervention designed to lower the elastic modulus in glaucomatous HTM. Indeed, recent results from our laboratory reveal that changes in substrate elastic modulus dramatically affect cellular responses to therapeutic agents.⁵⁰

It should be noted that while the age ranges of the normal and glaucomatous samples are different, there is substantial overlap in these sets of data between the ages of 72 and 83. Normal samples with donor ages from 70 to 83 continue to have a mean elastic modulus less than 10 kPa. In addition, the normal samples show no statistically significant correlation of elastic modulus with age (P = 0.3614). Each glaucomatous sample with a donor age from 72 to 82, however, had a mean elastic modulus greater than 60 kPa. These observations suggest that it is the presence of glaucoma and not simply an aging of the tissue that is responsible for the increase in the elastic modulus. In addition to the variation in ages, it should be noted that, within each set of samples, the number of days from date of death to analysis also varied. Measurements were completed on normal samples within 30 days of the date of death, ranging from 4 to 30 days and on the glaucomatous samples within 16 days of the date of death, ranging from 4 to 16 days. A correlation analysis of the elastic modulus and the number of days from the date of death revealed no statistically significant correlation for either the normal (P = 0.12) or glaucomatous (P = 0.95) samples.