Kinematic and behavioral evidence for a distinction between trotting and ambling gaits in the cockroach Blaberus discoidalis

SUMMARY

Earlier observations had suggested that cockroaches might show multiple patterns of leg coordination, or gaits, but these were not followed by detailed behavioral or kinematic measurements that would allow a definite conclusion. We measured the walking speeds of cockroaches exploring a large arena and found that the body movements tended to cluster at one of two preferred speeds, either very slow (<10 cm s^–1) or fairly fast (∼30 cm s^–1). To highlight the neural control of walking leg movements, we experimentally reduced the mechanical coupling among the various legs by tethering the animals and allowing them to walk in place on a lightly oiled glass plate. Under these conditions, the rate of stepping was bimodal, clustering at fast and slow speeds. We next used high-speed videos to extract three-dimensional limb and joint kinematics for each segment of all six legs. The angular excursions and three-dimensional motions of the leg joints over the course of a stride were variable, but had different distributions in each gait. The change in gait occurs at a Froude number of ∼0.4, a speed scale at which a wide variety of animals show a transition between walking and trotting. We conclude that cockroaches do have multiple gaits, with corresponding implications for the collection and interpretation of data on the neural control of locomotion.

INTRODUCTION

During locomotion, legged animals tend to move their limbs in distinct patterns of coordination, called gaits. As they change speeds, animals generally transition from one gait to another in such a way as to minimize the energy expenditure required to maintain the new speed (Alexander, 1989), which can also be influenced by load. However, energy cost is not the only reason one gait might be preferred over another (Reilly et al., 2007). One example is in escape responses, when an animal's choice of gait may be dictated by speed rather than efficiency. Another example is a predator stalking prey: a tiger creeping through the undergrowth in a crouch is not trying to conserve energy. Rather, the tiger enhances stealth by maximizing moment-to-moment control of its limbs. This emphasis on control enables the animal to actively adapt to changes in the height or composition of the substrate, at the cost of energy and speed. Humans make similar tradeoffs when choosing between walking and running (Hoyt and Taylor, 1981; Margaria, 1938). Running is more efficient in terms of distance traveled per unit of energy expended, but a walking gait allows considered responses to changes in situation or environment. Adaptation during running can be handled automatically or semi-automatically through biomechanics and reflexes, but feedback modification to walking motions may take place faster than the steps themselves.

The locomotion of cockroaches has been studied intently for many years. Cockroaches are possibly most famous for evading eradication by humans, and perhaps fittingly, cockroach locomotion and biomechanics have been particularly studied during escapes (Camhi and Tom, 1978; Full and Tu, 1990; Full and Tu, 1991; Nye and Ritzmann, 1992; Kram et al., 1997; Jindrich and Full, 2002; Cowan et al., 2006; Baba et al., 2010). When moving quickly, cockroaches are some of nature's most impressive runners. Their speed relative to their size and their ability to manage difficult terrain with minimal changes in leg motion (Jindrich and Full, 2002; Sponberg and Full, 2008) are exceptional among legged animals are unrivaled by human-engineered walking devices. Cockroaches generally step in a ‘tripod gait’, a trotting (running) gait in which the front and hind legs on one side of the body move synchronously with the middle leg on the other side (Hughes, 1952). The two mirror-image tripods step in an alternating pattern such that the animal always has at least three feet touching the ground, making this pattern both stable and efficient (Ting et al., 1994; Nishii, 2000).

It has long been known that the coordination of the legs within a tripod set is not strict, in the sense that the legs do not always lift off the ground or touch down at exactly the same time (Hughes, 1952; Bert, 1866). A tradition of behavioral investigation suggested that the synchrony grows gradually tighter as the animal's forward velocity increases (Hughes, 1952; Delcomyn, 1971; Spirito and Mushrush, 1979). However, a distinction between two different tripod gaits was noted in the cockroach Periplaneta americana and the stick insect Carausius morosus, based on step synchrony but using the forward–backward motion of the foot to monitor leg motion (Graham, 1972; Graham, 1985; Delcomyn and Usherwood, 1973). Delcomyn and Usherwood named the slower gait an amble, noting that at that speed, the animals “[do] not appear to want to go anywhere” (Delcomyn and Usherwood, 1973). However, measuring gait using the protraction and retraction of the feet or electromyograms (Kozacik, 1981) leaves open the possibility that the timing of ground contact would show a different pattern of coordination. Furthermore, we are not aware of any systematic observations on the running speeds of unconstrained cockroaches not performing escape maneuvers.

If cockroaches have multiple gaits, then it may be that their movements reflect multiple preferred walking speeds corresponding to the gaits. To test this hypothesis, we observed naïve blaberid cockroaches exploring a large (90×90 cm) arena. In addition to a wide distribution of slow locomotion speeds below 10 cm s^–1, freely walking animals also have a faster preferred speed at ∼30 cm s^–1, which is still only approximately half the escape speed (Full and Tu, 1990). This faster speed is mostly used when the animal is out of antennal-contact range of the arena walls. To determine whether walking at these two speeds is coordinated differently by the nervous system, or if kinematic differences are solely a consequence of biomechanics, we removed the mechanical coupling between the legs by placing tethered cockroaches above a slippery surface and found that they still displayed coordinated walking movements with two different preferred speeds. Kinematic information extracted from high-speed videos showed that animals stepping at frequencies above 7 Hz (equivalent to 15–20 cm s^–1) usually exhibited a tightly constrained tripod gait, but animals walking at slower rates were much more variable in their coordination. Sustained stepping at intermediate speeds was rare, and most kinematic parameters showed quantal shifts between slow and fast walking, thus justifying a distinction between ambling and trotting gaits in the cockroach.

MATERIALS AND METHODS

Arena

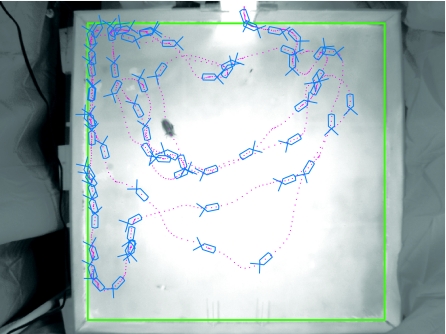
For all experiments, we used adult female cockroaches (Blaberus discoidalis Audinet-Serville) from a laboratory colony. Cockroaches were removed from the colony and isolated for at least 1 h, during their most active circadian phase at nightfall (Roberts, 1960). We constructed a 90×90 cm Plexiglas arena with walls 10 cm high, the upper 4 cm of which were coated with petroleum jelly to prevent the animals from climbing out. The arena was painted opaque white and was brightly lit (∼1500 lux) with incandescent bulbs. Cockroaches entered the arena from a 10×10 cm starting chamber in the center of one wall, gated by a painted, vertically sliding piece of Plexiglas. The starting chamber was lit even more brightly (7600 lux) to encourage the animals to vacate it after the gate was opened. Each cockroach (N=44) was placed in the starting chamber and left for ∼30 s to adapt to the new environment, and to reduce the likelihood of an escape response, we lifted the gate only when the animal was not touching the gate. Only one animal at a time was placed in the arena, and each animal was allowed to explore the arena only once, for 1 min. Between animals, the floor and walls of the arena were washed with detergent and water to remove any physical or chemical evidence of previous occupants. Sheets of black cloth were hung around the arena to remove perspective cues. Each animal's behavior was recorded at 20 frames s^–1 with a digital video camera using the Motmot image acquisition package (Straw and Dickinson, 2009). The position of the cockroach's (visual) center of mass and its body orientation in each frame of the videos were extracted using the Caltech Multiple Fly Tracker (version 0.1.5.6; http://ctrax.berlios.de/) and the associated FixErrors toolbox for MATLAB (MathWorks, Inc., Natick, MA, USA) (Branson et al., 2009). Fig. 1 shows the recording configuration and data obtained from a representative animal.

Fig. 1.

Free walking in B. discoidalis. The grayscale background image is a single frame from a captured video, with the contrast reduced by 50% for clarity. The green box represents the perspective-corrected position of the walls, forming a square 90×90 cm. The magenta points show the position of the cockroach at each frame in the movie (20 frames s^–1). Note that these dots are closer together along the walls, where the animal is walking more slowly. The blue drawings show the position and orientation of the cockroach at every 20th frame (1 frames s^–1), with ‘antennae’ drawn to disambiguate heading.

Oiled plate

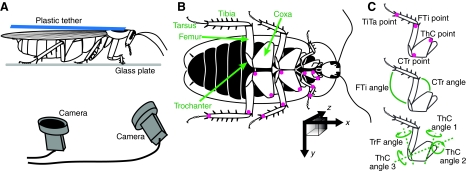
The protocols and video digitization for the oiled plate experiments were performed as previously described (Bender et al., 2010b). Briefly, each cockroach was anesthetized with ice (MacMillan and Sinclair, 2010) and a small piece of plastic was fixed to the dorsal surface of the pronotum. This tether was then used to position the cockroach above the surface of a glass plate prepared with several drops of transparent microtome oil (Lipshaw, Detroit, MI, USA). Two synchronized, high-speed, digital video cameras (MotionScope, Redlake Imaging, Morgan Hill, CA, USA) positioned beneath the glass plate provided different ventral views of the walking cockroach. Video frames were acquired at 500 frames s^–1 in sequences of 8 s at a time, each constituting one trial or bout of walking. Only trials or portions of trials (minimum 5 s) during which the animal appeared to perform limb motions corresponding to forward, straight walking at a constant step rate were used in subsequent analyses. Most walking bouts consisted entirely of spontaneous walking, and although some bouts were initiated with a tap to the abdomen, only sustained, consistent walking (after the initial startle response) was analyzed. A small amount of white paint applied to the leg joints aided us in locating these points in the video images. We report data from a total of 24 walking bouts by nine different cockroaches, the same dataset used in an earlier, preliminary analysis and methodological verification study (Bender et al., 2010b).

Extraction of the three-dimensional (3-D) joint positions was accomplished with a custom-written software package called Legger (Bender et al., 2010b). Our software served to assist a user in calculating the 3-D coordinates of each of the four points on all six legs (five points on the front legs), given synchronous pairs of 2-D images. Once extracted, the 3-D data were rotated into a common reference frame such that the x-axis pointed toward the animal's head, the y-axis was to the left, and the z-axis extended vertically, with the origin near the geometric center of all the data points. The swing and stance phases of a step cycle are defined by whether the leg is touching the ground or not – in our coordinate system, by whether the z-coordinate of the foot was equal to zero (stance) or greater than zero (swing). We first approximated the swing–stance transitions using the derivative of the x-coordinate of each foot and then made more precise calculations using the z-coordinates (Bender et al., 2010b).

RESULTS

Cockroaches freely exploring a large arena tended to walk at approximately one of two forward speeds, ∼30 cm s^–1 and <10 cm s^–1. Tethered animals walking in place on an oiled glass plate also displayed two preferred step rates, corresponding approximately to these forward speeds. Because the slippery surface partially decouples the movements of the various legs, this result suggests that both the coordination and the speed preference are determined internally, rather than arising spontaneously from biomechanical interactions. After extracting 3-D kinematics from high-speed videos of these cockroaches, we found that most motion parameters were significantly different between the two gaits.

Preferred speeds in free walking

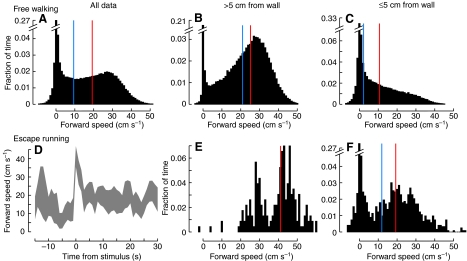
We constructed a 90×90 cm behavioral arena and captured video of cockroaches freely exploring the space without external stimulation. We extracted each animal's body position and orientation in each frame of the video (Fig. 1) automatically, using the Caltech Multiple Fly Tracker (Branson et al., 2009). These data revealed that the animals' forward speeds had a broad distribution, with a sharp peak at very slow speeds and another peak at speeds of ∼30 cm s^–1 (Fig. 2A). Observation of animals in the arena indicated qualitative differences between the walking behavior when the animals were in contact with the arena walls and when they were away from the walls. For example, inspection of Fig. 1 reveals that consecutive body positions are closer together near the walls, indicating that the cockroach appears to slow its walking during those times. To verify this observation, we separated the data by whether the animal's center of mass was within 5 cm of a wall (half the body length + antennal length=4.9 cm, averaged from 12 females of this species). Using this delimiter, clear quantitative differences in walking speed were evident between times when the animals were near a wall versus away from a wall (Fig. 2B,C). When near a wall (≤5 cm), the cockroaches' forward speed distribution had a peak at approximately 0 cm s^–1 and a linear decay at increasing speeds, with a median value of 10.6 cm s^–1. However, for animals not in contact with a wall, their non-zero speeds were noticeably clustered around ∼30 cm s^–1, with a median of 25.3 cm s^–1.

Fig. 2.

Forward walking speed in the open arena. (A–C) Free walking. (A) Pooled data from 44 animals, each walking for ∼1 min; at 20 samples s^–1. (B) Speeds when the animal was more than 5 cm from a wall, approximately the radius of antennal contact (n=31200 samples). (C) Speeds when the animal was less than 5 cm from the nearest wall (data in A minus data in B; n=28464 samples). (D-F) Escape running. (D) Average maximum speeds during escape behaviors (N=10 animals). The envelope represents a 90% confidence interval for the average maximum speed, based on 1000 bootstrap samples of the total 15 stimulation events. (E) Speeds used for escape (within 5 s after stimulation) when the cockroach was >5 cm from a wall, as in B (n=229 samples). (F) Escape speeds within 5 cm of a wall, as in C (n=1271 samples). The blue vertical lines indicate the median walking speed; the red lines show the median speed when data with an absolute value of less than 1 cm s^–1 (i.e. walking not continuous) are excluded.

In order to place in context the speeds we observed in the open arena relative to the well-studied escape behaviors, we collected a small sample of additional data from 10 cockroaches, comprising 15 total escape responses to an abdominal touch (supplementary material Fig. S1). The animals' forward speeds showed a transient peak (median 43 cm s^–1), decaying within 5 s back to speeds similar to free walking (Fig. 2D). Again, the escape speeds depended on wall proximity, such that animals escaping near (≤5 cm) a wall showed a median speed of 19.3 cm s^–1 and animals >5 cm from a wall ran at a median speed of 41.1 cm s^–1 (Fig. 2E,F). The distribution of the escape speeds used by cockroaches when not near a wall showed two clear peaks – one at 25–30 cm s^–1 and one at 45–50 cm s^–1, the latter speeds rarely achieved during free walking. Because this line of experiments was tangential to our purpose of studying the cockroach's usual modes of walking, we did not analyze these escape data further.

Aside from escape behaviors, the distribution of freely chosen speeds is strongly bimodal, with the choice of mode being influenced by the presence or absence of antennal contact with a wall, or potentially, other obstacles or objects (Ritzmann et al., 1991). A multimodal speed distribution is typical of animals that show multiple gaits, where each speed mode represents a single gait and a preferred speed within that gait. However, gaits are more commonly defined by distinct patterns of footfalls and biomechanical energy transfer, because one speed may be used with multiple gaits. We lacked the resolution to track the cockroaches' feet in this large arena, preventing us from determining whether different patterns of leg coordination are used at the different speeds we observed. Furthermore, the legs are mechanically coupled via the substrate, such that when one leg pushes, it alters the joint angles of all of the other legs that are touching the ground. Such passive joint motion could act to trigger active movements (Ekeberg et al., 2004), homogenizing leg coordination and obscuring any internal, neuromechanical effects on stepping. Therefore, we sought to decouple the legs by examining animals tethered and walking in place on a slippery surface, also allowing high-resolution kinematic measurements (Figs 3, 4) (Bender et al., 2010b).

Fig. 3.

Walking on the oiled plate. (A) A tethered cockroach walked in place on a lightly oiled glass plate in view of two synchronized cameras (500 frames s^–1). (B) Ventral view of a cockroach. The colored dots on the legs show the points that were marked with paint and then tracked in the movies. The black arrows (bottom, right) indicate the coordinate system used, with the z-axis extending into the page. The x- and y-vectors shown would be ∼1 cm in length. (C) Position of points and angles measured. CTr, coxa–trochanter (joint); FTi, femur–tibia; ThC, thorax–coxa; TiTa, tibia–tarsus; TrF, trochanter–femur.

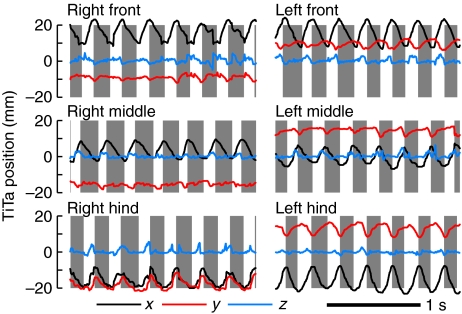
Fig. 4.

) Plots of 3-D position versus time of the TiTa data from one animal. The stance phase of each stride (approximately when the z-value of the TiTa point was zero) is indicated by gray boxes.

Bimodal step rates on the oiled plate

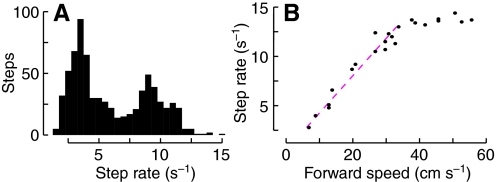
Cockroaches walking in place on the oiled plate consistently preferred one of two step rates, ∼3 Hz and ∼9 Hz (Fig. 5A). We correlated these step rates to forward speeds using data published by Full and Tu, who measured both simultaneously on a force platform (replotted in Fig. 5B) (Full and Tu, 1990). Separating the two gaits with a threshold at 7 Hz suggests that a transition occurs at forward speeds of 15–20 cm s^–1, speeds that were less common in unrestrained animals (Fig. 2A). Intriguingly, the Froude number for a cockroach walking at that speed is ∼0.5, similar to that at which virtually all animals transition from walking to running (see Discussion).

Fig. 5.

Step rates on the oiled plate. (A) Histogram of pooled data from 24 bouts of walking by nine animals, 5–8 s per bout. (B) Step rate is linearly related to walking speed except at very high rates [replotted from Ting et al. (Ting et al., 1994)]. The dashed line is a best fit to the speeds below 35 cm s^–1, and exceeds a step rate of 7 Hz at ∼15–20 cm s^–1 (compare with Fig. 2).

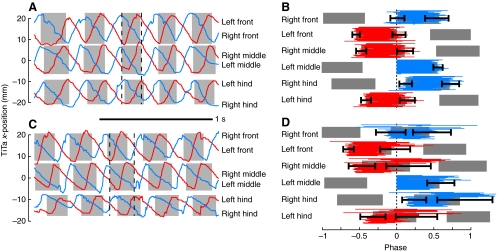
This bimodality is again suggestive of two distinct gaits at slow and fast speeds, which we will refer to as ambling (Delcomyn and Usherwood, 1973) and trotting (Full and Tu, 1990), respectively. However, gaits are generally defined according to leg coordination rather than speed alone. Cockroaches on the oiled plate did not obviously display any patterns of coordination other than the alternating tripod gait, unlike stick insects, which can walk with a quadrupedal gait at very slow speeds (Graham, 1972). However, there was considerable variability in the coordination of the legs within each tripod (Fig. 6). As a rule, the front leg in each tripod tended to move first, then the middle, then the hind leg (see gray boxes in Fig. 6A,C) but the relationship between the three legs in each tripod was not fixed (Fig. 6B,D). To calculate how tightly coordinated the motions of each tripod were, we converted the step times into terms of phase, where all the steps were aligned to the nearest step made by the left middle leg. We first computed the phase difference between the beginning of swing in the front leg of each tripod and the beginning of swing in the middle leg of the same tripod set (the contralateral middle leg). We recalculated the same measure using stance instead of swing, then using the hind leg and the middle leg, and then we calculated the same metrics within the other tripod set. The mean absolute value of these eight phase differences yielded a single ‘tripod coordination index’ (TCI) measuring how precisely the legs of each tripod set were moved together in each stride. A TCI of 0 would indicate perfect tripod synchrony and a value of 0.5 would imply a perfect antiphase relationship if swing and stance had equal durations. More intuitively, the TCI is roughly correlated with the average length of the ‘whiskers’ in Fig. 6B,D, suggesting that the choice of the left, middle leg as a reference is irrelevant. To verify this, we calculated the TCI using different reference legs and found only small effects (data not shown).

Fig. 6.

Motion plots and footfall rasters for two different bouts of walking by the same animal walking at the same speed. The upper bout had consistently low values (mean=0.09) of the tripod coordination index (TCI; see text), whereas the lower bout had much higher TCI (mean=0.19). (A,C) Forward–backward motion of the TiTa points of each of the six legs. Traces are colored according to the tripod set to which each leg belongs, i.e. the same color is used for all the legs that move together. (B,D) Phase-adjusted raster of step timing. The thin red and blue bars represent the stance period for a single stride by each leg, aligned to steps by the left middle leg. The colors of the bars match the colors in A and C. The gray boxes denote the average stance period for each leg (the mean of the colored bars), and the black whiskers show the standard deviation of the starting and ending phase of stance. The steps taken by the legs of a single tripod group were more tightly aligned, on average, and less variable for the trial in B than for the trial in D; i.e. the steps shown in B generally had smaller TCI values.

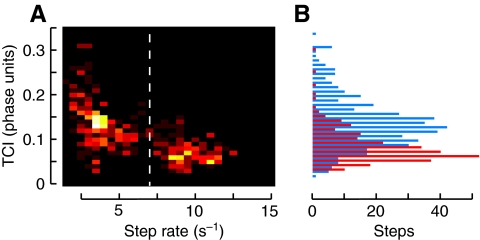
TCI showed a correlation with step rate (Fig. 7A), but not a simple linear trend. Rather, all fast stepping (trotting) had fairly low TCI values (<0.1), indicating that the legs moved fairly synchronously (Fig. 7B). In contrast, slow stepping (ambling) had quite a variable TCI, with an average value near 0.15 and considerably more variance. This is true even though TCI is calculated in phase units, meaning that the variance of leg synchrony as a fraction of the step cycle still decreases with decreasing step period. A t-test comparing the ambling and trotting TCI distributions yielded P<10^–47, indicating that interleg coordination is significantly different between the two gaits. Are changes to tripod coordination just an epiphenomenon, a mechanical consequence of gradually increasing coordination with speed, or are there other discontinuities consistent with a change in control strategies?

Fig. 7.

(A) Two-dimensional histogram of step rate versus TCI (see Fig. 6). (B) Histogram of TCI, divided between high-speed walking (red) and low-speed walking (blue) at a step-rate threshold of 7 Hz. A t-test comparing the high-speed and low-speed walking distributions resulted in a P-value of <10^–47.

Gait-dependent changes in kinematics

Early investigators concluded that the patterns of leg motion in walking cockroaches, though different at high and low speeds, “grade insensibly” into each other at intermediate rates (Hughes, 1952; Delcomyn, 1971). However, our large, high-resolution dataset gives us the opportunity to revisit this conclusion by looking for discontinuities that may be characteristic of different gaits. Motion parameters such as step length and angular excursion by the leg joints all vary slightly from step to step, but if they vary differently in the two gaits that would add support to their definition as distinct patterns of stepping.

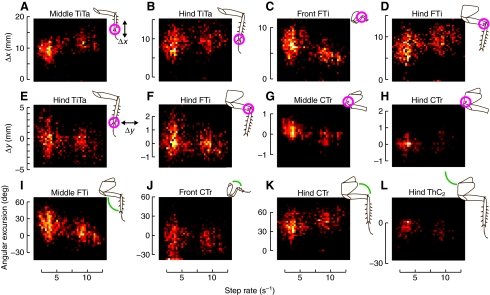
To test this hypothesis, in each step, we first found how far each tracked point moved along each body axis. For the x-axis, we subtracted the position of the point at the end of stance from its position at the beginning of stance, using the median position from five successive measurements. This metric of forward–backward motion along the animal's body during a step, when measured at the tibia–tarsus (TiTa) joint, corresponds to the stride length. However, each of the points corresponding to a leg joint has the ability to move in 3-D space, and each point can move quasi-independently because of kinematic redundancy. In addition to forward–backward motion, we also calculated side-to-side motion (along the y-axis) and vertical motion (along the z-axis) at each tracked point, subtracting the position halfway through swing from the position halfway through stance in each stride. These correspond to lateral and vertical excursions of that leg joint during each step. Finally, the joint flexion angles may be of more direct relevance to the nervous system than the Euclidean coordinates of the joints. We used the 3-D leg position data to calculate angles for each of the leg joints other than the TiTa joint and then calculated the angular excursion of each joint over the stance phase of each stride. This totaled three motion dimensions for each of four tracked points, plus five joint angles, for each of three pairs of legs, or 51 metrics of leg motion. The distributions of 43 (84%) of these measures differed significantly between ambling and walking speeds (t-test, P<0.01; Fig. 8). The only exceptions were the middle leg TiTa Δy (P=0.319), middle femur–tibia (FTi) Δz (P=0.328), hind trochanter–femur (TrF) angle (θ; P=0.197), front coxa–trochanter (CTr) Δz (P=0.263), front CTr θ (P=0.327), front and middle thorax–coxa (ThC) Δz (P=0.015 and P=0.034) and the front ThC θ₃ (ThC₃; P=0.453). Note that a significant change in ThC Δz would indicate that the entire thorax was bouncing differently during each step even though the tether tended to damp any vertical motion of the body; our data showed smaller but still strong trends in that direction. This vertical motion of the thorax shows a lack of complete mechanical decoupling between the various legs, but B. discoidalis will not walk on a very rigid tether. However, the enhancement of the speed preferences on the oiled plate relative to the open arena suggest that total isolation of the legs' mechanics would probably result in even larger kinematic changes between gaits.

Fig. 8.

Kinematic parameters vary between gaits. Each panel is a 2-D histogram of a different parameter for one joint of one leg pair. (A–D) Motion along the long axis of the body (Δx), measured at the beginning and end of stance, which for the TiTa point is roughly equivalent to stride length. (E–H) Lateral motion (Δy), measured halfway through stance and halfway through swing. (I–L) Joint excursion, which is the difference between the joint angle at the beginning and end of each stance phase; it is negative if the joint extended during stance.

The motion parameters in Fig. 8 show clear differences between steps in the two gaits. Some of these changes could be simple linear trends with speed, such as the FTi θ for the middle legs (Fig. 8I), in which the two gaits differ only because of their different average speeds. However, in some parameters, such as the front FTi Δx (Fig. 8C), the speed-dependent trends are opposite in the two gaits, indicating a qualitative shift in patterns of leg motion between gaits. These do not conclusively demonstrate a categorical change in gait mechanics, and only subtle changes appear in leg coordination (Fig. 7), but the preponderance of the evidence supports an interpretation of two distinct gaits in the cockroach.

DISCUSSION

Stepping behaviors in the cockroach B. discoidalis tended toward one of two preferred speeds, both in an open arena (Fig. 2) and in tethered preparations walking on a slippery surface (Fig. 5). These behaviors differed not only in step rate, but in patterns of coordination between the alternating tripods of support (Figs 5, 7) and in nearly all 3-D motion parameters (Fig. 8). Together, these data point to a change in the way leg motions are produced by the neuromuscular system at different speeds, consistent with the conclusions that cockroaches have multiple tripod gaits, a low-speed amble and a high-speed trot.

Locomotor behavior in the cockroach

Most of the literature on cockroach locomotion has focused on the tripod trot, and in particular on escape behaviors, but our large-scale behavioral observations in the open arena found extensive use of speeds typical to trotting and ambling gaits and only rare occurrences of escape-type speeds. One possible explanation for the bias in the literature may be the tendency of slower locomotion in the cockroach to be intermittent (Full and Tu, 1990). However, it is possible that cockroaches generally use the trotting gait as an escape response or when moving ‘purposefully’ from place to place, but normally amble when exploring and foraging in a smaller area. For example, our observations of freely walking animals indicated that cockroaches walking in close proximity to a wall almost always traveled slowly, presumably in an ambling gait. This is in contrast to nearly all of the studies on the wall-following behavior, which have been done predominantly in the context of trotting speeds (Camhi and Tom, 1978; Camhi and Levy, 1988; Camhi and Johnson, 1999; Cowan et al., 2006; Baba et al., 2010). From our data, it appears that high-speed wall following probably occurs mostly during escape behaviors rather than more common periods of foraging, a fact neuroethologists should note (compare Fig. 2C with F).

We found that the transition between ambling and trotting occurred at 6–7 Hz stepping, or 15–20 cm s^–1 forward speed (Figs 2, 5). Animals as diverse as humans (Margaria, 1938), horses (Hoyt and Taylor, 1981), rats (Alexander and Jayes, 1983), crows (Hayes and Alexander, 1983) and crabs (Blickhan and Full, 1987) change gaits at forward speeds that are predictable functions of the animal's size, even when the gaits themselves are not identical (e.g. hopping in birds). The constant factor is a dimensionless quantity known as the Froude number (F=u²/gl, where u is the velocity, g is gravitational acceleration, and l is a ‘characteristic length’, typically the length of the legs). Quadrupeds, for example, generally transition from walking to trotting at F≈0.35 and from trotting to galloping at values of F≈2.5 (Biknevicius and Reilly, 2006; Alexander, 1989). From our data, the Froude number for the amble–trot transition is in the range of 0.4–0.6, depending on what value is taken for l. Full and Tu used 0.4 cm for l in this species of cockroach, approximately the height of the animal's center of mass (Full and Tu, 1990). However, they did not specify what sex their animals were, whereas we used exclusively female cockroaches, which are somewhat larger than males. If a value of l as high as 0.6 cm could be justified on this basis, then the gait change occurs at F=0.4, precisely in the range where virtually all animals change gaits.

Intriguingly, a value of l=0.6 cm also yields F=2.5 just below 40 cm s^–1, raising the possibility of another gait transition between the two peaks in the distribution of escape speeds (Fig. 2E). This is the same speed at which B. discoidalis reaches its maximum step rate (Fig. 5B) and instead begins lengthening its strides in order to go faster, i.e. alters its gait (Full and Tu, 1990). We did not study escape-type speeds on the oiled plate because of their transient nature, but it is at least possible that cockroaches show equivalents of both of the standard gait transitions (walk–trot and trot–gallop). As with crows and crabs, however, the nature of the gaits themselves may be different from our somewhat anthropocentric standards. Walking, for example, is typically distinguished from trotting by its use of an inverted-pendulum pattern of energy exchange, which would be somewhat surprising to observe in a cockroach, given its small size and sprawled anatomy (Reilly et al., 2007); however, it might be feasible if the two tripods are considered as single legs (Schmitt et al., 2002). These questions may be worthy of future biomechanical studies.

Gait control

One obvious physiological correlate of the differences between slow and fast walking in cockroaches is the firing of the so-called ‘fast’ motor neuron pool (Pringle, 1939). In B. discoidalis, the slow motor neurons fire at all walking speeds, and their firing rate is the main determinant of the angular velocity of the joints (Watson and Ritzmann, 1998a; Watson and Ritzmann, 1998b). However, the fast motor neurons fire only at step rates of ∼5 Hz and higher, and their activity appears to sharpen the transition from swing to stance and increase the muscle torque available to overcome inertial motion of the legs and body (Watson and Ritzmann, 1998b). It would be surprising if it were coincidental that the fast motor neurons begin to fire at the same walking speeds that the animals switch from ambling to trotting. Testing the causality of this relationship should also be a goal of future studies.

In the stick insect C. morosus, Graham found the equivalent of both ambling and trotting gaits in first-instar nymphs, but noted that the tripod stepping pattern was “relatively rare in the adult and only occurred at the highest walking speeds” (Graham, 1972). At slower speeds, both juvenile and adult stick insects walked with a metachronal gait pattern. In a metachronal gait, a hindmost leg steps first, and each successive ipsilateral leg lifts off the ground immediately after its posterior neighbor touches down, suggesting that the legs are coordinated mainly by feedback (Borgmann et al., 2007; Borgmann et al., 2009). A mechanism for this was recently demonstrated in the cockroach P. americana by Zill and co-workers (Zill et al., 2010). They found that as a posterior foot sets down at the end of its swing phase, it touches down near the place where its anterior neighbor is reaching its posterior extreme position at the end of its stance phase. The beginning of stance in the posterior leg causes gravitational load to be reduced on the anterior leg, a change sensed by campaniform sensilla in (at least) the tibia. This unloading almost immediately causes the anterior leg to begin its swing phase. A series of studies in C. morosus showed that the entire chain of motor events during a step by even one leg could be successfully modeled using only such feedback interactions (Dürr et al., 2003; Ekeberg et al., 2004; Cruse et al., 2007). However, it is also known that central-pattern-generating (CPG) circuitry exists in the locomotor nervous system of adult stick insects and can be activated by the pharmacological application of pilocarpine, a muscarinic receptor agonist (Büschges et al., 1995). If the gait can emerge strictly through feedback interactions, do the CPGs act to complement the local feedback circuits rather than as the primary drivers of the muscles?

A third possible answer to the relationship between the CPGs and the feedback circuitry for leg coordination is suggested by combining our findings with the results of experiments by Noah et al. on P. americana (Noah et al., 2004). Noah and his colleagues cut the afferent nerves in a cockroach's hind legs at the level of the femur, removing feedback from the campaniform sensilla in the tibia that are responsible for coordinating the metachronal gait (Zill et al., 2010). Animals with this manipulation walked with abnormal gaits, as the hind legs stepped very irregularly relative to motions of the other legs. However, when startled, even deafferented cockroaches were capable of sustaining a fast tripod trot. In addition, a body of literature shows that running cockroaches do not readily adapt their leg motions to the shape of the substrate (Jindrich and Full, 1999; Jindrich and Full, 2002; Sponberg and Full, 2008; Spence et al., 2010). In particular, it has been suggested that feedback is too slow to be useful to a sprinting animal, at least within a single stride (Full et al., 2002).This distinction between the importance of afferent feedback in coordinating a slower gait and its apparent irrelevance to faster running could provide a simple explanation for our observations, and one that also resolves the dilemma of how a walking insect uses both CPGs and feedback in gait control. Perhaps the most parsimonious hypothesis is that ambling is a metachronal gait coordinated by sensory feedback, making it more variable (Fig. 5), whereas trotting is centrally coordinated by means of CPGs, similar to the proposal of Cruse et al. based only on the stick insect (Cruse et al., 2007). Intriguingly, some neurons in the central complex of the cockroach brain, a high-level sensorimotor area, were found to fire in phase with walking steps only at step rates faster than ∼5–8 Hz (Bender et al., 2010a), consistent with a switch to enhanced central control during faster locomotion.

LIST OF ABBREVIATIONS

CTr

coxa–trochanter

FTi

femur–tibia

TCI

tripod coordination index

ThC

thorax–coxa

TiTa

tibia–tarsus

TrF

trochanter–femur