A reappraisal of grandmothering and natural selection

Kachel et al. [1] conclude from simulations of their agent-based model that fitness benefits from helpful grandmothers do not select for increased longevity. We studied their assumptions and model, ran further simulations and found flaws that are fatal to their test.

Here, we explain four problems and show their consequences.

We agree that questions about evolutionary links between grandmothering and longevity are important. They arise from a puzzle in human life history and comparisons between humans and other living great apes. In all great apes, including humans, the childbearing years extend into the forties but not beyond. In all except humans, females rarely survive the childbearing years, but in all human populations, girls that survive to adulthood usually live well past menopause. The post-menopausal longevity of humans may be explained by the grandmother hypothesis that links the exceptional post-menopausal longevity of humans to other features that distinguish us from our closest living relatives [2,3]. Unlike other apes, human mothers can raise multiple offspring simultaneously because they often have help from their own mothers [4,5]. Supposing the ancestral life history of humans were once similar to life histories of other great apes, an ecological shift that reduced the foraging success of young juveniles would have presented a novel fitness opportunity to ageing females. As their fertility declined, they could subsidize the child rearing of their daughters by provisioning grandchildren. Their daughters would be able to bear children more frequently without compromising the survival of older offspring. Helpful grandmothers would thus leave more successful descendants. As longer lived grandmothers could help more, selection would favour allocating resources away from earlier subsidized reproductive effort towards somatic maintenance, thus shifting an ape-like lifespan towards human longevity.

Or would it? Kachel et al. propose a quantitative test of this argument. They conclude from their simulations that,

‘…despite the fact that the help provided by grandmothers significantly reduces the mean interbirth intervals of their adult daughters and significantly increases the survival of their matrilineal grandchildren in our model, grandmothering has no effect on the evolution of longevity relative to baseline simulations under any of the conditions tested here’. [1, p. 389]

This finding seems to contradict a previous simulation model constructed by Lee [6] who found that intergenerational sharing among a grandmother, her daughters and their children, maintained a mortality schedule with longevities like those of modern humans. The Kachel et al. [1] simulations differ in several ways from Lee's, among them is their aim to see whether grandmother effects could select for greater longevity in the first place. Kachel et al.'s findings are especially arresting because their model assumes that greater longevity has no cost. Life-history models, and the grandmother hypothesis in particular, generally assume that any increased allocation to somatic maintenance must reduce allocation to current reproduction. According to their conclusion, although increased longevity is beneficial and cost-free (1, p. 386), it does not spread. As the authors ask, ‘How can this be explained?’ (1, p. 389). They answer that, ‘this is due to the relatively weak selection that applies to women near or beyond the end of their reproductive period’ (1, p. 384). But even under weak selection—unless overwhelmed by drift—traits that increase fitness at no cost should spread.

The first and most important flaw we found in Kachel et al.'s [1] model is the assumption that increased longevity is costless. It places the initial condition of the model so far from equilibrium that every simulation results in the same outcome. While the authors conclude that this is owing to the weak impact of grandmothering on fitness because outcomes do not differ whether or not grandmothering is included, that result is simply the consequence of a race to increase ‘costless’ longevity.

Second, because they stopped their simulations at only 10 000 iterations and found similar results whether or not they included grandmother effects, they concluded that those effects did not select for longevity. Instead, Fisher's fundamental theorem identifies the reason:

‘…the rate at which a species responds to selection in favour of any increase or decrease of parts depends on the total heritable variance available’. [7, p. 16]

Even if maximum longevity were perfectly correlated with fitness, it could increase no faster than the rate at which genetic variance enters the population. Kachel et al. assume that at birth each individual inherits two alleles for longevity. Mutations occur at each locus at a rate of μ = 0.05, and the effect of each mutation is drawn from a truncated normal distribution with mean 0 and s.d. σ = 0.5. If we assume a generation time of 20 years, then 10 000 iterations is equivalent to t = 500 generations. Thus, we estimate the additive variance per generation to be

where v_t is the variance at time t, and N is the population size. Since,

and the mean effect per mutation is 0, we can simplify the expected complete variance as

In other words, given the model assumptions, the maximum rate that longevity can increase is about 6.25 years over 10 000 iterations. Kachel et al. [1] found increases in longevity of about 5 years regardless of the influence of grandmother effects. Rather than evidence against selection for longevity, this rate of change is close to the maximum allowed by their population size and mutation rate assumptions if longevity is perfectly correlated with fitness. Given the stochasticity of their model and the assumption that the population remains stationary at 1000, changes of about 5 years over 10 000 iterations indicate strong selection for increased longevity.

The third flaw is the authors' measure of longevity, x_L. We first note a problem with their treatment of mortality that we do not correct. They assume mortality follows a Siler model [8] and ‘use empirical data from recent hunter–gatherer populations [9]’ [1, p. 3] to fix all the Siler parameters except b₃, the exponent in the ageing term. Although they set x_L initially to longevity of 50 years, this is achieved with human values for two of the three Siler parameters for adult mortality, a₂ and a₃. Chimpanzee values for those parameters are notably higher than the human values [9]. On the other hand, the one adult mortality parameter they allow to vary, b₃, is actually lower in chimpanzees than in humans [9]. As noted above, other great ape females rarely outlive the childbearing years, while humans—if they survive to adulthood—usually do. The grandmother hypothesis proposes that grandmother effects propel that difference. We do not alter their assumptions about initial and unchanging human values for a₂ and a₃ or their singular focus on b₃. But their longevity measure makes results difficult to interpret, so we translate this problematic measure into a standard parameter, adult life expectancy. Their measure, x_L, is obtained by solving the Siler equation 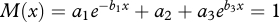for x. In other words, the solution x_L denotes the time at which age-specific mortality exceeds 1. This non-intuitive index depends on the age-specific mortality at one time and is not a good indicator of overall survivorship. We retain their Siler assumptions but evaluate resulting life expectancy at age 15, denoted by e₁₅, since 15 is the age that Kachel et al. [1] assume individuals are eligible to reproduce. Their measure x_L corresponds to adult life expectancy in a logarithmic manner. For example, when the other Siler parameters take the values they use, and b₃ is allowed to vary, adult life expectancies of e₁₅ = 20, 50, 80 and 99 years correspond to values of x_L = 49, 132, 342 and 1M yr, respectively.

Finally, the authors claim that,

‘…the data collected from our two-sex model provide no evidence to support the notion that old-age male reproduction had a large effect on the evolution of increased longevity’. [1, p. 389]

But their model assumes that when a female reproduces, she randomly chooses a mate among all adult males. This assumption strongly favours longer lived males, since they have a higher chance of reproductive opportunities. As longevity mutations are cost-free, the model should result in directional selection for increased longevity through males.

To quantify the effect of these features on simulation results, we modified Kachel et al.' model as follows. We ignored their assumptions about a fixed reproductive potential with mutations on the length of the fertile period beyond the initial age of 15 because this part of the model is not relevant to selection on longevity. We did not alter their assumption about longevity mutations just on b₃, but instead of assessing effects of selection on longevity with the problematic x_L, we evaluated the Siler mortality schedule with a standard survivorship equation to calculate life expectancy at 15. With these modifications, we ran 25 simulations for each of four scenarios. First, we compared unlimited male fertility with the case of age constraints on male reproduction:

• — Unlimited male fertility: females choose among all males with equal probability, as Kachel et al. [1] assumed.

• — Unlimited male fertility removed: we simply assumed that male fertility follows the same Brass polynomial as female fertility, and a female chooses male k with probability , where there are N males and m_i is the fertility of male i for i = 1, … , N.

Then to evaluate grandmother effects, we considered the following two cases:

• — No grandmothering: this is otherwise similar to their basic model with birth intervals of 5 years.

• — With grandmothering: no mortality for offspring with living grandmothers for up to 10 years and weaning age reduced to 1 year.

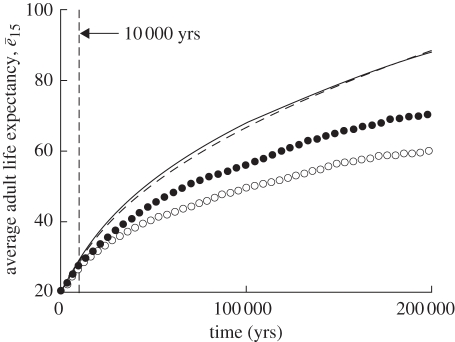
Results of simulating the four scenarios are shown in figure 1. They indicate first, as Kachel et al. [1] found, that within 10 000 years, little change is apparent. This is because their assumptions about mutations and population size strongly tether the possible rate of change. Second, when simulations are allowed to continue they reveal the strong effect of old-age male fertility on the evolution of longevity. Third, when the effect of old-age male fertility is controlled, grandmothering significantly improves the rate of increase in longevity. But, fourth, even with old-age male fertility controlled and no grandmothering, increased longevity evolves because it has no cost and higher longevity always improves the chance of surviving the childbearing years.

Figure 1.

Time evolution of average life expectancy of the population over four scenarios. Results are the average of 25 simulations. Solid line, unlimited male fertility, with grandmothering; dashed line, unlimited male fertility, no grandmothering; filled circles, limited male fertility, with grandmothering; open circle, limited male fertility, no grandmothering.

Kachel et al.'s [1] contribution has served to highlight the need for a quantitative model of grandmothering and shown this is not an easy need to fill. Flaws in their model preclude the conclusions drawn from it. We have shown that when the time scale is lengthened, longevity is measured in a conventional way, and the driving force of old-age male fertility is removed, simulations with their model do not produce the results they claim for it. We hope their attempt will stimulate others to tackle the problem of building a formal model that can quantify how much helpful grandmothering could shift a life history similar to that of the other apes towards the notably greater longevity that evolved in our lineage.