Abstract
The contribution of the three core components of working memory (WM) to the development of mathematical skills in young children is poorly understood. The relation between specific WM components and Numerical Operations, which emphasize computation and fact retrieval, and Mathematical Reasoning, which emphasizes verbal problem solving abilities in 48 2nd and 50 3rd graders was assessed using standardized WM and mathematical achievement measures. For 2nd graders, the central executive and phonological components predicted Mathematical Reasoning skills; whereas the visuo-spatial component predicted both Mathematical Reasoning and Numerical Operations skills in 3rd graders. This pattern suggests that the central executive and phonological loop facilitate performance during early stages of mathematical learning whereas visuo-spatial representations play an increasingly important role during later stages. We propose that these changes reflect a shift from prefrontal to parietal cortical functions during mathematical skill acquisition. Implications for learning and individual differences are discussed.
Keywords: Mathematical reasoning, Numerical operations, Central executive, Phonological loop, Visuo-spatial sketchpad
1. Introduction
Although the basics of mathematics are among the more important competencies that children need to master for successful living in modern societies, our understanding of the cognitive mechanisms that support mathematics learning is limited (Mazzocco, 2008). What is known suggests that working memory (WM) is pivotal to many aspects of learning mathematics (Bull, Epsy & Wiebe, 2008; Geary, 1990; Geary & Brown, 1991; Geary, Hamson & Hoard, 2000; Geary, Hoard, Byrd-Craven & DeSoto, 2004; Hitch & McAuley, 1991; Passolunghi & Siegel, 2001, 2004; Siegel & Ryan, 1989; Swanson, 1993, 1994; Swanson & Sachse-Lee, 2001; van der Sluis, van der Leij & de Jong, 2005; Wilson & Swanson, 2001). However, the relations between the different components of WM and mathematical competence are not as well established in children compared to adults (Ashcraft, 1992; Furst & Hitch, 2000; Hecht, 2002; Lemaire, Abdi & Fayol, 1996; Logie, Gilhooly & Wynn, 1994).
Many children begin school with an implicit understanding of aspects of number, counting, and arithmetic and WM may contribute to their ability to build on this informal knowledge during schooling (Geary & Brown, 1991; Geary & Burlingham-Dubree, 1989; Siegler & Jenkins, 1989). Children who excel in early mathematics learning tend to have high WM capacity (Hoard, Geary, Byrd-Craven & Nugent, 2008; Passolunghi, Mammarella & Altoe, 2008), and mathematically gifted adolescents tend to have enhanced visuo-spatial WM (Dark & Benbow, 1990). WM has also been reported to mediate the relationship between IQ and mathematical performance as early as the 1st grade (Passolunghi et al., 2008). Children’s learning of the mathematical number line is influenced by a combination of intelligence, the central executive, and visuo-spatial WM. However, the relative contributions of these WM components to learning changes from 1st to 2nd grade as the central executive increases in importance, whereas the roles of intelligence and visuo-spatial WM decline (Geary, Hoard, Nugent & Byrd-Craven, 2008). In short, we know that WM is critical for mathematics learning but we do not fully understand how the different components of WM contribute to learning in different areas of mathematics and we do not know whether the importance of one or more WM components changes as learning progresses. We begin with a brief review of the components of WM and their relation to mathematics learning, and then outline how the current study addresses the issue of whether the relative importance of the different WM systems changes from one grade to the next.
1.1. Working memory and mathematical cognition
WM is a cognitive system specialized for storage and manipulation of information (Baddeley, Hitch & Bower, 1974). Although different theoretical models of WM have been proposed (for a review see Miyake & Shah, 1999), Baddeley and Hitch’s model has been the most influential. In this model, WM is composed of a central executive and two subsystems for temporary storage and rehearsal of auditory-verbal and visuo-spatial information, the phonological loop and the visuo-spatial sketchpad, respectively (Baddeley, 1986, 1996; Baddeley et al., 1974; Miyake & Shah, 1999).
The central executive plays an important role in sequencing operations, coordinating the flow of information, and guiding decision-making (Baddeley, 1996; Baddeley, Emslie, Kolodny & Duncan, 1998), particularly when problems are more complex and facts cannot be easily retrieved from memory. The central executive is important for many aspects of mathematical performance, including use of complex arithmetic procedures that involve carrying and borrowing operations (Ashcraft, 1992; De Rammelaere, Stuyven & Vandierendonck, 1999, 2001; Frensch & Geary, 1993; Geary, Frensch & Wiley, 1993; Hecht, 2002; Lemaire et al., 1996). The two other components of WM are specialized for storage of domain-specific information. The phonological loop is involved in encoding and maintaining arithmetical operands (Furst & Hitch, 2000; Logie et al., 1994; Noel, Desert, Aubrun & Seron, 2001) and maintaining intermediate results (Heathcote, 1994), but not specifically in calculation of answers (De Rammelaere et al., 1999; Furst & Hitch, 2000; Hecht, 2002; Lemaire et al., 1996). Furst and Hitch showed that the phonological loop is involved in retaining and storing information about complex problems, but it is not critically involved in retrieving factual mathematical knowledge (Furst & Hitch, 2000). Consistent with this, the relationship between phonological loop and adults’ mathematical performance has been relatively weak, except in dual-task paradigms when the phonological loop is excessively taxed (Heathcote, 1994; Lehto, 1995; Logie & Baddeley, 1987; Logie et al., 1994). In adults, the visuo-spatial sketchpad has been implicated in solving multi-digit operations (Heathcote, 1994) and in more complex algebraic and geometric problem solving (Reuhkala, 2001). Notwithstanding these findings, the role of the visuo-spatial sketchpad in mathematical cognition remains poorly understood.
1.2. Role of specific WM components in mathematical learning
Each WM component has a specialized role in mathematical cognition that varies with expertise and development. Different levels of experience with numbers and mathematical concepts, familiarity of the stimuli and the strength of representations can lead to changes in the types of strategies applied to solve a mathematical task; this in turn calls upon different WM components (Gathercole & Adams, 1994; Henry & Miller, 1991).
Children under the age of seven tend to rely more on visual memory to remember material such as pictures of familiar and nameable objects rather than coding visual items to verbal labels (Hitch, Halliday, Schaafstal & Schraagen, 1988). Some researchers have suggested that preschoolers tend to perform better on nonverbal rather than verbal arithmetic tasks and that the visuo-spatial sketchpad capacity is the best predictor of these abilities in this age group (Levine, Jordan & Huttenlocher, 1992; McKenzie, Bull & Gray, 2003; Rasmussen & Bisanz, 2005; Simmons, Chris & Horne, 2008). From the age of seven onwards, however, children increasingly rely on verbal rehearsal to maintain information in memory, thus recruiting the phonological loop (Hitch et al., 1988). Consistent with this, Rasmussen and Bisanz found that by the 1st grade, performance becomes equivalent on nonverbal and verbal mathematical tasks, and that the phonological loop becomes the best predictor of performance on verbal mathematics problems (Rasmussen & Bisanz, 2005). WM also influences math performance in elementary school: in a large sample of 1st, 2nd and 3rd graders, Swanson found that younger children and children who were poor mathematical problem solvers performed less well on WM tasks than older children or children who were good problem solvers (Swanson & Beebe-Frankenberger, 2004). However, the specific contributions of each WM component across grades were not examined.
In 7- to 8-year-old children, one study found that mathematics performance is most strongly correlated with the central executive, followed by the phonological loop (L. Henry & MacLean, 2003). As the supervisory system, the central executive facilitates children’s problem solving by aiding in selection of appropriate strategies (Barrouillet & Lepine, 2005; Bull, Johnston & Roy, 1999; Geary et al., 2004) and by allocating attention resources to implement the strategy execution. Using a longitudinal design, Gathercole and Pickering found that central executive measures shared significant and unique links with children’s standardized test scores in mental arithmetic at 7 years of age and again at 8 years of age (Gathercole & Pickering, 2000). On the other hand, in a large sample of 8- to 11-year-old children, Adams and Hitch found that articulatory suppression significantly disrupted children’s ability to solve arithmetic problems (Adams, Hitch & Donlan, 1998), suggesting an important role for the phonological loop. Other studies have suggested that the phonological loop is engaged when children transform symbol and number strings into verbal code when using verbally mediated counting strategies during basic arithmetic problem solving (Baddeley & Logie, 1987; Geary, Bow-Thomas, Liu & Siegler, 1996; Geary et al., 1993; Logie et al., 1994; Miura, Yukari, Vlahovic-Stetic, Kim & Han, 1999). More recently, Holmes and Adams found that in a group of typically developing 8- and 9-year-olds, the central executive and the visuo-spatial sketchpad, but not the phonological loop scores, predicted overall curriculum-based mathematics achievement (Holmes & Adams, 2006). Interestingly, this study also found that for 8-year-olds, the visuo-spatial sketchpad was a stronger predictor of mathematics performance than the central executive. Similarly, Gathercole and Pickering (Gathercole & Pickering, 2000) found that 6- and 7-year-old children’s performance on national curriculum mathematics assessments correlate with performance on measures of visuo-spatial WM.
1.3. Current study
Taken together, these studies suggest that WM plays an important role in both mathematical performance and skill development in 7- to 11-year-old children. Current data also hint at the changing role of different WM components in relation to performance and skill development. However, the research to date has been contradictory; some studies implicate the phonological loop, others the visuo-spatial sketchpad, and still others the central executive (Henry & MacLean, 2003; Holmes & Adams, 2006) There are several reasons for such inconsistencies. The first is related to the large age-range across studies (Adams et al., 1998; Andersson, 2007; Durand, Hulme, Larkin & Snowling, 2005; Holmes & Adams, 2006; Swanson, 2006), resulting in high variability in the level of the participants’ mathematical competence and in the curricular content of the mathematical tasks. To address these issues, we focus on two groups of children in the 2nd and 3rd grades who are at an important stage in formal mathematical skill development.
A second reason for the inconsistencies in findings is the large variability in the types of tasks used to assess mathematical performance. For example, some studies have focused either on individual arithmetic operations, such as addition (Adams & Hitch, 1997), subtraction (Barrouillet, Mignon & Thevenot, 2008) or other more complex arithmetic problems (Henry & MacLean, 2003). The use of a single measure of mathematical ability is useful for studying particular cognitive questions, but is less useful for revealing more general links between WM and developmentally relevant mathematical abilities. To obtain a more complete and ecologically valid profile of mathematics competence, we administered two standardized mathematics achievement measures — the Numerical Operations and Mathematical Reasoning subtests of the Wechsler Individual Achievement Test (WIAT-II; (Wechsler, 2001)). A key distinction between the two measures is that Numerical Operations plays a greater emphasis on counting and computation whereas Mathematical Reasoning emphasizes word problems.
A third reason for inconsistencies across studies is the use of non-standardized instruments to assess WM. We use the Working Memory Test Battery for Children (WMTB-C), a comprehensive, standardized assessment of three core WM components (Pickering & Gathercole, 2001). Importantly, large-scale studies have found that the three-component model of WM best fits empirical data on the structure and development of WM in 6- to 16-year-old children (Gathercole, Pickering, Ambridge & Wearing, 2004). Additionally, WM capacity can be expressed both in terms of raw and standardized scores on each of the three WM components. Raw scores reflect age-related differences, whereas age-normed scores are useful in assessing performance differences after controlling for normative development. Accordingly, we used both raw and age-normed scores from the standardized WMTB-C measure to examine how each WM component influences mathematical abilities assessed by the Numerical Operations and Mathematics Reasoning. Based on previous research on 2nd and 3rd graders’ strategy use (Geary et al., 2004; Wu et al., 2008) we hypothesized that 2nd graders would rely more on the central executive compared to the 3rd graders because of the greater use of counting and other algorithmic strategies, whereas 3rd graders would rely more on automated retrieval processes that do not require the central executive to the same extent as 2nd graders.
2. Methods
2.1. Participants
Participants were recruited from a wide range of schools in the San Francisco Bay Area using mailings to schools, newspaper advertisements and postings at libraries. Participants include 48 2nd graders (17 girls, 31 boys) between the ages of 7 to 8.4 (M=7.59 years; SD=0.52), and 50 3rd graders, 24 girls, 26 boys) between the ages of 7.8 and 9.3 (M=8.52 years; SD=0.40). Participants were administered a demographic questionnaire and IQ was assessed using the Wechsler Abbreviated Scales of Intelligence (Weschler, 1999). As a part of the screening process, participants completed the Child Behavioral Checklist (Achenbach, 1991) to rule out behavioral and emotional problems. Participants with full-scale IQ between 80 and 120, who did not demonstrate signs of behavioral or emotional problems were selected for this study. All tests were administered in one session that lasted about 2 hours.
2.2. Standardized measures
2.2.1. Mathematical abilities
The WIAT-II was used to assess mathematical abilities. This achievement battery includes nationally standardized measures of children’s (grades K-12) academic skills and problem-solving abilities (Wechsler, 2001). The Numerical Operations subtest is a paper-and-pencil test that measures the ability to identify and write numbers, rote counting, number production, and solve written calculation problems and simple equations that require the child to draw from the basic operations of addition, subtraction, multiplication and division. The Mathematical Reasoning subtest is a verbal problem solving test that measures the ability to count, identify geometric shapes, and solve single- and multistep word problems. For example, items present problems in terms of time, money, and measurement with both verbal and visual prompts. The child is required to solve problems with whole numbers, fractions or decimals, interpret graphs, identify mathematical patterns, and solve problems of statistics and probability.
2.2.2. Working memory
Four subtests of the WMTB-C (Pickering & Gathercole, 2001) were used: Counting Recall, Backward Digit Recall, Digit Recall, and Block Recall. All of the subtests have six items at span levels ranging from one to six to one to nine. Passing four items at one level moves the child to the next. At each span level, the number of items to be remembered is increased by one. Failing three times at a span level terminates the subtest.
2.2.2.1. Central executive
Two central executive subsets were administered, Counting Recall and Backward Digit Recall. Counting Recall requires the child to count a set of 4, 5, 6, or 7 dots on a card, and then to recall the number of counted dots at the end of a series of cards. With Backward Digit Recall, the experimenter states a string of number words and the child repeats them in reverse order.
2.2.2.2. Phonological loop
Digit Recall was used to assess the phonological loop. The task requires the child to repeat in the same order a string of number words spoken by the experimenter.
2.2.2.3. Visuo-spatial sketchpad
Block Recall was used to assess the visuo-spatial sketchpad. The stimuli consist of a board with nine raised blocks in what appears to the child as a random arrangement. The blocks have numbers on one side that can only be seen by the experimenter. The experimenter taps a block (or series of blocks), and the child’s task is to duplicate the tapping in the same order as the experimenter.
3. Results
3.1. IQ, WIAT-II and WMTB-C in 2nd and 3rd graders
Although there was some overlap in the ages of the 2nd and 3rd graders, the two groups differed significantly in age (p<0.0001). There were no differences between the verbal IQ, performance IQ, and full-scale IQ scores of 2nd and 3rd graders (FSIQ M=108, SD=11.39, respectively), as shown in Table 1, suggesting comparable samples. As might be expected, Mathematical Reasoning and Numerical Operations raw scores were significantly higher in 3rd graders than 2nd graders, but there were no grade-level differences for age-normed scores (Table 1).
Table 1.
Comparison of WASI, WIAT-II and WMBT-C scores in 2nd and 3rd graders.
| Measure | Grade |
t-test |
||||
|---|---|---|---|---|---|---|
| 2nd (n=48) |
3rd (n=50) |
t-score | p | |||
| M | SD | M | SD | |||
| WASI | ||||||
| Verbal | 110.79 | 14.18 | 108.78 | 12.85 | 0.73 | 0.46 |
| Performance | 105.87 | 14.65 | 106.98 | 13.32 | 0.39 | 0.69 |
| Full | 108.93 | 11.39 | 108.68 | 11.73 | 0.11 | 0.91 |
| WIAT-II | ||||||
| Numerical Operations | 14.10 | 3.40 | 19.56 | 5.15 | 6.12 | 0.001 |
| Math Reasoning | 32.43 | 5.96 | 35.68 | 6.84 | 2.49 | 0.01 |
| WMTB-C | ||||||
| Counting Recall | 17.50 | 5.33 | 18.64 | 5.68 | 1.02 | 0.30 |
| Backward Digit Recall | 10.91 | 3.31 | 12.40 | 3.79 | 2.05 | 0.04 |
| Digit Recall | 29.14 | 5.25 | 29.74 | 5.02 | 0.57 | 0.56 |
| Block Recall | 23.02 | 3.40 | 23.76 | 3.72 | 1.02 | 0.30 |
Verbal, Performance and Full-Scale IQ were assessed using the Wechsler Abbreviated Scale of Intelligence (WASI). Numerical Operations and Math Reasoning were assessed using the Wechsler Individual Aptitude Test (WIAT-II). Counting Recall, Backward Recall, Digit Recall and Block Recall were used to assess the central executive, phonological loop and visuo-spatial sketchpad components of Working Memory, based on the Working Memory Test Battery for Children (WMTB-C). Raw scores were used for all Math Achievement and WM measures. Mean (M), Standard Deviation (SD) and results of t-tests comparing 2nd and 3rd graders are shown for each measure.
From WMBT-C subscales, only the raw Backward Digit Recall WMBT-C score was significantly different between 2nd and 3rd graders (t(1)=2.05, p<0.05). Once the scores were normed based on age, WM scores were not significantly different on any measure across grades (Table 2).
Table 2.
Comparison of age-normed WIAT-II and WMBT-C scores in 2nd and 3rd graders.
| Measure | Grade |
t-test |
||||
|---|---|---|---|---|---|---|
| 2nd (n=48) |
3rd (n=50) |
t-score | p | |||
| M | SD | M | SD | |||
| WASI | ||||||
| Numerical Operations | 103.66 | 14.37 | 105.00 | 13.88 | 0.46 | 0.64 |
| Math Reasoning | 112.16 | 14.10 | 106.76 | 13.42 | −1.94 | 0.06 |
| WMTB-C | ||||||
| Counting Recall | 95.08 | 16.35 | 90.34 | 19.09 | 1.31 | 0.19 |
| Backward Recall | 96.50 | 16.53 | 98.80 | 15.97 | −0.70 | 0.48 |
| Digit Recall | 111.52 | 18.70 | 107.98 | 16.97 | 0.98 | 0.32 |
| Block Recall | 95.54 | 15.36 | 94.40 | 15.01 | 0.37 | 0.71 |
Verbal, Performance and Full-Scale IQ were assessed using the Wechsler Abbreviated Scale of Intelligence (WASI). Numerical Operations and Math Reasoning were assessed using the Wechsler Individual Aptitude Test (WIAT-II). Counting Recall, Backward Recall, Digit Recall and Block Recall were used to assess the central executive, phonological loop and visuo-spatial sketchpad components of Working Memory, based on the Working Memory Test Battery for Children (WMTB-C). Age-normed scores were used for all Math Achievement and WM measures. Mean (M), Standard Deviation (SD) and results of t-tests comparing 2nd and 3rd graders are shown for each measure.
3.2. Relation between working memory and mathematics achievement
The relation between WM and mathematical achievement was first assessed with linear regression in 2nd and 3rd grade children (Table 3). As shown in Fig. 1 and Table 3, Counting Recall was a significant predictor of Mathematical Reasoning scores in 2nd grade but not 3rd grade. Backward Digit Recall did not predict Numerical Operations or Mathematical Reasoning scores in either grade.
Table 3.
Relation between WIAT-II math scores and working memory in 2nd and 3rd graders.
| WM component | Grade |
|||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 2nd Grade |
3rd Grade |
|||||||||
| B | SE (B) | β | R2 | p | B | SE (B) | β | R2 | p | |
| Numerical Operations | ||||||||||
| Counting Recall | 0.09 | 0.09 | 0.14 | 0.02 | 0.31 | 0.14 | 0.12 | 0.16 | 0.02 | 0.25 |
| Backward Digit Recall | 0.04 | 0.15 | 0.04 | 0.00 | 0.76 | 0.32 | 0.19 | 0.24 | 0.05 | 0.09 |
| Digit Recall | 0.03 | 0.09 | 0.05 | 0.00 | 0.73 | 0.12 | 0.14 | 0.11 | 0.01 | 0.41 |
| Block Recall | 0.01 | 0.15 | 0.01 | 0.00 | 0.94 | 0.54 | 0.18 | 0.39 | 0.15 | 0.005 |
| Math Reasoning | ||||||||||
| Counting Recall | 0.50 | 0.14 | 0.44 | 0.20 | 0.001 | 0.15 | 0.17 | 0.13 | 0.01 | 0.35 |
| Backward Digit Recall | −0.11 | 0.26 | 0.06 | 0.00 | 0.65 | 0.20 | 0.25 | 0.11 | 0.01 | 0.44 |
| Digit Recall | 0.41 | 0.15 | 0.36 | 0.13 | 0.01 | 0.19 | 0.19 | 0.14 | 0.02 | 0.31 |
| Block Recall | −0.14 | 0.25 | 0.08 | 0.00 | 0.58 | 0.62 | 0.24 | 0.33 | 0.11 | 0.01 |
Linear regression analysis was used to assess the relation between Numerical Operations and Math Reasoning components of the WIAT-II and each of the four working memory (WM) components of the WMTB-C in each grade. Similar results were observed with age-normed scores, as described in the text. Other details as in Table 1.
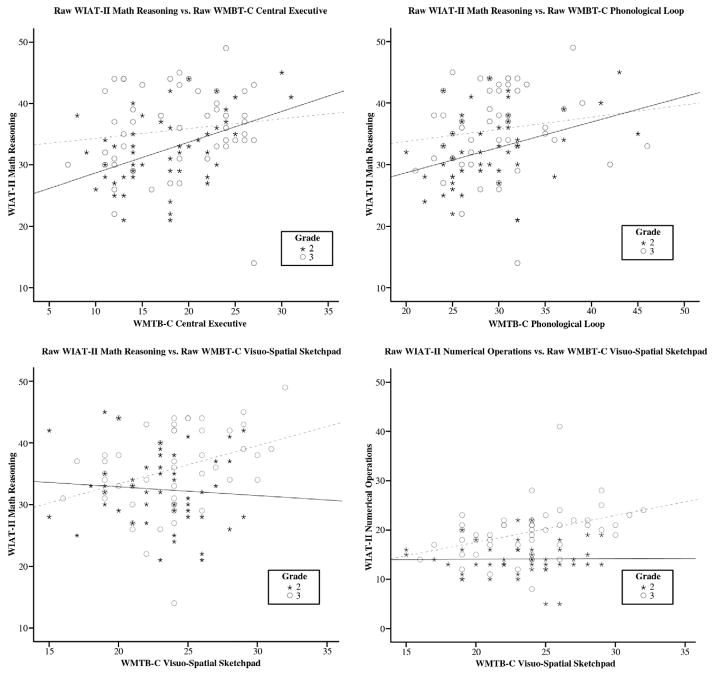
Fig. 1.
Relation between WIAT-II math scores and working memory components. Top row: In 2nd graders, WIAT-II Mathematical Reasoning scores were significantly correlated with the central executive and phonological loop. Bottom row: In 3rd graders, both WIAT-II Mathematical Reasoning and Numerical Operations scores were correlated with the visuo-spatial sketchpad. The solid regression line is for data from 2nd graders and dashed line is for data from 3rd graders. Analysis based on raw scores for both WIAT-II and working memory.
Digit Recall was a significant predictor of Mathematical Reasoning scores in 2nd grade (, but again not in 3rd grade, as shown in Fig. 1 and Table 3. Digit Recall did not predict Numerical Operations scores in either grade.
Block Recall did not predict Mathematical Reasoning or Numerical Operations scores of 2nd graders. For 3rd graders, Block Recall predicted both Numerical Operations, and Mathematical Reasoning, as shown in Table 3 and Fig. 1. The corresponding slopes differed significantly across grades for both Numerical Operations (standardized β = −1.221; t(1) = −2.214; p<0.05) and Mathematical Reasoning (standardized β = −1.364; t(1)=2.116; p<0.05).
There were two 2nd graders whose Digit Recall scores were three standard deviations above the mean score. We reanalyzed our data without these two subjects. There were no changes in any of the results noted above. No other WM or WIAT scores were identified as outliers.
To test whether gender was a significant predictor, we used hierarchical regression analysis to test the three-way interaction between working memory measures, math performance, and gender. Including gender as a covariate did not alter any of our findings.
We then conducted additional analyses using age-normed WMTB-C and WIAT-II scores. Linear regression analysis using age-normed scores showed similar relationships as raw scores. In 2nd graders, Counting Recall (standardized β = 0.42; p<0.01; R2=0.18) and Digit Recall (standardized β = 0.29; p<0.05; R2=0.08) predicted Mathematical Reasoning. In 3rd graders, Block Recall predicted Numerical Operations Mathematical Reasoning (standardized β = 0.50; p<0.001; R2=0.25) and Mathematical Reasoning (standardized β = 0.31; p<0.05; R2=0.09).
3.3. Hierarchical regression analysis of mathematics achievement and working memory
Hierarchical regression analyses were used to examine the unique and incremental contributions of the various components of WM to performance on the Numerical Operations and Mathematical Reasoning subtests; the order of entry of the predictors was based on the magnitude of the Pearson correlation between the WM subtest and the mathematics subtest scores. The order of predictors of 2nd grade Mathematical Reasoning scores was Counting Recall (r=0.44; p<0.01), Digit Recall (r=0.36, p<0.05), Block Recall (r=−0.08, p=0.724), and Backward Digit Recall (r=0.06; p=0. 87). The order of predictors of 3rd grade Mathematical Reasoning scores was Block Recall (r=0.33; p<0.05), Digit Recall (r=0.14; p=0.314), Counting Recall (r=0.13; p=0.359), and Backward Digit Recall (r=0.11; p=0.442). The order for Numerical Operations for 3rd graders was Block Recall (r=0.39; p<0.01), Backward Digit Recall (r=0.24; p=0.096), Counting Recall (r=0.16; p=0.256), and Digit Recall (r=0.11; p=0.413).
As shown in Table 4, Counting Recall contributed to 20% of the variance of 2nd graders Mathematical Reasoning scores (p<0.01), but the incremental contributions of Digit Recall, Backward Digit Recall, and Block Recall were not significant, although Digit Recall showed a trend towards significance (p=0.05). In 3rd graders’ Mathematical Reasoning scores, Block Recall accounted for 11% of the variance (p<0.05), but the incremental contributions of Digit Recall, Backward Digit Recall, and Counting Recall were not significant. Block Recall also contributed a significant 16% of the variance in 3rd graders’ Numerical Operations scores (p<0.05); again, the incremental contributions of Counting Recall, Backward Digit Recall, and Digit Recall were not significant predictors.
Table 4.
Hierarchical regression analysis of WIAT-II math scores and working memory in 2nd and 3rd graders.
| R2 | B | SE (B) | β | t | p | |
|---|---|---|---|---|---|---|
| Model 1 | ||||||
| 2nd graders’ Math Reasoning | ||||||
| Counting Recall | 0.20 | 0.45 | 0.15 | 0.40 | 2.87 | 0.006 |
| Digit Recall | 0.25 | 0.33 | 0.16 | 0.29 | 1.98 | 0.05 |
| Block Recall | 0.26 | −0.16 | 0.23 | −0.09 | −0.69 | 0.48 |
| Backward Digit Recall | 0.31 | −0.40 | 0.24 | −0.22 | −1.64 | 0.10 |
| Model 2 | ||||||
| 3rd graders’ Math Reasoning | ||||||
| Block Recall | 0.11 | 0.60 | 0.27 | 0.32 | 2.19 | 0.03 |
| Digit Recall | 0.12 | 0.11 | 0.19 | 0.08 | 0.56 | 0.57 |
| Backward Digit Recall | 0.12 | −0.13 | 0.31 | −0.07 | −0.43 | 0.66 |
| Counting Recall | 0.12 | 0.11 | 0.19 | 0.09 | 0.60 | 0.55 |
| Model 3 | ||||||
| 3rd graders’ Numerical Operations | ||||||
| Block Recall | 0.15 | 0.48 | 0.20 | 0.35 | 2.41 | 0.02 |
| Backward Digit Recall | 0.16 | 0.12 | 0.23 | 0.09 | 0.54 | 0.58 |
| Counting Recall | 0.17 | 0.04 | 0.14 | 0.04 | 0.29 | 0.77 |
| Digit Recall | 0.17 | 0.01 | 0.14 | 0.01 | 0.08 | 0.93 |
Hierarchical regression analysis was used to assess the effect of each of the four working memory (WM) components of the WMTB-C in each grade. Similar results were observed with age-normed scores, as described in the text. R2 values indicate initial and incremental variance explained. Other details as in Table 1.
In order to further examine the relation between WM and math achievement, we conducted additional analyses using age-normed measures of the WMTB-C and WIAT-II. Counting Recall predicted 18% of the variance (p<0.01) of 2nd graders’ Mathematical Reasoning, but the incremental contributions of Digit Recall, Backward Digit Recall, and Block Recall were not significant. In 3rd graders, Block Recall predicted 25% of the Numerical Operations (p<0.001) and 9% of the variance in Mathematical Reasoning (p<0.07) and; Counting Recall, Backward Digit Recall, and Digit Recall were not significant predictors.
4. Discussion
The purpose of our study was to clarify how the three WM systems differentially contribute to 2nd and 3rd grade children’s performance on standardized measures that assess basic number, counting, and arithmetic competencies (Numerical Operations) and more complex problem solving competencies (Mathematical Reasoning). We hypothesized that the central executive would more strongly predict 2nd graders’ than 3rd graders’ performance on both mathematics measures. We found that the central executive and the phonological loop significantly predicted performance on the Mathematical Reasoning scores of the WIAT-II in 2nd graders. For 3rd grade children, however, the central executive and the phonological loop were not correlated with either of the mathematics measures; instead, visuo-spatial sketchpad scores significantly predicted performance on both measures. Importantly, both raw and age-normed scores showed an identical profile of relations between WM and mathematics achievement, pointing to the robustness of our findings.
4.1. Developmental and instructional changes in mathematical competence and WM
Between 2nd and 3rd grade there were significant improvements in the Mathematical Reasoning and Numerical Operations components of the WIAT-II, but no changes in either the raw or the age-normed WM scores. The only exception was for the raw Backward Digit Recall test, which is not surprising as this central executive task is considerably more demanding than our other central executive task, Counting Recall. The overall pattern suggests that the window between 2nd and 3rd grades is too short a time frame for major changes in WM capacity. In spite of the lack of significant improvements in WM, there were changes in the contributions of WM components to the improvement in mathematical competence in 2nd and 3rd graders. Backward Digit Recall (raw scores), the only WM subscale that showed developmental changes across grades, was also the only WM measure that did not predict any of the mathematics performance scores. Critically, this profile allows us to rule out the possibility that differences in the observed relationships between mathematical abilities and specific WM components arise from developmental changes in WM capacity. Rather, because the observed relationships between mathematical abilities and specific WM components are virtually identical for raw and age-normed scores, they likely reflect changes in mathematical skill arising from instruction and practice.
4.2. Central executive and phonological loop
We confirmed our hypothesis that the central executive best predicts mathematics performance in 2nd graders. However, the central executive did not predict mathematics performance in 3rd graders. Further, hierarchical regression analysis was consistent with these results and confirmed that Counting Recall accounted for a significant fraction (p<0.01) of the variance in Mathematical Reasoning scores, while the incremental contributions of other WM components was not significant (p>0.3). It is noteworthy that no significant relation was found with Backward Digit Recall, our second measure of the central executive. One crucial difference between Backward Digit Recall and Counting Recall is that the former requires manipulation of information in WM, whereas the latter only requires maintaining information in WM. Our data suggest that Mathematical Reasoning in 2nd graders (as assessed by the WIAT and similar standardized tests) may rely more crucially on maintenance, rather than manipulation, of information in WM. Further studies are needed to disentangle the manner in which specific subcomponents of the central executive influence development of mathematical skills in children.
Our findings are consistent with several previous studies which have implicated the central executive in mathematical problem-solving in 7- to 12-year-old children (Adams & Hitch, 1997; Geary et al., 2004; Swanson, 2006; Swanson & Beebe-Frankenberger, 2004; Swanson, Cooney & Brock, 1993). Our results extend the findings of Swanson (2006) who found that maturation in the central executive was an important predictor of one-year longitudinal changes in children’s problem solving abilities in a sample of 1st, 2nd and 3rd graders. Swanson also showed that, in a combined 1st and 2nd grade group, central executive ability predicted mathematics performance on both Numerical Operations and Mathematical Reasoning one year after initial testing and that the central executive was the strongest predictor of mathematics skills even after accounting for phonological and visuo-spatial sketchpad capacities. These studies have, however, not examined the changing role of the central executive in mathematics achievement as learning progresses in the early grade levels. In this regard, our findings relate most closely to a study by Henry and Maclean (2003) who observed that in 7- to 8-year-old children, arithmetic reasoning ability was best predicted by memory measures tapping the central executive. In 11- to 12-year-old children, on the other hand, arithmetic reasoning was not predicted by the central executive. Our study identifies the interval between the 2nd (mean age=7.59) and 3rd grades (mean age 8.52) as an important period for this shift.
Mathematical skill development during this period is characterized by significant changes as children learn to rely less on finger and verbal counting to solve arithmetic problems, and shift to more complex procedural strategies and automatic retrieval of mathematical facts from long-term memory. The development of long-term storage of arithmetic facts depends on consistent pairing of numbers and operations with their associated responses as children are solving math problems through execution of counting strategies (Siegler, 1996). An important function of the central executive during early stages of mathematics skill acquisition may be to guide the use of counting strategies that young children typically use to solve arithmetic problems. Consistent with our findings, in a study of 1st, 3rd, and 5th grade children Geary et al. found that counting span (a CE measure) predicted use of finger and verbal counting in 1st graders, but not in older children (D. Geary et al., 2004). Digit Recall, a measure of the phonological capacity, was also correlated with Mathematical Reasoning abilities in 2nd grade, but not 3rd grade, children. The contribution of the phonological loop was weaker than that of the central executive. Nevertheless, the co-dependence on the central executive and the phonological loop is noteworthy because the Mathematical Reasoning subtest of the WIAT-II is comprised of verbal word problems, such as “Sally has a pie, cuts it into four pieces, and gives two pieces to her friend John. How many pieces does Sally have now?”. We suggest that the phonological loop and the central executive together facilitate the translation of verbal-codes in word problems into numeric–symbolic format, and this co-dependence may be most prominent in 2nd graders. We suggest that 3rd graders may extract numerical information more readily, and hence not have to rely on the central executive and the phonological loop to directly transform word problems into their core numerical representation. The results are also consistent with the finding that the solution of word problems, and especially multi-step word problems, which would be more frequent among the 3rd graders than 2nd graders, is often facilitated by use of visual–spatial representations (Geary, 1994). Our data instead suggest that 3rd graders may increasingly rely on visual–spatial representations to solve such problems.
4.3. Visuo-spatial sketchpad
In conjunction with the decreasing dependence on the central executive and the phonological loop in the 2nd grade, we found that dependence on the visuo-spatial sketchpad increases in the 3rd grade. In our 3rd grade sample, visuo-spatial sketchpad capacity was the only WM measure that significantly predicted mathematics achievement. We suggest that these changes reflect a greater reliance on visuo-spatial representations as a result of greater facility with numeric–symbolic representations of mathematics problems and increased practice in mathematical problem solving. Hierarchical regression analysis confirmed that Block Recall accounted for a significant fraction of the variance in Numerical Operations and Mathematical Reasoning, but incremental contributions of Counting Recall, Backward Digit Recall, and Digit Recall were not significant.
These results are consistent with those of three previous studies. In Swanson’s (2006) longitudinal study of a combined group of 1st, 2nd, and 3rd graders, the central executive, measured in Year 1, predicted word problem solving abilities in Year 2, whereas visuo-spatial sketchpad capacity in Year 2 was a significant predictor of math calculation skills in Year 3 (Swanson, 2006). In a group of 7- to 10-year-old children, Holmes and Adams (2006) found that the visuo-spatial sketchpad and central executive, but not phonological loop, predicted overall curriculum-based mathematical achievement. Consistent with our findings, Bull et al. found that performance on executive functioning and phonological WM tasks in preschoolers predicted later mathematical and reading ability in elementary school students (Bull et al., 2008). However, only visuo-spatial WM and visuo-spatial short-term memory performance in preschool uniquely predict mathematical performance by the end of the third grade. Our study extends these results by showing that greater reliance on visuo-spatial sketchpad emerges between the 2nd and 3rd grades. It is noteworthy that in a recent study, Holmes et al. assessed the contributions of the visual and spatial components of visuo-spatial sketchpad separately, and found that the spatial subcomponent is a better predictor of 7- to 8-year-old children’s mathematical performance, whereas the visual subcomponent is a better predictor of 9- to 10-year-old children’s performance (Holmes, Adams & Hamilton, 2007). Taken together, these studies suggest that the central executive and phonological loop may facilitate initial learning and performance, while visuo-spatial WM and visuo-spatial representations support mathematical performance during later stages.
Beyond these studies, compared to the central executive and the phonological loop, less attention has been paid to the development of visuo-spatial sketchpad and its role in facilitating mathematical problem solving during the early years of schooling (Hitch, 2006). In a study of older children (11- to 12-year-olds), the best predictor of mathematics achievement was the visuo-spatial sketchpad, followed by the phonological loop (Henry & MacLean, 2003). Moreover, Dark and Benbow (1990) found that 12- and 13-year-old children with mathematical talent show superior visuo-spatial sketchpad capacity compared to typically developing peers, and one study has reported that by 15 to 16 years of age, visuo-spatial sketchpad capacity was the only predictor of mathematics performance (Reuhkala, 2001). These effects appear to be developmentally stable over a protracted time frame, given that studies in adults have also reported that visuo-spatial working memory plays an important role in mathematical problem solving (Heathcote, 1994). Although these studies draw from older samples, the results parallel our findings in 3rd graders. Our findings are not only consistent with these studies, but they further suggest a greater role for the visuo-spatial sketchpad at a younger stage in mathematics learning than has been previously acknowledged.
We suggest that the interval between 2nd and 3rd grade is an important stage for a transformation to visuo-spatial representations in problem solving. The importance of the visuo-spatial sketchpad in children’s arithmetic skills can be traced to preschool, reflecting young children’s reliance on visuo-spatial representations of number and quantity for elementary tasks (Rasmussen & Bisanz, 2005). Furthermore, one recent study found that performance on two standardized tests of math achievement (the TEMA-2 and Woodcock-Johnson Calculation subtest) acquired in 6- to 11-year-old children was strongly correlated with their performance on a basic approximate number sense task at age 14 (Halberda, Mazzocco & Feigenson, 2008). Taken together, these findings suggest that the development of mathematical problem solving skills relies in part on visual problem representations.
4.4. Convergence with neurocognitive studies
The increased reliance on the visuo-spatial representations is consistent with neurocognitive studies that have provided evidence for a shift from reliance on prefrontal cortex functions to those mediated by the parietal cortex with increased mathematical skill acquisition. With development (Rivera, Reiss, Eckert & Menon, 2005), as with extended practice in adults (Ischebeck, Zamarian, Egger, Schocke & Delazer, 2007; Ischebeck et al., 2006), there is a shift from central executive processes subserved by the prefrontal cortex to more specialized mechanisms in the posterior parietal cortex.
Critically, a wide range of brain imaging and lesion studies have underscored the crucial role of the posterior parietal cortex, including the IPS region, more dorsally, and the angular gyrus, more ventrally, in efficient math performance. The posterior parietal cortex plays a more crucial role in number processing and fact retrieval as well as low-level computation (Ansari, 2008), while the prefrontal cortex is responsible for cognitive sequencing, executive control, decision-making and attention processes needed when more complex computation is required (Menon, Rivera, White, Glover & Reiss, 2000). The degree to which these processes are engaged may depend on individual proficiency and learning. Our findings suggest that 2nd graders are likely to engage the prefrontal cortex more as they perform these tasks because of greater reliance on the central executive and phonological rehearsal, especially in more proficient and high-performing children. In 3rd graders, a different pattern is suggested by our findings, with greater reliance on the visuo-spatial processes, again to greater extent in more proficient and high-performing children. Visuo-spatial representations that link to core magnitude systems in the parietal cortex are one mechanism by which mathematical skill development can occur. A shift away from central executive mechanisms frees the prefrontal cortex from low-level computation and thus makes available valuable processing resources for more complex problem solving and reasoning. Our study identifies the period between 2nd and 3rd grades as an important window in which such transformations might occur. The extent to which these changes depend on the development of spatial representations of number (Holloway & Ansari, 2008; Kucian, von Aster, Loenneker, Dietrich & Martin, 2008; Siegler & Opfer, 2003) remains to be investigated.
4.5. Implications for individual differences and learning
Our findings have important implications for understanding individual differences in mathematics performance. Previous research in children with poor mathematical skills has suggested distinctions between the three WM components and how they correlate with performance at different grades. The most consistent links with WM impairments are found with the central executive (Geary & Brown, 1991; Geary et al., 1999, 2000; Hitch & McAuley, 1991; McLean & Hitch, 1999; Passolunghi & Siegel, 2001; Siegel & Ryan, 1989; Swanson, 1993; Swanson & Sachse-Lee, 2001; Wilson & Swanson, 2001). Findings have been more mixed in the case of the visuo-spatial sketchpad, with some studies suggesting visuo-spatial sketchpad related impairments (D’Amico & Guarnera, 2005; McLean & Hitch, 1999; Passolunghi & Pazzaglia, 2005; Reuhkala, 2001; van der Sluis et al., 2005) whereas others have not found any consistent relationship (Bull et al., 1999; Geary et al., 2000). Similarly, studies of the phonological loop have been contradictory, and its role in poor mathematics learning is still debated (Bull et al., 1999; Geary & Brown, 1991; Geary et al., 1999, 2000; Hitch & McAuley, 1991; Landerl, Bevan & Butterworth, 2004; McLean & Hitch, 1999; Swanson & Sachse-Lee, 2001). Our findings suggest that in 2nd graders, in addition to the central executive, the phonological loop can also contribute to poor mathematics outcomes in reasoning tasks which require significant verbal processing. The impact on tasks which primarily taps symbolic fact retrieval is weaker, suggesting that poor performance on complex reasoning problems require good phonological skills, at least in the 2nd grade at a stage when children are beginning to be exposed to these types of problems (Jordan, Hanich & Kaplan, 2003). In children with poor central executive and phonological capacity, it is likely that math skills do not develop appropriately and these factors may continue to influence their performance in the 3rd grade. This is an important research question that the cross-sectional sample used in our study cannot address. Further research with longitudinal samples is required to address this issue.
4.6. Conclusion
Between 2nd and 3rd grades, there were significant changes in mathematics achievement, as assessed by the Numerical Operations and Mathematical Reasoning subtests of the WIAT-II. In comparison, changes in WM were weak — only Backward Digit Recall showed developmental changes related to WM but this measure did not predict mathematics performance. Taken together, these results ensure that the contributions of WM to math performance observed in our study are independent of developmental changes in WM capacity. Critically, we identified the period between 2nd (mean age 7.59) and 3rd grades (mean age 8.52) as an important period for a shift in the differential roles of specific WM components to mathematics achievement. Our data suggest that the central executive and phonological loop play a more important role in facilitating performance during the early stages of learning, and that their role diminishes with exposure and learning. In contrast, the visuo-spatial sketchpad plays an increasingly important role during the later stages of learning, suggesting a shift to an increasing role for visuo-spatial representations in mathematics problem solving. These changes may mirror increasing use of more specialized posterior parietal cortex mechanisms and decreasing use of prefrontal cortex mechanisms as facility with math problem solving develops and matures in this period (Rivera et al., 2005). The changing role of WM components in mathematics performance identified here may be useful in remediating poor math skills in young children (Fuchs et al., 2005) and in early identification of children at risk for mathematical learning disabilities.
Acknowledgments
We thank Kevin Holmes and Katherine Keller for assistance with data acquisition. This research was supported by grants from the National Institutes of Health (NICHD-HD047520 and NICHD-HD059205) and the National Science Foundation (BCS/DRL-075034) to VM. DG acknowledges support from grants from NICHD-HD38283 and NICHD-HD045914, co-funded by NIH and the Office of Special Education and Rehabilitation Services.
References
- Achenbach TM. Manual for the Child Behavior Checklist/4–18 and 1991 Profile. Burlington: University of Vermont, Department of Psychiatry; 1991. [Google Scholar]
- Adams JW, Hitch GJ. Working memory and children’s mental addition. Journal of Experimental Child Psychology. 1997;67(1):21–38. doi: 10.1006/jecp.1997.2397. [DOI] [PubMed] [Google Scholar]
- Adams JW, Hitch GJ, Donlan C. Children’s mental arithmetic and working memory. In: , editor. The development of mathematical skills. Studies in developmental psychology. Psychology Press/Taylor & Francis; (UK), Hove, England: 1998. pp. 153–173. [URL: http://www.psypress.co.uk] [Google Scholar]
- Andersson U. The contribution of working memory to children’s mathematical word problem solving. Applied Cognitive Psychology. 2007;21:1201–1216. [Google Scholar]
- Ansari D. Effects of development and enculturation on number representation in the brain. Nature Reviews. Neuroscience. 2008;9(4):278–291. doi: 10.1038/nrn2334. [DOI] [PubMed] [Google Scholar]
- Ashcraft MH. Cognitive arithmetic: A review of data and theory. Cognition. 1992;44:75–106. doi: 10.1016/0010-0277(92)90051-i. [DOI] [PubMed] [Google Scholar]
- Baddeley A. Working memory. Vol. 11. New York, NY: Clarendon Press/Oxford University Press; 1986. [Google Scholar]
- Baddeley AD. Exploring the central executive. Quarterly Journal of Experimental Psychology. 1996;49A:5–28. [Google Scholar]
- Baddeley A, Emslie H, Kolodny J, Duncan J. Random generation and the executive control of working memory. Quarterly Journal of Experimental Psychology A. 1998;51(4):819–852. doi: 10.1080/713755788. [DOI] [PubMed] [Google Scholar]
- Baddeley AD, Hitch GJ, Bower GA. Working memory. In: , editor. The psychology of learning and motivation: Advances in research and theory. Vol. 8. New York: Academic Press; 1974. pp. 47–89. [Google Scholar]
- Baddeley AD, Logie RH. Working memory: The multi-component model. In: Miyake A, Priti S, editors. Models of working memory: Mechanisms of active maintenance and executive control. Cambridge: Cambridge University Press; 1987. pp. 28–54. [Google Scholar]
- Barrouillet P, Lepine R. Working memory and children’s use of retrieval to solve addition problems. Journal of Experimental Child Psychology. 2005;91(3):183–204. doi: 10.1016/j.jecp.2005.03.002. [DOI] [PubMed] [Google Scholar]
- Barrouillet P, Mignon M, Thevenot C. Strategies in subtraction problem solving in children. Journal of Experimental Child Psychology. 2008;99(4):233–251. doi: 10.1016/j.jecp.2007.12.001. [DOI] [PubMed] [Google Scholar]
- Bull R, Epsy K, Wiebe S. Short-term memory, working memory, and executive functioning in preschoolers: Longitudinal predictors of mathematical achievement at age 7 years. Developmental Neuropsychology. 2008;33(3):205–208. doi: 10.1080/87565640801982312. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bull R, Johnston RS, Roy JA. Exploring the roles of the visual–spatial sketch pad and central executive in children’s arithmetical skills: Views from cognition and developmental neuropsychology. Developmental Neuropsychology. 1999;15(3):421–442. [Google Scholar]
- D’Amico A, Guarnera M. Exploring working memory in children with low arithmetical achievement. Learning and Individual Differences. 2005;15(3):189–202. [Google Scholar]
- Dark VJ, Benbow CP. Enhanced problem translation and short-term memory: Components of mathematical talent. Journal of Educational Psychology. 1990;82:420–429. [Google Scholar]
- De Rammelaere S, Stuyven E, Vandierendonck A. The contribution of working memory resources in the verification of simple mental arithmetic sums. Psychological Research. 1999;62(1):72–77. [Google Scholar]
- De Rammelaere S, Stuyven E, Vandierendonck A. Verifying simple arithmetic sums and products: Are the phonolgical loop and the central executive involved? Memory & Cognition. 2001;29:267–273. doi: 10.3758/bf03194920. [DOI] [PubMed] [Google Scholar]
- Durand M, Hulme C, Larkin R, Snowling M. The cognitive foundations of reading and arithmetic skills in 7- to 10-year-olds. Journal of Experimental Child Psychology. 2005;91:113–136. doi: 10.1016/j.jecp.2005.01.003. [DOI] [PubMed] [Google Scholar]
- Frensch PA, Geary DC. Effects of practice on component processes in complex mental addition. Journal of Experimental Psychology. Learning, Memory, and Cognition. 1993;19(2):433–456. doi: 10.1037//0278-7393.19.2.433. [DOI] [PubMed] [Google Scholar]
- Fuchs LS, Compton DL, Fuchs D, Paulsen K, Bryant JD, Hamlett CL. The prevention, identification, and cognitive determinants of math difficulty. Journal of Educational Psychology. 2005;97(3):493–513. [Google Scholar]
- Furst AJ, Hitch GJ. Separate roles for executive and phonological components of working memory in mental arithmetic. Memory & Cognition. 2000;28(5):774–782. doi: 10.3758/bf03198412. [DOI] [PubMed] [Google Scholar]
- Gathercole SE, Adams AM. Children’s phonological working memory: Contributions of long-term knowledge and rehearsal. Journal of Memory and Language. 1994;33(5):672–688. [Google Scholar]
- Gathercole S, Pickering S. Assessment of working memory in six- and seven-year-old children. British Journal of Educational Psychology. 2000;70(2):177–194. doi: 10.1348/000709900158047. [DOI] [PubMed] [Google Scholar]
- Gathercole SE, Pickering SJ, Ambridge B, Wearing H. The structure of working memory from 4 to 15 years of age. Developmental Psychology. 2004;40(2):177–190. doi: 10.1037/0012-1649.40.2.177. [DOI] [PubMed] [Google Scholar]
- Geary DC. A componential analysis of an early learning deficit in mathematics. Journal of Experimental Child Psychology. 1990;49(3):363–383. doi: 10.1016/0022-0965(90)90065-g. [DOI] [PubMed] [Google Scholar]
- Geary DC. Children’s mathematical development: Research and practical applications. Washington, DC: American Psychological Association; 1994. [Google Scholar]
- Geary DC, Bow-Thomas C, Liu F, Siegler RS. Development of arithmetical competencies in Chinese and American children: Influence of age, language, and schooling. Child Development. 1996;67(5):2022–2044. [PubMed] [Google Scholar]
- Geary DC, Brown SC. Cognitive addition: Strategy choice and speed-of-processing differences in gifted, normal, and mathematically disabled children. Developmental Psychology. 1991;27:398–406. [Google Scholar]
- Geary DC, Burlingham-Dubree M. External validation of the strategy choice model for addition. Journal of Experimental Child Psychology. 1989;47:175–192. [Google Scholar]
- Geary DC, Frensch PA, Wiley JG. Simple and complex mental subtraction: Strategy choice and speed-of-processing differences in younger and older adults. Psychology and Aging. 1993;8(2):242–256. doi: 10.1037//0882-7974.8.2.242. [DOI] [PubMed] [Google Scholar]
- Geary DC, Hamson CO, Hoard MK. Numerical and arithmetical cognition: A longitudinal study of process and concept deficits in children with learning disability. Journal of Experimental Child Psychology. 2000;77(3):236–263. doi: 10.1006/jecp.2000.2561. [DOI] [PubMed] [Google Scholar]
- Geary D, Hoard M, Byrd-Craven J, DeSoto M. Strategy choices in simple and complex addition: Contributions of working memory and counting knowledge for children with mathematical disability. Journal of Experimental Child Psychology. 2004;88(2):121–151. doi: 10.1016/j.jecp.2004.03.002. [DOI] [PubMed] [Google Scholar]
- Geary DC, Hoard MK, Hamson CO. Numerical and arithmetical cognition: Patterns of functions and deficits in children at risk for a mathematical disability. Journal of Experimental Child Psychology. Special Issue: The Development of Mathematical Cognition: Arithmetic. 1999;74(3):213–239. doi: 10.1006/jecp.1999.2515. [DOI] [PubMed] [Google Scholar]
- Geary DC, Hoard MK, Nugent L, Byrd-Craven J. Development of number line representations in children with mathematical learning disability. Developmental Neuropsychology. 2008;33(3):277–299. doi: 10.1080/87565640801982361. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Halberda J, Mazzocco MM, Feigenson L. Individual differences in non-verbal number acuity correlate with maths achievement. Nature. 2008;455(7213):665–668. doi: 10.1038/nature07246. [DOI] [PubMed] [Google Scholar]
- Heathcote D. The role of visuo-spatial working memory in the mental addition of multi-digit addends. Current Psychology of Cognition. 1994;13:207–245. [Google Scholar]
- Hecht SA. Counting on working memory in simple arithmetic when counting is used for problem solving. Memory & Cognition. 2002;30(3):447–455. doi: 10.3758/bf03194945. [DOI] [PubMed] [Google Scholar]
- Henry L, MacLean M. Relationships between working memory, expressive vocabulary and arithmetical reasoning in children with and without intellectual disabilities. Educational and Child Psychology. 2003;20(3):51–63. [Google Scholar]
- Henry LA, Miller S. Memory span increasee with age: A test of two hypotheses. Journal of Experimental Child Psychology. 1991;51:459–484. [Google Scholar]
- Hitch GJ. Working memory in children: A cognitive approach. In: Bialystok E, Craik F, editors. Lifespan cognition. Oxford: Oxford University Press; 2006. [Google Scholar]
- Hitch GJ, Halliday MS, Schaafstal A, Schraagen JM. Visual working memory in young children. Memory & Cognition. 1988;16:120–132. doi: 10.3758/bf03213479. [DOI] [PubMed] [Google Scholar]
- Hitch GJ, McAuley E. Working memory in children with specific arithmetical learning difficulties. British Journal of Psychology. Special Issue: The Retirement of Donald Broadbent. 1991;82(3):375–386. doi: 10.1111/j.2044-8295.1991.tb02406.x. [DOI] [PubMed] [Google Scholar]
- Hoard MK, Geary DC, Byrd-Craven J, Nugent L. Mathematical cognition in intellectually precocious first graders. Developmental Neuropsychology. 2008;33(3):251–276. doi: 10.1080/87565640801982338. [DOI] [PubMed] [Google Scholar]
- Holloway ID, Ansari D. Domain-specific and domain-general changes in children’s development of number comparison. Developmental Science. 2008;11(5):644–649. doi: 10.1111/j.1467-7687.2008.00712.x. [DOI] [PubMed] [Google Scholar]
- Holmes J, Adams JW. Working memory and children’s mathematical skills: Implications for mathematical development and mathematics curricula. Educational Psychology. 2006;26:339–366. [Google Scholar]
- Holmes J, Adams JW, Hamilton CJ. The relationship between visuospatial sketchpad capacity and children’s mathematical skills. European Journal of Cognitive Psychology. 2007;20(2):272–289. [Google Scholar]
- Ischebeck A, Zamarian L, Egger K, Schocke M, Delazer M. Imaging early practice effects in arithmetic. NeuroImage. 2007;36(3):993–1003. doi: 10.1016/j.neuroimage.2007.03.051. [DOI] [PubMed] [Google Scholar]
- Ischebeck A, Zamarian L, Siedentopf C, Koppelstatter F, Benke T, Felber S, et al. How specifically do we learn? Imaging the learning of multiplication and subtraction. NeuroImage. 2006;30(4):1365–1375. doi: 10.1016/j.neuroimage.2005.11.016. [DOI] [PubMed] [Google Scholar]
- Jordan NC, Hanich LB, Kaplan D. Arithmetic fact mastery in young children: A longitudinal investigation. Journal of Experimental Child Psychology. 2003;85(2):103–119. doi: 10.1016/s0022-0965(03)00032-8. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kucian K, von Aster M, Loenneker T, Dietrich T, Martin E. Development of neural networks for exact and approximate calculation: A FMRI study. Developmental Neuropsychology. 2008;33(4):447–473. doi: 10.1080/87565640802101474. [DOI] [PubMed] [Google Scholar]
- Landerl K, Bevan A, Butterworth B. Developmental dyscalculia and basic numerical capacities: A study of 8–9-year-old students. Cognition. 2004;93(2):99–125. doi: 10.1016/j.cognition.2003.11.004. [DOI] [PubMed] [Google Scholar]
- Lehto J. Working memory and school achievement in the Ninth Form. Educational Psychology. 1995;15(3):271–281. [Google Scholar]
- Lemaire P, Abdi H, Fayol M. The role of working memory resources in simple cognitive arithmetic. European Journal of Cognitive Psychology. 1996;8(1):73–103. [Google Scholar]
- Levine SC, Jordan NC, Huttenlocher J. Development of calculation abilities in young children. Journal of Experimental Child Psychology. 1992;53(1):72–103. doi: 10.1016/s0022-0965(05)80005-0. [DOI] [PubMed] [Google Scholar]
- Logie RH, Baddeley AD. Cognitive processes in counting. Journal of Experimental Psychology: Learning, Memory, & Cognition. 1987;13:310–326. [Google Scholar]
- Logie RH, Gilhooly KJ, Wynn V. Counting on working memory in arithmetic problem solving. Memory & Cognition. 1994;22(4):395–410. doi: 10.3758/bf03200866. [DOI] [PubMed] [Google Scholar]
- Mazzocco M. Introduction: Mathematics ability, performance, and achievement. Developmental Neuropsychology. 2008;33(3):197–204. doi: 10.1080/87565640801982270. [DOI] [PubMed] [Google Scholar]
- McKenzie B, Bull R, Gray C. The effects of phonological and visual–spatial interference on children’s arithmetical performance. Educational and Child Psychology. 2003;20(3):93–108. [Google Scholar]
- McLean JF, Hitch GJ. Working memory impairments in children with specific arithmetic learning difficulties. Journal of Experimental Child Psychology. Special Issue: The Development of Mathematical Cognition: Arithmetic. 1999;74(3):240–260. doi: 10.1006/jecp.1999.2516. [DOI] [PubMed] [Google Scholar]
- Menon V, Rivera SM, White CD, Glover GH, Reiss AL. Dissociating prefrontal and parietal cortex activation during arithmetic processing. NeuroImage. 2000;12(4):357–365. doi: 10.1006/nimg.2000.0613. [DOI] [PubMed] [Google Scholar]
- Miura IT, Yukari O, Vlahovic-Stetic Vesna, Kim Chungsoon C, Han JongHye. Language supports for children’s understanding of numerical fractions: Cross-national comparisons. Journal of Experimental Child Psychology. Special Issue: The Development of Mathematical Cognition: Numerical Processes and Concepts. 1999;74(4):356–365. doi: 10.1006/jecp.1999.2519. [DOI] [PubMed] [Google Scholar]
- Miyake A, Shah P. Toward unified theories of working memory: Emerging general consensus, unresolved theoretical issues, and future directions. In: Miyake A, Shah P, editors. Models of Working Memory: Mechanisms of Active Maintenance and Executive Control. Cambridge: Cambridge University Press; 1999. pp. 442–483. [Google Scholar]
- Noel MP, Desert M, Aubrun A, Seron X. Involvement of short-term memory in complex mental calculation. Memory & Cognition. 2001;29(1):34–42. doi: 10.3758/bf03195738. [DOI] [PubMed] [Google Scholar]
- Passolunghi M, Mammarella I, Altoe G. Cognitive abilities as precursors of the early acquisition of mathematical skills during first through second gradts. Developmental Neuropsychology. 2008;33(3):229–250. doi: 10.1080/87565640801982320. [DOI] [PubMed] [Google Scholar]
- Passolunghi MC, Pazzaglia F. A comparison of updating processes in children good or poor in arithmetic word problem-solving. Learning and Individual Differences. 2005;15(4):257–269. [Google Scholar]
- Passolunghi MC, Siegel LS. Short-term memory, working memory, and inhibitory control in children with difficulties in arithmetic problem solving. Journal of Experimental Child Psychology. 2001;80(1):44–57. doi: 10.1006/jecp.2000.2626. [DOI] [PubMed] [Google Scholar]
- Passolunghi MC, Siegel LS. Working memory and access to numerical information in children with disability in mathematics. Journal of Experimental Child Psychology. 2004;88(4):348–367. doi: 10.1016/j.jecp.2004.04.002. [DOI] [PubMed] [Google Scholar]
- Pickering S, Gathercole S. Working memory test battery for children. London: The Psychological Corporation; 2001. [Google Scholar]
- Rasmussen C, Bisanz J. Representation and working memory in early arithmetic. Journal of Experimental Child Psychology. 2005;91(2):137–157. doi: 10.1016/j.jecp.2005.01.004. [DOI] [PubMed] [Google Scholar]
- Reuhkala M. Mathematical skills in ninth-graders: Relationship with visuo-spatial abilities and working memory. Educational Psychology. 2001;21:387–399. [Google Scholar]
- Rivera SM, Reiss AL, Eckert MA, Menon V. Developmental changes in mental arithmetic: Evidence for increased functional specialization in the left inferior parietal cortex. Cerebral Cortex. 2005;15(11):1779–1790. doi: 10.1093/cercor/bhi055. [DOI] [PubMed] [Google Scholar]
- Siegel LS, Ryan EB. The development of working memory in normally achieving and subtypes of learning disabled children. Child Development. 1989;60(4):973–980. doi: 10.1111/j.1467-8624.1989.tb03528.x. [DOI] [PubMed] [Google Scholar]
- Siegler RS. Emerging minds: The process of change in children’s thinking. New York: Oxford University Press; 1996. [Google Scholar]
- Siegler RS, Jenkins E. How children discover new strategies. Hillsdale, NJ: Lawrence Erlbaum Associates; 1989. [Google Scholar]
- Siegler RS, Opfer JE. The development of numerical estimation: Evidence for multiple representations of numerical quantity. Psychological Science. 2003;14(3):237–243. doi: 10.1111/1467-9280.02438. [DOI] [PubMed] [Google Scholar]
- Simmons F, Chris S, Horne Joanna. Brief report—Phonological awareness and visual–spatial sketchpad functioning predict early arithmetic attainment: Evidence from a longitudinal study. European Journal of Cognitive Psychology. 2008;20(4):711–722. [Google Scholar]
- Swanson HL. Working memory in learning disability subgroups. Journal of Experimental Child Psychology. 1993;56(1):87–114. doi: 10.1006/jecp.1993.1027. [DOI] [PubMed] [Google Scholar]
- Swanson HL. Short-term memory and working memory: Do both contribute to our understanding of academic achievement in children and adults with learning disabilities? Journal of Learning Disabilities. 1994;27(1):34–50. doi: 10.1177/002221949402700107. [DOI] [PubMed] [Google Scholar]
- Swanson HL. Cross-sectional and incremental changes in working memory and mathematical problem solving. Journal of Educational Psychology. 2006;98(2):265–281. [Google Scholar]
- Swanson LH, Beebe-Frankenberger M. The relationship between working memory and mathematical problem solving in children at risk and not at risk for serious math difficulties. Journal of Educational Psychology. 2004;96(3):471–491. [Google Scholar]
- Swanson HL, Cooney JB, Brock S. The influence of working memory and classification ability on children’s word problem solution. Journal of Experimental Child Psychology. 1993;55(3):374–395. [Google Scholar]
- Swanson HL, Sachse-Lee C. Mathematical problem solving and working memory in children with learning disabilities: Both executive and phonological processes are important. Journal of Experimental Child Psychology. 2001;79(3):294–321. doi: 10.1006/jecp.2000.2587. [DOI] [PubMed] [Google Scholar]
- van der Sluis S, van der Leij A, de Jong PF. Working memory in Dutch children with reading- and arithmetic-related LD. Journal of Learning Disabilities. 2005;38(3):207–221. doi: 10.1177/00222194050380030301. [DOI] [PubMed] [Google Scholar]
- Wechsler D. The Wechsler Individual Achievement Test. 2. The Psychological Corporation; 2001. (WIAT-II) [Google Scholar]
- Weschler D. Wechsler abbreviated scale of intelligence. San Antonio, TX: Psychological Corporation; 1999. [Google Scholar]
- Wilson KM, Swanson HL. Are mathematics disabilities due to a domain-general or a domain-specific working memory deficit? Journal of Learning Disabilities. 2001;34(3):237–248. doi: 10.1177/002221940103400304. [DOI] [PubMed] [Google Scholar]
- Wu SS, Meyer ML, Maeda U, Salimpoor V, Tomiyama S, Geary DC, et al. Standardized assessment of strategy use and working memory in early mental arithmetic performance. Developmental Neuropsychology. 2008;33(3):365–393. doi: 10.1080/87565640801982445. [DOI] [PMC free article] [PubMed] [Google Scholar]