Age at menopause: imputing age at menopause for women with a hysterectomy with application to risk of postmenopausal breast cancer

Abstract

Purpose

Age at menopause, a major marker in the reproductive life, may bias results for evaluation of breast cancer risk after menopause.

Methods

We follow 38,948 premenopausal women in 1980 and identify 2,586 who reported hysterectomy without bilateral oophorectomy, and 31,626 who reported natural menopause during 22 years of follow-up. We evaluate risk factors for natural menopause, impute age at natural menopause for women reporting hysterectomy without bilateral oophorectomy and estimate the hazard of reaching natural menopause in the next 2 years. We apply this imputed age at menopause to both increase sample size and to evaluate the relation between postmenopausal exposures and risk of breast cancer.

Results

Age, cigarette smoking, age at menarche, pregnancy history, body mass index, history of benign breast disease, and history of breast cancer were each significantly related to age at natural menopause; duration of oral contraceptive use and family history of breast cancer were not. The imputation increased sample size substantially and although some risk factors after menopause were weaker in the expanded model (height, and alcohol use), use of hormone therapy is less biased.

Conclusions

Imputing age at menopause increases sample size, broadens generalizability making it applicable to women with hysterectomy, and reduces bias.

Introduction

Patterns of hormone therapy vary by presence or absence of a uterus and duration of use varies with age at menopause. Furthermore, as Pike described ¹, and data have shown ^2,3, appropriate control for age at menopause is necessary to accurately assess the relations of duration of use of postmenopausal hormones and breast cancer risk. Substantial bias towards the null, or underestimating the adverse effect is observed when age at menopause is misclassified ². Hence variation in results for hormone use and risk of breast cancer may arise due to approaches commonly used to model age at menopause for women who have undergone hysterectomy prior to menopause.

Age at menopause has long been identified as a risk factor for breast cancer⁴, ovarian cancer ⁵, osteoporosis ⁶, and mortality ⁷. Hysterectomy is a major common surgical procedure with some 600,000 women discharged following this procedure each year from US hospitals ⁸. Major indications for hysterectomy in the US include uterine leiomyomas (30%), dysfunctional uterine bleeding (20%), genital prolapsed (15%), endometriosis and adenomyosis (20%), chronic pelvic pain (10%) and endometrial hyperplasia (6%)⁹. The proportion of hysterectomies accompanied by bilateral oophorectomy rises with age at surgery from approximately 33% at ages 25 to 29 to more than 70% at ages 45 to 54 ⁸. For these women age at menopause equals age at hysterectomy, for those with ovaries not removed menopause occurs years later with the programmed senescence of the ovary and no clinical marker of menopause, i.e. cessation of menses. This surgical hysterectomy without bilateral oophorectomy leads to a prevalence of hysterectomy and / or missing age at menopause by age 50 that ranges up to more than 38% ¹⁰ in recent US based studies. In the UK Million Women Study, reflecting lower rates of hysterectomy in the UK, approximately 8.6% of women reported hysterectomy without bilateral oopohorecetomy when their mean age was 55.9 at baseline ¹¹.

Missing age at menopause is addressed in a variety of ways in epidemiologic investigations. In the combined analysis of 50 studies completed before 1995, 11% of cases had hysterectomy before menopause and were eliminated from analysis ². A recent French cohort excluded 14.3% of postmenopausal women because age at menopause was not accurate ¹². Other epidemiologic studies allow women into analysis but stratify on type of surgery to account for missing age at menopause. Even others assume age at menopause is the age at hysterectomy ¹³. These approaches to analysis of epidemiologic data often ignore the fact that age at menopause is directly impacting estimation of the adverse effect of use, and duration of use, of postmenopausal hormone therapy and that misspecification leads to biased estimates ¹. Furthermore, exclusion of women with hysterectomy reduces sample size limiting evaluation of other risk factors for postmenopausal breast cancer. In addition, application of models to predict risk of subsequent breast cancer either must exclude these women or provide biased estimates. One recent approach imputed age at menopause using baseline cohort data and assuming the distribution of age at menopause among women undergoing hysterectomy was the same as for women with natural menopause ¹⁴.

While numerous studies have identified factors that may relate to age at natural menopause, most have not had sufficiently large sample size to provide stable estimates, or did not control for confounding variables ^15,16. Few have addressed these factors in combination including reproductive factors (menarche, nulliparity, oral contraceptive use, cigarette smoking, and socioeconomic status)^17-20, though Gold and colleagues comprehensively address predictors in their national study of 14,620 women. They observed that current smoking, lower education attainment, being separated/widowed/divorced, non-employment and history of heart disease were independently associated with earlier natural menopause, while parity, prior oral contraceptive use, and Japanese ancestry were associated with later age at natural menopause ²¹. In Poland, similar results were observed for lifestyle factors including smoking, parity, and oral contraceptive use ²². Among African American women Palmer et al showed that smoking, parity, and body mass index had similar relations to natural menopause as reported for Caucasian populations ²³. Cramer estimates age at menopause by combining multiple risk factors and provides a logistic regression formulation for dichotomized exposures including pack years of smoking; ovulatory cycles; depression; unilateral oophorectomy; and family history of early menopause ²⁴. Simpson et al use an imputation approach with 9 years of follow-up in the Melbourne Collaborative Cohort Study ¹⁴. Neither of these models uses updated exposures over time to predict future age of natural menopause.

In this paper, we describe the methods we used to draw on the rich array of data from the Nurses’ Health Study to impute age at menopause conditional on age at hysterectomy for women who have had a hysterectomy and did not have both ovaries removed. We then evaluate the impact of this imputation on estimates for the effects of postmenopausal hormone therapy dividing time after surgery into two intervals, from surgery to imputed age at menopause – a period during which endogenous hormones may determine risk of breast cancer ²⁵, and from imputed age at menopause through the postmenopausal years when the effect of exogenous hormones may be more directly related to risk ²⁶. This provides the opportunity to include women with hysterectomy without bilateral oophorectomy in clinical applications of risk prediction models, increasing generalizability of models, and providing less biased estimates of future breast cancer risk.

Material and Methods

Participant Characteristics

The Nurses’ Health Study cohort was established in 1976, when 121,701 female, U.S. registered nurses between the ages of 30 and 55 years responded to a mailed questionnaire that inquired about risk factors for cancer and heart disease. The details of the establishment of this cohort have been previously reported ^27,28. In 1976, women reported their age at first full-term pregnancy and the number of pregnancies lasting 6 months or more. In 1978, this information was updated and the women were asked to record the ages of their living children. Every 2 years, follow-up questionnaires have been mailed to the women to bring the information on risk factors up to date and to ascertain whether major medical events have occurred. Data on parity were updated through 1984, and in 1996 we asked women to report their age at each birth. This added information allowed for refinement of data when questionnaires had been missing during earlier follow-up. Deaths in the cohort are reported by family members or the postal service or identified by a search of the National Death Index. It is estimated that mortality ascertainment in this cohort of women is 98% complete ^29,30.

Menopause is assessed on each follow-up questionnaire (http://www.channing.harvard.edu/nhs/questionnaires/index.shtml). A woman is asked,

“Have your periods ceased permanently”?

If yes, “Have you had either of your ovaries removed?”

If yes, “How many remain (none; one)”?

The short questionnaire administered to the last 5 percent of women in a follow-up cycle. Through the 1992 follow-up cycle this questionnaire only asked:

Have your periods ceased permanently (yes / no)?

All data are used prospectively with the classification at the beginning of a 2-year interval carrying through the whole of that interval to the next questionnaire.

Identification of Breast Cancer Cases

On each questionnaire, the participant is asked whether breast cancer had been diagnosed and, if so, the date of diagnosis. All women who report having breast cancer (or the next of kin for decedents) are contacted for permission to review their relevant medical records to confirm the diagnosis.

Population for Analysis

The approach included two stages. First we imputed age at menopause for women in the population with hysterectomy without bilateral oophorectomy. We then evaluated incidence of breast cancer among the total study population, including premenopausal women and women with either natural menopause or surgical menopause.

As depicted in Figure 1, we excluded from the analysis those who submitted duplicate responses at entry (n=123), those with an unknown date of diagnosis (n=7), and all women (n = 3,293) who reported breast or other cancer (excluding non-melanoma skin cancer) on the 1976 questionnaire. This left 118,278 women eligible for follow-up. A total of 12,643 women were missing parity in 1976, reported age at menarche less than 9 or greater than 21, were missing age at menopause on the baseline questionnaire, reported age at any birth greater than age at menopause, did not return any questionnaire after 1978, had an unknown age at first birth, or were missing duration of postmenopausal hormone therapy or unknown history of use. These women were excluded. This left a cohort of 105,635 women eligible for follow-up after exclusions 1 through 7. From this follow-up cohort, we further excluded women with missing height or unknown weight at age 18 years (n=18,237); 87,398 women remained. For analysis of breast cancer incidence, we further excluded women with type of menopause unknown who were postmenopausal at the first NHS questionnaire and those with type of postmenopausal hormone therapy unknown on all questionnaires. 86, 834 women remained for analysis and are included in models B and C in table 3.

Figure 1.

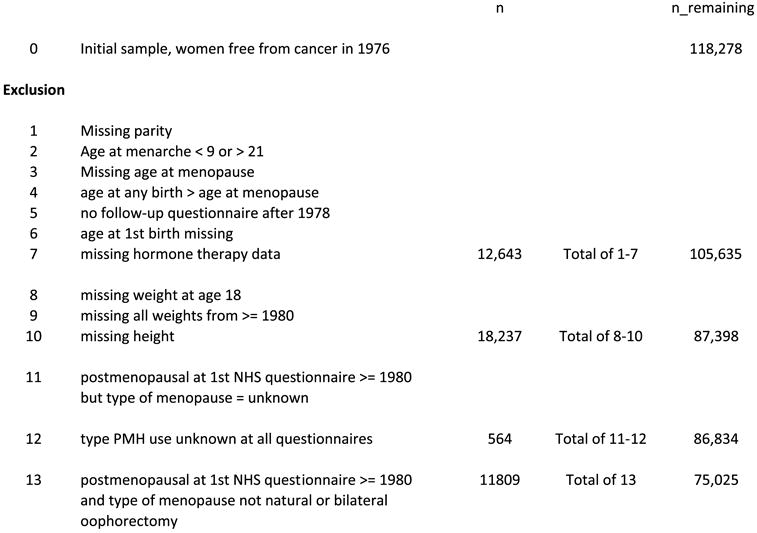
Flow of population with exclusions, Nurses’ Health Study

Note: number in exclusion 11 is not the same as in Table 3, since table 3 includes women who were premenopausal in 1980, but became postmenopausal at a later questionnaire, but with unknown type of menopause (n=6305).

TABLE 3.

Survival curve for natural menopause, natural + surgical menopause, and natural + imputed natural menopause

	Natural menopause		Natural menopause plus surgical menopause*		Natural menopause plus imputed natural menopause†
Age	Number of survivors	Survival probability	Number of survivors	Survival probability	Number of survivors	Survival probability
30	53,249	1.0	53,249	1.0	53,249	1.0
35	53,186	1.0	53,183	0.999	53,189	1.0
40	52,959	0.997	51,735	0.982	52,285	0.993
45	47,138	0.966	46,633	0.915	48,008	0.942
50	29,130	0.713	28,857	0.625	29,873	0.651
55	4,203	0.181	4,011	0.138	4,476	0.147
60	163	0.028	161	0.019	161	0.015
65	15	0.014	15	0.009	15	0.007
70	0	0	0	0	0	0
Censored	21,623‡		14,301§		14,301§
Postmenopausal	31,626		38,948		38,948

^*Age at menopause = age at surgery.

^†Age at menopause for women with surgical menopause women other than bilateral oophrecomty = imputed age at natural menopause: age at menopause = reported age at menopause for women with natural menopause or bilateral oophorecetomy.

^‡Censored includes 4656 bilateral oophorectomy; 630 unilateral oophorectomy; 1956 with hysterectomy; 56 with surgery and uncertain number of ovaries; 24 with radiation; 6305 women with unknown type of menopause; and 7996 women who were premenopausal as of 2002. Unknown menopause is caused by women answering a short follow-up questionnaire that does not obtain any details beyond “periods ceased (yes/no)?”

^§Censored includes 6305 women with unknown type of menopause and 7996 women who were premenopausal as of 2002.

Imputing age at menopause

2,586 women, who were premenopausal in 1980, reported hysterectomy or unilateral oophorectomy during follow-up. These women have been omitted from previous analyses using our log-incidence model due to missing age at menopause ³¹. In the majority of NHS analyses these women are added to follow-up time and considered postmenopausal based on the 90^th percentile for age at natural menopause classified according to smoking status (54 for current smokers and 56 for non smokers). In such analyses age at menopause is defined as age at hysterectomy ¹³.

We developed a Cox Regression Model to identify risk factors associated with early or late natural menopause. We identified a study population of 53,249 women who were premenopausal in 1980 and had no missing covariate data (Table 1 presents data for 38,948 of these women who were premenopausal in 1980 and during 22 years had a natural menopause or hysterectomy) and case / non-case status. We then used a Cox regression model with hazard function defined by:

$$\mathit{hi}(t)=\mathit{ho}(t)\exp \lfloor \sum _{j=1}^k{\beta }_j{X}_{\mathit{ij}}\rfloor$$ (1)

where X are the risk factors defined in 1980. This process was repeated for each 2-year interval, 1982-1984, …, 1998-2000, with risk factors updated every two years. At the beginning of each 2-year interval, women who had realized natural menopause in the previous 2-year interval were considered as “failures” and women who realized surgical or other types of menopause, or who died or developed cancer, were considered as censored. 31,626 women reported natural menopause during follow-up of whom 29,482 had no missing covariates. Covariates were updated in each 2-year interval. All 2-year intervals were considered in the same analysis. We used the AGGREGATE option of PROC PHREG of SAS to account for the correlation between outcomes for multiple 2-year time periods for the same woman³².

TABLE 1.

Distribution of covariates by type of menopause, Nurses’ Health Study, 1980 through 2002^*

			Hysterectomy plus		Hysterectomy without
	Natural menopause		Bilateral oophorectomy		Bilateral oophorectomy
Variable	mean ± SD	n	mean ± SD	n	mean + SD	n
Age at menarche	12.5 ± 1.4	31626	12.3 ± 1.4	4656	12.4 ± 1.4	2586
Age at first birth†	24.9 ± 3.2	30098	24.4 ± 2.8	4423	24.4 ± 2.8	2479
Parity	2.9 ± 1.5	31626	2.8 ± 1.4	4656	2.8 ± 1.3	2586
Nulliparous, %	4.8	31626	5.0	4656	4.1	2586
Current BMI, kg/m2	25.5 ± 5.0	31593	25.6 ± 4.9	4646	24.8 ± 4.7	2582
BMI at age 18	21.3 ± 2.9	28678	21.4 ± 3.0	4299	21.2 ± 2.9	2414
Height, in	64.5 ± 3.2	31626	64.5 ± 3.6	4656	64.6 ± 3.3	2586
Alcohol, g/day	6.2 ± 10.4	29997	5.8 ± 10.5	4438	5.7 ± 9.6	2470
Age at menopause	51.1 ± 3.2	31626	48.0 ± 4.3	4656	44.6 ± 4.8‡	2586
Current smoking, %	21.3	31558	16.9	4651	19.0	2583
Past smoking, %	34.6	31558	34.7	4651	30.9	2583
Family Hx br ca, %	8.7	31626	8.3	4656	5.9	2586
Benign Br disease, %	39.4	31626	41.6	4656	35.2	2586
	Median IQR		Median IQR		Median IQR
Pack-years, (among ever smokers)	16 (6–30)	15391	11 (4–24)	2049	11 (4–22)	1142
Ever OC use, %	56.4	31586	65.0	4652	68.6	2585
Duration OC use (months, among ever users)	37 (13–80)	17816	33 (12–71)	3023	32 (12–68)	1774

BMI = body mass index; IQR = interquartile range; OC = oral contraceptives.

^*38,868 women were premenopausal in 1980 and become postmenopausal during follow-up to 2002. Exposure variable values are on the basis of the survey administered just before the women became postmenopausal.

^†Among parous women.

^‡For women with hysterectomy without bilateral oophorectomy this represents age at surgery.

We then estimated the hazard of reaching natural menopause in the next 2 years for a woman with 0 values (referent values for age 45-9; never smoker; parous; parity 3; age at first birth 24; age at menarche 12; no OC use; no benign breast disease; no family history; and no history of incident breast cancer, and zero for continuous covariates) for all covariates from the equation

$$\mathit{ho}(t)=\mathrm{29,482}/\sum _{i=1}^n\exp \left[\sum _{j=1}^k{\beta }_j{x}_{\mathit{ij}}\right]=0.16325$$ (2)

Upon combining equation 1 and 2, we then estimated the hazard function for each 2 year interval for each woman and thus estimated the survival curve for each woman based on the Kaplan-Meier product limit method given by

$$S_i(t_s+t\mid t_s)=\prod _{s=2(2)}^t[1-h_i(t_s,s-2)],t=2,4,6\dots ,60-t_s,$$ (3)

where t_s = age at surgery.

For each woman with surgical menopause at age t_s we then estimated the probability of natural menopause from age t to t+2 by.

$$P_i(t\mid t_s)=[S_i(t_s+t\mid t_s)-S_i(t_s+t+2\mid t_s)],t=0,2,,\dots 18.$$ (4)

We than generated random uniform (U (0,1)) deviates using the RANUNI function of SAS to impute the age at natural menopause for each woman with surgical menopause. Specifically, if U_i⁽⁰⁾, …, U_i^(l) are a set of U (0,1) random deviates, then if U_i (0)<P_i(0) then the imputed age at natural menopause (t_m) was set to t_s +2; else if U_i (1)< P_i(1) then t_m was set to age t_s +4, …, etc. If a woman did not reach an imputed age at natural menopause by age 60, then t_m was set to 60. Thus, each woman with surgical menopause at age t was assigned an imputed age at natural menopause (t_m) by this process where t<t_m ≤60. During the imputation process, the covariate values were updated up to the year of the last survey, after which they were carried forward at subsequent years. As an example, we estimate the survival curve for a typical woman to impute her age at menopause (See Appendix).

Breast cancer incidence

After all traditional exclusions, including missing age at menopause, a total of 75,025 women were followed for 1,257,005 person-years from 1980 to 2002 during which time 3669 cases of incident breast cancer occurred. After imputing age at menopause we included 86,834 women followed for 1,503,182 person years who reported 4,386 cases of invasive breast cancer. Analysis began in 1980 because this is the year when weight at age 18 years and alcohol intake were first reported. For the total cohort before exclusions, the observed incidence of breast cancer is comparable to the expected incidence based on the SEER rates ³³ for white women, 1988-1992 (O/E = 1.0; 95%CI 0.98-1.03).

Description of the Log - Incidence Model of Breast Cancer

We fit the log-incidence model of breast cancer ^34,35 to incident cases of invasive breast cancer that were identified during follow-up of the Nurses’ Health Study cohort from 1980 to 2002. The approach to model fitting was to assume that incidence at time t(I_t) is proportional to the number of cell divisions (C_t) accumulated throughout life up to age t, that is, I _t = kC_t. The cumulative number of breast cell divisions is calculated as follows:

$$C_t=C_0\mathrm{x}\prod _{i=0}^{t-1}(C_{i+1}/C_i)\equiv C_0\mathrm{x}\prod _{i=0}^{t-1}{\lambda }_i$$

Thus, λ_i = C_i+1/C_i represents the rate of increase in the number of breast cell divisions from age i to age i+1. Log (λ_i) is assumed to be a linear function of risk factors that are relevant at age i. The set of relevant risk factors and their magnitude may vary according to the stage of reproductive life. The details for the representation of C_i are given in Colditz and Rosner ³⁵. The overall model is given by

$$\begin{array}{l}\mathrm{Log}\mathrm{I}=\\ \alpha +{\beta }_o(t^{\ast }-t_o)+{\beta }_{1}b+(t_1-t_0)b_{1,t-1}+{\gamma }_1(t-t_m)m_A\\ +{\gamma }_2(t-t_m)m_B+{\delta }_1{\mathit{pmh}}_A\\ +{\delta }_2{\mathit{pmh}}_B+{\delta }_3{\mathit{pmh}}_C+{\delta }_4{\mathit{pmh}}_{\mathit{cur},t}\\ +({\delta }_4+{\delta }_5){\mathit{pmh}}_{\mathit{past},t}+{\beta }_3{\mathit{BMI}}_1+{\beta }_3^{\ast }{\mathit{BMI}}_2+{\beta }_4{h}_1\\ +{\beta }_4^{\ast }{h}_2+{\alpha }_1\mathit{bbd}+{\alpha }_2\mathit{bbd}{t}_0+{\alpha }_3\mathit{bbd}(t^{\ast }-t_0)\\ +{\alpha }_4\mathit{bbd}(t-t_m)m_t+\varphi \mathit{fhx}+{\beta }_5{\mathit{alc}}_1\\ +{\beta }_5^{\ast }{\mathit{alc}}_2+{\beta }_5^{\ast \ast }{\mathit{alc}}_3\end{array}$$

where t =age; t_o = age at menarche; t_m = age at menopause; t^*= minimum (age, age at menopause); m_t=1 (if postmenopausal at age t, 0 otherwise); s_t =parity at age t; t_i =age at i^th birth, i= 1,. ., s_t; $\mathrm{b}=\mathrm{birth\; index}={\sum }_{i=1}^{s_t}(t^{\ast }-t_i)b_{\mathit{it}}$; b_it = 1 if parity ≥ i at age t, 0 otherwise; m_A =1 (if natural menopause, 0 otherwise); bbd=1 (if benign breast disease=yes, 0 otherwise); fhx = 1 (if family history of breast cancer in mother or sister = yes, 0 otherwise); pmh_A = number of years on oral estrogen; pmh_B = number of years on oral estrogen and progesterone; pmh_C = number of years on other types of postmenopausal hormones; pmh_cur,t =1 (if current user of postmenopausal hormones at age t, 0 otherwise); pmh _past,t =1 (if past user of postmenopausal hormones at age t, 0 otherwise); BMI_j = BMI at age j (kg/m²); alc_j=alcohol use (grams) at age j; h=height (inches).

In these analyses, we treated age at menopause in 3 different ways. In model A, we censored women once they reported a surgical menopause other than bilateral oophorectomy. In model B, we included women with both surgical and natural menopause but assumed that the age at surgery = age at menopause. In model C, we included women with surgical menopause other than bilateral oophorectomy but assumed their age at menopause = age at imputed natural menopause. Furthermore, in Model C, for women with surgical menopause other than bilateral oophorectomy, person-time was divided into 2 segments; pre-menopausal person-time from age at menarche to age at surgery; and postmenopausal person-time after age at imputed natural menopause. For women with natural menopause or bilateral oophorectomy, person-time was divided into pre and postmenopausal person-time. For model C we take the average of 10 imputations per woman. For model C, we estimated age at menopause for women with hysterectomy and combined data from premenopausal women and women with natural menopause, bilateral oophorectomy and hysterectomy with one or no ovaries removed, and ran our breast cancer incidence model on the combined data set. This process was repeated 10 times, yielding 10 separate estimates of relative risk and se for each breast cancer risk factor. We then used standard methods of multiple imputation to obtain overall estimates and standard errors for each risk factor based on the 10 completed data sets.

Finally, to evaluate the hormonal status of women prior to menopause we compare the luteal phase estradiol and estrone levels of participants in the Nurses Health Study II cohort who were premenopausal and free from cancer at the time of blood draw and subsequently reported hysterectomy without bilateral oophorectomy or natural menopause during follow-up ²⁵. Analyses controlled for age, laboratory batch and luteal day.

This study was approved by the Institutional Review Board at Partners Health Care, Boston, MA.

Results

In Table 1 we list the distribution of risk factors for natural menopause, hysterectomy plus bilateral oophorectomy, and hysterectomy without bilateral oophorectomy. We note that women reporting hysterectomy plus bilateral oophorectomy have a higher prevalence of nulliparity and women reporting hysterectomy a lower prevalence. Age at menopause, interpreted as date of surgery for surgical menopause, varies substantially according to type of menopause decreasing from 51.1 for natural menopause to 48.0 for hysterectomy plus bilateral oophorectomy, and 44.6 for average age at hysterectomy plus unilateral oophorectomy. Women with natural menopause are more likely to be current smokers and to have smoked a greater number of pack-years. Prevalence of benign breast disease varied somewhat by type of menopause, and was highest among women with bilateral oophorectomy. Duration of OC use was longest among women reporting hysterectomy without bilateral oophorectomy.

We next evaluated time to natural menopause among women who were premenopausal in 1980. 29,482 reached natural menopause during follow-up from 1980 to 2002 (we censored women when they reported other types of menopause) (table 2). The hazard ratio for natural menopause increases with age, as expected. As previously reported in this cohort ³⁶, current smokers have earlier natural menopause and accordingly increased hazard for natural menopause (Hazard Ratio 1.33; 95% CI 1.30 - 1.37). Later age at menarche was associated with a later age at natural menopause and nulliparous women were at increased risk of natural menopause (Hazard Ratio 1.20; 95%CI 1.14 - 1.25). Parity was also related to decreased risk of menopause, but later age at first birth was not related to risk of earlier menopause. Longer duration of OC use was not related to risk, higher body mass index reduced the risk of natural menopause while benign breast disease was related to earlier menopause and family history of breast cancer was not related to risk of natural menopause. Diagnosis of breast cancer in a 2-year cycle was associated with a substantially lower hazard of becoming menopausal.

TABLE 2.

Association between breast cancer risk factors and time to natural menopause, based on Nurses’ Health Study Data from 1980 through 2002^*

Variable	Regression coefficient	Standard error	p-value	Hazard ratio	95% CI
Age
30–34	−5.311	0.301	<.001	0.01	(0.00–0.01)
35–39	−3.699	0.069	<.001	0.03	(0.02–0.03)
40–44	−1.847	0.024	<.001	0.16	(0.13–0.18)
45–49	(ref)			1.0
50–54	1.137	0.011	<.001	3.12	(3.05–3.18)
55+	1.582	0.028	<.001	4.87	(4.61–5.14)
Smoking
Never	(ref)			1.0
Current	0.287	0.014	<.001	1.33	(1.30–1.37)
Ex	0.046	0.011	<.001	1.05	(1.02–1.07)
Pregnancy history†
Age at menarche −12	−0.021	0.004	<.001	0.92‡	(0.89–0.95)
Nulliparous	0.180	0.024	<.001	1.20	(1.14–1.25)
Parity −3	−0.010	0.004	.012	0.99	(0.98–1.00)
Age at 1st birth −24	0.003	0.002	.11	1.03§	(0.99–1.06)
Other risk factors
Duration of OC use, months	−0.0001	0.0001	.31	0.99¶	(0.98–1.01)
Body mass index, kg/m2	−0.007	0.0001	<.001	0.93∥	(0.91–0.95)
Benign breast disease	0.035	0.011	.001	1.04	(1.00–1.07)
Family history of breast cancer	0.016	0.018	.36	1.02	(0.98–1.05)
Incident breast cancer
Yes	−0.457	0.089	<.001	0.63	(0.53–0.75)

CI = confidence interval; OC = oral contraceptive.

^*Based on 308,065 person–years during which 29,482 women with covariates reported natural menopause. Women were censored if they were premenopausal at the end of follow-up or had other types of menopause.

^†Age at menarche-12 is age at menarche centered using the mean. Parity and age at first birth are also mean centered.

^‡Four-year difference in age at menarche.

^§10-year difference in age at first birth.

^¶Per 5 years of use.

^∥Per 10 kg/m².

We then imputed age at menopause for the 2662 women with surgical menopause without bilateral oophorectomy and no missing covariates (Table 2). The average age at hysterectomy was 44.8 ± 4.9 years, and the average imputed age at menopause was 51.7 ± 3.7 years, close to the age at natural menopause for women in Table 1. We next present the survival probability for 5-year age groups from 30 to 70 (Table 3). Compared to women reporting natural menopause we observed that the curve is shifted to the left if natural menopause and surgical menopause are combined (using age at surgery as the age at menopause) (see Figure 2). On the other hand, after imputing age at natural menopause for women with hysterectomy without bilateral oophorectomy, the probabilities are comparable to those for natural menopause alone. For example, the probability of remaining premenopausal for a 45 year old woman is 96.6% for natural menopause alone; 91.5% for natural plus surgical menopause; and 94.2% after imputing natural menopause (the later probability also includes women with bilateral oophorectomy).

Figure 2.

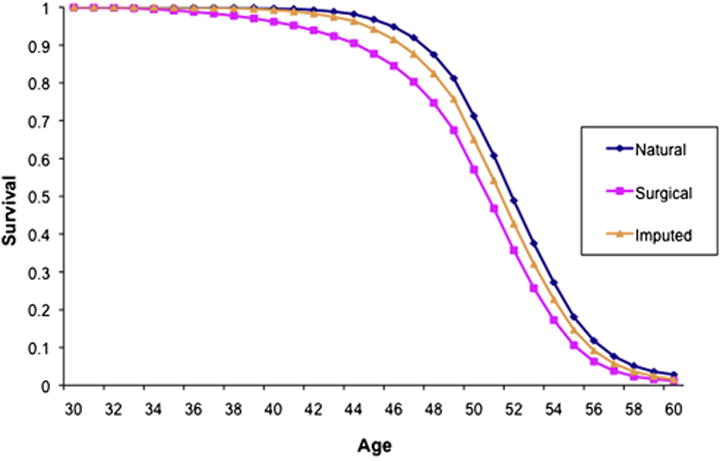
Probability of remaining premenopausal according to age, by type of menopause, among participants in the Nurses’ Health Study.

We next fit the log incidence model for breast cancer and report the different parameter estimates after defining age at menopause in different ways (Table 4). Model A includes natural menopause and bilateral oophorectomy only, and thus represents an unbiased estimate of the effect of hormone therapy after menopause. It includes 3,669 cases diagnosed during 1.257M person-years. In Model B we include 4386 cases during 1.503M person years and set age at menopause to the age at hysterectomy. In Model C we include 4386 cases during 1.503M person years and age at menopause is set as the imputed age at menopause with follow-up time set to missing from surgery to imputed age at menopause. First, for duration of premenopause, we observe that the increase in risk per year is relatively constant across the 3 models.

TABLE 4.

Effect of different approaches to defining age at menopause on fitted breast cancer incidence model, Nurses’ Health Study, 1980–2002

	Model A*			Model B†			Model C‡
Variable	Beta	SE	p-value	Beta	SE	p-value	Beta	SE	p-value
Intercept	−9.205	0.191		−9.021	0.164		−9.051	0.259
Duration of premenopause, yrs	0.081	0.005	<.001	0.076	0.005	<.001	0.077	0.007	<.001
Menopause, duration
Natural	0.028	0.004	<.001	0.029	0.003	<.001	0.029	0.005	<.001
Bilateral oophorectomy	0.019	0.005	<.001	0.023	0.004	<.001	0.023	0.007	<.001
Unilateral oophorectomy	–	–	–	0.036	0.005	<.001	0.037	0.006	<.001
Hysterectomy	–	–	–	0.038	0.004	<.001	0.038	0.005	<.001
Other types of menopause	–	–	–	0.045	0.008	<.001	0.045	0.010	<.001
Pregnancy history
Age at 1st birth - age at menarche	0.0062	0.0034	.068	0.0066	0.0032	.038	0.0066	0.0035	.063
Birth index	−0.0037	0.0005	<.001	−0.0038	0.0005	<.001	−0.0038	0.0005	<.001
BBD
BBD (yes vs no)	0.214	0.373	.57	0.351	0.310	.26	0.282	0.430	.51
BBD x age at menarche	0.049	0.018	.005	0.043	0.016	.006	0.045	0.019	.018
BBD x duration of premenopause	−0.009	0.007	.22	−0.010	0.006	.064	−0.009	0.007	.19
BBD x duration of menopause	−0.013	0.004	<.001	−0.013	0.003	<.001	−0.012	0.004	.005
Postmenopause hormone (PMH)
Duration of oral estrogen use alone, yrs during postmenopause	0.033	0.006	<.001	0.022	0.005	<.001	0.022	0.005	<.001
Duration of oral estrogen and progesterone, yrs during postmenopause	0.065	0.008	<.001	0.065	0.008	<.001	0.066	0.010	<.001
Duration of use of other types of postmenopausal hormones
During postmenopause	0.030	0.008	<.001	0.017	0.007	.011	0.018	0.007	.019
Current PMH use	0.045	0.053	.39	0.053	0.047	.26	0.050	0.081	.58
Past PMH use	−0.164	0.053	.002	−0.162	0.048	<.001	−0.167	0.065	.001
BMI
(Average BMI during premenopause-21.8) × duration of premenopause§	−0.0011	0.0002	<.001	−0.0010	0.0002	<.001	−0.0010	0.0002	<.001
(Average BMI during postmenopause-24.4) × duration of postmenopause	0.0034	0.0004	<.001	0.0025	0.0004	<.001	0.0025	0.0004	<.001
Height
(Height - 64.5) x duration of premenopause§	0.00076	0.00022	<.001	0.00057	0.00020	.004	0.00058	0.00022	.009
(Height - 64.4) x duration of postmenopause	−0.00070	0.00095	.46	0.00045	0.00076	.56	0.00039	0.00084	.64
Alcohol
Cumulative grams premenopause	0.00032	0.00006	<.001	0.00026	0.00006	<.001	0.00026	0.00006	<.001
Cumulative grams postmenopause
While on PMH	−0.00068	0.00029	.020	−0.00038	0.00024	0.11	−0.00038	0.00026	.14
While not on PMH	−0.00013	0.00023	.56	0.00011	0.00018	0.54	0.00009	0.0002	.63
Family history of breast cancer (yes vs no)	0.41	0.04	<.001	0.42	0.04	<0.001	0.42	0.04	<.001
Number of women		75,025			86,834			86,834
Number of cases		3,669			4,386			4,386
Number of person-years		1,257,005			1,503,182			1,503,156

BBD = benign breast disease; BMI = body mass index.

Model results updated for C: brcnmodel.alcbmi.clustered2a.saslog Dec 1, 2009.

^*Model A includes person-time before menopause or after natural menopause or bilateral oophorectomy; women are censored after surgical menopause or menopause of unknown type.

^†Model B includes person-time both before and after menopause; for surgical menopause, age at menopause is set to age at surgery.

^‡Model C includes person-time both before and after menopause; for women with surgical menopause, age at menopause is set to imputed age at natural menopause, where imputed age at natural menopause is forced to be greater than age at surgery. Average of 10 imputations.

^§Include time postmenopausal when on postmenopausal hormones.

Let us consider a woman with age at surgery = 45 and imputed age at menopause = 50 and use of estrogen for 10 years from age 45 to 55. This woman would be excluded from analysis after age 45 in Model A. Including women with surgical menopause and treating age at menopause as age at surgery (model B) increases the number of cases to 4,386 during 1.503M person-years. The relative risk for 10 years of use of unopposed estrogen is exp(0.066+10×0.024) = 1.36 and the increase in risk per year is 2.4% per year of use. On the other hand, in Model C with the same population, this woman is not included in analysis from surgery to imputed age at menopause (50), but accrues only 5 years of postmenopausal person-time from imputed age at menopause to current age. Thus, the relative risk = exp[0.050 +5×(0.022)] = 1.17. After the imputed age at menopause, risk increases at 2.2% per year. When only person-time after imputed age at menopause is included in Model C the association for estrogen plus progestin therapy is comparable to the unbiased estimate in Model A, but 717 additional cases are included in the population for analysis.

In the expanded population (Models B and C) we adjust for type of menopause adding terms for unilateral oophorectomy, hysterectomy without oophorectomy, and other type of menopause, and observed that the rate of increase in risk per year after menopause is somewhat lower for body mass index (0.0025 vs. 0.0034). A reduction was observed for height after menopause. We also note that after adding women who have had a hysterectomy, the strength of association for each year of use of alcohol after menopause was reduced.

We also address hormone levels in premenopausal women prior to menopause to determine if those who undergo hysterectomy are different from those who proceed to natural menopause. We evaluated luteal phase estradiol and estrone levels collected while the women were premenopausal. The ICC over a 2 to 3 year period for luteal phase estrone was 0.44 and for estradiol it was 0.45 ³⁷. We observed no significant difference in either hormone level approximately 5 to 6 years before surgery or natural menopause. See Table 5.

TABLE 5.

Geometric mean^* hormone levels measured in luteal phase and subsequent type of menopause, Nurses’ Health Study II

	Simple hysterectomy		Natural menopause
	Number	Mean (95% CI)	Number	Mean (95% CI)	p value
Estrone	36	81.9 (70.7–94.9)	346	81.2 (77.7–85.0)	.92
Estradiol	34	111.5 (92.1–135.1)	322	116.8 (110.1–123.8)	.66

CI = confidence interval; ICC = intraclass correlation coefficient.

ICC for luteal phase blood measures 0.44 for E1 and 0.45 for E2.

^*Adjusted for age, laboratory batch, and luteal day

Discussion

We report a model to impute expected age at natural menopause conditional on age at hysterectomy without bilateral oophorectomy. Given the prevalence of hysterectomy without bilateral oophorectomy in the US population, and widespread exclusion of these women from epidemiologic evaluations of postmenopausal breast cancer risk factors, this imputation offers the potential to include age at menopause in analysis of contemporary studies. Women can accurately report whether or not they have had both ovaries removed at surgery³⁸, enabling multiple potential applications. This imputed age at menopause allowed us to evaluate use of postmenopausal hormones and showed that unopposed estrogen may be misspecified in some settings, but combination estrogen plus progestin has comparable magnitude across models reflecting use by women with natural menopause and limited impact of how women with hysterectomy are handled in analysis. The expanded model includes substantially more cases, generates lower standard errors for some postmenopausal risk estimates, and will enable applications such as use of risk prediction models to counsel women regarding breast cancer prevention strategies tailored to their level of risk. The expanded population for analysis will also be valuable in such settings as histological subsets of breast cancer or disease classified according to receptor status, where power is reduced compared to the study of total incidence.

Strength of the imputation is the array of reproductive and other variables we could evaluate as predictors of age at menopause. While we do not have measures of depression that were included by Cramer, the findings are nevertheless consistent with his work ²⁴. The Melbourne cohort had 17% of women missing age at menopause ¹⁴. They imputed age at menopause and recommend this approach to evaluate relations for postmenopausal hormone therapy. While other approaches to classification of menopausal status may be applied in clinical settings or with more comprehensive exposure assessment ³⁹, evidence from the majority of epidemiologic studies evaluating hormone therapy shows that stratification, exclusion, or other methods for modeling missing age at menopause have been the standard approach ^2,10,12,40.

One concern with respect to imputing future age at menopause is that the hormonal milieu of women undergoing surgery is different from that of women proceeding to natural menopause. Nurses’ Health Study II data reported in this paper support no major differences for luteal estradiol and estrone levels approximately 5 years before natural menopause (or surgery). Although not definitive, these are consistent with cross-sectional data reported for Australian women ages 40 to 69 years. 152 women with hysterectomy were compared with 1423 women with an intact uterus who had never used hormone therapy. Women under 55 years of age with hysterectomy had slightly higher estradiol levels than those with an intact uterus ⁴¹.

We also note that we observed an association between history of benign breast disease and type of menopause, and that women with benign breast disease had an earlier age at natural menopause. To the best of our knowledge this association has not been reported previously, though many studies have evaluated a range of reproductive and socio-cultural predictors.

A limitation includes the lack of ethnic diversity and the potential for risk factors to vary with race and ethnicity. Henderson et al ⁴² have shown that Latina have an earlier age at natural menopause and like Gold et al ²¹ that Japanese Americans a later age at natural menopause, while smoking, age at menarche, parity and body mass index are not related to risk. However, we note that the majority of breast cancer risk factors carry common effects for cancer risk regardless of ethnicity in the Multiethnic Cohort, the same cohort that showed variation in age at menopause according to race/ethnicity ⁴³.

In conclusion, the ability to impute age at natural menopause for women reporting hysterectomy without bilateral oophorectomy allows for inclusion of substantially more women into analyses relating hormone therapy to risk of breast cancer without inducing bias in the risk estimates. Furthermore, as models become available for risk prediction for women regardless of type of menopause and extent of surgery, they will be more clinically useful if all women can be included. Likewise, the expanded population available for evaluation of risk factors after menopause will be valuable as studies evaluate subtypes of breast cancer.

Appendix Calculation of Imputed Age at Natural Menopause for a Particular Woman

We consider a woman with surgical menopause at age 40. First, the baseline hazard h₀(t) was estimated by 29,482/180594 = 0.16325. This represents the probability of reaching natural menopause over 2 years in our dataset for a 45-49 year-old woman who never smoked, had age at menarche = 12 years, age at 1^st birth = 24 years, parity =3 and 0 values for all other covariates. Second, the woman had the following covariate values:

1. age	40	42	44	46	48	50	52	54
2. smoking	cur	past	past	past	past	past	past	past
3. age at menarche	10	10	10	10	10	10	10	10
4. nulliparous	no	no	no	no	no	no	no	no
5. parity	3	3	3	3	3	3	3	3
6. age at 1st birth	24	24	24	24	24	24	24	24
7. dur OC use (mth)	23	23	23	23	23	23	23	23
8. BMI	29.8	29.8	31.3	29.8	31.3	31.3	29.8	29.8
9. BBD	yes	yes	yes	yes	yes	yes	yes	yes
10. Fam Hx Br ca	no	no	no	no	no	no	no	no
Exp(Σ βj Xij)	0.183	0.144	0.142	0.914	0.904	2.817	2.848	2.848
hi(t)	0.030	0.024	0.023	0.149	0.148	0.460	0.465	0.465
x(t)	0.605	0.499	0.736	0.488	0.168	0.799	0.686	0.343
Natural menop.	no	no	no	no	no	no	no	yes

For this woman with surgical menopause at age 40, there was a probability of 0.030 of becoming menopausal by age 42. However, since the random uniform deviate for this woman at age 40 (x(t) = 0.605) was greater than h_i(t) (0.030), the woman was considered to remain premenopausal until age 42. The process was repeated at age 42,44,…,52 with the same result. However, at age 54, since x(t) < h_i(t), the imputed age at natural menopause for this woman was set at 54.