Religion, fertility and genes: a dual inheritance model

Abstract

Religious people nowadays have more children on average than their secular counterparts. This paper uses a simple model to explore the evolutionary implications of this difference. It assumes that fertility is determined entirely by culture, whereas subjective predisposition towards religion is influenced by genetic endowment. People who carry a certain ‘religiosity’ gene are more likely than average to become or remain religious. The paper considers the effect of religious defections and exogamy on the religious and genetic composition of society. Defections reduce the ultimate share of the population with religious allegiance and slow down the spread of the religiosity gene. However, provided the fertility differential persists, and people with a religious allegiance mate mainly with people like themselves, the religiosity gene will eventually predominate despite a high rate of defection. This is an example of ‘cultural hitch-hiking’, whereby a gene spreads because it is able to hitch a ride with a high-fitness cultural practice. The theoretical arguments are supported by numerical simulations.

1. Introduction

It is widely agreed that religion has biological foundations—that belief in the supernatural, obedience to authority or susceptibility to ceremony and ritual depend on genetically based features of the human brain [1–6]. However, there is a disagreement about the extent to which causality also flows in the opposite direction, from religion to biology. One view is that religion is a ‘spandrel’—a cultural phenomenon based on features of the brain that were already in existence in their present form when religion first appeared (e.g. [7–12]). Another view is that the existence of religion promotes the evolution of genes that predispose people towards religious belief or behaviour. The latter view rests upon two plausible assumptions: (i) individuals have diverse endowments of the genes that predispose humans towards religion; and (ii) religion-induced selection is strong enough to have an appreciable impact within the relevant time-frame on the frequency of such genes.

The fact that religion has a genetic basis of great antiquity does not imply that genetic variation has disappeared. Koenig & Bouchard [13] survey twin studies that quantify the genetic and environmental determinants of what they call the ‘traditional moral triad’ of authoritarianism, conservatism and religiousness. In most cases, 40 to 60 per cent of the observed variation in such personality traits is explained by genotypic variation. The authors argue that these are large genetic effects in comparison with typical findings in the social sciences.

There is no direct evidence regarding the speed with which religion affects genetic evolution. However, there is evidence that the rise of complex human culture in general has greatly accelerated the pace of genetic evolution, by altering the frequency of long-standing genes in the global population and by spreading or preserving new mutations [14–19].

For religion to influence genetic evolution it must convey some kind of selective advantage. Such an effect might come about through social bonding via ritual, formation of group identity through myth, honest signalling through participation in costly ceremonies and adherence to social norms through love or fear of God [20–24]. In most cases, religious individuals gain personal advantage from their activities or beliefs. However, religion may also induce behaviour that has a fitness cost to the individual but is beneficial to the group. If group selection is strong, this should favour the emergence of a type of religion that induces adherence to pro-social norms, which in turn should favour the evolution of genes that predispose individuals towards this type of religion [25–27].

Another channel through which religion might influence genetic evolution is via its impact on fertility. The link between religion and fertility has been extensively discussed by demographers, but, as Bouchard [28] notes, the potential genetic implications of this link have been largely ignored by evolutionary theorists.

(a). Religion and fertility

In the modern world, religious people, even controlling for income and education, have more children on average than people without religion [29,30]. The reasons are cultural in the broad sense, and include social norms and the influence exerted by religious organizations [31–34]. The more devout people are, the more children they are likely to have. The World Values Survey for 82 nations over the period 1981–2004 reveals that adults attending divine service more than once a week averaged 2.5 children, those attending once per month averaged 2.01 and those never attending averaged 1.67 (cited in [12]). Similar findings for a variety of European countries are reported by Philipov & Berghammer [35]. Sects such as the Amish, the Hutterites and Haredi (‘ultra-orthodox’) Jews have total fertility rates three to four times greater than the secular average [36,37].

Modern fertility differentials partly reflect diverse responses to the ‘demographic transition’. Global birth rates have fallen dramatically in recent times and in a number of countries, including Japan and most of Europe, they are now well below replacement [38–40]. However, the transition to lower fertility has been slower and less complete among religious people than among those without religion, since it is driven partly by individualistic values of self-fulfilment that are inimical to traditional religious teaching [32,41]. The pace and extent of the transition also vary across religions and denominations [42–44]. Some religious groups have been largely unaffected by the demographic transition and still have extremely high birth rates, which explains why there is now such an enormous fertility gap between them and the rest of the population.

The impact of differential fertility on the religious composition of society depends on the scale of switching between religious groups, and between them and the secular population. With no defections at all, ultra-high fertility groups would rapidly outgrow the rest of the population and soon become a majority. In practice, there may be limits to their expansion. As they get larger, their members may come into closer contact with other members of society and acquire secular values, causing them to have fewer children [45]. Some members may leave to join a less strict group, whereas others may give up religion altogether. For example, the Old Order Amish have a total fertility rate of 6.2, whereas the more modern New Order Amish have a somewhat lower total fertility rate of 4.8 (p. 36 in [37]). What will happen in the future is a matter of speculation. Kaufmann [37] argues that the stricter religious groups will continue to exhibit high fertility and will also be effective at transmitting their beliefs to their children, so that relatively few of them will defect in later life. This would represent a continuation of recent history, which has seen the number of Amish in the USA rise from 123 000 in 1991 to 249 000 in 2010—due almost entirely to internal growth [46]. Sustained growth at this rate would take the Amish population to almost 7 million by the end of the century and 44 million by 2150. Rapid fertility-driven growth has also been observed among other groups such as Hutterites and Haredi Jews [36,37]. If growth at such rates were to continue, it would speedily turn what is currently a tiny fraction of the population into a majority. Indeed, this will inevitably happen unless the forces of secularism constrain the growth of these groups by reducing their birth rates or increasing their defection rates.

(b). Genetic implications

There are two channels through which a high fertility group can influence the composition of the overall gene pool: internal growth and defection. If most of the children born within a high fertility group remain in the group when they grow up, the size of this group will increase rapidly and its share of the overall gene pool will rise accordingly. Conversely, if most of the children eventually leave, this will limit the size of the group. However, such defections will also spread the group's genes into the surrounding population, thereby increasing their share in the overall gene pool. The role of defection in this context is similar to that of migration in Sewall-Wright's [47] shifting balance theory of evolution, whereby a mutation may spread outwards from a high-fitness group to the rest of the population. These two channels—internal growth and diffusion through defection—may have radically different implications for the eventual size of the high fertility group, but their long-run genetic implications may be similar. In each case, the genes associated with the high-fertility group may eventually predominate in the overall gene pool. The picture is further complicated by the existence of conversion, whereby individuals join the high-fertility group, and by exogamy, whereby individuals remain in the group but marry an outsider who does not convert.

To explore these issues we shall consider some simple mathematical models. These models are in the gene-culture tradition [19] and draw heavily on the work of Boyd & Richerson [48] regarding direct bias, vertical transmission and the natural selection of cultural variants. The models are of the dual inheritance type, in which children inherit both their genes and their initial religious (or non-religious) allegiance from their parents. They retain their genes throughout their lives, but they may eventually change their allegiance. Children who are brought up as religious may abandon religion when they grow up, whereas those brought up without religion may later convert and become religious. The probability of switching allegiance in our models depends on the genetic endowment of the individual concerned. A child who is genetically predisposed towards religion is more likely than other children to remain or become religious as an adult. Throughout the analysis we shall assume that fertility is an entirely cultural phenomenon: genes affect the likelihood that particular individuals will be religious, but do not directly influence their reproductive behaviour. All religious adults (‘believers’) have the same fertility irrespective of their genes; likewise non-believers have the same fertility irrespective of their genes. There is some evidence from a Danish twin study that genes have an independent influence on the desire of people to have children [49], but we shall ignore this complication. We initially assume complete assortative mating, whereby religious people mate only with other religious people. One or both of the partners may be converts, but there are no truly mixed couples in which the partners retain distinct allegiances. This assumption is realistic for ultra-high-fertility Jewish and Christian groups [50], and also for Muslims in general [51], but is less realistic for mainstream Christian Churches whose members nowadays frequently marry members of other Churches or people of no religion at all. We later consider how the existence of mixed marriages between religious and non-religious people affects the results.

We begin with a haploid model in which the rules of genetic transmission are simple and rates of religious defection and conversion are fixed. This model is later modified to allow for more complex genetic transmission and for variable rates of defection and conversion. Such modifications do not affect the qualitative results, although they may have important quantitative implications. In all of the models we consider, religious predisposition (‘religiosity’ for short) is determined by a single gene. This is unlikely to be true in practice, but without this simplification the analysis would be intractable.

2. A haploid model of dual inheritance

Society is divided into two distinct allegiance groups indexed by i = r, n. Members of group r are religious ‘believers’ whereas members of group n are ‘non-believers’. All individuals have two cultural parents who are also their genetic parents. Individuals live for two periods. They acquire their genes from their parents and during the first period as children they acquire their initial allegiance from their parents. They then become adults and choose whether to retain or modify their allegiance. Next they have children of their own, bring them up and then die. The probability that a child will switch allegiance on becoming an adult depends on its genetic endowment, which is specified by a single gene at a specific locus. Each individual carries exactly one copy of this gene. The gene comes in two forms (‘alleles’) that are indexed by j = R, N. The ‘religiosity’ allele R codes for religious predisposition and allele N codes for non-religious predisposition. Thus, there are four distinct types in the population:

Dynamics depend on fertility and mating. We assume that adults mate only with adults from the same allegiance group. Each couple in group i has 2c_i (>0) children. These children initially have the same allegiance as their parents, and the genetic endowment of each child is inherited with equal probability from either parent. Let 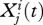be the number of adults at time t with allegiance i and allele j. Every adult of type (i, j) gives rise to c_i children of type (i, j). For example, if two adults of type (r, R) mate they will have 2c_r children of type (r, R), which is equivalent to c_r children of this type for each parent. If an adult of type (r, R) mates with an adult of type (r, N) they will on average have c_r children of type (r, R). The total number of children of type (r, R) is therefore .

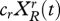The genetic composition of an individual is fixed for life, but there may be a change of allegiance when a child reaches adulthood. This will alter the distribution of adult types in the next period. Let 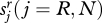be the probability that a child of type (r, j) will switch allegiance to type (n, j) as an adult. Likewise, 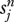is the probability that a child of type (n, j) will switch to type (r, j) as an adult. In principle, these switching parameters could vary through time as a result of wider social trends or they might be density-dependent. For the moment we shall assume they are constant. Taking switches into account, the number of adults of each type in the next period is given by the system of difference equations

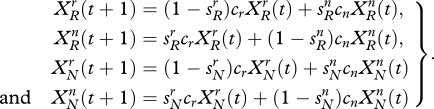2.1

The right-hand side of each equation contains two components. One component denotes individuals who have retained their childhood allegiance and the other denotes newcomers who have switched allegiance from the other group.

For any type (i, j) define the fraction of this type in the adult population as follows:

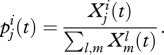Thus, 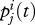is the fraction of the adult population who belong to group i(= r, n) and carry allele j(=R, N). Also, define

and

Thus, pⁱ(t) is the fraction of the adult population who belong to group i and p_j(t) is the fraction who carry allele j. Note that p^r(t) + pⁿ(t) = p_R(t) + p_N(t) = 1.

(a). Modelling predisposition

The religiosity allele R codes for a genetic predisposition towards religion. In the present context, this means that at least one of the following conditions must hold:

• — Among children who are brought up without religion, those who carry allele R are more likely to become religious in adult life than those with allele N.

• — Among children who are brought up as religious, those who carry allele R are less likely to abandon their religion in adult life than those with allele N.

To express the above conditions mathematically we impose the following conditions on the switching parameters:

2.2

with at least one strict inequality. These conditions are symmetric. If R codes for a predisposition towards membership of group r then allele N codes for a predisposition towards membership of group n. The above definition of predisposition is similar to that of Lumsden & Wilson [52].

(b). Long-run behaviour

We can now state a simple but powerful result. We assume that 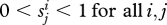and also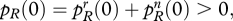 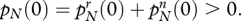Thus, for each type there is a non-zero switching between groups and both alleles are initially present in the population.

Proposition 2.1. Suppose that the predisposition conditions (2.2) are satisfied. Suppose further that members of group r have more children than those of group n, such that

2.3

Then, in the long-run, the distribution of types in the population will converge to a limit of the following kind:

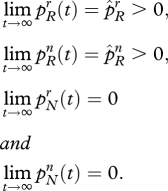Hence

2.4

Proof. See the electronic supplementary material. ▪

Thus, if religious allegiance is associated with a higher-than-average birth rate, and there is an allele that predisposes individuals towards religion, this allele will eventually predominate. The fraction of individuals who are ‘believers’ will stabilize at less than 100 per cent and there will remain a finite percentage of adults who are without religion. In the limit, all of the latter will carry the religiosity allele R. It must be stressed that this result is contingent on the assumption that c_r > c_n. In the opposite case (c_r < c_n), allele N would eventually predominate.

(c). Role of the predisposition conditions

To understand the role of the predisposition conditions (2.2), consider what happens when these conditions do not hold. Suppose the probability of switching between groups is positive and independent of a person's genotype, so that 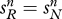and 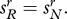Suppose also that both alleles are initially present in the population. In this case, the system will eventually settle down to a stable genetic polymorphism in which the genetic composition of groups r and n is identical, and alleles R and N coexist in finite proportions. The ultimate frequency of these alleles in the population as a whole will depend on the starting point.

The outcome is different if the predisposition conditions (2.2) hold. These conditions establish a bias in the pattern of inter-group flows and ensure that, irrespective of starting point, allele R will eventually become over-represented in the high fertility group r. From then onwards R will enjoy a growth advantage over allele N, whose share in the overall gene pool will converge to zero. The correlation between cultural phenotype (allegiance r) and genotype (allele R) is endogenous and is subject to forces that ensure that, in the long run, this correlation is positive. This is an example of ‘cultural hitch-hiking’, whereby a gene spreads because it is able to hitch a ride with a high-fitness cultural practice [18].

(d). Fertility and the pace of evolution

The pace of evolution and the eventual share of religion in the population both depend on the fertility differential between religious and non-religious people. This is illustrated in table 1. When the relative fertility of the religious group is ultra-high (c_r/c_n = 3), the pace of change is extremely fast. Within 10 generations, the share of adults with a religion rises from 0.5 to 50 per cent and the share of the religiosity allele R in the overall gene pool rises to more than 95 per cent. With c_r/c_n = 2, the pace of change is still fast. Within 10 generations, the share of adults with religion rises from 0.5 initially to 14.3 per cent and the share of allele R in the gene pool rises to 22.6 per cent. As relative fertility is further reduced, the pace of change slows down dramatically and the eventual share of religion is much lower. With c_r/c_n = 1.3, the share of adults with a religion is only 1.4 per cent after 100 generations and the share of allele R is only 3.8 per cent. It takes 500 generations for the share of R to surpass 40 per cent.

Table 1.

Effect of fertility on the evolution of religion and genes. This table illustrates how differential fertility affects social and genetic evolution. The table assumes that 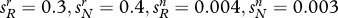. All trajectories begin at the point .

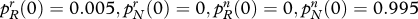	generations elapsed
differential fertility	0	10	20	100	500	∞
cr/cn	share of population who are religious = pr
3.0	0.005	0.500	0.545	0.552	0.552	0.552
2.0	0.005	0.143	0.347	0.406	0.406	0.406
1.5	0.005	0.026	0.035	0.135	0.147	0.147
1.3	0.005	0.014	0.014	0.014	0.024	0.038
1.1	0.005	0.009	0.009	0.009	0.009	0.017
cr/cn	frequency of religiosity allele R = pR
3.0	0.005	0.782	0.955	1.000	1.000	1.000
2.0	0.005	0.226	0.754	1.000	1.000	1.000
1.5	0.005	0.034	0.076	0.905	1.000	1.000
1.3	0.005	0.015	0.019	0.038	0.414	1.000
1.1	0.005	0.007	0.007	0.008	0.011	1.000

(e). Ultra-high fertility sects

The rate of expansion of ultra-high fertility sects depends on their ability to retain their membership. If all of their children were to remain members for life and had the same ultra-high fertility as their parents, then within just a few generations these sects would dominate the social landscape. If a large number of children leave these sects on reaching adulthood, this will limit their expansion. However, it will not prevent the spread of the genes that predispose individuals towards religion (assuming such genes exist).

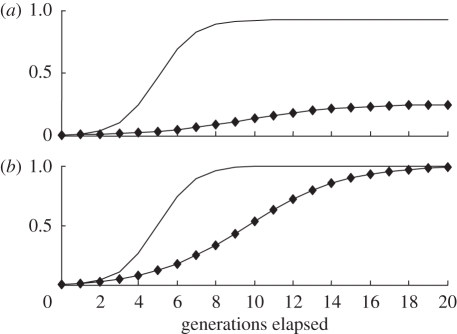
Figure 1 provides a numerical illustration of a closed ultra-high fertility sect that recruits no one from the outside. The birth rate of its members is three times as high as that of outsiders. The membership of the sect is initially 0.5 per cent of the adult population. All members of the sect carry the religiosity allele R, whereas initially no one outside the sect carries this allele. What happens in the course of time depends on the ability of the sect to retain its members. Two scenarios are shown in figure 1. In the low defection scenario, 5 per cent of adult members leave the sect each generation. This is the observed defection rate for Old Order Amish children born in the 1960s [53]. In the high defection scenario, the figure is 50 per cent. The most striking feature of the low defection scenario is the pace of change. Within four generations, one fifth of the adult population belong to the sect and after 10 generations its share stabilizes at 92 per cent. By then almost every adult in the population carries allele R, and the other allele is well on the way to oblivion. Under the high defection scenario, the pace of change is much slower and the eventual share of the population who are religious is much lower. Even so, after 20 generations, the vast majority of the population carry allele R. Such a situation comes about because in each generation a large number of adults who are carrying R abandon their religion and feed this allele into the non-religious population. This example illustrates the paradoxical role of secularization in spreading the religiosity allele. Secularization reduces the growth rate of the ultra-high fertility sect, but it does so by importing into the non-religious population a large number of people who carry the religiosity allele. Religion may be kept in check by defections from the sect, but this does not prevent the ultimate triumph of the religiosity allele.

Figure 1.

How religious defections influence social and genetic evolution. The diagrams refer to an ultra-high-fertility religious group. (a) Share of believers; (b) share of allele R. A high defection rate reduces the eventual share of religious believers (group r) in the population. Whatever the defection rate, the religiosity allele R eventually tends to fixation. The diagram assumes: both variants: c_r/c_n = 3.0; p_R^r (0)=0.005, p^r_N (0)=0, p_Rⁿ (0)=0, p_Nⁿ (0)=0.995; low defection: s_R^r=0.05, s_N^r=0.07, s_Rⁿ=0, s_Nⁿ=0; high defection: s_R^r=0.5, s_N^r=0.07, s_Rn=0, s_Nⁿ=0. Line without diamonds, low defection; Line with diamonds, high defection.

3. A diploid model

The above analysis can be extended to the diploid case in which individuals carry two alleles rather than one. In this version, there are three genetic types and two cultural types, which can be combined as follows:

We assume that adults mate at random with adults from the same group and that one gene is inherited with equal probability from each parent. Each type (i, jk) has a given probability of switching to the other allegiance group. Note that 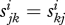. The difference equations are more complicated than in the haploid case (see the electronic supplementary material).

In the diploid case, allele R codes for a genetic predisposition towards r provided the following inequalities are satisfied:

3.1

with at least one strict inequality. The level of dominance is specified by the equations

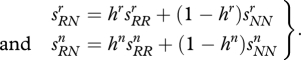3.2

If 1 ≥ h^r, hⁿ ≥ 0, then

3.3

with at least one strict inequality. Allele R is completely dominant if h^r = hⁿ = 1 and completely recessive if h^r = hⁿ = 0. The properties of the diploid version were explored by simulation, using 2700 distinct parameter combinations for R dominant and the same number for R recessive, together with a further 2700 for the intermediate case h^r = hⁿ = 0.5. All of these parameter combinations satisfy the predisposition conditions (3.3) and in all of them c_r > c_n. 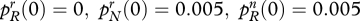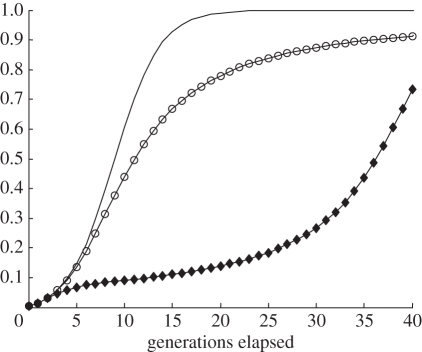Initial gene frequencies are and 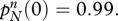Thus only a very small proportion of the adult population are initially religious and all of these individuals carry allele N. Even so, in every case, the gene R eventually takes over and the other allele goes to oblivion. This is identical to the result obtained in the haploid case. However, the pace of convergence is slower in the diploid case, especially when R is recessive. Figure 2 shows what happens to the overall frequency of R in one particular example.

Figure 2.

What happens to the share of allele R in the haploid and diploid versions of the model. R achieves fixation fastest in the haploid version of the model and slowest in the recessive diploid version. The diagram assumes: all variants: c_r/c_n = 3.0; p_R^r (0)=0.005, p_N^r (0)=0, p_Rⁿ (0)=0, p_Nⁿ (0)=0.995; haploid: s_R^r=0.3, s_N^r=0.6, s_Rⁿ=0.05, s_Nⁿ=0.05; diploid: s_RR^r=0.3, s_NN^r=0.6, s_RRⁿ=0.05, s_NNⁿ=0.05; R dominant: s_RN^r=s_RR^r, s_RNⁿ=s_RRⁿ; R recessive: s_RN^r=s_NN^r, s_RNⁿ=s_NNⁿ. Line with circles, diploid + R dominant; line with diamonds, diploid + R recessive; line without circles or diamonds, haploid.

(a). Density dependence

The decision to switch may depend on the relative size of the two groups. We model this by assuming that, for i = r, n and j, k = R, N

3.4

where 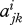and bⁱ are constant and 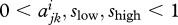. These assumptions ensure that .

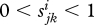If bⁱ > 0, the probability of defecting from group i increases as this group gets larger. In the case of religion, this could occur because the religious group becomes ‘softer’ and less able to police its boundaries as its relative size increases. The group may also become less isolated as it gets larger and a greater fraction of its members come into contact with the secular world [45].

If bⁱ<0, the probability of defecting from group i decreases as this group gets larger. This could be because group i becomes a world unto itself as it gets larger, so that individuals from this group have fewer contacts with outsiders and, in consequence, are less frequently exposed to ideas or pressures that subvert their allegiance.

For R to code for a predisposition towards religion, the following conditions must be satisfied:

3.5

with at least one strict inequality.

(b). Simulations

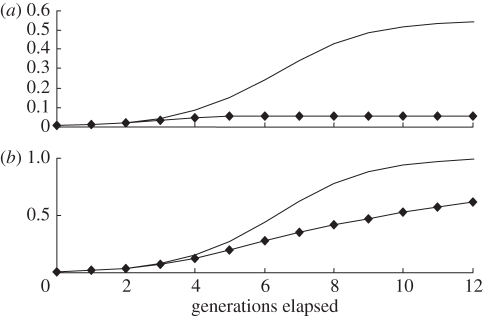
The simulations reported in the online material indicate that proposition (2.1) holds even in the diploid model with density-dependent behaviour. The allele R will eventually take over, provided it satisfies the relevant predisposition conditions and c_r > c_n. Moreover, the share of the religious group r will eventually stabilize at less than 100 per cent. Figure 3 provides an illustration in which the growth of the religious group is severely constrained by defection as it gets larger, so that it never attains more than 5.5 per cent of the total population. Even so, because of this group's high birth rate, there is a stream of defectors who take the religiosity allele R with them into the non-religious population.

Figure 3.

The evolutionary effects of a constraint on the growth of a high-fertility religious group. Defections increase as the group gets bigger, which limits the eventual size of this group. However, large-scale defections spread the religiosity allele R into the rest of the population. The diagram assumes: both variants: c_r/c_n = 3.0; p_R^r (0)=0.005, p_N^r (0)=0, p_Rⁿ (0)=0, p_Nⁿ (0)=0.995; a_RR^r=a_RN^r=0.3, a_NN^r=0.4, a_RRⁿ=a_RNⁿ=a_NNⁿ=0; bⁿ=0; no constraint: b^r=0, constraint: b^r =−20. Note: s_jkⁱ (t)=a_jkⁱ [1−bⁱ pⁱ (t)]. Line without diamonds, no constraint; line with diamonds, constraint.

(c). Mixed marriages

The discussion has so far assumed strict endogamy within the two allegiance groups. The partners in a couple may come from different backgrounds, but they have a common allegiance by the time they mate. In this section, we consider briefly what happens if there are mixed marriages in which the two partners retain distinct allegiances. Our approach draws on the biology literature on imprinting (especially [54], but also [55,56]). We make the following assumptions. A constant fraction α_i of adults from allegiance group i will only mate with someone from their own group. This preference is unrelated to their genetic type. The remaining adults are indifferent towards the allegiance of their partner and they mate randomly with each other. Some of them find a partner from their own group and some a partner from the other group. Mixed couples have 2c_m children, of which a fraction f_r receives a religious upbringing. The relevant difference equations are derived in the electronic supplementary material.

The evolution of the system was explored numerically for a number of parameter combinations. The conclusions are as follows. Provided the religious group has higher fertility than the non-religious group, there is always a cultural polymorphism: the population share of each group will eventually stabilize at a non-zero level. If mixed marriages are ruled out, as in the preceding sections of this paper, allele R always tends to fixation. However, if mixed marriages are permitted, there are some parameter combinations for which the frequency of allele R tends to 0. These are characterized by a low preference for endogamy, combined with a low-to-medium fertility advantage for the religious group. This can be seen in the examples shown in rows (9), (13), (17), (18), (21) and (22) of table 2. In most cases, even where mixed marriages occur, R tends to fixation. Moreover, the greater is the preference for endogamy, as indicated by the α parameters, the faster is the approach to fixation. This can be seen from the penultimate column of the table. The effect of mixed marriages in these examples is to slow down the fixation of R and sometimes prevent fixation of this allele altogether. Similar effects were observed in other simulations not reported here. None of these simulations gave rise to a stable genetic polymorphism.

Table 2.

The effect of endogamy on the evolution of genes. This table illustrates how preferences for endogamy influence the overall frequency p_R of allele R in the adult population. The fraction of religious (non-religious) adults with a preference for endogamy is α_r (α_n). The number of children per mixed marriage is 2c_m of which a fraction f_r receive a religious upbringing; couples in which both partners are religious (non-religious) have 2c_r (c_n) children. The table assumes that 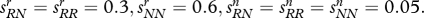Trajectories all start from the point .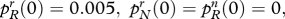

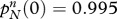row	αr	αn	fr	cr/cn	cm/cn	pR(0)	pR(20)	pR(106)
(1)	0	0	1	3	2	0.005	0.024	1.000
(2)	0.4	0.4	1	3	2	0.005	0.474	1.000
(3)	0.8	0.8	1	3	2	0.005	0.724	1.000
(4)	1	1	1	3	2	0.005	0.780	1.000
(5)	0	0	0	3	2	0.005	0.005	0.997
(6)	0.4	0.4	0	3	2	0.005	0.072	1.000
(7)	0.8	0.8	0	3	2	0.005	0.773	1.000
(8)	1	1	0	3	2	0.005	0.780	1.000
(9)	0	0	1	2	1.5	0.005	0.003	0.000
(10)	0.4	0.4	1	2	1.5	0.005	0.003	1.000
(11)	0.8	0.8	1	2	1.5	0.005	0.304	1.000
(12)	1	1	1	2	1.5	0.005	0.495	1.000
(13)	0	0	0	2	1.5	0.005	0,004	0.000
(14)	0.4	0.4	0	2	1.5	0.005	0.010	1.000
(15)	0.8	0.8	0	2	1.5	0.005	0.191	1.000
(16)	1	1	0	2	1.5	0.005	0.495	1.000
(17)	0	0	1	1.2	1.1	0.005	0.001	0.000
(18)	0.4	0.4	1	1.2	1.1	0.005	0.003	0.000
(19)	0.8	0.8	1	1.2	1.1	0.005	0.007	1.000
(20)	1	1	1	1.2	1.1	0.005	0.012	1.000
(21)	0	0	0	1.2	1.1	0.005	0.003	0.000
(22)	0.4	0.4	0	1.2	1.1	0.005	0.003	0.000
(23)	0.8	0.8	0	1.2	1.1	0.005	0.007	1.000
(24)	1	1	0	1.2	1.1	0.005	0.012	1.000

4. Discussion

This paper assumes that there exist genetic differences between individuals that affect their predisposition towards religion. In the same cultural environment, some individuals are for genetic reasons more likely to become or remain religious than others. It also assumes that religious people have more children on average than secular people. This gap may currently be quite small in the case of mainstream Churches, but there are some religious groups that have fertility rates several times larger than their secular counterparts. They also have high rates of endogamy. If individuals from these groups marry outsiders they must normally either leave the group or else their partner must convert. Some of these groups have been growing rapidly for the past century but their future is uncertain. There are several possible scenarios. Such groups may continue on their present trajectory for the rest of this century and beyond, leading to a spectacular and ultimately unsustainable increase in their numbers. Or maybe their growth rates will slow down quite soon because they experience more defections as members leave to join more liberal religious groups or give up religion altogether. Or perhaps their fertility will decline as they absorb secular values or face practical obstacles to expansion. These scenarios have different implications for the genetic evolution of society. If religious people continue to have a higher birth rate on average than secular people and they are sufficiently endogamous, then any genes which predispose people towards religion will spread. This may be true even if mainstream religions shrink or become increasingly secular in their breeding habits. Provided a core of high-fertility sects continues to exist, and those sects remain highly endogamous, they will transform the genetic composition of society through either internal growth or defection. This has been demonstrated in the present paper using a single gene for religiosity. In reality, a phenomenon as complex as religious predisposition is likely to be influenced by many different genes, but this does not alter the main argument.

One issue of interest in this context is genetic polymorphism. Such a phenomenon is widespread in nature. It may arise through genetic drift if some trait is selectively neutral or because different variants enjoy selective advantage in different environments. It may also arise because heterozygotes have superior fitness when compared with homozygotes. Alternatively, an observed polymorphism may be transitory and scheduled to disappear as some particular variant eventually triumphs. In the case of religion, heritability studies suggest there is currently significant variation in genetic predisposition towards religion [13]. There are several possible explanations for this. Religious predisposition may be associated with genes that were, or still are, differentially advantageous in specific natural or social environments. The current variation in predisposition may simply reflect environmental diversity. To the extent that religious predisposition is the result of group selection, variation is to be expected, since group selection requires variation to operate. Or perhaps we are living in a transitional era in which selective forces will eventually marginalize certain variants. This is one possible interpretation of the analysis presented in this paper. However, even within our framework, there are several avenues through which a stable polymorphism could arise. If there is a convergence of religious and non-religious birth rates, the share of the religiosity allele R will eventually stabilize at less than unity. Or there might be some form of heterozygote advantage, whereby heterozygotes have a stronger religious predisposition than homozygotes. This will be the case within our framework if h^r, hⁿ > 1. Simulations not reported here suggest that even a modest effect of this kind will generate a stable polymorphism and allow N to survive. However, heterozygote advantage is merely a theoretical possibility and there is currently no evidence to support it. The most plausible mechanism that could generate a stable polymorphism is a future convergence of religious and non-religious birth rates.

Some of the present fertility differentials are so large that, if they persist, they may have a significant genetic effect within the space of a few generations. This may not be because of an increase in the share of individuals who are actively religious. If high fertility rates are combined with high defection rates, the share of the population who belong to high-fertility religious groups may stabilize at quite a low level. However, defections from such groups will spread religiosity genes to the rest of society. There will be an increasing number of people with a genetic predisposition towards religion but who lead secular lives. It is interesting to speculate how such a predisposition might manifest itself in a secular context. The findings of Koenig & Bouchard [13] suggest that a genetic predisposition towards religion is associated with obedience to authority and conservatism. If this is correct, then the diffusion of religiosity genes into the rest of society should see an increase in the number of secular people who are genetically inclined towards such values. The implications of such a development are beyond the scope of this paper to consider.