Abstract
Purpose/Objectives
We have created an automated process utilizing real-time tracking data to evaluate adequacy of PTV margins in prostate cancer, allowing for a process of adaptive radiotherapy with minimal physician workload. We present an analysis of PTV adequacy and a proposed adaptive process.
Materials/Methods
Tracking data was analyzed for 15 patients who underwent SMLC IMRT with uniform 5mm PTV margins for prostate cancer utilizing the Calypso® Localization System. Additional plans were generated with 0 and 3 mm margins. A custom software application utilizing the planned dose distribution and structure location from CT simulation was developed to evaluate the dosimetric impact to the target due to motion. The dose delivered to the prostate was calculated for the initial 3, 5, and 10 fractions, and for the entire treatment. Treatment was accepted as adequate if minimum delivered prostate dose (Dmin) was at least 98% of the planned Dmin.
Results
For 0, 3, and 5 mm PTV margins, adequate treatment was obtained in 3/15, 12/15, and 15/15 patients, and the delivered Dmin ranged from 78–99, 96–100, and 99–100 percent of the planned Dmin. Changes in Dmin did not correlate with magnitude of prostate motion. Treatment adequacy during the first 10 fractions predicted sufficient dose delivery for the entire treatment for all patients and margins.
Conclusions
Our adaptive process successfully utilized real-time tracking data to predict the need for PTV modifications, without the added burden of physician contouring and image analysis. Our methods are applicable to other utilizations of real-time tracking, including hypofractionated treatment.
Keywords: Prostate, cancer, radiotherapy, intrafraction, adaptive
INTRODUCTION
Multiple randomized clinical trials have demonstrated a dose escalation benefit for prostate radiotherapy(1–5). Recent data suggests both inter- and intra-fraction prostate motion is not negligible(6–11). Selection of planning target volume (PTV) margins must balance assurance of adequate dose delivery to the prostate with the hazard of increased dose to normal tissues(12).
Given the heterogeneity of intra-fraction motion among patients previously described(10, 11, 13), patients with minimal intra-fraction motion may benefit from PTV margin reductions. Although numerous methods are available to quantify isolated translational prostate motion, the dosimetric consequences of translational and rotational motion are not well described. Current methods of dose verification rely on serial imaging and contouring, which creates a segmentation and image analysis workload for the physician. These methods cannot account for all intra-fraction motion since images are not sampled continuously throughout treatment.
We have created an automated process utilizing real-time electromagnetic tracking to evaluate adequacy of PTV margins in prostate cancer, allowing for a practical method of adaptive radiation therapy with reduced physician workload.
The purpose of this study was to 1) determine if patient-specific rotations and translations of the prostate could predict adequacy of plans with 0, 3 and 5 mm margins and 2) determine if rotations and translations from the first 3, 5, or 10 fractions could predict adequacy of the margins for the remainder of therapy.
METHODS
Patient Population
Records were analyzed for 15 patients who underwent definitive IMRT for prostate cancer utilizing the Calypso® 4D Localization System (Calypso Medical, Seattle WA) between November 2007 and March 2009. Most patients had low risk disease (14). Detailed patient characteristics are shown in Table 1.
Table I.
Patient Characteristics
| Number of patients | 15 |
| Median Age (Range) | 71 yrs. (58–83 yrs.) |
| Tumor Stage | |
| T1c | 12 |
| T2a | 1 |
| T3a | 1 |
| T3b | 1 |
| Risk Stratification | |
| Low | 9 |
| Medium | 4 |
| High | 2 |
| Median Prescribed Dose (Range) | 7380 cGy (7000 – 7920 cGy) |
Simulation and Treatment Planning
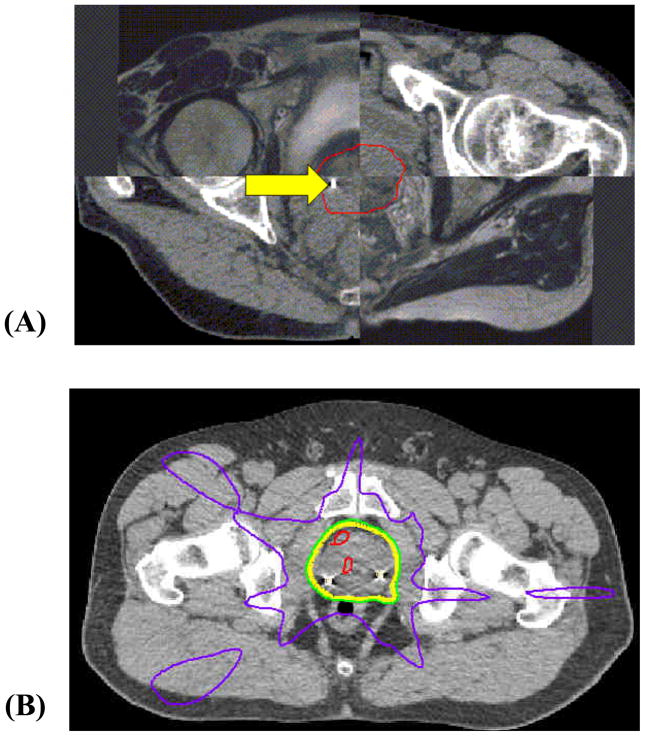
Details of our methods for MRI simulation, subsequent transponder placement and CT simulation have been previously described (15). Planning CT and MR images were imported into Pinnacle v8.0m (Philips Medical Systems, Cleveland, OH) and fused with manual, rigid image registration using prostate anatomy contoured on both the CT and MRI scans. The clinical target volume (CTV) was formed by contouring the prostate on the CT and MR fusion images (Figure 1).
Figure 1.
(A) Checkerboard axial image of the fused MR and CT scans, and the contoured prostate, illustrated for one patient. The final contoured prostate (CTV) is shown in red. A fiducial beacon placed at the right base of the prostate is seen on this CT slice (arrow). (B) Isodose distribution of a 5-field IMRT prostate plan, showing the 50% (purple), 95% (green), 100% (yellow), and 105% (red) isodose lines.
For each patient, 0 mm, 3 mm, and 5 mm expansions were applied about the prostate to create PTV volumes. Five–field IMRT plans using a step-and-shoot multi-leaf collimation (SMLC) technique were generated with a planning goal of 100% CTV and 98% PTV coverage by prescription dose. Rectum constraints were applied such that the rectum volumes receiving greater than 65 Gy (V65) and 40 Gy (V40) were less than 17% and 35%, respectively. Bladder constraints were applied such that the volumes of bladder receiving dose greater than 65 Gy and 40 Gy were less than 25% and 50%, respectively. Figure 1 illustrates a representative plan for one patient treated with a 5 mm PTV margin.
Treatment Delivery
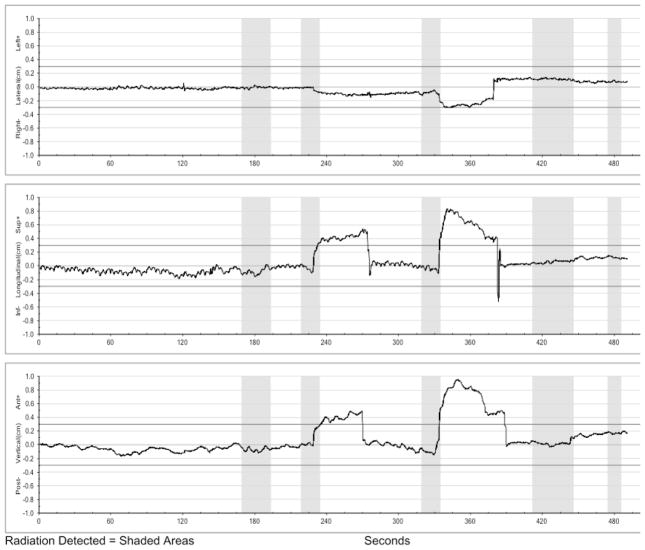
Real-time electromagnetic tracking was utilized for all patients obtained at 0.1 second intervals. The Calypso system reports set-up rotations/translations and intra-fraction prostate position. Therapists are alerted to displacements exceeding motion limits via feedback provided by the System’s graphical display and audible alarms. Radiation delivery was initiated only if reported setup rotation was less than 10°. If setup rotation exceeded 10°, patients were repositioned and then, if necessary, asked to urinate in order to adjust bladder filling. Treatment was delivered only while isocenter deviation was less than 3mm from setup during real-time tracking to prevent radiation delivery during large anatomical variations. An example motion trace of a treatment delivery pause followed by couch repositioning is shown in Figure 2.
Figure 2.
Real-time tracking data for one treatment, including right/left (top), superior/inferior (middle), and anterior/posterior (bottom) tracking data. The shaded regions illustrate the time when the treatment beam was turned on for each of the 5 planned fields. Following delivery of the second field, treatment was paused due to a persistent shift in the superior and anterior directions which exceeded tolerance. This was corrected with intra-fraction repositioning 270 seconds into treatment. Additional repositioning was utilized at 380 seconds following delivery of the 3rd field, due to motion in the right, superior, and anterior directions which exceeded tolerance.
Motion Analysis
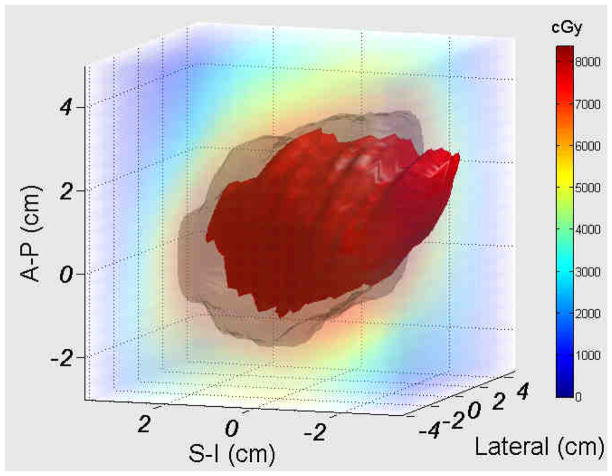
Using a research feature of the Calypso system that allows for tracking data export, the three-dimensional spatial coordinates for each transponder were sampled at 0.1 second intervals during treatment. A custom Matlab program called SWIFTER (Semi-Automatic Workflow using Intra-fraction Fiducial-based Tracking for Evaluation of Radiotherapy) was used to estimate delivered dose using the static dose cloud from treatment planning along with the measured intra-fraction motion. The details and validation of the software application have been previously described(16). Briefly, rotation was calculated about each axis (superior/inferior, anterior/posterior, right/left), with the origin set at the centroid of the three transponders. Using SWIFTER, target motion within the planned dose cloud was simulated using measured translations and rotations collected over each patient’s course of therapy in order to gauge the “actual delivered dose” to the prostate. This analysis requires no physician input or recontouring, since the prostate structure is obtained from the planning CT and rigidly translated and rotated through the static dose cloud from the treatment plan. A simulation of the prostate structure moving relative to the dose cloud for a sample translation/rotation is shown in Figure 3. For each patient, the dose delivered to the prostate was calculated for the first 3, 5, and 10 fractions, and for the entire treatment.
Figure 3.
Illustration of the impact of translational and rotational motion for a prostate (red) plan using a 3mm PTV margin (grey), with prostate rotation of 20 degrees pitch, and translational shifts of 3 mm in the anterior and inferior directions. Real-time tracking translational and rotational data was used to virtually move the prostate within the treatment dose cloud, in order to estimate the ‘actual’ dose delivered for each patients’ motion.
Evaluation of Rigid Body Approximation
To assess validity of this approximation in our cohort, inter-transponder distances (sampled at 0.1 second intervals throughout treatment) were analyzed for variation. Histogram plots of the distribution of tracked inter-transponder distances compared to the mean for each patient were generated. To evaluate deformation, variability of the measured inter-transponder distances was compared to the known positional accuracy of the tracking system (<0.5 mm) (17).
Evaluation of Variable Rectum Anatomy
Day-to-day changes in rectum composition could potentially modify the radiation dose distribution, particularly at the rectum-prostate interface. Because the dose distribution was modeled as unchanging, inter-fraction change in dose cloud shape could potentially introduce a calculation error. To evaluate our static dose cloud approximation, we studied the effect of a “worst-case” change in rectum composition by manual assignment of air-equivalent density (0 gm/cc) to the rectum for one patient with rectum distension at simulation. Aside from rectum tissue density, all parameters for the IMRT plan were kept constant. To evaluate dose at the rectum-prostate interface, a new structure (RP Interface) was created by expanding the prostate 1 mm posteriorly, followed by subtraction of the initial prostate structure. Maximum, minimum, and mean doses to the prostate and RP Interface were calculated for comparison between the standard and worst-case plans. The percent volume of prostate receiving 95% prescription dose was also calculated, and isodose lines for 95% and 50% of prescription dose were also compared.
Evaluation of PTV Adequacy
PTV adequacy was assessed using dose statistics of the prostate structure. The “actual” minimum dose delivered to the prostate (Dmin) based on our simulation was calculated both for the first 3, 5, and 10 treatments, and for the entire treatment course. Additionally, dose delivered to 95% of the prostate volume (D95) was calculated for the entire course. In accordance with our planning constraints, adequate treatment was defined as a course in which the actual delivered dose to the prostate was no less than 98% of the planned minimum dose.
Correlation of Patient Motion Parameters With Treatment Adequacy
Pitch, roll, and yaw rotation, and isocenter translational displacement along each axis (superior/inferior, anterior/posterior, right/left) and in total, was tested for correlation with the decrement in delivered prostate dose to determine if patient motion parameters could predict adequacy of treatment. Total displacement was defined as mean three-dimensional isocenter displacement over an entire treatment course from the planned position as defined by the distance formula. Scatter plots and linear regression was performed to determine whether specific rotations and translations predict inadequate Dmin as measured by SWIFTER.
Treatment Adequacy Prediction Using Analysis of Initial Treatments
We evaluated if dose coverage during the first few radiotherapy fractions predicted adequacy for an entire treatment course, and also determined the number of fractions required to successfully predict treatment adequacy. Delivered Dmin values as a percentage of planned Dmin were calculated using the tracking data from the first 3, 5, and 10 fractions. Coverage adequacy based on Dmin during each initial treatment period was compared to the Dmin for each patient’s entire treatment course. Dmin was calculated as the cumulative delivered dose using all available data at a given time point, rather than for each fraction individually.
Impact of Translational and Rotational Motion on Rectum Dose
To assess the impact of translational and rotational motion on rectum dose, contours were generated to contain the portion of the rectum lying within 2 cm of the prostate contour. The 2 cm cut-point was arbitrarily chosen due to concern regarding the applicability of a rigid body transformation if the entire rectum contour were used. Using SWIFTER, ‘actual’ rectum doses were calculated using each patient’s real-time tracking data and comparisons were made with the planned rectum doses (V40 and V65), both for the 3 mm and 5 mm PTV plans.
RESULTS
Treatment Plans
Treatment plan parameter results are illustrated in Table 2. No significant planning differences existed in PTV coverage for the 0 mm, 3 mm, and 5 mm margin plans (p=NS, Student’s paired two tailed t-test) as gauged by the percentage of the prescription dose delivered to 98% of each PTV. Statistically significant decrements (Table 2) were noted in the volumes of rectum and bladder receiving 65 Gy and 40 Gy as PTV margins were reduced from 5 mm to 3 mm, and from 3 mm to 0 mm (Student’s paired two tailed t-test, Table 2).
Table II.
Impact of PTV margins on doses delivered to rectum and bladder. V65 = percentage of organ receiving greater or equal to 65 Gy. V40 = percentage of organ receiving greater or equal to 40 Gy. A significant reduction of rectum and bladder V65 and V40 values was seen for reductions in PTV margins.
| PTV margin | % Rx dose Delivered to 98% of PTV | Rectum V65 | Rectum V40 | Bladder V65 | Bladder V40 |
|---|---|---|---|---|---|
| 0 mm | 100.6 ± 0.7 | 3.5 ± 3 | 14.6 ± 6 | 4.3 ± 5 | 16 ± 10 |
| 3 mm | 100.7 ± 0.7 | 7.5 ± 4 | 19.8 ± 6 | 9 ± 10 | 22 ± 20 |
| 5 mm | 100.5 ± 2 | 10.0 ± 4 | 23.3 ± 7 | 12 ± 10 | 26 ± 20 |
| p value (0 mm, 3 mm) | 0.6 | <.001 | <.001 | 0.003 | <.001 |
| P value (3 mm, 5 mm) | 0.7 | <.001 | 0.005 | 0.001 | <.001 |
Translational and Rotational Motion
Across all patients, compared with position at the time of simulation, the mean and standard deviation values of the magnitude of rotation about the x (pitch), y (roll), and z (yaw) axes were 5.7° ± 5°, 2.0° ± 2°, and 1.6 ± 1°. The mean and standard deviation values of the magnitude of translation in the x (anterior-posterior), y (left-right), and z (superior-inferior) directions were 0.39 mm ± 0.4 mm, 0.64 mm ± 0.5 mm, and 0.69 ± 0.6 mm. Table III illustrates the mean rotation about the x, y, and z axes, as well as the mean translational displacement along each axis and in total for each patient. Substantial variation in the magnitude of patient motion was seen across the study’s population (Table 3).
Table III.
Characteristics of each patient’s rotational and translational motion.
| Patient Number | Mean Magnitude of Rotation (degrees) | Mean Isocenter Displacement (mm) | |||||
|---|---|---|---|---|---|---|---|
| x-axis (pitch) | y-axis (roll) | z-axis (yaw) | Sup-Inf | Ant-Post | Left-Right | Composite | |
| 1 | 3.1 ± 3 | 0.71 ±0.7 | 0.31 ±0.5 | 0.48 ± 0.4 | 0.51 ± 0.5 | 0.71 ± 0.6 | 1.18 ±0.6 |
| 2 | 4.3 ± 3 | 2.3 ± 1 | 4.5 ± 1 | 0.58 ± 0.5 | 0.68 ± 0.5 | 0.37 ± 0.3 | 1.11 ± 0.5 |
| 3 | 5.9 ± 3 | 3.4 ± 1 | 1.6 ± 0.9 | 0.46 ± 0.4 | 0.76 ± 0.7 | 0.30 ± 0.4 | 1.13 ± 0.5 |
| 4 | 12.5 ± 4 | 1.9 ± 1 | 2.38 ± 0.9 | 0.74 ± 0.5 | 0.51 ± 0.4 | 0.35 ± 0.4 | 1.14 ± 0.5 |
| 5 | 4.0 ± 3 | 2.9 ± 2 | 2.18 ± 0.8 | 0.60 ± 0.5 | 0.60 ± 0.6 | 0.40 ± 0.4 | 1.10 ± 0.7 |
| 6 | 5.3 ± 2 | 1.33 ± 0.9 | 1.84 ± 0.9 | 0.46 ± 0.4 | 0.62 ± 0.6 | 0.29 ± 0.3 | 0.99 ±0.6 |
| 7 | 3.8 ± 3 | 2.6 ± 2 | 2.5 ± 1 | 0.68 ± 0.6 | 0.42 ± 0.5 | 0.57 ± 0.5 | 1.13 ±0.6 |
| 8 | 12.0 ± 5 | 1.3 ± 1 | 1.40 ± 0.9 | 0.77 ± 0.6 | 0.89 ± 0.7 | 0.34 ± 0.4 | 1.43 ± 0.7 |
| 9 | 2.4 ± 2 | 1.4 ± 1 | 0.74 ± 0.6 | 0.57 ± 0.5 | 0.90 ± 0.7 | 0.43 ± 0.4 | 1.34 ± 0.6 |
| 10 | 5.2 ± 4 | 2.5 ± 2 | 0.90 ± 0.8 | 0.70 ± 0.5 | 0.84 ± 0.7 | 0.42 ± 0.4 | 1.40 ± 0.7 |
| 11 | 4.4 ± 3 | 2.2 ± 2 | 1.5 ± 1 | 0.83 ± 0.7 | 0.85 ± 0.7 | 0.39 ± 0.4 | 1.43 ± 0.8 |
| 12 | 3.7 ± 2 | 2.7 ± 1 | 0.70 ± 0.7 | 0.72 ± 0.6 | 0.74 ± 0.7 | 0.37 ± 0.3 | 1.27 ± 0.7 |
| 13 | 1.9 ± 2 | 1.14 ± 0.9 | 0.63 ± 0.6 | 0.64 ± 0.5 | 0.58 ± 0.5 | 0.49 ± 0.4 | 1.16 ± 0.5 |
| 14 | 6.4 ± 2 | 1.26 ± 0.9 | 3.1 ± 1 | 0.49 ± 0.5 | 0.56 ± 0.6 | 0.52 ± 0.4 | 1.08 ± 0.5 |
| 15 | 2.5 ± 2 | 1.18 ± 0.9 | 1.39 ± 0.8 | 0.63 ± 0.5 | 0.72 ± 0.6 | 0.21 ± 0.3 | 1.13 ± 0.6 |
Evaluation of Rigid Body Approximation
Figure 4 displays the histogram of inter-transponder distances for all patients across all fractions. Inter-transponder distances varied by less than 0.5, 1, 1.5, and 2 mm from the average for 55, 84, 95, and 99% of total treatment time, respectively. Given the known positional accuracy of 0.5 mm (17), inter-transponder deformations greater than 0.5 and 1.5 mm occurred less than 16 % and 1% of the total treatment time, respectively.
Figure 4.
Histogram of the intra-fraction intertransponder distance variation from mean distance (mm) observed throughout treatment for all patients and fractions.
Evaluation of Variable Rectum Anatomy
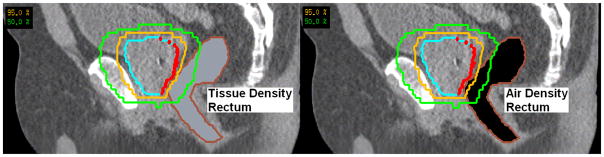
Figure 5 shows a sagittal image of the rectum contour with 95 % and 50 % isodose lines for actual tissue density and overridden (air-equivalent) tissue density, respectively. A slight, but perceivable increase in the volume receiving 95% prescription is noted for the air-equivalent density calculation. The prostate minimum, maximum, and mean doses as a percentage of prescription were 100.7, 107.3, and 104.5 % for the normal tissue density calculation, and 95.3, 108.0, and 104.7 % for the air-equivalent density calculation. The RP interface, minimum, maximum, and mean doses as a percentage of prescription were 102.0, 107.2, and 104.8 % for the actual tissue density calculation, and 93.4, 107.3, and 103.8 % for the air-equivalent density calculation. 100% of the prostate received 95% prescription dose for both air and tissue density rectum calculations. A dose decrement anterior to the rectum was noted for the air-equivalent density calculation, due to loss of lateral scatter at the air-tissue interface.
Figure 5.
Sagittal image showing 95% (orange) and 50% (green) isodose lines for a patient planned using a tissue density (left) compared to air-equivalent density (right) rectum structure. The rectum-prostate interface structure is shown in red.
Dose Effect of Prostate Motion
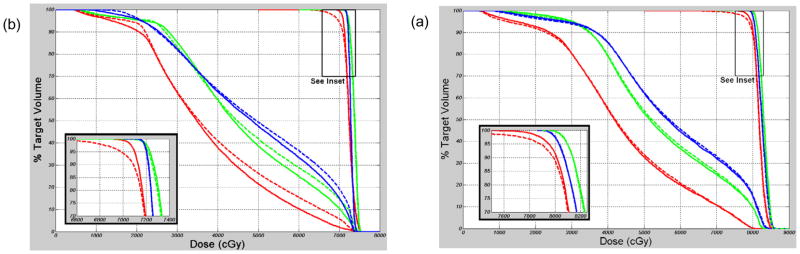
For 0, 3, and 5 mm PTV margins, adequate treatment was obtained in 3/15, 12/15, and 15/15 patients, and the delivered Dmin ranged from 78–99, 96–100, and 99–100 percent of the planned Dmin, respectively. In Table IV, the actual delivered prostate Dmin is shown for each of the 15 patients and each PTV expansion as a percentage of the planned Dmin, Delivered dose is shown both for the first 10 fractions and for the entire radiotherapy course of each patient. The D95 (minimum dose covering 95% of the prostate) was not affected by motion for any of the PTV margins. Delivered D95 remained within 1 and 2% of planned D95 for 44/45 and 45/45 cases. Figure 6 shows example “actual” and planned prostate and rectum (within 2 cm of prostate) DVH curves for two patients (#4 and #6).
Table IV.
Minimum dose delivered to the prostate as a percentage of planned minimum dose for 0, 3, and 5 mm PTV margins, based on the first 10 fractions and the entire treatment course of each patient.
| Minimum Dose Delivered to Prostate as a percentage of planned minimum dose (Goal >98%) | ||||||
|---|---|---|---|---|---|---|
| 0 mm PTV | 3 mm PTV | 5 mm PTV | ||||
| Patient Number | 10 fx | All | 10 fx | All | 10 fx | All |
| 1 | 99 | 99 | 100 | 100 | 100 | 100 |
| 2 | 94 | 93 | 99 | 99 | 100 | 100 |
| 3 | 89 | 89 | 96 | 96 | 99 | 99 |
| 4 | 89 | 89 | 96 | 96 | 100 | 99 |
| 5 | 92 | 97 | 99 | 100 | 99 | 100 |
| 6 | 86 | 78 | 100 | 99 | 100 | 100 |
| 7 | 96 | 97 | 100 | 100 | 100 | 100 |
| 8 | 90 | 88 | 99 | 100 | 101 | 101 |
| 9 | 94 | 97 | 100 | 100 | 101 | 100 |
| 10 | 95 | 93 | 101 | 101 | 100 | 100 |
| 11 | 92 | 96 | 98 | 99 | 101 | 101 |
| 12 | 92 | 92 | 100 | 100 | 100 | 100 |
| 13 | 98 | 99 | 100 | 100 | 100 | 100 |
| 14 | 88 | 87 | 97 | 97 | 100 | 100 |
| 15 | 98 | 99 | 100 | 100 | 100 | 100 |
Figure 6.
Dose Volume Histogram (DVH) curves for patient #4 (a) and patient #6 (b), illustrating “actual” (dotted) and planned (solid) dose for the CTV (see inset) and rectum, for 0 mm (red), 3 mm (green), and 5 mm (blue) PTV margins.
Correlation of Prostate Motion With Treatment Adequacy
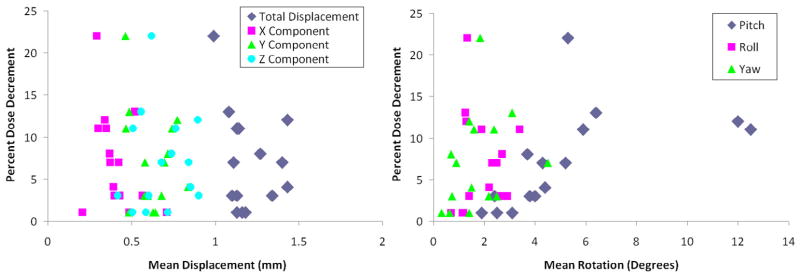
Figure 7 shows the dose decrement (change in actual vs. planned minimum prostate dose) as a function of mean pitch, roll, yaw, and displacement along each x (left-right), y (superior-inferior), and z (anterior-posterior) direction for all patients, using a 0 mm PTV expansion. No correlation was noted between dose decrement and mean pitch, roll, yaw, or any component of translational motion for any of the PTV expansions.
Figure 7.
Left: Dose decrement as a function of mean isocenter displacement (left) along the x (left-right), y (superior-inferior), z (anterior-posterior) axes, and composite displacement calculated using the distance formula. Right: Dose decrement as a function of mean intra-fraction pitch, roll, and yaw of the prostate for all patients based on 0 mm PTV plans.
Prediction of Treatment Adequacy Based on Initial Treatments
For 30 cases of adequate treatment, motion analysis using only the first 3, 5, and 10 fractions predicted adequacy of the entire radiotherapy course in 26/30, 28/30, and 30/30 cases. For 15 cases of inadequate treatment, analysis using only the first 3, 5, and 10 fractions predicted inadequacy in 13/15, 14/15, and 15/15 cases, revealing a need for PTV expansion. Table IV shows delivered dose to the prostate as a percentage of planned minimum dose for each PTV margin, based on the first 10 fractions and the entire treatment course for each patient.
Impact of Translational and Rotational Motion on Rectum Dose
The volumes of rectum within 2 cm of the prostate and receiving greater than 40 Gy (V40) or greater than 65 Gy (V65) were not uniformly affected by prostate translational and rotational motion (Student’s paired two tailed t test, p= 0.8 and 0.6 for 3 mm and 5 mm comparison of V40, and p= 0.6 and 0.9 for 3 mm and 5 mm comparison of V65), although some patient specific variability was observed (Figure 6)
DISCUSSION
Our data confirms variation in intra-fraction motion among patients that has been previously described(10, 11, 13) and provides the first analysis of the dosimetric implications of intra-fraction motion, taking into account both translation and rotation. Li et al(18) studied intra-fraction translational motion using tracking data from 35 patients, applied to IMRT plans from two patients; and found that a 2mm margin was sufficient. In our analysis, we accounted both for translational and rotational motion, and used each patient’s treatment plan for the dose calculation, which showed the need for a larger margin in some patients.
Prior analyses of rotational motion have not accounted for individual patient anatomy. A previously published dosimetric study(19) suggested that prostate rotations greater than 5 degrees may reduce 98% PTV coverage. For patients in our study (#3, #4, #14) where 3 mm plans were insufficient, mean pitch rotation was greater than 5 degrees. However, such a degree of rotational motion did not always correlate with a decrement in dose delivered to the prostate.
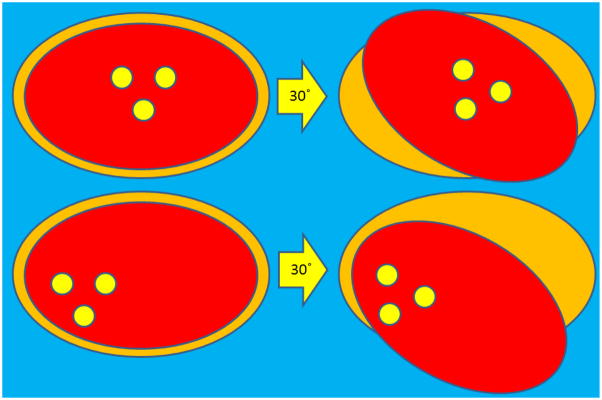
We attempted to determine a correlation between specific motion factors (pitch, roll, yaw, displacement) and decrement in dose delivered. Interestingly, we were unable to determine any generalized motion parameters that would predict a decrement in delivered prostate dose. Figure 7 shows the poor correlation between the degree of prostate rotation/translation and dose decrement. The weakness of a generalized rotation threshold is shown by different effects of implant geometry, illustrated in Figure 8. Rotations may take on increased or decreased significance due to a “lever arm” effect if the axis of measurement is at the periphery of the organ of interest. This effect likely contributes to the poor correlation between measured patient motion factors and dose decrement.
Figure 8.
Schematic to illustrate the variable effect of transponder geometry as a gauge for rotation measurements. The fiducial markers are depicted by yellow circles, within the prostate (red) and PTV (orange). 30 degree rotations are shown about the centroid of the three beacons for idealized (top) and skewed (bottom) fiducial geometry. A rotation of a given magnitude (30 degrees in this example) will carry different implications depending on the reference axis of rotation.
Our clinic uses Dmin as a metric to define treatment adequacy, and we propose Dmin as the most applicable parameter for prostate motion analysis due to the small prostatic volumes affected. The absence of a significant change in D95 for any patient confirms that dose alterations were only seen in a relatively small volume (<5%) of prostate.
Treatment plans using 5 mm PTV margins resulted in sufficient dose delivered to the prostate for all 15 patients in the cohort. This suggests that when using real-time tracking with careful setup to <10 degrees of initial rotation and a 3 mm action level, 5 mm PTV margins are sufficient to overcome any adverse effects of intra-fraction organ rotation. Using our institutional definition of a dosimetrically “sufficient” treatment, 12/15 patients would have received sufficient dose to the prostate with 3 mm PTV margins. Using only the first 3, 5, and 10 fractions, adequacy or inadequacy of treatment was predicted for 39/45, 42/45, and 45/45 cases. The relatively high predictive accuracy (87%) using motion only during the first 3 fractions is surprising, but consistent with prior reports of motion heterogeneity between patients, although not necessarily between fractions for a given patient(10, 11, 13).
Our model applied rigid transformations to structures of interest within a static dose cloud. To gauge the impact of tissue density variation, we calculated prostate and rectum-prostate interface dose for a patient with a distended rectum, with air-equivalent density manually assigned to the entire rectum structure. Loss of lateral scatter resulted in decreased dose at the air-tissue interface, and reduced attenuation in air resulted in a slight increase to mean and maximum prostate dose. Review of isodose lines (Figure 5) and full dosimetric parameters (see Results) revealed that although the effect was relatively subtle, a radical change in rectum composition can cause inadequate treatment delivery, when adequacy is defined by Dmin, actual ≥ 98% Dmin, planned. While this example illustrates the potential dose effect of a radical change in rectal composition, this has not been observed in clinical practice. For recalculation of daily dose using megavoltage CT imaging, Kupelian et al reported <2% variation in delivered compared to planned dose to 95% of the prostate(20).
We used inter-transponder distance variability to evaluate the rigid body approximation and found deformation greater than 0.5 and 1.5 mm occurred less than 16% and 1% of the total treatment time, respectively. Our data support observations from a recent CT-based imaging study suggesting that the prostate may be treated as a rigid body(21). An MRI-based deformation study by Nichol et al(22) revealed random inter-fraction deformation uncertainty of 1.5 mm, and average reduction in prostate volume of 0.5% per fraction during radiotherapy. Given the uncertainties of 0.8 mm and 0.4 mm with regard to the position of serial prostate contours and fiducial centroid localization, the data supports minimal impact of prostate deformation in the presence of physiologic rectal filling. Although reductions in prostate volume during radiotherapy were not accounted for in our model, a reduced prostate volume could potentially improve target coverage, since a reduction in target size effectively increases the PTV margin. The integration of real-time volumetric imaging during radiation therapy delivery would definitively answer questions about deformation and volume change, but were beyond the scope of the current study. Several groups are currently working towards development of magnetic resonance guided radiotherapy, which would potentially solve this problem by real-time dose recalculation(23–25).
Of note, our analysis applies only to treatment of the prostate while supine, and not treatment of the seminal vesicles or regional lymph nodes. Also, application of this technique to proton radiation therapy may be limited since changes in tissue homogeneity can have significant dosimetric consequences.
CONCLUSION
We have provided a description of intra-fraction translational and rotational motion of the prostate and a method to evaluate the sufficiency of individualized patient margins using real-time tracking. Our “automated” adaptive process does not require additional segmentation or image analysis workload for the physician and is ready for implementation in the clinic.
Acknowledgments
This research was first presented at the 2009 ASTRO annual meeting
Footnotes
Conflict of Interest Notification:
Research interface provided by Calypso Medical Technologies. Dr. Parikh receives research funding from Calypso Medical Technologies. Supported in part by NCI R01CA134541.
Publisher's Disclaimer: This is a PDF file of an unedited manuscript that has been accepted for publication. As a service to our customers we are providing this early version of the manuscript. The manuscript will undergo copyediting, typesetting, and review of the resulting proof before it is published in its final citable form. Please note that during the production process errors may be discovered which could affect the content, and all legal disclaimers that apply to the journal pertain.
References
- 1.Beckendorf V, Guerif S, Prise EL, et al. 70 Gy versus (vs) 80 Gy Dose Escalation Getug 06 French Trial for Localized Prostate Cancer: Mature Results. International Journal of Radiation Oncology, Biology, Physics. 2008;72:S96–97. [Google Scholar]
- 2.Dearnaley DP, Sydes MR, Graham JD, et al. Escalated-dose versus standard-dose conformal radiotherapy in prostate cancer: first results from the MRC RT01 randomised controlled trial. [see comment] Lancet Oncology. 2007;8:475–487. doi: 10.1016/S1470-2045(07)70143-2. [DOI] [PubMed] [Google Scholar]
- 3.Kuban DA, Tucker SL, Dong L, et al. Long-term results of the M. D. Anderson randomized dose-escalation trial for prostate cancer. [see comment] International Journal of Radiation Oncology, Biology, Physics. 2008;70:67–74. doi: 10.1016/j.ijrobp.2007.06.054. [DOI] [PubMed] [Google Scholar]
- 4.Peeters ST, Heemsbergen WD, Koper PC, et al. Dose-response in radiotherapy for localized prostate cancer: results of the Dutch multicenter randomized phase III trial comparing 68 Gy of radiotherapy with 78 Gy. [see comment] Journal of Clinical Oncology. 2006;24:1990–1996. doi: 10.1200/JCO.2005.05.2530. [DOI] [PubMed] [Google Scholar]
- 5.Zietman AL, DeSilvio ML, Slater JD, et al. Comparison of conventional-dose vs high-dose conformal radiation therapy in clinically localized adenocarcinoma of the prostate: a randomized controlled trial. [see comment][erratum appears in JAMA. 2008 Feb 27;299(8):899–900] JAMA. 2005;294:1233–1239. doi: 10.1001/jama.294.10.1233. [DOI] [PubMed] [Google Scholar]
- 6.Aubry JF, Beaulieu L, Girouard LM, et al. Measurements of intrafraction motion and interfraction and intrafraction rotation of prostate by three-dimensional analysis of daily portal imaging with radiopaque markers. International Journal of Radiation Oncology, Biology, Physics. 2004;60:30–39. doi: 10.1016/j.ijrobp.2004.02.045. [DOI] [PubMed] [Google Scholar]
- 7.Chen J, Lee RJ, Handrahan D, et al. Intensity-modulated radiotherapy using implanted fiducial markers with daily portal imaging: assessment of prostate organ motion. International Journal of Radiation Oncology, Biology, Physics. 2007;68:912–919. doi: 10.1016/j.ijrobp.2007.02.024. [DOI] [PubMed] [Google Scholar]
- 8.Britton KR, Takai Y, Mitsuya M, et al. Evaluation of inter- and intrafraction organ motion during intensity modulated radiation therapy (IMRT) for localized prostate cancer measured by a newly developed on-board image-guided system. Radiation Medicine. 2005;23:14–24. [PubMed] [Google Scholar]
- 9.Kotte AN, Hofman P, Lagendijk JJ, et al. Intrafraction motion of the prostate during external-beam radiation therapy: analysis of 427 patients with implanted fiducial markers. International Journal of Radiation Oncology, Biology, Physics. 2007;69:419–425. doi: 10.1016/j.ijrobp.2007.03.029. [DOI] [PubMed] [Google Scholar]
- 10.Kupelian P, Willoughby T, Mahadevan A, et al. Multi-institutional clinical experience with the Calypso System in localization and continuous, real-time monitoring of the prostate gland during external radiotherapy. International Journal of Radiation Oncology, Biology, Physics. 2007;67:1088–1098. doi: 10.1016/j.ijrobp.2006.10.026. [DOI] [PubMed] [Google Scholar]
- 11.Willoughby TR, Kupelian PA, Pouliot J, et al. Target localization and real-time tracking using the Calypso 4D localization system in patients with localized prostate cancer. International Journal of Radiation Oncology, Biology, Physics. 2006;65:528–534. doi: 10.1016/j.ijrobp.2006.01.050. [DOI] [PubMed] [Google Scholar]
- 12.Kuban D, Pollack A, Huang E, et al. Hazards of dose escalation in prostate cancer radiotherapy. International Journal of Radiation Oncology, Biology, Physics. 2003;57:1260–1268. doi: 10.1016/s0360-3016(03)00772-7. [DOI] [PubMed] [Google Scholar]
- 13.Nederveen AJ, van der Heide UA, Dehnad H, et al. Measurements and clinical consequences of prostate motion during a radiotherapy fraction. International Journal of Radiation Oncology, Biology, Physics. 2002;53:206–214. doi: 10.1016/s0360-3016(01)02823-1. [DOI] [PubMed] [Google Scholar]
- 14.D’Amico AV, Whittington R, Malkowicz SB, et al. Pretreatment nomogram for prostate-specific antigen recurrence after radical prostatectomy or external-beam radiation therapy for clinically localized prostate cancer. Journal of Clinical Oncology. 1999;17:168–172. doi: 10.1200/JCO.1999.17.1.168. [DOI] [PubMed] [Google Scholar]
- 15.Olsen JR, Parikh PJ. Image Guided Radiation Therapy. 1. McGraw Hill; 2011. Calypso real-time localization and tracking for treatment of prostate cancer with external beam radiotherapy. [Google Scholar]
- 16.Noel CE, Santanam L, Olsen JR, et al. An automated method for adaptive radiotherapy for prostate cancer patients using continuous fiducial based tracking. Physics in Medicine & Biology. 2010;55:18. doi: 10.1088/0031-9155/55/1/005. [DOI] [PubMed] [Google Scholar]
- 17.Santanam L, Malinowski K, Hubenshmidt J, et al. Fiducial-based translational localization accuracy of electromagnetic tracking system and on-board kilovoltage imaging system. Int J Radiat Oncol Biol Phys. 2008;70:892–899. doi: 10.1016/j.ijrobp.2007.10.005. [DOI] [PubMed] [Google Scholar]
- 18.Li HS, Chetty IJ, Enke CA, et al. Dosimetric consequences of intrafraction prostate motion. International Journal of Radiation Oncology, Biology, Physics. 2008;71:801–812. doi: 10.1016/j.ijrobp.2007.10.049. [DOI] [PubMed] [Google Scholar]
- 19.Cranmer-Sargison G. A treatment planning investigation into the dosimetric effects of systematic prostate patient rotational set-up errors. Medical Dosimetry. 2008;33:199–205. doi: 10.1016/j.meddos.2007.06.005. [DOI] [PubMed] [Google Scholar]
- 20.Kupelian PA, Langen KM, Zeidan OA, et al. Daily variations in delivered doses in patients treated with radiotherapy for localized prostate cancer. Int J Radiat Oncol Biol Phys. 2006;66:876–882. doi: 10.1016/j.ijrobp.2006.06.011. [DOI] [PubMed] [Google Scholar]
- 21.Deurloo KE, Steenbakkers RJ, Zijp LJ, et al. Quantification of shape variation of prostate and seminal vesicles during external beam radiotherapy. [erratum appears in Int J Radiat Oncol Biol Phys. 2005 Apr 1;61(5):1609] International Journal of Radiation Oncology, Biology, Physics. 2005;61:228–238. doi: 10.1016/j.ijrobp.2004.09.023. [DOI] [PubMed] [Google Scholar]
- 22.Nichol AM, Brock KK, Lockwood GA, et al. A magnetic resonance imaging study of prostate deformation relative to implanted gold fiducial markers. International Journal of Radiation Oncology, Biology, Physics. 2007;67:48–56. doi: 10.1016/j.ijrobp.2006.08.021. [DOI] [PubMed] [Google Scholar]
- 23.Raaymakers BW, Lagendijk JJ, Overweg J, et al. Integrating a 1.5 T MRI scanner with a 6 MV accelerator: proof of concept. Phys Med Biol. 2009;54:N229–237. doi: 10.1088/0031-9155/54/12/N01. [DOI] [PubMed] [Google Scholar]
- 24.Kirkby C, Stanescu T, Rathee S, et al. Patient dosimetry for hybrid MRI-radiotherapy systems. Med Phys. 2008;35:1019–1027. doi: 10.1118/1.2839104. [DOI] [PubMed] [Google Scholar]
- 25.Dempsey J, Dionne B, Fitzsimmons J, et al. A Real-Time MRI Guided External Beam Radiotherapy Delivery System. Medical Physics. 2006;33:2254. [Google Scholar]