Abstract
Cataliotti and Gilchrist (1995) reported that, consistent with anchoring theory, the lightness of a black step in a reflectance staircase was not altered by moving a white step from a remote to an adjacent location. Recently, Economou, Zdravkovic and Gilchrist (2007) reported data supporting three additional predictions of the anchoring model (Gilchrist et al., 1999): 1) equiluminant incremental targets in staircase simultaneous lightness contrast stimuli appeared equally light; 2) the simultaneous lightness contrast effect was due mainly to the lightening of the target on the black surround; and 3) the strength of lightness induction was greatest for darker targets. We investigated similar stimuli using brightness/lightness matching and found, contrary to these reports, that: 1) the relative position of the steps in a luminance staircase significantly influenced their brightness/lightness; 2) equiluminant incremental targets in staircase simultaneous brightness/lightness contrast stimuli did not all appear equally bright/light; 3) an asymmetry due to a greater brightening/lightening of the target on the black surround was not general; and 4) darker targets produced larger effects only when plotted on a log scale. In addition, the ODOG model (Blakeslee & McCourt, 1999) did an excellent job of accounting for brightness/lightness matching in these stimuli.
INTRODUCTION
Cataliotti and Gilchrist (1995) sought to distinguish between local-contrast and anchoring explanations of lightness (perceived reflectance). In their view, contrast explanations of lightness depended on inhibitory interactions between neural units responding to luminance differences exclusively at the borders between targets and their immediate surrounds, and were therefore highly dependent on distance. Anchoring explanations, on the other hand, were posited to be independent of distance, requiring only that the highest luminance in the stimulus serve as an “anchor” and appear white. Cataliotti and Gilchrist (1995) tested these competing explanations by obtaining lightness matches to a series of stimuli containing one to five reflectance steps presented under Gelb lighting conditions. The five step stimulus had two configurations: 1) a sequential staircase in which the luminance of the steps was ordered from lowest to highest and 2) a disrupted staircase in which the highest luminance step (the white step), originally at the end of the staircase, was moved to a position between the two lowest luminance steps (the black step and the dark-gray step). Figure 1A illustrates an example of a five step sequential staircase stimulus on a low luminance background. The disrupted staircase is illustrated in Fig. 1B, although in that figure it appears on a background set to the mean luminance rather than on a dark background. Cataliotti and Gilchrist (1995) reported that the lightness of the black square was not affected by the proximity of the white (highest luminance) square. This result was obtained for both a within-subjects design, where all observers were exposed to both stimulus configurations, and for a between-subjects design in which individual subjects observed only one of the two staircase conditions. Cataliotti and Gilchrist (1995) concluded that their results favored an anchoring, as opposed to a local-contrast, explanation since a local-contrast mechanism would predict greater darkening of the black square when it was adjacent to the white square.
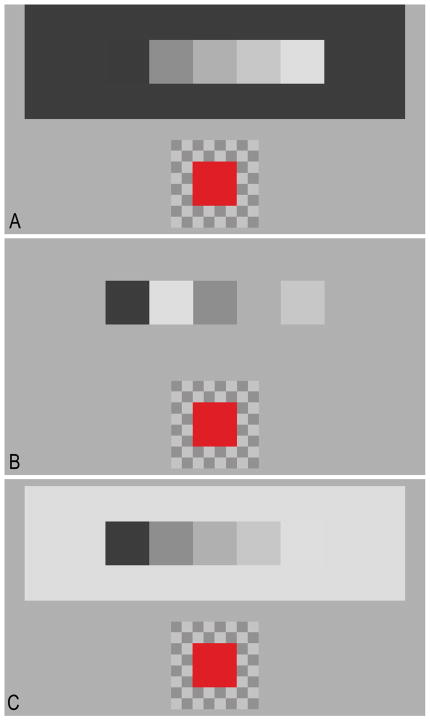
Figure 1.
The sequential (A and C) and disrupted (B) staircase stimuli on the 5 cd/m2 (A), 62 cd/m2 (B) and 119 cd/m2 (C) backgrounds used in Experiment 1. The matching patch (in red) and its checkerboard background are also illustrated.
Similarly, Economou, Zdravkovic and Gilchrist (2007) recently conducted a study using both paper and CRT displays in which they examined classical simultaneous lightness contrast stimuli and staircase simultaneous lightness contrast stimuli to determine whether a “lateral-inhibition” model or an anchoring model (Gilchrist et al., 1999; Gilchrist, 2006) provided a better explanation of simultaneous lightness contrast. In the classical simultaneous lightness contrast stimulus (also known as simultaneous brightness contrast1), a mean luminance/mid-gray test patch on a dark/black surround appears brighter/lighter than an identical test patch on a bright/white surround. An example of the staircase version of this stimulus, in which an incremental sequence of intermediate surrounds has been inserted between the dark/black (far left) and bright/white (far right) surrounds of the classical stimulus, is shown in Figure 4B. Economou et. al. (2007) used the term “lateral-inhibition models” to refer to a wide range of single- and multi-scale receptive field models (Cornsweet, 1970; Jameson & Hurvich,1964; Watt & Morgan, 1985; Kingdom & Moulden, 1992; Morrone & Burr, 1988; Pessoa, Mingolla & Neumann, 1995; Grossberg & Todorovic, 1988; Heinemann & Chase, 1995; and McArthur & Moulden, 1999), however, they specifically identified the oriented difference-of-gaussians (ODOG) model of Blakeslee and McCourt (1999; 2001; 2004) as representative of modern lateral-inhibition models.
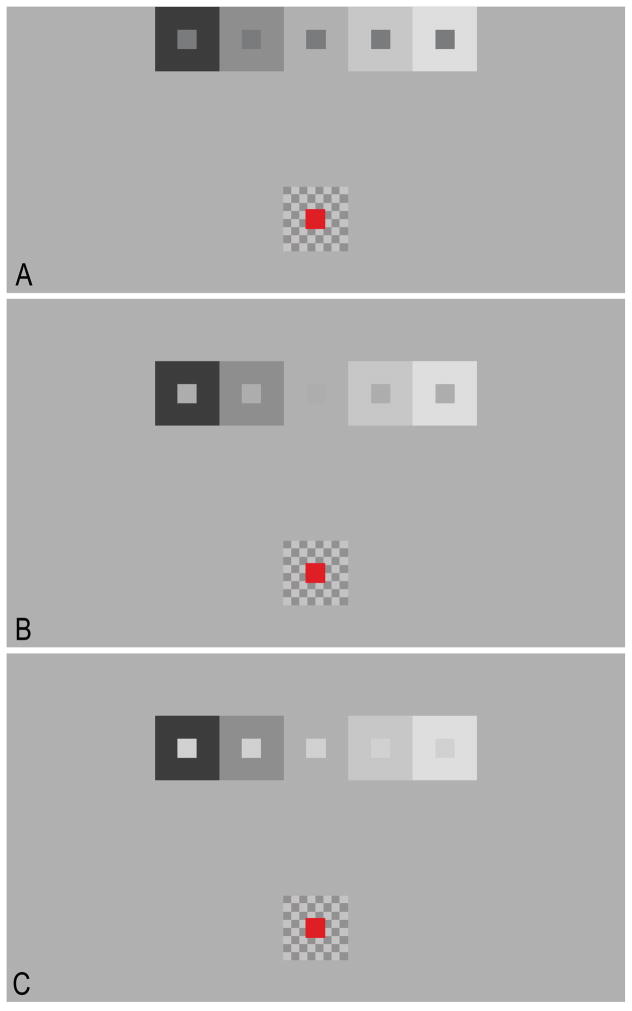
Figure 4.
The staircase simultaneous brightness/lightness contrast stimuli used in Experiment 2. Background luminance was held constant and equiluminant test patches were added to the centers of each step of the sequential staircase to produce a staircase simultaneous brightness/lightness contrast stimulus. Three test patch luminances: 24 cd/m2 (A), 60 cd/m2 (B), and 100 cd/m2 (C) were used. The matching patch (in red) and its checkerboard background are also shown.
Economou et al. (2007) tested three predictions of the anchoring model. First, the anchoring model predicts that in staircase simultaneous lightness contrast stimuli equiluminant targets that are luminance increments against their local surround, irrespective of the luminance of the surround, will appear equal. In other words, the anchoring model predicts no differential simultaneous lightness contrast effect for equiluminant incremental targets due to the fact that each is assigned a value of white in its local framework (i.e., the target and its immediate surround), and since the targets are equiluminant, each is assigned the same value (relative to the highest luminance in the display) in the global framework. Second, the model predicts that the majority of the lightness difference between the equiluminant targets in a simultaneous lightness contrast display will be due to a lightening of the target on the black surround as a result of the mismatch between the target’s assigned lightness value in the local framework (white) and its assigned value in the global framework. Third, the lightening of the target on the black surround is predicted to be greater for darker targets because the mismatch between the target’s assigned value in the local framework (white) and its assigned value in the global framework will be larger for the darker targets. Economou et al. (2007) reported evidence to support all of these predictions and argued that lateral inhibition models in general, and the ODOG model in particular (Blakeslee & McCourt, 1999; 2001; 2004), could not account for these effects.
There are several reasons to carefully reexamine the findings from both of the above studies. First, lateral inhibition in multiscale models such as the ODOG model differs significantly from the older local-contrast conception (Cornsweet, 1970; Fiorentini et al., 1977) which was tested against anchoring theory in the Cataliotti and Gilchrist (1995) study. In the local-contrast conception, lateral-inhibition is ascribed to the operation of filters (receptive fields) at a single and relatively small spatial scale. The responses of small filters are, of course, restricted to spatial regions of stimuli containing high spatial frequencies, such as edges. The local contrast conception stemmed from the pervasive, but erroneous, notion that lateral inhibition was a mechanism exclusively devoted to detecting and/or enhancing local edge contrast. In a multiscale filter model, such as the ODOG model, lateral-inhibition occurs across multiple spatial scales and the distances involved depend on the space-constants of the various filters. For the largest filter of the ODOG model the surround space constants for the two orientations are 3° and 6°, resulting in inhibitory interactions that extend across very large distances (20° or more). Like a local-contrast model, a multiscale filter model will also predict differences in the brightness/lightness of the patches in the sequential and disrupted luminance staircases. Cataliotti and Gilchrist (1995); however, measured only the lightness effect on the black (lowest luminance) step. Since one would not expect induced lightness differences to be particularly large for this stimulus configuration, in which the inducing regions share only a single edge (Heinemann, 1972), and since there is no reason to expect that lightness induction will be largest for the lowest luminance step, it is of interest to carefully reexamine brightness/lightness matching for all of the patches in sequential and disrupted staircases and to compare them with the predictions of the ODOG (Blakeslee & McCourt,1999; 2004) and anchoring models (Gilchrist et al.,1999; Gilchrist, 2006).
In addition, the finding of Economou et al. (2007) that lightness matches were identical for equiluminant incremental target patches placed on variable surrounds is itself controversial. Economou et al. (2007) cite eight studies that, in their view, also failed to find differential simultaneous brightness/lightness contrast effects for incremental targets (Agostini & Bruno, 1996; Arend & Spehar, 1993b; Diamond, 1953; Heinemann, 1955; Kozaki, 1963;1965; Gilchrist, 1988; Jacobsen & Gilchrist, 1988). However, a careful examination of these studies reveals that this conclusion may not be justified. For example, in Heinemann’s experiment (1955, Fig. 3; 1972, Fig. 2) a matching patch (which he called a comparison patch) on a dark surround was adjusted to match a target patch (which he called a test patch) as a function of target (test) patch inducing surround. Within the range where the target (test) patches were increments relative to their inducing surrounds, Heinemann found, at first, a slight but consistent increase in the brightness of the target (test) patches, followed by a depression in target (test) patch brightness that became precipitous as the luminance of the target (test) patch approached that of the inducing surround. It seems inaccurate, therefore, to cite Heinemann’s work as evidence for no effect of inducing surround on the brightness of target (test) patches that are increments. Arend and Spehar (1993b) also found a differential effect of surround luminance on matching (test) patch brightness/lightness judgments for target (standard) patch increments. Interestingly, they found that the magnitude of this differential effect depended on the particular stimulus conditions in the far surround of the stimulus (see their Fig. 3 and Fig. 8). The effect was quite small and, in agreement with Economou et al. (2007), appeared absent for one of the three subjects for matching (test) patch increments on variable surrounds placed on Mondrian backgrounds of fixed luminance. The effect, however, was very apparent for uniform outer backgrounds that were the same luminance (i.e., that co-varied with) the inner surround. Note that the brightness and lightness matching criteria in this study produced equivalent results under both of these surround conditions. The Kozaki (1963) study also showed that the inducing surround exerts an effect on the brightness of target (test) patches that are increments. Equal reflectance test patches decreased in brightness/lightness with increasing inducing surround reflectance. Diamond (1953), in agreement with Economou et al. (2007), reports little effect. However, it is possible that a small effect could easily have been missed in the Diamond (1953) study since the inducing and target (test) patches were adjacent squares, a stimulus configuration that produces much weaker induction than when the inducing field completely surrounds the target (test) patch (Heinemann, 1972). In addition, two older demonstrations (Cornsweet, 1970, pg 279; Shapley, 1986) as well as two recent studies specifically designed to test the hypothesis that surround luminance has no differential effect on incremental target patch brightness/lightness (Bressan & Actis-Grosso, 2001; Rudd & Zemach, 2005) clearly show an effect of background luminance on the brightness of test patch increments and contradict the results of Economou et al. (2007). Bressan and Actis-Grosso (2001) demonstrated that while a differential simultaneous lightness contrast effect is observed for incremental target patches, and thus does not support the predictions of the anchoring model (Gilchrist et al., 1999; Gilchrist, 2006), the strength of the effect depends critically on both the surround and target patch luminances. For example, they found that the inducing effect of surround luminance, i.e., the magnitude of perceptual darkening of the target patch as a function of increasing surround luminance, increased with target patch luminance, but that the effect was not significant until target patch luminance exceeded 29.75 cd/m2. Bressan and Actis-Grosso (2001) explained what they thought were failures of previous studies or conditions (Heinemann, 1955; Gilchrist, 1988; Arend & Spehar, 1993b) to demonstrate a differential lightness contrast effect for incremental target patches on variable surrounds, to surround and/or target patch luminances that were not optimal for producing the effect. According to this explanation the target patches used in the Economou et al. (2007) study should have been of sufficient luminance (34.26 cd/m2) to elicit only a small effect. Interestingly, however, Rudd and Zemach (2005) used very low luminance targets (tests) and surrounds (e.g., the incremental test patches never exceeded 3.16 cd/m2) but found nonetheless that luminance matches to incremental target (test) patches were influenced by the luminance of their surrounds and, like Bressan and Actis-Grosso (2001), concluded that their results contradicted predictions of the anchoring model (Gilchrist et al.,1999). Rudd and Zemach (2005) discussed the possibility that one reason their results differed from previous studies was the limited number of matching steps available with the 16-step Munsell matching scales used in several of the previous studies (Gilchrist, 1988; Jacobsen & Gilchrist, 1988; Agostini & Bruno, 1996). The coarseness of the matching scales could easily mask the differences observed in studies employing finer scale luminance matching (Heinemann, 1955; Arend & Spehar, 1993b; Bressan & Actis-Grosso, 2001; Rudd & Zemach, 2005). This explanation for the failure to detect an effect of background luminance on the brightness/lightness of incremental target (test) patches could also apply to the Economou et al. (2007) study. The controversy associated with this topic made it of interest to reexamine staircase simultaneous brightness/lightness contrast stimuli for both target patch increments and decrements and to compare these data with the predictions of the ODOG (Blakeslee & McCourt, 1999; 2001; 2004) and anchoring models (Gilchrist et al., 1999; Gilchrist, 2006).
Figure 3.
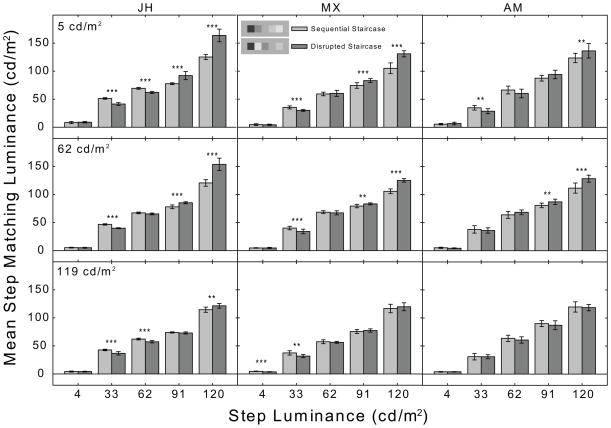
Bar graph plotting mean matching luminance for each step of the sequential (light-gray) and disrupted (dark-gray) staircases for observers JH, MX, and AM. See Fig. 2 for details.
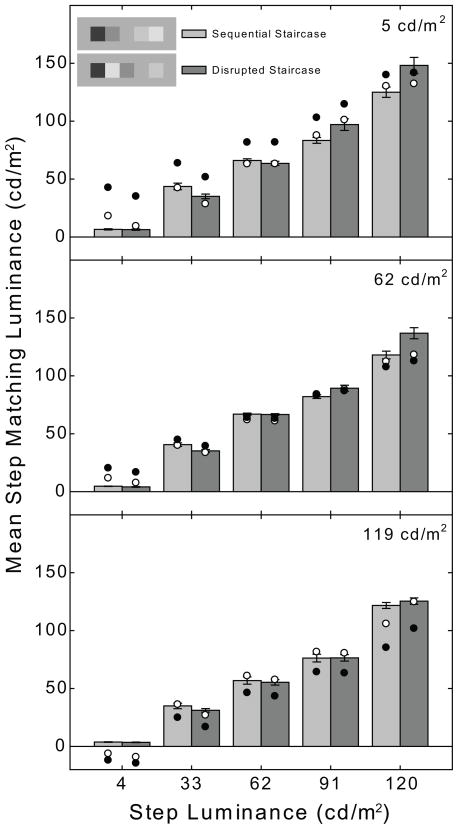
Figure 2.
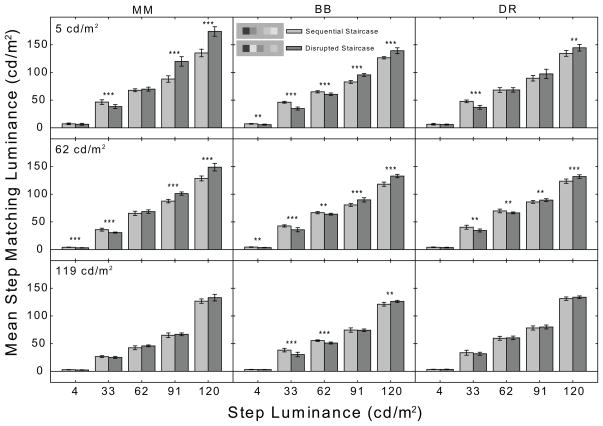
Bar graph plotting mean matching luminance for each step of the sequential (light-gray) and disrupted (dark-gray) staircases for observers MM, BB, and DR. The matches on the 5 cd/m2, 62 cd/m2, and 119 cd/m2 backgrounds are plotted separately in the top, middle and bottom panels, respectively. The error bars are 95% confidence intervals. The step luminances at which significant mean matching luminance differences were found between the sequential and disrupted staircases are indicated by asterisks.
Figure 8.
Bar graphs showing the group-averaged mean matching luminance for each step of the sequential (light-gray bars) and disrupted (dark-gray bars) staircases from Experiment 1. Group-averaged mean matching luminance to steps on the 5 cd/m2, 62 cd/m2, and 119 cd/m2 backgrounds is plotted separately in the top, middle and bottom panels, respectively. The error bars are 95% confidence intervals. Black symbols represent the predictions of the ODOG model with no adjustment for mean luminance (K=2) and white symbols plot predictions of the mean luminance-adjusted model (K=1).
Experiment 1 examines sequential and disrupted staircase configurations presented on a CRT rather than as papers under Gelb illumination (Cataliotti and Gilchrist,1995). In addition, it measures the brightness/lightness of each step, not just that of the lowest luminance step, and extends the results to three background luminance levels (Fig. 1). In Experiment 2 the background is set to the mean luminance of the display and equiluminant test patches are positioned at the center of each step of the sequential staircase to produce a staircase simultaneous brightness/lightness contrast stimulus. The brightness of each test patch is measured for three levels of test patch luminance which allows a test of the various predictions from the Economou et al. (2007) study (Fig. 4). The matching data from both experiments are compared directly to the predictions of the anchoring model (Gilchrist et al., 1999; Gilchrist, 2006) and to predictions of the multiscale ODOG filtering model (Blakeslee & McCourt, 1999; 2001; 2004).
EXPERIMENT 1
Methods
The three authors (BB, DR, and MM) and three naive observers (JH, MX, and AM) participated in the experiments. All six observers possessed normal or corrected-to-normal vision. Each observer provided informed consent and protocols were approved by the NDSU IRB.
Stimuli were presented on a BrightSide DR37-P (Dolby Laboratories, Inc.) high dynamic range display. This display possesses a backlight consisting of an array of 1380 individually controlled high-intensity LEDs, and a front panel consisting of a high-resolution (1920 × 1080 pixel) LCD. The Brightside DR37-P has two operating modes, HDR and LCD. In HDR mode, it performs inverse gradient correction on edges which exceed the resolution of the spatially modulated LED backlight. We did not use the display in this mode for any of our experiments. Rather, we used the display in LCD mode where the backlight is uniform and the LCD image is unprocessed, functioning similarly to a standard LCD display. The advantage conferred by this display is that the overall luminous intensity of the backlight can be set to much higher intensities than those available using standard LCD displays. From a viewing distance of 110 cm the entire display subtended 40.9° × 23.0°. LCD frame refresh rate was 60 Hz. Detailed photometric calibration of each stimulus was performed using a spot photometer (Konica Minolta LS-110).
Observers viewed the display by placing their face against a molded viewing aperture. The aperture was mounted on a tripod, and was attached to the margins of the display by a black felt hood which excluded any other source of light from the observer’s field of view. The stimulus consisted of five spatially abutting homogeneous square patches (4.26° × 4.26°) which formed the steps of a luminance staircase. Step luminances were 4 cd/m2, 33 cd/m2, 62 cd/m2, 91 cd/m2, and 120 cd/m2. Two versions of the staircase were used, one in which the luminance steps were arranged sequentially (Fig. 1A, C), and another in which the staircase was disrupted by placing the highest luminance step in the second position of the staircase (Fig. 1B). Each staircase (sequential and disrupted) was presented on three different background luminances: 5 cd/m2 (Fig. 1A); 62 cd/m2 (Fig. 1B); and 119 cd/m2 (Fig. 1C), that subtended 36.92° × 11.11°. A matching patch (4.26° × 4.26°) was located 11.83° (center-to-center) below the staircase, and was presented on a checkerboard background (8.52° × 8.52°) whose individual checks measured (1.06° × 1.06°). The luminances of the dark and bright checks of the matching patch background checkerboard were 38 cd/m2 and 86 cd/m2, respectively (i.e., 38.7% contrast). All regions of the display not occupied by the staircase and its background, or by the matching patch and its background, were set to a luminance of 62 cd/m2.
On each trial a small dark dot appeared beneath the step of the luminance staircase which was to be matched, and observers adjusted the luminance of the matching patch (in steps of 0.5% maximum luminance) using arrow keys until the brightness of the matching patch was judged to equal that of the target step in the luminance staircase. Observers were instructed to make brightness matches by “adjusting the matching patch to match the intensity of light coming from the test patch”. Note, however, that under the homogeneous illumination conditions of the present experiment lightness and brightness matches would produce equivalent results (Arend & Spehar, 1993a; 1993b; Blakeslee, Reetz & McCourt, 2008). Observers indicated a satisfactory match by depressing a “done” button. Final adjustment settings were recorded by computer, which also controlled the matching sequences and presentation of stimuli. On each trial the initial luminance of the matching patch was randomized, as was the step within the staircase which was to be matched. Observers made 10–12 brightness match settings for each of the five staircase steps of the sequential and disrupted staircases, on each of the three background luminances.
Results and Discussion
The bar graphs in Figures 2 and 3 plot individual observers’ mean matching luminances for each step of the sequential (light-gray bars) and disrupted (dark-gray bars) staircases. The matching luminances on the 5 cd/m2, 62 cd/m2, and 119 cd/m2 backgrounds are plotted separately in the top, middle and bottom panels, respectively. The error bars are 95% confidence intervals. At each background luminance we performed a two-way between-subjects ANOVA with staircase type (sequential versus disrupted) and step luminance (4 cd/m2, 33 cd/m2, 62 cd/m2, 91 cd/m2 and 120 cd/m2) as factors. In cases where a significant interaction term was observed the source of the interaction was traced using a series of five independent-samples t-tests which compared mean matching luminance in the two staircase conditions at each level of step luminance. Each post-hoc comparison was conducted using a Bonferroni-corrected alpha level of 0.01.
On the 5 cd/m2 background, the condition most similar to that in the Gilchrist and Cataliotti (1995) study, (Figs. 2 & 3, upper panels) MM, BB, JH, and MX showed a significant main effect of staircase type [MM: F(1, 90) = 152.87, p<.001; BB: F(1, 90) = 10.67, p<.002; JH: F(1, 90) = 62.03, p<.001; MX: (1, 90) = 45.02, p<.001]. All six observers showed a significant main effect of step luminance [MM: F(4, 90) = 2422.08, p<.001; BB: F(4, 90) = 7345.95, p<.001; DR: F(4,100) = 2381.73, p<.001; JH: F(4, 90) = 2391.77, p<.001; MX: F(4, 90) = 1859.19, p<.001; AM: F(4, 90) = 1054.05, p<.001], and a significant staircase type x step luminance interaction [MM: F(4, 90) = 83.13, p<.001; BB: F(4, 90) = 92.21, p<.001; DR: F(4,100) = 15.73, p<.001; JH: F(4, 90) = 93.70, p<.001; MX: F(4, 90) = 36.23, p<.001; AM: F(4, 90) = 7.46, p<.001]. Neither of the main effects is particularly informative for the purposes of the present study. The main effect of staircase type, where present, reveals that the mean matching luminance (collapsed across all steps) is significantly greater in the disrupted staircase condition. The main effect of step luminance simply reveals that mean matching luminance generally tracks step luminance when collapsed across the sequential and disrupted staircase conditions. Of primary interest to the present study, however, is the significant interaction which is due to significant differences in mean matching luminance between specific steps of the sequential and disrupted staircases. The source of this interaction was traced using five independent-samples t-tests. Bonferroni-corrected (alpha level = 0.01) significant differences for each luminance step are indicated by asterisks in Figs. 2 and 3. All six subjects showed significantly higher matching luminances for the 120 cd/m2 step when it was located next to the 4 cd/m2 step in the disrupted staircase, and lower matching luminances for the 33 cd/m2 step when it was located next to the 120 cd/m2 step in the disrupted staircase. All subjects except DR and AM also showed significantly higher matching luminances for the 91 cd/m2 step in the disrupted staircase; however, only BB and JH showed a significant darkening of the 62 cd/m2 step in the disrupted staircase. Importantly, note that only BB showed a significant darkening of the 4 cd/m2 step in the disrupted staircase condition.
The results for the 62 cd/m2 background condition were very similar. There was a significant main effect of staircase type for all subjects except DR [MM: F(1, 90) = 86.01, p<.001; BB: F(1, 90) = 26.28, p<.001; JH: F(1, 90) = 59.44, p<.001; MX: F(1, 90) = 31.29, p<.001; AM: F(1, 90) = 23.17, p<.001]. All six subjects showed a significant main effect of step luminance [MM: F(4, 90) = 4966.12, p<.001; BB: F(4,90) = 6203.50, p<.001; DR: F(4,100) = 6172.92, p<.001; JH: F(4, 90) = 2798.21, p<.001; MX: F(4, 90) = 4324.69, p<.001; AM: F(4, 90) = 1440.15, p<.001] and a significant staircase type x step luminance interaction [MM: F(4, 90) = 50.22, p<.001; BB: F(4,90) = 58.98, p<.001; DR: F(4,100) = 22.02; JH: F(4, 90) = 73.58, p<.001; MX: F(4, 90) = 57.69, p<.001; AM: F(4, 90) = 10.31, p<.001]. As indicated by the asterisks in Figs. 2 and 3, observer BB again showed significant differences in step brightness for sequential and disrupted staircases for all step luminances; MM showed significant differences for all but the 62 cd/m2 step and DR for all but the 4 cd/m2 step. MX and JH showed a significant difference in step brightness for all but the 4 cd/m2 and 62 cd/m2 steps while for AM only the 91 cd/m2 and 120 cd/m2 steps showed a significant difference.
Finally, on the 119 cd/m2 background, MM, BB, and JH showed a significant main effect of staircase type [MM: F(1, 90) = 10.19, p =.002; BB: F(1,90) = 9.25, p =.003; JH: F(1, 90) = 4.04, p =.047], all six subjects showed a significant main effect of step luminance [MM: F(4, 90) = 5169.21, p<.001; BB: F(4,90) = 6259.51, p<.001; DR: F(4,100) = 5185.43, p<.001; JH: F(4, 90) = 5939.31, p<.001; MX: F(4, 90) = 2321.47, p<.001; AM: JH: F(4, 90) = 1368.64, p<.001] and there was a significant staircase type x step luminance interaction for MM, BB, JH, and MX but not for DR or AM [MM: F(4, 90) = 5.20, p=.001; BB: (F(4,90) = 18.31, p<.001; JH: F(4, 90) = 21.80b, p<.001; MX: F(4, 90) = 3.50, p = .011]. BB and JH showed significant differences in mean matching luminance between the sequential and disrupted staircase conditions at step luminances of 33 cd/m2, 62 cd/m2 and 120 cd/m2 while MX showed significant differences at 4 cd/m2 and 33 cd/m2.
The above analysis clearly demonstrates that there are significant effects on the brightness of the steps in a luminance staircase when the highest luminance step in a sequential staircase is moved from its position at the end of the staircase to a position between the two lowest luminance steps. Although significant differences in brightness were observed for steps on all three background luminance levels, the number of steps showing a significant difference was clearly reduced on the highest background luminance compared to the other two, and was slightly larger for the 62 cd/m2 background. This pattern of results is generally consistent with the idea that the visual system is most sensitive to the small brightness differences resulting from the order of the staircase when the staircase is situated on a background equal to its mean (Whittle, 1986; 1992) due to luminance gain control mechanisms (Hood, 1998; Reeves, 2004; Mante et al. 2005).
EXPERIMENT 2
Methods
In the second experiment background luminance was held constant (62 cd/m2) and equiluminant test patches (1.28° × 1.28°) were added to the centers of each step of the sequential staircase (4 cd/m2, 33 cd/m2, 62 cd/m2, 91 cd/m2, and 120 cd/m2) to produce a staircase simultaneous brightness/lightness contrast stimulus. The brightness of each of the test patches was measured for three test patch luminances: 24 cd/m2 (Fig. 4A), 60 cd/m2 (Fig. 4B), and 100 cd/m2 (Fig. 4C). A matching patch (1.28° × 1.28°) was located 11.83° (center-to-center) below the staircase, and was situated on a checkerboard background (4.26° × 4.26°) whose individual checks measured 0.53° × 0.53°. The luminances of the dark and bright checks of the matching patch background checkerboard were 38 cd/m2 and 86 cd/m2, respectively (i.e., 38.7% contrast). All regions of the display not occupied by the staircase, or by the matching patch and its background, were set to a luminance of 62 cd/m2. All other details of Experiment 2 were identical to Experiment 1.
Results and Discussion
Figure 5 plots the data for the six observers separately in the six panels. Mean matching luminance is plotted as a function of surround luminance for three test patch luminance levels: 24 cd/m2 (black circles); 60 cd/m2 (gray circles); and 100 cd/m2 (white circles). Dotted symbols denote test patches that are decrements relative to the surround luminance. The error bars are 95% confidence intervals. We performed a two-way between-subjects ANOVA with test patch luminance (24 cd/m2, 60 cd/m2, and 100 cd/m2) and surround luminance (4 cd/m2, 33 cd/m2, 62 cd/m2, 91 cd/m2 and 120 cd/m2) as factors. In cases where a significant interaction term was observed the source of the interaction was traced using one-way ANOVAs and post-hoc pair-wise comparisons (Tukey HSD).
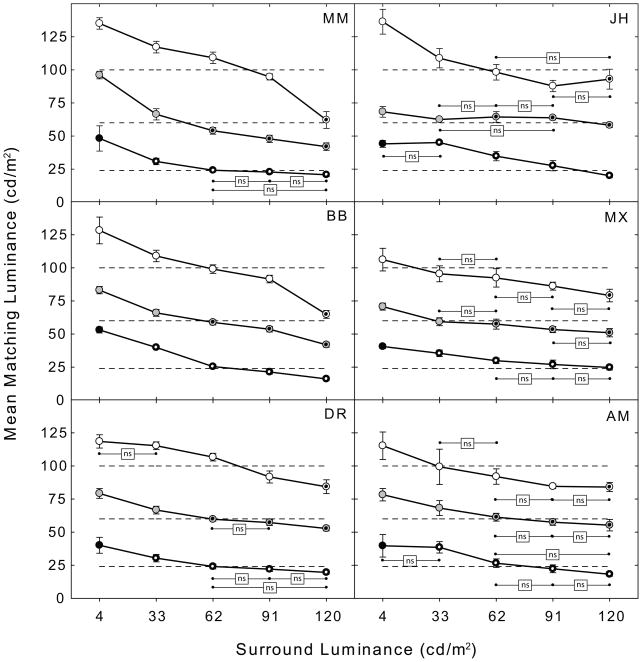
Figure 5.
Mean matching luminance as a function of surround luminance for three test patch luminance levels: 24 cd/m2 (black circles); 60 cd/m2 (gray circles); and 100 cd/m2 (white circles), are plotted separately for the six observers in the six panels. The dotted symbols denote test patches that are decrements relative to the surround luminance. The error bars are 95% confidence intervals. Non-significant pairwise post-hoc comparisons between the test patches (p< 0.05) are marked by the initials (ns).
All six subjects showed a significant main effect of test patch luminance [MM: F(2,135) = 4322.79, p<.001; BB: F(2,145) = 5484.37, p<.001; DR: F(2,150) = 6265.26, p<.001; JH: F(2,135) = 3024.00, p<.001; MX: F(2,135) = 2766.66, p<.001; AM: F(2,135) = 1623.53, p<.001], a significant main effect of surround luminance [MM: F(4,135) = 880.78, p<.001; BB: F(4,145) = 880.78, p<.001; DR: F(4,150) = 302.13, p<.001; JH: F(2,135) = 155.93, p<.001; MX: F(2,135) = 116.37, p<.001; AM: F(2,135) = 97.35, p<.001], and a significant test patch luminance x surround luminance interaction [MM: F(8,135) = 70.07, p<.001; BB: F(8,145) = 31.71, p<.001; DR: F(8,150) = 19.53, p<.001; JH: F(2,135) = 42.53, p<.001; MX: F(2,135) = 55.33, p<.002; AM: F(2,135) = 2.90, p<.005]. The main effect of test patch luminance is due to matching luminance in general tracking test patch luminance. Of more interest to the present study is the main effect of surround luminance and the test patch luminance x surround luminance interaction. The main effect of surround luminance is due to the relative decrease in matching luminance as surround luminance increases and the interaction is due to an asymmetry in the effect of surround luminance that is most prominent at low test patch luminances (black circles). All of the pair-wise post-hoc comparisons were significantly different (p< 0.05) except those marked by the initials (ns) in Fig. 5. Note that for the 100 cd/m2 test patches (white circles) the test patch is an increment relative to the surround luminance for all but the 120 cd/m2 surround. Nevertheless, all six subjects show clear decreases in matching luminance as a function of background luminance. This is also the case for the 60 cd/m2 test patches on the 4 cd/m2 and 33 cd/m2 backgrounds where the test patches are increments as well. In addition, although we observe asymmetries in induction on the lowest and highest luminance surrounds, a greater lightening of the targets on the lowest luminance surround was not general across all test patch luminance levels. For the 100 cd/m2 test patches (white circles) the magnitude of the induction effect from the 4 cd/m2 and 120 cd/m2 surrounds appears fairly symmetric (except for subject JH) relative to that on the 62 cd/m2 background. The results for the 60 cd/m2 test patches are somewhat mixed, however, a clear asymmetry i.e., a greater magnitude of induction on the lowest luminance surround, is observed for all subjects except JH for the 24 cd/m2 test patches. Finally, although matches to the test patch on the 4 cd/m2 background do not appear to show any clear trend to suggest that induction magnitude increases as test patch luminance decreases (Fig. 5, compare the white, gray and black circles on the 4 cd/m2 surround), darker test patches did produce larger induction effects when plotted as ratios relative to their surrounds i.e., on a log scale.
MODELING
The results of Experiments 1 and 2 indicate: 1) that the relative position of the steps in a luminance staircase significantly influences their brightness; 2) that the brightness of equiluminant incremental targets in staircase simultaneous brightness/lightness contrast stimuli is not constant; 3) that an asymmetry due to a greater lightening of the target on the dark (as opposed to the bright) surround is not general; and 4) that darker test patches produce larger effects only when plotted as ratios relative to their surrounds. Points one and two clearly contradict the predictions of the anchoring model (Cataliotti and Gilchrist, 1995; Gilchrist et al., 1999); however, they appear consistent with simultaneous brightness contrast explanations that posit effects resulting from the influence of neighboring regions on the brightness of the target. This class of explanation was tested by comparing the matching data from Experiments 1 and 2 with the predictions of the ODOG multiscale filtering model of brightness perception (Blakeslee & McCourt, 1999; 2001; 2004) for these same stimuli.
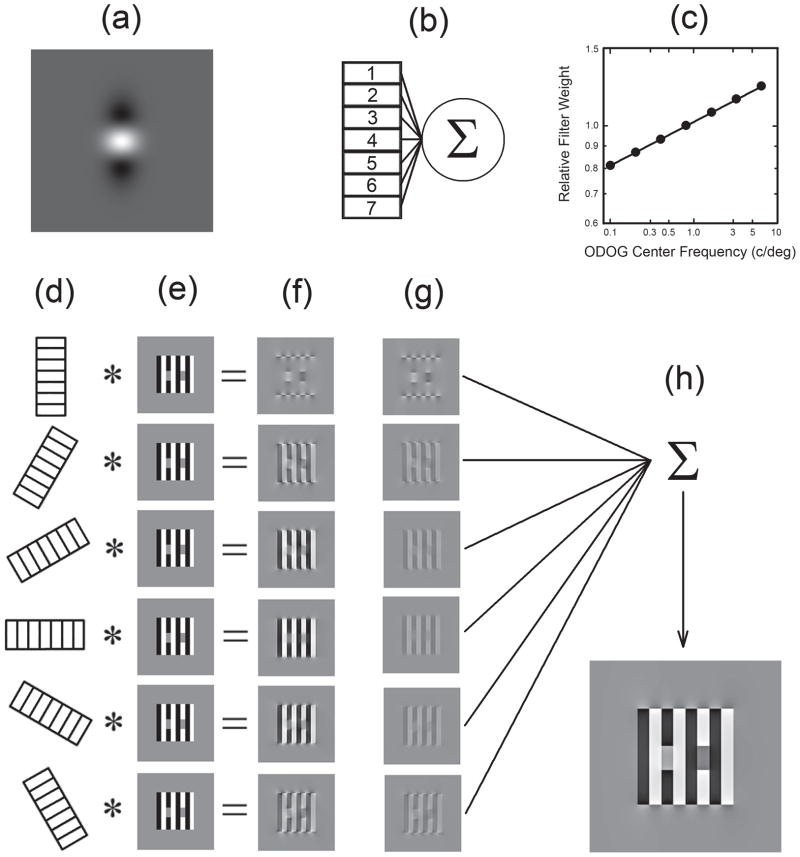
The oriented filters of the ODOG model are produced by setting the ratio of DOG center/surround space constants to 1:2 in one orientation and to 1:1 in the orthogonal orientation (Table 1). A gray level representation of an ODOG filter appears in Figure 6(a). The ODOG model is implemented in six orientations (0, 30, 60, 90 −30 and −60 degrees relative to vertical). Each orientation is represented by seven volume-balanced (i.e., integrate to 0) filters that possess center frequencies arranged at octave intervals (from 0.1–6.5 c/d). The seven filters [Fig. 6(b)] within each orientation are summed after weighting across frequency using a power function with a slope of 0.1 [Fig. 6(c)]. This slope is consistent with the shallow low-frequency fall-off of the suprathreshold contrast sensitivity function (Georgeson & Sullivan, 1975). The resulting six broadband (multiscale) spatial filters, one per orientation, are convolved with the stimulus of interest [Fig. 6(d–e)]. The six filter outputs [Fig. 6(f)] are normalized across orientation such that their RMS contrasts, as computed across the entire convolution output, are equal. To preserve the contrast response of the model this is achieved by multiplying the output images by a factor that makes their RMS contrast equal to that of the output image with the lowest RMS contrast [Fig. 6(g)]. The six normalized outputs are summed to produce the final ODOG model output [Fig. 6(h)]. The psychophysical linking hypothesis employed is that the univariate output of the ODOG model at each point in space is proportional to brightness (perceived luminance).
Table 1.
Oriented Difference of Gaussian Space Constants
| Mechanism | Space Constant (deg) | |||
|---|---|---|---|---|
| Center | Surround | |||
| X | Y | X | Y | |
| 1 | .047° | .047° | .047° | .093° |
| 2 | .094° | .094° | .094° | .188° |
| 3 | .188° | .188° | .188° | .375° |
| 4 | .375° | .375° | .375° | .75° |
| 5 | .75° | .75° | .75° | 1.5° |
| 6 | 1.5° | 1.5° | 1.5° | 3° |
| 7 | 3° | 3° | 3° | 6° |
Figure 6.
A diagrammatic representation of the oriented difference-of-Gaussian (ODOG) model. (a) A gray level representation of an ODOG filter. The oriented filters of the ODOG model are produced by setting the ratio of DOG center/surround space constants to 1:2 in one orientation and to 1:1 in the orthogonal orientation. (b) The ODOG model is implemented in 6 orientations (0, 30, 60, 90 −30 and −60 degrees relative to vertical). Each orientation is represented by seven volume-balanced (i.e., integrate to 0) filters that possess center frequencies arranged at octave intervals (from 0.1–6.5 c/d). The seven filters (b) within each orientation are summed after weighting across frequency using a power function with a slope of 0.1 (c). This slope is consistent with the shallow low-frequency fall-off of the suprathreshold contrast sensitivity function (Georgeson and Sullivan, 1975). The resulting six multiscale spatial filters, one per orientation, are convolved with the stimulus of interest (d–e). The filter outputs (f) are normalized across orientation by dividing each by its space-averaged root-mean-square contrast, as computed across the entire convolution output (g). The six normalized outputs are summed to produce the final ODOG model output (h).
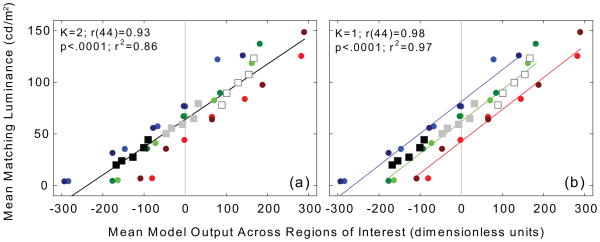
The scatterplot in Figure 7(a) illustrates the relationship between raw ODOG model output and psychophysical luminance matching. Mean matching luminance (collapsed across all observers) is plotted against mean ODOG model output for all 45 regions of interest in the stimuli of Experiments 1 and 2 (i.e., the five individual steps of the sequential and disrupted staircases on three background luminances, and the five test patches of the simultaneous brightness/lightness contrast stimuli at three levels of test patch luminance). Circular symbols plot data from the staircase stimuli of Experiment 1: red, green and blue refer to steps on the 5, 62, and 119 cd/m2 backgrounds, and bright and dark colors denote the sequential and disrupted staircase conditions, respectively. Square symbols plot data from simultaneous brightness/lightness contrast stimuli: black, gray and white refer to the 24, 60, and 100 cd/m2 test patches, respectively. The staircase data from the three background conditions and the simultaneous brightness/lightness contrast data for the three test patch luminance conditions were fit using least-squares regression (black line) to a linear function possessing two free parameters (K=2): a y-intercept and a slope. The fitted y-intercept is 63.9 cd/m2, and the slope is 0.268 cd/m2 per unit model output. The correlation between matching luminance and ODOG model output is highly significant, r(44)=0.93, p<.0001, and accounts for 86.5% of the total variance. It is, however, obvious that this single regression equation predicts luminance matches in some conditions, e.g., the 62 cd/m2 background staircase (green symbols) and all three simultaneous brightness/lightness contrast stimuli (black, gray and white symbols) – which are also situated on a 62 cd/m2 background – much better than others, e.g., the 5 and 119 cd/m2 background staircases (red and blue symbols, respectively). This systematic departure from precise correspondence prompted a secondary analysis.
Figure 7.
Mean matching luminance (collapsed across all observers) is plotted against mean ODOG model output for all 45 regions of interest in the stimuli of Experiments 1 and 2. Circular symbols plot data from staircase stimuli: red, green and blue refer to steps on the 5, 62, and 119 cd/m2 backgrounds, and bright and dark colors denote the sequential and disrupted staircase conditions, respectively. Square symbols plot data from simultaneous brightness/lightness contrast stimuli: black, gray and white refer to the 24, 60, and 100 cd/m2 test patches, respectively. In panel (a) the data are fit to a linear function possessing two free parameters (K=2): a y-intercept and a slope. The fitted y-intercept is 63.9 cd/m2, and the slope is 0.268 cd/m2 per unit model output. The correlation is highly significant, r(44)=0.93, p<.0001, and accounts for over 86% of the total variance. In panel (b) mean stimulus luminances are introduced as a priori y-intercepts, and the data were fit to a system of three linear functions with just a single free parameter (K=1): the slope. The value of the fitted slope parameter is 0.309 cd/m2 per unit model output. The correlation of matching luminance and ODOG model output is again highly significant, r(44)=0.98, p<.0001, and accounts for 97% of the total variance in matching luminance.
We remind the reader that the ODOG model responds exclusively to spatial contrast, although it does so over multiple spatial scales and differs in this respect from older single-channel models. Because ODOG filters are volume-balanced they cannot record or represent mean luminance, around which ODOG model output is centered, and for which its output is always zero. On previous occasions where ODOG model output has been compared with luminance matching data (Blakeslee & McCourt, 1999; 2001; 2004, 2005; Blakeslee, Pasieka & McCourt, 2005), the regions of interest (i.e., the test patches) always possessed identical luminance (while appearing different in brightness due to their spatial context), and mean stimulus luminance was held constant. Under these circumstances the relative difference in ODOG model output to physically identical test regions successfully predicted their relative brightness in the case of many brightness illusions. Here, however, the situation is more complex in that some regions of interest (i.e., stair steps or test patches) not only possess unequal luminance, but are themselves situated on variable luminance backgrounds which cause large shifts in mean luminance.
Fig. 7(b) shows the relationship between ODOG model output and psychophysical luminance matching after compensating for these differences in mean stimulus luminance to which the ODOG model is insensitive. Mean stimulus luminance was computed by averaging the luminance of each stimulus over a region corresponding to the size of the largest ODOG filter (a circular aperture approximately 21° visual angle in diameter). These luminances are 42.65, 62.0, and 81.25 cd/m2 for the staircase stimuli situated on the 5, 62, and 119 cd/m2 backgrounds, respectively. The mean luminance of the simultaneous brightness/lightness contrast stimuli is 62.0 cd/m2 (±0.05). These mean luminances were introduced as a priori y-intercepts, and the data were fit to a system of three linear functions with just a single free parameter (K=1): the slope. The value of the fitted slope parameter is 0.309 cd/m2 per unit model output. The correlation of matching luminance and ODOG model output is again highly significant, r(44)=0.98, p<.0001, and now accounts for 97% of the total variance in matching luminance.
The bar graphs of Figure 8 plot the group-averaged mean matching luminance for each step of the sequential (light-gray bars) and disrupted (dark-gray bars) staircases from Experiment 1. Group-averaged mean matching luminance to steps on the 5 cd/m2, 62 cd/m2, and 119 cd/m2 backgrounds is plotted separately in the top, middle and bottom panels, respectively. The error bars are 95% confidence intervals. Black symbols represent the predictions of the ODOG model with no adjustment for mean luminance (K=2) and white symbols plot predictions of the mean luminance-adjusted model (K=1). While the mean luminance-adjusted model is obviously superior in predicting the values of the luminance matches with changes in mean stimulus luminance, the output of either model captures the qualitative relationships, i.e., the direction of the brightness changes, between the sequential and disrupted staircases on each background luminance.
Despite the excellent qualitative agreement of model results with empirical findings, we note that some quantitative differences remain. For example, the ODOG model predicts that the largest brightness difference between sequential and disrupted staircase steps will occur for the 120 cd/m2 step on the 119 cd/m2 background, and that the smallest difference in brightness for this step will occur when it is situated on the 5 cd/m2 background. The psychophysical data clearly trend in the opposite direction. It is possible that such discrepancies are due to known nonlinearities such as luminance gain control (Hood, 1998; Mante et. al., 2005; Geisler, Albrecht & Crane, 2007) which are not included in the ODOG model.
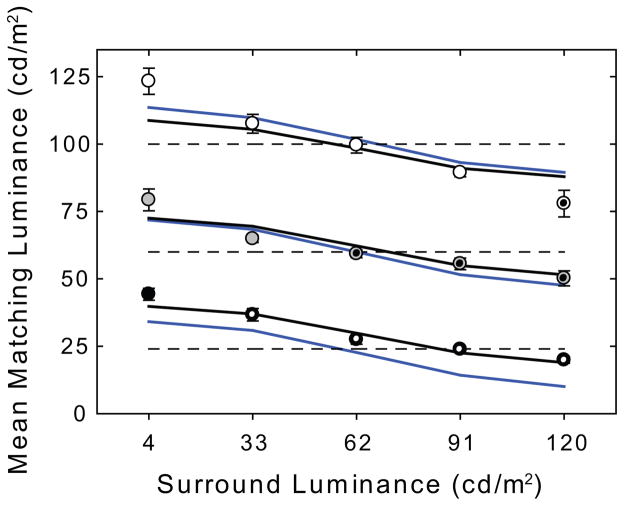
Fig. 9 plots the group averaged data and model output for the staircase simultaneous brightness/lightness contrast stimuli from Experiment 2. The group averaged mean matching luminances for the 24 cd/m2 (black symbols), 60 cd/m2 (gray symbols) and 100 cd/m2 (white symbols) test patches are plotted as a function of surround luminance. The dotted symbols denote test patches that are decrements relative to the surround luminance. The error bars are 95% confidence intervals. The black line plots the predictions of the K=2 ODOG model and the blue line plots the predictions of the K=1 mean luminance-adjusted model. Unlike the large disparity between the predictions of the two models for the staircase stimuli, the discrepancies between the predictions of the K=1 and K=2 models are small for the simultaneous brightness/lightness contrast stimuli because there is practically no difference in mean luminance between them. While the ODOG model does a good job of capturing the effect of surround luminance on mean matching luminance, the model underestimates the magnitude of induction on the 4 cd/m2 surround for all three test patch luminances, and also underestimates induction for the 100 cd/m2 test patch on the 120 cd/m2 surround. Again, we suggest that these quantitative discrepancies may result from nonlinearities of visual processing which have not yet been incorporated into the ODOG model. In this instance, the accelerating nonlinearity which characterizes the spike generation stage of visual cortical processing (Albrecht, Geisler, Frazor, and Crane, 2002; Albrecht, Geisler, & Crane, 2003) is a likely candidate. Interestingly, the (K=1) model overestimates induction for the 24 cd/m2 test patch on the 91 cd/m2 and 120 cd/m2 surround. This is consistent with our proposal, discussed in greater detail below, that asymmetric induction in this condition is attributable to the effect of scattered light.
Figure 9.
Group averaged data and model output for the staircase simultaneous brightness/lightness contrast stimuli from Experiment 2. The group averaged mean matching luminances for the 24 cd/m2 (black symbols), 60 cd/m2 (gray symbols) and 100 cd/m2 (white symbols) test patches are plotted as a function of surround luminance. The dotted symbols denote test patches that are decrements relative to the surround luminance. The error bars are 95% confidence intervals. The black line plots the predictions of the K=2 ODOG model and the blue line plots the predictions of the K=1 mean luminance-adjusted model.
This analysis naturally leads us to consider what modifications to the ODOG model might establish a unique correspondence between matching luminance and raw model output. Whereas ODOG model output is necessarily centered around mean stimulus luminance (as integrated within an approximately 21° diameter aperture) the visual system appears to possess some ability to encode absolute luminance. Although the classical receptive fields of simple cells generally fail to respond to homogeneous changes in mean luminance (Hubel & Wiesel, 1968), recent evidence in cat suggests that, when total spikes within a fixed integration interval are counted, changes in local mean luminance have a large effect on the scale of the transient contrast-response function without changing its shape (Geisler et. al., 2007) This scaling effect of local luminance on the contrast response of cortical neurons is similar to the scaling effects of orientation, spatial frequency, phase, and direction of motion (Geisler & Albrecht, 1997) and results in tuning functions for these parameters that are contrast invariant. In addition, transient changes in local mean luminance (unlike contrast changes) also have a large effect on the shape of the temporal response profiles of cortical simple and complex cells (Geisler et. al., 2007). Thus, local luminance information is contained in the responses of most neurons in primary visual cortex and may be coded in part due to fast luminance and contrast gain control mechanisms (Geisler et. al., 2007). Attempts to evaluate or propose such modifications to the ODOG model are clearly beyond the scope of the present paper; however, the modeling results in Figs. 7, 8 and 9 clearly illustrate the strong potential of physiologically-informed multiscale filtering explanations to account for a wide variety of brightness matching data.
GENERAL DISCUSSION
The comparison of the brightness matching data from the sequential and disrupted staircases indicates that the relative position of the various steps in the staircase significantly influences their brightness (Figs. 2 & 3). This result contradicts the predictions of the anchoring model (Gilchrist et al., 1999; Gilchrist, 2006), which posits that step position in the staircase should have no effect on brightness/lightness matching. The data are consistent, however, with models based on multiscale spatial filtering. This is demonstrated by the ability of the ODOG filtering model (Blakeslee & McCourt, 1999; 2001; 2004) to predict the overall pattern of the brightness matching data (Fig. 8). Note that the ODOG model predicts that the brightness/lightness of the dark step in the context of the disrupted staircase will be lower than in the sequential staircase condition. Interestingly, however, in the 5 cd/m2 background condition, the condition closest to that in the Cataliotti and Gilchrist (1995) study, only observer BB showed a significantly lower matching luminance for the darkest step in the disrupted staircase. Thus, our data for this step are largely in agreement with those of Cataliotti and Gilchrist (1995). In other words, by restricting their analysis to the black step, where the brightness/lightness effects are experimentally small, Cataliotti and Gilchrist (1995) may have simply missed the significant effects produced by staircase configuration that are more conspicuous for the other steps.
The results of Experiment 2 clearly indicate that there is a differential simultaneous brightness contrast effect for increments (Fig 5, solid white circles). Although this finding contradicts the predictions of the anchoring model (Gilchrist et al., 1999; Gilchrist 2006) it is clearly accounted for by the ODOG model (Fig 9). These results conflict with those of Economou et al. (2007) and several earlier studies (Gilchrist, 1988; Jacobsen & Gilchrist, 1988; Agostini & Bruno, 1996). They are, however, consistent with other results (Heinemann, 1955; Kozaki, 1963; Cornsweet, 1970; Shapley, 1986; Arend & Spehar, 1993b) including those of two recent studies which were specifically designed to address the question of whether there is a differential simultaneous brightness/lightness contrast effect for test patch increments on different surrounds (Bressan & Actis-Grosso, 2001; Rudd & Zemach, 2005). As suggested by Bressan and Actis-Grosso (2001), the failure of some studies or conditions to demonstrate a differential lightness effect for incremental test patches on variable surrounds may have been due partly to surround and/or test patch luminances that were not optimal for producing the effect. It also seems likely, however, as discussed by Rudd and Zemach (2005), that the failure to detect these induction effects may be due to the use of a 16-step Munsell matching scale (Gilchrist, 1988; Jacobsen & Gilchrist, 1988; Agostini & Bruno, 1996; Economou et al., 2007). This coarse matching scale may simply not be sensitive enough to reveal the differences observed in the studies employing finer scale luminance matching (Heinemann, 1955; Arend & Spehar, 1993b; Bressan & Actis-Grosso, 2001; Rudd & Zemach, 2005).
Economou et al. (2007) also claim that the majority of the simultaneous lightness contrast effect is due to the lightening of the target on the black surround. The data for the 24 cd/m2 test patch (black circles) clearly show this asymmetry. Relative to the match on the 62 cd/m2 surround, the brightening effect of the less luminous surround (4 cd/m2) is greater than the darkening effect of the more luminous surround (120 cd/m2). Thus, in the 24 cd/m2 test patch condition, one could conclude, consistent with anchoring theory, that the majority of the simultaneous brightness/lightness contrast effect is due to the brightening/lightening of the target on the dark/black surround. This does not, however, appear to be true for the 100 cd/m2 test patch (Fig. 5, white circles) for any of the observers with the possible exception of JH. Although this might be interpreted as contradicting the anchoring model, it has been suggested that the majority of the simultaneous brightness/lightness contrast effect may shift to the target on the white background as target luminance increases and that this shift can also be explained by anchoring theory (for details of this explanation see Gilchrist & Economou, 2003). Another plausible explanation for this particular pattern of results, however, is that intraocular light scatter from the higher luminance surrounds physically increases test patch luminance and reduces the magnitude of the contrast effect. Since the amount of scatter from each of the surrounds is a fixed percentage of the surround luminance, and is constant across test patch luminance, we would expect the greatest effect of scatter to occur at the lowest test patch luminances, where scattered light represents a larger proportion of the total luminance. If this is indeed the case it would also explain why the ODOG model, which is not affected by stray light, overestimates the size of the induction effect for the 24 cd/m2 test patch (Fig. 9, gray line) on the 91 cd/m2 and 120 cd/m2 surrounds.
Finally, Economou et al. (2007) reported that darker targets result in larger effects. Although this does not appear to be supported by the brightness matching data (compare the black, gray and white symbols on the 4 cd/m2 surround luminance in Fig. 5) it is important to note that after Economou et al. (2007) converted their CRT luminance values to reflectance values by assigning the “white” background a reflectance of 90%, and assigning lower values in proportion to relative luminance, they plotted their matching data in terms of log reflectance. When our matching data are similarly plotted on a log scale the magnitude of the effect also appears greater for the lower test patch luminances. This is because a constant difference in mean matching luminance from the veridical luminance of the test patch translates into a larger luminance ratio as test patch luminance decreases. Both types of plot describe the matching behavior accurately and it is, therefore, important to note the axes so that this transformation of the data is not confused with a property of the visual system. In a matching paradigm, as opposed to a direct measurement paradigm such as magnitude estimation or single-unit recording, any log transformation of the visual stimulus by the visual system is applied both to the stimulus and matching patch and would not be expected to influence the matching data.
CONCLUSIONS
The comparison of the brightness matching data from the sequential and disrupted staircases in Experiment 1 indicates that the relative position of the various steps in the staircase significantly influences their brightness. This result does not support the conclusions of Cataliotti and Gilchrist (1995) and contradicts the predictions of anchoring theory which posits that position in the staircase should not have an effect on brightness/lightness matching. The data are consistent, however, with a lateral-inhibition model of the multiscale filtering type. This is demonstrated by the ability of the ODOG model (Blakeslee & McCourt, 1999; 2001; 2004) to predict the overall pattern of the brightness matching data.
Brightness matching data for the staircase simultaneous brightness/lightness contrast stimuli in Experiment 2 indicate first that incremental test patches do not all appear equal, as predicted by anchoring theory (Gilchrist et al., 1999; Gilchrist, 2006) and reported by Economou et al. (2007); rather, matching luminance decreased significantly with increases in background luminance. Second, we found that although the simultaneous lightness contrast effect appeared symmetrical for all observers for the 100 cd/m2 test patch, the data for the 24 cd/m2 test patch, in agreement with Economou et al. (2007), clearly show an asymmetry relative to the matching luminance on the 62 cd/m2 background such that the brightening effect of the less luminous backgrounds was greater than the darkening effect of the more luminous backgrounds. Although it has been suggested that anchoring theory can explain this pattern of results (Gilchrist & Economou, 2003) we suggest an alternative explanation in terms of intraocular scattered light. Third, Economou et al. (2007) reported that darker targets result in larger simultaneous brightness contrast effects. Although at first glance this does not appear to be supported by the brightness matching data from this study, it is important to note that Economou et al. (2007) converted their CRT luminance values to log reflectance values. When our matching data are plotted on a log scale the magnitude of the effect also appears greater for the lower test patch luminances. Finally, although, Economou et al. (2007) argued that contrast models, and the ODOG model in particular (Blakeslee & McCourt, 1999; 2001; 2004), could not account for their data, we find that the ODOG model does an excellent job of qualitatively accounting for the brightness effects produced by the sequential and disrupted staircase and the staircase simultaneous brightness/lightness contrast stimuli.
Acknowledgments
This work was supported by grants from the National Science Foundation (IBN-0212789), the National Eye Institute (NEI) (R01 EY014015) and the National Center for Research Resources (NCRR) (P20 RR020151). NEI and NCRR are components of the National Institutes of Health (NIH). The contents of this report are solely the responsibility of the authors and do not necessarily reflect the official views of the NIH, NCRR or NSF. The authors thank Dan Gu for programming assistance and the reviewers for providing helpful feedback on earlier versions of the manuscript. Commercial relationships: none.
Footnotes
Although some authors use the term “simultaneous lightness contrast”, and describe the stimulus and effect in terms of perceived reflectance (e.g., white, black) others prefer the term “simultaneous brightness contrast” and describe the stimulus and effect in terms of perceived luminance i.e., brightness (e.g., dark, bright). Under homogeneous illumination, lightness and brightness matches produce equivalent results (Arend & Spehar, 1993a, b; Blakeslee, Reetz & McCourt, 2008) and these descriptions are functionally equivalent. Illumination was homogeneous in all of the studies discussed in this paper and although we will use the terms preferred by the authors when we are reporting the results of specific studies, these results are comparable. In order to emphasize this fact the results from the present study are discussed using the combined term “brightness/lightness”.
References
- Agostini T, Bruno N. Lightness contrast in CRT and paper-and-illminant displays. Perception and Psychophysics. 1996;58:250–258. doi: 10.3758/bf03211878. [DOI] [PubMed] [Google Scholar]
- Albrecht DG, Geisler WS, Frazor RA, Crane AM. Visual cortex neurons of monkeys and cats: temporal dynamics of the contrast response function. Journal of Neurophysiology. 2002;88:888–913. doi: 10.1152/jn.2002.88.2.888. [DOI] [PubMed] [Google Scholar]
- Albrecht DG, Geisler WS, Crane AM. Nonlinear properties of visual cortex neurons: temporal dynamics, stimulus selectivity, neural performance. In: Chalupa AWJ, editor. The Visual Neurosciences. Boston: MIT; 2003. pp. 747–764. [Google Scholar]
- Arend LE, Spehar B. Lightness, brightness, and brightness contrast: 1. Illuminance variation. Perception and Psychophysics. 1993a;54(4):446–456. doi: 10.3758/bf03211767. [DOI] [PubMed] [Google Scholar]
- Arend LE, Spehar B. Lightness, brightness, and brightness contrast: 2. Reflectance variation. Perception and Psychophysics. 1993b;54(4):457–468. doi: 10.3758/bf03211768. [DOI] [PubMed] [Google Scholar]
- Blakeslee B, McCourt ME. A multiscale spatial filtering account of the White effect, simultaneous brightness contrast and grating induction. Vision Research. 1999;39:4361–4377. doi: 10.1016/s0042-6989(99)00119-4. [DOI] [PubMed] [Google Scholar]
- Blakeslee B, McCourt ME. A multiscale spatial filtering account of the Wertheimer-Benary effect and the corrugated Mondrian. Vision Research. 2001;41:2487–2502. doi: 10.1016/s0042-6989(01)00138-9. [DOI] [PubMed] [Google Scholar]
- Blakeslee B, McCourt ME. A unified theory of brightness contrast and assimilation incorporating oriented multiscale spatial filtering and contrast normalization. Vision Research. 2004;44:2483–2503. doi: 10.1016/j.visres.2004.05.015. [DOI] [PubMed] [Google Scholar]
- Blakeslee B, McCourt ME. A multiscale filtering explanation of gradient induction and remote brightness induction effects: a reply to Logvinenko (2003) Perception. 2005;34:793–802. doi: 10.1068/p5303x. [DOI] [PubMed] [Google Scholar]
- Blakeslee B, Pasieka W, McCourt ME. Oriented multiscale spatial filtering and contrast normalization: a parsimonious model of brightness induction in a continuum of stimuli including White, Howe and simultaneous brightness contrast. Vision Research. 2005;45:607–615. doi: 10.1016/j.visres.2004.09.027. [DOI] [PubMed] [Google Scholar]
- Blakeslee B, Reetz D, McCourt ME. Coming to terms with lightness and brightness: effects of stimulus configuration and instructions on brightness and lightness judgments. Journal of Vision. 2008;8:1–14. doi: 10.1167/8.11.3. http://journalofvision.org/8/11/3/ [DOI] [PMC free article] [PubMed]
- Bressan P, Actis-Grosso R. Simultaneous lightness contrast with double increments. Perception. 2001;30:889–897. doi: 10.1068/p3103. [DOI] [PubMed] [Google Scholar]
- Cataliotti J, Gilchrist A. Local and global processes in surface lightness perception. Perception and Psychophysics. 1995;57(2):125–135. doi: 10.3758/bf03206499. [DOI] [PubMed] [Google Scholar]
- Cornsweet TN. Visual Perception. New York: Academic Press; 1970. [Google Scholar]
- Diamond AL. Foveal simultaneous brightness contrast as a function of inducing-and test-field luminances. Journal of Experimental Psychology. 1953;45:304–314. doi: 10.1037/h0060230. [DOI] [PubMed] [Google Scholar]
- Economou E, Zdravkovic S, Gilchrist A. Anchoring versus spatial filtering accounts of simultaneous lightness contrast. Journal of Vision. 2007;7:1–15. doi: 10.1167/7.12.2. http://journalofvision.org/7/12/2/ [DOI] [PubMed]
- Fiorentini A, Baumgartmer G, Magnussen S, Schiller PH, Thomas JP. The perception of brightness and darkness: Relations to neuronal receptive fields. In: Spillman LW, JS, editors. Visual Perception the Neurophysiological Foundations. San Diego: Academic Press; 1977. pp. 129–159. [Google Scholar]
- Georgeson MA, Sullivan GD. Contrast constancy: deblurring in human vision by spatial frequency channels. Journal of Physiology. 1975;252:627–656. doi: 10.1113/jphysiol.1975.sp011162. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Geisler WS, Albrecht DG. Visual cortex neurons in monkeys and cats: detection, discrimination, and identification. Visual Neuroscience. 1997;14:897–919. doi: 10.1017/s0952523800011627. [DOI] [PubMed] [Google Scholar]
- Geisler WS, Albrecht DG, Crane AM. Responses of neurons in primary visual cortex to transient changes in local contrast and luminance. Journal of Neuroscience. 2007;27:5063–5067. doi: 10.1523/JNEUROSCI.0835-07.2007. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gilchrist A, Kossyfidis C, Bonato F, Agostini T, Cataliotti J, Li X, Spehar B, Annan V, Economou E. An anchoring theory of lightness perception. Psychological Review. 1999;106:795–834. doi: 10.1037/0033-295x.106.4.795. [DOI] [PubMed] [Google Scholar]
- Gilchrist AL. Lightness contrast and failures of constancy: a common explanation. Perception and Psychophysics. 1988;43:415–424. doi: 10.3758/bf03207877. [DOI] [PubMed] [Google Scholar]
- Gilchrist A. Seeing Black and White. New York: Oxford University Press; 2006. [Google Scholar]
- Grossberg S, Todorovic D. Neural dynamics of 1-D and 2-D brightness perception: A unified model of classical and recent phenomena. Perception & Psychophysics. 1988;43:241–277. doi: 10.3758/bf03207869. [DOI] [PubMed] [Google Scholar]
- Heinemann EG. Simultaneous brightness induction as a function of inducing and test-field luminances. J Exp Psychol. 1955;50(2):89–96. doi: 10.1037/h0040919. [DOI] [PubMed] [Google Scholar]
- Heinemann EG. Simultaneous brightness induction. In: Jameson DH, LM, editors. Handbook of Sensory Physiology. VII/4. Berlin: Springer; 1972. pp. 147–169. [Google Scholar]
- Heinemann EG, Chase S. A quantitative model for simultaneous brightness induction. Vision Research. 1995;35:2007–2020. doi: 10.1016/0042-6989(94)00281-p. [DOI] [PubMed] [Google Scholar]
- Hood DC. Lower-level visual processing and models of light adaptation. Annual Review of Psychology. 1998;49:503–535. doi: 10.1146/annurev.psych.49.1.503. [DOI] [PubMed] [Google Scholar]
- Jacobsen A, Gilchrist A. Hess and Pretori revisited: resolution of some old contradictions. Perception & Psychophysics. 1988;43:7–14. doi: 10.3758/bf03208967. [DOI] [PubMed] [Google Scholar]
- Jameson D, Hurvich LM. Theory of brightness and color contrast in human vision. Vision Research. 1964;4:135–154. doi: 10.1016/0042-6989(64)90037-9. [DOI] [PubMed] [Google Scholar]
- Kingdom F, Moulden B. A multi-channel approach to brightness coding. Vision Research. 1992;32:1565–1582. doi: 10.1016/0042-6989(92)90212-2. [DOI] [PubMed] [Google Scholar]
- Kozaki A. A further study in the relationship between brightness constancy and contrast. Japanese Psychological Research. 1963;5:129–136. [Google Scholar]
- Kozaki A. The effect of co-existent stimuli other than the test stimulus on brightness constancy. Japanese Psychological Research. 1965;7:138–147. [Google Scholar]
- Mante V, Frazor RA, Bonin V, Geisler WS, Carandini M. Independence of luminance and contrast in natural scenes and in the early visual system. Nature Neuroscience. 2005;8:1690–1697. doi: 10.1038/nn1556. [DOI] [PubMed] [Google Scholar]
- McArthur JA, Moulden B. A two-dimensional model of brightness perception based on spatial filtering consistent with retinal processing. Vision Research. 1999;39:1199–1219. doi: 10.1016/s0042-6989(98)00216-8. [DOI] [PubMed] [Google Scholar]
- Morrone MC, Burr DC. Feature detection in human vision: A phase-dependent energy model. Proceedings of the Royal Society of London B: Biological Sciences. 1988;235:221–245. doi: 10.1098/rspb.1988.0073. [DOI] [PubMed] [Google Scholar]
- Pessoa L, Mingolla E, Neumann H. A contrast- and luminance-driven multiscale network model of brightness perception. Vision Research. 1995;35:2201–2223. doi: 10.1016/0042-6989(94)00313-0. [DOI] [PubMed] [Google Scholar]
- Reeves A. Visual adaptation. In: Chalupa AW, editor. The visual neurosciences. Boston: MIT; 2004. pp. 851–862. [Google Scholar]
- Rudd ME, Zemach IK. The highest luminance anchoring rule in achromatic color perception: some counterexamples and an alternative theory. Journal of Vision. 2005;5:983–1003. doi: 10.1167/5.11.5. http://journalofvision.org/5/11/5/ [DOI] [PubMed]
- Shapley R. The importance of contrast for the activity of single neurons, the VEP and perception. Vision Research. 1986;26:45–61. doi: 10.1016/0042-6989(86)90070-2. [DOI] [PubMed] [Google Scholar]
- Watt RJ, Morgan MJ. A theory of the primitive spatial code in human vision. Vision Research. 1985;11:1661–1674. doi: 10.1016/0042-6989(85)90138-5. [DOI] [PubMed] [Google Scholar]
- Whittle P. Increments and decrements: Luminance discrimination. Vision Research. 1986;26:1677–1691. doi: 10.1016/0042-6989(86)90055-6. [DOI] [PubMed] [Google Scholar]
- Whittle P. Brightness, discriminability and the “Crispening Effect”. Vision Research. 1992;32:1493–1507. doi: 10.1016/0042-6989(92)90205-w. [DOI] [PubMed] [Google Scholar]