The Solution Structure of Heparan Sulfate Differs from That of Heparin

Abstract

The highly sulfated polysaccharides heparin and heparan sulfate (HS) play key roles in the regulation of physiological and pathophysiological processes. Despite its importance, no molecular structures of free HS have been reported up to now. By combining analytical ultracentrifugation, small angle x-ray scattering, and constrained scattering modeling recently used for heparin, we have analyzed the solution structures for eight purified HS fragments degree of polymerization 6–18 (dp6–dp18) and dp24, corresponding to the predominantly unsulfated GlcA-GlcNAc domains of heparan sulfate. Unlike heparin, the sedimentation coefficient s_20,w of HS dp6–dp24 showed a small rotor speed dependence, where similar s_20,w values of 0.82–1.26 S (absorbance optics) and 1.05–1.34 S (interference optics) were determined. The corresponding x-ray scattering measurements of HS dp6–dp24 gave radius of gyration (R_G) values from 1.03 to 2.82 nm, cross-sectional radius of gyration (R_XS) values from 0.31 to 0.65 nm, and maximum lengths (L) from 3.0 to 10.0 nm. These data showed that HS has a longer and more bent structure than heparin. Constrained scattering modeling starting from 5000–8000 conformationally randomized HS structures gave best fit dp6–dp16 molecular structures that were longer and more bent than their equivalents in heparin. No fits were obtained for HS dp18 or dp24, indicating their higher flexibility. We conclude that HS displays an extended bent conformation that is significantly distinct from that for heparin. The difference is attributed to the different predominant monosaccharide sequence and reduced sulfation of HS, indicating that HS may interact differently with proteins compared with heparin.

Introduction

Heparan sulfate (HS)² is a sulfated glycosaminoglycan that is found extensively on animal cell surfaces and other extracellular surfaces (1, 2). HS has key roles in biological recognition processes at the cell-tissue-organ interface through its interactions with a wide range of proteins (3, 4). Specific interactions involving HS include roles in cell growth and development (5), cell adhesion (6), inflammation and wound healing (7), angiogenesis and cancer (8–10), viral invasion (11, 12), and anticoagulation (13). The breadth of these HS-protein interactions offers potential strategies for therapeutic intervention at the cell-tissue-organ interface.

HS is a sulfated polysaccharide composed of uronic acid and d-glucosamine residue pairs linked by 1→4 glycosidic bonds (Fig. 1) (14, 15). The uronic acid residue is either unmodified β-d-glucuronic acid (GlcA), alternating with N-acetylated glucosamine (Fig. 1A), or α-l-iduronic acid (IdoA), often 2-O-sulfated, alternating with N-sulfated glucosamine (GlcNS) (Fig. 1B). In the latter, sulfation often occurs at C6 and rarely also at C3 (16, 17). HS has a distinct domain organization that is composed of short S domains (IdoA2S and GlcNS residues), long NA domains with GlcA and GlcNAc residues, and mixed domain regions at the junctions between the S domains and NA domains (15, 16). The S domains and mixed domain regions are termed the hypervariable regions that result in different functional characteristics for HS from different cell types (16).

FIGURE 1.

Chemical structures of the two disaccharide repeats of HS and heparin. A, the major repeating disaccharide unit of HS (glucuronic acid → N-acetylglucosamine). The NH·CO·CH₃ group in the second ring is replaced by NH·SO₃⁻ in 50% of this structure. The resulting molecular mass of this averaged disaccharide is 483 Da. B, the minor repeating unit of HS, this being the major repeating disaccharide unit in 90% of heparin (iduronic acid 2-sulfate → glucosamine 2,6-disulfate). For comparison with this study, heparin is considered to be 50% in the trisulfate form as shown and 50% in a disulfate form where a sulfate group is lost. The resulting molecular mass of this averaged disaccharide is 628 Da.

Three-dimensional structural studies of HS are required in order to complete an understanding of the physiological significance of HS-protein interactions. Many structural studies have been carried out for heparin, which is an analog for HS but possesses a higher degree of sulfation, being predominantly S region-like in sequence, and for at least 19 heparin-protein co-crystal complexes. This abundance results from the ease with which heparin is obtained and its strong binding to many of the cell surface proteins whose physiological ligand is HS. An NMR structure is known for heparin (18). Solution structures are known for six heparin dp6–dp36 forms from constrained scattering modeling; these were shown to be similar in conformation to heparin when observed in heparin-protein crystal structures (19). In distinction, up to now, no molecular structures for free HS are known, and only one crystal structure at 0.21 nm resolution for a dp4 HS oligosaccharide complexed with heparinase II is available (20).

Given the importance of understanding the HS solution structure, we have used a multidisciplinary approach to determine molecular structures for HS based on the combination of three methods, namely analytical ultracentrifugation, small angle x-ray scattering, and constrained scattering modeling (21, 22). This approach is well established for solution structure determinations of large multidomain complement and antibody proteins and was recently applied to small heparin oligosaccharide fragments (19, 23). Here, we apply this approach for the second time for oligosaccharide solution structures, this time for eight HS fragments ranging in sizes from dp6 to dp24, thus permitting detailed comparisons with heparin. The HS fragments exhibited solution structures that were distinct from those of the heparin fragments. In particular, their overall lengths are longer compared with heparin, and their structures display a greater degree of bending with an increase in size compared with heparin. Our results are attributed to the difference in monosaccharide sequence between HS and heparin fragments, combined with a much reduced degree of sulfation in the HS fragments, which possessed greater structural flexibility than heparin. These results provided new insights into the potential binding modes of HS to proteins.

EXPERIMENTAL PROCEDURES

Purification of HS Fragments

HS oligosaccharide fragments were prepared according to a method similar to that previously used for heparin oligosaccharides (19, 24–26). Exhaustive heparinase digestion was used to minimize the content of fully sulfated sequences. About 100 mg of HS (prepared from a crude glycosaminoglycan mixture, the kind gift of Laboratori Derivati Organici, Italy; a mixture of HS-I and HS-II as described in Ref. 27) was weighed out and dissolved in about 2 ml of phosphate buffer, pH 7. An aliquot of 200 μl of heparinase I stock solution was added and left to digest at room temperature for at least 2 h, long enough for the reaction to run to completion. The reaction mixture was evaporated to dryness, using a rotary evaporator at 50 °C.

To isolate the HS fragments, the dried digest was dissolved in 1.5 ml of 2% ammonium bicarbonate solution and filtered through a 0.45-μm syringe filter before injection onto the preparative gel filtration column. The filtered digested HS was then applied to a preparative gel permeation chromatography column (100 × 1.6 cm; packed with Biogel P10) (Bio-Rad). The HS fragments were eluted using 2% ammonium bicarbonate at a flow rate of 0.2 ml/min in 2-ml fractions. The absorbance of the fractions was measured at 234 nm, and the top fractions corresponding to each individual resolved peak were pooled. The HS oligosaccharides larger than dp12 were not completely resolved (Fig. 2). The pooled fractions were evaporated under reduced pressure and lyophilized before assessment of their sizes by analytical gel permeation chromatography (25). Like previous heparin chromatography, gel permeation chromatography was carried out using two columns (TSK G3000 SW-XL (30 cm) and TSK G2000 SW-XL (30 cm), Anachem) connected in series. The eluant was 0.1 m ammonium acetate solution at a flow rate of 0.5 ml/min, and HS was detected with a refractive index detector (RI-1530, Jasco). The chromatography system was calibrated using the First International Reference Reagent Low Molecular Weight Heparin for Molecular Weight Calibration (National Institute for Biological Standards and Control 90/686). HS quantification was achieved by integration of the area under each refractive index peak and comparison with a standard curve prepared using known concentrations of low molecular weight heparin. An absorption coefficient of 5500 m⁻¹ cm⁻¹ at 232 nm was used for HS experiments (28).

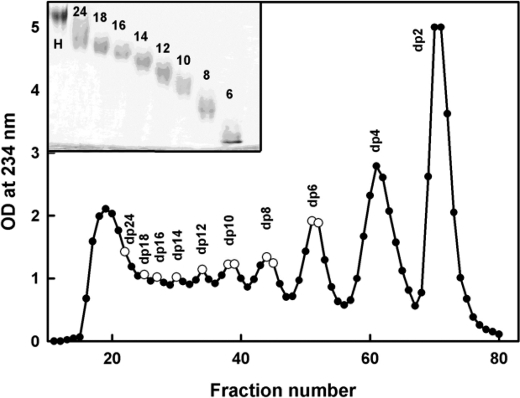
FIGURE 2.

Purification profile of the HS fragments. The HS fragments were eluted with a flow rate of 0.2 ml/min using a Biogel P-10 column in 2% ammonium bicarbonate solution. Fractions of 2 ml/10 min were collected, and their HS concentrations were measured spectrophotometrically at 234 nm. The fractions taken for this study are shown by open circles. The inset shows 25% PAGE of the HS fragments dp6–dp24 (labeled 6–24) with heparin dp24 (labeled H) as marker.

PAGE of HS Fragments

The HS fragments were analyzed by PAGE to determine the level of purity of each one according to a previously described method (29). Each HS fragment (5 μg) was mixed with 20% glycerol up to a maximum volume of 10–15 μl and then loaded into separate wells (Fig. 2, inset). Phenol red in 20% glycerol in a maximum volume of 10 μl was also applied to a separate well as a marker. Initially, samples were run through a stacking gel (5% acrylamide, 0.5% bisacrylamide) at 150 V for 20–30 min until the phenol red started to enter the resolving gel. In the resolving gel (25% acrylamide, 1% bisacrylamide), samples were run at a constant current of 18 mA until the phenol red reached the bottom of the gel. The discontinuous buffer system of Laemmli (30) consisted of 0.125 m Tris/HCl, pH 6.8, in the stacking gel and 0.375 m Tris/HCl, pH 8.8, in the resolving gel. The gel running buffer was 25 mm Tris, 0.192 m glycine, pH 8.3. The gel was stained with 0.08% aqueous Azure A for 10 min to visualize HS bands. The gel was then destained in water to remove excess dye and clear the gel background.

Analytical Ultracentrifugation of HS Fragments

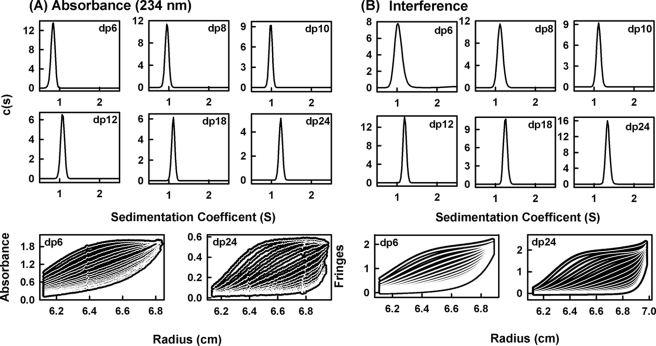
Sedimentation velocity data for eight HS fragments (dp6, dp8, dp10, dp12, dp14, dp16, dp18, and dp24) were obtained on two Beckman XL-I analytical ultracentrifuges (Beckman-Coulter Inc., Palo Alto, CA) using both absorbance and interference optics. Experiments with the dp6–dp24 fragments were performed at concentrations of 0.5 mg/ml in 10 mm HEPES and 137 mm NaCl (pH 7.4). The buffer density was measured at 20 °C using an Anton-Paar DMA5000 density meter to be 1.00480 g/ml. A partial specific volume of 0.467 ml/g determined for heparin (31) was used for HS. An alternative higher value of 0.55 ml/g for HS has been reported elsewhere and was used for data processing only when required to confirm that the partial specific volume has no effect on the outcome of this study (32). Analytical ultracentrifugation runs were carried out in an eight-hole AnTi50 rotor with standard double-sector cells with column heights of 12 mm at 20 °C using absorbance optics at 234 nm and interference optics. Sedimentation velocity data were collected at 40,000, 50,000, and 60,000 rpm using absorbance optics and interference optics. The continuous c(s) analysis method was used to determine the sedimentation coefficients s_20,w of the eight HS fragments using SEDFIT software (version 9.4) (33, 34). The c(s) analysis directly fits the experimental sedimentation boundaries using the Lamm equation, the algorithm for which assumes that all species have the same frictional ratio f/f_o in each fit. The final SEDFIT analyses used a fixed resolution of 200, and optimized the c(s) fit by floating the meniscus and cell bottom when required, and holding the f/f_o value, base line, and cell bottom fixed until the overall root mean square deviations and visual appearance of the fits were satisfactory (Fig. 3). The individual f/f_o values calculated previously for the heparin fragments were used for the equivalent HS fragments (19).

FIGURE 3.

Sedimentation velocity size distribution analyses c(s) of six HS dp6–dp24 fragments. The absorbance and interference boundary scans were fitted using SEDFIT software for the HS fragments, each at 0.5 mg/ml. The mean s_20,w values and their S.D. values are reported in Table 1. A, the absorbance data using a wavelength of 234 nm and a rotor speed of 50,000 rpm gave s_20,w peaks at 0.84 S for dp6, 0.95 S for dp8, 0.98 S for dp10, 1.08 S for dp12, 1.11 S for dp18, and 1.23 S for dp24. Below these panels, representative boundary fits are shown for dp6 and dp24, in which only every sixth scan of the 120 fitted boundaries is shown for clarity. B, the interference data using a rotor speed of 50,000 rpm gave s_20,w peaks at 1.04 S for dp6, 1.12 S for dp8, 1.11 S for dp10, 1.19 S for dp12, 1.25 S for dp18, and 1.34 S for dp24. Below these panels, representative boundary fits are shown for every sixth scan of the 120 fitted boundaries for dp6 and dp24.

Synchrotron X-ray Scattering of HS Fragments

X-ray solution scattering of the above eight HS fragments dp6–dp24 were performed on beamline ID02 at the European Synchrotron Radiation Facility in Grenoble, France in two sessions with a ring energy of 6.0 GeV (35). In the first session, data were collected for six HS fragments in 16-bunch mode using beam currents from 63 to 89 mA. In the second session, data were collected for all eight HS fragments in 16-bunch mode using beam currents from 65 to 78 mA. Data were acquired using an improved fiber optically coupled high sensitivity and dynamic range CCD detector (FReLoN) with a smaller beam stop. The sample-to-detector distance was 3.0 meters. Experiments used the same HS concentrations of 0.5 mg/ml and buffers used in the sedimentation velocity experiments. For each HS fragment, samples were measured in a flow cell that moved the sample continuously during beam exposure in 10 time frames with different exposure times of 0.1, 0.25, 0.5, and 1 s to check for the absence of radiation damage effects. This exposure was optimized using on-line checks for the absence of radiation damage to show that this was not detectable.

Guinier analyses give the radius of gyration, R_G, which measures the degree of structural elongation in solution if the internal inhomogeneity of scattering within the macromolecules has no effect. Guinier plots at low Q values (where Q = 4 π sin θ/λ; 2θ is the scattering angle, and λ is the wavelength) gives the R_G and the forward scattering at zero angle I(0) (36).

This expression is valid in a Q·R_G range up to 1.5. If the structure is elongated (i.e. rod-shaped), the radius of gyration of the cross-sectional structure R_XS and the mean cross-sectional intensity at zero angle (I(Q)·Q)_Q→0 parameters are obtained from fits in a higher Q range.

The R_G and R_XS analyses were performed using an interactive PERL script program SCTPL7 (J. T. Eaton and S. J. Perkins, unpublished software) on Silicon Graphics OCTANE Workstations. Indirect Fourier transformation of the full scattering curve I(Q) in reciprocal space gives the distance distribution function P(r) in real space. This yields the maximum dimension of the macromolecule L and its most commonly occurring distance vector M in real space.

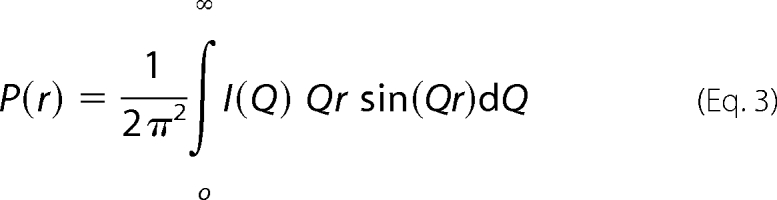The transformation was carried out using GNOM software (37). For dp6–dp16, the full x-ray I(Q) curves contained 295–343 data points in similar Q ranges between 0.29 and 1.80 nm⁻¹.

Molecular Modeling of HS Fragments

Linear HS models were created using the crystal structure of the HS tetrasaccharide (dp4) observed in its complex with heparinase II (Protein Data Bank code 3E7J) and using Discovery Studio (version 2.5) molecular graphics software (Accelrys, San Diego, CA). The residues in HS dp4 were N-acetyl-d-glucosamine (NAG), d-glucuronic acid (GCU), and 4,5-dehydro-d-glucuronic acid (GCD) in the order NAG-GCU-NAG-GCD (Protein Data Bank three-letter nomenclature). The NAG-GCU disaccharide was taken to be the base structure, and these disaccharide units (NAG-GCU) were joined by glycosidic linkages to generate a fully extended linear HS dp30 structure. In this, the Φ and Ψ angles were maintained at similar values observed in the starting dp4 structure. Linear HS dp6-dp24 models were created from this extended dp30 starting model by the removal of non-required disaccharides.

Totals of 5000 conformationally randomized models each for dp6, dp8, dp10, and dp12 and 8000 similar models each for dp14 and dp16 were created starting from each linear model. In the original HS dp4 structure, the Φ and Ψ angles were −90 and 124°, respectively, for the GlcA-GlcNAc (GCU-NAG) disaccharide and 85 and 95°, respectively, for the GlcNAc-GlcA (NAG-GCU) disaccharide. These Φ and Ψ angles were randomized to take any value in a maximum range of ±45° from their starting values using the TorsionKick function in a PERL script that was modified from the ExtractAngle.pl script provided with the Discovery Studio software. For example, in the case of dp16, a total of eight Φ and Ψ angles for GlcA-GlcNAc and seven Φ and Ψ angles for GlcNAc-GlcA were randomized in this way. To avoid steric clashes between the dp16 atoms in each randomized structure, a constant force field termed DREIDING minimization provided in Discovery Studio was used to correct this. DREIDING minimization was useful in generating structures by providing accurate geometries and a reasonably accurate steric barrier for organic, biological, and inorganic main groups (38).

Constrained Scattering and Sedimentation Coefficient Modeling

Each HS model was used to calculate the x-ray scattering curve for comparison with the experimental curve using Debye sphere models (39–41). A cube side length of 0.520 nm in combination with a cut-off of 4 atoms was used to create the spheres for the HS dp6–dp16 models. The hydration shell corresponding to 0.3 g/g H₂O was created by adding spheres to the unhydrated sphere models using HYPRO (42), where the optimal total of hydrated spheres is listed in Table 1. The x-ray scattering curve I(Q) was calculated using the Debye equation as adapted to spheres and assuming a uniform scattering density for the spheres (43). Other details are given elsewhere (39–41, 44). X-ray curves were calculated without instrumental corrections because these were considered to be negligible for the pinhole optics used in synchrotron x-ray instruments. First, the number of spheres N in the dry and hydrated models after grid transformation was used to assess steric overlap between the HS disaccharides, where models showing less than 95% of the optimal totals (Table 1) were discarded. This procedure was found to be insensitive to steric overlap in the case of oligosaccharides and was discontinued in favor of the DREIDING minimization procedure (above). Next, the models were assessed by calculation of the x-ray R_G values from Guinier fits of the modeled curves using the same Q ranges used for the experimental Guinier fits in order to allow for any approximations inherent in the use of the Q·R_G range up to 1.5. Models that passed the N and R_G filters were then ranked using a goodness of fit R factor in order to identify the best fit eight models for each HS fragment.

TABLE 1.

X-ray scattering and sedimentation coefficient modeling fits for eight HS fragments

HS fragment	Filter	Number of models	Hydrated spheresa	RGb	RXS	R factor	Length L	s20wc
				nm	nm	%	nm	S
dp6	None	5000	9–23	0.82–1.19	0.04–0.43	4.4–7.8	NAd	NA
	RG, RXS, R factor	8	15–20	1.01–1.03	0.30–0.31	4.4–4.5	3.0–3.5	0.52–0.79
	Best fit	1	19	1.01	0.30	4.4	3.0	0.52
	Experimental			1.03 ± 0.08	0.31 ± 0.06		3.0	0.82 ± 0.05
				0.98 ± 0.05				1.05 ± 0.04
dp8	None	5000	12–30	1.04–1.44	0.16–0.52	4.4–8.3	NA	NA
	RG, RXS, R factor	8	20–23	1.17–1.18	0.39–0.40	4.4	3.7–3.8	0.45–0.80
	Best fit	1	20	1.18	0.39	4.4	3.7	0.77
	Experimental			1.19 ± 0.08	0.40 ± 0.03		3.5	0.94 ± 0.06
				1.16 ± 0.02				1.06 ± 0.08
dp10	None	5000	15–37	1.17–1.63	0.24–0.63	4.3–8.0	NA	NA
	RG, RXS, R factor	8	25–30	1.40–1.43	0.42–0.47	4.3–4.4	4.7–4.8	0.48–095
	Best fit	1	26	1.42	0.42	4.3	4.8	0.91
	Experimental			1.41 ± 0.07	0.44 ± 0.04		4.5	0.95 ± 0.09
				1.37 ± 0.04				1.09 ± 0.06
dp12	None	5000	16–43	1.33–184	0.23–0.72	4.1–9.9	NA	NA
	RG, RXS, R factor	8	30–37	1.64–1.65	0.49–0.50	4.2	5.3–5.8	0.47–0.98
	Best fit	1	34	1.64	0.49	4.2	5.5	0.92
	Experimental			1.65 ± 0.09	0.49 ± 0.04		5.5	1.08 ± 0.09
				1.62 ± 0.03				1.16 ± 0.05
dp14	None	8000	24–50	1.52–1.94	0.18–0.76	4.2–9.0	NA	NA
	RG, RXS, R factor	8	35–40	1.75–1.78	0.48–0.51	4.4	6.0–6.5	0.74–1.05
	Best fit	1	37	1.75	0.49	4.4	6.2	0.96
	Experimental			1.76 ± 0.07	0.51 ± 0.02		6.0	1.07 ± 0.07
				1.82 ± 0.09				1.18 ± 0.04
dp16	None	8000	28–54	1.57–235	0.36–0.91	6.3–19.4	NA	NA
	RG, RXS, R factor	8	40–50	1.98–2.02	0.49–0.52	6.3–6.4	6.5–7.2	0.61–1.1
	Best fit	1	43	1.96	0.52	6.3	6.8	1.10
	Experimental			2.03 ± 0.07	0.52 ± 0.01		7.0	1.10 ± 0.03
				2.11 ± 0.11				1.24 ± 0.08
dp18	Experimental			2.34 ± 0.03	0.61 ± 0.05		8.5	1.12 ± 0.06
				2.44 ± 0.11				1.25 ± 0.07
dp24	Experimental			2.82 ± 0.10	0.65 ± 0.05		10.0	1.26 ± 0.06
				3.0 ± 0.05				1.34 ± 0.06

^a The optimal totals of hydrated spheres were 16 for dp6, 21 for dp8, 26 for dp10, 31 for dp12, 37 for dp14, and 42 for dp16.

^b The first experimental value is from the Guinier R_G analyses, and the second one is from the GNOM P(r) analyses.

^c The averaged experimental s_20,w value is reported, the first value being from the absorbance (234 nm) data sets and the second value from the interference data sets. The absorbance and interference data were recorded at rotor speeds of 40,000, 50,000, and 60,000 rpm.

^d NA, not applicable.

Sedimentation coefficients s⁰_20,w for each of the eight best fit HS scattering models were calculated directly from molecular structures using the HYDROPRO shell modeling program (45). The default value of 0.31 nm for the atomic element radius for all atoms was used to represent the hydration shell surrounding HS.

The best fit dp6–dp16 models are currently available in supplemental material.

RESULTS

Sedimentation Velocity Data Analysis for Eight HS Fragments

The purification profile from the Biogel P-10 column shows that the four smallest HS fragments, dp6–dp12, were eluted as well resolved peaks, whereas the four larger fragments, dp14–dp18 and dp24, were less well resolved (Fig. 2). Analytical high performance size exclusion chromatography of the HS oligosaccharide fractions as described (25) and PAGE were performed to show that all eight peak fractions showed altered sizes as expected and were relatively homogenous in size. Proton NMR spectroscopy (not shown) showed that the GlcA-GlcNAc disaccharide was the predominant structure present with a minor content of sulfated saccharide residues, presumably originating from transition sequences between the NA and S domains. Signals typical of heparin-like S domains were almost completely absent.

Analytical ultracentrifugation studies macromolecular structures and sizes through quantitative measurements of sedimentation rates in a high centrifugal field (46). Sedimentation velocity experiments at three rotor speeds were performed for the eight HS fragments (dp6–dp18 and dp24) to determine their shapes and degree of polydispersity. The sedimentation coefficient distribution function c(s) was calculated by direct fitting of the sedimentation boundaries using SEDFIT software. The absorbance optics analyses for each HS fragment reproducibly resulted in good boundary fits that resulted in single major peaks (Fig. 3A). The mean sedimentation coefficient s_20,w values at three speeds ranged from 0.82 ± 0.05 S for dp6 to 1.26 ± 0.08 S for dp24. The corresponding interference optics analyses for dp6–dp24 also resulted in good boundary fits and single major c(s) peaks with mean s_20,w values that ranged from 1.05 ± 0.04 S for dp6 to 1.35 ± 0.04 S for dp24 (Fig. 3B). The comparisons in Fig. 4, A and B, indicate that the difference between the absorbance and interference s_20,w values results from statistical variability, and there is no bias between the two data sets. The number of peaks and their widths assess the polydispersity of each HS fragment. In this regard, both the absorbance and interference optics showed better resolution and single narrower major peaks when compared with the equivalent data sets for heparin (Fig. 3 in Ref. 19). The most likely explanation for this appears to be variability in the sulfate content within the heparin fragments, causing variations in mass that resulted in a broader peak width. This effect would not be present in HS because of the reduced sulfation level in HS. The similar single peak widths from either absorbance or interference optics suggest that all eight HS fragments showed narrow size distributions and are relatively homogenous, in agreement with the chromatography results above. The smaller HS fragments show slightly broader peak widths than the larger ones, and this is attributed to a higher back-diffusion effect. Unlike heparin, a slight decrease in the s_20,w values of the absorbance and interference data was observed with increase of rotor speed; this indicated that the s_20,w values depend on the rotor speed (Fig. 4, A and B). Inspection of the boundary fits showed that this slight rotor speed dependence resulted from a contribution from back-diffusion in the c(s) fits that was reduced with increase in rotor speed. Accordingly, although the values at the highest speed were taken to be more valid, these were within error of the averaged values (Table 1).

FIGURE 4.

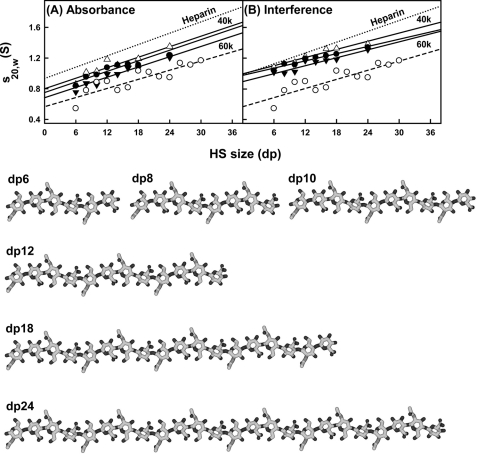
Comparison of the experimental and predicted sedimentation coefficients for eight HS dp6–dp24 fragments. The filled circles and triangles represent the experimental values for dp6–dp24. The open circles (○) represent the predicted values for linear dp6–dp30 models. For comparison, the equivalent heparin experimental data from Ref. 19 are shown as a dotted line in A and B. A, comparison with the experimental sedimentation coefficients at rotor speeds of 40,000 (△), 50,000 (●), and 60,000 (▾) rpm using absorbance optics. B, comparison with the experimental sedimentation coefficients at rotor speeds of 40,000 (△), 50,000 (●), and 60,000 (▾) rpm using interference optics. Bottom, the linear models for HS dp6–dp24 that were created starting from the HS dp4 crystal structure (Protein Data Bank code 3E7J).

Like heparin, the HS analyses revealed s_20,w values that increased with increase in size of the fragments (Fig. 4, A and B). A typical molecular mass of the most abundant HS dp2 structure shown in Fig. 1A is 483 Da, whereas the corresponding value for heparin dp2 is 628 Da (Fig. 1A of Ref. 19). Because of the differences in molecular size, the Svedberg equation predicts that the s_20,w values of the HS fragments will be 77% of those for the equivalent sized heparin fragments. The mean s_20,w value for HS dp24 is 1.30 ± 0.06 S (Table 1), which is 86 ± 8% of the corresponding value for heparin dp24 of 1.52 ± 0.07 S (Table 1 of Ref. 19). This is almost within error of the mass-predicted reduction in s_20,w value. If real, the difference between the 77 and 86% values would correspond to a 10% smaller frictional coefficient for HS compared with heparin (i.e. HS may have a slightly more compact solution structure than heparin).

The sedimentation coefficient s⁰_20,w values were calculated using HYDROPRO software from molecular models of HS. For this, 13 linear HS models (dp6–dp30) were computed, starting from the HS dp4 crystal structure seen in its complex with heparinize II (20). Although the rate of increase of the s⁰_20,w values with size was predicted correctly, the theoretical s⁰_20,w values for HS were consistently lower than those seen experimentally (Fig. 4, A and B). The lower theoretical values for the HS fragments when compared with their experimental values show that HS sediments more rapidly than predicted (i.e. the overall solution structures are more compact through bending than the linear HS structures). Below, this difference in Fig. 4, A and B, is explained by the best fit bent HS structures that were modeled from the scattering data. It is noteworthy that this difference between experimental and theoretical values was not seen for heparin (19); this shows that the properties of the HS and heparin solution structures differ. In addition, the linear HS and heparin structures differ in their degree of elongation. The theoretical s⁰_20,w value for a linear HS structure is 70% lower than the corresponding theoretical value for a linear heparin structure, in reflection of a longer linear HS structure.

X-ray Solution Scattering Data for Eight HS Fragments

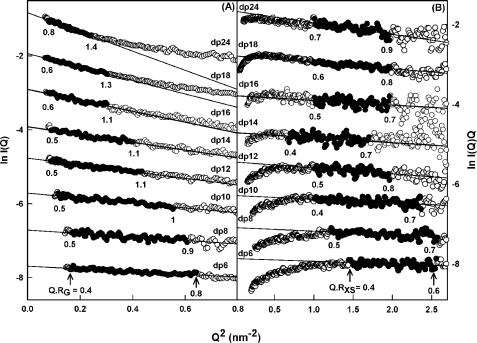
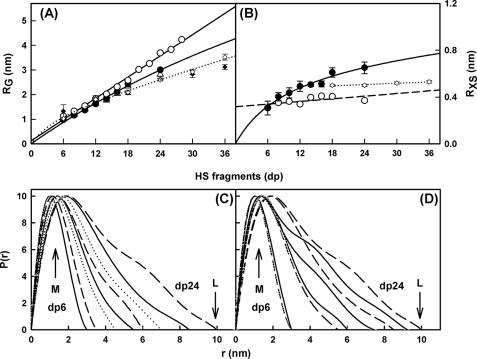
Solution scattering is a diffraction technique that provides structural information on biological macromolecules in random orientations in solution (21, 22). In order to complement the analytical ultracentrifugation data in more detail, the solution structures of the same eight HS fragments, dp6–dp24, at 0.5 mg/ml were characterized by synchrotron x-ray scattering. The scattering experiments report the scattering curve I(Q) as a function of scattering angle Q. Tests for possible radiation damage effects (see “Experimental Procedures”) showed that these were not detectable; accordingly, data were acquired with the longest exposure time of 1 s in order to maximize signal/noise ratios. Guinier analyses of ln I(Q) versus Q² at low Q values gives the radius of gyration R_G, which monitors the degree of macromolecular elongation. Because of the very different sizes of the HS fragments, different Q ranges were required for each fragment in order to work within acceptable linear Q·R_G ranges (Fig. 5A). Thus, the Guinier fit Q range of 0.4–0.8 nm⁻¹ for dp6 was successively reduced in stages to that of 0.28–0.55 nm⁻¹ for dp24 (Fig. 5A). The mean Guinier R_G values increased from 1.03 ± 0.08 nm for dp6 up to 2.82 ± 0.10 nm for dp24 (Table 1). These R_G values for the eight HS fragments do not show a linear relationship with the size of the HS fragments, unlike the R_G values calculated from linear models, showing reduced values instead (Fig. 6A). Thus, these HS fragments show bending in solution. In addition, the experimental HS R_G values are larger for the dp18–dp36 fragments than those seen for the heparin dp18 and dp24 fragments (Fig. 6A). This shows that HS has a more elongated structure than that of heparin.

FIGURE 5.

Experimental Guinier x-ray scattering analyses of eight HS dp6–dp24 fragments. A, Guinier R_G plots for dp6–dp24 at concentrations of 0.5 mg/ml. The filled circles were used to determine the radius of gyration R_G, based on the best fit lines as shown. The Q ranges used for the R_G analyses were 0.40–0.8 nm⁻¹ for dp6, 0.42–0.78 nm⁻¹ for dp8, 0.34–0.74 nm⁻¹ for dp10, 0.30–0.66 nm⁻¹ for dp12, 0.30–0.64 nm⁻¹ for dp14, 0.29–0.55 nm⁻¹ for dp16, 0.28–0.55 nm⁻¹ for dp18, and 0.28–0.55 nm⁻¹ for dp24. B, Guinier R_XS plots for dp6–dp24. The filled circles represent the Q ranges used to determine the cross-sectional radius of gyration R_XS, based on the best fit lines as shown. The Q ranges used for R_XS analyses were 1.2–1.6 nm⁻¹ for dp6, 1.1–1.6 nm⁻¹ for dp8, 1.0–1.54 nm⁻¹ for dp10, 1.0–1.44 nm⁻¹ for dp12, 0.82–1.3 nm⁻¹ for dp14, and 1.0–1.4 nm⁻¹ for dp16, dp18, and dp24.

FIGURE 6.

Experimental Guinier and P(r) x-ray data analyses of eight HS dp6–dp24 fragments. A, comparison of the experimental R_G values from Guinier plots (△) and P(r) curves (●) with the predicted R_G values calculated from the linear models of Fig. 4 (○). The six corresponding values for heparin from (19) are denoted by open and filled diamonds, respectively, and fitted to a dotted line. B, comparison of the experimental cross-sectional R_XS values (●) with the predicted R_XS values calculated from the linear models of Fig. 4 (○). The corresponding four values for heparin dp18–dp36 from Ref. 19 are denoted by open diamonds and fitted to a dotted line. C, the distance distribution function P(r) analyses for dp6–dp24. The r values of the maximum at M were 1.02 nm (dp6), 1.15 nm (dp8), 1.30 nm (dp10), 1.43 nm (dp12), 1.44 nm (dp14), 1.61 nm (dp16), 1.87 nm (dp18), and 1.90 nm (dp24). The eight fragments are denoted by continuous, dashed, and dotted lines in alternation. D, comparison of the P(r) analyses for HS dp6–dp24 with heparin dp6–dp24. The curves corresponding to the four HS fragments dp6, dp12, dp18, and dp24 are denoted by dashed lines, whereas the corresponding four curves for heparin are denoted by continuous lines. The heparin P(r) data are from Ref. 19.

Macromolecules that are sufficiently elongated in shape will show a cross-sectional radius of gyration R_XS value. The R_XS value monitors the degree of bending within the macromolecular length. As for the R_G analyses, different Q ranges were used for the R_XS fits for the different HS fragments, depending on the size of the HS fragment, all of which were larger than the Q ranges used for the R_G analyses (Fig. 5B). In all cases, despite the worsened signal/noise ratios of the I(Q) data, linear fit ranges were identified in the plots of ln I(Q)·Q versus Q². These gave experimental R_XS values of 0.31 nm for dp6 that increased up to 0.65 nm for dp24 (Fig. 6B and Table 1). This increase in the R_XS values correlated with the deviation of the R_G values from linearity (Fig. 6A). They were larger than the calculated R_XS values from 0.31 to 0.40 nm for the linear HS dp6–dp30 models, thus supporting the conclusion that the HS fragments become progressively more bent with increase in size. Combination of the R_G and R_XS values according to the relationship L² = 12 (R_G² − R_XS²) for an elliptical cylinder (36) showed that HS dp6, dp8, dp10, dp12, dp14, dp16, dp18, and dp24 have approximate lengths of 3.4, 3.9, 4.6, 5.5, 5.8, 6.8, 7.8, and 9.5 nm in that order. Similar lengths of 7.0, 9.1, 9.6, and 10.7 nm were observed for the heparin dp18, dp24, dp30, and dp36 fragments (19). In conclusion, the comparison of the dp18 and dp24 lengths showed that HS has a longer structure than that of heparin, in addition to being more bent than heparin.

The distance distribution function P(r) is calculated from the full Q range of the I(Q) scattering curve (see “Experimental Procedures”). This provides R_G values and model-independent determinations of the overall length L following an assumption of the maximum dimension D_max (Fig. 6C); note that L is not a contour length. The mean R_G values obtained from the P(r) curves increase from 0.98 ± 0.05 nm for dp6 to 3.0 ± 0.05 nm for dp24 (Table 1). These P(r) R_G values are in excellent accord with the corresponding Guinier R_G values from the low Q values and follow the same trends with size (Table 1 and Fig. 6A). Model-independent L values are determined from the r value where the P(r) curve reaches zero at large r. These experimental L values were 3.0 nm for dp6, 3.5 nm for dp8, 4.5 nm for dp10, 5.5 nm for dp12, 6.0 nm for dp14, 7.0 nm for dp16, 8.5 nm for dp18, and 10.0 nm for dp24. These values show increasing deviation with size from the longer lengths measured for the linear HS dp6–dp24 models (i.e. 3.5 nm for dp6, 4.1 nm for dp8, 5.6 nm for dp10, 6.5 nm for dp12, 7.2 nm for dp14, 7.8 nm for dp16, 9.2 nm for dp18, and 11.2 nm for dp24), noting that a hydration shell of thickness 0.6 nm (2 × 0.3 nm) is added to these linear model lengths (44). These experimental L values from the P(r) curves are more accurate compared with the approximate L values calculated from the R_G and R_XS values that assumed an elliptical cylinder shape for HS; however, these approximate L values show that the R_G and R_XS values are consistent with the P(r) analyses. The P(r) curves also provide the most frequently occurring interatomic distance M within the heparin structure from the r value of the peak maximum. M was observed at r values that started at 1.02 nm for dp6 and increased to 1.90 nm for dp24 (Fig. 6C). In conclusion, these comparisons show that HS has a progressively more bent solution structure with increase in size. Concurrently with this, the lengths L of HS dp18 and dp24 are longer than those for the corresponding heparin dp18 and dp24 fragments (Fig. 6D). Hence, HS is also longer as well as being more bent than heparin.

Constrained Modeling of Six HS Fragments

The experimental x-ray R_G and R_XS values showed that the solution structures for HS are longer and more bent than those of heparin. Here, constrained scattering modeling was performed with HS in order to provide a molecular explanation of these scattering data. The linear models created from the HS dp4 crystal structure were the starting constraint. All eight HS fragments dp6–dp24 were subjected to modeling. They were considered as belonging to a structurally homologous series. As illustrated in the previous modeling of heparin, the linkage connectivity between the oligosaccharide rings was maintained (Fig. 1A), whereas the Φ and Ψ rotational angles at each glycosidic linkage were varied randomly in a range of up to ±45° from their starting values. In all, 5000–8000 models for each of the eight HS fragments were generated. For each fragment, x-ray scattering curves were calculated from these randomized models and fitted to the experimental curve. The R_G, R_XS, and R factor values were calculated for each modeled curve, where the R_G and R_XS values were calculated using the same Q range used for the experimental Guinier fits (Fig. 5, A and B), and the R factor is a measurement of goodness of fit. The R factor distributions (supplemental Fig. S1, A–F) showed that all of the dp6–dp16 models (yellow circles) encompassed the experimental R_G values (dashed lines) and that the R factor values were well below the level of 5% usually expected for excellent curve fits (47). Totals of 5000 models for dp6–dp10 and 8000 models for dp12–dp16 provided enough randomized conformers in order to be able to determine best fit HS solution structures. The lowest R factors showed the best agreement with the experimental x-ray curves. At these minima, the best fit R_G values (red and cyan circles) agreed well with the experimental R_G values. The best fit R_XS values also showed good agreement with the experimental R_XS values (supplemental Fig. S1, G–L). In distinction, the linear models for the dp6–dp16 HS fragments showed more marked deviations from the experimental R_G and R_XS values (green circles). Of interest was that this modeling procedure could not be applied to HS dp18 or dp24 (not shown). There, no minimum R factor could be identified in what turned out to be comparatively flat distributions, unlike those in supplemental Fig. S1, A–F. In addition, the curve fits showed better agreement for the linear models of dp18 or dp24 than the bent HS models, which was contrary to the analyses of Figs. 4 and 6. The inability to fit the x-ray curve was attributed to the lack of a single well defined solution structure for dp18 and dp24, unlike the cases of dp6–dp16.

For HS dp6, the modeling analyses showed that a nearly linear structure with slight bending accounted for the x-ray and analytical ultracentrifugation data. The eight best fit models gave R factors of 4.4–4.5%, R_G values of 1.01–1.03 nm, R_XS values of 0.30–0.31 nm, and maximum lengths L of 3.0–3.5 nm. These agree well with the experimental R_G value of 1.03 ± 0.08 nm, R_XS value of 0.31 ± 0.06 nm, and the P(r) length of 3.0 nm (Table 1). Contrary to these agreements, the linear dp6 model gave a higher R factor of 5.3%, an R_G value of 1.10 nm, and an L value of 3.5 nm. The visual agreement between the experimental and modeled I(Q) curves and P(r) curves was excellent (Fig. 7A). The calculated s⁰_20,w values from the eight best fit models gave 0.52–0.79 S. These are lower but comparable with the experimental s_20,w values of 0.82 ± 0.05 S and 1.05 ± 0.04 S, given that the typical accuracy of the s⁰_20,w calculation is ±0.21 S (22).

FIGURE 7.

X-ray modeling curve fits for best fit and poor fit HS dp6–dp16 models. The main panels depict the I(Q) curve fits, and the insets show the P(r) distance distribution function fits. The experimental I(Q) and P(r) scattering data are represented by black circles and lines, respectively; the red lines and models correspond to the best fit dp6 (A), dp8 (B), dp10 (C), dp12 (D), dp14 (E), and dp16 (F) models from the trial-and-error searches; and the green lines and models correspond to the linear poor fit dp6–dp16 models from Fig. 4. The best fit and linear models are shown in the bottom left corner, together with their maximum lengths L in nm for comparison with the experimental L values in the P(r) curves.

For HS dp8, the modeling analyses indicated slightly bent structures similar to those seen for dp6. The eight best fit models gave R factors of 4.4%, R_G values of 1.17–1.18 nm, R_XS values of 0.39–0.49 nm, and L values of 3.7–3.8 nm. These values agree well with the experimental R_G value of 1.19 ± 0.08 nm, R_XS value of 0.40 ± 0.03 nm, and the P(r) length of 3.5 nm (Table 1). Contrary to these agreements, the linear model again showed a higher R factor of 5.3% and R_G value of 1.28 nm, a lower R_XS value of 0.35 nm, and a larger L value of 4.1 nm. The visual agreement of the experimental and modeled I(Q) and P(r) curves was excellent (Fig. 7B). The eight calculated s⁰_20,w values of 0.45–0.80 S are smaller than the experimental s_20,w values of 0.94 ± 0.06 S and 1.06 ± 0.08 S; however the best fit model gave a calculated value of 0.77 S, which is within the error of experiment and the typical accuracy of ± 0.21 S (22).

For HS dp10, the modeling analyses showed good agreements with slightly bent structures, in which the deviation from a linear dp10 structure for dp10 was slightly increased. The eight best fit dp10 models gave R factors of 4.3–4.4%, R_G values of 1.40–1.43 nm, R_XS values of 0.42–0.47 nm, and L values of 4.7–4.8 nm. These correspond well with the experimental R_G value of 1.41 ± 0.07 nm, R_XS value of 0.44 ± 0.04 nm, and L value of 4.5 nm (Table 1). The deviations from a linear dp10 model are larger, for which the R factor is 4.5%, the R_G value is 1.46 nm, the R_XS value is 0.36 nm, and the L value is 5.6 nm. The visual agreement of the experimental and modeled I(Q) and P(r) curves was again excellent (Fig. 7C). The eight modeled s⁰_20,w values of 0.48–0.95 S are similar to the experimental s_20,w values of 0.95 ± 0.09 S and 1.09 ± 0.06 S, with the best fit model giving a modeled value of 0.91 S, within the typical accuracy of ±0.21 S (22).

For HS dp12, the modeling analyses were also successful, in which the deviation from a linear dp12 structure was greater. The eight best fit models gave R factors of 4.2%, R_G values of 1.64–1.65 nm, R_XS values of 0.49–0.50 nm, and L values of 5.3–5.8 nm. These agree well with the experimental R_G value of 1.65 ± 0.09 nm, R_XS value of 0.49 ± 0.04 nm, and L value of 5.5 nm (Table 1). The linear model showed a higher R factor of 4.6%, a higher R_G value of 1.74 nm, a reduced R_XS value of 0.34 nm, and a longer L value of 6.5 nm. The visual agreement of the experimental and modeled I(Q) and P(r) curves was excellent (Fig. 7D). The eight modeled s⁰_20,w values of 0.47–0.98 S are comparable with the experimental s_20,w values of 1.08 ± 0.09 and 1.16 ± 0.05 S, with the best fit model giving a value of 0.92 S.

For HS dp14, good agreements between the models and experimental data were obtained, whereas the deviation from a linear dp14 structure was larger. The eight best fit dp14 models gave R factors of 4.4%, R_G values of 1.75–1.78 nm, R_XS values of 0.48–0.51 nm, and L values of 6.0–6.5 nm. These agree well with the experimental R_G value of 1.76 ± 0.07 nm and R_XS value of 0.51 ± 0.02 nm, and L value of 6.0 nm (Table 1). These deviated from the linear dp14 model, which had an R factor of 4.5%, a higher R_G value of 1.88 nm, a lower R_XS value of 0.39 nm, and an L value of 7.2 nm. Again the visual agreement of the experimental and modeled I(Q) and P(r) curves was excellent (Fig. 7E). The eight modeled s⁰_20,w values of 0.74–1.05 S compare well with the experimental s_20,w value of 1.07 ± 0.07 and 1.18 ± 0.04 S, respectively, where the best fit model gave a value of 0.96 S.

For HS dp16, the outcome of the modeling analyses was similar to that of dp14. The eight best fit models gave R factors of 6.3–6.4%, R_G values of 1.92–2.02 nm, R_XS values of 0.49–0.52 nm, and L values of 6.5–7.2 nm. These agree well with the experimental R_G value of 2.03 ± 0.07 nm, R_XS value of 0.52 ± 0.01 nm, and L value of 7.0 nm (Table 1). The linear model gave a poorer fit with an R factor of 6.5%, R_G value of 2.12 nm, R_XS value of 0.41 nm, and L value of 7.8 nm. The experimental and modeled I(Q) and P(r) curves showed excellent agreement (Fig. 7F) The eight modeled s⁰_20,w values of 0.61–1.1 S are similar to the experimental s_20,w values of 1.10 ± 0.03 and 1.24 ± 0.08 S, with the best fit model giving a value of 1.10 S, again within the typical accuracy of ±0.21 S (22).

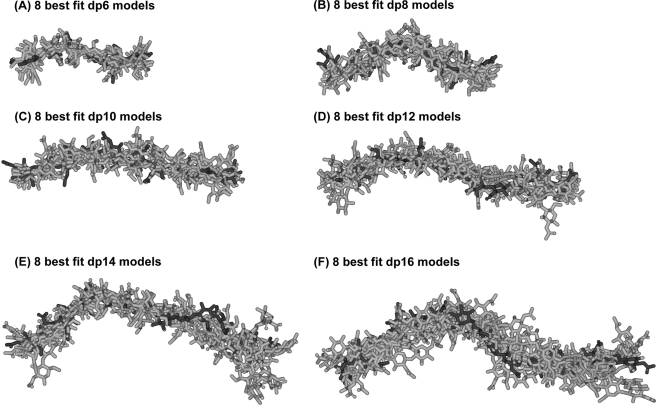
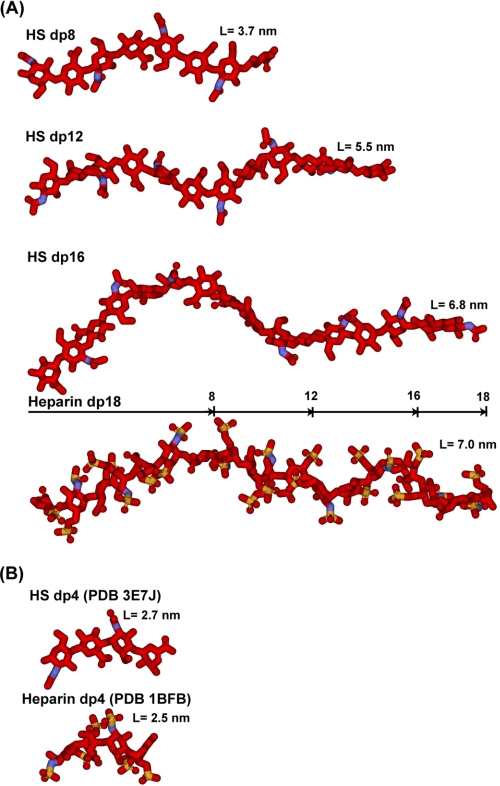
In conclusion, the best fit models for HS dp6–dp16 show progressively more bent structures in solution with increase in HS size. This is visible from the superimposition of the eight best fit models for each HS fragment (Fig. 8). In terms of lengths, crystal structures containing HS and heparin showed that the glycosidic linkage in HS has a length similar to that in heparin (Fig. 9B). In HS, the separation between the C1 and C4 atoms of GlcA-GlcNAc is 0.237 ± 0.003 nm, and that between the C1 and C4 atoms of GlcNAc-GlcA is 0.235 ± 0.002 nm. For heparin, analyses of five crystal structures containing dp6 showed that very similar C1 and C4 separations were seen between IdoA-GlcNS of 0.241 ± 0.004 nm and between GlcNS-IdoA of 0.243 ± 0.006 nm. Thus, the increased length of HS in solution compared with heparin is therefore principally the consequence of altered Φ and Ψ angles. In terms of rotational bend, it is noteworthy that the crystallographic Φ and Ψ angles for smaller HS and heparin structures are in agreement with each other within error (Table 2 and supplemental Fig. S2). However, the comparison of the Φ and Ψ angles of HS and heparin showed that these values differed (supplemental Fig. S2). The mean Φ and Ψ angles for the GlcA-GlcNAc glycosidic linkage were −93 and 134° in HS and are similar to the corresponding IdoA-GlcNS values of −61 and 132° in heparin, although the S.D. values are large at ±21–36° (Table 2). In distinction, the mean Φ and Ψ angles for the GlcNAc-GlcA linker were 4 and 138° in HS, which are more different from the corresponding values of 98 and 86° for the GlcNS-IdoA linker in heparin, although the S.D. values are again large (Table 2). The distribution of the Φ and Ψ values in supplemental Fig. S2B suggests that there is more rotational variability at this second glycosidic linkage in HS when compared with those for heparin in supplemental Fig. S2D. In conclusion, when compared with the corresponding solution structures of large heparin fragments, the modeling of the large fragments of HS showed structures that were longer and more bent in solution than those of heparin (Fig. 9A).

FIGURE 8.

Superimposition of the eight best fit models for each of the six HS dp6–dp16 fragments. Each set of eight best fit models for the six HS fragments (dp6 (A), dp8 (B), dp10 (C), dp12 (D), dp14 (E), and dp16 (F)) was superimposed globally using Discovery Studio VISUALISER software, and then their non-hydrogen atoms were displayed as shown. Each best fit model from Fig. 7 is shown in black, whereas the seven related best fit structures are shown in gray.

FIGURE 9.

Comparison of the best fit HS dp8–dp16 structures with the equivalent heparin dp8–dp16 structures. Red, carbon and oxygen; blue, nitrogen; sulfur, yellow. A, three best fit HS models (dp8, dp12, and dp16) are compared with the heparin dp18 model at the bottom, all drawn to the same scale. The lengths of heparin dp8, dp12, and dp16 are indicated in the heparin dp18 structure for comparison with HS. B, the glycosidic linkages in the crystal structure of HS dp4 complexed with heparinase II (Protein Data Bank code 3E7J) are compared with the crystal structure of the complex of heparin dp4 with fibroblast growth factor (Protein Data Bank code 1BFB).

TABLE 2.

Summary of the Φ and Ψ angle in the solution and crystal structures of HS and heparin

	GlcA-GlcNAc		GlcNAc-GlcA
	Φ	Ψ	Φ	Ψ
	degrees		degrees
HS crystal structurea	−90 ± 1	127 ± 4	83 ± 3	94 ± 1
HS dp6	−101 ± 38	140 ± 19	−16 ± 27	129 ± 48
HS dp8	−93 ± 26	121 ± 29	34 ± 55	152 ± 15
HS dp10	−121 ± 26	126 ± 17	18 ± 41	143 ± 23
HS dp12	−78 ± 30	114 ± 26	24 ± 35	121 ± 30
HS dp14	−64 ± 32	149 ± 21	−12 ± 53	139 ± 42
HS dp16	−112 ± 38	136 ± 45	−11 ± 88	143 ± 52
Average (27 of 35 values)	−93 ± 36	134 ± 32	4 ± 58	138 ± 37

	IdoA-GlcNS		GlcNS-IdoA
	Φ	Ψ	Φ	Ψ
19 heparin crystal structures	−79 ± 20	132 ± 19	84 ± 22	100 ± 19
Heparin dp18	−52 ± 22	135 ± 15	104 ± 27	96 ± 25
Heparin dp24	−61 ± 17	127 ± 20	100 ± 26	78 ± 17
Heparin dp30	−64 ± 21	136 ± 21	87 ± 25	82 ± 22
Heparin dp36	−63 ± 23	129 ± 27	103 ± 22	89 ± 24
Average (50 of 54 values)	−61 ± 21	132 ± 22	98 ± 25	86 ± 22

^a The mean value from two HS dp4 molecules seen in the crystal structure (Protein Data Bank code 3E7J).

DISCUSSION

The size and spacing of S domains in heparan sulfate are proposed to be as important to its biologically significant interactions with proteins as are the detailed sequences of the S domains themselves (48). Heparin, a commonly used model compound for heparan sulfate, consists of lengthy S domains, made up largely of the repeating trisulfated disaccharide shown in Fig. 1B, separated by much smaller, unsulfated NA domains. In heparan sulfate, the position is reversed, and long NA domain sequences (Fig. 1A) act as spacers to separate the short S domains. Although the S domain conformation, exemplified by heparin, has been the subject of numerous studies (49), the NA domain has not. It has been proposed that the NA domain repeating sequences are both less flexible (50) and more flexible (51) than the S domains.

By this study, we have determined molecular solution structures for small and medium sized HS fragments dp6 to dp16 and obtained insights into the structures of HS dp18 and dp24. The fragments we used were produced by extensive depolymerization using heparinase 1, an enzyme that cleaves only within the S domains (28) leaving NA domains untouched. It is therefore likely that some minor degree of sulfation remains at the reducing and non-reducing ends of our fragments but that internal disaccharides are unsulfated. Such fragments bear a closer resemblance to NA domains of intact HS than the most commonly used model compound for this sequence, the capsular polysaccharide from Escherichia coli K5 (50, 51). These HS structures comprise extended ones with flexibility around the glycosidic linkages between monosaccharide residues and further evidence of flexibility in the larger structures. These structures provide novel comparative insight into the structures of HS and heparin and the likely manner in which these two polyanionic oligosaccharides interact with their protein ligands. Previously, we reported that heparin dp6–dp36 exhibited a semirigid structure in solution (19). HS and heparin both share similar covalent structures (Fig. 1). The comparison of our two sets of structures for HS and heparin becomes essentially that between NA domains and S domains. The greater length and bending of HS compared with heparin (Fig. 9) may be attributed to the difference in uronic acid residue, in which GlcNAc alternates with GlcA in HS, causing HS to adopt a conformation distinct from that of fully sulfated heparin (52). The regular repeats of sulfate groups in heparin that are absent in HS are also expected to contribute to the relative rigidity of heparin.

The application of constrained scattering modeling has proved to be as effective for the HS fragments as it was for heparin previously. Usually, scattering fits are performed for protein structures with masses of 20–100 kDa and higher (21, 22). The HS fragments dp6–dp24 and the heparin fragments dp6–dp36 are an order of magnitude smaller in size with masses of 1–7 and 2–11 kDa, respectively. The ability to measure these scattering curves was attributed to improved detector technology at the European Synchrotron Radiation Facility synchrotron and the low background levels at the instrument. The constrained scattering modeling technique determines a three-dimensional molecular structure that best accounts for the observed scattering curve through trial-and-error searches that rule out structures that are incompatible with the observed scattering curves. By fixing the analyses to what is already known about the macromolecule, namely the carbohydrate rings, and varying only the flexible regions, namely the Φ and Ψ angles of each glycosidic linker, significantly fewer modeling variables are involved in the scattering fits. Through the variation of these Φ and Ψ angles, the analysis of 5000–8000 models provided sufficient statistical detail to result in clear V-shaped graphs of R factor versus R_G and R factor versus R_XS values. The best fit models were identified by the lowest R factor values, and these were verified by the agreement of the modeled and experimental R_G and R_XS values at this point. The quality of the HS dp6–dp16 scattering fits was similar to that of the heparin dp18–dp36 fits (19). Interestingly, no good fits could be obtained for the HS dp18 and dp24 structures. The monodispersity of these two fragments had been established by the single peaks seen in the ultracentrifugation c(s) analyses (Fig. 3); thus, the possibility of sample heterogeneity can be ruled out as an explanation for the lack of fit. It is more likely that the accumulation of conformational variability at 17 and 23 glycosidic linkages in HS dp18 and dp24, compared with the variability between 5 and 15 linkages in HS dp6–dp16, has resulted in the appearance of multiple conformations for HS dp18 and dp24 that precluded the ability to fit one single conformation to these two scattering curves. This modeling outcome is distinct from that for heparin dp6–dp36 when good fits could be obtained for all six structures. This outcome suggested that the heparin structures show greater rigidity than the HS fragments.

The solution structures of the HS dp6–dp16 fragments exhibited a degree of bending (Figs. 8 and 9A). In addition, the HS structures are longer by reason of alterations in the glycosidic Φ and Ψ angles (supplemental Fig. S2). The physical basis of these changes in HS is likely to arise from the GlcA-GlcNAc sequences (as opposed to the IdoA-GlcNS sequences in heparin), together with less repulsion between sulfate and sulfate, between sulfate and carboxylate, and between carboxylate and carboxylate groups in HS.

This study has provided fresh insight into potential HS-protein interactions and heparin-protein interactions. The outcome of 19 protein-heparin crystal structures has been discussed previously (19). In distinction, only one protein-HS crystal structure is known (20). It was of interest that the Φ and Ψ angles for this latter structure are similar to those seen in the 19 heparin-protein crystal structures (Table 2). Several studies of HS-protein interaction have noted that SAS sequences, in which two short S domains are separated by an NA sequence, are preferred for optimum binding (48). This is particularly true for oligomeric proteins, such as, for example, MIP-1a or platelet factor 4 (7, 53). In these cases, the multiple heparin binding sites on the oligomer are not always arranged in a linear way, so that a single long S domain cannot readily bind to more than one site on the same multimer. It has been reasonable to suppose that NA domains, composed of alternating α-(1→4)- and β-(1→4)-linked hexopyranoses, would be more flexible than the unusually stiff heparin structure of S domains, allowing an SAS domain to bend in order to present two S domains to heparin binding sites on opposite sides of a protein complex. The conclusion that can be drawn from our present study of HS NA fragments supports this intuitive reasoning.