Skip to main content
PMC home page
Applied Physics Letters logoLink to Applied Physics Letters
. 2011 Jul 1;98(26):264107–264107-3. doi: 10.1063/1.3608155

Optimal signal-to-noise ratio for silicon nanowire biochemical sensors

Nitin K Rajan 1, David A Routenberg 2, Mark A Reed 1,3,a)
PMCID: PMC3144966  PMID: 21799538

Abstract

The signal-to-noise ratio (SNR) for silicon nanowire field-effect transistors operated in an electrolyte environment is an essential figure-of-merit to characterize and compare the detection limit of such devices when used in an exposed channel configuration as biochemical sensors. We employ low frequency noise measurements to determine the regime for optimal SNR. We find that SNR is not significantly affected by the electrolyte concentration, composition, or pH, leading us to conclude that the major contributions to the SNR come from the intrinsic device quality. The results presented here show that SNR is maximized at the peak transconductance.

Silicon nanowire field-effect transistors (SiNW-FET) have shown great sensitivity when employed as biological∕chemical sensors (bioFETs).1, 2, 3 The principle of operation is that a charged species bound to the nanowire (NW) surface (modified with some receptor molecules) induces a change in surface potential at the NW surface, which translates into a change in drain-to-source current (ΔI) which is then measured. It is well-known that the sensitivity (defined as ΔI∕I for a current based sensing experiment) is maximized in the subthreshold regime.4, 5, 6 However, it is also known that the normalized current noise power amplitude (SI∕I2) reaches a plateau and is highest in the subthreshold regime for silicon-silicon oxide devices7, 8 and concerns have been expressed that signal-to-noise ratio (SNR) would be impacted for measurements carried out in subthreshold.4, 9 On the other hand, SI∕I2 is lower in the linear regime but the sensitivity is also lower. In order to determine the ideal regime for optimal SNR, we carry out both I-V and noise measurements for solution gated devices. Our measurements indicate that the current noise is independent of electrolyte concentration, composition, or pH, leading us to conclude that the intrinsic electronic properties of the SiNW bioFETs determine the optimal SNR achievable by these sensors. We also find that SNR is maximized in the linear regime at the point where the transconductance is largest.

The SiNWs were fabricated from SOI wafers (Soitec) with a high resistivity boron doped active layer (>2000 ohm cm) as described previously.10 Devices used for the experiments were nominally 100 nm wide and 5 μm long. The devices were covered with a passivation layer of SU-8 (an epoxy based negative photoresist) with windows opened for the NW channel and the contact pads. An optical micrograph of the devices is shown in Fig. 1b. The NW surfaces were functionalized with a monolayer of APTES (3-aminopropyltriethoxysilane) using the protocol described earlier,11 which increases device stability and reduces gate leakage current in solution. A fluidic well was then glued on top of each chip for the sensing experiments, with a platinum wire used as the solution gate electrode. The device structure and experimental setup is shown schematically in Fig. 1a. For all noise and transfer characteristics measurements, the back-gate contact was left floating and the source-drain voltage was kept fixed at 100 mV. A typical transfer (Id-Vsg) curve is shown in Fig. 1c, including the leakage current (Isg). For the noise measurements, the current, Id, was amplified using a current pre-amplifer (SRS 570) and current fluctuations recorded by a National Instruments DAQ card. The power spectral density was then determined using Welch’s modified periodogram method12 implemented in matlab. Similar 1∕f power spectra have been explicitly shown previously.13

Figure 1.

Figure 1

Open in a new tab

(Color online) (a) Schematic of the device structure and experimental setup. (b) Optical micrograph showing two parallel devices. The doped contact regions (pink) and the metal electrodes can be clearly seen as well as the openings in the SU8 layer (passivation layer) atop the nanowires (NWs). An SEM image of a single nanowire is shown. (c) I-V curve for a NW device (after APTES functionalization) showing the drain current (Id) and solution gate leakage current (Isg).

For number fluctuation noise, including correlated mobility fluctuations, the current noise power spectral density is given by7

SI=(1+αμ0CoxIdgm)2gm2SVFB, (1)

where α is the Coulombic scattering coefficient, µ0 is the low-field mobility, Cox is the oxide capacitance per unit area, and SVFB is the flatband-voltage noise spectral density due to fluctuations in the interface∕oxide charge. The low-field mobility was determined to be approximately 520 cm2∕Vs.

The root-mean-square (rms) current noise amplitude (δi) is obtained by integrating SI over the measurement bandwidth and taking the square root. The measured signal response (ΔI) is given by the change in surface potential (ψ0) multiplied by the transconductance (gm). Thus we arrive at the SNR which is given by

SNR=ΔIδi=Δψ0BW'gm(Vg)SI(f=1Hz), (2)

where BW′ = ln (f2∕f1) for low frequency cutoff f1 and high frequency cutoff f2 in the measurement bandwidth. For the rest of the paper we shall consider BW′ = 1 and refer to SNR for a change of 1 volt in surface potential. We carried out measurements in phosphate buffered saline (PBS) at three different concentrations as well as measurements using a different salt system, namely potassium chloride solution (KCl – 0.1X). The concentrations were limited to those values used commonly in sensing experiments. The results of the noise measurements are shown in Fig. 2. We observe negligible differences both in the noise spectra and the transfer characteristics, which result in similar SNR for the different concentrations and solutions investigated. This leads us to conclude that the gate response and the noise properties are solely a function of device properties, resulting in similar SNR across different electrolyte systems and concentrations. It is true that Debye screening is a function of buffer concentration and composition, and affects the sensitivity of the sensing experiment,14 but this variation only affects the Δψ0 seen at the NW surface. The SNR (defined per volt change in surface potential) is therefore independent of the actual change in surface potential and intrinsic to the device as our results indicate, which means that we can safely define and use this figure-of-merit to predict the detection limit of these bioFET sensors. We also observe that SNR for our devices is maximized in the linear regime, close to the peak transconductance (gm,peak), which is contrary to what was observed in other works,15, 16 where SNR was determined to be maximum in subthreshold. The difference in the operating regime at which maximum SNR occurs can be explained by different mobility fluctuation noise regimes13 (potentially caused by different material systems used, by different gate configurations, or by contact resistance dominated regimes). The power spectral density due to gate voltage fluctuations can be extracted using SVG = SI∕gm2. For a negligible scattering coefficient α, in the linear regime of operation, SVG = SVFB. Fig. 3 shows that SVG is not linearly proportional to gate voltage, which indicates a negligible α and for lower gate voltages, SVG is found to increase which is most likely due to the gate voltage dependence of SVFB as observed previously.13 From Fig. 3, we can also see that the gate voltage noise, which is important in the case of measurements of threshold voltage shifts and which corresponds to the inverse of SNR, is minimized in the region of peak transconductance. SVFB can be modeled as17

SVFB=λkTq2NtfWLCox2, (3)

where λ is the tunneling attenuation distance, W is the width, L is the length, Cox is the capacitance per unit area, and Nt is the trap density. For the data shown in Fig. 3. we estimate the minimum flatband-voltage noise at about 1.3 × 10−4 V2∕Hz and using dimensions W = 100 nm and L = 5 μm with a capacitance of 4.3 × 10−7 F∕cm2 (as previously modeled in Silvaco10), we extract a trap density of Nt = 2.3 × 1018 eV−1 cm−3. This trap density is comparable to values obtained for gate-all-around silicon nanowire devices8, 18 and slightly larger than values obtained for ultra-thin Si-FinFETs.19

Figure 2.

Figure 2

Open in a new tab

(Color online) (a) Normalized current noise power density at f = 1 Hz is plotted against drain current. The noise profile does not change significantly with changes in PBS (phosphate buffered saline) concentration or by changing the electrolyte to KCl (potassium chloride). The proportionality to (gm∕Id)2  confirms that our data are well fitted by the number fluctuation model. (b) Signal-to-noise ratio (as defined in text) is plotted against solution gate voltage to highlight the regime at which SNR is maximized. (c) Transconductance values extracted from I-V measurements are also plotted against solution gate voltage to point out that maximum SNR occurs close to the point of peak transconductance.

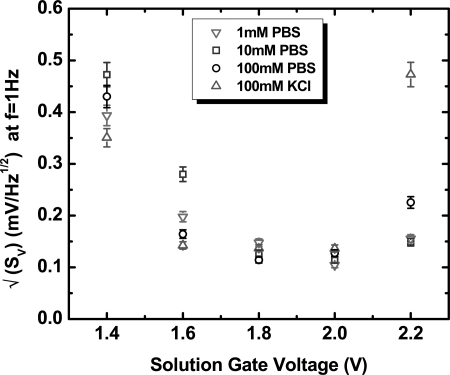
Figure 3.

Figure 3

Open in a new tab

(Color online) The gate voltage noise fluctuations (√SV) are plotted against solution gate voltage (limited to the linear regime of operation). The absence of a linear dependence indicates that number fluctuations are the dominant cause of the noise of these bioFET devices. The dip in the data also highlights the region of maximum signal-to-noise ratio (1∕√SV), which is again shown to occur around the region of peak transconductance.

Similar and consistent results were obtained for measurements carried out at different pH values, which show that the charge state of the NW surface (due to different solution pH) does not have a significant impact on the noise properties or SNR. The I-V curves show the expected threshold voltage shifts but, again, the peak transconductance and the peak SNR are both minimally affected by the change in pH and SNR is still maximized around the peak transconductance region. SNR is also a more important figure-of-merit in determining the limit of detection. For example, assuming a measurement bandwidth of 100 Hz, with a peak SNR of approximately 8000 per 1 V change in surface potential, we have Δψ0,min ≈ 3 × 10−4 V (assuming the minimum SNR to be 1) and assuming a NW capacitance of 2 × 10−15 F we obtain the minimum detectable charge to be around 4 elementary charges for our bioFETs. This of course does not consider screening or any surface chemistry which would reduce the effective charge that can be detected or affect the surface potential produced by such a charge. However, this represents the best case scenario of the minimum number of charges that can be detected by this bioFET sensor, given the intrinsic device SNR.

In this report, we study and characterize the SNR of Si NWs under a solution gate bias and different electrolyte composition, concentration, and pH. We conclude that SNR is an intrinsic device property and is maximized at the region of peak transconductance. SNR is a figure-of-merit which can be used to estimate the best possible detection limit for bioFET sensors and compare across different material systems and device designs.

Acknowledgments

This work was supported by the National Institute of Health under grant number 1RO1EB008260-1 and the Defense Threat Reduction Agency under grant number HDTRA1-10-1-0037.

References

  1. Cui Y., Wei Q. Q., Park H. K., and Lieber C. M., Science 293(5533), 1289 (2001). 10.1126/science.1062711 [DOI] [PubMed] [Google Scholar]
  2. Li Z., Chen Y., Li X., Kamins T. I., Nauka K., and Williams R. S., Nano Lett. 4(2), 245 (2004). 10.1021/nl034958e [DOI] [Google Scholar]
  3. Stern E., Klemic J. F., Routenberg D. A., Wyrembak P. N., Turner-Evans D. B., Hamilton A. D., LaVan D. A., Fahmy T. M., and Reed M. A., Nature 445(7127), 519 (2007). 10.1038/nature05498 [DOI] [PubMed] [Google Scholar]
  4. Cheng Y., Xiong P., Yun C. S., Strouse G. F., Zheng J. P., Yang R. S., and Wang Z. L., Nano Lett. 8(12), 4179 (2008). 10.1021/nl801696b [DOI] [PMC free article] [PubMed] [Google Scholar]
  5. Gao X. P. A., Zheng G. F., and Lieber C. M., Nano Lett. 10(2), 547 (2010). 10.1021/nl9034219 [DOI] [PMC free article] [PubMed] [Google Scholar]
  6. Routenberg D. A., “ Fabrication and Characterization of Silicon Nanowire Field-Effect Sensors,” Ph.D. thesis, Yale University, 2009. [Google Scholar]
  7. Ghibaudo G., Roux O., Nguyenduc C., Balestra F., and Brini J., Physica Status Solidi A 124(2), 571 (1991). 10.1002/pssa.v124:2 [DOI] [Google Scholar]
  8. Zhuge J., Wang R. S., Huang R., Tian Y., Zhang L. L., Kim D. W., Park D., and Wang Y. Y., IEEE Electron Device Lett. 30(1), 57 (2009). 10.1109/LED.2008.2007752 [DOI] [Google Scholar]
  9. Lin Y. M., Appenzeller J., Knoch J., Chen Z. H., and Avouris P., Nano Lett. 6(5), 930 (2006). 10.1021/nl052528d [DOI] [PubMed] [Google Scholar]
  10. Rajan N. K., Routenberg D. A., Jin C., and Reed M. A., IEEE Electron Device Lett. 31(6), 615 (2010). 10.1109/LED.2010.2047000 [DOI] [Google Scholar]
  11. Stern E., Vacic A., Rajan N. K., Criscione J. M., Park J., Ilic B. R., Mooney D. J., Reed M. A., and Fahmy T. M., Nat. Nanotechnol. 5(2), 138 (2010). 10.1038/nnano.2009.353 [DOI] [PMC free article] [PubMed] [Google Scholar]
  12. Welch P. D., IEEE Trans. Audio Electroacoust. Au15(2), 70 (1967). 10.1109/TAU.1967.1161901 [DOI] [Google Scholar]
  13. Rajan N. K., Routenberg D. A., Chen J., and Reed M. A., Appl. Phys. Lett. 97(24), 3 (2010). 10.1063/1.3526382 [DOI] [PMC free article] [PubMed] [Google Scholar]
  14. Stern E., Wagner R., Sigworth F. J., Breaker R., Fahmy T. M., and Reed M. A., Nano Lett. 7(11), 3405 (2007). 10.1021/nl071792z [DOI] [PMC free article] [PubMed] [Google Scholar]
  15. Heller I., Mannik J., Lemay S. G., and Dekker C., Nano Lett. 9(1), 377 (2009). 10.1021/nl8031636 [DOI] [PubMed] [Google Scholar]
  16. Tarasov A., Fu W., Knopfmacher O., Brunner J., Calame M., and Schonenberger C., Appl. Phys. Lett. 98(1) 012114 (2011). 10.1063/1.3536674 [DOI] [Google Scholar]
  17. Jayaraman R. and Sodini C. G., IEEE Trans. on Electron Devices 36(9), 1773 (1989). 10.1109/16.34242 [DOI] [Google Scholar]
  18. Wei C. Q., Jiang Y., Xiong Y. Z., Zhou X., Singh N., Rustagi S. C., Lo G. Q., and Kwong D. L., IEEE Electron Device Lett. 30(10), 1081 (2009). 10.1109/LED.2009.2027614 [DOI] [Google Scholar]
  19. Lim Y. F., Xiong Y. Z., Singh N., Yang R., Jiang Y., Loh W. Y., Bera L. K., Lo G. Q., and Kwong D. L., IEEE Electron Device Lett. 27(9), 765 (2006). 10.1109/LED.2006.880640 [DOI] [Google Scholar]

Articles from Applied Physics Letters are provided here courtesy of American Institute of Physics

RESOURCES