Abstract
Food restriction (FR) retards animals' growth. Understanding the underlying mechanisms of this phenomenon is important to conceptual problems in life-history theory, as well as to applied problems in animal husbandry and biomedicine. Despite a considerable amount of empirical data published since the 1930s, there is no relevant general theoretical framework that predicts how animals vary their energy budgets and life-history traits under FR. In this paper, we develop such a general quantitative model based on fundamental principles of metabolic energy allocation during ontogeny. This model predicts growth curves under varying conditions of FR, such as the compensatory growth, different age at which FR begins, its degree and its duration. Our model gives a quantitative explanation for the counterintuitive phenomenon that under FR, lower body temperature and lower metabolism lead to faster growth and larger adult size. This model also predicts that the animals experiencing FR reach the same fraction of their adult mass at the same age as their ad libitum counterparts. All predictions are well supported by empirical data from mammals and birds of varying body size, under different conditions of FR.
Keywords: energy allocation, food restriction, growth, metabolism
1. Introduction
Animals often face food scarcity and must vary their life-history characteristics in response. These responses can include foraging behaviours, the ages at which a certain developmental stage is reached, or reproductive efforts and so on [1–4]. Perhaps the most profound and direct life-history changes associated with low food availability or food restriction (FR) are retarded growth and reduced adult body size. Understanding the effects of FR on animals' energy budgets during growth is not only important to conceptual problems in life-history theory, but also to many applied problems. For example, in animal husbandry it has been suggested that appropriate FR on domestic birds can lighten body mass and improve total egg production (e.g. [5,6]). In biomedicine, it has been shown that FR (also known as caloric restriction) extends animals' lifespans and enhances their somatic maintenance functions (e.g. [7–9]). Despite the wealth and significance of empirical data derived from FR studies since the era of McCay in the 1930s [10], there has been no relevant theoretical framework that predicts how FR affects energy budgets and growth characteristics. Here, we present a general quantitative model based on fundamental principles of metabolic energy allocation during ontogeny, which provides a deeper understanding of the changes in life-history traits associated with the growth of mammals and birds under different conditions of FR.
We build on two ontogenetic growth models that together specify the complete metabolic energy allocation for animals fed ad libitum. The first model provides quantification of the fact that energy from food fuels growth [11]. When an animal is growing, a fraction of the energy assimilated from food is synthesized and stored as new biomass. The remaining fraction is used to fuel the total metabolic rate, Btot, which is dissipated as heat and not conserved in stored biomass [11]. This is described by
| 1.1 |
where A is the rate of intake of metabolizable energy from food, S (=EC(dm/dt)) is the rate of energy stored as new biomass, EC is the combustion energy content of one unit biomass and dm/dt is the rate of change in body mass, m, at time, t. The total metabolic rate, Btot, is the sum of the resting metabolic rate, Brest, and the rate of energy expenditure for locomotion, feeding and other activities, Bact. Btot can be expressed as Btot = Brest + Bact = fBrest, where f is a dimensionless parameter that reflects the activity level of the organism [11]. For wild mammals and birds, the value of f ranges from 2 to 3 with an average of 2.7. For caged animals, f is usually below 2 [11]. This relationship between total and resting metabolic rate is strongly supported by empirical data [11–13].
In the second model [14], the resting metabolic rate, Brest, is further partitioned into the rate of energy allocated to synthesizing new biomass, Bsyn, and the rate of energy allocated to maintenance of existing biomass Bmaint. Hence, we write: Brest = Bsyn + Bmaint [14]. The term Bsyn is expressed as Bsyn = Em(dm/dt), where Em is the amount of metabolic energy required to synthesize one unit of biomass. Em differs from EC in equation (1.1) in that EC is the amount of energy stored in one unit of biomass. Likewise, Bsyn differs from S in equation (1.1) in that S is the rate at which energy is accumulated as new biomass; the maintenance of existing biomass is expressed as Bmaint = Bmm, where Bm is the mass-specific maintenance metabolic rate. Empirical measurements and theoretical predictions provide evidence that resting metabolic rate Brest(m) is roughly equal to B0m3/4 [15–17], where B0 is a normalization constant for a given taxon and exponentially increases with body temperature as B0 = b0e−E0/(KT). The exponential factor e−E0/(KT) is called Boltzmann–Arrhenius (B–A) factor, where E0 is the average activation energy of metabolism (ca 0.65 eV), K is Boltzmann's constant (8.62 × 10−5 eV K−1) and T is body temperature in Kelvin [18]. The coefficient b0 is a constant within a taxon, and independent of body mass and temperature. Taken together, this gives a growth equation of the form:
| 1.2 |
When growth stops, that is, dm/dt = 0, and an animal reaches its adult mass, M, equation (1.2) gives B0M3/4 = BmM, and Bm = B0M−1/4.
A combination of equations (1.1) and (1.2) allows the food assimilation rate, A, to be expressed as a function of body mass during growth;
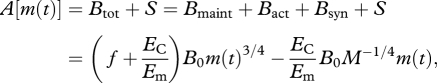 |
1.3 |
with four parameters, B0, f, EC and Em. Data for mammals and birds of diverse body sizes and taxa support the predictions of equation (1.3) [11].
When animals are under FR, their metabolic energy intake from food is lowered to a fraction, β, of that received by ad libitum animals, so that the assimilation rate of FR animals becomes AFR(t) = β × A(t). Under laboratory conditions, β ranges from 30 to 80 per cent and is usually set as a constant or a segment function of time [7]. In the field, β is a result of seasonally determined variations in abundance [1,2]. To derive the growth equation for animals under FR from equation (1.3), we assume that parameters, EC, Em and f, do not change under FR. EC and Em represent the combustion energy content of one unit biomass and the energy required to synthesize one unit of biomass, respectively. Their values correspond to the energetics of basic physico-chemical processes, quantified by heats of reaction, energies of formation and degradation, bond energies, etc. These values are elemental and do not vary. Empirical data also support our assumption that f will not change under FR. Activity levels are typically measured by motion/activity counts per unit time, and studies have shown that FR elicits no change in this value (see review of empirical evidence in appendix A and table 1). FR animals expend the same multiple of their resting metabolic rate on activities as do their ad libitum counterparts, and f does not change under FR. In other words, if Brest is reduced by FR, Bact is also reduced, but the ratio of these two is assumed to remain constant.
Table 1.
Changes in mass-specific metabolic rate (B/M or B/M3/4), body temperature (Tb) and activity level in different species and strains under FR. (B is the resting or starving metabolic rate (unless noted otherwise); M is the adult body mass. The percentages in the columns of B/M and B/M3/4 are relative to data for ad libitum animals. The symbols =, ⇑ and ⇓ denote same as, higher than and lower than the ad libitum, respectively.)
| species | strain | FR level (%) | B/M | B/M3/4 | Tb drop (°C) | activity | source |
|---|---|---|---|---|---|---|---|
| chronic effects (stabilized) | |||||||
| mice | B10C3F1 | 50 | 2 | [19] | |||
| mice | C57BL/6 (B6) | 90 | 1.2 | [20] | |||
| mouse | SHN/C3HF1 | 50 | 2.2 | [21] | |||
| mice | CD2F1 | 75 | 1.5 | [22] | |||
| mouse | B6 | 40 | 1 | [23] | |||
| mouse | DBA/2 | 1.5 | [23] | ||||
| mice | B6C3F1 | 60 | 1.2 | 73% ⇑ | [24] | ||
| mice | QS | ⇑ | = | [25] | |||
| mice | golden spiny | 70 | 1.2–1.5 | slight⇑ | [26] | ||
| rat | Fishcher 344 | 50 | 1 | [27] | |||
| rat | Sprague-Dawley (S-D) | moderate | 10% | [28] | |||
| rat | S-D | severe | 17% | [28] | |||
| rat | F344 | 60 | = | = | [29] | ||
| rat | F344 | 60 | = | [30] | |||
| rat | Wistar | 50 | = | [31] | |||
| rat | FBNF1 | 60–70 | slightly⇓ | = | [32] | ||
| rat | F344 | 60 | 0.8 | slight⇑ | [24] | ||
| rat | S-D | 80 | = a | slight⇑ | [33] | ||
| rat | S-D | 70 | = a | = or⇓ | [33] | ||
| rat | S-D | 60 | 25%a | ⇑or⇓ | [33] | ||
| rat | 14%a | [34] | |||||
| rat | F344 | 60 | 12% | 9–10% | [35] | ||
| rat | 60 | slightly⇑ | = | [36] | |||
| rat | F344 | 60 | 0.9 | slight⇑ | [37] | ||
| Rhesus monkey | 11–16% | = | [38] | ||||
| Rhesus monkey | 70 | slight⇓ | [39] | ||||
| Rhesus monkey | 70 | 1.3% | [40] | ||||
| Rhesus monkey | 70 | 0.5 | [41] | ||||
| Rhesus monkey | 70 | = | [42] | ||||
| dog | 75 | = | [43] | ||||
| ewec | 50 | = | [44] | ||||
| broiler chicken | 50 | 10% and = b | [45] | ||||
| hen | Babcock B300 | 80 | 12% | [46] | |||
| hen | Warren SSL | 80 | 12% | [46] | |||
| hen | 80 | 10% | [47] | ||||
| cockerels | 80 | 22% | [47] | ||||
| short-term effects | |||||||
| mouse | B6 male | 50 | 4.5 | [21] | |||
| mouse | B6 female | 50 | 4.4 | [21] | |||
| mouse | SHN/C3HF1 | 50 | 3.3 | [21] | |||
| mice | B6C3F1 | 60 | 62% | 3.2 | 50% ⇑ | [24] | |
| rat | F344 | 60 | 39% | = | [29] | ||
| rat | Wistar | 70 | 6% | [48] | |||
| rat | Wistar | 16 | 5% | [48] | |||
| rat | S-D | 60 | 8%d | [49] | |||
| rat | Long-Evans | 60 | 15%d | [49] | |||
| rat | S-D | 50 | 8.20% | 15% ⇓ | [50] | ||
| rat | S-D | 35 | 18% | 20% ⇑ | [50] | ||
| rat | S-D | 65 | 3%e | [51] | |||
| rat | S-D | moderate | 6% | [28] | |||
| rat | S-D | severe | 13% | [28] | |||
| Rhesus monkey | 70 | 1 | [41] | ||||
aTotal energy expenditure rate.
b10% lower on day of age 10 (d10) but no change on d18, expressed as B/M0.67.
cNon-catheterized ewes; the mass-corrected metabolic rate, B/M3/4, drops 22% in catheterized ewes.
dAveraged over values during light and dark periods.
eAveraged over values on d10, d11 and d12.
However, there are some cases in which FR does slightly reduce animals' mass-corrected resting metabolic rates, expressed as either Brest/M or Brest/M3/4. In cases of severe restriction, FR animals can respond with mass-corrected metabolic rates that are as much as 15–20% lower than those of their ad libitum counterparts. As is often the case in biology, evidence to the contrary exists, and shows that the mass-corrected metabolic rates of FR animals do not differ from those of their ad libitum counterparts (appendix A and table 1). We take this drop in resting metabolic rate as evidence that the FR animals have a smaller normalization coefficient, B0,FR(=B/M3/4). Some empirical studies have also reported body temperature drops in FR cohorts, e.g. 1–2°C in mice, approximately 1°C in rats and 0.5°C in Rhesus monkeys (appendix A and table 1). Since B0 = b0 e−E0/(KT) and b0 is a fixed constant, independent of body mass and temperature, the drops in body temperature (TFR − T) and the reduction in mass-corrected metabolic rate (B0,FR/B0) can be reconciled by the B–A factor, B0,FR/B0 = e−E0/K/(TFR−T). For example, if B0 decreases by 15 per cent, the B–A factor predicts that the drop in body temperature is approximately 2°C, in agreement with most empirical observations. Our model will draw from multiple sources of empirical data, some of which report their results as changes in mass-corrected basal metabolic rates, and others report resultant data in terms of body temperature changes. Only B0 is used to account for metabolic rate in our model, so where source data are presented in terms of body temperature, we use the B–A factor to convert the value of temperature change to B0. Among 32 studies that reported the effect of long-term FR on metabolic rate or body temperature, the mean value of B0 reduction is 9.6 per cent (s.d. = 7.7%). The drop in B0 (ΔB0) is not correlated to the degree of FR (β), ΔB0 = 0.08 − 0.02β (n = 32, r2 = 0.03, p = 0.86; data listed in table 1).
Accounting for altered (B0,FR) and unaltered (f, EC, Em) parameters, equation (1.1) for an FR animal becomes:
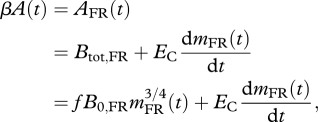 |
1.4 |
where mFR(t) is the body mass of FR animals during growth. Recalling that the assimilation rate of ad libitum animals, A(t), is determined by its growth via equation (1.3), we can substitute equation (1.3) into equation (1.4) to yield:
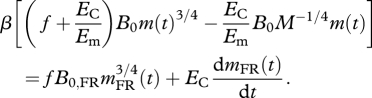 |
1.5 |
Equation (1.5) is the major result of this model. We now make several predictions based on equation (1.5).
Prediction 1: the adult mass of FR animals, MFR. When both FR and ad libitum animals stop growing, i.e. m(t) = M, dmFR/dt = 0, and mFR(t) = MFR, equation (1.5) reduces to MFR = M × (β × B0/B0,FR)4/3.
Prediction 2: the growth curve of FR animals, mFR(t). Equation (1.5) establishes the relationship between m(t) and mFR(t). If the species-specific growth parameters of the ad libitum animals are known empirically, namely: initial mass, m0; adult mass, M; and energy required to synthesize biomass, Em; then the growth curve of the ad libitum animal, m(t), can be expressed by the solution of equation (1.1), m(t) = (1 − [1 − (m0/M)1/4]e−B0t/4EmM1/4)4M [14]. Since we assume that f, Em and EC should not change between FR and ad libitum animals, substituting m(t) into equation (1.5) and numerically solving it will produce the growth curve of an FR animal, mFR(t), without any extra free-adjusting parameters. The only empirical inputs required to solve equation (1.5) for mFR(t) are the degree of FR, β and B0,FR; the latter of which can be calculated, if necessary, from the reduction in body temperature using the B–A factor as discussed.
Prediction 3: we predict that FR and ad libitum animals reach the same fraction of their adult mass at the same age, m(τ)/M = mFR(τ)/MFR, where τ is the age of the animals after a transient period. It is commonly thought that FR slows growth rate and delays the age associated with certain development stages, such as certain fraction of adult mass and puberty (e.g. [52,53]). However, theoretical consideration of our model and analyses of empirical data suggest that this is only true under certain FR conditions. If FR starts early in life and β, the fraction of ad libitum food given to FR animals, is kept constant, then equation (1.5) predicts that after the transient period, during which FR causes negative growth, the FR and ad libitum animals will reach the same percentage of their adult mass at the same age, i.e. m(τ)/M = mFR (τ)/MFR.We present the detailed proof below.
During the transient period FR animals experience negative growth; they lose body mass immediately following the initiation of FR. The negative growth is predicted by equation (1.5) based on the same assumption introduced in predictions 1 and 2, i.e. parameters f, Em and EC are the same for FR animals and for ad libitum animals; and changes in body temperature (and therefore B0) are constant during entire period of FR. After the transient period, growth rates can be expressed as dmFR (t)/dt = B0,FR mFR(t)3/4 − Bm,FRmFR(t), which is the analogue of equation (1.1) for FR animals. When growth stops, this yields 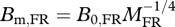 . Substituting both equations into equation (1.5) gives:
. Substituting both equations into equation (1.5) gives:
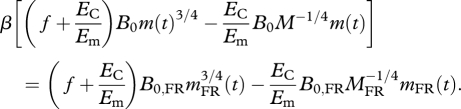 |
Letting f + EC/Em = a and EC/Em = b, yields 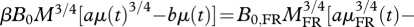
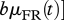 , where μ (t) = m(t)/M is the relative mass of ad libitum animals, and μFR(t) = mFR(t)/MFR is the relative mass for FR animals. Using MFR = M × (β × B0/B0,FR)4/3 we have:
, where μ (t) = m(t)/M is the relative mass of ad libitum animals, and μFR(t) = mFR(t)/MFR is the relative mass for FR animals. Using MFR = M × (β × B0/B0,FR)4/3 we have:
| 1.6 |
Now, let us assume that there exists a time T, such that μ(T) ≠ μFR(T). Since both μ(t) and μFR(t) monotonically increase from 0 to 1, 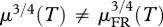 , and therefore,
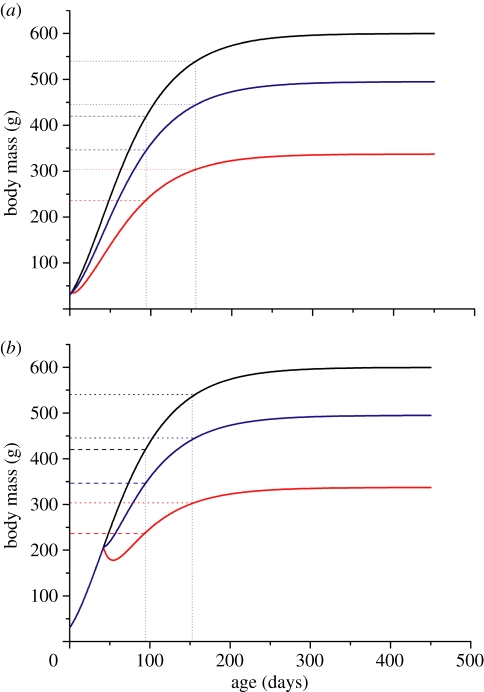
, and therefore, 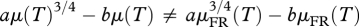 . This is in contradiction to equation (1.6), which holds for all times, t, after the transient period. So, for any time, t, after the transient period, it will be true that μ(t) = μFR(t), regardless of the degree of FR or the age at which FR initiates. In figure 1, we illustrate this relationship.
. This is in contradiction to equation (1.6), which holds for all times, t, after the transient period. So, for any time, t, after the transient period, it will be true that μ(t) = μFR(t), regardless of the degree of FR or the age at which FR initiates. In figure 1, we illustrate this relationship.
Figure 1.
Theoretically, FR and ad libitum animals reach the same fraction of adult mass at the same age. (a) FR starts on day 2 of age; (b) FR starts on day 42 of age. Dotted lines, 90% of adult body mass; dashed lines, 70% of adult body mass; black line, ad libitum fed; blue line, 80% FR; red line, 60% FR.
2. Results and discussion
We evaluate predictions using laboratory and field data for the growth of FR and ad libitum animals.
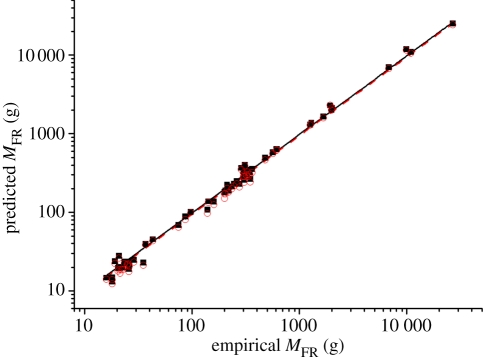
In figure 2, we plot predicted values (black squares) against empirical values of adult mass for FR animals, MFR, derived from 62 studies of mammals and birds across a broad range of body sizes and taxa, including rodents, monkeys, dogs, chickens and quails. Empirical data strongly support our first prediction MFR = M × (β × B0/B0,FR)4/3; predictions plotted against empirical data have a line of best fit (solid black) with a slope of 0.98 and include the predicted value of 1. Our predictions are based on the scaling power of resting metabolic rate over ontogeny, 3/4, which has been used in allometric theories and supported by data on a diverse set of animals, including mammals, birds and fishes [15–17,54]. In figure 2, we also show that if the scaling power is taken to be 2/3 instead of 3/4, our predictions of MFR (red circles) would deviate only slightly from the empirical values. The slope of the line (red dashed) is 0.94, which indicates that a 2/3 scaling power underestimates the adult mass of FR animals. The confidence interval for this slope does not include the predicted value of 1. In appendix B, we show that the prediction of MFR is not very sensitive to the scaling power; varying scaling power from 0.65 to 0.85 generates 0.7–9% variation in MFR from this prediction.
Figure 2.
Predicted values of ultimate body mass of FR animals, MFR = M × (β × B0/B0,FR)4/3, against the empirical values. (Empirical data are in table S1 of the electronic supplementary material.) Black filled squares, predictions based on scaling power = 3/4; solid line, y = 0.98x (fixed intercept = 0; r2 = 0.99; 95% CI: 0.96, 1); red open circles, predictions based on scaling power = 2/3; dashed line, y = 0.94x (fixed intercept = 0; r2 = 0.99; 95% CI: 0.92, 0.97).
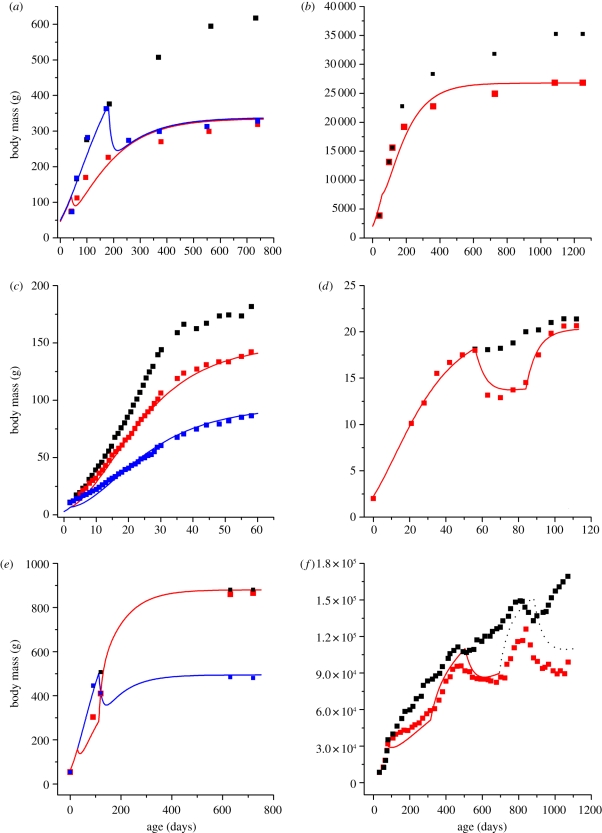
In figure 3, we plot predicted and empirical growth curves for the FR animals, mFR(t). We first fit the empirical growth curves of ad libitum animals to the solution of equation (1.2), in order to obtain the species-specific growth parameters; m0, M and Em; and the analytic expression of m(t). The values of fitted parameters and statistics are listed in the electronic supplementary material, table S2. We then substituted m(t) into equation (1.5), assuming that the combustion energy in one unit of biomass, EC, is constant within an individual and across mammalian and bird species with a value of roughly 7000 J g−1 [55,56]. Solving equation (1.5) numerically with a defined level of FR, β and known values of B0, we determine the growth curves of FR animals, mFR(t). So, the predicted mFR(t) in figure 3 were not obtained by fitting the empirical FR data. The model successfully predicts how FR affects growth for different mammals and birds of diverse body sizes. Predicted and empirical data have strong linear relationships; the slopes and r2 values are nearly identical to 1, and p < 10−5 (detailed statistics are shown in the electronic supplementary material, table S3). More importantly, our model predicts growth curves under different FR conditions, e.g. different ages at which FR starts (figure 3a,e); different levels of FR, β (figure 3c,d); and alternations between FR and ad libitum (figure 3d–f).
Figure 3.
Empirical (dots) and predicted (solid lines) growth curves of ad libitum and FR animals. In (f), the accuracy of the prediction is lost after day ca 700 of age. This is because both ad libitum and FR deer stags had the rut, which causes irregular food intake and body mass change (empirical data and statistics are in table S2, electronic supplementary material). (a) Rat: black squares, ad libitum fed; red squares, 60% FR from day 42; blue squares, 60% FR from day 180; (b) dog: black squares, ad libitum fed; red squares, 75% FR from day 56; (c) quail: black squares, ad libitum fed; red squares, 70% FR from day 2; blue squares, 40% FR from day 2; (d) mouse: black squares, ad libitum fed; red squares, 67% FR from day 56 then switched to 95% FR from day 84; (e) rat: black squares, ad libitum fed; blue squares, ad libitum fed for 12 week after weaning then 60% FR; red squares, 60% FR for 12 week after weaning then ad libitum; (f) red deer: black squares, ad libitum fed; red squares, 70% FR and refeeding; dotted line, accuracy lost due to rut.
Body temperature plays an important role throughout ontogenesis. The effects of variable body temperature on growth have been studied extensively in ectotherms (e.g. [57]). However, mammals, especially small rodents, also vary their body temperatures over ontogeny in response to FR (e.g. [58] and review in appendix A and table 1). Figure 3 shows predictions based on constant body temperature throughout the entire period of FR. Empirical evidence has shown that in many cases, body temperature drops severely after implementation of FR, and after a transient period it increases to a stabilized level (e.g. [29]). One study on two strains of mice [21] reported growth curves and temperature drops at different ages under FR. We take the reported, variable temperature drops to predict the growth curves of FR mice (figure 4). Our model predicts that under FR, lower body temperature, meaning lower B0,FR by virtue of the B–A factor, B0 = b0 e−E0/KT, leads to a relatively larger body size (MFR = M × (β × B0/B0,FR)4/3 and figure 4). Equation (1.1) gives a quantitative explanation. The rate of new biomass storage (growth) is the difference between food intake rate, A, and metabolic rate, B, which increases with body temperature. When A is restricted during FR, lower temperatures lead to lowered metabolic rates, therefore, leaving a relatively larger amount of energy to be allocated to growth.
Figure 4.
Predicted growth curves with accurate variable temperature (solid lines) drops during whole periods of FR. (a) B6 mice; (b) SHN/C3H mice. Dashed lines indicate the predictions with constant, stabilized temperature drops. For FR mice in this study [21], the body temperature changed from T1 at age d1 to T2 at age d2 (T1 < T2 and d1 < d2). The body temperature of ad libitum mice is roughly a constant, TAL. We assume that the temperature of FR mice is a function of time, and increases smoothly from T1 at age dm, which is between d1 and d2, to reachT2 at age d2. The function we took for the calculation has the form (T2 − T1) × [1 − e−a(t−dm)], where a is a dimensionless constant that controls the rate of increase. Note: any function that smoothly increases from T1 to T2 would give a similar result. The values of T1, T2, d1, d2, dm and a are listed in table 2. The growth curves of FR animals are obtained by numerically solving equation (1.5). This was completed using the B–A factor to determine B0 from temperature data.
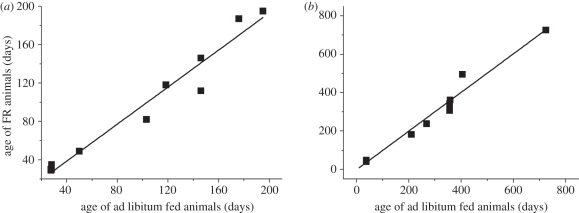
To test our third prediction, we plotted in figure 5 the ages of 11 FR animals against those of their ad libitum counterparts, at which 70 per cent (figure 5a) and 90 per cent (figure 5b) of the adult body masses were reached. Our model's prediction is well supported by empirical data. Life-history theories suggest that mammals and birds need to reach a critical fraction of adult mass for sexual maturity [59–61]. So, minimizing the time to reach the fraction of adult mass associated with reproductive maturity will maximize the animals' fitness. Theoretical predictions by our model and empirical data shown in figure 5 illustrate the finding that, despite the stress of FR, FR animals with a constant β reach the same fraction of adult mass at the same age as their ad libitum counterparts. Many studies reported that FR delays puberty (e.g. [62,63]), but in most of those studies FR was not set as a constant fraction of the amount of food that ad libitum animals obtain, which is the condition of our prediction. By contrast, empirical evidence shows that rats [64] and quails [65] under FR with constant β reach puberty at the same age as their ad libitum fed counterparts, in agreement with our theoretical prediction.
Table 2.
Parameters for predicting growth curves in figure 3.
| strain | T1 (°C) | T2 (°C) | d1 | d2 | dm | ab |
|---|---|---|---|---|---|---|
| B6 (FR) | 33.2 | 35.3a | day 90 | day 390 | day 150 | 0.025 |
| B6 ad libitum | 37.7 | 37.7 | ||||
| SHN/C3H (FR) | 34.1 | 34.8 | day 90 | day 390 | day 210 | 0.025 |
| SHN/C3H ad libitum | 37.2 | 37.2 |
aAt d2 (day 390), the body temperature drop in SHN/C3H mice is 2.4°C. The authors did not report T2 at d2 for B6 mice. We assume the same drop (2.4°C) for B6 mice at age d2.
bWe set the value of a to be 0.025 so that the temperature smoothly increases from Tm to T2 at an appropriate rate. A too large/small a will make the temperature increase too fast/slow and reach to T2 too much before/after age d2.
Figure 5.
The age of FR animals against the age of ad libitum counterparts at which they reach to the same percentage of the adult masses, M and MFR. (a) Age at 70% of adult body mass (solid line, y = 0.97x (fixed intercept = 0; r2 = 0.99 95% CI: 0.89, 1.04)); (b) age at 90% of adult body mass (solid line, y = 1.00x (fixed intercept = 0; r2 = 0.99 95% CI: 0.93, 1.08)). The points are derived from empirical studies instead of predictive curves (empirical data are in tables S4 and S5, electronic supplementary material). Where the exact value, i.e. exact 70 or 90% of M or MFR was not available, we used the closest value having error less than 4%. Dashed line, y = 0.97x (fixed intercept = 0; r2 = 0.99% CI: 0.89, 1.04).
One of the fundamental issues in ontogenetic growth is whether growth is constrained by food intake or metabolism [66]. Together with previous ontogenetic growth models [11,14], the model presented here illustrates that both provide constraints on growth. When available food is unlimited, metabolic rate is the dominant influence on growth, and is positively correlated with the growth rate. Under FR, however, food intake has more influence on growth, and is positively correlated to growth. More importantly, under FR, owing to the trade-off between metabolism and new biomass storage, higher metabolism leads to slower growth. This negative correlation has been reported in experiments on rats, in which food was restricted and elevated metabolic rates were found to be associated with severely reduced growth [67].
In summary, we have derived a general quantitative model for understanding growth under FR, which is based on the first principles of energy balance and allometries of metabolism. This model predicts growth curves under different conditions of FR (figures 2 and 3), and explores the effects of body temperature and metabolic rate on growth (figure 4). The model also predicts that animals reach the same fraction of their adult mass at the same age, regardless of whether they endure FR or are allowed to eat ad libitum (figures 1 and 5). In its general form, this model contributes to our current understanding of the pattern of energy budgeting under FR. In addition, it presents a conceptual framework from which more detailed, species- or strain-specific studies may be possible. The model partitions the metabolic rate between the rate of energy allocated to growth and the rate of energy allocated to maintenance of the existing biomass (equations (1.2) and (1.5)). Since FR greatly suppresses growth but only slightly reduces mass-specific metabolism, it channels extra energy for mass-specific maintenance. Therefore, this model offers a departure point for quantitatively understanding how FR enhances organisms' maintenance functions. From an energetic point of view, this enhancement in maintenance provides a feasible and quantifiable explanation for the phenomenon of lifespan extension that has been observed in food-restricted animals [7,8].
Acknowledgements
This work has been supported by grants from the Ellison Medical Foundation Senior Scholar Award AG-SS-2235, and NIH grants R01-AG028872 and P01-AG027734. We gratefully acknowledge the careful reviews and excellent suggestions of two anonymous reviewers of earlier versions of this manuscript.
Appendix A. Body temperature, metabolic rate and activity level under food restriction
Numerous empirical studies of FR in mammals, such as rodents, ewes, dogs, and primates; and on birds, such as quail and chicken; have shown that the mass-specific metabolic rates of FR animals, expressed per gram of body mass or per gram of body mass to 3/4 power (metabolic mass), either decreases slightly or sometimes remains roughly the same as those of their ad libitum fed counterparts [25,26,28,29–31,33,36,37,43,44,46,47,68–69]. Under severe FR (50 or 60%), the mass-specific metabolic rates may drop up to 15–20% in some cases [28,33–35,40], although in one case, rates showed an increase in severely FR animals [25]. Studies have also shown that animals under FR keep the same or even slightly increased activity levels [25,26,33,38,42,70,71]. Only one study reports increased activity levels as pronounced as 50–70% above normal [24].
Some empirical studies have reported slight body temperature drops, e.g. approximately 1°C for rats [24,27,37], 1–2°C for mice [19,22–24,26,58,72] (but up to 4°C for a few strains [58]), and 0.5–1°C for Rhesus monkeys [41]. Studies have also reported that drops in body temperatures and metabolic rates are more severe immediately after FR starts [21,24,29,41], but one study showed the opposite result [28]. We summarize the reported changes in mass-specific metabolic rates, body temperatures and activity levels of different species and strains under FR in table 1.
Appendix B. Sensitivity of MFR Estimates to the value of metabolic rate scaling power
Our estimate of MFR depends on the value of the scaling power of the metabolic rate. In general, if the power is α, the predicted value of MFR from equation (1.5) would be MFR = M(β × B0/BFR)1/α, where M, β, B0 and BFR are empirically determined. We now show how deviation from α = 3/4 affect our prediction of MFR.
First, we take the natural logarithm of both sides of the prediction of MFR, giving ln MFR = ln M + 1/α ln(β × B0/BFR). Then, we take the derivative of ln(MFR) with respect to α, and get d ln MFR/dα = −ln(β × B0/B0,FR)/α2. Since d ln MFR = dMFR/MFR = ΔMFR/MFR, then ΔMFR/MFR = −Δα × ln(β × B0/B0,FR)/α2. The value of β ranges from 0.6 to 0.8, and the ratio of B0/B0,FR ranges from 1 to 1.2, so the value of ln(β × B0/BFR) varies from −0.5 to −0.04. In the main text, we assume α = 3/4. If for some particular species the empirical value of α = 0.65 or 0.85, i.e. Δα = −0.1 or 0.1, then ΔMFR/MFR = ∓ (0.007–0.089), so the value of MFR will be 0.7–9% lower or greater than our estimate based on the 3/4 power.
References
- 1.Schoener T. W. 1971. Theory of feeding strategies. Annu. Rev. Ecol. Syst. 2, 369–404 10.1146/annurev.es.02.110171.002101 (doi:10.1146/annurev.es.02.110171.002101) [DOI] [Google Scholar]
- 2.Roff D. A. 2001. Life history evolution. Sunderland, MA: Sinauer Associates [Google Scholar]
- 3.Stearns S. C. 1992. The evolution of life histories. Oxford, UK: Oxford University Press [Google Scholar]
- 4.Kooijman S. A. L. M. 2000. Dynamic energy and mass budgets in biological systems. Cambridge, UK: Cambridge University Press [Google Scholar]
- 5.Hocking P. M. 1992. Effects of photostimulation at 18 weeks, 24 weeks and 30 weeks of age on the productivity of female turkeys fed ad libitum or restricted until point of lay. Br. Poultry Sci. 33, 253–269 10.1080/00071669208417464 (doi:10.1080/00071669208417464) [DOI] [PubMed] [Google Scholar]
- 6.Ottinger M. A., Mobarak M., Abdelnabi M., Roth G., Proudman J., Ingram D. K. 2005. Effects of calorie restriction on reproductive and adrenal systems in Japanese quail: are responses similar to mammals, particularly primates? Mech. Ageing Dev. 126, 967–975 10.1016/j.mad.2005.03.017 (doi:10.1016/j.mad.2005.03.017) [DOI] [PubMed] [Google Scholar]
- 7.Weindruch R., Walford R. L. 1988. The retardation of aging and disease by dietary restriction. Springfield, IL: Thomas [Google Scholar]
- 8.Masoro E. J. 2005. Overview of caloric restriction and ageing. Mech. Ageing Dev. 126, 913–922 10.1016/j.mad.2005.03.012 (doi:10.1016/j.mad.2005.03.012) [DOI] [PubMed] [Google Scholar]
- 9.Kirkwood T. B. L., Shanley D. P. 2005. Food restriction, evolution and ageing. Mech. Ageing Dev. 126, 1011–1016 10.1016/j.mad.2005.03.021 (doi:10.1016/j.mad.2005.03.021) [DOI] [PubMed] [Google Scholar]
- 10.McCay C. M., Crowell M. F., Maynard L. A. 1935. The effect of retarded growth upon the length of the lifespan and upon the ultimate body size. J. Nutr. 10, 63–79 [PubMed] [Google Scholar]
- 11.Hou C., Zuo W., Moses M. E., Woodruff W. H., Brown J. H., West G. B. 2008. Energy uptake and allocation during ontogeny. Science 322, 736–739 10.1126/science.1162302 (doi:10.1126/science.1162302) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 12.Speakman J. R. 2005. Body size, energy metabolism and lifespan. J. Exp. Biol. 208, 1717–1730 10.1242/jeb.01556 (doi:10.1242/jeb.01556) [DOI] [PubMed] [Google Scholar]
- 13.Nagy K. A., Girard I. A., Brown T. K. 1999. Energetics of free-ranging mammals, reptiles, and birds. Annu. Rev. Nutr. 19, 247–277 10.1146/annurev.nutr.19.1.247 (doi:10.1146/annurev.nutr.19.1.247) [DOI] [PubMed] [Google Scholar]
- 14.West G. B., Brown J. H., Enquist B. J. 2001. A general model for ontogenetic growth. Nature 413, 628–631 10.1038/35098076 (doi:10.1038/35098076) [DOI] [PubMed] [Google Scholar]
- 15.Zuo W., Moses M. E., Hou C., Woodruff W. H., West G. B., Brown J. H. 2009. Response to comments on ‘energy uptake and allocation during ontogeny’. Science 325, 1206. 10.1126/science.1171949 (doi:10.1126/science.1171949) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 16.Moses M. E., Hou C., Woodruff W. H., West G. B., Nekola J. C., Zuo W., Brown J. H. 2008. Revisiting a model of ontogenetic growth: estimating model parameters from theory and data. Am. Nat. 171, 632–645 10.1086/587073 (doi:10.1086/587073) [DOI] [PubMed] [Google Scholar]
- 17.Brody S. 1964. Bioenergetics and growth. Darien, CT: Hafner [Google Scholar]
- 18.Gillooly J. F., Brown J. H., West G. B., Savage V. M., Charnov E. L. 2001. Effects of size and temperature on metabolic rate. Science 293, 2248–2251 10.1126/science.1061967 (doi:10.1126/science.1061967) [DOI] [PubMed] [Google Scholar]
- 19.Weindruch R. H., Kristie J. A., Cheney K. E., Walford R. L. 1979. Influence of controlled dietary restriction on immunologic function and aging. Fed. Proc. 38, 2007–2016 [PubMed] [Google Scholar]
- 20.Leto S., Kokkonen G. C., Barrows C. H. J. 1976. Dietary protein, life-span, and physiological variables in female mice. J. Gerontol. 31, 149–154 [DOI] [PubMed] [Google Scholar]
- 21.Koizumi A., Tsukada M., Wada Y., Masuda H., Weindruch R. 1992. Mitotic activity in mice is suppressed by energy restriction-induced torpor. J. Nutr. 122, 1446–1453 [DOI] [PubMed] [Google Scholar]
- 22.Nelson W., Halberg F. 1986. Meal-timing, circadian rhythms and life span of mice. J. Nutr. 116, 2244–2253 [DOI] [PubMed] [Google Scholar]
- 23.Ferguson M., Sohal B. H., Forster M. J., Sohal R. S. 2007. Effect of long-term caloric restriction on oxygen consumption and body temperature in two different strains of mice. Mech. Ageing Dev. 128, 539–545 10.1016/j.mad.2007.07.005 (doi:10.1016/j.mad.2007.07.005) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 24.Duffy P. H., Leakey J. E. A., Pipkin J. L., Turturro A., Hart R. W. 1997. The physiologic, neurologic, and behavioral effects of caloric restriction related to aging, disease, and environmental factors. Environ. Res. 73, 242–248 10.1006/enrs.1997.3714 (doi:10.1006/enrs.1997.3714) [DOI] [PubMed] [Google Scholar]
- 25.Faulks S. C., Turner N., Else P. L., Hulbert A. J. 2006. Calorie restriction in mice: effects on body composition, daily activity, metabolic rate, mitochondrial reactive oxygen species production, and membrane fatty acid composition. J. Gerontol. A. Biol. Sci. Med. Sci. 61, 781–794 [DOI] [PubMed] [Google Scholar]
- 26.Ehrhardt N., Heldmaier G., Exner C. 2005. Adaptive mechanisms during food restriction in Acomys russatus: the use of torpor for desert survival. J. Comp. Physiol. B Biochem. Syst. Environ. Physiol. 175, 193–200 10.1007/s00360-005-0475-3 (doi:10.1007/s00360-005-0475-3) [DOI] [PubMed] [Google Scholar]
- 27.Jin Y. H., Koizumi A. 1994. Decreased cellular proliferation by energy restriction is recovered by increasing housing temperature in rats. Mech. Ageing Dev. 75, 59–67 10.1016/0047-6374(94)90028-0 (doi:10.1016/0047-6374(94)90028-0) [DOI] [PubMed] [Google Scholar]
- 28.Ballor D. L. 1991. Effect of dietary restriction and or exercise on 23-H metabolic-rate and body-composition in female rats. J. Appl. Physiol. 71, 801–806 [DOI] [PubMed] [Google Scholar]
- 29.McCarter R. J., McGee J. R. 1989. Transient reduction of metabolic rate by food restriction. Am. J. Physiol. 257, E175–E179 [DOI] [PubMed] [Google Scholar]
- 30.McCarter R., Palmer J. 1992. Energy metabolism and aging: a lifelong study of Fischer 344 rats. Endocrinol. Metab. 26, E448–E452 [DOI] [PubMed] [Google Scholar]
- 31.Mohan P. F., Rao B. S. N. 1985. Adaptation to underfeeding in young growing-rats. Nutr. Res. 5, 1409–1418 10.1016/S0271-5317(85)80051-8 (doi:10.1016/S0271-5317(85)80051-8) [DOI] [Google Scholar]
- 32.Evans S. A., Parsons A. D., Overton J. M. 2005. Homeostatic responses to caloric restriction: influence of background metabolic rate. J. Appl. Physiol. 99, 1336–1342 10.1152/japplphysiol.01380.2004 (doi:10.1152/japplphysiol.01380.2004) [DOI] [PubMed] [Google Scholar]
- 33.Rising R., Lifshitz F. 2006. Energy expenditures and physical activity in rats with chronic suboptimal nutrition. Nutr. Metab. 3, 11. 10.1186/1743-7075-3-11 (doi:10.1186/1743-7075-3-11) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 34.Dulloo A. G., Girardier L. 1993. 24 Hour energy-expenditure several months after weight-loss in the underfed rat: evidence for a chronic increase in whole-body metabolic efficiency. Int. J. Obes. 17, 115–123 [PubMed] [Google Scholar]
- 35.Gonzales Pacheco D. M., Buss W. C., Koehler K. M., Woodside W. F., Alpert S. S. 1993. Energy restriction reduces metabolic-rate in adult male Fisher-344 rats. J. Nutr. 123, 90–97 [DOI] [PubMed] [Google Scholar]
- 36.McCarter R., Masoro E. J., Yu B. P. 1985. Does food restriction retard aging by reducing the metabolic rate? Am. J. Physiol. 248, E488–E490 [DOI] [PubMed] [Google Scholar]
- 37.Duffy P. H., Feuers R. J., Leakey J. A., Nakamura K. D., Turturro A., Hart R. W. 1989. Effect of chronic caloric restriction on physiological variables related to energy-metabolism in the male Fischer-344 rat. Mech. Ageing Dev. 48, 117–133 10.1016/0047-6374(89)90044-4 (doi:10.1016/0047-6374(89)90044-4) [DOI] [PubMed] [Google Scholar]
- 38.Ramsey J. J., Roecker E. B., Weindruch R., Kemnitz J. W. 1997. Energy expenditure of adult male Rhesus monkeys during the first 30 mo of dietary restriction. Am. J. Physiol. Endocrinol. Metab. 35, E901–E907 [DOI] [PubMed] [Google Scholar]
- 39.Moscrip T. D., Ingram D. K., Lane M. A., Roth G. S., Weed J. L. 2000. Locomotor activity in female Rhesus monkeys: assessment of age and calorie restriction effects. J. Gerontol. A. Biol. Sci. Med. Sci. 55, B373–B380 [DOI] [PubMed] [Google Scholar]
- 40.Blanc S., Schoeller D., Kemnitz J., Weindruch R., Colman R., Newton W., Wink K., Baum S., Ramsey J. 2003. Energy expenditure of Rhesus monkeys subjected to 11 years of dietary restriction. J. Clin. Endocrinol. Metab. 88, 16–23 10.1210/jc.2002-020405 (doi:10.1210/jc.2002-020405) [DOI] [PubMed] [Google Scholar]
- 41.Lane M. A., Baer D. J., Rumpler W. V., Weindruch R., Ingram D. K., Tilmont E. M., Cutler R. G., Roth G. S. 1996. Calorie restriction lowers body temperature in Rhesus monkeys, consistent with a postulated anti-aging mechanism in rodents. Proc. Natl Acad. Sci. USA 93, 4159–4164 10.1073/pnas.93.9.4159 (doi:10.1073/pnas.93.9.4159) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 42.Weed J. L., Lane M. A., Roth G. S., Speer D. L., Ingram D. K. 1997. Activity measures in Rhesus monkeys on long-term calorie restriction. Physiol. Behav. 62, 97–103 10.1016/S0031-9384(97)00147-9 (doi:10.1016/S0031-9384(97)00147-9) [DOI] [PubMed] [Google Scholar]
- 43.Lawler D. F., et al. 2008. Diet restriction and ageing in the dog: major observations over two decades. Br. J. Nutr. 99, 793–805 10.1017/S0007114507871686 (doi:10.1017/S0007114507871686) [DOI] [PubMed] [Google Scholar]
- 44.Ortigues I., Durand D. 1995. Adaptation of energy-metabolism to undernutrition in ewes. Contribution of portal-drained viscera, liver and hindquarters. Br. J. Nutr. 73, 209–226 10.1079/BJN19950024 (doi:10.1079/BJN19950024) [DOI] [PubMed] [Google Scholar]
- 45.Zubair A. K., Leeson S. 1996. Compensatory growth in the broiler chicken: a review. Worlds Poultry Sci. J. 52, 189–201 10.1079/WPS19960015 (doi:10.1079/WPS19960015) [DOI] [PubMed] [Google Scholar]
- 46.MacLeod M. G., Shannon D. W. F. 1978. Effects of food intake regulation on the energy metabolism of laying hens. Br. Poult. Sci. 19, 349–363 10.1080/00071667808416487 (doi:10.1080/00071667808416487) [DOI] [PubMed] [Google Scholar]
- 47.MacLeod M. G., Tullett S. G., Jewitt T. R. 1979. Effects of food intake regulation on the energy metabolism of hens and cockerels of a layer strain. Br. J. Nutr. 20, 521–531 [DOI] [PubMed] [Google Scholar]
- 48.Even P. C., Nicolaidis S. 1993. Adaptive-changes in energy-expenditure during mild and severe feed restriction in the rat. Br. J. Nutr. 70, 421–431 10.1079/BJN19930136 (doi:10.1079/BJN19930136) [DOI] [PubMed] [Google Scholar]
- 49.Evans S. A., Messina M. M., Knight W. D., Parsons A. D., Overton J. M. 2005. Long-Evans and Sprague-Dawley rats exhibit divergent responses to refeeding after caloric restriction. Am. J. Physiol. Regul. Integr. Comp. Physiol. 288, R1468–R1476 10.1152/ajpregu.00602.2004 (doi:10.1152/ajpregu.00602.2004) [DOI] [PubMed] [Google Scholar]
- 50.Boyle P. C., Storlien L. H., Harper A. E., Keesey R. E. 1981. Oxygen consumption and locomotor activity during restricted feeding and realimentation. Am. J. Physiol. Regul. Integr. Comp. Physiol. 241, 392–397 [DOI] [PubMed] [Google Scholar]
- 51.Rothwell N. J., Stock M. J. 1982. Effect of chronic food restriction on energy balance, thermogenic capacity, and brown adipose tissue activity in the rat. Biosci. Rep. 2, 543–549 10.1007/BF01314214 (doi:10.1007/BF01314214) [DOI] [PubMed] [Google Scholar]
- 52.Kirkwood R. N., Cumming D. C., Aherne F. X. 1987. Nutrition and puberty in the female. P. Nutr. Soc. 46, 177–192 [DOI] [PubMed] [Google Scholar]
- 53.Robinson J. J. 1990. Nutrition in the reproduction of farm animals. Nutr. Res. Rev. 3, 253–276 10.1079/NRR19900015 (doi:10.1079/NRR19900015) [DOI] [PubMed] [Google Scholar]
- 54.Brown J. H., Gillooly J. F., Allen A. P., Savage V. M., West G. B. 2004. Toward a metabolic theory of ecology. Ecology 85, 1771–1789 10.1890/03-9000 (doi:10.1890/03-9000) [DOI] [Google Scholar]
- 55.Cummins K. W., Wuycheck J. C. 1971. Calorific equivalents for investigations in ecological energetics. Mitt int Verein theor angew Limnol 18, 1–158 [Google Scholar]
- 56.Robbins C. T. 1983. Wildlife feeding and nutrition. New York, NY: Academic Press [Google Scholar]
- 57.Atkinson D. 1994. Temperature and organism size: a biological law for ectotherms. Adv. Ecol. Res. 25, 1–58 10.1016/S0065-2504(08)60212-3 (doi:10.1016/S0065-2504(08)60212-3) [DOI] [Google Scholar]
- 58.Rikke B. A., Yerg J. E., III, Battaglia M. E., Nagy T. R., Allison D. B., Johnson T. E. 2003. Strain variation in the response of body temperature to dietary restriction. Mech. Ageing Dev. 124, 663–678 10.1016/S0047-6374(03)00003-4 (doi:10.1016/S0047-6374(03)00003-4) [DOI] [PubMed] [Google Scholar]
- 59.Charnov E. L. 2001. Evolution of mammal life histories. Evol. Ecol. Res. 3, 521–535 [Google Scholar]
- 60.Peters R. H. 1986. The ecological implications of body size. New York, NY: Cambridge University Press [Google Scholar]
- 61.Taylor S. C. S. 1968. Time taken to mature in relation to mature weight for sexes, strains and species of domesticated mammals and birds. Anim. Prod. 10, 157–169 10.1017/S0003356100026106 (doi:10.1017/S0003356100026106) [DOI] [Google Scholar]
- 62.Merry B. J., Holehan A. M. 1979. Onset of puberty and duration of fertility in rats fed a restricted diet. J. Reprod. Fertil. 57, 253–259 10.1530/jrf.0.0570253 (doi:10.1530/jrf.0.0570253) [DOI] [PubMed] [Google Scholar]
- 63.Glass A. R., Harrison R., Swerdloff R. S. 1976. Effect of undernutrition and amino acid deficiency on the timing of puberty in rats. Pediatr. Res. 10, 951–952 10.1203/00006450-197611000-00009 (doi:10.1203/00006450-197611000-00009) [DOI] [PubMed] [Google Scholar]
- 64.Engelbregt M. J. T., Houdijk M. E. C. A. M., Popp-Snijders C., Delemarre-Van de Waal H. A. 2000. The effects of intra-uterine growth retardation and postnatal undernutrition on onset of puberty in male and female rats. Pediatr. Res. 48, 803–807 10.1203/00006450-200012000-00017 (doi:10.1203/00006450-200012000-00017) [DOI] [PubMed] [Google Scholar]
- 65.Hassan S. M., Mady M. E., Cartwright A. L., Sabri H. M., Mobarak M. S. 2003. Effect of early feed restriction on reproductive performance in Japanese quail (Coturnix coturnix japonica). Poultry Sci. 82, 1163–1169 [DOI] [PubMed] [Google Scholar]
- 66.Ricklefs R. E. 2003. Is rate of ontogenetic growth constrained by resource supply or tissue growth potential? A comment on West et al's model. Funct. Ecol. 17, 384–393 10.1046/j.1365-2435.2003.00745.x (doi:10.1046/j.1365-2435.2003.00745.x) [DOI] [Google Scholar]
- 67.Derting T. L. 1989. Metabolism and food availability as regulators of production in juvenile cotton rats. Ecology 70, 587–595 10.2307/1940210 (doi:10.2307/1940210) [DOI] [Google Scholar]
- 68.Ramsey J. J., Harper M. E., Weindruch R. 2000. Restriction of energy intake, energy expenditure, and aging. Free Radic. Biol. Med. 29, 946–968 10.1016/S0891-5849(00)00417-2 (doi:10.1016/S0891-5849(00)00417-2) [DOI] [PubMed] [Google Scholar]
- 69.Van der Ziel C. E., Visser G. H. 2001. The effect of food restriction on morphological and metabolic development in two lines of growing Japanese quail chicks. Physiol. Biochem. Zool. 74, 52–65 10.1086/319314 (doi:10.1086/319314) [DOI] [PubMed] [Google Scholar]
- 70.DeLany J. P., Hansen B. C., Bodkin N. L., Hannah J., Bray G. A. 1999. Long-term calorie restriction reduces energy expenditure in aging monkeys. J. Gerontol. A. Biol. Sci. Med. Sci. 54, B5–B11 10.1093/gerona/54.1.B5 (doi:10.1093/gerona/54.1.B5) [DOI] [PubMed] [Google Scholar]
- 71.McCarter R. J. M. 1995. Role of caloric restriction in the prolongation of life. Clin. Geriatr. Med 11, 553–565 [PubMed] [Google Scholar]
- 72.Duffy P. H., Feuers R., Nakamura K. D., Leakey J., Hart R. W. 1990. Effect of chronic caloric restriction on the synchronization of various physiological measures in old female Fischer-344 rats. Chronobiol. Int. 7, 113–124 10.3109/07420529009056963 (doi:10.3109/07420529009056963) [DOI] [PubMed] [Google Scholar]