Sensory versus motor loci for integration of multiple motion signals in smooth pursuit eye movements and human motion perception

Abstract

We have investigated how visual motion signals are integrated for smooth pursuit eye movements by measuring the initiation of pursuit in monkeys for pairs of moving stimuli of the same or differing luminance. The initiation of pursuit for pairs of stimuli of the same luminance could be accounted for as a vector average of the responses to the two stimuli singly. When stimuli comprised two superimposed patches of moving dot textures, the brighter stimulus suppressed the inputs from the dimmer stimulus, so that the initiation of pursuit became winner-take-all when the luminance ratio of the two stimuli was 8 or greater. The dominance of the brighter stimulus could be not attributed to either the latency difference or the ratio of the eye accelerations for the bright and dim stimuli presented singly. When stimuli comprised either spot targets or two patches of dots moving across separate locations in the visual field, the brighter stimulus had a much weaker suppressive influence; the initiation of pursuit could be accounted for by nearly equal vector averaging of the responses to the two stimuli singly. The suppressive effects of the brighter stimulus also appeared in human perceptual judgments, but again only for superimposed stimuli. We conclude that one locus of the interaction of two moving visual stimuli is shared by perception and action and resides in local inhibitory connections in the visual cortex. A second locus resides deeper in sensory-motor processing and may be more closely related to action selection than to stimulus selection.

to a large degree, the initiation of smooth pursuit eye movements is driven by visual motion (Lisberger and Westbrook 1985; Rashbass 1961), and the motor output provides a good probe for the properties of sensory processing. Systematic overestimates of target speed for apparent motion appear to originate from shifts in the population response in visual area MT toward neurons with larger preferred speeds (Churchland and Lisberger 2001). Trial-by-trial variation in the initiation of pursuit seems to arise from the properties and structure of correlated noise in the responses of MT neurons (Huang and Lisberger 2009; Osborne et al. 2005). The transfer of the properties of sensory processing directly to the motor output implies a reflexive nature to the initiation of pursuit, yet pursuit is a voluntary movement that is subject to extensive cognitive control, at least in humans (Barnes 2008). Perhaps some features of the initiation of pursuit arise in the properties of sensory processing, while others originate in top-down modulation.

Vector averaging is a strategy used by the pursuit system to combine signals originating from the motion of two separate targets. When identical spot targets move in orthogonal directions across different parts of the visual field, the resulting initiation of pursuit can be modeled best as the vector average of the pursuit initiation evoked by the two stimuli singly (Lisberger and Ferrera 1997). However, winner-take-all behavior emerges when a monkey or human is cued about which target to track (Ferrera and Lisberger 1995; Garbutt and Lisberger 2006; Recanzone and Wurtz 1999; Shichinohe et al. 2009). Attempts to determine the site of the vector averaging for two spot targets have led to the conclusion that vector averaging is located downstream from the site(s) of motor learning in pursuit (Kahlon and Lisberger 1999) and the site(s) where the gain of visual-motor transmission is controlled (Tanaka and Lisberger 2002) by outputs from the smooth eye movement region of the frontal eye fields (Tanaka and Lisberger 2001). Thus it seems unlikely that the vector averaging previously seen in pursuit occurs in the primary sensory representation of visual motion in the primary visual cortex or extrastriate area MT.

The eye movements evoked by combinations of two moving stimuli have been analyzed in detail for the visual-motor reflex of “ocular following.” Comparison of the interactions of superimposed stimuli versus stimuli that appear in different locations has led to the conclusion that some interactions occur during earlier sensory processing while others occur downstream (Sheliga et al. 2008). In ocular following, the interactions that occur in early sensory processing result in complete suppression of the motion signals from a low-contrast stimulus when a higher-contrast stimulus shares the same visual field location. The interactions that occur downstream include a divisive normalization that prevents summation of motion signals from separate parts of the visual field.

Following the lead of Sheliga et al. (2008), we regard the eye movements evoked by the motion of two stimuli as a probe that can reveal how visual stimuli normally are processed to create smooth eye movements. Given the existence of both similarities and differences between pursuit and ocular following, it seems possible that the mechanisms proposed for visual drive of ocular following will have partial or perfect homologs for pursuit eye movements. Because prior experimental designs for the analysis of pursuit have employed stimuli that are separated in the visual field, we do not know whether suppressive interactions take place in early vision for pursuit, as they do for early vision in ocular following. The divisive normalization proposed for ocular following is one mechanism to implement vector averaging such as that seen downstream from sensory processing in pursuit. Given how much is known about the neural basis for sensory, sensory-motor, and motor processing in pursuit (Lisberger 2010), it seems important to explore interactions between multiple visual motion stimuli thoroughly for pursuit.

Our goal in the present paper was to put our knowledge of the interaction of multiple moving stimuli for pursuit on the same broader ground as Sheliga et al. (2006, 2008) and Matsuura et al. (2008) have done for ocular following. A major goal was to determine how fully the initiation of pursuit eye movements follows directly from the features of visual motion processing, and to localize different forms of interactions of multiple motion stimuli in the pursuit circuit.

We varied the relative luminance of two targets and their degree of overlap in the visual field. Eye movement responses are explained well by vector averaging whenever the two stimuli have equal luminance. However, a brighter stimulus dominates a dimmer stimulus, presumably because of interactions in visual processing, when the two stimuli are superimposed. In contrast, when the stimuli move across separate regions of the visual field, vector averaging is affected little by luminance differences. Human perception follows the same pattern of interaction as the initiation of pursuit eye movements in monkeys. Thus pursuit seems to follow the same rules as ocular following. The locus of interaction of multiple superimposed stimuli appears to be in visual processing. The interaction between two separated stimuli occurs at a second locus deeper in the pursuit system.

METHODS

We recorded eye movements from four male rhesus monkeys (Macaca mulatta), using the scleral search coil method. Two of the monkeys were used for almost all the experiments. The additional two monkeys were used only for the experiments at the end of the article that studied the interaction of overlapping or separated noisy and coherent patches of dots. Before experiments began, we conducted sterile surgery with isoflurane for general anesthesia and implanted a socket to stabilize the monkey's head and eye coils to measure eye position (Ramachandran and Lisberger 2005). The monkeys also had been trained to sit in a primate chair and perform a visual-tracking task in exchange for liquid rewards. Daily experiments lasted 2–3 h. All methods had been approved in advance by the Institutional Animal Care and Use Committee at the University of California, San Francisco (UCSF) and followed the National Institutes of Health Guide for the Care and Use of Laboratory Animals.

We also recorded perceptual judgments from four human subjects, all naive to the purpose of the experiment. Subjects viewed the visual stimuli on the same monitors used for the monkey experiments. They viewed presentations of moving stimuli of 150-ms duration while fixating a stationary spot at the center of the screen. In the experiment we instructed subjects to use buttons to report their perceptions in two steps. First, they reported whether they saw 0, 1, or 2 moving stimuli. Second, if they saw only 1 moving stimulus, they reported whether it moved in a horizontal, vertical, or oblique direction. Subjects gave their informed consent before the experiments. All methods had been approved in advance by the Human Subjects Committee at UCSF.

Visual stimuli and experimental paradigm.

We presented visual stimuli on a CRT monitor with a spatial resolution of 2,304 × 1,440 pixels and a refresh rate of 80 Hz. The screen was 30 cm from the monkey and subtended a visual angle of 66.7 × 50.8°. In most experiments, visual stimuli consisted of patches of 100% correlated random dots plotted within in a 3° × 3° aperture on a black background. The size of each dot was 0.05°, and the dot density was 1 dot/degree².

Experiments were conducted as a series of trials, each of which began when the monkey brought his eyes within 1° of a fixation spot at the center of the screen for 200 ms. The fixation spot then disappeared, and one or two stimuli appeared and began to move immediately. For the majority of the stimuli that comprised patches of dots, the dots moved inside a stationary, virtual aperture for 150 ms (Fig. 1, left). If dots reached the edge of the virtual aperture in this brief interval, they disappeared and were replaced by new dots at the other side of the aperture. Then, the aperture and dots moved together for 600–800 ms (Fig. 1, right). Because the direction and speed were the same for dot and aperture motion during this later interval, no dots reached the edge of the aperture and the stimulus moved as a static object. In trials that began with two moving stimuli, one of the stimuli disappeared 150 ms after the onset of motion, at the time when dot motion within the aperture turned to en bloc motion of the dots and aperture. Stimulus speed was always 20°/s.

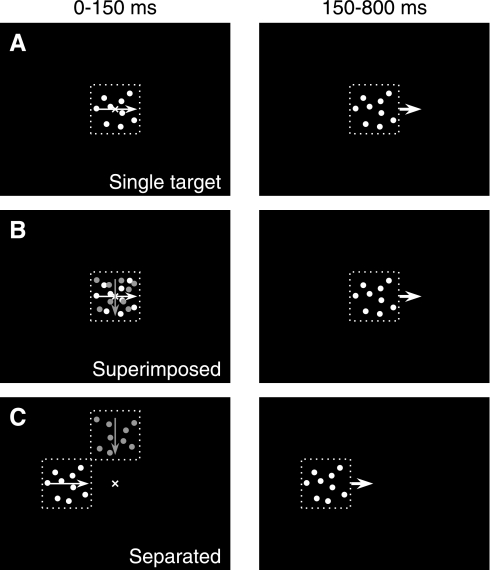
Fig. 1.

Schematic showing the visual stimuli and the sequence of presentation. Left: the first 150 ms of stimulus motion, when 1 or 2 patches of dots are visible and move behind an invisible, stationary aperture. Right: the next 650 ms of stimulus motion, when only 1 patch of dots is visible and the aperture and dots move together, en bloc, across the screen. A: single patch. B: 2 superimposed patches. C: 2 separated patches. Dashed squares represent the invisible apertures around the patches, white and gray dots represent bright or dim stimuli, thin arrows inside patches represent the directions of dots moving within the aperture, and thick arrows outside patches represent the directions of combined dot and aperture motion. Crosses in images on left represent the initial fixation spots, which disappeared as soon as the stimuli started to move.

The main manipulation in our experiments was to vary the relative luminance of pairs of patches that moved simultaneously and then to determine the weighting of the two stimulus motions in determining the initiation of pursuit. For most of the experiments with two-patch stimuli, the luminance of the dots in one patch was 40 cd/m² and the luminance of the dots in the other patch was chosen to be one of 1.25, 2.5, 5, 10, 20, and 40 cd/m² in different trials. Thus the luminance ratio between the two patches was 1, 2, 4, 8, 16, or 32. In one set of experiments, we kept the summed luminance of the dots in the two patches equal at either 41.25 or 80 cd/m² by varying the luminance of the dots in both patches within a range from 1.25 to 77.6 cd/m² so that the luminance ratio had three values of 1, 4, and 32.

Each trial presented the motion of one or two patches of dots in configurations that were either superimposed or separated (Fig. 1). Superimposed patches appeared in the center of the screen and remained superimposed during the 150 ms when the dots moved within the virtual, stationary aperture (Fig. 1B). Separated patches appeared along the horizontal or vertical meridian 3° or 6° from the center of screen (Fig. 1C). Motion always was toward the center of the screen. Each experiment also included trials that presented single patches of different luminance (Fig. 1A), with motion in each of the four cardinal directions: rightward, leftward, upward, or downward. The two components of paired patches were created from pairs of the patches presented singly, with the constraint that we always presented orthogonal motion directions. To improve the efficiency of data collection and the number of repetitions for each pair of moving stimuli, each monkey was shown only the two pairs of stimulus directions that evoked the strongest pursuit. Monkeys were allowed a grace time of 300 ms after the onset of target motion when tracking accuracy was not monitored and were required thereafter to keep their eyes within 3° of the target throughout its motion to obtain a reward. To minimize the monkey's ability to anticipate target motion, each of the two stimuli in a trial was equally likely to disappear, causing the other stimulus to become the tracking target.

In various control experiments, we introduced a number of minor modifications to the basic experiment. To explore the effect of latency of tracking for the two targets, we introduced a 25-ms delay to the motion onset of one patch. To allow comparison with prior work on pursuit of two-target stimuli (Lisberger and Ferrera 1997), we used spots instead of patches as visual stimuli. Each spot was 0.4° square, and the luminance was chosen in the same way as for the patch stimuli. Spots appeared 3° eccentric, providing step-ramp target motion (Rashbass 1961). To explore the relationship between perception and pursuit for identical stimuli, we introduced stochastic noise so that one patch contained random dots with a motion coherence of 0%. To create a similar appearance of the normal and noise patches we increased the dot density to 4 dots/degree².

Data acquisition and analysis.

Eye velocity signals were created by analog differentiation of voltages proportional to the angle of the eye in the orbit, using a circuit that differentiated signals at frequencies below 25 Hz and filtered out those at higher frequencies (−20 dB/decade). Horizontal and vertical eye position and velocity were sampled at 1 kHz on each channel. Before we analyzed the data, we reviewed the eye movement records and removed from further analysis any trials with saccades in the interval from 20 ms before to 180 ms after the onset of target motion. We then pooled all responses to the same stimulus and calculated the time course of the mean and variance of horizontal and vertical eye velocity. Because our analysis would be based only on the responses during the initiation of pursuit, before there had been time for any visual feedback, we pooled responses to the same initial stimuli without regard for which of the two stimuli became the target the monkey received a reward for tracking.

To identify the latency of pursuit in each condition, we computed the mean and standard deviation of eye velocity at each time point and averaged both across the interval from 20 ms before to 80 ms after the onset of target motion. We then used the averaged mean ± 1.5 SD of the baseline eye velocity as the threshold to identify the onset time of pursuit. We analyzed pursuit in three different intervals within the first 100 ms after the time chosen as the initiation of pursuit. Most of our analyses were based on the first 50-ms interval after pursuit onset, with a few based on the second 50-ms interval or the first 20-ms interval. We chose to focus on the first 100 ms of pursuit as the response that precedes visual feedback and can be considered to be within the “open-loop” interval of pursuit. We used linear regression to calculate the average horizontal and vertical eye acceleration during the chosen analysis interval and combined the horizontal and vertical components of acceleration to obtain the vector of eye acceleration. We measured eye direction from the horizontal and vertical eye acceleration in the first 50 ms of the response. We then quantified direction error on each trial as the difference between eye direction on that trial and the mean eye direction, and then estimated trial-by-trial variation as the standard deviation of the direction error.

RESULTS

Effect of relative luminance and visual field location on the weighting of two stimuli for the initiation of pursuit.

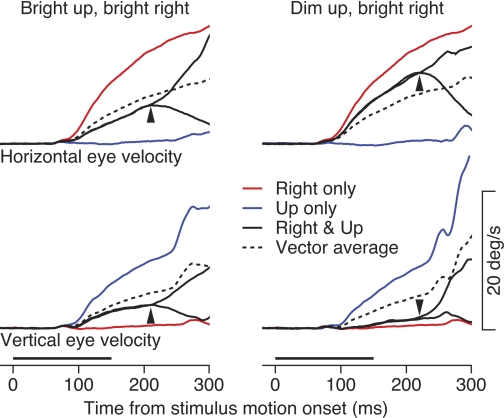
The pursuit evoked by the combined motion of two stimuli depended on the relative luminance of the stimuli and on whether or not they occupied the same part of the visual field. When the two stimuli were of equal luminance, the responses to each singly combined linearly in approximately the way predicted by vector averaging. As shown in Fig. 2, left, rightward motion of a bright stimulus (red traces) evoked a strong rightward eye velocity that started ∼100 ms after the onset of target motion, along with essentially zero change in vertical eye velocity. Upward motion of a bright stimulus (blue traces, Fig. 2, left) evoked eye velocities that were almost perfectly vertical. When the bright upward and rightward stimuli were presented simultaneously (continuous black traces, Fig. 2, left), both horizontal and vertical eye velocity started “on time” ∼100 ms after the onset of stimulus motion and both started with approximately the trajectory predicted by the vector average of the responses to the two target motions singly. The initial horizontal and vertical eye velocities both had slightly lower amplitudes than did the vector average prediction (dashed black trace, Fig. 2, left), indicating that eye direction conformed to the prediction while eye speed was a little lower than predicted. The two black traces in each panel in Fig. 2, left, indicate responses to the paired stimuli, under conditions when one or the other disappeared after 150 ms of motion so that the other became the tracking target. The time when the two traces separate (arrowheads) indicates the end of the interval of pursuit that was driven by the simultaneous motion of two stimuli.

Fig. 2.

Average eye velocity traces showing examples of the initiation of pursuit for motion of 2 superimposed stimuli. Left: data for upward and rightward motion of bright stimuli. Right: data for rightward motion of a bright stimulus and upward motion of a dim stimulus, of luminance 1/8 of the bright stimulus. Each trace shows average horizontal or vertical eye velocity as a function of time. Red and blue traces show eye velocities evoked by the rightward and upward targets presented singly. Continuous black traces show eye velocities evoked by the simultaneous motion of the 2 stimuli. Dashed black traces show the predictions from the use of vector averaging to combine the responses to the 2 stimuli presented singly. Arrowheads show the time when the 2 black traces separated because the monkey had started to track 1 stimulus after the other disappeared. Bold horizontal lines at bottom indicate the time of simultaneous motion of 2 stimuli.

When the two stimuli differed in luminance (Fig. 2, right), in this case a ratio of 8 to 1, the results for single target motions looked quite similar but the pursuit evoked by simultaneous motion of the two stimuli was quite different. As before, rightward motion of a bright target (red traces, Fig. 2, right) evoked strong, purely horizontal eye velocity, while upward motion of a dim target (blue traces, Fig. 2, right) evoked purely vertical eye velocity that was only slightly weaker than that evoked by a brighter stimulus moving in the same direction. Simultaneous motion of the two stimuli across the same locus of the visual field, however, revealed strong dominance of the rightward motion from the bright target (continuous black traces, Fig. 2, right). The resulting horizontal eye velocity was larger than the vector averaging prediction (dashed black trace, Fig. 2, right), while the vertical eye velocity was much smaller, indicating that the direction of the eye movement was biased toward the brighter stimulus. Again, the two black traces separated ∼225 ms after the onset of target motion, indicating tracking of the remaining target ∼75 ms after one stimulus disappeared.

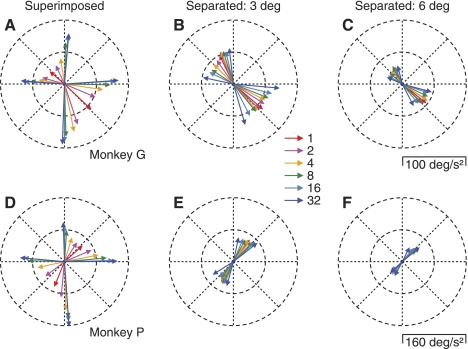
Results from the full experimental sequence and a wide range of luminance ratios are summarized in Fig. 3. If the patches were superimposed in the center of the visual field (Fig. 3, A and D), then pursuit was in the direction of the brighter patch when the ratio of the luminance was 16 or 32 (blue arrows) but was in an intermediate direction when the ratio of the luminance was 1 or 2 (red and purple arrows). If the two patches appeared at separate locations 3° or 6° eccentric along orthogonal axes (Fig. 3, B, C, E, and F), the ratio of the luminance of the two stimuli had a less profound effect on the direction of the resulting eye movement. Pursuit was biased in the direction of the brighter patch when the luminance ratio was high but never ignored the dimmer patch, as it had when the two patches were superimposed. Figure 3 also suggests some differences in the effect of luminance ratio on the amplitude of the resulting smooth pursuit initiation, but these did not hold up as reliable effects across the two monkeys.

Fig. 3.

Effect of changing luminance of the dimmer target on the initiation of pursuit. Each arrow represents an eye acceleration vector for the first 50-ms pursuit, where angle shows the direction of the eye acceleration and length shows the amplitude of the eye acceleration. Different colors indicate different luminance ratios, where the brighter target always had the same luminance. Top and bottom: data for monkeys G and P, respectively; to allow enough repetitions of each stimulus pair, we used only 2 pairs of orthogonal directions in each monkey. A and D: superimposed stimuli. B and E: stimuli presented 3° eccentric along orthogonal axes. C and F: stimuli presented 6° eccentric along orthogonal axes.

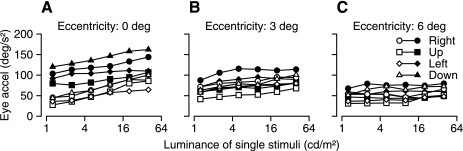
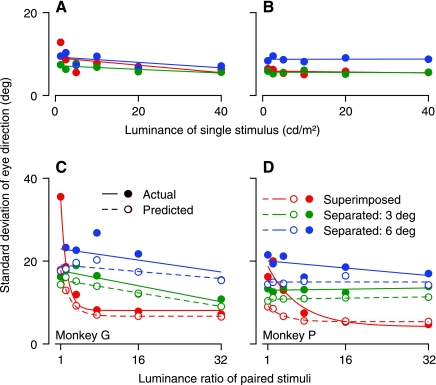
To understand and quantify the interaction of moving visual stimuli that differed in luminance, we started by analyzing the effect of luminance on the magnitude of the eye acceleration for single stimuli at different locations. For the central location used for the superimposed stimuli, eye acceleration increased as a function of the luminance of the target (Fig. 4A), doubling over the 32-fold range of luminance we used in monkey G (open symbols) and increasing by 50% in monkey P (filled symbols). The effects of luminance were less profound when the stimuli appeared either 3° or 6° eccentric along the horizontal or vertical meridian (Fig. 4, B and C).

Fig. 4.

Effect of luminance on eye acceleration for single stimuli. Each point shows mean eye acceleration in the first 50 ms of pursuit, plotted as a function of the luminance of the dots in the stimulus. Different symbols show data for different directions of stimulus motion, and solid vs. open symbols plot data for monkeys P and G, respectively. A: superimposed stimuli. B: stimuli presented 3° eccentric along orthogonal axes. C: stimuli presented 6° eccentric along orthogonal axes.

With the responses to single stimuli of different luminance documented, we were able to quantify the effect of the ratio of luminance on the responses to two moving stimuli. To do so, we computed the weights assigned by the initial pursuit response to each stimulus in a two-patch stimulus using

$${\overrightarrow{E}}_{i,j}=w_i{\overrightarrow{E}}_i+w_j{\overrightarrow{E}}_j$$ (1)

where E⃗_i,j represents the eye acceleration vector for paired patches moving in directions i and j (shown in Fig. 3),E⃗_i and E⃗_j represent the eye acceleration vectors for single patches moving in the two directions (shown in Fig. 4), and w_i and w_j represent the weighting of the two stimuli when presented as part of paired patches. In this formulation, perfect vector averaging occurs when w_i and w_j are 0.5, perfect summation occurs with the two weights are 1, one stimulus completely suppresses the response to the other when one weight is 1 and the other is 0, and the overall gain of pursuit is decreased by the presence of two stimuli when the sum of the two weights is <1.

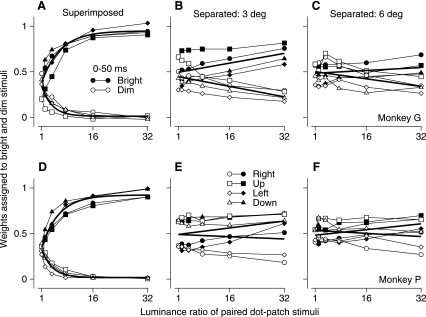
For superimposed stimuli (Fig. 5, A and D), the weights assigned to the two stimuli were approximately equal when the two stimuli had equal luminance and averaged 0.36 and 0.33 in monkeys G and P. As the luminance ratio increased, the weight assigned to the brighter component increased rapidly and reached a value close to 1 for the largest luminance ratio. At the same time, the weight assigned to the dimmer stimulus decreased quickly and approached 0. When the luminance ratio was 8 for the superimposed stimuli, the weights for the brighter and dimmer components were 0.79 and 0.04 for monkey G and 0.78 and 0.05 for monkey P. We fitted the data for each monkey with exponential curves and found very similar parameters for the two monkeys (Table 1). If we use w_b = 0.8 as threshold for winner-take-all behavior for the brighter stimulus, then according to the fitting results the relevant luminance ratio is 8.4 for monkey G and 8.9 for monkey P.

Fig. 5.

Effect of luminance ratio on the weighting of each patch of dots for the first 50 ms of pursuit during simultaneous motion of 2 stimuli. Each symbol shows weights for 1 of the 2 targets, plotted as a function of the ratio of the brighter to the dimmer luminance. Filled and open symbols plot data for the bright and dim stimuli, respectively. Different symbol shapes indicate data for different directions of stimulus motion. A–C: data for monkey G. D–F: data for monkey P. A and D: superimposed stimuli. B and E: stimuli presented 3° eccentric along orthogonal axes. C and F: stimuli presented 6° eccentric along orthogonal axes. Solid curves (A, D) and lines (B, C, E, F) show fits to the data.

Table 1.

Equations used to fit relationship between weighting of each target and luminance ratio and best fitting parameters

Stimulus Location	Fitting Function	Parameter	Monkey G 0–50 ms	Monkey G 50–100 ms	Monkey P 0–50 ms	Monkey P 50–100 ms
Superimposed	wb = wb0 + ab(1 − e−bbc)	wb0	0.27	0.16	0.22	0.33
		ab	0.68	0.83	0.70	0.87
		b	0.18	0.09	0.20	0.03
		r2	0.9868	0.9710	0.9933	0.9013
	wd = wd0 + ade−bdc	wd0	0.01	0.07	0.02	0.04
		ad	0.46	0.55	0.43	0.41
		bd	0.33	1.16	0.32	0.13
		r2	0.8953	0.7980	0.9534	0.7270
Separated by 3°	wb = wb0 + abc	wb0	0.49	0.46	0.49	0.38
		ab	0.007	0.004	0.005	0.001
		r2	0.9531	0.9620	0.9417	0.9313
	wd = wd0 + adc	wd0	0.45	0.35	0.45	0.40
		ad	−0.007	0.004	−0.002	0.003
		r2	0.9388	0.8365	0.8811	0.9071
Separated by 6°	wb = wb0 + abc	wb0	0.48	0.38	0.48	0.44
		ab	0.002	0.005	0.004	0.000
		r2	0.9740	0.9370	0.9746	0.9742
	wd = wd0 + adc	wd0	0.51	0.22	0.54	0.38
		ad	−0.005	0.003	−0.002	0.001
		r2	0.9436	0.9152	0.9488	0.9660

All values, including r², are averaged across stimuli that presented different pairs of target directions. Subscripts b and d indicate brighter and dimmer stimuli, respectively.

For separated stimuli, the weight for the brighter component increased only slightly and never approached a value of 1 (Fig. 5, B, C, E, and F). When the luminance ratio was 8, the weights assigned to the brighter/dimmer stimuli for monkeys G and P were about 0.54/0.40 and 0.52/0.48 when the stimuli appeared 3° eccentric along orthogonal axes and 0.50/0.46 and 0.51/0.52 when the stimuli appeared 6° eccentric. We used linear regression to fit the data for the separated stimuli, again obtaining parameters that agreed well for the two monkeys (Table 1). We chose linear fits because they seemed to describe the data well. Exponential fits provided the same agreement between the two monkeys, while accounting for somewhat less of the variance in the data.

In the experiments described so far, the luminance of one stimulus was fixed and the luminance of the other stimulus was varied, so that the total luminance of the two-patch stimulus varied systematically with luminance ratio. We obtained the same results in control experiments in which we changed the luminance of both patches to keep the total luminance of the paired patches constant (not shown).

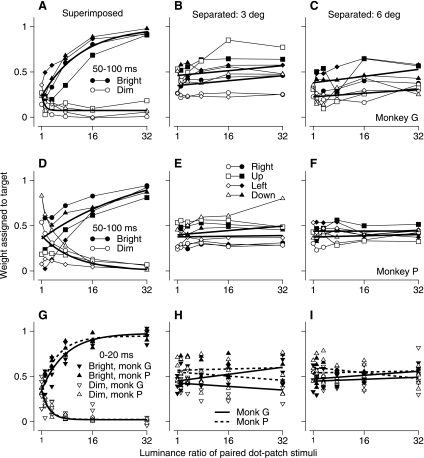
The data plotted and discussed so far were measured from the first 50 ms of eye velocity after the onset of pursuit. Figure 6, A–F, show that the data for the second 50 ms of eye velocity were similar. If the stimuli were superimposed (Fig. 6, A and D), then the data showed the same progression from vector averaging when the luminance ratio was 1 to winner-take-all pursuit for the brighter stimulus when the luminance ratio was 8 or larger. The general trend seems consistent even though the results for the second 50 ms of pursuit showed considerable variation at a luminance ratio of 1 in monkey P (Fig. 6D), where different combinations of stimulus directions produced quite different weights. If the two stimuli appeared in separate locations, then the luminance ratio had a smaller effect on the weights of vector averaging in the second 50 ms of pursuit (Fig. 6, B, C, E, and F) than it did in the first 50 ms of pursuit (Fig. 5, B, C, E, and F). As shown in Table 1, most of the parameters of the equations used to fit the data were quite similar for the analysis of the first versus second 50 ms of pursuit.

Fig. 6.

Effect of luminance ratio on the weighting of each patch of dots for the second 50 ms and first 20 ms of pursuit during simultaneous motion of 2 stimuli. Each symbol shows weights for 1 of the 2 targets, plotted as a function of the ratio of the brighter to the dimmer luminance. Filled and open symbols plot data for the bright and dim stimuli, respectively. A–F: data for the second 50 ms of pursuit. Different symbol shapes indicate data for different directions of stimulus motion. A–C: data for monkey G. D–F: data for monkey P. G–I: data for the 2 monkeys measured during the first 20 ms of pursuit. Upward and downward triangles show averages across target directions for the 2 monkeys. Continuous and dashed curves show fits for monkeys G and P, respectively. A, D, and G: superimposed stimuli. B, E, and H: stimuli presented 3° eccentric along orthogonal axes. C, F, and I: stimuli presented 6° eccentric along orthogonal axes.

We also conducted an analysis designed to control for the fact that we had analyzed pursuit in a 50-ms window that was triggered on the latency of pursuit initiation and therefore could be different for the two single patches and the two-patch stimulus. We analyzed eye acceleration in a time window of 20-ms duration that started at the longest of the three latencies of the pursuit evoked by the two stimuli presented singly or together. The short analysis window was chosen to keep all analyses within the first 50 ms of all responses. The data in Fig. 6, G–I, show that the results from this 20-ms interval agree well with the data analyzed during the first 50 ms of pursuit in both monkeys.

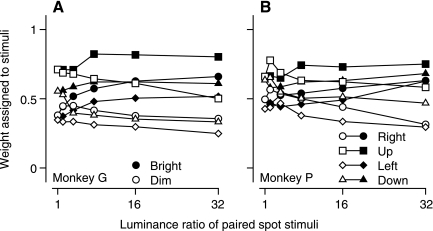
To allow comparison with the results of prior two-target experiments (Lisberger and Ferrera 1997), we also varied the luminance ratio for stimuli that comprised one or two moving spots. As in the prior work, the spots appeared 3° eccentric along two orthogonal axes. We calculated the weighting assigned to each spot with the strategy outlined above for patches of dots. When the two spots were of equal luminance, the weights assigned to them for the initiation of pursuit were approximately equal. As the luminance ratio increased, there was a slight tendency to assign a higher weight to the brighter target (Fig. 7, filled symbols) and a lower weight to the dimmer target (Fig. 7, open symbols). The effect of changing the luminance of spot targets was similar to that for patches of dots that appeared in separate locations.

Fig. 7.

Effect of luminance ratio on the weighting of spot stimuli during simultaneous motion of 2 stimuli. Each symbol shows weights for 1 of the 2 targets, plotted as a function of the ratio of the brighter to the dimmer luminance. Filled and open symbols plot data for the bright and dim stimuli, respectively. Different symbol shapes indicate data for different directions of stimulus motion. Symbols show data for the first 50 ms of pursuit. A: monkey G. B: monkey P.

We have shown so far that the relative weighting of two simultaneously moving stimuli in the initiation of smooth pursuit eye movements depends strongly on the relative luminance of the two stimuli and on whether they cover the same region of the visual field. We turn next to a number of control experiments to understand better the cause of the interactions.

Effect of pursuit acceleration and latency on relative weighting of two stimuli.

We asked next whether the relationship between the weighting of the bright/dim targets and their luminance ratio could be explained as a consequence of the difference between the eye accelerations caused by each target presented singly. To do so, for each pair of targets we computed the “eye acceleration ratio,” defined as the acceleration caused by the bright stimulus presented singly divided by that caused by the dim stimulus. We then plotted the weight assigned to each target as a function of eye acceleration ratio. To obtain the largest possible range of ratios for eye acceleration, we used data obtained when the sum of the luminance of the paired patches was held constant as the luminance ratio changed and luminance varied from 1.25 to 77.5 cd/m².

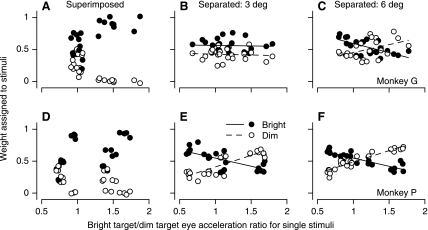
When the patches were superimposed (Fig. 8, A and D), there was a clear relationship between weighting and acceleration ratio for monkey G (Fig. 8A). The same basic relationship appears for monkey P (Fig. 8D) but is bimodal. The bimodality results because, for example, the eye accelerations for upward and rightward motion were quite different. For example, a bright rightward motion provoked much larger eye acceleration than a bright upward motion, so that the acceleration ratios were >1 even though the targets were of equal luminance and the weighing of each stimulus was 0.5. Furthermore, a bright upward motion evoked smaller eye acceleration than a dim rightward motion, creating acceleration ratios (bright/dim) that were <1 even when the weighting favored the upward target motion strongly. The same situation obtained for downward and leftward motion. As a consequence, there are two exponential relationships between weighting and acceleration ratio starting at 0.7 and 1.4 on the x-axis in Fig. 8D. The bimodal relationship in Fig. 8D provides some evidence that the eye acceleration caused by a stimulus motion presented singly is not the sole determinant of the weighting assigned to that stimulus when it is presented as part of a pair of stimulus motions. The absence of an important role for the acceleration ratio is supported more strongly by the data for separated pairs of patches. In Fig. 8, B, C, E, and F, the acceleration ratios cover the same range as for the superimposed patches, but the weights of the bright and dim patches do not progress toward 1 and 0 as the eye acceleration ratio increases. Indeed, in three of the four graphs, there is a paradoxical increase in the weight of the dimmer target as the eye acceleration of the brighter target becomes relatively larger. We have no explanation for this unexpected observation.

Fig. 8.

Relationship between weighting of 2 stimuli and the ratio of eye acceleration evoked by single stimuli. Each symbol shows weights for 1 of the 2 targets, plotted as a function of the ratio of the eye acceleration produced by the brighter to the dimmer stimulus when presented singly. Filled and open symbols plot weights for the bright and dim stimuli, respectively. Data were obtained from the first 50 ms of pursuit. A–C: monkey G. D–F: monkey P. A and D: superimposed stimuli. B and E: stimuli presented 3° eccentric along orthogonal axes. C and F: stimuli presented 6° eccentric along orthogonal axes. In the graphs for separated stimuli, the solid and dashed lines show the regression lines for the bright and dim targets, respectively.

Pursuit latency is longer for dimmer stimuli. The average differences in pursuit latency between the brightest (40 cd/m²) and dimmest (1.25 cd/m²) single-patch stimuli presented at the center of gaze or 3° or 6° eccentric were 40.8 ± 2.3 ms, 31.5 ± 1.0 ms, and 21.5 ± 3.0 ms for monkey G and 18.3 ± 4.3 ms, 19.3 ± 3.8 ms, and 17.8 ± 3.8 ms for monkey P. Therefore, we also tested the possibility that a stimulus causing a response with a shorter latency would be weighted more heavily when presented at the same time as a stimulus that caused pursuit after a longer latency.

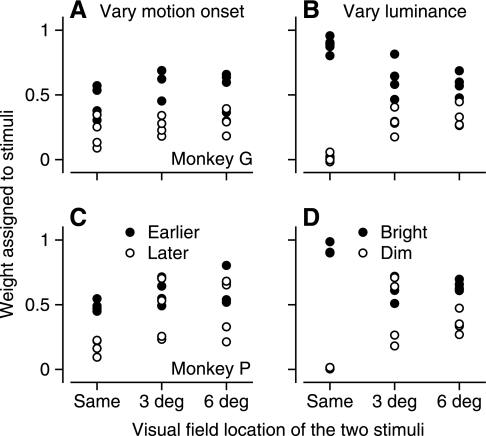
To test the effect of latency on the weights assigned each target, all patches of dots had the same luminance of 40 cd/m², but we introduced a 25-ms delay into the onset of one of the two patches. The resulting difference in latency between the pursuit responses to the two stimuli averaged 25 ms and ranged from 18 to 32 ms. We then compared the weights assigned to the stimuli that started earlier and later with the weights in the experiment where differences in luminance caused comparable differences in pursuit response latency (15–35 ms, average 21.5 ms). In both monkeys, the earlier response (filled symbols, Fig. 9, A and C) tended to be weighted somewhat more heavily than the later response (open symbols) for both superimposed and separated stimuli. However, the effect was quite small by comparison with the strong favoritism toward the brighter stimulus (filled symbols, Fig. 9, B and D), especially when the two stimuli were superimposed.

Fig. 9.

Small effect on weights assigned to each target by latency difference in the responses to each target presented singly. A and C: experiments that varied the onset time of 2 moving stimuli with equal luminance. B and D: experiments that varied the luminance of 2 moving stimuli and indirectly the latency of pursuit initiation for each stimulus singly. Filled and open symbols plot weights for the earlier and later stimuli, respectively, in A and C and the bright and dim stimuli, respectively, in B and D. Data were obtained from the first 50 ms of pursuit. A and B: monkey G. C and D: monkey P.

The data in Figs. 8 and 9 suggest that the weights assigned to the motion of each stimulus were decided mainly by the luminance ratio of the two stimuli rather than by the property of the eye movements evoked by the two targets singly. The different effects for superimposed versus separated stimuli imply the existence of two loci of interaction with different properties. We suggest that the interaction of two superimposed stimuli occurs during early sensory processing, while the interaction of two spots or separated patches of dots may occur later in sensory-motor integration or even in the motor pathways for pursuit (e.g., Kahlon and Lisberger 1999).

Trial-by-trial variation of pursuit direction.

Analysis of the direction of initial pursuit on each individual trial revealed that the variation of pursuit direction for two-stimulus motions could be accounted for by the variances of the responses to each stimulus singly, in almost all cases. The only notable exception occurred when the moving stimuli were superimposed and had equal luminance. For stimuli presented singly (Fig. 10, A and B), the standard deviation of pursuit direction either was flat or decreased slightly as luminance increased. To determine whether the variance of the responses to pairs of motions could be accounted for by the variance of the responses to each motion singly, we used a sampling analysis to predict the variance of the responses to pairs of target motions. We drew all possible pairs of responses from the full distributions of all the individual-trial responses to each stimulus singly. For each paired draw, we simulated the direction of eye acceleration as the vector average of the two responses. We then used the full set of simulated pairs of responses to predict the standard deviation of the direction for pursuit evoked by the two-stimulus motion.

Fig. 10.

Trial-by-trial variation of pursuit direction. A and B: standard deviation of eye direction as a function of the luminance of single stimuli. C and D: standard deviation of eye direction as a function of the luminance ratio for 2 simultaneous stimuli. Filled symbols show measurements from the data; open symbols show predictions for simultaneous stimuli based on the variation in responses to the 2 stimuli singly. Continuous and dashed lines show linear regression fits to the points for actual and predicted standard deviation, except for superimposed stimuli in C and D, where the curves show exponential fits to the points. Red, green, and blue symbols show data for superimposed stimuli, stimuli presented 3° eccentric along orthogonal axes, and stimuli presented 6° eccentric along orthogonal axes, respectively. A and C: monkey G. B and D: monkey P.

Comparison of the predicted standard deviations of direction (Fig. 10, C and D, open symbols and dashed curves) with the actual standard deviations (filled symbols and continuous curves) revealed excellent agreement for all pairs of target motions when the stimuli appeared in separate locations (green and blue symbols). When the moving stimuli were superimposed (red symbols, Fig. 10, C and D), the predicted and actual standard deviations differed if the luminance of the two stimuli was similar but agreed well if luminance differed between the two stimuli. The excess variation of direction for superimposed, equal-luminance stimuli implies that this particular stimulus condition causes a considerable increase in the variation of estimates of target direction obtained by decoding the population response in extrastriate area MT (Huang and Lisberger 2009).

Comparison of motion perception and pursuit initiation.

The strong dominance of bright over dim stimuli presented at the same visual field location supports the idea that the interaction occurs in sensory processing (i.e., Sheliga et al. 2008) and provoked us to design experiments that would compare monkeys' pursuit with humans' perceptual responses for the same sets of stimuli. Our rationale was that an interaction that occurred in early sensory processing should have equivalent effects on motion perception and pursuit.

We chose an experimental design based on our finding that pursuit behaved as though only one moving stimulus was present when that stimulus was 1) brighter than a second stimulus and 2) covered the same region of the visual field. Our design was constrained by the need to use stimuli that would work for both pursuit and perceptual tasks, and the need to obtain comparable measures. Our logic was that the vector averaging pursuit indicated that the pursuit system “saw” two moving stimuli, while winner-take-all pursuit indicated that the pursuit system “saw” only one moving stimulus. Therefore, our perceptual experiment asked whether the subjects saw one or both of two stimuli. If they saw only one stimulus, we asked them to indicate whether the direction of motion was horizontal, vertical, or oblique so that we could be sure that the two physical stimuli were not being merged by the perceptual system into a single unified percept that was an average of the two real motions. As before, we used two stimuli positioned either at the center of the screen or along orthogonal meridia. In some trials, the stimuli comprised different luminance patches of 100% correlated dots. In other trials, one stimulus was made up of 100% correlated moving dots, another was made up of 0% correlated noisy dots, and we controlled the luminance of both stimuli.

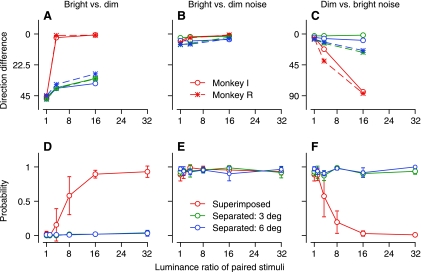
To analyze the pursuit of monkeys for pairs of moving dot patterns, we computed the difference between the direction of the initiation of pursuit for two-patch stimuli and that for its brighter component presented singly. With such a metric, a direction difference of 0 would imply a 100% probability of tracking the brighter stimulus and ignoring the dimmer stimulus; a direction difference of 45° would imply equal weighting of the two stimuli. For the interaction of bright versus dim patches of 100% correlated moving dots (Fig. 11A), the direction difference was close to 45° for all luminance ratios when the patches appeared in separate locations (green and blue symbols), as expected if the pursuit system emitted behavior based on vector averaging of the two stimuli. When the patches were superimposed (red symbols, Fig. 11A), the direction difference transitioned smoothly from around 45° (vector averaging) to 0° as the luminance ratio became larger, indicating winner-take-all behavior (100% probability of tracking) for the brighter of the two stimuli.

Fig. 11.

Monkey pursuit and human perception for bright vs. dim patches of coherent dot motion vs. noise. A–C: difference between the direction of 2 monkeys' pursuit initiation for paired patches and the direction of their pursuit initiation for the brighter (A, B) or dimmer (C) patch presented singly. D–F: probability that human reported a single moving stimulus in the direction of the brighter (D, E) or dimmer (F) stimulus. In each graph, data are plotted as a function of the luminance ratio of the brighter divided by the dimmer stimulus. Red, green, and blue symbols show data for superimposed stimuli, stimuli presented 3° eccentric along orthogonal axes, and stimuli presented 6° eccentric along orthogonal axes, respectively.

Perceptual experiments with two 100% correlated dot patterns produced parallel reports (Fig. 11D). When the dot patterns appeared in separate visual field locations, subjects always reported that they saw two moving patterns (superimposed green and blue symbols). Thus, in Fig. 11D, the probability of reporting a single pattern that moved in the direction of the brighter stimulus was always zero. When the moving dot patterns were superimposed (red symbols, Fig. 11D), subjects always reported two, transparent, moving stimuli when the luminance of the patterns was the same. As the luminance difference between the patterns increased, they transitioned to an increased probability of reporting a single moving stimulus in the direction of the brighter stimulus. These patterns of perceptual reports make intuitive sense, and are noteworthy mainly by comparison with the results of the parallel experiment on pursuit, reported in Fig. 11A.

The pursuit behavior of monkeys and the perceptual reports of motion detection also agreed well when the stimuli were a bright or dim patch of 100% correlated moving dots and a dim or bright patch of 0% correlated dots. When the stimuli comprised bright moving dots versus equally bright or dimmer noise, pursuit was in the direction of the bright dots for all conditions (Fig. 11B). For the same stimuli, perceptual reports indicated a 100% probability of seeing a single motion in the direction of the bright stimulus (Fig. 11E). In both pursuit and perception, bright motion suppressed responses to dimmer noise.

When the stimuli comprised dim moving dots and bright noise, the dominance of the bright noise over the dim motion required a somewhat different analysis metric. For pursuit, we computed the difference between the direction of the initiation of pursuit for two-patch stimuli and that for the single dimmer patch. The initiation of pursuit in monkeys always was in the direction of the dimmer motion when the two stimuli were separated, and when the correlated dots and noisy dots were superimposed and of equal luminance (Fig. 11C). As the moving dots became dimmer, the direction difference increased to 90°, which is orthogonal to the direction of the dimmer motion. In fact, this orthogonal direction corresponds to the direction of pursuit initiation for the bright noise presented alone, which was biased away from the position of the stimulus along the orthogonal axis, even though there was no coherent motion (see also Tanaka and Lisberger 2000). For perception (Fig. 11F), we plotted the probability of reporting a single moving stimulus in the direction of the dimmer stimulus. Humans reported seeing a single stimulus in the direction of the dimmer stimulus every time when the two stimuli appeared in separate locations (green and blue symbols, Fig. 11F) and when the two stimuli were superimposed and had equal luminance (red symbols, Fig. 11F). When the two stimuli were superimposed, however, humans transitioned to reporting that they saw no motion as the 100% correlated dots became dimmer. In both pursuit and perception, bright noise suppressed responses to dimmer motion.

DISCUSSION

Interactions of pairs of motion signals.

Our study uses tracking conditions in which multiple motion signals are present to evaluate how visual motion signals are pooled to generate a command for the initiation of smooth pursuit eye movements. Our prior studies of pursuit have emphasized the use of vector averaging to combine two stimuli that move in different directions and across different parts of the visual field (Lisberger and Ferrera 1997). Initially, we had thought of presentation of two targets as a way to probe the mechanisms that transform the population code in visual area MT into commands for movement. However, more recent experiments showed that vector averaging occurs downstream from the loci of both motor learning in pursuit (Kahlon and Lisberger 1999) and control of the gain of visual-motor transmission (Tanaka and Lisberger 2002). Both of these functions are thought to occur outside of the sensory representation of visual motion. Thus the form of vector averaging we studied before does not seem to occur within the parts of the pursuit circuit responsible for visual processing.

Others have used different approaches and obtained somewhat different, but compatible, data. Ferrera and Lisberger (1995) used a cuing task, while Recanzone and Wurtz (1999) altered the timing and location of the two targets: both found conditions where pursuit could show winner-take-all behavior. The locus of these modulatory effects is unknown, although it again seems likely that they occur outside of area MT (Ferrera and Lisberger 1997), with some debate about whether they might occur in area MST (Recanzone and Wurtz 1999, 2000). Sheliga et al. (2006, 2008) studied a related visual tracking behavior called “ocular following” and found evidence for interactions between multiple motion stimuli that depended on the spatial relationship of the stimuli. In the present study, we evaluated the pursuit evoked by two moving stimuli with a wide range of stimulus parameters, to determine whether some of the interactions between pairs of targets occurred within versus after sensory processing. Our goal was to leverage the two-stimulus paradigm to obtain new insight into the neural mechanisms of visual-motor transformations.

Local, nonlinear interactions in sensory processing.

In good agreement with the data obtained for ocular following (Sheliga et al. 2006, 2008), we found that two superimposed stimulus motions interact in a competitive manner. The initiation of pursuit is dominated by the brighter of the two stimuli, and increasing dominance turns to winner-take-all behavior as the luminance ratio of the two stimuli increases. Human perception shows a similar phenomenon for two superimposed stimuli, with the dimmer stimulus suppressed completely as the luminance ratio increases. On the basis of the similarity of the effects in pursuit and perception, and the presence of winner-take-all behavior only for stimuli that share the same part of the visual field, we conclude that the interaction of two superimposed stimuli occurs as part of early sensory processing and could be mediated by inhibitory mechanisms within topographically restricted regions of the visual cortex.

Extrastriate area MT seems like a good candidate site for both the vector averaging of two superimposed stimuli of equal luminance and their nonlinear interaction leading to winner-take-all behavior for the brighter stimulus in both pursuit and perception. MT neurons are selective for motion, and the response amplitudes of many MT neurons depend on stimulus contrast (Cheng et al. 1994; Heuer and Britten 2002; Krekelberg et al. 2006; Sclar et al. 1990). MT neurons integrate visual inputs successfully only if they are superimposed, implying that MT is a site of local but not global integration (Majaj et al. 2007), an effect that can be understood in terms of mechanisms of normalization in MT (Britten and Heuer 1999; Heuer and Britten 2002). The output from MT is important for both motion perception and the initiation of pursuit eye movements (Newsome et al. 1985; Newsome and Pare 1988). When there is only a single stimulus, vector averaging is successful as a way of decoding the population response in MT to produce the eye movements observed for a wide range of stimuli (Churchland and Lisberger 2001; Huang and Lisberger 2009; Lisberger and Movshon 1999; Yang and Lisberger 2009). Finally, the striking inflation of pursuit variation for superimposed, equal-luminance stimuli in our data can be understood in terms of the population response in MT. Superimposed stimuli moving in orthogonal directions would cause a profound broadening of the population response in MT, defined as a function of the preferred directions of the activated neurons. Huang and Lisberger (2009) showed that such a broadening of the population response would increase pursuit variation.

It is possible that the nonlinear interaction of overlapping stimuli occurs in the inputs to MT from the primary visual cortex (V1), where contrast normalization is an important feature (Geisler and Albrecht 1992; Rust et al. 2005; Tsui et al. 2010). Sheliga et al. (2006, 2008) have proposed the primary visual cortex as the locus of the local interaction of multiple stimuli for ocular following. We favor area MT because the properties of our fairly sparse random dot stimuli are better matched to the larger receptive fields of MT neurons than the very small receptive fields of V1 neurons in the central visual field. Although Sheliga et al. (2006, 2008) favor a locus of interaction in V1, their data also would be compatible with an interaction that occurs in MT. In any event, our data on the interaction of superimposed moving stimuli emphasize the large extent to which measures of the initiation of pursuit are a probe for the properties of visual processing, a point that has been made abundantly before for both pursuit eye movements (Lisberger 2010) and ocular following (Masson et al. 1997).

Vector averaging and normalization in sensory-motor processing.

In agreement with the data from ocular following, again, the interaction between stimuli that appear in separate visual field locations is different from that between superimposed stimuli. Pursuit follows much more closely the expectations for vector averaging of the responses to each stimulus singly, even when the luminance ratio of the two stimuli is quite large. For ocular following the results were partly compatible with our findings during pursuit, but more complicated. Ocular following shows normalization like that needed for vector averaging when two separate stimuli move in the same direction, but largely linear summation when the stimuli move in opposite directions. We expect a similar normalization in pursuit for stimuli moving in the same direction, on the basis that pursuit of a patch of dots is only slightly stronger than pursuit of a single small spot (Osborne and Lisberger 2009). It is difficult to evaluate the interaction of stimuli that move in opposite directions for pursuit because the evoked eye movements are small and do not discriminate strongly between different models of the interaction (S. G. Lisberger, unpublished observations).

We suggest that a site outside the area of primary sensory processing is the locus for the more linear form of vector averaging seen for stimuli presented in separate locations. This form of vector averaging could even reflect linear interactions between separate motor commands for the two separate visual stimuli, possibly occurring much later in sensory-motor processing (Kahlon and Lisberger 1999). The absence of a strongly nonlinear interaction between separated stimuli and the similar interactions for stimuli that appear 3° or 6° eccentric suggest that vector averaging for separate stimuli occurs on a spatial scale that is too large for area MT and therefore must be located downstream. Although area MST is one candidate locus (Churchland et al. 2007; Recanzone et al. 1997; Sheliga et al. 2008), a locus deeper in the motor system is suggested by the previous findings that vector averaging in pursuit for two moving spot stimuli occurs downstream from where the smooth eye movement region of the frontal eye fields regulates the gain of pursuit (Tanaka and Lisberger 2002), and also downstream from motor learning (Kahlon and Lisberger 1999). Possible loci may include the visual-cerebellar relay nuclei in the brain stem, or even the cerebellum itself.

Stimulus interactions for pursuit, ocular following, and other behaviors.

Our findings are similar to those for ocular following (Sheliga et al. 2006, 2008) but also go beyond them in several ways. First, pursuit and ocular following have different functions and respond to different visual stimuli: ocular following is designed to track the motion of large stimuli that are fixed in the world, while pursuit tracks the motion through the world of smaller objects. We have shown how interactions work at two levels within the pursuit system, where we have considerable knowledge about neural mechanisms beyond visual motion processing (Lisberger 2010). Second, the ocular following response is quite small (1–2°/s in Sheliga et al. 2006), while we have studied eye velocities that are a much larger fraction of target velocity (5–10°/s), verifying the functional importance of the mechanisms that mediate the interactions we have studied. Third, we have added observations on human perception and shown that they agree well with the results for superimposed moving stimuli, supporting the idea that the interactions of superimposed stimuli arise during sensory processing. Finally, studies of ocular following have looked only at stimuli of the same or opposite direction of motion, while we have considered the more natural situation for pursuit where different stimuli move in more congruent directions. The work of Sheliga et al. (2006, 2008) did have the advantage of using stimuli that probe the bank of spatial-frequency-tuned motion detectors believed to sense and react to motion energy, but this advantage is not particularly relevant to the specific issues addressed here, namely, of the loci of vector averaging behavior in pursuit. Still, to achieve the goal of understanding how the brain generates ocular following and smooth pursuit, it will be necessary to understand the similarities and differences between the two behaviors, and their neural circuits, including the low-level mechanisms of visual motion processing for each.

In the specific examples of pursuit eye movements and the ocular following response, the interaction of multiple stimuli across different spatial scales has been studied in relation to the continuum between vector averaging and winner-take-all behavior. A similar division of different effects according to different spatial scales has been observed in other behavioral systems as well, albeit with different measurement end points. In saccades (Walker et al. 1997), a distractor modifies the amplitude or latency of a saccade to a nearby target, depending on the exact spatial relationship between the target and the distractor. In salience measures, luminance salience operates on a very small spatial scale and can be tentatively assigned to precortical processing, while orientation salience operates on a spatial scale of 1–2° and can be tentatively assigned to neural processing in the primary visual cortex (Northdurft 2000). Thus all behavioral systems may have features that operate on different spatial scales, and experiments like those conducted in pursuit, ocular following, saccades, and salience have the potential to identify the relevant neural loci for stimulus interactions on the basis of behavioral observations alone.

Conclusions.

We have found different effects of altering the relative luminance of superimposed versus separate stimuli. Our findings imply that interactions occur at two (or more) sites in the sensory-motor circuit for pursuit. We suggest that the first site processes local motion information competitively during early vision, while the second site produces more linear interactions during sensory-motor or motor command formation. We can think of the first site as a sensory interaction based on stimuli from a single object and the second site as a locus that may be better related to response choice than to stimulus choice.

GRANTS

This research was supported by the Howard Hughes Medical Institute and National Eye Institute Grant EY-03878.

DISCLOSURES

No conflicts of interest, financial or otherwise, are declared by the author(s).