Trends in Labor Force Participation: How Much is Due to Changes in Pensions?

Abstract

In the United States, beginning in the late 1980s there was a substantial increase in the labor force participation of men and women in their 60s. Over the same time period the type of pension plans offered by employers shifted strongly from defined benefit plans to defined contribution plans. Defined benefit plans typically have optimal retirement ages embedded in their structure which induce early retirement, whereas defined contribution plans do not favor any particular retirement age. Based on panel data, this paper quantifies the increase in participation due to the change in pension structure. The main result is that the pension changes account for a considerable part of the increase, but other factors also made a contribution.

Introduction

The aging of the populations in developed economies is expected to lead to a greater ratio of retirees to workers, putting financial pressure on pensions (public and private) and health insurance systems. For example, in the United States in 2000, the population aged 65 or over was 21% of the working age population (20–64), but it is forecast to increase to 36% by 2030.

One way to mitigate this pressure is to design public policies that will encourage a longer work life via later retirement. Such a solution may be practical because of the increased life expectancy that is partly responsible for the trend toward more retirees: if workers have increasingly better health in conjunction with greater life expectancy, they will be able and possibly willing to work longer.

The trend toward later retirement has apparently already begun in the United States. The labor force participation rates of older men in the United States have increased according to data from the Current Population Survey (CPS). For example, the labor force participation rate of men 60–64 rose from 55.5% in 1990 to 58.6% in 2006. The increase was even greater for those aged 65–69: from 26.0% to 34.4%. Trends for women have been similar.

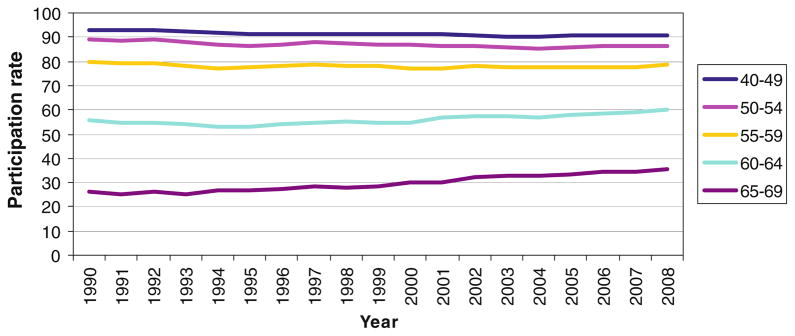
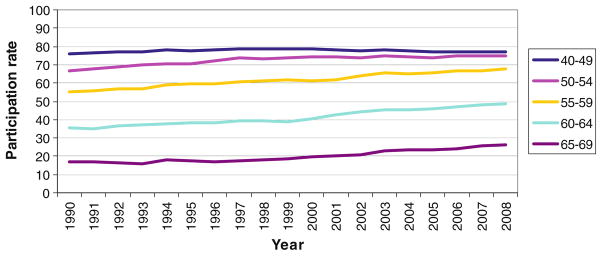
As a simple matter of accounting, these trends could have been due to a stable rate of retirement at older ages combined with an increase in labor force participation at younger ages resulting in a larger fraction of the population working at older ages. Or the trends could have been due to stable labor force participation at younger ages and reduced retirement rates at older ages. For men the latter is the case. As Fig. 1 shows, there has actually been a slight decline in labor force participation among men at younger ages: a smaller fraction of 40-to-49-year-olds was in the labor force in 2006 than in 1990. Thus, relatively fewer men entered the pool of potential retirees in 2006 than in 1990, and the higher post-60 labor force participation is the result of lower retirement rates among older men conditional on reaching their 60s. The situation for women is more complex. Figure 2 shows that the labor force participation rate among women aged 40–49 increased slowly until year 2000, but among women 50–54, participation increased by about 12% (eight percentage points) between 1990 and 2006, and among older women the increase was even greater: 32% and 42% in the age bands 60–64 and 65–69 respectively. Thus part of the increase in labor force participation by women in their 50s and 60s was the result of an increasing pool of working women reaching those ages and partly the result of changes in retirement behavior.

Fig. 1.

Labor Force Participation by age. Men. Source: CPS Civilian Labor Force Participation Rates. See Table 1 for detailed series. Note: The top line refers to ages 40–49, and the other lines follow the same order as in the legend

Fig. 2.

Labor Force Participation by age. Women. Source: CPS Civilian Labor Force Participation Rates. See Table 1 for detailed series. Note: The top line refers to ages 40–49, and the other lines follow the same order as in the legend

Especially for women, we would like to separate trends in inflow to the retirement pool from retirement rates at older ages. To do that we assembled synthetic cohort data from the CPS, taking advantage of the large sample sizes in the CPS. We used these data to calculate transition rates out of the labor force, that is, the retirement hazard rate. Specifically, we computed the transition rates out of the labor force in five-year age bands across five-year intervals. For example, we found the labor force transition rate among those who are 60 to 64 in 1990 as they aged to 65 to 69 in 1995 by dividing their labor force participation rate in 1995 by that in 1990, and subtracting the quotient from 1.

In 1990 the labor force participation rate of men aged 60–64 was 55.5% and in 1995 the rate of 65–69 year-old men was 27.0%. Thus, our synthetic panel estimate of the retirement hazard rate for that cohort over those five years was 51.4% as shown in Table 1.¹ The table shows that the age-specific, five-year retirement hazards for working women are about one percentage point greater than for men except in the top age band where they are about three percentage points greater. Thus conditional on reaching age 50 and being in the labor force, women are approximately as likely to remain in the labor force as men until they reach their late 60s. There has been a substantial decline in the retirement hazards of both men and women in their late 50s and 60s. For example among men in their late fifties, the retirement hazard declined from 33.4% to 24.1%. The decrease in the retirement hazard of women was approximately the same. The implication is that for men in their 60s the labor force participation rate increased even as the participation rate of younger men decreased because of a sharp decline in the retirement hazard rate. For women the increase was partly the result of an increase in the participation rate among younger women and partly the result of a sharp decline in the retirement hazard. Thus, the increase in participation by women in their early 60s was particularly large: from 35.5% in 1990 to 47.0% in 2006 (Fig. 2).

Table 1.

Five-year rates (percent) of transition into retirement

Initial and final year	Men			Women
	50–54 to 55–59	55–59 to 60–64	60–64 to 65–69	50–54 to 55–59	55–59 to 60–64	60–64 to 65–69
1990–1995	12.8	33.4	51.4	11.1	31.3	50.7
1991–1996	11.9	31.4	49.7	11.8	31.4	51.0
1992–1997	11.6	31.0	48.1	11.6	30.5	51.6
1993–1998	11.0	29.2	48.2	12.2	31.5	51.9
1994–1999	9.6	28.7	46.0	12.6	34.5	51.3
1995–2000	10.9	29.1	43.0	13.2	32.4	48.7
1996–2001	11.2	27.3	44.4	14.2	28.9	47.6
1997–2002	11.2	26.8	40.9	13.2	27.3	47.6
1998–2003	11.1	27.0	40.8	10.3	26.1	41.9
1999–2004	10.8	27.3	40.5	12.2	26.5	39.9
2000–2005	10.6	24.7	38.8	11.5	25.4	41.0
2001–2006	10.3	24.1	39.2	10.0	23.8	43.1

Note: Transition rates pertain to workers in an initial five-year age band and after five years in the ending age band. The initial year is the beginning year of the transition and the final year is the last year of the transition

Source: Authors’ calculations based on CPS Civilian Labor Force Participation Rates. For men the data series are LNU01300179, LNU01300181, LNU01300188, LNU01300189, LNU01300197, LNU01300203, and for women the data series are LNU01300338, LNU01300340, LNU01300345, LNU01300346, LNU01300352, LNU01300358. Data accessed at http://www.bls.gov/data/ on October 27, 2010

Although there are a number of possible causes for the decline in the retirement hazard, of particular note is a trend in the U.S. in the type of employer-provided pensions. Traditionally, pensions were defined benefit (DB) pension plans which provide an annuity in retirement and which typically focus retirement at particular retirement ages. They do this by providing large financial incentives to work until the normal retirement age and financial disincentives for working past the normal retirement age (Kotlikoff and Wise 1987). However, there has been a very substantial shift from defined benefit plans to defined contribution (DC) plans. Defined contribution plans offer a stock of pension wealth at retirement (which could be annuitized but is not required to be annuitized), but they do not focus retirement at any particular age (Johnson 2009). Because the normal retirement age under many DB pension plans is in the late 50s or early 60s, a shift from defined benefit to defined contribution plans could be responsible for the trend toward later retirement (Friedberg and Webb 2005).

Joint or coordinated retirement could also be responsible for some of the decline in the retirement hazard. Working husbands and working wives tend to retire at the same time (Hurd 1990; Blau 1998; Gustman and Steinmeier 2000; Blau and Gilleskie 2006). If working wives retire at typical retirement ages, 62–65, husbands would tend to retire in their mid- to late-60s rather than in their early 60s because on average husbands are about three years older than their wives. In that female labor force participation rates have been increasing, more men will be coordinating their retirement, and so retiring later, than was previously the case. Indeed, Schirle (2007) attributes a substantial fraction of the increase in men’s labor force participation to joint retirement. However, even assuming that explanation is correct, we would like to know what caused the increase in women’s labor force participation.

Health is an important determinant of retirement (Bound 1991; Bound et al. 1999). While there is evidence that disability rates have been declining in the population older than 65 (Freedman et al. 2002; Schoeni et al. 2001), which would lead us to conclude that better health could be part of the explanation for the trend toward later retirement, it is not clear that health has been improving in the working-age population. For example, self-reported health has declined as measured in the Health and Retirement Study in the population 51–56 (Weir, 2007; Soldo et al., 2007). Thus, the evidence about the effect of any trend in health on retirement is not strong.

The education level of the retirement-age population increased substantially over the time period we are considering, and Blau and Goodstein (2008) attribute the increase in participation by men to the change in education. The explanation would be that jobs associated with less education require physical effort making it difficult for the older population to remain working. While perhaps this is true for men it does not seem likely for women, who typically do not have physically demanding jobs. A second factor is the well-documented decline in cognitive functioning with age (Salthouse 2006), which at least potentially would make it difficult for older people to remain in the types of jobs filled by the well educated.

Additional possible explanations include increases in longevity necessitating more retirement wealth and therefore a longer work life, and changes in the economic circumstances of workers such as increases over time in wealth. Finally, changes in public policy especially changes in Social Security rules have likely had an effect on retirement; we will return to this issue in the concluding section.

The goal of this paper is to provide evidence about the shift in pension type in explaining the increase in labor force participation at older ages and the corresponding delay in retirement. In panel data we will estimate the effects of pensions on retirement. Then using the prevalence of defined benefit and defined contribution pensions in 1992 and in 2004 among workers in their early 50s, we will simulate labor force participation under the two pension regimes. The difference in the simulations will show the effects of the change in pensions on retirement and on labor force participation at older ages for these two cohorts. While we cannot directly compare our difference with the trends in labor force participation shown in Fig. 1 because those trends involve earlier cohorts, we can compare our difference with the expected labor force participation of the two cohorts. We find that the pension changes are likely responsible for part of the increase in expected labor force participation, but it is also likely that public policy played an important role.

There are at least three reasons for wanting to quantify the effects of the shift in pension type. First, if the shift continues we will have an ability to forecast continuing changes in the labor force participation of the older workforce from observed changes in the structure of pensions. Second, the forecast will help policy makers evaluate the magnitude of the labor market problem associated with the aging of the population. Third, the results will illustrate the potential for influencing retirement by altering private pensions and possibly public pensions.

Pensions and Retirement Behavior

Public pensions—those provided by the government—are available in the United States in the form of Social Security and in Europe through various state-run arrangements. Public pensions have been the subject of considerable research. Social Security and other public pensions have been found to have had a strong influence on the timing of retirement (Gruber and Wise 1999).

Private pensions are those provided by an employer, and they have a substantial influence on retirement (Samwick 1998; Kotlikoff and Wise 1987; Stock and Wise 1990). Private pensions are, broadly speaking, of two types—defined-contribution (DC) and defined-benefit (DB). Persons with DC plans accumulate pension wealth, but do not face incentives to retire at any particular age, although a firm may not allow access to DC pension wealth before some particular age. People with DC plans may retire early if they have accumulated substantial wealth in their plans and access to that wealth is allowed by the firm, but not because of any incentives inherent in the plan’s structure.

In contrast, DB pensions have sharp incentives embedded in them. There can be large gains in pension value from working until the retirement age set by the pension plan (which can vary across employers). The gains create an incentive to remain until that retirement age. Those who work past that age can accrue more personal wealth, but their pension income is typically not increased, even though, to be actuarially fair, it should be adjusted upward because the expected years of payout decline with age. Said differently, benefits must be increased to keep pension wealth (the expected discounted value of future pension income) constant. Thus, working past the retirement age embedded in the pension plan results in loss of pension wealth (Kotlikoff and Wise 1987). Not surprisingly, research has shown a strong tendency for workers to retire at the retirement age as specified by the DB pension plan. Because that age is typically 62 or earlier in DB plans, workers with DB plans are unlikely to work beyond their early 60s. A shift from DB to DC plans could result in less early retirement and, hence, more later retirement.

Data

The data for this analysis are from the Health and Retirement Study (HRS) (Juster and Suzman 1995), a survey funded by the National Institute on Aging, with additional funding from the Social Security Administration. The HRS is a biennial longitudinal survey of individuals 51 or older. In this study we use data from eight waves, fielded from 1992 through 2006. The sample is representative of the U.S. population, except for certain oversamples, for which weights are used to calculate population averages. In the initial wave the target population was from the cohorts born in 1931 through 1941, and they were approximately aged 51–61 at the time of the interview. The initial sample size was about 12,000, including age-eligible spouses but also spouses who themselves were not age-eligible but were married to age-eligible persons. In 1998 new cohorts were added making the HRS representative of the population aged 51 or over. In 2004 new cohorts aged 51–56 were again added. With the addition of these cohorts the sample size is about 20,000.

The HRS questionnaire has sections on health, economic status, labor market activity, and family linkages, among other topics. Respondents report labor force status, information about employment, and, if working, whether they participate in a pension on their job. If they do participate, they report their pension plan type (DB, DC, or both) and the age at which benefits are fully available as well as any earlier age at which they may be partially available.

We take advantage of the addition of new cohorts to make cohort comparisons of pension entitlement. In particular, we compare the cohorts who were 51–56 in 1992 with the cohorts who were 51–56 in 1998 and 51–56 in 2004. Because in each case the cohorts were freshly recruited into the HRS, they are cross-sections that are population representative and do not suffer from any differential attrition that may occur in panel data. Table 2 shows the percentage of workers who have DB plans, DC plans or both on their jobs, conditional on having a pension.² After taking into account joint holdings of DB and DC plans, approximately the same percentage of men as women held DB plans. But, for both men and women, there has been a large decline in those having a DB plan only and a corresponding large increase in those having a DC plan only. If DB plans induce early retirement and DC plans are neutral with respect to the timing of retirement, the shift from DB to DC would cause later retirement on average in the population of workers.

Table 2.

Percentage of workers aged 51 to 56 having plans of various types, conditional on having a pension

	Men			Women
	1992	1998	2004	1992	1998	2004
DB	38.8	28.1	21.3	44.7	30.7	26.8
DC	29.3	36.4	48.7	33.5	43.2	51.4
Both DB and DC	31.9	35.6	30.0	21.8	26.1	21.8
All	100.0	100.0	100.0	100.0	100.0	100.0

Note: About 60% of workers had a pension on their current job in all periods

Source: Authors’ calculations based on HRS 1992, 1998 and 2004

Results

We begin with the relationship between DB pension eligibility and retirement, specifically, the probability of leaving the labor force or the retirement hazard rate.³ We calculate the retirement hazard rate as the fraction of workers in wave t who are not working in wave t+1 in the HRS panel data.⁴ We group workers in age bands according to their ages in wave t+1 rather than according to their ages in wave t because for some particular ages, such as age 62, it is important that all workers in the age group will have achieved that age by wave t+1. Workers are further grouped according to their eligibility for DB plan benefits (no DB plan, DB plan but not yet eligible for benefits, became eligible between waves t and t+1, became eligible before wave t). We calculate as many as six transitions for each worker based on seven data waves, or about 25,000 transitions in total. The process is repeated for DC plans.

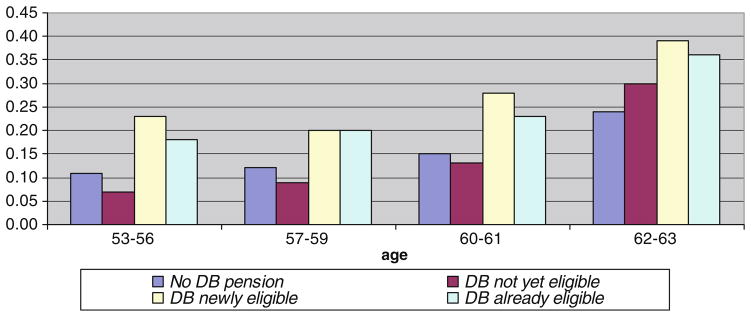
Figure 3 shows two-year retirement rates, or hazards, for workers at various ages with various DB plan benefit eligibilities. For example, among workers aged 53–56 at wave t+1, the retirement rate among those lacking a DB pension on their current job was 11% over the previous two years. Among those who had a DB plan but were not yet eligible to receive benefits, the retirement rate was just 7%. Those newly eligible or previously eligible retired at rates of 23% and 18% respectively. The figure suggests that becoming eligible for benefits increases the retirement hazard by 16 percentage points. The pattern of retirement rates—higher for those eligible for DB pensions—was similar for workers in other age bands, although the differences were not as great in relative terms for those aged 62–63. These workers were 60–61 in wave t and they retired at a high rate on reaching 62. Age 62 is the modal retirement age in the U.S. because it is the earliest age at which a worker can claim retirement benefits under Social Security, which is the public pension system in the U.S.

Fig. 3.

Two-year transition rates from working to not working. Variation by eligibility for DB pension benefits. Source: Authors’ calculations based on observed labor force transitions from HRS waves 1 to 8 (1992 – 2006). Note: “No DB pension” means worker is not enrolled in a DB plan; “DB not yet eligible” means worker is enrolled in a DB plan but is not yet age-eligible for full DB benefits; “DB newly eligible” means worker is enrolled in a DB plan and became age-eligible between the prior HRS wave and the current HRS wave; “DB already eligible” means worker is enrolled in a DB plan and was already age-eligible before the prior HRS wave

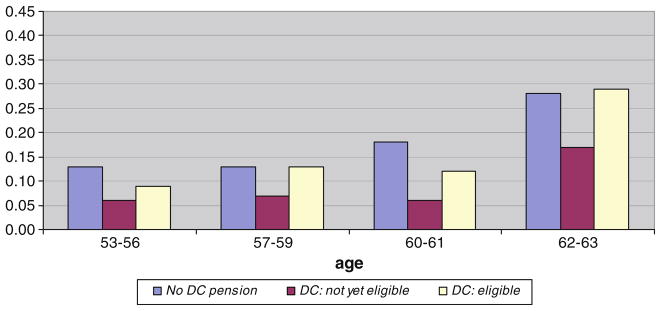
DC plans are associated with later retirement. As Fig. 4 shows, retirement hazards among workers eligible for DC plan benefits are similar to or less than the rates among workers having no DC plan. For example, in the age band 53–56 those with a DC plan but not yet eligible retired at a rate of only five percent in two years. Even those eligible for benefits retired at a rate of just nine percent. A comparison with Fig. 3 shows that for this age band the retirement rate of those eligible for DB benefits was 18 to 22 percentage points higher than the retirement rate of those eligible for DC benefits. The general pattern of retirement rates shown in Figs. 3 and 4 is consistent with the notion that, because of the earlier retirement ages typical of DB plans, a shift away from DB plans has the capability of increasing employment of older workers.⁵

Fig. 4.

Two-year transition rates from working to not working. Variation by eligibility for DC pension benefits. Source: Authors’ calculations based on observed labor force transitions from HRS waves 1 to 8 (1992 – 2006). Note: “No DC pension” means worker is not enrolled in a DC plan; “DC not yet eligible” means worker is enrolled in a DC plan but is not yet age-eligible to draw benefits; “DC eligible” means worker is enrolled in a DC plan and is age-eligible to draw benefits

To isolate the effects of DB plans from the effects of DC plans we performed a logistic estimation of the probability of leaving employment by wave t+1, conditional on employment at wave t. Covariates are indicators for the sex of the worker, for age bands, for wealth quartiles, for survey wave, and for worker eligibilities for DB and/or DC benefits. The pension variables include an indicator as to whether a worker has a plan, but also whether in any wave the worker had attained the age required to be eligible for benefits.⁶

These estimates are in Table 3, shown in terms of relative odds ratios, along with the P-value for the null hypothesis that the relative odds are 1.0. The reference group is a female, aged 53–56 in wave 2, in the lowest wealth quartile, lacking a pension, and observed in waves 1 and 2. The table also has the relative odds for specifications that exclude the wealth quartiles and that also exclude the indicator for sex.⁷ Men are less likely than women to leave the labor force. There is a sharp increase in the hazard with age with a particularly large increase at age 62, the youngest age at which retired worker benefits are available under Social Security. Workers aged 62–63 in wave t+1 were not eligible for Social Security benefits in wave t but they became eligible for benefits by wave t+1. This illustrates the powerful effect public pensions can have on retirement behavior. Those in the first wealth quartile have a somewhat greater likelihood of a transition into retirement but there is little variation across the other quartiles.⁸

Table 3.

Logistic estimation of retirement hazard. Relative odds. N=36730

	Odds ratio	Odds ratio	Odds ratio	p-value
Female		1.00	1.00	–
Male		0.75	0.75	<.001
Age 53–56	1.00	1.00	1.00	–
Age 57–59	1.13	1.15	1.15	0.003
Age 60–61	1.50	1.53	1.54	<.001
Age 62–63	2.79	2.88	2.90	<.001
Age 64–65	2.64	2.73	2.76	<.001
Age 66–68	2.88	3.04	3.08	<.001
Wealth quartile lowest			1.00	–
2			0.91	0.033
3			0.94	0.149
highest			0.86	0.000
No pension	1.00	1.00	1.00	–
DB only, not yet eligible	0.71	0.72	0.72	<.001
DB only, eligible	1.59	1.63	1.64	<.001
DC only, not yet eligible	0.44	0.45	0.45	<.001
DC only, eligible	0.85	0.87	0.88	0.023
DC & DB not yet eligible	0.42	0.43	0.44	<.001
DC eligible, DB not yet eligible	0.64	0.67	0.68	0.015
DC not yet eligible, DB eligible	1.21	1.28	1.30	0.370
Both eligible	1.55	1.62	1.65	<.001
Wave 1	1.00	1.00	1.00	–
Wave 2	1.01	1.00	0.99	0.919
Wave 3	0.89	0.87	0.87	0.007
Wave 4	0.94	0.92	0.91	0.074
Wave 5	1.05	1.02	1.01	0.859
Wave 6	0.81	0.78	0.78	<.001

Note: P-values are for a significant difference of the odds ratio from 1.0 for the specification in the last column

Source: Authors’ calculations based on HRS waves 1–8

The pension effects are relative to workers who have no pension on their current job. Someone with a DB plan who has not yet reached the age where he or she is eligible for benefits has a reduced likelihood of leaving the labor force whereas someone who is eligible has a much elevated likelihood. Indeed the odds of someone eligible for benefits relative to someone not yet eligible is 2.29 (= 1.64/0.72 from the next-to-last column). Workers with a DC plan who have not yet reached the age to be eligible for access to the DC balance are considerably less likely to leave the labor force than someone lacking a DC plan, and even someone who is eligible is marginally less likely.⁹ The other coefficients show the effects of various combinations of DB and DC plan eligibility among those who have both types. Retirement hazards from these combinations show progressive increases from ineligibility under either plan to eligibility for DC benefits alone to eligibility for DB benefits alone to eligibility for both. The increase is sharp, from a relative odds ratio of 0.44 for those ineligible under either plan to 1.65 for those eligible for both.

The wave indicators do not exhibit any consistent time trend in the hazard even though several are statistically significant.

Simulations

We would like to quantify the effects of a shift from DB to DC pensions as shown in Table 2. Our method is to simulate the labor force participation paths of workers who were aged 51–54 in 1992 (the “1992 cohort”) using their distribution of pension types. We begin the simulations at age band 51–54, to capture the full range of retirement behavior, including that of the many people with DB plans specifying normal retirement ages in their late 50s. Based on the logistic estimations of the effects of pensions on the retirement hazards, for each worker we calculated the probability of leaving the labor force as a function of his or her pension attributes including the ages for benefit eligibility. For example, a worker aged 54 with a DB plan that specifies an eligibility age of 57 would be assigned a 2-year probability of about 0.07, which is the predicted probability of retirement as estimated by the simplest of the logistic models of retirement. This low probability of transition is due to the worker not being eligible for DB benefits during the two years under consideration. Based on Monte Carlo methods the worker will either leave the labor force or survive in the labor force. If the worker survives in the labor force, we increase age by two years, compare the new age with the retirement ages of any pensions, and calculate the updated retirement hazard. Continuing the example, we assign a retirement probability of about 20% because the worker would be 58 in the following wave and would be newly eligible for benefits. With this updated retirement probability we repeat the Monte Carlo. We do this for all workers in the cohort to generate a path of labor force participation from age 51–54 to ages 65–68. Then we repeat these same calculations but use the pension characteristics of workers 51–54 in 2004 (the “2004 cohort”). These characteristics include ages for eligibility for benefits as reported by the 2004 cohort. A comparison of the two paths will show the estimated difference in labor force participation due to a shift in pensions.

The sample size for the simulations was 2788 for the 1992 cohort and 1751 for the 2004 cohort. We used the simple model that only included the pension variables and the wave indicators because of our desire to isolate changes associated with pension changes: had we used the more complex models, differences in the simulations could be due to differences in the fraction of women in the samples, differences in the wealth quartiles, and interactions between those variables and the pension variables. As a practical matter, the pension effects across the models in Table 3 are trivial.

As shown in Table 4, 40.9% of workers from the 1992 cohort (men and women combined) would still be working 10 years later when they were 61–64, and 23.5% would still be working 14 years later, when they were 65–68. The analogous rates for the 2004 cohort would be 43.0%, or 2.1 percentage points higher, at ages 61–64 and 26.1%, or 2.6 percentage points higher, at ages 65–68. The only differences between the two cohorts are the pension structures they face. The results suggest that the shift from DB to DC pensions explains an increase in labor force participation at older ages of about 2.6 percentage points or 11%.¹⁰

Table 4.

Simulated labor force participation rates conditional on working at age 51–54 (percent) and average subjective probability of working past 62 or 65

Age	1992 cohort	2004 cohort	Difference
Simulated labor force participation
51–54	100.0	100.0	0.0
53–56	88.2	88.1	−0.1
55–58	77.0	77.6	0.5
57–60	67.2	68.4	1.2
59–62	55.4	56.8	1.4
61–64	40.9	43.0	2.1
63–66	31.2	33.7	2.5
65–68	23.5	26.1	2.6
Average subjective probability reported at age 51–54
Working past 62 (P62)	46.7	49.8	3.1
Working past 65 (P65)	26.2	32.7	6.5

Note: Simulations of the 1992 cohort use the pension structure of 51–54 year-olds workers in 1992. Simulations of the 2004 cohort use the pension structure of 51–54 year-olds workers in 2004. Simulations based on model shown in first column of Table 3. P62 and P65 are averages of the same groups of workers as stated in 1992 and 2004 respectively

Source: Authors’ calculations based on HRS waves 1–8

Comparison of Simulated Labor Force Participation with the Subjective Probabilities of Working

As we discussed in the Introduction, according to the CPS the labor force participation rates of older Americans increased during the 1990s and 2000s. For example, between 1992 and 2006, the labor force participation rate of 60–64 year-olds (men and women combined) increased from 45.0% to 52.5%, and for 65–69 year-olds it increased from 20.6% to 29.0%. Our simulated increase is considerably smaller, about 2.6%. However, the populations we are comparing are not the same: The simulation based on 1992 HRS pension characteristics follows the same cohort that is observed in the CPS in 1992 as 51–54 year-olds and in 2006 as 65–68 year-olds. But the simulation based on 2004 pension characteristics pertains to the cohort that will be in its mid-to-late 60s in 2016 in the CPS. Thus the observed change in the CPS is relevant to different cohorts than the change we have simulated in the HRS. For a comparison of the same populations we use anticipated labor force participation for the cohorts. Our measures are the subjective probabilities of working past 62 and past 65 which are asked of workers in all waves.¹¹ Average values of these responses, which we call P62 and P65, are given in Table 4, as reported by the cohorts of 51-to-54-year-olds in 1992 and 2004. If expectations are rational, the averages of P62 and P65 would equal the average labor force participation rate at ages 62 and 65 of these cohorts.¹²

We can infer the importance of the pension changes relative to all other changes combined by comparing the difference in the simulation results (which holds constant all factors except for the structure of pensions) with the difference in P62 or P65. Were a change in pension structure the only cohort change, we would expect that the change in P62 or P65 would be the same as the change in predicted employment from the simulations. However, as shown in Table 4, the difference in pensions approximately at age 62 (61–64 age band) accounts for 2.1 percentage points of the difference out of a predicted 3.1 percentage points, or 68%, while the difference in pensions at age 65 (63–66 age-band) accounts for 2.5 percentage points out of 6.5 percentage points, or 38%. Thus, the change in the structure of pensions explains a substantial part of the change in anticipated employment, but not all.¹³

Conclusions

This study shows that pensions, in particular DB pensions, play an important role in determining labor force participation rates in the HRS, and that changes in the prevalence of DB and DC pensions are associated with changes in retirement rates. Specifically, simulations predict that the shift in private pension plans from predominantly DB to predominantly DC between 1992 and 2004 will increase labor force participation rates of people in their 60s by about 2.5 percentage points. Subjective probabilities of working at ages 62 or 65 have also gone up, and the pension shift explains 68% of the change in the age 62 prediction and 38% of the change in the age 65 prediction.

What accounts for the remainder of the changes in predicted labor force participation probabilities? There are several potential explanations. We have already discussed the possible roles of joint retirement, health and education. In addition there were several important policy changes. The Social Security “earnings test” was eliminated in 2000. Before then, the Social Security benefits of workers aged 65–69 were reduced if a worker had earnings above a threshold amount. The threshold was low so that most workers were affected. This penalty, often viewed as a tax by workers, is likely to have induced some workers to retire who might have otherwise continued to work.¹⁴ The elimination of the earnings test was accompanied by predictions that some workers would delay retirement past age 65, and it appears that abolition of the earnings test has led to later retirement (Song 2006; Song and Manchester 2007b).

Full retirement age under Social Security was age 65 for the cohorts born in 1937 or earlier. But a law change increased full retirement age for those reaching age 65 in 2003 to 65 and two months. The full retirement age of each succeeding birth year was increased by two months until it reached age 66 for those born in 1943.¹⁵ This cohort will reach age 65 in 2009, when it would have been eligible for full Social Security benefits under the old rules. Under the new rules it must wait until age 66 to get full benefits. Analysis of Social Security data on claiming ages shows clearly that there was a peak in the retirement hazard at age 65 prior to 2003, but that the retirement hazard of these transition cohorts increased by two months per year during the time period from 2003 to the present (Song and Manchester 2007a).

The largest increase in labor force participation has been among those 65–69. Our simulations indicate that pensions had a role in the increase, but it is likely that changes in the Social Security law were more important. Among those 60–64, however, our results show that pension changes were of greater importance than among 65–69 year-olds: the pattern of pension entitlement explained more of the change in expected participation in the younger age group. Furthermore, the changes in the Social Security law most directly affected the older group both via the increase in the Full Retirement Age and the elimination of the earnings test.

The direct effect on the younger group of the increase in the Full Retirement Age came from a reduction in benefits payable at early retirement at age 62: benefits were reduced by five percentage points on a base of 80% of full benefits or 6%. This reduction could have made retirement unaffordable for some workers but it is likely that the average effect in the population was small. The elimination of the earnings test could have affected the younger group indirectly. It would have made remaining in the labor force at younger ages somewhat more attractive because of the option of working past full retirement age while collecting Social Security benefits.

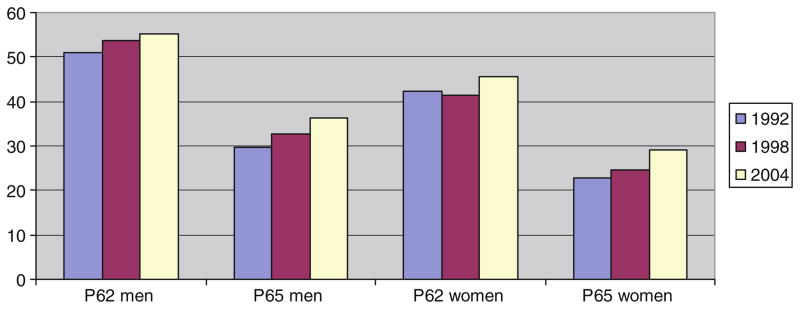
While the trend to later retirement is clear among workers in their 60s, we would like to know whether this trend will continue. Figure 5 shows the averages of P62 and P65 among workers aged 51–56 in 1992, 1998 and 2004. They are generally increasing for both men and women, and the increase is particularly strong for P65.¹⁶ The increase suggests that participation will continue to rise. For example, the average P65 among 51–56 year-old male workers in 1992 was 29.7%; among 51–56 year-old male workers in 2004 it was 36.3%. These figures predict that the participation rate of men shortly after they turn age 65 will have increased by 5.6 percentage points between the early 2000s and the mid-2010s.

Fig. 5.

Average values of P62 and P65 among workers age 51–56 in 1992, 1998 and 2004. Source: Authors’ calculations based on data from HRS 1992, 1998 and 2004. Note: P62 is the subjective probability of working past age 62 and P65 is the subjective probability of working past age 65