Abstract
Prestin was found in the membrane of outer hair cells (OHCs) located in the cochlea of the mammalian inner ear. These cells convert changes in the membrane potential into dimensional changes and (if constrained) to an active electromechanical force. The OHCs provide the ear with the mechanism of amplification and frequency selectivity that is effective up to tens of kHz. Prestin is a crucial part of the motor complex driving OHCs. Other cells transfected with prestin acquire electromechanical properties similar to those in the native cell. While the mechanism of prestin has yet to be fully understood, the charge transfer is its critical component. Here we investigate the effect of the mechanics of the surrounding membrane on electric charge transfer by prestin. We simulate changes in the membrane mechanics via the corresponding changes in the free energy of the prestin system. The free energy gradient enters a Fokker-Planck equation that describes charge transfer in our model. We analyze the effects of changes in the membrane tension and membrane elastic moduli. In the case of OHC, we simulate changes in the longitudinal and/or circumferential stiffness of the cell’s orthotropic composite membrane. In the case of cells transfected with prestin, we vary the membrane areal modulus. As a result, we show the effects of the membrane mechanics on the probabilistic characteristics of prestin-associated charge transfer for both stationary and high-frequency conditions. We compare our computational results with the available experimental data and find good agreement with the experiment.
Keywords: Electromechanical coupling, Membrane moduli, Membrane tension, Protein conformational states, High-frequency electric field
1 Introduction
The protein prestin was originally found in the membrane of the outer hair cells located in the cochlea of the mammalian ear (Zheng et al. 2000). These cells provide the ear with the amplification and sharp frequency selectivity via generating an active force and energy. The outer hair cell has two active sites, the soma (Brownell et al. 1985; Spector et al. 2006 for review) and stereocilia bundle on the cell’s top (Kennedy et al. 2005). Through the somatic activity (termed electromotility), the cylindrical cell converts changes in its membrane potential into length as well as radius changes (Brownell et al. 1985; Dallos et al. 1993). If cell is constrained, its electromotility results in an active force production (Hallworth 1995; Adachi and Iwasa 1997; Liao et al. 2007). The outer hair cell is capable of the electromotile force production up to frequencies of several tens of kHz (Frank et al. 1999). Prestin is a critical part of the motor complex driving outer hair cell electromotility (Zheng et al. 2000; Liberman et al. 2002; Dallos et al. 2008), and it is probably the fastest known membrane protein. The secondary and tertiary structures of this protein are being debated, however, it is commonly assumed that prestin undergoes conformational changes as a result of transfer of an electric charge through the membrane. In the whole cell or membrane patch clamp experiments, this charge is manifested as nonlinear current and nonlinear (voltage-dependent) capacitance (Ashmore 1987; Santos-Sacchi 1989). What is the nature of this charge? Its understanding evolved from purely protein charges (Ashmore 1994) through purely extrinsic chloride ions (Oliver et al. 2001) to the later appreciation that the transferred charge probably includes the interrelated intrinsic prestin charges and intracellular chloride ions (Rybalchenko and Santos-Sacchi 2003; Muallem and Ashmore 2006; Bai et al. 2009; Sun et al. 2009). Coupling (interconnection) of the mechanical and electrical processes is the physical basis of outer hair cell electromotility, and the molecular manifestation of such coupling is the interrelated conformational changes and electric charge transfer. Several modifications (treatments) of membranes associated with changes in their mechanical properties or tension indeed resulted in changes in the charge transferred by prestin and nonlinear capacitance (Kakehata and Santos-Sacchi 1995; Adachi and Iwasa 1997; Dong and Iwasa 2004; Murdock et al. 2005). Thus, the interaction with the surrounding membrane plays an important role in prestin function.
Previously, the mechanical effects on charge transfer in outer hair cells and cells transfected with prestin were analyzed on the basis of 2(3)-state models (Iwasa 1994; Adachi and Iwasa 1997; Adachi et al. 2000) under the conditions of application of a DC electric field. Here, our analysis of the effects of the membrane mechanics considers novel features of prestin-associated charge transfer, including a probabilistic characterization of the charge being at a given position inside the membrane and high frequency effects. While the exact nature of the prestin-associated charge and prestin structure are not fully understood our approach is general: depending on the free energy as a function of the z-coordinate different scenarios can be simulated, including a finite set of the states of the charge. In our consideration, the high-frequency charge is composed of capacitive and resistive components, also a feature that was not considered before our model.
In our modeling of the effects of membrane mechanics, we consider changes in the membrane in-plane moduli and membrane tension. We consider two types of cells: outer hair cell that has a cylindrical shape and an orthotropic membrane and a representative cell transfected with prestin (e.g., HEK cell) whose membrane is assumed to be isotropic and spherical. The changes in the membrane mechanics are introduced via changes in the system’s free energy that enters a Fokker-Planck equation describing the process of charge transfer in our model. We show that the considered changes in the membrane properties can significantly affect the probability of the charge being at a particular position inside the membrane as well as the amplitude of such probability in the DC+AC case. These effects are greatest near the DC-potential value that corresponds to the point of highest sensitivity of the system. In addition to the probability densities, we also consider the total probabilities of charge being transferred. In the case DC fields, we compare the total computed probabilities with the experimental values of the transferred charge and show good qualitative and quantitative agreement with the experiment. The proposed approach can be extended to include other membrane effects such as bending, strain in the direction normal to the membrane, and membrane thickness change. The model can also be used in studies of other membrane proteins, including voltage-gated and mechano-sensitive channels.
2 Model
2.1 Prestin-associated charge transfer
Prestin was found in the membrane of outer hair cells, one of two sensory cells located in the organ of Corti of the mammalian cochlea (upper introductory panel in Fig. 1). While inner hair cells passively convert the information on the mechanics of the cochlear structures into a neural signal going to the brain, outer hair cells, affect the mechanics of these structures by generating an active force and energy. Prestin, a critical part of the outer hair cell motor complex that includes the protein, surrounding membrane, and external ions, undergoes conformational changes associated with electric charge transfer across the membrane. We assume that a system of charges that involves external charges (chloride ions shown as—circles in Fig. 1) and internal protein charges (amino acid residues shown as × circles in Fig. 1) is perturbed by the application of an electric field (changes in the transmembrane potential). Since experiments report charge translocation in terms of a single coordinate across the membrane, z, we will describe the evolution of the prestin-associated charges in terms of its average position as a function of z. The average position of the prestin-associated charges is characterized by a probability density, ρ(z, t), of taking z-coordinate at a moment of time, t (shown as black squares in Fig. 1). This function satisfies a stochastic Fokker-Planck equation (Sun et al. 2009):
| (1) |
where D is the effective diffusion coefficient, kB is the Boltzmann constant, T is the absolute temperature, and V is the free energy of the system. The chloride ions are a necessary part of the charge transfer and prestin conformational change. Thus we supplement Eq. 1 with a kinetic equation of chloride ion-protein binding
Fig. 1.
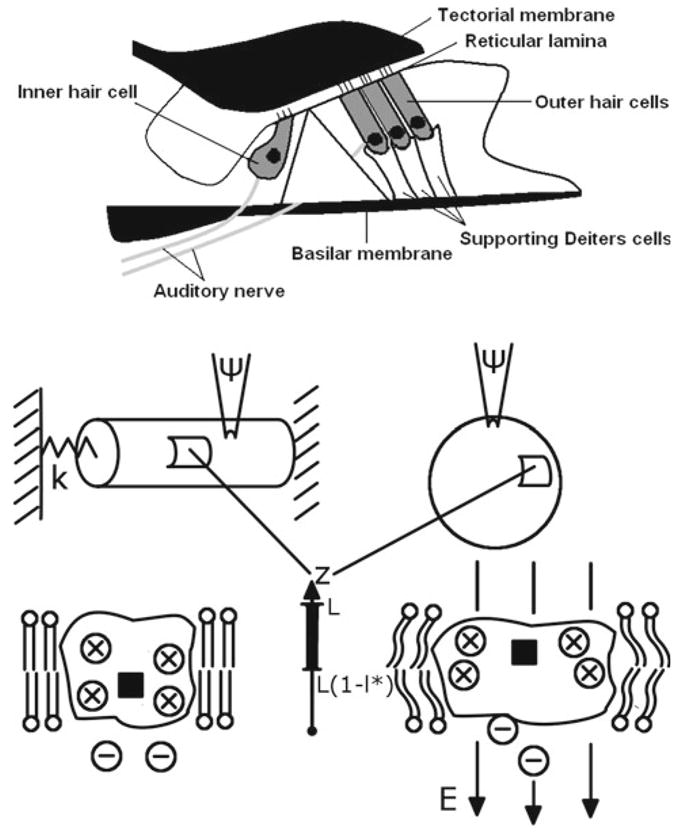
Upper panel: cross section of the organ of Corti where outer hair cells (three rows) are located. This cell affects the mechanics of the cochlear structures, including basilar membrane, tectorial membrane, and reticular lamina. The inner hair cell converts the resulting information on the cochlear mechanics into a neural signal. Middle panel: electrically stimulated cylindrical outer hair cell (left) and spherical prestin-transfected cell (right). The spring of a stiffness, k, attached to the outer hair cell represents constraints imposed on the cell. The details of the processes inside the cellular membranes in both cases are shown via representative cuts. Lower panel: prestin in two, “short” (left) and “expanded” (right) states surrounded by the membrane. The molecular-level electromechanical coupling occurs via the deformation of the membrane due to the protein conformational change and via the changes in the probabilities of charge position due to the mechanical contribution to the electromechanical driving force. The model is in terms of the average position of the charge system (black square) that includes chloride ions (– circles) and internal protein charges (× circles). The charges are driven by electric field, E, and the average position moves along the z-axis across the membrane where L is the thickness of the membrane. The charge is considered transferred when it reaches the upper part of the protein, of a relative thickness, l*
| (2) |
where P is the probability of chloride ion binding and k1 and k−1 are the attachment and detachment rate constants. The boundary conditions for Eq. 1 are formulated in terms of flux, J, where
| (3) |
We assume that
The first of the above boundary conditions ensures the continuity of flux at the point of chloride ion binding, and the second condition assumes that there is no charge transport outside the protein. Thus Eqs. 1 and 2 are related through the first boundary condition at z = 0. The second boundary condition at z = L can be easily modified if experimental evidence for prestin transferring charge through the membrane becomes available. We assume that prestin undergoes a conformational change when the charge (average position of the charge system) reaches an upper part of the protein of fractional length, l* (Fig. 1). Thus, this event is characterized by a probability, P*,
In general case, P* is a function of time. Below we consider DC and AC+DC electric fields applied across the protein, and we use constant limiting P* (its amplitude) that corresponds to the steady state (steady oscillation) regime of charge transfer. As it was previously shown (Sun et al. 2009), the solution of the system (1), (2) in the AC + DC-case has an intrinsic feature of a phase shift (delay) with respect to the applied electric field. The phase shift is determined by the effective diffusion coefficient, and it increases with frequency. Such phase shift constitutes two, capacitive (in-phase with the applied field) and resistive (at 90° to the applied field) components of the transferred charge.
2.2 Free energy of the system
The main focus of the consideration below is the effect of changes in the membrane elastic (in-plane) properties or membrane tension on charge transfer by prestin. Mathematically, this effect is analyzed via the corresponding changes in the z-gradient of the free energy of the system, which is the charge driving force in Fokker-Planck equation (1). We assume that the free energy of the system, V, can be presented as the sum of electrical and mechanical components
The electrical component of the free energy is determined by the externally applied electric field as well as by the field generated by the system of involved charges, extrinsic ions, internal protein charges, membrane surface charges, etc., but we limit our consideration to the contribution of the applied electric field. We approximate the z-gradient of the electrical energy that enters Eq. 1 as
where q is a typical charge and Ψ is the applied transmembrane potential. We associate the elastic component of the free energy with the energy accumulated in the elastic membrane surrounding prestin as a result of the conformational change in the protein. We approximate the z-gradient of this component of the free energy as
| (4) |
where ΔVmech is the increment in the membrane elastic energy related to a conformational change of prestin. Note, that such approximation of the z-gradient of the free energy allows us not to consider the intermediate strains in the surrounding membrane that correspond to the changing position of the charge moving along the z-axes. Instead, we can use the ultimate membrane strain associated with the protein conformational change, and this strain corresponds to the fully transferred charge. Note also that while we can neglect the intermediate positions of the charge in the consideration of the free energy gradient, all these positions are under consideration when we analyze the effect of membrane mechanical changes of the electric charge.
In the case of changes in the elastic moduli of the membrane, the mechanical energy in Eq. 4 is a quadratic function of the strains generated in the membrane by conformational changes of prestin. The coefficients in this function are determined by the elastic moduli of the membrane. In the case of variation of the membrane tension, the mechanical energy is a linear function of the change in the membrane area caused by the conformational change of prestin. The coefficients in this linear function are determined by the membrane tension. Below, we consider several examples of changes in the membrane properties coming from experiments or in vivo models of prestin performance, derive the corresponding changes in the free energy (its mechanical component), and estimate the resulting effect on the transferred electric charge.
2.3 Mechanical component of the free energy and outer hair cell membrane moduli
Here we consider the outer hair cell case and find how the elastic energy accumulated in the membrane due to the conformational change of an individual prestin molecule depends on the membrane elastic moduli. The outer hair cell membrane is a tri-layer composite that can be effectively described by a linear orthotropic model (Tolomeo and Steele 1995; Spector et al. 1998, 2002). Note that prestin molecules distributed along the cylindrical surface of the outer hair cell also contribute to the effective mechanical properties of the composite outer hair cell membrane. The elastic energy that we seek depends on membrane/cell constraints, and a sketch of such constraints for the outer hair is shown in Fig. 1. The model used here can be applied to the in vivo case where the cell is constrained by the cochlear structures (Fig. 1) as well as the experiment to measure the cell active force where the cell is stimulated electrically and interacts with an elastic fiber. The total (observable) strain ε(εx, εθ) can be presented as the sum of two parts, and associated with the membrane and prestin, respectively. Then the force balance equations for an orthotropic cylindrical cell in terms of the membrane strains take the form
Here Δp is reactive pressure occurring due to incompressibility of the cell’s liquid core when the cell deforms under the action of the applied electric field, k is the stiffness of the constraint (for the simplicity, we neglect here the viscous and inertial properties of the constraints), and R is the radius of the cylindrical cell. The volume preservation of the cylindrical cell yields to the condition of εθ = −0.5εx. If we use convenient asymptotic formulas for the membrane moduli (Spector et al. 1998) then
| (5) |
| (6) |
where the dimensionless parameter, γ, is defined as
| (7) |
The numerator in Eq. 7 for γ is proportional to the longitudinal cell stiffness, and this parameter is small within a realistic range of the cell stiffness. As a result, the membrane strains can be expressed in terms of the prestin-associated strain by the following equations
| (8) |
| (9) |
where α = k/γCθθ.
For the simplicity, we use a relationship between two components of the prestin-related strain that was observed in the microchamber experiment with outer hair cell electrical stimulation (Dallos et al. 1993; Hallworth et al. 1993). This experiment is suitable to extract the circumferential component of the prestin-associated strain or its ratio to the longitudinal one. First, this is the only available experiment that provides both the length and radius changes of the electromotile cell. Also, the liquid volumes of the cell’s parts inside and outside of the microchamber are not constrained, and the reactive pressure and tension are relatively small. Thus in this case, the observable strain can be interpreted as the active strain (e.g., Tolomeo and Steele 1995; Spector and Jean 2004). The prestin-associated strain used in our model can be extracted from the active strain that corresponds to the conditions of extreme negative and positive voltages. When the electric field changes between these extremes all prestin molecules located along the cell (membrane) surface fully transfer their electric charges and undergo conformational changes. We use the data of Dallos et al. (1993) that correspond to the equal lengths of included and excluded parts of the cell to extract the ratio of two components of the prestin-associated strain. We found this ratio being close to . It should be noted that not all the data of Dallos et al. (1993) are from the direct experimental measurements. However, we interpret them as an effective analytical extrapolation that is based on numerous direct measurements (Dallos et al. 1993; Hallworth et al. 1993) of the electromotile diameter changes for various voltages.
The surface density of the elastic energy accumulated as a result of changes in the membrane moduli, Cxx, Cxθ, and Cθθ, is expressed by the equation
| (10) |
Taking into account Eqs. 5–6 and 8–9, the energy density in Eq. 10 can be presented as
| (11) |
where
The free energy change is the product of the energy density, ΔV̂mech, and a characteristic membrane surface area, Sm, involved in the deformation due to the conformational change of an individual prestin molecule. Assuming (for the simplicity) that the prestin molecules are circular with a radius of 10 nm and an edge-to-edge distance of 3 nm, such membrane area can be estimated as 100 nm2. In this case, the prestin molecules occupy about 50% of the area in the outer hair cell membrane which is quite reasonable for the estimated densities of prestin. The longitudinal component of the prestin-associated strain can also be extracted from the microchamber experiment (Dallos et al. 1993). This strain corresponds to the experimental regime of the limiting cell length change when the applied voltage changes from extreme depolarization to extreme hyperpolarization. Thus, the prestin-related longitudinal strain can be estimated as . Dimensional changes in the outer hair cell prestin caused by prestin conformational change have previously been estimated by Dong and Iwasa (2004). The above value of extracted from the microchamber experiment is consistent with the calculation of this strain as the ratio of the longitudinal dimensional change of prestin to the reference dimension of the molecule based on the results of Dong and Iwasa (2004). With the obtained estimates of the membrane area per prestin and of the prestin-related strain, the free energy (mechanical component) becomes a function of two membrane elastic constants, Cxx − Cxθ + 0.25Cθθ = γCθθ and Cθθ, that characterize the longitudinal and circumferential stiffness of the cell and the membrane. In addition, the free energy depends on k, the stiffness of the constraints. The dependence of the free energy on the outer hair cell membrane moduli is presented in Fig. 2. There the free energy is plotted as a function of the two combinations of membrane elastic moduli for three different values of the stiffness of the constraint that is much higher than (Fig. 2, upper panel), close to (Fig. 2, middle panel), and much lower than (Fig. 2, lower panel) than that of the cell.
Fig. 2.

The changes in the free energy of the prestin system due to changes in the orthotropic properties of the outer hair cell membrane. The changes in the free energy are associated with the changes in the elastic energy accumulated in the membrane surrounding prestin as a result of its conformational state. The free energy change is a function of the longitudinal, Cxx − Cxθ +0.25Cθθ, and circumferential, Cθθ, stiffness where Cxx, Cxθ, and Cθθ are the orthotropic moduli of the composite membrane. The results are presented for three, 1 × 10−3, 7× 10−3, and 30 × 10−3 N/m, values of the stiffness of the constraint, k
2.4 Free energy and outer hair cell membrane tension
The cylindrical outer hair cell is under the action of turgor pressure, and we assume that changes in the cell membrane tension are caused by changes in the cell turgor pressure. Thus the mechanical component of the free energy associated with changes in the longitudinal, and circumferential, , components of membrane tension (turgor pressure, p,) is expressed by the following equation
Based on previous estimates (Dong and Iwasa 2004) of the components of the membrane area change in the outer hair cell due to prestin conformational change and direct comparison with experimental data (see below), we use and .
2.5 Free energy and membrane mechanics of cells transfected with prestin
We consider a membrane of an incompressible prestin-transfected cell of spherical shape and assume that its tension changes. We are interested in what would be the corresponding change in the elastic energy accumulated in the membrane surrounding an individual prestin molecule in response to its conformational change. The electrical stimulation does not change the volume of the spherical cell, which means that its surface area does not change either. This is true for any tension (pressure). It means that Sm = −Spr where Sm and Spr are the surface area changes in the membrane and prestin, respectively, due to the molecule conformational change. Thus, the changes in the free energy of the prestin system can be expressed as
where tm (p) is the tension (pressure) change, and R is the radius of the spherical membrane.
If a spherical membrane undergoes changes in its elastic properties (areal expansion modulus K) then the corresponding free energy density takes the form
| (12) |
where S0 is the membrane area associated with a single prestin molecule. Thus, the total energy can be determined by the following equation
| (13) |
3 Results
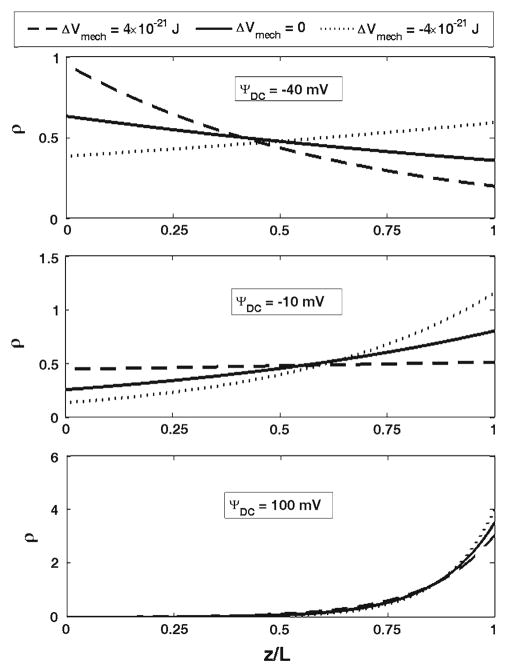
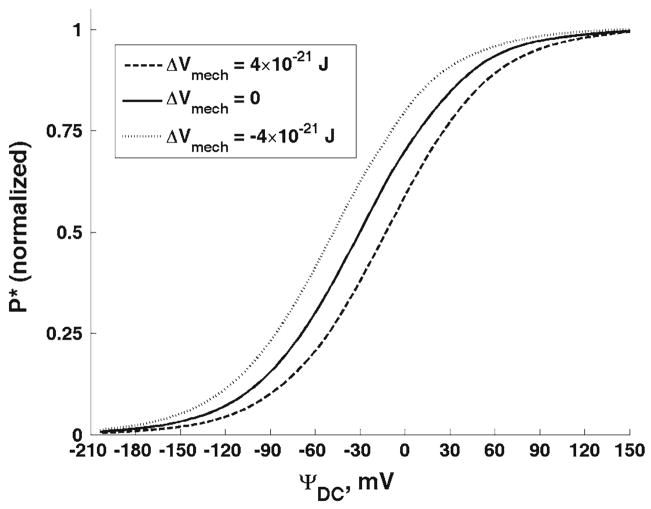
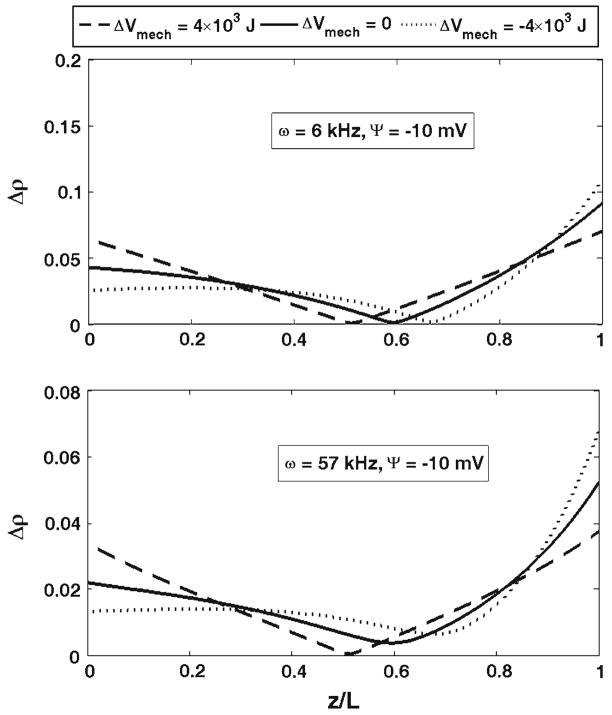
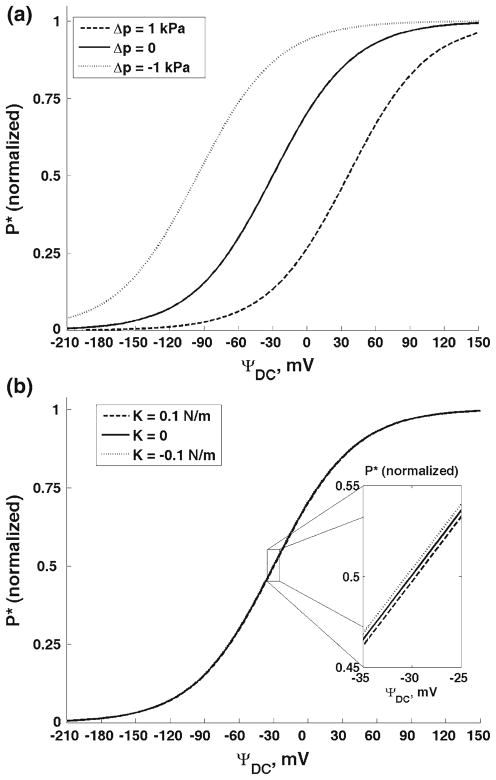
First, we present the results for the outer hair cell cylindrical membrane. Figure 3 shows the effect of changes in the membrane elastic moduli on the probability density of the charge being at a position, z, and at a moment of time, t. The considered charge is moved by a DC electric field. The results in Fig. 3 are presented for three different values of the DC potential, Ψ = −10, 40, and 100 mV, respectively. Previously (Fig. 2), we showed the free energy change as a result of changes in the longitudinal and circumferential stiffness of the cell composite membrane. Here, we consider two changes in the free energy resulting in its increase by 4 × 10−21 J (dashed lines in Fig. 3) or its decrease by the same value (dotted lines). Such free energy increase can be achieved, for example, by a simultaneous increase in the longitudinal, from 0.001 to 0.012 N/m, and circumferential, from 0.02 to 0.5 N/m, stiffness of the membrane (Fig. 2). The solid lines in Fig. 3 correspond to the reference state without perturbation of the elastic properties of the membrane. In Fig. 4, we present the total probabilities, P*, (normalized) of the charge being transferred as a function of DC voltage. We also consider the effect of the membrane mechanics on charge transfer under the action of an AC+DC field. In Fig. 5, we present the amplitudes of the probability density, Δρ(z, t), for two different frequencies, 6 and 57 kHz (upper and lower panels, respectively). The results in Fig. 5 are given for the same DC potential, Ψ = −10 mV. Here we consider the same perturbations in the mechanical properties of the composite membrane as those above in Figs. 3 and 4. Finally in Fig. 6, we present the amplitudes of the total probability, ΔP*, as a function of the DC component of the electric field for the same two frequencies. The amplitudes are normalized by their low-frequency value. The presented curves for each frequency show the results for the same increase and decrease in the free energy that we considered for the purely DC field in Figs. 3 and 4. Figure 7 presents the results of our study of the effect of tension changes in the outer hair cell cylindrical membrane. We assume that tension changes via changes in the cell turgor pressure by its increase and decrease by 1 kPa (shown, respectively, in dashed and dotted lines in Fig. 7a). The results corresponding to the unperturbed pressure are given as a solid line. Here we consider the DC-field case and show the total probability, P*, of charge being transferred. Figure 7b shows a comparison of our computational results for the total probability in the purely DC case with the available experimental data.
Fig. 3.
The probabilities, ρ, of the charge being at z (dimensionless)-position inside the protein for two changes in the electromechanical driving force (free energy of the system), 4×10−21 (dashed lines) and −4 × 10−21 J (dotted lines). The solid lines correspond to the unperturbed state of the membrane. The set of three curves is presented for three values of the DC-potential, −40, −10, and 100 mV
Fig. 4.
The total (normalized) probability of the charge being transferred as a function of the DC potential. The free energy change due to changes in the membrane orthotropic properties are 4 × 10−21 (dashed line) and −4 × 10−21 J (dotted line). The solid line corresponds to the unperturbed case
Fig. 5.
The amplitudes of the probability of the charge being at the position z (dimensionless) for two frequencies, 6 and 57 kHz for the same DC potential of −10 mV. The results are presented for the changes in the free energy equal to 4 × 10−21 (dashed lines) and −4 × 10−21 J (dotted lines). The solid lines correspond to the unperturbed case
Fig. 6.
The amplitude of the total probability as a function of the DC potential for two frequencies, 6 (in black) and 57 kHz (in red). The dashed and dotted lines correspond, respectively, to changes in the free energy equal to 4 × 10−21 and −4 × 10−21 J. The solid lines correspond to the unperturbed case
Fig. 7.
a The total probability of the charge being transferred (normalized) as a function of the DC-potential for two changes in the outer hair cell turgor pressure, 1 (dashed line) and −1 kPa (dotted line). The solid line corresponds to the unperturbed case. b Comparison with experimental data: open and black circles are measurements of Adachi et al. (2000) and Kakehata and Santos-Sacchi (1995), respectively, and crosses are our computational results approximated by the solid line
Finally, we consider the effect of changes in tension (Fig. 8a) and elastic properties (Fig. 8b) in membranes of spherical prestin-transfected cells. Figure 8a shows the total (normalized) probability, P*, as a function of the DC potential for an increase (dashed line) and decrease (dotted line) in turgor pressure by 1 kPa. The curve corresponding to the unperturbed cell is presented as a solid line. Based on Dong and Iwasa (2004), we use Spr ≈ 2 nm2 and the radius of the cell (membrane), R ≈ 15 μm. We assume that cells transfected with prestin have an isotropic membrane that under the condition of pressure changes can be characterized by a single area expansion modulus, K. In Fig. 8b, we consider the effect of changes in this modulus by its increase and decrease by 0.1 N/m. To compute the free energy change, we use the value of the membrane area per prestin, Sm ≈ 1, 000 nm2, which was calculated assuming the prestin density of about 1/10 of that in its native outer hair cell (Dong and Iwasa 2004).
Fig. 8.
a The total probability of the charge being transferred (normalized) as a function of the DC-potential for two changes in turgor pressure in the spherical prestin-transfected cell, 1 (dashed line) and −1 kPa (dotted line). The solid line corresponds to the unperturbed case. b The total probability of the charge being transferred (normalized) as a function of the DC-potential for two changes in the membrane areal modulus, 0.1 (dashed line) and −0.1 N/m (dotted line). The solid line corresponds to the unperturbed case. The three lines almost overlap, and the insert shows a slight difference in the highest sensitivity region
4 Discussion
4.1 Prestin-level model vs. continuum model
The dependence of prestin-associated charge transfer and nonlinear capacitance on the mechanics of the surrounding membrane is a manifestation of electromechanical coupling, a fundamental aspect of the outer hair cell motor complex and this cell’s active properties. The phenomenon of electromotility can be effectively described by continuous nonlinear or linearized piezoelectric relations (e,g., Tolomeo and Steele 1995). In continuum relations, the electrical displacement (charge per unit area) depends on the local strains. Here we develop a prestin-level model and analyze a similar effect of the mechanics of the surrounding membrane on the prestin-associated charge.
4.2 Effect of the membrane mechanical properties
We found that the changes in the membrane mechanical properties or its tension have a significant effect on the process of electric charge transfer by prestin. In our model this process is described by the probability density (its amplitude) of the charge being at a given position at a particular moment of time. In the DC-case, it is convenient to use the probability densities corresponding to the steady state of the system (Fig. 3). The effect of the outer hair cell membrane mechanics (Fig. 3) is mainly determined by two factors: the closeness of the DC-potential to the point of highest sensitivity and the sign of the change in the mechanical component of the free energy. The electric charge measured in isolated outer hair cells as a function of voltage has the point of highest sensitivity close to −30 mV (e.g., Gale and Ashmore 1997a). In our model, the physical charge is represented by the probability density and the total probability of charge being transferred. Previously, Sun et al. (2009) adjusted the parameters of the solution of the Fokker-Planck equation to reflect that experimentally observed point of the highest sensitivity. The effect is most pronounced if the applied DC-potential is close to the point of highest sensitivity: compare changes due to the same amount of free energy change in the cases of ΨDC = −10 mV and ΨDC = 100 mV. Interestingly in all cases, there is a point inside the membrane (z-position) that changes the response of the local value of the probability density to a given increment in the free energy. This particular z-position is determined by the value of the DC-potential, ΨDC. Thus, the probability density does not uniformly follow the changes in the mechanical component of the free energy. If this free energy increases the probability density decreases at all points above the point of separation. The change in the probability density is in the opposite direction at all points below the point of separation. Thus, the changes in the outer hair cell membrane mechanical properties results in an increase in the probability of the charge being in certain part of the protein, and in a decrease in that probability for the its other part. We also consider the effect of the membrane mechanics in the DC+AC case. The corresponding results are presented in Fig. 5 in terms of the amplitude of the probability density. Regarding the frequency effect, the probability density almost uniformly decreases about two times when the frequency increases from 6 to 56 kHz. This is consistent with our previous analysis with purely electrical component of the free energy (Sun et al. 2009). The phenomenon of a decrease in the transferred charge for higher frequencies is associated with the finite speed of the charge moving through prestin and a delay in charge transfer relative to the applied field. Previously, a frequency decrease in outer hair cell nonlinear capacitance was measured experimentally (Gale and Ashmore 1997b), and it was also described using two-(three) state models (Iwasa 1997). How the frequency-associated decrease in the transferred charge depends on a particular position of the outer hair cell in the cochlea has not been investigated. In terms of Δρ-distribution across the membrane, there is an internal point where the amplitude is close to zero with further increase toward to bottom and top of the protein (membrane). A detailed analysis (not presented) shows that in these two characteristic areas the probability density oscillates in opposite directions. This is consistent with the pattern for the DC-case where the probability density increases above and decreases below a separation point in response to an increase in the DC-potential (compare the solid-line distributions in the cases of −40, −10, and 100 mV in Fig. 3).
In addition to the local characteristics of charge transfer, we analyze the effect of the membrane mechanics on the total probability of the charge being transferred (Fig. 4). In the DC-case, the increase in the mechanical component of the free energy results in a uniform shift of the curve toward more negative potentials (hyperpolarization direction). A decrease in the free energy results in a shift in the depolarization direction. Such pattern means that a decrease (increase) in the mechanical component of the free energy causes a uniform decrease (increase) in the total probability of the charge being transferred. Similar shifts were found in the amplitude of the total probability for both frequencies in Fig. 6. Note that the total probability in Fig. 6 reflects both capacitive and resistive components of the transferred charge (Sun et al. 2009).
The experimental information on the effect of changes in the mechanical properties of the orthotropic outer hair cell membrane is limited. The diamide treatment (Adachi and Iwasa 1997) of the outer hair cell destroys the connections between the spectrin and actin fibers in the cytoskeleton, changing the composite membrane elastic properties. It has been shown that the longitudinal stiffness of the cell decreases with increasing concentration of diamide. Although the resulting changes in nonlinear capacitance or transferred charge have not been reported, there are results for electromotility of unconstrained cells. Electromotility (whose behavior is normally similar to that of nonlinear capacitance) exhibits a shift toward the hyperpolarization direction in response to cell treatment with diamide. Our model predicts that a decrease in the longitudinal stiffness of the cell shifts the P*-probability, that represents the transferred charge, in the depolarization direction. This qualitative result is fully consistent with the experimental data of the diamide treatment. Quantitatively, Adachi and Iwasa (1997) reported a shift of about 37 mV in electromotility of unconstrained cells treated with 3 mM concentration of diamide. This treatment results in an about three-time decrease in the cell longitudinal stiffness. The computed here P*-shift that corresponds to a three-time decrease in the parameter Cxx − Cxθ + 0.25Cθθ is to some extent smaller, but this discrepancy can be explained by a difference between the shift in the charge and that in electromotility as well as by an additional effect that the diamide treatment can have on the circumferential stiffness of the membrane.
In addition to the effect of changes in the outer hair cell in-plane moduli, we also consider changes in the mechanical properties of the cells transfected with prestin. Since we assume that membranes of such cells are isotropic the only elastic modulus describing the membrane in-plane elastic properties is the areal modulus, K. The estimates of this parameter under normal conditions are 0.16 N/m for a pure lipid bilayer, 0.45 N/m, for the red blood cell membrane (Evans and Skalak 1980), and 0.07 N/m for the outer hair cell membrane (Iwasa and Chadwick 1992). Although in the two latter cases the membranes are composite, the outermost plasma membrane is probably responsible for the effective areal modulus. The estimate for the lipid bilayer is probably most relevant to this study. Thus, we consider an increase and decrease in this modulus by 0.1 N/m. We found a very small effect of such changes on the charge transfer. This small change is enlarged in the insert in Fig. 8 that corresponds to the voltage range of the highest sensitivity of the total probability, P*.
4.3 Effect of membrane tension. Comparison with the experiment
We also analyze the effects of changes in the membrane tension in the cylindrical (outer hair cell) and spherical (cell transfected with prestin) membranes. We assume that tension is changed via changes in cell turgor pressure. The resulting voltage dependence of P* shows shifts in the depolarization (hyperpolarization) direction if turgor pressure increases (decreases). This shift is about 25 mV per unit turgor pressure change in the cylindrical membrane (Fig. 7a), and it is about 61 mV/kPa in the spherical membrane (Fig. 8a). The tension effect due to changes in cell turgor pressure has been studied experimentally (Kakehata and Santos-Sacchi 1995; Adachi et al. 2000; Dong and Iwasa 2004) and theoretically (Adachi et al. 2000; Dong and Iwasa 2004). It is important here to compare our computational results on the tension effect in the purely-DC case with the available experimental data. Our computational probability, P*, exhibits shifts similar to those observed in the experimental charge and nonlinear capacitance. The experimental data have also shown a slight decrease in the magnitude of the capacitance as a function of pressure. We do not discuss this effect here because we present the normalized values of the probability, P*. To make a quantitative comparison with experimental data, we combine in Fig. 7b the data from two groups (Kakehata and Santos-Sacchi 1995 and Adachi et al. 2000; black and open circles, respectively). Figure 7b shows good qualitative and quantitative agreement of our results (solid line as a linear approximation) and the experimental data. In addition, Dong and Iwasa (2004) analyzed the turgor pressure (tension) effect in prestin-transfected HEK cells. In order to study the purely membrane effect, the cells were treated with trypsin that destroyed the cell cytoskeleton. Our computational result for spherical cells under variable turgor pressure is about 61 mV/kPa which is quite close to the experimental shift in experimental nonlinear capacitance of about 59 mV/kPa.
4.4 Other membrane effects
Some effects of the membrane mechanics on electric charge transfer by prestin are not associated with the in-plane mechanical properties or tension, and their explanation requires a modification of the current version of the model. One of such examples is the treatment of prestin-containing membranes with chlorpromazine. Lue et al. (2001) observed an about 20 mV shift of outer hair cell nonlinear capacitance in the depolarization direction in outer hair cells treated with chlorpromazine. On the other hand, Murdock et al. (2005) probed the outer hair cell membrane mechanical properties pulling membrane tethers and measuring the equilibrium tether holding force. This force decreases with the application of chlorpromazine. These results can not be explained from the standpoint of changes of membrane in-plane properties or tension. However, these results could be consistent with an effect on other membrane mechanical properties. Chlorpromazine is known to affect the membrane bending properties (Sheetz and Singer 1976) and the above observations are consistent with changes in the membrane bending modulus. Indeed, the tether holding force is proportional to the square root of the membrane bending modulus (Derényi et al. 2002). Thus, a chlorpromazine-related decrease in the membrane bending modulus can result in decreases in the tether force, and it could also cause the observed shift in nonlinear capacitance toward the depolarization direction. Another example of this type is the modulation of the membrane properties via changes the concentration of cholesterol. It has been shown that nonlinear capacitance in membranes with prestin shifts up to 100 mV in the depolarization direction in response to loading the membranes with cholesterol (Rajagopalan et al. 2007; Sfondouris et al. 2008). It was also shown (Nguyen and Brownell 1998) that such treatment is associated with an increase in the membrane stiffness. This is also inconsistent with changes in in-plane membrane moduli or tension and points out at other mechanisms involved. In addition, the damping in the membrane can affect prestin-associated charge transfer under high-frequency conditions. Such phenomenon can be analyzed by introducing a bi-directional coupling where the transferred charge is modulated by the time-dependence of free energy and the time-dependent mechanics of the surrounding membrane charge, in turn, is affected by the prestin charge. Our approach is general and it can be used for the analysis of various mechanical effects, such as membrane bending, thinning, and viscosity as well as effects of non-mechanical nature by incorporation of the corresponding components in the free energy of the system.
5 Conclusions
The proposed model describes the effects of the membrane mechanics on fine properties of electric charge transfer by the protein prestin, including the probability density of charge being at a given position inside the protein and its amplitude in the case of DC+AC-fields. This analysis covers high-frequency regimes that are characteristic to this protein that is functional throughout the whole frequency range. The elastic moduli of the outer hair cell orthotropic membrane can have a significant effect on electric charge transferred by prestin, especially at DC-voltages close to the point of the highest sensitivity of the system. In isotropic membranes of cells transfected with prestin, a variation of the area modulus has a little effect on the transferred charge. Changes in membrane tension can affect transfer of the electric charge significantly both in outer hair cells and cells transfected with prestin. A comparison of the tension-caused shifts in the total probability of charge being transferred show good qualitative and quantitative agreement with the available experimental data for the transferred charge and nonlinear capacitance. The current model can be extended to include other properties of the membrane such as bending modulus, membrane stiffness in the normal direction, and membrane thickness.
Acknowledgments
This research was supported by research grants DC 002775 and DC 000354 from National Institute on Deafness and Other Communication Disorders (NIH). We thank Dr. Aleksander Popel for the discussion of the obtained results.
Contributor Information
Natalie Nilsen, Department of Biomedical Engineering, Johns Hopkins University, 720 Rutland Ave, Traylor 411, Baltimore, MD 21205, USA.
William E. Brownell, Bobby A. Alford Department of Otolaryngology, Head & Neck Surgery, Baylor College of Medicine, Houston, TX 77030, USA
Sean X. Sun, Department of Biomedical Engineering, Johns Hopkins University, 720 Rutland Ave, Traylor 411, Baltimore, MD 21205, USA. Department of Mechanical Engineering, Johns Hopkins University, Baltimore, MD 21218, USA
Alexander A. Spector, Email: aspector@jhu.edu, Department of Biomedical Engineering, Johns Hopkins University, 720 Rutland Ave, Traylor 411, Baltimore, MD 21205, USA. Department of Mechanical Engineering, Johns Hopkins University, Baltimore, MD 21218, USA
References
- Adachi M, Iwasa KH. Effect of diamide on force generation and axial stiffness of the cochlear outer hair cell. Biophys J. 1997;73:2809–2818. doi: 10.1016/S0006-3495(97)78310-3. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Adachi M, Sugawara M, Iwasa KH. Effect of turgor pressure on outer hair cell motility. J Acoust Soc Am. 2000;108:2299–2306. doi: 10.1121/1.1314396. [DOI] [PubMed] [Google Scholar]
- Ashmore JF. A fast motile response in guinea-pig outer hair cells: the cellular basis of the cochlear amplifier. J Physiol. 1987;388:323–347. doi: 10.1113/jphysiol.1987.sp016617. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Ashmore JF. The G.L. Brown prize lecture. The cellular machinery of the cochlea. Exp Physiol. 1994;79:113–134. doi: 10.1113/expphysiol.1994.sp003746. [DOI] [PubMed] [Google Scholar]
- Bai J-P, Surguchev A, Montoya S, Aronson PS, Santos-Sacchi J, Navaratnam D. Prestin’s anion transport and voltage-sensing capabilities are independent. Biophys J. 2009;96:3179–3186. doi: 10.1016/j.bpj.2008.12.3948. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Brownell WE, Bader CR, Bertrand D, de Ribaupierre Y. Evoked mechanical responses of isolated cochlear outer hair cells. Science. 1985;227:194–196. doi: 10.1126/science.3966153. [DOI] [PubMed] [Google Scholar]
- Dallos P, Hallworth R, Evans BN. Theory of electrically driven shape changes of cochlear outer hair cells. J Neurophysiol. 1993;70:299–323. doi: 10.1152/jn.1993.70.1.299. [DOI] [PubMed] [Google Scholar]
- Dallos P, Wu X, Cheatham MA, Gao J, Zheng J, Anderson CT, Jia S, Wang X, Cheng WHY, Sengupta S, He DZZ, Zuo J. Prestin-based outer hair cell motility is necessary for mammalian cochlear amplification. Neuron. 2008;58:333–339. doi: 10.1016/j.neuron.2008.02.028. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Derényi I, Jülicher F, Prost J. Formation and interaction of membrane tubes. Phys Rev Lett. 2002;88:238101. doi: 10.1103/PhysRevLett.88.238101. [DOI] [PubMed] [Google Scholar]
- Dong XX, Iwasa KH. Tension sensitivity of prestin: comparison with the membrane motor in outer hair cells. Biophys J. 2004;86:1201–1208. doi: 10.1016/S0006-3495(04)74194-6. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Evans EA, Skalak R. Mechanics and thermodynamics of bio-membranes. CRC; Boca Raton: 1980. [Google Scholar]
- Frank G, Hemmert W, Gummer AW. Limiting dynamics of high-frequency electromechanical transduction of outer hair cell. Proc Natl Acad Sci. 1999;96:4420–4425. doi: 10.1073/pnas.96.8.4420. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gale JE, Ashmore JF. The outer hair cell motor in membrane patches. Pflugers Arch Eur J Physiol. 1997a;434:267–271. doi: 10.1007/s004240050395. [DOI] [PubMed] [Google Scholar]
- Gale JE, Ashmore JF. An intrinsic frequency limit to the cochlear amplifier. Nature. 1997b;389:63–66. doi: 10.1038/37968. [DOI] [PubMed] [Google Scholar]
- Hallworth R. Passive compliance and active force generation in the guinea pig outer hair cell. J Neurophysiol. 1995;74:2319–2328. doi: 10.1152/jn.1995.74.6.2319. [DOI] [PubMed] [Google Scholar]
- Hallworth R, Evans BN, Dallos P. The location and mechanism of electromotility in guinea pig outer hair cell. J Neurophysiol. 1993;70:549–558. doi: 10.1152/jn.1993.70.2.549. [DOI] [PubMed] [Google Scholar]
- Iwasa KH. A membrane motor model for the fast motility of the outer hair cell. J Acoust Soc Am. 1994;96:2216–2224. doi: 10.1121/1.410094. [DOI] [PubMed] [Google Scholar]
- Iwasa KH. Current noise spectrum and capacitance due to the membrane motor of the outer hair cell: theory. Biophys J. 1997;73:2965–2971. doi: 10.1016/S0006-3495(97)78325-5. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Iwasa KH, Chadwick RS. Elasticity and active force generation of cochlear outer hair cells. J Acoust Soc Am. 1992;92:3169–3173. doi: 10.1121/1.404194. [DOI] [PubMed] [Google Scholar]
- Kakehata S, Santos-Sacchi J. Membrane tension directly shifts voltage dependence of outer hair cell motility and associated gating charge. Biophys J. 1995;68:2190–2197. doi: 10.1016/S0006-3495(95)80401-7. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kennedy HJ, Crawford AC, Fettiplace R. Force generation by mammalian hair bundles supports a role in cochlear amplification. Nature. 2005;433:880–883. doi: 10.1038/nature03367. [DOI] [PubMed] [Google Scholar]
- Liao Z, Feng S, Popel AS, Brownell WE, Spector AA. Outer hair cell active force generation in the cochlear environment. J Acoust Soc Am. 2007;122:2215–2225. doi: 10.1121/1.2776154. [DOI] [PubMed] [Google Scholar]
- Liberman MC, Gao J, He DZZ, Wu X, Jia S, Zuo J. Prestin is required for electromotility of the outer hair cell and for the cochlear amplifier. Nature. 2002;419:300–304. doi: 10.1038/nature01059. [DOI] [PubMed] [Google Scholar]
- Lue AJC, Zhao HB, Brownell WE. Chlorpromazine alters outer hair cell electromotility. Otolaryngol Head Neck Surg. 2001;125:71–76. doi: 10.1067/mhn.2001.116446. [DOI] [PubMed] [Google Scholar]
- Muallem D, Ashmore J. An anion antiporter model of prestin, the outer hair cell motor protein. Biophys J. 2006;90:4035–4045. doi: 10.1529/biophysj.105.073254. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Murdock DR, Ermilov SA, Spector AA, Popel AS, Brownell WE, Anvari B. Effects of chlorpromazine on mechanical properties of the outer hair cell plasma membrane. Biophys J. 2005;89:4090–4095. doi: 10.1529/biophysj.105.069872. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Nguyen TV, Brownell WE. Contribution of membrane cholesterol to outer hair cell lateral wall stiffness. Otolaryngol Head Neck Surg. 1998;119:14–20. doi: 10.1016/S0194-5998(98)70167-6. [DOI] [PubMed] [Google Scholar]
- Oliver D, He DZZ, Klöcker N, Ludwig J, Schulte U, Waldegger S, Ruppersberg JP, Dallos P, Fakler B. Intracellular anions as the voltage sensor of prestin, the outer hair cell motor protein. Science. 2001;292:2340–2343. doi: 10.1126/science.1060939. [DOI] [PubMed] [Google Scholar]
- Rajagopalan L, Greeson JN, Xia A, Liu H, Sturm A, Raphael RM, Davidson AL, Oghalai JS, Pereira FA, Brownell WE. Tuning of the outer hair cell motor by membrane cholesterol. J Biol Chem. 2007;282:36659–36670. doi: 10.1074/jbc.M705078200. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Rybalchenko V, Santos-Sacchi J. Cl− flux through a non-selective, stretch-sensitive conductance influences the outer hair cell motor of the guinea-pig. J Physiol. 2003;547:873–891. doi: 10.1113/jphysiol.2002.036434. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Santos-Sacchi J. Asymmetry in voltage-dependent movements of isolated outer hair cells from the organ of corti. J Neurosci. 1989;9:2954–2962. doi: 10.1523/JNEUROSCI.09-08-02954.1989. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Sfondouris J, Rajagopalan L, Pereira FA, Brownell WE. Membrane composition modulates prestin-associated charge movement. J Biol Chem. 2008;283:22473–22481. doi: 10.1074/jbc.M803722200. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Sheetz MP, Singer SJ. Equilibrium and kinetic effects of drugs on the shapes of human erythrocytes. J Cell Biol. 1976;70:247–251. doi: 10.1083/jcb.70.1.247. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Spector AA, Brownell WE, Popel AS. Estimation of elastic moduli and bending stiffness of the anisotropic outer hair cell wall. J Acoust Soc Am. 1998;103:1007–1011. doi: 10.1121/1.421217. [DOI] [PubMed] [Google Scholar]
- Spector AA, Deo N, Grosh K, Ratnanather JT, Raphael RM. Electromechanical models of the outer hair cell composite membranes. J Membrane Biol. 2006;209:135–152. doi: 10.1007/s00232-005-0843-7. [DOI] [PubMed] [Google Scholar]
- Spector AA, Ameen M, Charalambides PG, Popel AS. Nano-structure, effective properties, and deformation pattern of the cochlear outer hair cell cytoskeleton. J Biomech Eng. 2002;124:180–187. doi: 10.1115/1.1448521. [DOI] [PubMed] [Google Scholar]
- Spector AA, Jean RP. Modes and balance of energy in the piezoelectric cochlear outer hair cell wall. ASME J Biomech Engin. 2004;126:17–25. doi: 10.1115/1.1644564. [DOI] [PubMed] [Google Scholar]
- Sun SX, Farrell B, Chana MS, Oster G, Brownell WE, Spector AA. Voltage and frequency dependence of prestin-associated charge transfer. J Theoret Biol. 2009;260:137–144. doi: 10.1016/j.jtbi.2009.05.019. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Tolomeo JA, Steele CR. Orthotropic piezoelectric properties of the cochlear outer hair cell wall. J Acoust Soc Am. 1995;97:3006–3011. doi: 10.1121/1.411865. [DOI] [PubMed] [Google Scholar]
- Zheng J, Shen W, He DZZ, Long KB, Madison LD, Dallos P. Prestin is motor protein of cochlear outer hair cells. Nature. 2000;405:149–155. doi: 10.1038/35012009. [DOI] [PubMed] [Google Scholar]