Cell motility: the integrating role of the plasma membrane

Abstract

The plasma membrane is of central importance in the motility process. It defines the boundary separating the intracellular and extracellular environments, and mediates the interactions between a motile cell and its environment. Furthermore, the membrane serves as a dynamic platform for localization of various components which actively participate in all aspects of the motility process, including force generation, adhesion, signaling, and regulation. Membrane transport between internal membranes and the plasma membrane, and in particular polarized membrane transport, facilitates continuous reorganization of the plasma membrane and is thought to be involved in maintaining polarity and recycling of essential components in some motile cell types. Beyond its biochemical composition, the mechanical characteristics of the plasma membrane and, in particular, membrane tension are of central importance in cell motility; membrane tension affects the rates of all the processes which involve membrane deformation including edge extension, endocytosis, and exocytosis. Most importantly, the mechanical characteristics of the membrane and its biochemical composition are tightly intertwined; membrane tension and local curvature are largely determined by the biochemical composition of the membrane and the biochemical reactions taking place; at the same time, curvature and tension affect the localization of components and reaction rates. This review focuses on this dynamic interplay and the feedbacks between the biochemical and biophysical characteristics of the membrane and their effects on cell movement. New insight on these will be crucial for understanding the motility process.

Introduction

The plasma membrane is essential in many cellular processes; we focus here on its involvement in motility and cell shape determination. The overall morphology of a cell is defined by the membrane which typically conforms to the underlying cytoskeletal structures. Cell movement, which involves a continuous reorganization of the cytoskeleton, must be accompanied by appropriate restructuring of the plasma membrane. Extensive research has shown that the plasma membrane is tightly coupled to the motility machinery; many proteins physically link the membrane to the cytoskeleton, and much of the regulation of cytoskeletal dynamics occurs at the membrane. Despite its importance, the role of the plasma membrane in the motility process is still far from understood.

The plasma membrane defines the physical boundary of the cell by serving as a permeability barrier between the intracellular and the extracellular environments. Transport through the membrane occurs primarily through an array of transmembrane channels and pores and is important in maintaining and regulating cell volume and composition. In addition to phospholipids, glycolipids, and cholesterol derivatives the membrane contains trans-membrane proteins and proteins with domains which insert into or bind the membrane. In general, lipids and membrane proteins are free to diffuse within the membrane, unless they are restricted by binding to cytoskeletal structures. The heterogeneous and dynamic composition of the membrane is determined by this movement within the bilayer and by transport between internal membranes and the plasma membrane. The composition of the plasma membrane is intimately linked with its shape; curvature-sensing lipids and proteins localize to curved regions, and accumulation of such lipids and proteins tends to induce local curvature.

The plasma membrane in motile cells is under tension. This tension arises in part from the in-plane tension generated by the motility machinery pushing from within on an essentially inextensible membrane. In addition, a significant part of the apparent membrane tension in cells arises from the adhesion between the cytoskeleton and the membrane. The membrane tension applies an opposing load which resists membrane extension. This is relevant both for actin-based protrusions, for example lamellipodia and filopodia, and for blebs in which the membrane transiently detaches from the cytoskeleton and protrusion is driven by a pressure gradient across the membrane. Tension also affects membrane transport processes in which vesicles bud off or fuse with the plasma membrane. Although we still do not understand how membrane tension is set and regulated in motile cells, it is obviously important in regulating cell boundary dynamics and physically coupling processes along the boundary.

Importantly, various feedbacks exist between the biochemical composition of the membrane and its biophysical characteristics. As discussed in more detail below, the lipid and protein composition of the membrane affects the local curvature of the membrane; at the same time, curvature can direct non-uniform localization of curvature-sensing lipids and proteins (Liu et al. 2009; McMahon and Gallop 2005; Zimmerberg and Kozlov 2006). Similarly, membrane tension affects various processes including actin polymerization, endocytosis, and exocytosis, processes which, in turn, are involved in determining membrane tension (Keren et al. 2008; Kozlov and Mogilner 2007; Mogilner and Keren 2009; Sheetz 2001; Sheetz and Dai 1996). This review focuses on this complex and dynamic interplay between biophysical and biochemical processes at the membrane which we are only beginning to understand. Progress in this direction will be essential for understanding the important role of the plasma membrane in motility.

The plasma membrane as a platform for localizing components at the cell boundary

The plasma membrane acts as a dynamic platform for localization of a variety of structural and functional components involved in the motility process (Ridley 2011; Saarikangas et al. 2010; Sheetz et al. 2006). The membrane is composed of a heterogeneous and dynamic mixture of lipids and membrane proteins which regulate and activate essential aspects of the motility machinery either directly or by affecting the localization and activity of cytosolic proteins. As described below, the distribution of specific lipids and membrane proteins within the plasma membrane is highly non-uniform, and there is evidence that these localization patterns have functional significance for motility. The mechanisms responsible for generating and maintaining these non-uniform distributions remain elusive in many cases. For example, despite extensive research and substantial progress we still do not know what determines the special composition of lipids and proteins at the leading edge of motile cells.

External stimuli induce and direct cell movement. A myriad of signaling molecules at the membrane respond to these cues and activate the motility machinery and, in particular, actin polymerization. Rho GTPases including Rho, Rac, and Cdc42 are of crucial importance in transmitting signals from membrane receptors to the cytoskeleton and to adhesions (Hall 1998). Rho GTPases act as molecular switches which are active in their GTP-bound conformation and inactive when they are GDP-bound. The exchange between these states is mostly catalyzed and regulated by GTPase-activating proteins (GAPs) and guanine nucleotide exchange factors (GEFs). Rho GTPases can interact directly with the membrane through post-translational modifications (e.g. prenylation) and indirectly through, for example, activation by membrane-bound GEFs (Ridley 2006).

Rho GTPases are of crucial importance in regulating actin dynamics at the cell periphery (Ridley 2006). Actin nucleation is typically the rate-limiting step in actin polymerization in vivo, so cells regulate where and when polymerization occurs by controlling the localization and activation of actin-nucleation promoting factors, for example members of the WASP–WAVE protein family. Activation of these proteins typically depends on multiple inputs and leads to Arp2/3 activation which in turn nucleates new actin filaments leading to actin network growth (Fig. 1a). Rho GTPases are involved in recruiting and activating proteins from the WASP–WAVE family and other actin nucleators, including formins. Their ability to interact with membranes and activate actin nucleation enables Rho GTPases to direct actin assembly to specific sites along the cell periphery and regulate actin protrusions.

Fig. 1.

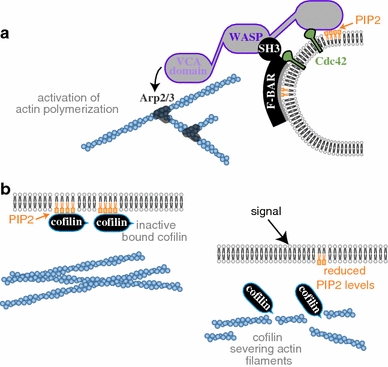
Regulation of actin dynamics at the membrane. a An example of Arp2/3 mediated activation of actin polymerization by membrane localized factors. WASP is localized and activated at the membrane through multiple signals including binding to PIP2 lipids, Cdc42 protein, and a protein from the BAR-domain family containing an SH3 domain (e.g. Toca). WASP is further activated by dimerization/oligomerization (not shown) (Padrick and Rosen 2010). Activated WASP activates Arp2/3 which induces actin filament nucleation and network growth. Membrane curvature is induced by the BAR domain, and affected by the forces generated by actin polymerization. b Membrane composition regulates actin disassembly. PIP2 lipids in the membrane bind and inactivate cofilin (left panel). Reduced PIP2 levels induced by signaling events lead to release of membrane bound cofilin, which becomes active and severs actin filaments (right panel)

Membrane proteins are essential for coupling the cell to its environment both biochemically and mechanically. Transmembrane protein receptors enable cells to sense and respond to chemical cues from their environment (Ridley et al. 2003), and adhesion molecules, for example integrins, mediate attachment between the cytoskeleton and the environment. Integrins preferentially localize to the leading edge of motile cells (Lawson and Maxfield 1995; Lee and Jacobson 1997), where nascent adhesions form and stabilize the actin network by anchoring it to the substrate. As new actin is polymerized, older actin, with the adhesion molecules bound to it, flow rearward relative to the leading edge. Regulation of the localization and dynamics of adhesions involves a large number of proteins with a myriad of interactions and mechanical inputs (Zaidel-Bar and Geiger 2010).

Other important classes of transmembrane proteins are the ion channels and aquaporins, which enable transport of ions and water across the membrane and are essential for regulating the intracellular environment and cell volume. Various channels including NHE1 sodium channels and aquaporins localize preferentially to the leading edge in several motile cell types (Schwab et al. 2007). It has been suggested that these channels may play an active role in protrusion by generating hydrostatic/osmotic pressures that could assist the protrusion process (Keren et al. 2009; Saadoun et al. 2005; Schwab et al. 2007).

The highly dynamic and heterogeneous membrane composition is determined by movement within the bilayer and by extensive transport between internal membranes and the plasma membrane (see the section "Membrane transport and flow", below). The cell membrane is typically fluid, so diffusive transport of lipids and proteins within the bilayer is relatively fast. Extensive tracking of membrane lipids and proteins (Fujiwara et al. 2002) and photobleaching/photoactivation experiments (Dai and Sheetz 1995b; Lee et al. 1993; Weisswange et al. 2005) have shown that movement within the bilayer is essentially diffusive, but the diffusion rates are typically several-fold slower than in artificial bilayers in vitro. Membrane-associated proteins and cytoskeletal structures which form dynamic microdomains in the membrane are thought to be responsible for this reduction in diffusion rates (Fujiwara et al. 2002). In particular, high local concentrations of membrane proteins and attachments to the cytoskeleton can lead to the formation of diffusion barriers. This was observed, for example, at the leading edge of motile keratocytes which harbor a high concentration of proteins (Weisswange et al. 2005).

The composition of the plasma membrane

The lipid composition of the plasma membrane is highly diverse. The lipid species in the membrane differ in their head group and in the length and saturation of their fatty acid tails, and this diversity is only beginning to be characterized (Shevchenko and Simons 2010). Typically the plasma membrane contains large amounts of phosphatidylcholines and phosphatidylethanolamines, as well as phosphatidylserines, sphingolipids, phosphoinositides, and cholesterol. Moreover, the composition of the plasma membrane is highly asymmetric between the inner and outer leaflet. The inner leaflet contains phosphatidylethanolamines, phosphatidylserines, and phosphoinositides whereas the outer leaflet contains mostly phosphatidylcholines and sphingolipids, with cholesterol residing in both leaflets. This asymmetric distribution is dynamically maintained by the membrane translocation machinery which consumes large amounts of ATP in the process.

In addition to lipids, the plasma membrane contains a substantial protein component which is made up of transmembrane proteins and proteins with membrane-binding domains, for example amphipathic alpha-helices or lipid anchors. Protein–lipid interactions are known to have a large effect on the relative distribution of lipids and proteins in the membrane, and, in particular, on the formation of dynamic membrane domains, for example lipid rafts. Despite extensive work in this area, we are only beginning to understand the importance of the diversity in the lipid and protein composition of the membrane and the complexity of lipid–protein interactions.

The local composition of the membrane has significant effect on the behavior and morphology of the cell boundary. On small scales, formation of self-organized membrane domains which vary in composition from their surroundings are precursors for sites of vesicle formation, invaginations, or protrusions (Shnyrova et al. 2009). On larger scales, the membrane organization is polar (reflecting the inherent polarity of motile cells which protrude at the front and retract at the rear) so the membrane composition at the leading edge differs from that at the trailing edge. An important family of lipids which are non-uniformly distributed are the phosphoinositides, including phosphatidylinositol(4,5)bisphosphate (PIP2) and phosphatidylinositol(3,4,5)triphosphate (PIP3). The head groups of the phosphoinositides are easily modified, enabling their rapid regulation by enzymes such as phosphoinositide 3-kinase and the phosphatase PTEN (Kolsch et al. 2008). Phosphoinositides bind and activate actin nucleation promoting factors and inhibit actin filament capping and disassembly, and hence promote actin polymerization at the membrane (Fig. 1) (Kolsch et al. 2008; Saarikangas et al. 2010). PIP3 has been shown to be enriched along the leading edge of several motile cell types including HL60 and Dictyostelium and this has been implicated in establishing polarity and directed motility in these cells (Kolsch et al. 2008), although it is not essential (Hoeller and Kay 2007).

The effect of lipid composition on actin dynamics is clearly demonstrated by in vitro experiments in which supported membrane bilayers of different composition were tested for their ability to catalyze actin polymerization and induce the formation of filopodia-like structures (Lee et al. 2010). Incorporation of phosphoinositides, and in particular PIP2, dramatically enhanced the formation of these structures, which resemble filopodia in live cells. Furthermore, direct regulation of actin-binding proteins by PIP2 has been demonstrated in live mammary carcinoma cells in which cofilin, which actively promotes actin network disassembly by severing filaments, was found to be regulated by binding to PIP2 (Van Rheenen et al. 2007). Activation of cells led to a rapid decrease in PIP2 levels in the membrane which triggered release and activation of membrane-bound cofilin, leading to actin network disassembly (Fig. 1b).

Membrane curvature

A motile cell contains regions characterized by high membrane curvature, most notably the leading edge of the cell, which has a radius of curvature of ~50–100 nm (Abraham et al. 1999) and intermediates in membrane transport including intracellular vesicles and endosomes. The motility process involves continuous reorganization of the morphology and curvature of these membrane domains. The spontaneous curvature of a membrane (i.e. the curvature at which the bending energy is minimum) and its bending elasticity (i.e. the energy cost of bending the membrane beyond its spontaneous curvature) are determined by the composition of the membrane (Fuller and Rand 2001; Leikin et al. 1996). The local curvature of the membrane is determined by these characteristics together with the forces acting on the membrane including cytoskeletal-generated forces and pressure gradients across the membrane (McMahon and Gallop 2005; Zimmerberg and Kozlov 2006). Rather than being a passive consequence, local membrane curvature is dynamically determined by a continuous interplay between shape and composition; curvature-sensing lipids and proteins respond to membrane curvature and at the same time induce spontaneous curvature by energetically favoring curved morphology and by inducing changes in local cytoskeletal dynamics and force generation.

The structure of lipids and membrane proteins and their interactions can favor membrane deformations; e.g. wedge-shaped lipids or proteins incorporated within the bilayer induce local curvature. The correlation between lipid composition and local curvature is clearly demonstrated by in vitro experiments in which giant unilamellar vesicles composed of several lipid species spontaneously phase separate into domains which vary in both composition and local curvature (Baumgart et al. 2003). Furthermore, expression of membrane proteins, for example the Shiga toxin in cells, can induce the formation of highly curved membrane tubules in which the toxin is highly enriched (Romer et al. 2007). Proteins with domains that insert into the bilayer, for example an amphipathic alpha-helix domain, can also sense and induce curvature (Drin et al. 2007).

Scaffolding proteins that bind to the membrane provide yet another important mechanism for inducing curvature (McMahon and Gallop 2005; Zimmerberg and Kozlov 2006). One of the best characterized families of scaffolding proteins is the BAR domain protein family. These proteins contain a rigid curved domain which interacts with membranes via hydrophobic and electrostatic interactions (Frost et al. 2008; Gallop et al. 2006; Masuda et al. 2006). Different BAR domain proteins are characterized by different natural curvature; in particular F-BAR and N-BAR domain proteins induce positive curvature (membrane invaginations) (Peter et al. 2004) whereas the I-BAR domain protein induces negative curvature (membrane protrusions) (Mattila et al. 2007). In vitro experiments have shown that the BAR-domain proteins can spontaneously bind and deform membranes on their own and that the types of structure formed depend on the proteins’ structures (Frost et al. 2008; Saarikangas et al. 2009). In general, the action of curvature-favoring lipids and proteins and scaffolding proteins is viewed as being highly cooperative; membrane deformation in cells (e.g. the formation of an endocytic vesicle) typically involve a very large number of curvature-sensing and curvature-inducing molecules (Shnyrova et al. 2009).

The BAR domain proteins affect membrane curvature by other means also; many BAR domain proteins have additional domains, for example the SH3 domain which binds and activates proteins from the WASP–WAVE family and thus promotes actin nucleation and polymerization (Fig. 1a) (Takenawa and Suetsugu 2007). In vitro work has shown that activation of N-WASP-mediated actin polymerization by proteins containing an F-BAR domain depends on membrane curvature (Takano et al. 2008). The actin polymerization-enhancing activity of mixtures containing N-WASP and F-BAR domain proteins was measured by use of a pyrene assay. Addition of liposomes (i.e. highly-curved membranes) to the mixture significantly enhanced actin polymerization rates. Moreover, the actin polymerization rates were found to vary for liposome preparations that differed in their average size. These results directly suggest the possibility of feedback between curvature and actin dynamics: curvature-inducing proteins stimulate actin polymerization in a curvature-dependent manner, and actin polymerization can generate forces which induce curvature.

The leading edge of motile cells is characterized by a thin protruding region with high membrane curvature (Abraham et al. 1999). This thin structure is maintained by restricting actin polymerization to the apex and it is clear that localized pushing forces induced by growing actin filaments are important for generating and maintaining this high curvature. Although it is known that Arp2/3, which nucleates actin filaments, is regulated by membrane-bound activators, primarily proteins from the WASP–WAVE family (Takenawa and Suetsugu 2007), it is still unclear why activation is concentrated only at the very leading edge. It is, moreover, unclear how the preferred directionality of the nucleated filaments toward the leading edge is established.

The key effect of the enveloping membrane on the morphology of the actin network at the leading edge is highlighted by experiments on permeabilized cells (Shao et al. 2006). Activation of Arp2/3-dependent actin polymerization in these permeabilized cells resulted in the formation of thick 3D networks whose structure and morphology are very different from the normal, essentially 2D flat networks found at the leading edge of intact cells. The mechanisms responsible for this are still unclear; is it related to curvature-dependent localization of nucleators or rather to steric and mechanical hindrance by the membrane? Theoretically, a positive feedback mechanism between localization of actin nucleation-promoting factors and curvature induced by the force generated by actin polymerization has been suggested (Atilgan et al. 2005; Gov and Gopinathan 2006): localization of curvature-sensitive actin nucleation promoting factors induce actin polymerization and local force generation which deforms the membrane and induces high curvature at the leading edge. This high curvature in turn promotes further localization of the curvature-sensitive actin nucleation-promoting factors, closing a positive feedback loop which forms and maintains the high curvature at the leading edge. Although this idea is certainly plausible and appealing, experimental verification is still lacking. Overall, the mechanisms determining curvature and morphology of the leading edge are still an intriguing and open question in the field.

Membrane transport and flow

Transport between internal membranes and the plasma membrane through endocytosis and exocytosis are essentially the only way for lipids and transmembrane proteins to enter or leave the plasma membrane. Endocytosis includes several different processes, including clathrin-mediated and caveolar endocytosis, by which patches of the plasma membrane invaginate inward and bud off to form vesicles, thus reducing the area of the plasma membrane. Conversely, in exocytosis intracellular vesicles fuse with the plasma membrane, so their lipid and membrane protein content is incorporated into the plasma membrane and their internal cargo is released to the extracellular environment. Together these processes are of central importance in determining the composition of the membrane and its overall surface area (Morris and Homann 2001).

Membrane transport between internal membranes and the plasma membrane accompanies movement in all cell types. Furthermore, in some cases there is evidence of polarized membrane trafficking in which either the rates or composition of vesicle trafficking vary along the front-to-rear axis of the cell (Bretscher 1984, 1996; Fletcher and Rappoport 2010). The overall rates of transport between internal membranes and the plasma membrane vary among different cell types. In Dictyostelium amoeba it takes ~3–10 min to replace an area equal to the cell surface area (Aguado-Velasco and Bretscher 1999) whereas in fibroblasts it takes approximately one hour (Steinman et al. 1983). Rates of endocytosis will depend on the availability of internal membranes, which also depends on membrane biogenesis. Moreover, as discussed below, the rates of endocytosis and exocytosis depend on the tension in the membrane.

Inhibition of membrane trafficking in several cell types, for example fibroblasts, endothelial cells and Dictyostelium, reduces persistent migration and reduces cell speed (Fletcher and Rappoport 2010; Thompson and Bretscher 2002; Wessels et al. 2000). Although these perturbation experiments clearly implicate membrane transport in the motility process, the mechanisms and pathways involved are still debated (Fletcher and Rappoport 2010). One of the main functions suggested for membrane trafficking is the recycling of membrane components such as cell-adhesion molecules (Fig. 2; see below) (Caswell et al. 2009; Jones et al. 2006). Other possible functions include lipid transport to induce membrane flow to the leading edge and the establishment and maintenance of cell polarity. The importance of membrane transport to motility probably varies among different cell types, and research is needed to clarify its functions (Fletcher and Rappoport 2010).

Fig. 2.

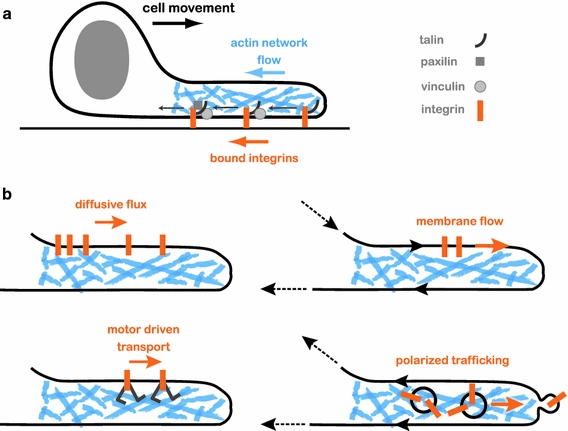
Membrane transport and recycling of adhesions. a Adhesion complexes assemble in a hierarchical fashion from integrins embedded in the membrane and cytosolic proteins. Nascent adhesions form at the leading edge and attach to the substrate. Actin network growth and flow away from the leading edge leads to a rearward flux of adhesions. In particular, rearward flux is observed for bound integrins. b Various possible mechanisms for recycling integrins back to the leading edge have been suggested. Passive transport by diffusion will tend to equilibrate the integrin density but may not be fast enough for efficient recycling. Active transport to the leading edge can occur with the help of motor proteins which drag the integrins within the membrane, by drift induced by bulk membrane flow, or by polarized membrane trafficking

Cell movement requires continuous recycling of essential membrane components. For example, membrane proteins such as integrins, which couple the actin cytoskeleton to the substrate, must be continuously replenished at the leading edge; as the actin flows rearward in the cell frame of reference, a continuous flux of bound integrins moves with it (Fig. 2a). To maintain motion there must be a counter-flux of adhesion molecules entering the leading edge. Recycling of transmembrane proteins, for example integrins, can occur via several mechanisms (Fig. 2b) (Bretscher 1996). Within the bilayer, passive diffusion can contribute, but it is probably too slow to compensate for the retrograde flow of adhesions away from the leading edge. Thus, at least in rapidly moving cells, active transport is likely to be needed. Directed motion through the membrane can be driven by bulk membrane flow (see below) or motor-driven transport. Alternatively, membrane transport via polar membrane trafficking in which integrin-containing vesicles are targeted preferentially to the leading edge can provide means for directed transport (Fletcher and Rappoport 2010; Pellinen and Ivaska 2006). Although integrin transport has been investigated to some extent (Jones et al. 2006; Lawson and Maxfield 1995), the question of how integrins (and other components) are recycled back to the leading edge is still largely unanswered (Pellinen and Ivaska 2006).

In most motile cells in which membrane dynamics have been directly measured, either by single particle tracking of lipid-bound particles or by photobleaching/photoactivation, no net membrane flow has been observed. This includes fibroblasts, fish keratocytes (Kucik et al. 1989, 1990; Lee et al. 1993), leukocytes (Lee et al. 1990), and Dictyostelium amoebae (Traynor and Kay 2007), in all of which the membrane was found to passively move along with the cell with no net membrane flow in the cell frame of reference. Notable exceptions are neuronal growth cones, in which a continuous membrane flow from the growth cone toward the cell body was observed and is thought to be involved in replenishing the components of the axon plasma membrane (Dai and Sheetz 1995a).

Changes in the area of the plasma membrane accompany movement in some cell types. Because the plasma membrane is essentially inextensible (it can be stretched by only 2–4% before rupturing (Morris and Homann 2001)), such changes must be generated by an imbalance between the processes of exocytosis and endocytosis. Excess endocytosis reduces plasma membrane area, whereas surplus exocytosis increases it. The extent of change in surface area during motility varies among different cell types. During motility of Dictyostelium amoeba, substantial (more than ~20%) dynamic changes in surface area have been observed for individual cells over time (Traynor and Kay 2007) whereas rapidly moving fish keratocytes have essentially constant surface area as they move, despite changes in their overall morphology and speed (Keren et al. 2008). The function of surface-area changes during motility is still unclear; whereas in Dictyostelium amoeba it seems that surface area regulation is an active part of the motility process, in keratocytes membrane area remains fixed and the membrane seems to be stretched taut around the cytoskeleton imposing constraints on shape and movement.

Rapid changes in cell surface area can also be mediated by budded and folded membrane domains that act as a membrane reservoir (Ting-Beall et al. 1993) and enable cells to respond to sudden changes in membrane tension (Sens and Turner 2006). To probe the membrane reservoir in motile fibroblasts, membrane tethers were extracted from the cell membrane (see below). The initial force required to pull tethers was found to be independent of tether length, indicating that the membrane flowing into the tether was taken from a reservoir (Raucher and Sheetz 1999a); at some point the tether force started increasing abruptly in an exponential fashion, indicating depletion of the reservoir. The extent of the membrane reservoir in fibroblasts is relatively small (<1%) but it could be larger in other cell types in which membrane folds and invaginations are more abundant. Recent work has shown that caveolae, which are cup-shaped membrane invaginations, enable endothelial cell and muscle cells to undergo fast changes in cell surface area (Sinha et al. 2011). Caveolae are actively generated through an ATP and actin-dependent mechanism, and can then flatten out rapidly in response to cell stretching or swelling (Fig. 3d). This enables cells to buffer changes in membrane tension and prevent rupture of the plasma membrane.

Fig. 3.

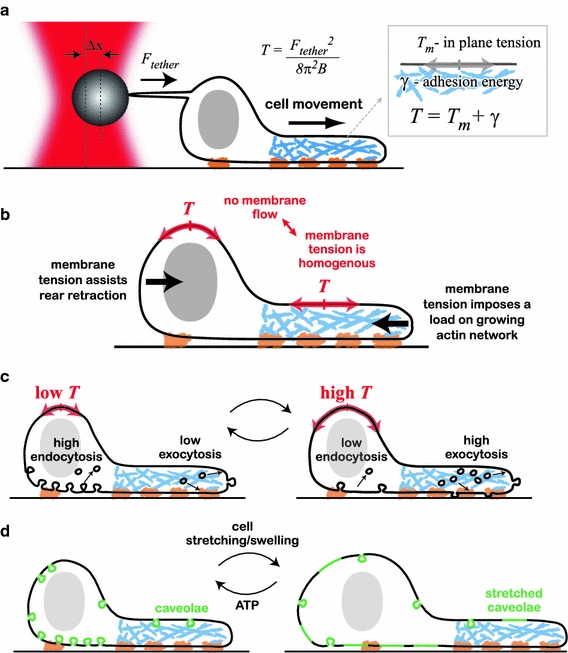
Membrane tension in motile cells. a Membrane tension measurements using the tether-pulling assay. A membrane tether is generated by pulling a coated bead away from the cell surface by use of optical tweezers. The force the tether exerts on the bead can be measured from the displacement of the bead from the center of the trap. The apparent membrane tension, which is equal to the sum of the in-plane tension and the adhesion energy per unit area, can be calculated from the measured tether force. b The apparent membrane tension exerts forces perpendicular to the cell boundary (black arrows). In the absence of membrane flow, the apparent membrane tension has to be spatially homogenous. The membrane tension opposes actin network growth at the leading edge, while assisting retraction at the rear. c Endocytosis rates increase with decreasing tension whereas exocytosis rates decrease. It has been suggested that tension-dependent endocytosis and exocytosis are involved in surface area regulation and buffering of membrane tension: increased tension leads to excess exocytosis, leading to an increase in cell surface area and a decrease in tension, and vice versa. d Membrane invaginations, for example caveolae, provide means for rapidly increasing cell surface area and buffering membrane tension. Caveolae require ATP and actin for their synthesis. A rapid increase in tension (because of stretching or swelling of the cells) is buffered by rapid flattening of the caveolae, providing additional surface area

Overall the questions of how cells regulate their surface area and how changes in surface area relate to the motility process are still largely unanswered. Although a cell probably cannot directly measure its surface area, it is thought that cells respond to changes in membrane tension. As discussed in the next section, endocytosis and exocytosis rates are tension-dependent, suggesting the possible involvement of mechanical feedback in cell surface area regulation (Morris and Homann 2001). Many questions remain. What determines the surface area of a motile cell? How are membrane proteins such as integrins recycled? When and why are changes in membrane surface area required for motion? Is tension-mediated surface area regulation sufficient to explain surface area dynamics?

Membrane tension

Motile cells are characterized by an active actin cytoskeleton that is constantly pushing from within. As a result, the plasma membrane is stretched and tension is generated in the membrane. The membrane tension exerts a force along the cell boundary which is proportional to the local mean membrane curvature and is directed perpendicular to the cell surface. Unlike artificial vesicles in which tension is often negligible in comparison with other contributions, for example the membrane-bending energy (Safran 1994), in motile cells membrane tension is significant. The energy cost of any process which involves membrane deformation or reorganization will include a tension-dependent term and hence the rates of such processes are expected to be tension-dependent. Specifically, as described in detail below, the rates of extension and retraction of the cell boundary, blebbing, endocytosis, and exocytosis all depend on tension.

The apparent membrane tension T can be thought of as the energy cost of adding membrane area (per unit area). In giant vesicles which lack a cytoskeleton, the energy cost of adding unit area of membrane arises only from the in plane membrane tension, T _m. Cells, however, are characterized by a dense cytoskeleton which underlies the plasma membrane and substantial interactions exist between the membrane and the cytoskeleton. In cells, therefore, the apparent membrane tension is the sum of the in plane tension and the membrane–cytoskeleton adhesion energy per unit area, γ, so that T = T _m + γ (Dai and Sheetz 1999; Hochmuth et al. 1996; Sheetz et al. 2006). Moreover, the extent of the membrane–cytoskeleton adhesion is thought to be substantial (Dai and Sheetz 1999). Generally, the contributions of the in-plane tension and membrane–cytoskeleton adhesion are not separable, and the force felt, e.g., by a filament impinging on the membrane or a bead during a tether-pulling assay (Fig. 3a; see below) incorporates contributions from both (Hochmuth et al. 1996).

The exact nature of membrane–cytoskeleton adhesion is not entirely clear; although it is known that discrete protein-mediated interactions occur between the cytoskeleton and the membrane, the relative contribution of these compared with a continuum of weaker interactions between the membrane and the cytoskeleton is not clear (Sheetz 2001). Recent experiments have demonstrated the involvement of class I myosins (which have both actin and membrane-binding domains) in membrane–cytoskeleton adhesion (Nambiar et al. 2009). In these experiments, membrane tension was measured in epithelial cells and found to be lower in cells which express dominant-negative myosin I and higher in cells over-expressing myosin I, largely because of the contribution of myosin to membrane–cytoskeleton adhesion. Experiments on the formation of blebs and their retraction after reformation of an actin cortex suggest that proteins, for example ezrin, that crosslink actin filaments to the membrane make a major contribution to actin–membrane adhesion energy (Charras et al. 2006). However, the adhesion energy between the membrane and actin filaments can also be substantial in the absence of specific proteins that directly link them, as demonstrated by in vitro experiments in which the continuous interaction between actin filaments and the membrane was sufficient to stabilize filopodial-like protrusions (Liu et al. 2008).

The apparent membrane tension can in principle vary along the cell boundary. In particular, the local energy of adhesion to the cytoskeleton is expected to vary as the morphology and the composition of the cytoskeleton and the membrane change along the boundary. Gradients in the apparent membrane tension result in in-plane forces on the lipids in the fluid bilayer and are therefore expected to induce membrane flows. Such gradients were measured between the cell body and the growth cone in neurons (Dai and Sheetz 1995a) where they were, indeed, accompanied by membrane flow. However, in most motile cells in which no net membrane flow is found (Kucik et al. 1989, 1990; Lee et al. 1990, 1993; Traynor and Kay 2007), the apparent membrane tension is expected to be constant along the boundary (Fig. 3b). Importantly, local application of force to the membrane (either internally, e.g. by a polymerizing actin filament of a membrane-bound cytoskeletal motor, or externally, by pulling a membrane tether) will rapidly propagate across the entire cell and equilibrate within milliseconds (Kozlov and Mogilner 2007). Thus on the time scales relevant for motility, the apparent membrane tension can be regarded as spatially constant (in cases where no net membrane flow is observed).

The most common way of measuring membrane tension in adherent cells is the tether-pulling assay (Fig. 3a). Membrane tethers are pulled from the plasma membrane by use of an AFM tip (Sun et al. 2005) or a micropipette, but most commonly using a bead manipulated by optical tweezers (Dai and Sheetz 1995b). The bead is coated with a membrane binding moiety, held against the membrane for a few seconds to establish binding, and subsequently moved away from the cell to generate a membrane tether. The force opposing tether formation (“the tether force”) can be extracted from the displacement of the bead from the center of the optical trap. A typical force–extension curve contains an initial peak in the tether force related to tether formation followed by a broad region in which the tether force is independent of tether length (Raucher and Sheetz 1999a).

The tether force acting on a tether pulled from a flat membrane with no spontaneous curvature is related to the membrane tension by: 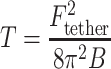, where B is the bending modulus of the membrane (characterizing the energy cost of bending the membrane) (Hochmuth et al. 1996). Note that this relationship is independent of the length of the tether. Thus, knowing the bending modulus of the membrane, one can relate the measured tether force to the tension in the membrane. In cells the situation is somewhat more complex; as discussed above the membrane adheres to the cytoskeleton. Because membrane tethers are typically devoid of cytoskeleton, pulling a tether involves detaching an area of the membrane from the cytoskeleton (in addition to the work done against the in-plane tension). Assuming a cell remains essentially at steady state during a tether-pulling assay, the measured tether force on the bead (F _tether) can be related to apparent membrane tension, T = T _m + γ, by: 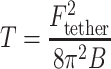. This simplified expression neglects dynamic contributions from dissipative losses during tether formation because of viscosity (Marcus and Hochmuth 2002), any spontaneous curvature in the cell membrane or non-homogenous segregation of lipids or proteins into the tether (Sorre et al. 2009), and the possibility of dynamic rearrangements within the cell during the measurement.

Notwithstanding these complications, the tether-pulling assay has been used to estimate membrane tension in a variety of cells and, more importantly, to investigate how changes in membrane tension correlate with changes in cellular behavior. Tether forces have been measured in several types of adherent motile cells and found to range from F _tether ~ 7 pN in neuronal growth cones (Hochmuth et al. 1996) and fibroblasts (Raucher and Sheetz 2000) to ~30 pN in endothelial cells (Sun et al. 2005) and melanoma cells (Dai and Sheetz 1999). The apparent membrane tension is extracted from tether-force measurements based on either measured (Hochmuth et al. 1996) or estimated values for the bending modulus of the cell membrane in each cell type, with values ranging from T ~ 3 pN/μm in neuronal growth cones to ~50 pN/μm in melanoma cells (Dai and Sheetz 1999). These values are much lower than the lytic tension (the tension at which the membrane ruptures) which is ~3,000–10,000 pN/μm (Morris and Homann 2001). Membrane tension measurements have not yet been reported for rapidly moving cells such as fish keratocytes.

Tether-pulling experiments have also demonstrated the important contribution of the adhesion between the membrane and the cytoskeleton to the apparent membrane tension (Dai and Sheetz 1999; Sheetz 2001). Tether-force measurements were preformed on blebbing cells (see below) from the blebbing regions (which are devoid of cytoskeleton) and from regions outside the blebs (which have an underlying cytoskeleton). The tether force required to pull tethers from the cell membrane was found to be substantially higher in regions outside blebs in which membrane–cytoskeleton adhesion is present (Dai and Sheetz 1999). Although it is difficult to quantify the adhesion energy in these experiments, because blebs are not in steady state and hence the tension is expected to vary both in space and in time, these results obviously demonstrate the significant contribution of membrane–cytoskeleton adhesion to the apparent membrane tension.

Changes in membrane tension can be induced by osmotic shock; hyperosmotic media causes an outward water flux from the cell and hence a decrease in membrane tension whereas hypoosmotic shock leads to cell swelling accompanied by an increase in membrane tension. Osmotic perturbations thus provide a rapid and easy way to induce changes in tension and investigate how these affect cell behavior. This approach has proved useful (Dai and Sheetz 1999; Raucher and Sheetz 1999a, 2000), even though it should be kept in mind that osmolarity shifts invoke a broad range of cellular responses which are not all related to tension and, hence, the results from these experiments should be interpreted carefully. Changes in membrane tension can also be induced by adding substances which are incorporated into the membrane or change its properties, for example detergents or fluorescent lipid analogs (Raucher and Sheetz 1999a, 2000). Here again isolating the effect of tension is not straightforward; these compounds can affect, e.g., the localization of membrane proteins and, hence, induce changes not directly related to the changes in tension. By use of hyper and hypoosmotic shocks and membrane-perturbing agents it was possible to correlate the rates of endocytosis, exocytisis, and membrane extension with membrane tension (Dai and Sheetz 1995c; Raucher and Sheetz 2000).

Importantly, there is dynamic feedback between membrane tension and processes which involve deformation of the plasma membrane (Fig. 3b–d). As discussed in detail below, membrane tension affects the rates of extension at the leading edge and retraction at the trailing edge, and membrane transport in which vesicles bud off and fuse with the plasma membrane. All these processes, which have been shown to be affected by membrane tension, are at the same time effectors of membrane tension. For example, it is well known that actin assembly is force-dependent (Footer et al. 2007; Kovar and Pollard 2004; Marcy et al. 2004) and hence the rate of polymerization at the leading edge will depend on the load due to membrane tension (Keren et al. 2008; Raucher and Sheetz 2000); at the same time, the tension in cells will depend on the overall pushing forces exerted by the actin cytoskeleton within an essentially inextensible membrane.

Thus, membrane tension is a dynamic variable which has a unique role as a physical regulator of cell behavior; tension integrates inputs from multiple sources and feeds back to regulate the rates of these processes. Because membrane tension equilibrates rapidly (Kozlov and Mogilner 2007), it effectively serves as a global regulator which enables biochemical reactions at distant parts of the cell to communicate through the membrane. This is relevant to coordination of rates of actin polymerization along the cell boundary (Keren et al. 2008; Lacayo et al. 2007) and the coordination between protrusion at the front and retraction at the rear in keratocyte fragments (N. Ofer and K. Keren, unpublished results). Such communication is important for large-scale coordination of the motility machinery and cell-shape determination (Mogilner and Keren 2009).

The integrating role of membrane tension as a global mechanical regulator is analogous to the role of the cell’s membrane potential as a regulator of its electrophysiological behavior. Membrane potential arises from the collective action of all the channels in the membrane, and at the same time directly affects transport through channels and, in particular, regulates the activity of voltage-dependent channels (Kandel et al. 2000). Similarly, membrane tension arises from the integrated mechanical action of all the processes occurring at the cell boundary, and at the same time affects them. In both cases, because the time scale for equilibration (of potential or tension, respectively) is fast compared with other time scales in the problem, membrane potential and membrane tension are homogenous along the boundary and hence act as global regulators which effectively induce coupling between local processes occurring at distal locations along the cell periphery.

Although research in recent decades has established the importance of membrane tension as a physical regulator of cell motility, and membrane tension has been measured in a variety of cell types, it is completely unclear how membrane tension is set and regulated. A variety of processes are known to affect membrane tension, but how they all combine to determine and maintain tension in motile cells remains a mystery. This is also an unanswered question in the context of other cellular processes in which membrane deformation is extremely important, for example cell growth and cell division. Below we discuss in more detail the relationship between membrane tension and the dynamics of the cell boundary.

Membrane tension affects cell boundary dynamics

Actin-based protrusions

Actin-based protrusions including lamellipodia, filopodia, and invadopodia are obviously central to motility (Ridley 2011). The membrane imposes a load on the growing actin filaments within these protrusions, and the rate of polymerization is known to be force-dependent (Footer et al. 2007; Kovar and Pollard 2004; Marcy et al. 2004). The effect of the load force is typically described within the framework of molecular ratchet models (Mogilner and Oster 1996, 2003; Peskin et al. 1993) in which fluctuations in the position of both the membrane and the filament tip are responsible for opening a gap and enabling monomer insertion and filament elongation. Within such models, filament growth depends on the tension in the membrane and on the filament’s anchoring and orientation relative to the membrane.

Despite extensive research in recent years, the relationship between the rate of growth of an actin network and force is still not clear (Brangbour et al. 2011; Carlsson 2003; Mogilner and Oster 2003; Parekh et al. 2005; Prass et al. 2006). The actin network is composed of many filaments which cooperatively push against the membrane. The structure and dynamics of the network and the membrane determine how the mechanical work is shared among the filaments and will determine the relationship between network growth and force (Schaus and Borisy 2008). Experimental research suggests that rates of network growth are relatively insensitive to load force at weak forces (compared with the stall force) and then rapidly drop as force increases (Parekh et al. 2005; Prass et al. 2006). Moreover, because the morphology of the network is predicted to be force-dependent (Carlsson 2003), the so-called force–velocity relationship characterizing the dependence of network growth on the load force becomes history-dependent (Parekh et al. 2005; Weichsel and Schwarz 2010). Although the details of the force–velocity relationship governing actin network growth are still not known, it is clear that load force will, in general, slow network growth and eventually stall growth at the so-called stall force.

In accordance with the idea that membrane tension hinders actin polymerization (Fig. 3b), experiments in fibroblasts demonstrated an inverse relationship between membrane tension and lamellipodial extension rates (Raucher and Sheetz 2000). Membrane tension was reduced by adding amphiphilic compounds or lipids, which are incorporated into the membrane and increase its area, or by stimulating cells with PDGF, which is thought to reduce membrane–cytoskeleton adhesion. Conversely, membrane tension was increased by hypoosmotic shock which caused cell swelling. In all cases, a decrease in tension was associated with higher extension rates whereas tension increase was accompanied by reduced rates of lamellipodial extension.

The extent to which tension determines protrusion rates, cell speed, and cell morphology varies among different cell types (Mogilner and Keren 2009). In rapidly moving keratocytes recent work suggests that the graded extension rate along the leading edge (Lee et al. 1993) is a result of the graded variation in the force-per-filament imposed by the membrane tension. The actin network density along the leading edge in keratocytes peaks at the center (Keren et al. 2008; Lacayo et al. 2007), so the force per filament, which is equal to the tension divided by the local filament density, is minimum at the center of the leading edge and increases toward the sides. In particular, the front corners of the cell are assumed to be determined by where the force per filament reaches the stall force for polymerization. This model predicts a clear correlation between cell shape and the distribution of actin filaments along the leading edge. Quantitative analysis of shape variation within a population of keratocytes and its correlation with the distribution of actin filaments provides support for this picture (Keren et al. 2008; Lacayo et al. 2007). In addition to coupling rates of protrusion along the leading edge, the membrane provides means for mechanical feedback between extension at the leading edge and retraction at the rear; higher rates of extension will tend to increase tension and hence increase retraction.

Blebbing

Blebbing is an alternative from of edge protrusion which is driven by intracellular hydrostatic pressures rather than actin polymerization (Charras and Paluch 2008). The actin cytoskeleton is still, however, essential for bleb formation; the intracellular pressure driving bleb formation results from myosin II-generated contraction of the actin cytoskeleton which “squeezes” the cytosol leading to increased pressure. Migration driven by the formation of blebs has been observed in a variety of cell-types including embryonic stem cells and tumor cells (Charras and Paluch 2008); some cells, for example embryonic cells, rely primarily on bleb migration whereas others switch between actin-based protrusions and blebbing. The nucleation of a bleb involves an initial detachment between the actin cortex and the cell membrane which continues to grow as a result of increased intracellular pressure. The dynamics of bleb formation and the final size of blebs depend on membrane tension, because tension imposes a load resisting bleb expansion (Charras et al. 2008). Experiments have shown that increasing membrane tension by switching to hypoosmotic media reduces both the number of blebs formed and their extension (Charras et al. 2008).

Rear retraction

Whereas at the leading edge, the load due to membrane tension opposes protrusion, at the trailing edge membrane tension assists in rear retraction and de-adhesion (Fig. 3b). The extent of the force contributed by membrane tension will obviously depend on the magnitude of the membrane tension and on the local curvature at the rear (which is typically smaller than at the leading edge). Although myosin II-generated contraction of the actin cytoskeleton is thought to dominate rear contraction in several cell types, for example Dictyostelium (Clow and McNally 1999), the contribution of membrane tension can be important in other cell types. In particular, in rapidly moving keratocytes, membrane tension is thought to be crucially involved in rear retraction (Keren et al. 2008). Inhibition of myosin II by blebbistatin in these cells, which should essentially suppress the contribution of myosin II to rear retraction, does not impair retraction and is characterized by only a moderate reduction in cell speed (Keren et al. 2008).

Apart from the obvious direct contribution of membrane tension to rear retraction, membrane tension can assist in retraction by activation of mechanosensitive channels. Stretch-activated channels are known to respond to membrane tension by modifying their gating characteristic (Morris 1990). In particular, stretch-activated calcium channels have been shown to be important in rear retraction in motile keratocytes which are transiently stuck at the rear (Doyle and Lee 2005; Lee et al. 1999). The influx of calcium is likely to contribute to retraction by enhancing myosin II contraction and adhesion disassembly. This mechanism provides feedback in which reduced rear contraction leads to a buildup of tension (because the front continues to protrude forward) which is then relieved by activation of the stretch-activated channels. It should be noted that stretch-activated channels are known to respond both to membrane tension and to mechanical forces transmitted through connections to the cytoskeleton; both membrane tension and cytoskeletal tension are expected to increase when the cell rear gets stuck, and it is currently unclear which is responsible for channel activation.

Endocytosis and exocytosis

As described above, membrane transport into and out of the plasma membrane occurs through exocytosis and endocytosis, respectively. Both processes involve extensive membrane deformation, and are hence expected to depend on membrane tension (Apodaca 2002; Sheetz 2001). Formation of an endocytic vesicle from the plasma membrane is similar to initiation of a membrane tether in a tether-pulling assay (Sheetz 2001): as membrane tension increases the energy cost of both is expected to increase, and, hence, the rate of endocytosis is predicted to decrease with increasing tension (Fig. 3c). Measurements have revealed that rates of endocytosis do, indeed, decrease as membrane tension increases (Dai and Sheetz 1995c). Moreover, rates of endocytosis were found to correlate inversely with membrane tension during the cell cycle; as cells enter mitosis, membrane tension increases as the rate of endocytosis decreases, whereas the rate of endocytosis increases as membrane tension decreases when cells exit mitosis (Raucher and Sheetz 1999b). Exocytosis, on the other hand, involves insertion of vesicles into the bilayer. The energy cost of exocytosis is expected to decrease as membrane tension increases, so the rates are expected to increase (Fig. 3c). The dependence of the rates of endocytosis and exocytosis on tension provides a means of simple mechanical feedback for surface area homeostasis (Apodaca 2002; Sheetz and Dai 1996); as membrane tension increases, exocytosis is stimulated and this in turns tends to reduce membrane tension. Conversely, a decrease in membrane tension stimulates endocytosis which tends to increase tension (Fig. 3c). It has been suggested that this simple feedback between cell surface area and membrane tension is a central mechanism for cell surface area regulation (Morris and Homann 2001), but it has yet to be characterized.

Concluding remarks

The objective of this review is to emphasize the important (and often unappreciated) involvement of the membrane in cell motility and to stress the significance of mechanical feedback at all levels, i.e. from the molecular level to the cellular level. The localization and function of individual molecules are affected by membrane curvature and tension. Because the interactions between lipids and membrane proteins are typically highly cooperative, they continue to affect the behavior of larger sub-cellular structures, for example actin protrusions. Finally, at the cellular level, membrane tension acts as a global mechanical regulator of cell boundary dynamics. The interplay between biophysical and biochemical aspects of membrane behavior at all levels is essential for establishing and coordinating cell crawling. It is similarly important for more complex processes, for example cell movement in 3D or collective cell migration (see below), and many other cellular processes including cell growth and cell division.

This review focuses on the crawling of individual cells in 2D. However, in vivo, cell motion often occurs within a 3D environment composed of a matrix of extracellular filaments and/or other cells. Movement in 3D is thought to be qualitatively different from movement in 2D (Lammermann and Sixt 2009; Mogilner and Keren 2009). For example, the requirements for adhesion are different for 2D and 3D motion (Lammermann and Sixt 2009). Specifically, although integrins are thought to be essential for crawling on a substrate in 2D, integrin-free movement has been demonstrated in 3D (Lammermann et al. 2008). As a result of these differences in adhesion, cells in 2D are typically more spread out than cells in 3D. Although little is known about the behavior of the plasma membrane during 3D movement, reduced cell spreading is likely to be associated with lower membrane tension (Mogilner and Keren 2009). Experiments are needed to corroborate this, as there are few if any reports of measurements of membrane tension or dynamics for cells moving in 3D. More generally, the interplay between the cell membrane and the motility machinery in 3D is still largely unexplored and awaits research.

Collective migration, in which cells move in cohesive groups rather than individually, is also important for many physiological processes in morphogenesis, regeneration, and cancer (Friedl and Gilmour 2009; Padrick and Rosen 2010). Much less is known about collective cell migration, and in particular about its biophysical aspects. However, it is clear that the plasma membrane is of crucial importance; in addition to its involvement in regulating the behavior of individual cells, the membrane mediates the interactions between cells in the group. Understanding how mechanical and chemical inputs from neighboring cells and the environment integrate together and lead to coherent multi-cellular movement is one of the central questions in collective cell migration. Although research is needed to answer this question, the dynamics of the plasma membrane will certainly be an important part of the answer.

The interplay between cytoskeletal dynamics and the plasma membrane is also highly relevant to the motility of nematode sperm cells, which is based on polymerization of the major sperm protein (MSP) (Bottino et al. 2002; Stewart and Roberts 2005). Although this system is biochemically remote from actin-based crawling, the biophysical aspects of movement are rather similar. Recent work has revealed a clear connection between membrane tension and sperm cell motility (Batchelder et al. 2011). The membrane tension in sperm cells was perturbed by osmotic shock or detergents, and although this seemed to have a negligible effect on rates of MSP polymerization, it had a substantial effect on lamellipodial organization; higher tension promoted longer and more oriented filaments which resulted in increased speed, whereas lower tension resulted in a less organized, slower moving lamellipodium with shorter filaments. Research is needed to better understand the interplay between MSP dynamics and the membrane in sperm cells; the insights gained from this will surely also shed light on actin-based motility.

This review emphasizes the important role of the plasma membrane in cell motility and stresses the inherent coupling between the biochemical composition of the membrane and its biophysical characteristics. Research in recent years has led to substantial progress in this direction through experiments in simple in vitro model systems and in vivo in live cells. Yet many fundamental questions remain unanswered even at the level of a single migrating cell. What determines the composition of membrane proteins and lipids in a motile cell? How are membrane components recycled? What is the function of membrane transport? What determines the size and shape of the cell? What determines membrane tension? While, obviously, knowledge of the biochemical components and reactions involved is necessary to answer these questions, it is becoming apparent that one cannot ignore biophysical aspects of membrane structure and dynamics. Research will need to address these important questions and achieve more comprehensive understanding of the dynamics of the cell membrane and its relationship to the motility process.