Variation of Stereothreshold with Random-Dot Stereogram Density

Abstract

Purpose

Reports of dissimilar stereothresholds for contour and random-dot (RD) targets may reflect differences in stimulus properties or differences between local and global stereoscopic processing mechanisms. In this study, we evaluated whether the stereothresholds obtained using low- and high-density RD stimuli are consistent with a distinction between local and global disparity processing.

Methods

Stereothresholds were measured in eight normal subjects for a small disparate line segment superimposed on RD surrounds with densities that ranged between 0.07% and 28.3%.

Results

Stereothresholds averaged 0.23 arc min for a RD density of 0.39% and approximately doubled for lower and higher densities. The increase in stereothresholds at low densities is likely due to the increased spacing between elements, which reduces their usefulness as a reference for relative disparity judgments. The increase in stereothresholds at high densities is attributed to a crowding effect.

Conclusions

Because the stereothresholds measured with RD stimuli of low and high density are limited by different constraints, they can be considered to be different types of stereotargets. However, because the stereothresholds measured for RD targets of varying densities are similar to those determined previously for a local, two-rod stereotarget, it is likely that all of these stimuli are processed by a single disparity-processing mechanism.

Stereograms consist of two-dimensional monocular half-images that are combined by the brain into a three-dimensional depth percept. The two half-images contain reference elements that are in the same relative locations and test elements that are offset in their relative spatial locations in the two eyes. This offset, or relative binocular disparity, causes a perception of depth. The stereothreshold is the smallest relative binocular disparity that yields a perception of depth. Stereopsis has been categorized as “local” and “global,” but the definitions of these terms are ambiguous.

Julesz¹ distinguished between local and global stereograms based on the number of dots contained within the stereogram. This definition was adopted by Westheimer & McKee² who formed a stereogram that they considered “local” because it only contained 49 dots. In support of the distinction made by Julesz, Pressmar & Hasse³ reported phenomenologically different responses to low density and high density random dot stereograms (RDSs), which the authors considered to be local and global stereograms, respectively.

Some researchers describe local stereograms as stimuli composed of individual features or contours seen in depth, such as line stereograms, and global stereograms as complex, patterned stimuli composed of many features, such as RDSs.^{4,5, 6} This definition is also consistent with the distinction between the stereogram types based on element density, because RDSs that contain very few dots are essentially isolated contours seen in depth; whereas RDSs that contain a high density of dots comprise textures with many features.

Clinically, stereopsis is assessed commonly using local contour stereograms (e.g. the Titmus circles or the Randot stereotest for fine disparities),^{7, 8} although global random-dot based tests also are available (e.g., Preschool Randot test and TNO Stereo Test). Discrepant results for stereothresholds assessed with local and global stereograms have been reported.^8–10 In contrast, Fawcett⁷ only found differences between the thresholds for local and global targets in patients with abnormal binocularity, although the similar thresholds obtained for normal subjects could reflect a floor effect because the tests used in this study could not measure stereothresholds lower than 40 arc seconds. However, Stevenson, Cormack, & Schor¹¹ reported similar average thresholds on the order of a few arc seconds using global RDSs and the local Howard Dolman Apparatus. Disagreement about the similarity between thresholds using local and global stereotargets may be explained by differences in the properties of the targets,^12,11 such as the spacing between the constituent elements,^{8, 13, 14} size,^15–17 contrast,^18–22 viewing duration,^{9, 22, 23} and spatial frequency content,^24–26 or, alternatively, by differences in the neural mechanisms that underlie the processing of local and global stereograms.²⁷

Consistent with the second alternative, Westheimer²⁸ distinguished between local processes that ascribe depth values to individual features and global processes that ascribe depth values to the overall configuration and are relatively unaffected by the depth values of the constituent internal features. The perception of depth in a dense RDS presumably would reflect the operation of the latter, global process.

Other authors distinguish between local and global processing mechanisms based on the extent of the correspondence problem – the matching ambiguity that exists in global stimuli.^{1, 29–32}

Attributing their definition to Julesz, Bruce, Green, & Georgeson³³ define local stereopsis as the mechanism that processes edge or dot stimuli, and global stereopsis as the mechanism that processes complex pattern stimuli that involve the evaluation of many possible local matches. Similarly, global stereopsis has been described as the integration across multiple pattern elements to solve the correspondence problem by selecting the disparity for which the maximum number of elements in the two half views are matched.^{32, 34}

Still other authors claim that local and global processing mechanisms share a common disparity-processing stage, followed by a subsequent, global level of analysis to resolve the ambiguity that is produced when multiple possible stereoscopic matches exist.^{1, 35}

To determine whether different neural mechanisms process local and global stimuli, it is necessary to form local and global stereograms that have similar properties. Based on the definition that a small number of dots should appear as local, isolated targets in depth and that many dots should appear as a random-dot texture and not as individual dots in depth,¹ it should be possible to obtain both local and global stereograms by varying the density of the same RDS. Although previous studies examined changes in correlation thresholds³⁶ and stereoscopic efficiency³⁷ as a function of RD density, to our knowledge, the effect of RD density on stereothresholds for depth discrimination has not been evaluated systematically.

Following Tyler,²⁷ we provisionally define a local disparity mechanism as one that processes the disparity at one location in the field without reference to the disparities at other regions of the field, and a global disparity mechanism as one that involves interactions between local disparity mechanisms. One outcome of this interaction may be the assignment of depth information to empty regions between texture elements to form a disparity-defined surface, which Wilcox & Duke³⁸ found to occur when approximately 10% of the stereogram area is covered by texture elements. In addition, densely spaced texture elements in a stereogram undergo disparity averaging^{11, 39, 40} and result in a reduced sensitivity to differences in disparity.^{2, 41} For example, Tsirlin et al.⁴² reported that the disambiguation of two (or more) stereoscopic surfaces separated by 1.9 arc minutes of disparity worsens with increasing RD density.

To evaluate whether the stereothresholds obtained using low- and high-density RDSs are consistent with the distinction made above between local and global targets, in this study we superimposed a small disparate line segment (i.e. a single feature) within RDS surrounds of various densities. If the RDS stimulus is processed by a local disparity mechanism, then the disparities of the random dots and the line segment should be determined independently and the depth value of the line segment should remain conspicuous regardless of the RD density. However, if the stimulus is processed by a global disparity mechanism, then the depth of the line segment should be less easily discriminated as its disparity is averaged, at least to some extent, with the disparity of the surrounding dots. A hierarchical processing scheme, in which local disparity extraction is followed by global interactions between local disparity mechanisms²⁷ also is consistent with a decrease in the ease of discrimination of the disparate line segment. We therefore expected that the stereothreshold for the line segment would increase when it was embedded in RDSs of high density, if the processing of high-density stereotargets reflects the operation of a global disparity mechanism.^*

METHODS

Subjects

Eight healthy, fully corrected subjects between the ages of 24 and 58, including the two authors, participated in the experiment. Each subject had a stereothreshold of at least 40 arc seconds for both crossed and uncrossed disparities, as measured with the Titmus Stereo Test (Stereo Optical Co., Chicago, IL, USA). The research adhered to the tenets of the Declaration of Helsinki, and the experimental protocol was reviewed by the University of Houston Committee for the Protection of Human Subjects. Informed consent was obtained before the experiments were conducted.

Stimuli

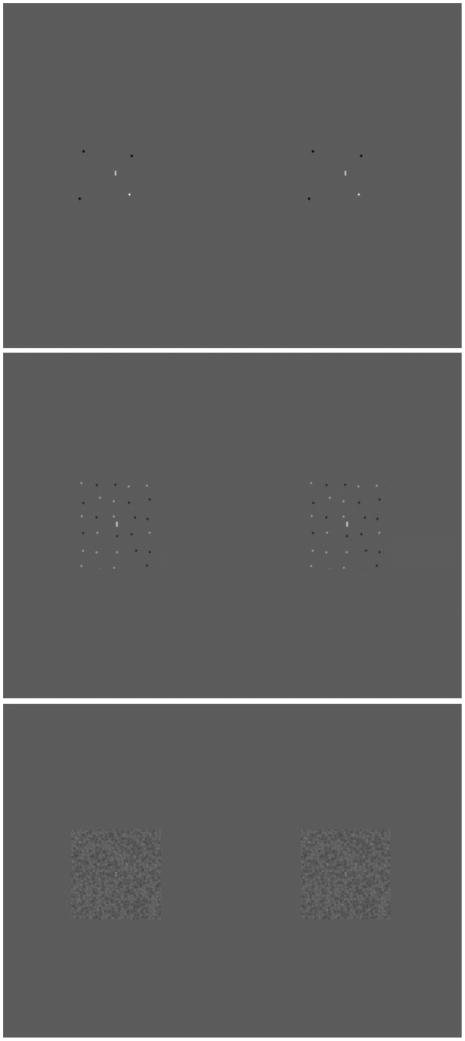
The stimuli were created in Matlab (The MathWorks; Natick, MA) with the Psychophysics toolbox ^{43, 44} and displayed on a gamma-corrected, 29-cm wide LCD monitor of 1024 X 768 pixels. Each pixel is 0.03 cm in size and subtends 0.85 arc minutes at the viewing distance of 114 cm. As illustrated in Figure 1, the stimuli comprised two identical half images of static 171 arc minutes X 171 arc minutes RDSs that were presented side-by-side on the laptop monitor. To ensure that the mean luminance of the display did not vary with dot density, each dot contained within the RDS was randomly either darker or lighter than the gray background.

Figure 1.

Stimuli to assess stereothresholds. One frame of two identical half images of (a) 0.07%, (b) 0.92%, and (c) 28.3% density 171 arc minutes X 171 arc minutes RDSs, presented side-by-side on the laptop monitor, with a superimposed line segment. In the experiment, the contrast of the RDs and the line segments shown in each panel were adjusted to the same multiple of the observers’ contrast detection thresholds.

To measure stereothresholds for dots that are offset in the stereogram by less than one pixel,⁴⁵ each 1-pixel dot was convolved with a Gaussian envelope (SD= 1.75 pixels), to yield dots with a full width at half height (corresponding to ±1.15 standard deviations) of approximately 4 pixels (3.5 arc minutes). Each RDS was constructed from a regularly spaced array of Gaussian dots, to which horizontal and vertical position jitter was added with a SD equal to 15% of the average dot-to-dot spacing. To produce relative disparities, the Gaussian dots (but not the superimposed line segment) were offset horizontally by half of the required positional offset in opposite directions in the two half images. Eight densities of the RDS background were selected, ranging from 0.07% to 28.3%. We define the area of each dot as the proportion of its Gaussian amplitude that is either 50% of the maximum value above or 50% of the maximum value below the mean luminance. Because the dot diameter corresponded to 4 pixels, the area of each dot was 4π, or 12.57 square pixels. The RDS density is defined as the percentage of the total stereogram area that is covered by dots.

A single 1 X 13 pixel bright line segment was convolved with the same Gaussian envelope, and transparently pasted at the center of the RDS, plus or minus 1.8 arc minutes (SD) of horizontal and vertical position jitter. The transparent paste operation inserted the line into the RD image without obscuring the neighboring background dots and prevented wrap around of the luminance values^*.

During each stimulus presentation, one of 15 possible arrangements of dots for each density was randomly selected and presented for 500 ms.

Stimulus Presentation

Experiments were performed in a dark room. A mirror haploscope and an opaque divider mounted between the haploscope mirrors in the median plane provided full separation between the half images presented to each eye.^46,47 Prior to the experiment, the positions of the haploscope mirrors were adjusted for each subject to produce perceived alignment of a pair of vertically separated flashed Nonius lines, in order to minimize horizontal fixation disparity.

Variation of Stereothreshold with RD Density

To match the detectability of the vertical line segment that was superimposed on RDSs with different densities, contrast-detection thresholds for both the line segment and the RDS images were measured prior to the experimental sessions. For each subject, the RDS dot and line contrasts were adjusted for equal visibility across dot densities (see the Appendix - available at [LWW insert link]).

Although retinal image disparities were introduced into the background of RDs and the superimposed line segment always had zero disparity, subjects judged whether the line segment was in front or behind the RD surround. Prior to the experiment, subjects ran a set of approximately 20 practice trials at each RD density to become familiar with the task. No additional practice was deemed necessary, as the subjects were familiar with the feedback sounds and the use of the auxiliary button box from the preceding contrast-detection experiments.

In each session, one of eight RD densities was tested. A session consisted of a total of 70 trials, in which 7 disparities (3 crossed, 3 uncrossed, and 0 disparity) were presented in a random order using the method of constant stimuli. After each response, audio feedback was provided to indicate if it was correct or incorrect. Within a session, the range of disparities was selected individually to obtain a suitable psychometric function, which plotted the percentage of “near” responses as a function of disparity. The psychometric function was fit with a cumulative Gaussian and the threshold was estimated as the semi-interquartile range (=0.675 X SD) of the fitted cumulative Gaussian function. Stereothresholds were measured at least twice for each observer, in a random order.

RESULTS

Stereothresholds for depth discrimination of a line superimposed on RDSs with different dot densities average approximately 0.23 arc minutes, corresponding to 13.8 arc seconds, for a RD density of 0.39% and approximately double for both lower and higher RD densities (Figure 2, upper axis). The effect of dot density on the stereothresholds is significant (Repeated Measures ANOVA, F_df=7,49=3.01, p=0.03, Huynh-Feldt corrected). Pairwise comparisons indicated that the stereothreshold measured with 0.07% density differs significantly from all but the two highest RD densities (p values range from 0.002 to 0.038, Huynh-Feldt corrected) and that the stereothreshold measured with 0.39% density differs significantly from that for 28.28% density (F_df=1,49= 5.87, p= 0.035, Huynh-Feldt corrected).

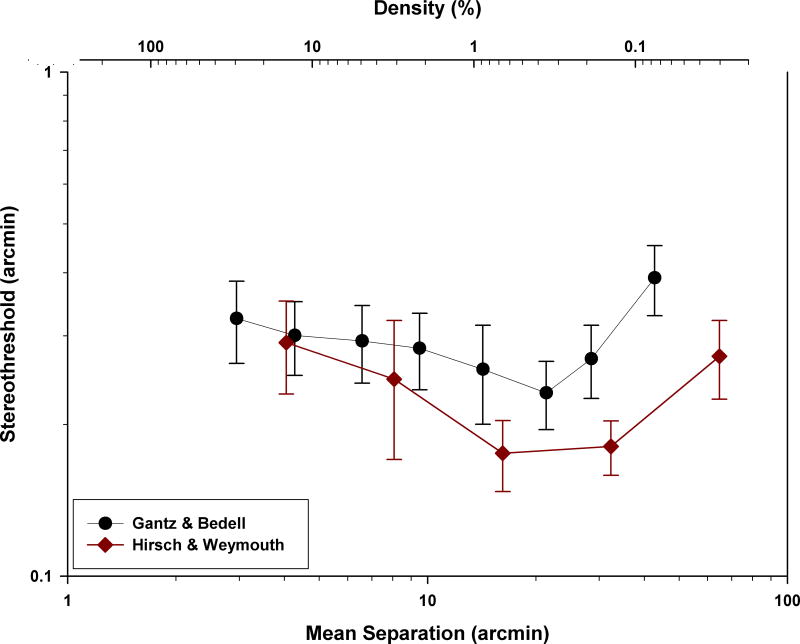
Figure 2.

Mean stereothresholds for depth discrimination of a line superimposed on RDSs with various dot densities (black circles) are plotted as a function of the mean line-to-dot separation, equal to one half of the mean RD separation (lower axis) and the RD density (upper axis). Error bars represent the standard error of the mean. Stereothresholds are approximately 0.23 arc minutes for a RD density of 0.39%, and increase at both lower and higher densities. For comparison, data are shown from a previous study (filled diamonds) that measured stereothresholds for two rods that varied in their horizontal separation.

DISCUSSION

Stereothresholds are lowest at a background RD density of 0.39% (mean line-to-dot separation of 21.4 arc minutes) and increase for lower and higher RD densities. For small mean line-to-dot separations (high RD densities), the results are consistent with a study by Westheimer and McKee² that measured stereothresholds with a 49-element random-dot stimulus with various inter-element separations. They found that stereothresholds increase for small inter-element separations (i.e., at high densities), starting when the separation reaches approximately 10 arc minutes. Because they varied the element separation by scaling the entire target’s size, a change in the number of dots can not account for the increase in stereothreshold at high element densities. Instead, they and other authors attributed the increase in stereothreshold that occurs at small inter-element separations to a crowding effect – an interference in localizing one element produced by the presence of neighboring elements.^{2, 48, 49} Disparity averaging also is reported to occur for dense stereoscopic stimuli^{11, 39, 40, 42} and has been suggested to contribute to the crowding effect for stereothresholds.⁴⁹ In support of this suggestion, studies that examined crowding for orientation discrimination and letter acuity found that the features and locations of the surrounded and flanking targets undergo averaging, even when observers can clearly differentiate the individual component stimuli.^{50, 51}

Although the study by Westheimer & McKee² did not measure stereothresholds for inter-element separations larger than 15 arc minutes, other studies demonstrated an increase in stereothreshold with a further increase in element separation.^{46, 52} Large separations between the test and reference elements may degrade the usefulness of the reference target for determining the relative disparity of the test elements. As illustrated by the filled diamonds in Figure 2, Hirsch & Weymouth⁵² also reported that stereothresholds increase at both small and large target separations, using a target that consisted of two horizontally separated rods. The similar trend shown for these local rod targets and for the small line segment superimposed on our static RD targets suggests that both types of targets are processed by the same disparity-processing mechanism. This suggestion is consistent with the results of a recent perceptual-learning experiment, in which the improvement of stereothreshold produced by training observers with sparse RD targets transferred to dense RD targets, and vice versa.⁴⁷

The goal of this study was to examine which RD densities could be considered to represent local and global stereograms. Under the assumption that local and global stereotargets are processed by different mechanisms we hypothesized that stereothresholds for an embedded line segment would be best for low densities of the background RDS, when individual elements are processed separately by local disparity mechanisms, and would be worse for high RDS densities, when the disparities of the line and nearby RDs can interact within a global disparity mechanism. However, we found that stereothresholds for static RDSs are optimal for 0.39% density RDSs, and worsen for both lower and higher densities. For low densities, these findings are consistent with those of other authors, who attribute the results to the increased spacing between elements, which reduces the usefulness of the reference target for relative disparity judgments. For high densities, our findings are consistent with an elevation of stereothresholds because ofcrowding, which may be attributable to disparity averaging.^{11, 39, 40, 42} and occurs regardless of whether the individual elements in the stereogram are distinguishable.^{2, 41, 48} Because the stereothresholds measured with RDSs of low and high density are limited by different constraints, they can be considered to be different types of stereotargets. Low density RDSs (<0.39%) may be considered “local” stereotargets, and high density RDSs (>0.39%) may be considered “global” stereotargets. However, because of the similarity between the stereothresholds measured using a range of RD densities and the stereothresholds measured with a two-rod stereotarget (a “local” stereotarget), it is likely that all of these stimuli are processed by a single disparity-processing mechanism.

APPENDIX

The appendix is available at [LWW insert link].