Membrane tension leads the way

Tension in the plasma membrane of adherent cells arises from the in-plane tension in the lipid bilayer, as well as from the adhesion between the membrane and the cytoskeleton (1). The membrane tension exerts a force along the cell boundary that affects all processes involving membrane deformations, including edge extension, endocytosis, and exocytosis. As such, membrane tension has been implicated as an important factor in a wide range of cellular phenomena, including cell migration, cytokinesis, mitosis, and intracellular trafficking. Despite its importance, little is known about the mechanisms by which membrane tension regulates these processes and in particular about the feedbacks between tension and the biochemical pathways involved. In PNAS, Gauthier et al. (2) show that membrane tension coordinates the activation of exocytosis and contraction during actin-based cell spreading. Their results nicely illustrate how membrane tension can serve as a global mechanical regulator that couples different biochemical processes occurring at diverse locations along the cell boundary.

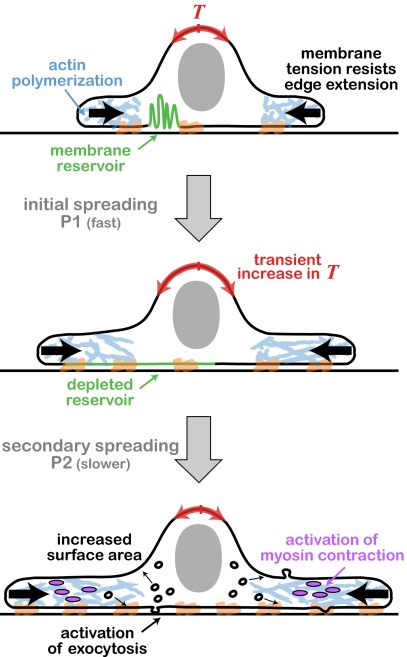
Spreading fibroblasts provide a convenient model system to investigate the relation between membrane and cytoskeletal dynamics. The spreading process consists of two distinct phases (Fig. 1) (3). Initial cell spreading after plating the cells on a substrate (P1) is driven by actin polymerization, while the effective cell surface area increases by drawing from a membrane reservoir of folded regions and blebs (2). After the depletion of this reservoir, there is an abrupt transition into the second spreading phase (P2), whereby additional plasma membrane is recruited from internal membranes through the activation of exocytosis (4). Actin-driven protrusion is typically slower in this phase, which is further characterized by periodic cycles of myosin-induced contraction (5).

Fig. 1.

Schematic illustration of the phases observed during fibroblast spreading (2). A suspended fibroblast is plated on a coated glass substrate (Top). The initial phase (P1) is characterized by rapid spreading driven by actin polymerization. This spreading is accompanied by gradual unfolding of membrane reservoirs and stretching out of the plasma membrane. The depletion of the membrane reservoir at the end of P1 leads to a transient increase in membrane tension (Middle). The increase in tension activates exocytosis and myosin contraction. Subsequent spreading (P2) is enabled by an increase in cell surface area due to the enhanced exocytic activity. This spreading phase is characterized by a gradual reduction in edge velocity and local cycles of protrusion and acto-myosin contraction (Bottom).

What is the signal that coordinates the simultaneous changes in protrusion dynamics, myosin contraction, and cell surface area at the transition between these two spreading phases? Gauthier et al. hypothesize that membrane tension might be involved (2). To measure tension they used a tether-pulling assay in which a bead pulled by optical tweezers extracts a membrane tether from the cell surface, and the restoring force is measured (6). Using this assay they were able to monitor the tension in a spreading fibroblast as it underwent the transition between the initial rapid spreading phase (P1) and the subsequent contractile spreading phase (P2). They found that this transition is accompanied by a transient increase in membrane tension (Fig. 1). To show that this transient increase in membrane tension is indeed the signal responsible for activating the transition, Gauthier et al. increase tension artificially by placing cells in hypoosmotic media (2). These conditions cause an abrupt arrest of edge extension and an induction of exocytosis, indicating that tension increase not only accompanies but can also trigger the transition between the two different spreading modes.

Overall the work of Gauthier et al. demonstrates that membrane tension acts as a mechanical regulator of multiple cellular processes, including actin-driven edge extension, exocytosis, and myosin contraction. A transient increase in membrane tension due to the depletion of the membrane reservoir coordinates changes in all these processes that together produce the sharp transition observed in the global spreading behavior of fibroblasts.

Two recent studies (7, 8) provide further support for the important role of membrane tension as a mechanical regulator of cell morphology and movement. Sinha et al. (7) showed that caveolae, which are cup-shaped membrane invaginations, allow endothelial and muscle cells to quickly change their surface area. Caveolae rapidly flatten out in response to transient increases in membrane tension induced by cell stretching or swelling, thus allowing cells to increase their effective cell surface area and buffer changes in tension. Cells invest large amounts of energy in generating and maintaining caveolae through an ATP- and actin-dependent mechanism, likely because these dynamic membrane structures allow for response to mechanical insults and prevent rupture of the plasma membrane.

Batchelder et al. (8) recently investigated the effect of membrane tension on the dynamics of nematode sperm cell motility, which is driven by the polymerization of the major sperm protein (MSP) rather than actin. The membrane tension in these sperm cells was perturbed by osmotic shock or detergents, and although this seemed to have a negligible effect on MSP polymerization rates, it had a substantial influence on lamellipodial organization: higher tension promoted longer and more oriented filaments, which resulted in increased speed, whereas lower tension resulted in a less organized, slower moving lamellipodium with shorter filaments. This work shows that membrane tension plays a similar role in coordinating local biochemical reactions over cellular scales in a system that is biochemically distinct from the actin-based cell spreading (2).

The work by Gauthier et al. (2), along with these and other recent results (7–9), highlights membrane tension as an important mechanical regulator of cell behavior. Moreover, because membrane tension equilibrates rapidly over cellular scales (10), it provides a simple means for global coordination of local molecular processes across the cell. However, much remains unknown. What actually determines membrane tension? How does the transient increase in tension trigger the onset of exocytosis and myosin contraction? Why do exocytotic activity and contraction remain high during subsequent spreading, even though after the transient increase the membrane tension decreases to a level below its initial value?

In general, it is becoming apparent that biochemical pathways are intricately coupled to the biophysics of dynamic cellular processes. In particular, biophysical characteristics such as membrane tension play a central role in coordinating biochemical processes across the cell. Thus, future progress in understanding complex cell behaviors will likely depend on our ability to integrate the biochemical and biophysical aspects of cellular biology into a coherent and dynamic picture.