Abstract
Brightness induction is the modulation of the perceived intensity of a region by the luminance of surrounding regions and reveals fundamental properties of neural organization in the visual system. Grating induction affords a unique opportunity to precisely measure the temporal properties of induction using a quadrature motion technique. Contrary to previous reports that induction is a sluggish process with temporal frequency cutoffs of 2-5 Hz (DeValois, Webster, Devalois, & Lingelbach, 1986; Rossi & Paradiso, 1996), we find that induction is nearly instantaneous. The temporal response of induced brightness differs from that of luminance gratings by a small time lag (< 1 ms), or by a small temporal phase lag (< 0.016 cycle), and remains relatively constant across wide variations in test field height. These data are not easily explained by an edge-dependent, homogeneous filling-in process (Rossi & Paradiso, 1996), however, they are consistent with an explanation of brightness induction based on spatial filtering by cortical simple cells (Blakeslee & McCourt, 1999).
Keywords: Brightness induction, grating induction, temporal vision, spatial vision, psychophysics
Introduction
Brightness induction is the modulation of the perceived intensity of a region by the luminance of its surround. Induction reveals fundamental properties of neural organization in the visual system and its spatial characteristics have been extensively studied (Heinemann,1972; Yund & Armington,1975). The temporal properties of brightness induction, however, are less well understood. DeValois, Webster, DeValois and Lingelbach (1986) measured the temporal response of simultaneous brightness contrast (SBC), where a gray patch on a dark background looks brighter than an equivalent gray patch on a bright background (Fig. 1 a-c). Observers adjusted the luminance modulation of a comparison patch to match the changing brightness of a test patch that underwent either luminance-modulation or surround-induced brightness modulation. Brightness changes in the luminance-modulated test patch were stable over temporal frequencies from 0.5 to 8 Hz. Surround-induced brightness changes, however, decreased rapidly for frequencies above 2.5 Hz, leading to the conclusion that brightness induction was a slow process.
Figure 1.
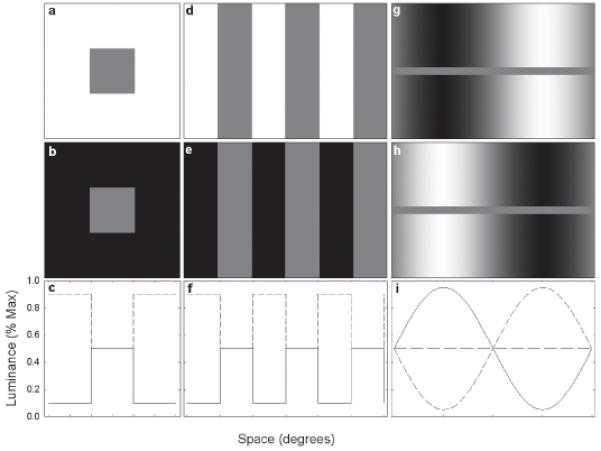
Comparison of the SBC stimuli used by DeValois et al. (1986) (a-c) and Rossi and Paradiso (1996) (d-f) with the GI stimulus (g-i). The lower row of panels illustrates horizontal one-dimensional slices through the stimuli in the upper row (short-dashed lines) and middle row (solid lines) representing the two extreme temporal phases. Note that for the GI stimulus the homogeneous test field is represented by a separate horizontal slice (long-dashed line).
Rossi & Paradiso (1996) investigated whether the size of the induced region influenced the temporal properties of induction. They temporally modulated every other bar of a square-wave grating while holding the luminance of the intervening bars (the induced regions) constant (Fig. 1 d-f). The temporal frequency cutoff for induction decreased as bar width increased. Narrow bars (0.25°) possessed cutoff frequencies of 1.5 to 5 Hz; for wider bars (16.7°) this decreased to 0.5 to 2.0 Hz. The authors noted that the critical flicker-fusion frequency (CFF) for luminance-modulated stimuli under the conditions of their experiment would, if measured, have been much higher, and would have been expected to increase (rather than decrease) with increasing bar size. Rossi & Paradiso (1996) proposed that a “fast” process accounted for the high CFF for luminance modulation and for the increase in the CFF with the size of the modulated area, and that a slower “filling-in” process was responsible for the induced brightness modulations. DeValois et al. (1986) and Rossi and Paradiso (1996) also attempted to measure the phase (time) lag of induction, but found that these measurements were extremely difficult and imprecise.
Grating induction (GI) is a brightness effect in which a sinusoidal luminance grating induces a counterphase spatial brightness variation (a grating) in an extended homogeneous test field (Fig. 1 g-i) (Foley & McCourt, 1985; McCourt, 1982). Unlike other brightness induction effects, including SBC and the square-wave stimulus employed by Rossi and Paradiso (1996), the spatial pattern of brightness induced in the test field of the GI stimulus is not readily explained by an edge-dependent, homogeneous filling-in process like that proposed by Rossi and Paradiso (1996). Blakeslee and McCourt (1997; 1999; 2001; 2004; 2005) have provided strong evidence for an alternative explanation in which GI, SBC and a host of other brightness illusions result from the normalized outputs of oriented spatial filters summed across multiple spatial scales and argue that a filling-in mechanism is not required to explain any of these brightness effects.
If the brightness of both surround-induced and luminance-modulated stimuli is the product of oriented spatial filtering across multiple spatial scales, one would expect the temporal properties of luminance- and surround-induced brightness modulation to be similar. GI offers a way to test this hypothesis using a novel and precise technique to measure the temporal properties of induction. Because the brightness variations induced in the test field of the GI stimulus are sinusoidal (Fig. 1 g-i) (Foley & McCourt, 1985; McCourt, 1982; McCourt, 1994), a quadrature motion technique (Stromeyer, Kronauer, Madsen & Klein, 1984) can be used to exploit the visual system’s excellent motion sensitivity to leverage even small temporal phase differences into conspicuous changes in motion direction. Two counterphasing gratings (standing waves) whose phases are in spatial and temporal quadrature (i.e., differ by ¼ cycle) sum linearly to produce a moving grating (a traveling wave) (Fig. 2). The traveling wave moves leftward when the temporal phase difference is −¼ cycle (Fig. 2 a-c) and rightward when the temporal phase difference is +¼ cycle (Fig 2 g-i). When the temporal phase difference is 0 cycles the sum is itself a standing wave, for which the leftward and rightward motion energy is equal and motion direction is ambiguous (Fig. 2 d-f).
Figure 2.
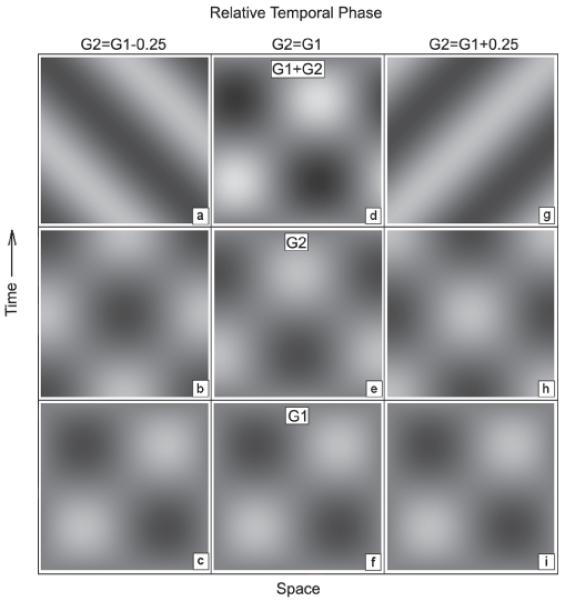
Space-time diagrams illustrating the quadrature-motion experimental technique. Panels (c, f, i) illustrate one temporal cycle of a counterphasing grating (G1: luminance or induced). Panels (b, e, h) illustrate a luminance grating (G2) added to the test field in quadrature spatial phase to G1, in three relative temporal phases: −.25 cycles (b); 0 cycles (e) and +.25 cycles (h). Panels (a, d, g) illustrate the compound grating G1 + G2 for each combination. Leftward motion results when the temporal phase of G2 < G1, rightward motion occurs when G2 > G1, and motion energy is balanced when G2 = G1. Our technique identifies the temporal phase of an added luminance grating which matches the temporal phase of an induced grating (experimental condition) or of a luminance grating (control condition) and results in an ambiguous motion percept.
By counterphasing the inducing grating of a GI stimulus we produce a counterphasing induced grating whose spatial phase is precisely opposite to that of the inducing grating (Blakeslee & McCourt, 1997; McCourt, 1994), but whose temporal phase lags the inducing grating by 180° plus some unknown quantity whose value depends on the time lag of brightness induction. To the induced (test field) grating we add a like-frequency luminance grating counterphasing in spatial quadrature to the inducing grating (and to the induced grating), but varying in temporal phase. The temporal phase of the added luminance grating producing a sum whose motion energy is perceptually left/right balanced reveals the temporal phase of the induced grating.
Methods
The authors (BB and MM) and two naïve observers (NP and AZ) participated in the experiments. All four subjects possessed normal or corrected-to-normal vision. Each subject provided informed consent and protocols were approved by the NDSU IRB.
Stimuli were initially presented on a 22” Mitsubishi DiamondPro (model 2070) CRT display with a frame refresh rate of 140 Hz. To ensure that temporal resolution exceeded 8 temporal phases per cycle, temporal modulation frequency was capped at 16 Hz. An additional 20” flat-screen Clinton monoray (DP104 phosphor) display with a refresh rate of 199 Hz was employed to study induction at counterphase frequencies up to 24 Hz. Data collected under identical conditions for the two displays were compared and no differences were found. Stimuli were generated and presented using MATLAB routines to control a Cambridge Research Systems ViSaGe system (14-bit intensity resolution per channel). Gamma linearization was accomplished via look-up tables following photometric calibration. Display format was 1024 (w) × 768 (h) pixels for the Mitsubishi monitor. Viewing distance was 57 cm resulting in a stimulus field that was 40° in width and 30° in height. Individual pixels measured 0.039° × 0.039°. Display format was 640 (w) × 480 (h) pixels for the Clinton monitor. The viewing distance was adjusted to 51 cm producing a stimulus field that was 38° in width and 28° in height. Individual pixels measured 0.059° × 0.059°. Mean display luminance for both displays was 50 cd/m2.
The inducing gratings of the GI stimuli filled the stimulus field surrounding the homogeneous test field. Inducing gratings were presented at 100% contrast with a spatial frequency of 0.0625 c/d. Inducing gratings were counterphased at 2, 8, 16 and 24 Hz. Test field heights were 0.5°, 3.0°, 6.0° and 9.0°. The luminance grating added to the test field was of the same spatial frequency as the inducing grating, was in spatial quadrature phase to the inducing grating, possessed a contrast that linearly ramped from 0.0 to 0.80 over the 1.5 sec stimulus presentation interval, and completely filled the test field. The test field luminance grating possessed 41 temporal phases ranging from ± 0.10 cycles. The psychophysical task was a forced-choice “left” versus “right” motion judgment of the induced + luminance grating compound in the test field. Because motion judgments are not criterion-free, a control condition (luminance + luminance grating) was included in which there was no inducing grating. Instead, a luminance grating was added to the test field which possessed the contrast, spatial frequency and temporal frequency necessary to mimic the induced grating. To this induced grating mimic was added the same luminance grating in fixed spatial quadrature phase but variable temporal phase used in the experimental condition. Based on results from previous studies (McCourt & Blakeslee, 1994), the contrast of the mimic grating was set to the mean of the luminance ramp of the added luminance grating. Stimuli were presented with central fixation for 1500 ms. Blocks of 41 trials were run for each combination of temporal frequency and test field height. The temporal phase of the added luminance grating was randomized within blocks. Subjects participated in 10-30 blocks of trials for each condition.
Psychometric data were fitted with a two-parameter (midpoint and slope) cumulative-normal function using a maximum-likelihood criterion. The fitted midpoint parameter corresponds to the temporal phase of the added luminance grating yielding 50% “right” motion responses and was taken as an estimate of the point of subjective equality (PSE). For each condition we obtained confidence intervals (± 2 s.e.m.) using a bootstrap analysis (Foster & Bischof, 1991) of 1000 iterations where each iteration resampled the data at each level of temporal phase from a binomial process whose mean was equal to the proportion of “right” motion responses at that level. Resampled psychometrics from each iteration were fitted in the same manner as the original data; this process yields a distribution of PSEs whose standard deviation was used to compute 95% confidence intervals.
Results
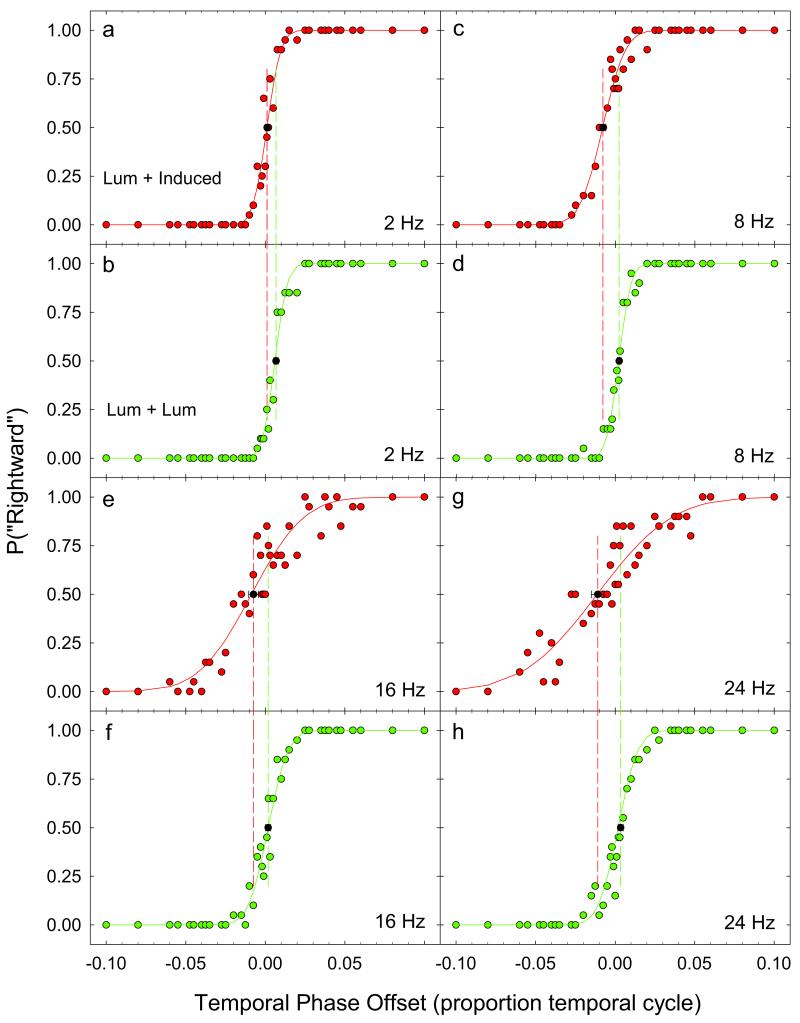
Psychometric functions from one subject (MM) are plotted for the 2, 8, 16 and 24 Hz temporal frequency conditions in Fig. 3 a-h. The upper and lower panels plot data from the experimental (luminance + induced) and control conditions (luminance + luminance), respectively, for a 0.0625 c/d spatial frequency inducing grating and a 0.5° test field height. Proportion “right” motion judgments are plotted against the temporal phase offset (expressed in proportion of a temporal cycle) of the added luminance grating, and are fit with cumulative normal distributions. The point of subjective equality (PSE) corresponds to the temporal phase offset that results in a “right” motion judgment by the subject on 50% of the trials. In order to determine the error associated with this measure we calculated the bootstrap PSE and the 95% confidence intervals (Foster & Bischof, 1991), indicated in each figure by the filled symbols and horizontal error bars. The difference between bootstrap PSEs in the experimental and control conditions was taken as a measure of the phase (time) lag of induction for that experimental condition. Fig. 4 (a-d) plots separately for each subject bootstrap PSE differences as a function of temporal frequency. Test field height (0.5°, 3.0°, 6.0° and 9.0°) is indicated by the different symbols.
Figure 3.
Psychometric functions from one subject (MM) for the 2, 8, 16 and 24 Hz temporal frequency conditions. The upper and lower panels plot data from the experimental (luminance + induced) and control conditions (luminance + luminance), respectively. For each condition, proportion “right” motion judgments are plotted against the temporal phase offset (expressed in proportion of a temporal cycle) of the added luminance grating and are fit with cumulative normal distributions. The point of subjective equality (PSE) corresponds to the temporal phase offset that results in a “right” motion judgment by the subject on 50% of the trials. The bootstrap PSE and the 95% confidence intervals (15) are indicated in each figure by the filled black symbols and horizontal error bars. The difference in the value of the bootstrap PSE between the experimental (luminance + induced) condition (upper panel, red symbols) and the corresponding control (luminance + luminance) condition (lower panel, green symbols) was taken as a measure of the phase (time) lag of induction for that condition. This difference is illustrated by the dashed lines drawn through the bootstrap PSE for the experimental (red dashes) and control (green dashes) conditions.
Figure 4.
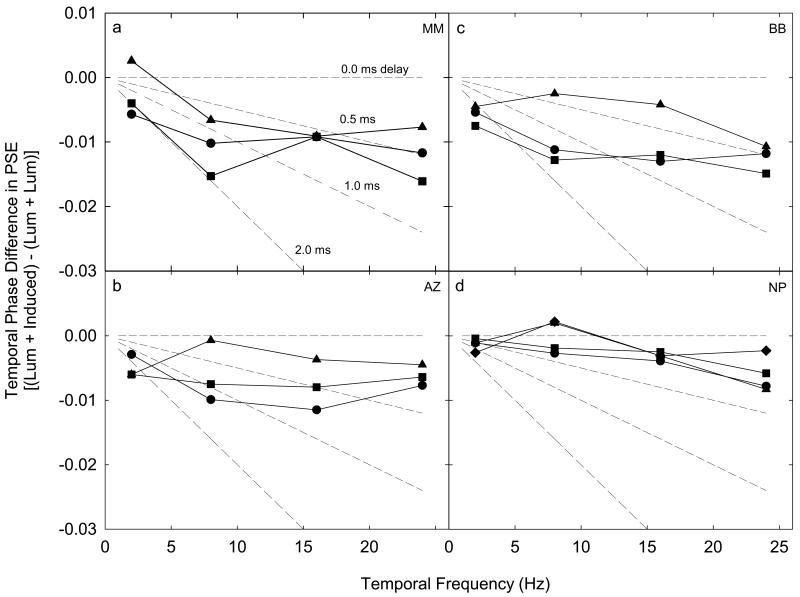
The phase (time) lag of induction as a function of temporal frequency and test field height. Panels (a-d) plot separately for each subject the difference in the bootstrap PSEs between the experimental (luminance + induced) and control (luminance + luminance) conditions as a function of temporal frequency. Test field height is indicated by the different symbols (circles = 0.5°, squares = 3.0°, triangles = 6.0°, diamonds = 9.0°). The dotted lines depict the predicted differences in the PSEs between experimental and control conditions as a function of temporal frequency which would correspond to fixed induction time lags of 0.0, 0.5, 1.0 and 2.0 ms.
In all conditions subjects show very small temporal phase lags of less than 0.016 of a cycle (5.7 degrees temporal phase). The dotted lines in each panel depict predicted differences corresponding to fixed induction time lags of 0.0, 0.5, 1.0 and 2.0 ms. At 24 Hz, where measurement of the time lag is most accurate, the time lag is consistently less than 1.0 ms. A constant time lag will produce an increasing phase lag as temporal frequency increases. Such a trend is absent from the data indicating that the fixed time lag is either very short (i.e., very shallow slopes), or that induction is characterized by a small but nearly constant phase lag. Either interpretation leads to the conclusion that the mechanism underlying the perception of the luminance + induced (experimental) condition has nearly identical temporal characteristics to that underlying the perception of the luminance + luminance (control) condition. Note that for systematic motion judgments to be possible at all, counterphase brightness variations must be induced in test fields even at temporal frequencies of 24 Hz, indicating that brightness induction is much faster than previously reported (DeValois et al., 1986, Rossi & Paradiso, 1996). In addition, the size of the induced area (test field height) does not affect the temporal properties of induction.
Discussion
Unlike previous studies suggesting that brightness induction is a sluggish process our results show that induction is nearly instantaneous. Why are the temporal properties of induction we measure so much faster than those described by previous investigators (DeValois et al., 1986; Rossi & Paradiso, 1996)? One idea is that GI, measured in the present study, and SBC, measured in previous studies, arise from fundamentally different brightness induction mechanisms with different temporal characteristics. Although possible, the work of Blakeslee and McCourt (1997) argues against this idea. They obtained detailed point-by-point brightness matching data in a series of stimulus displays that transitioned smoothly from GI to SBC. These data indicated that both the structure and magnitude of induction in both stimulus types could be parsimoniously accounted for by multiscale spatial filtering. It seems unlikely, therefore, that they should exhibit totally different temporal properties.
Another possible reason for the large discrepancy in the temporal properties of induction across studies is that the indirect quadrature motion technique and direct brightness matching reveal different brightness induction mechanisms with different temporal characteristics. This interpretation is especially intriguing in view of the differences in qualitative appearance of luminance- and surround-induced brightness modulations at high temporal frequencies reported in the DeValois et. al. (1986) study. While luminance-modulated test fields appeared to oscillate between black and white (bright and dark) at all temporal frequencies, DeValois et. al. (1986) found that the brightness of surround-induced test fields only appeared to change from black to white at low frequencies. At higher frequencies the center appeared a constant gray that flickered very strongly, particularly around the edges. It is important to note, however, that subjects in these experiments were specifically instructed to ignore the appearance (or nonappearance) of flicker when making their brightness judgments. Considering that any flicker in the center test patch of the surround-modulated display must be due to induction effects, their description of the SBC stimulus indicates that some form of induction was present up to higher frequencies than they reported. DeValois et. al., (1986) suggested that this somewhat strange dissociation between flicker and a change in brightness (or lightness) might result from having simultaneous access to the output from cells or channels involved in detecting flicker and the output from cells or channels involved in specifying the brightness (lightness) of a region.
Still another possibility is that induction is fast and that the temporal frequency cut-off reported in previous studies of SBC is artificially low. The argument for this interpretation is that it is exceedingly difficult to directly match induction magnitude and phase in temporally varying displays and this difficulty increases with increasing temporal frequency. DeValois et al. (1986), attempted to address this problem in their direct brightness matching experiments by comparing brightness matches to test fields that were luminance-modulated at 60% contrast with induced brightness changes produced by surround-modulation of 60% contrast. In this experiment, however, surround modulation of 60% contrast produced induced brightness modulations of at most 30% contrast. Therefore, a better comparison would be between a direct luminance-modulation and a surround-induced brightness modulation of the same apparent contrast (i.e., 30%). This discrepancy may, at least in part, be responsible for the differences in the matching behavior to direct and surround-modulated variations. Qualitative comparisons performed in our laboratory between a SBC stimulus matching that in the DeValois et al. (1986) study and a GI stimulus with similar parameters lend support to this idea. They indicate that flicker is visible in the test field of both displays up to at least 24 Hz (the highest frequency we examined) and that the appearance of the test fields for surround-modulated and luminance modulated patterns appear similar and match the descriptions reported by DeValois et al. (1986). When the contrast of the luminance-modulated test field for either stimulus is decreased to 30%, however, it no longer appears to modulate as clearly between bright and dark at high temporal frequencies and more closely resembles the appearance of the surround-modulated test field. These observations are consistent with the idea that a similar induction mechanism underlies both GI and SBC, and support the hypothesis that at least some of the differences in the appearance of surround- and luminance-modulated brightness at high temporal frequencies reported by DeValois et al. (1986) is due to a mismatch in the contrast of the stimuli being compared. Rossi and Paradiso (1996) did not perform a luminance control in their brightness matching experiments and therefore it is again possible that the low temporal frequency cut-offs they reported stem from the difficulty of the matching task with temporally varying displays.
Our finding that brightness induction, or one form of brightness induction, is nearly instantaneous requires a reevaluation of the physiological mechanisms proposed to account for induction and its temporal characteristics. In order to explain their brightness masking data, Paradiso and Nakayama (1991) proposed that filling-in signals travel at a rate of 6.7-9.2 ms/degree. In the GI stimulus it is test field height, rather than bar width (or spatial frequency), that determines the distance over which filling-in would need to propagate. We find that induction phase (time) lag is small and relatively constant across wide variations in test field height. These data, like the spatial pattern of brightness induced in the test field of the GI stimulus (Blakeslee & McCourt,1997), cannot easily be explained as the outcome of an edge-dependent, homogeneous filling-in process (Rossi & Paradiso, 1996). The data, however, are consistent with an explanation of brightness induction based on spatial filtering by cortical simple cells (Blakeslee & McCourt, 1997; Blakeslee & McCourt, 1999). Cortical filtering predicts that induction will be nearly instantaneous since the ON and OFF sub-regions of these cells arise through excitatory inputs from ON and OFF center LGN cells, respectively, and have similar latencies (Ferster & Lindstrom, 1983; Hirsch & Martinez, 2006). Blakeslee and McCourt (1997) parsimoniously accounted for SBC and GI with a linear multiscale difference-of Gaussians (DOG) model. Both effects, however, occur over distances too large to be explained on the basis of retinal or geniculate receptive fields making a cortical origin for these effects likely (Blakeslee & McCourt, 1997; DeValois et al., 1986; Yund & Armington, 1975). The DOG model has since been elaborated to include both orientation selectivity and contrast normalization (gain control), response characteristics routinely observed at early cortical stages of visual processing in both cat and monkey (Albrecht, Geisler, Frazor, & Crane, 2002; Carandini, Heeger & Movshon, 1997; Hirsch & Martinez, 2006). The ODOG model accounts for GI and SBC as well as for a number of additional brightness effects, including White’s effect, which cannot be explained by the linear DOG model (Blakeslee & McCourt, 1999; Blakeslee & McCourt, 2001; Blakeslee & McCourt, 2004; Blakeslee & McCourt, 2005).
Conclusions
Grating induction provides a unique opportunity to precisely measure the temporal properties of induction using a quadrature motion technique. Unlike previous studies suggesting that brightness induction is a sluggish process with temporal frequency cutoffs of 2-5 Hz (DeValois, Webster, Devalois, & Lingelbach, 1986; Rossi & Paradiso, 1996), we find using this technique that induction is nearly instantaneous. The temporal response of induced brightness differs from that of luminance gratings by a small time lag (< 1 ms), or by a small temporal phase lag (< 0.016 cycle), and remains relatively constant across wide variations in test field height. These data are not easily explained by an edge-dependent, homogeneous filling-in process of the type described by Rossi and Paradiso (1996), however, they are consistent with an explanation of brightness induction based on spatial filtering by cortical simple cells (Blakeslee & McCourt, 1999). The quadrature motion technique provides evidence that brightness induction, or a form of brightness induction, is nearly instantaneous. It remains to be determined, however, whether the sluggish process reported in previous studies represents a separate induction mechanism or stems from the inherent difficulty of using the direct brightness matching task with temporally varying stimuli.
Acknowledgments
This work was supported by grants R01 EY014015 and NIH P20 RR020151. The National Center for Research Resources (NCRR) and the National Eye Institute (NEI) are components of the National Institutes of Health (NIH). The contents of this report are solely the responsibility of the authors and do not necessarily reflect the official views of the NIH, NCRR, or NEI. The authors thank Huanzhong (Dan) Gu for programming the experiments and Nate Pikalek for help conducting the experiments.
Footnotes
Commercial relationships: none.
References
- Albrecht DG, Geisler WS, Frazor RA, Crane AM. Visual cortex neurons of monkeys and cats: Temporal dynamics of the contrast response function. Journal of Neurophysiology. 2002;88:888–913. doi: 10.1152/jn.2002.88.2.888. [DOI] [PubMed] [Google Scholar]
- Blakeslee B, McCourt ME. Similar mechanisms underlie simultaneous brightness contrast and grating induction. Vision Research. 1997;37:2849–2869. doi: 10.1016/s0042-6989(97)00086-2. [DOI] [PubMed] [Google Scholar]
- Blakeslee B, McCourt ME. A multiscale spatial filtering account of the White effect, simultaneous brightness contrast and grating induction. Vision Research. 1999;39:4361–4377. doi: 10.1016/s0042-6989(99)00119-4. [DOI] [PubMed] [Google Scholar]
- Blakeslee B, McCourt ME. A multiscale spatial filtering account of the Wertheimer-Benary effect and the corrugated Mondrian. Vision Research. 2001;41:2487–2502. doi: 10.1016/s0042-6989(01)00138-9. [DOI] [PubMed] [Google Scholar]
- Blakeslee B, McCourt ME. A unified theory of brightness contrast and assimilation incorporating oriented multiscale spatial filtering and contrast normalization. Vision Research. 2004;44:2483–2503. doi: 10.1016/j.visres.2004.05.015. [DOI] [PubMed] [Google Scholar]
- Blakeslee B, McCourt ME. Oriented multiscale spatial filtering and contrast normalization: a parsimonious model of brightness induction in a continuum of stimuli including White, Howe and simultaneous brightness contrast. Vision Research. 2005;45:607–615. doi: 10.1016/j.visres.2004.09.027. [DOI] [PubMed] [Google Scholar]
- Carandini M, Heeger DJ, Movshon JA. Linearity and normalization in simple cells of the macaque primary visual cortex. Journal of Neuroscience. 1997;17:8621–8644. doi: 10.1523/JNEUROSCI.17-21-08621.1997. [DOI] [PMC free article] [PubMed] [Google Scholar]
- DeValois RL, Webster MA, DeValois KK, Lingelbach B. Temporal properties of brightness and color induction. Vision Research. 1986;26:887–897. doi: 10.1016/0042-6989(86)90147-1. [DOI] [PubMed] [Google Scholar]
- Ferster D, Lindstrom S. An intracellular analysis of geniculo-cortical connectivity in area 17 of the cat. Journal of Physiology. 1983;342:181–215. doi: 10.1113/jphysiol.1983.sp014846. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Foley JM, McCourt ME. Visual grating induction. Journal of the Optical Society of America A. 1985;2:1220–1230. doi: 10.1364/josaa.2.001220. [DOI] [PubMed] [Google Scholar]
- Foster DH, Bischof WF. Thresholds from psychometric functions: superiority of bootstrap to incremental and probit variance estimators. Psychological Bulletin. 1991;109:52–159. [Google Scholar]
- Heinemann EG. Simultaneous brightness induction. In: Jameson D, Hurvich LM, editors. Handbook of Sensory Physiology, VII-4 Visual psychophysics. Springer; Berlin: 1972. pp. 147–169. [Google Scholar]
- Hirsch JA, Martinez LM. Circuits that build visual cortical receptive fields. Trends in Neuroscience. 2006;29:30–39. doi: 10.1016/j.tins.2005.11.001. [DOI] [PubMed] [Google Scholar]
- McCourt ME. A spatial frequency dependent grating-induction effect. Vision Research. 1982;22:119–134. doi: 10.1016/0042-6989(82)90173-0. [DOI] [PubMed] [Google Scholar]
- McCourt ME. Grating induction: A new explanation for stationary visual phantoms. Vision Research. 1994;34:609–1618. doi: 10.1016/0042-6989(94)90118-x. [DOI] [PubMed] [Google Scholar]
- McCourt ME, Blakeslee B. A contrast matching analysis of grating induction and suprathreshold contrast perception. Journal of the Optical Society of America, A. 1994;11:14–24. doi: 10.1364/josaa.11.000014. [DOI] [PubMed] [Google Scholar]
- Paradiso MA, Nakayama K. Brightness perception and filling-in. Vision Research. 1991;31:1221–1236. doi: 10.1016/0042-6989(91)90047-9. [DOI] [PubMed] [Google Scholar]
- Rossi AF, Paradiso MA. Temporal limits of brightness induction and mechanisms of brightness perception. Vision Research. 1996;36:1391–1398. doi: 10.1016/0042-6989(95)00206-5. [DOI] [PubMed] [Google Scholar]
- Stromeyer CF, III, Kronauer RE, Madsen JC, Klein SA. Opponent-movement mechanisms in human vision. Journal of the Optical Society of America, A. 1984;1:876–884. doi: 10.1364/josaa.1.000876. [DOI] [PubMed] [Google Scholar]
- Yund EW, Armington JC. Color and brightness contrast effects as a function of spatial variables. Vision Research. 1975;15:917–929. doi: 10.1016/0042-6989(75)90231-x. [DOI] [PubMed] [Google Scholar]