Lattice thermal conductivity of lower mantle minerals and heat flux from Earth’s core

Abstract

The amount of heat flowing from Earth’s core critically determines the thermo-chemical evolution of both the core and the lower mantle. Consisting primarily of a polycrystalline aggregate of silicate perovskite and ferropericlase, the thermal boundary layer at the very base of Earth’s lower mantle regulates the heat flow from the core, so that the thermal conductivity (k) of these mineral phases controls the amount of heat entering the lowermost mantle. Here we report measurements of the lattice thermal conductivity of pure, Al-, and Fe-bearing MgSiO₃ perovskite at 26 GPa up to 1,073 K, and of ferropericlase containing 0, 5, and 20% Fe, at 8 and 14 GPa up to 1,273 K. We find the incorporation of these elements in silicate perovskite and ferropericlase to result in a ∼50% decrease of lattice thermal conductivity relative to the end member compositions. A model of thermal conductivity constrained from our results indicates that a peridotitic mantle would have k = 9.1 ± 1.2 W/m K at the top of the thermal boundary layer and k = 8.4 ± 1.2 W/m K at its base. These values translate into a heat flux of 11.0 ± 1.4 terawatts (TW) from Earth’s core, a range of values consistent with a variety of geophysical estimates.

The 46 ± 3 terawatts (TW) of heat flowing from the solid Earth (1, 2) is one of the prime geophysical constraints on the dynamic state of the planet. As an integrated quantity, this global value does not distinguish individual contributions from the mantle and the core. Knowledge of the proportion of surface heat flow originating from the core is of enormous importance to our understanding of mantle dynamics (3–5), the generation of the magnetic field by flow in the outer core (6, 7), and the distribution of radiogenic isotopes between mantle and core (8). Current estimates place core-mantle boundary (CMB) heat flux at around 10–30% of the surface value (4), an uncertainty which significantly hampers constraints on the thermal evolution of the core. This large spread of values further implies notable uncertainty in the extent of heat generated in the lower mantle, because heat not originating from the core must necessarily come from the overlying mantle.

The principle factor controlling the heat flux from the core is the ability of the thermal boundary layer overlying the core-mantle boundary to conduct heat. Thermal conductivity of the lowermost mantle is therefore a key physical property to constrain if we are to narrow the bounds of uncertainty on core-mantle boundary heat flux. The polycrystalline nature of the lower mantle aggregate, combined with the presence of minor elements, most notably Fe, will likely suppress a strong radiative contribution to thermal conduction in the lowermost mantle (9, 10), making heat transport via lattice vibrations the dominant factor to consider.

Existing estimates of lattice thermal conductivity for the lowermost mantle range between k = 4–16 W/m K, based on extrapolation of measurements for pure end member phases (MgO, MgSiO₃, SiO₂) at upper mantle pressures (11–16). While minor elements have been shown to have a large effect on k in upper mantle minerals (17), their effect at high pressure in lower mantle minerals has not been rigorously considered. A nontrivial source of uncertainty in k of the lowermost mantle is the means of extrapolation to the conditions of the lowermost mantle (11, 18). Recent first-principles computations of lattice k in MgO periclase has shown that Debye theory (Eq. 2, see below) gives an excellent representation of the density dependence of k in a simple oxide (19–21), while a recent set of experimental measurements (22) indicates that the density dependence of k in more complex solids can be represented by removing the assumption that the phonon group velocities are proportional to the Debye temperature (13; Eq. 3, see below).

In this study we seek to constrain the lattice thermal conductivity of a realistic lower mantle mineral assemblage. We performed high pressure and temperature measurements of k for the following systems: Pure MgSiO₃ (Pv), MgSiO₃ + 3 mol% FeSiO₃ (Pv_Fe03), and MgSiO₃ + 2 mol% Al₂O₃(Pv_Al02) at 26 GPa up to 1,073 K; pure MgO (Pe), MgO + 5 mol% FeO(Pe_Fe05), and MgO + 20 mol% FeO(Pe_Fe20) at 8 and 14 GPa, up to 1,273 K. We then apply these results to reassess the value of k for the lowermost mantle and propose a refined value for core-mantle boundary heat flux.

Results

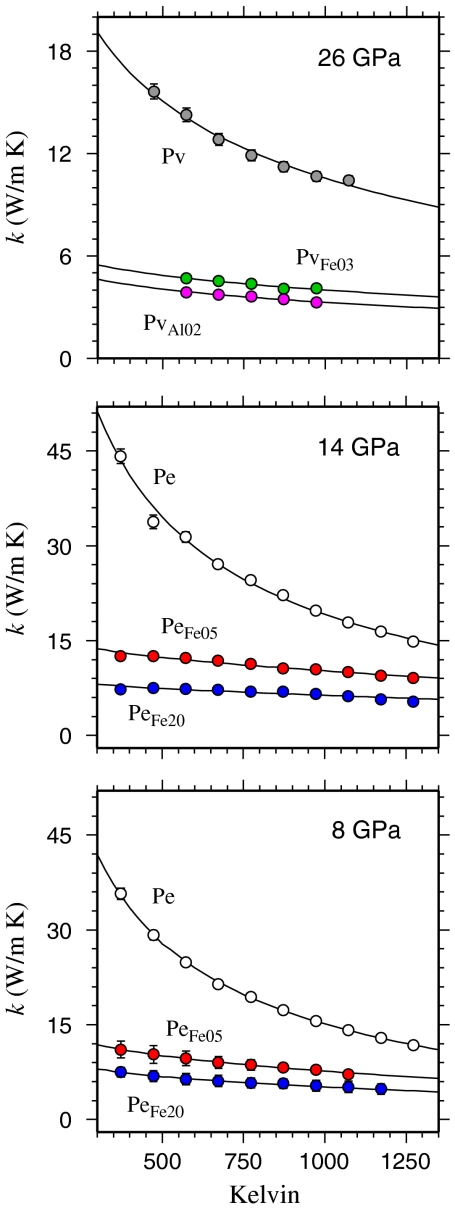
We find that addition of even a small amount of minor elements to the mineral composition strongly reduces the lattice conductivity (Fig. 1). This reduction is of similar magnitude for larger concentrations of Fe in ferropericlase, as well as for substitution of Al rather than Fe in perovskite. The measured k for MgSiO₃ perovskite is notably higher than the values Osako and Ito measured at ambient pressure and supercooled conditions (14), while our measurements for MgO periclase are somewhat lower than the previous experimental determination of Katsura (12).

Fig. 1.

Measured lattice thermal conductivities for the respective mineral compositions considered (see SI Appendix for more detail). We use the values determined from the amplitude ratio (Θ), in order to minimize contributions from direct radiative transfer. Solid lines indicate the fits of Eq. 1, using the parameters reported in Table 1.

To construct a set of models for the pressure-temperature dependence of lattice conductivity in lower mantle minerals, we combine our thermodynamic models (SI Appendix, Eqs. S6–S12) with the relation

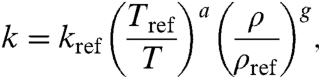[1]

in which a allows for deviation from the theoretically expected 1/T relation (23, 24), and g = (∂ ln k/∂ ln ρ)_T , with

[2]

for the oxide phases (Debye theory; 19, 20), and

[3]

for the perovskite phases (modified Debye theory; 13, 22). 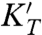is the pressure derivative of the isothermal bulk modulus, γ the Grüneisen parameter, and q = -(∂ ln γ/∂ ln ρ)_T. This approach further allows us to correct for the fact that our measurements are at constant pressure while theoretical descriptions of k(T) are strictly valid at constant volume. With ρ and g constrained from the respective thermodynamic models, we determine the optimal values of k_ref and a in Eq. 1 for each set of measurements (Table 1).

Table 1.

Parameters for k(P,T) in the respective compositions, modeled through Eq. 1

	Pe	PeFe05	PeFe20	Pv	PvFe03	PvAl02
ρref (cm3/mol)	3.71	3.84	4.21	4.45	4.49	4.45
kref (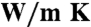)	21.0	8.94	6.02	12.8	4.46	3.69
a	0.76	0.24	0.24	0.43	0.20	0.22

In all cases T_ref = 700 K, with P_ref = 8 GPa for periclase and 26 GPa for perovskite.

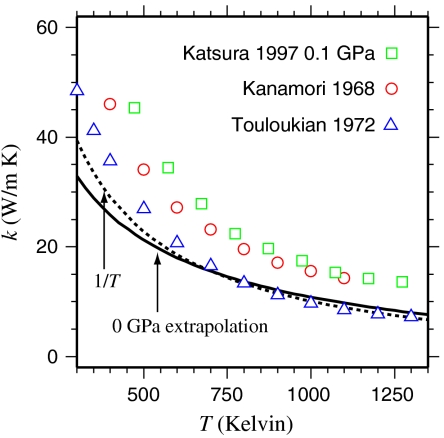
We find that the T-dependence for MgO is somewhat more gradual than 1/T, while k flattens out for the more complex structured MgSiO₃ perovskite as well as for samples containing Fe or Al. This behavior is consistent with larger values to the lower limits of the phonon mean free path lengths in the more complex structured and/or disordered crystal lattices, in which case it is theoretically expected that a = 1/2 (23). We also note that the density dependence of the k_ref values in all three ferropericlase compositions considered is consistent with the use of Eq. 2 for the oxide systems. Ambient pressure thermal conductivity for MgO determined from our model agrees well with experiment, although it fails to capture the very steep increase in k seen at room temperature (Fig. 2). The agreement is very encouraging for the larger extrapolation to high P and T at which we perform below.

Fig. 2.

Thermal conductivity of MgO periclase at ambient pressure, extrapolated from our high pressure measurements using Eq. 2 and our thermodynamic model. Values compare well at high temperatures with the measurements of Touloukian, et al. (25) for polycrystalline MgO, though it falls somewhat below those of Kanamori, et al. (26) and Katsura (12). The model underestimates the lowest T values, as does a simple 1/T relation.

Discussion

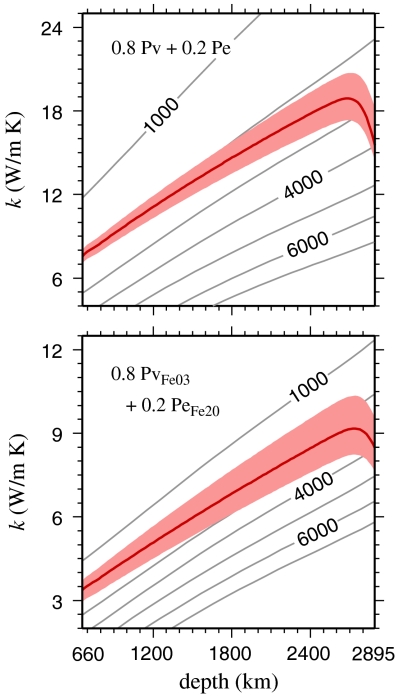
Representing the lower mantle as a randomly oriented polycrystalline aggregate of 20% ferropericlase and 80% perovskite, and assuming Fe to partition preferentially into ferropericlase (27), we construct a k(P,T) model for the lowermost mantle by combining our models for Pe_Fe20 and Pv_Fe03 given in Table 1. Fig. 3 shows the subsequent Hashin-Shtrikman averaged mantle k model thus obtained, computed along a model geotherm (29). Our subsequent prediction of the lattice thermal conductivity in the lowermost mantle is 9.1 ± 1.2 W/m K at the top of the thermal boundary layer, and 8.4 ± 1.2 W/m K at its base, notably lower than the corresponding values of 18.9 ± 1.6 and 15.4 ± 1.4 W/m K we predict for a pure MgO-MgSiO₃ aggregate.

Fig. 3.

Thermal conductivity models computed from our results using Hashin-Shtrikman averaging (28) for aggregates of 20% Pe + 80% Pv (top) and 20% Pe_Fe20 + 80% Pv_Fe03 (bottom). The mantle geotherm is constructed by combining a 1,600 K pyrolite adiabat (29) with a superadiabatic temperature increase in the bottom 200 km through the thermal boundary layer to reach the range of temperature estimates for the core-mantle boundary (30, 31). Temperatures in the thermal boundary layer thus increase from 2,600 ± 200 K at its top to 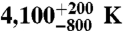at the CMB.

In the light of the relatively small differences seen between Fe- and Al-bearing perovskite, and between different Fe-concentrations in ferropericlase, it is unlikely that the small change in ionic radius resulting from a transition in the spin state of Fe will affect the lattice conductivity significantly. Indeed, at mantle temperatures the transition will be very gradual and to an intermediate rather than low spin state (32, 33). In contrast to metals, dielectric materials do not exhibit a simple direct relation between thermal conductivity and electrical resistivity (34), so that room temperature changes in the latter associated with the spin transition (35, 36) do not imply a corresponding change in k in the mantle. To account for possible variations in our models for k due to these factors, we increase the uncertainty on the k_ref value from 5% to 10%.

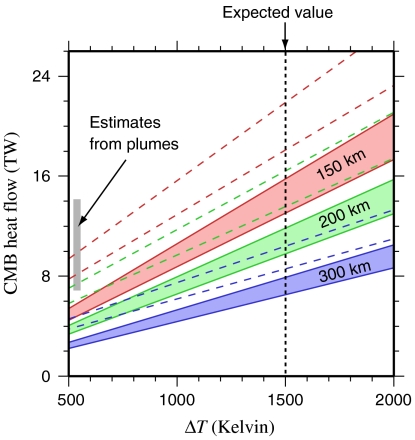
The estimated heat flux computed from our thermal conductivity model is 11.0 ± 1.4 TW (Fig. 4), which is within the range of values based on geophysical arguments (2, 4), and also consistent with the minimum bounds of heat flux (3-4 TW) required by geodynamic models to sustain the geomagnetic field (2). Due to the steep thermal gradient in the basal thermal boundary layer, postperovskite will occur as lenses surrounded by perovskite (29, 38). Using a simplified scaling based on measurements in analogue materials (37), we estimate that a layer of postperovskite would increase CMB heat flow by about 40%.

Fig. 4.

CMB heat flux as a function of the temperature contrast across the D’’, for different thermal boundary layer thicknesses, computed from our model values for the lower-most mantle by iteratively adjusting the thermal profile for steady state. Solid envelopes represent a 20% Pe_Fe20 + 80% Pv_Fe03 assemblage, accounting for uncertainty on the k values. Dashed lines indicate the positions of these envelopes if the silicate component of the thermal boundary layer was in the postperovskite (PPv) phase for T < 3,300 K, assuming k_PPv = 2 × k_Pv (37). Independent core-mantle boundary heat flux values based on mantle plume buoyancy flux estimates are shown for comparison (see ref. 2).

Our results indicate that lateral compositional heterogeneities of Fe and Al in major lower mantle mineral assemblages will not result in large variations of thermal conductivity on its own. However, to the extent that lateral variations in temperature and chemistry bring about changes in mineral abundances, either by changing the perovskite-ferropericlase ratio, or by substituting perovskite for postperovskite, lateral variations in heat flux from the core on the order of 50% can be expected.

Experimental Methods

Thermal conductivity measurements were performed on presynthesized polycrystalline samples using the Ångström method (e.g., ref. 39) in a 5,000 tonne multianvil apparatus at the Bayerisches Geoinstitut (refer to SI Appendix, section S2). To accurately represent the temperature dependence of lattice conductivity, we use thermal diffusivity values determined from the amplitude ratio of temperature signals between the two thermocouples (SI Appendix, Eq. S2) to minimize spurious effects due to direct radiative transfer between the thermocouples. In Fe-containing samples, Fe⁺³ concentrations are measured using Mössbauer spectroscopy (refer to SI Appendix, section S1). To represent the thermodynamic properties of the various phases as a function of pressure and temperature, we use a self-consistent Mie-Grüneisen formulation of the Helmholtz free energy (40, 41; refer to SI Appendix, section S3).