There is always a trade-off between speed and force in a lever system: comment on McHenry (2010)

In a recent Biology Letters article, McHenry [1] makes a distinction between levers that operate under ‘quasi-static’ and ‘dynamic’ conditions, concluding that ‘no trade-off between force and velocity exists in a lever with spring–mass dynamics’. As evidence, McHenry uses a computer model to simulate a kicking locust leg powered by a spring. While we concur with aspects of McHenry's analysis—and we agree that complex relationships between forces and speeds may emerge from some biological levers—we offer a different interpretation of McHenry's data.

1. What distinguishes levers under quasi-static and dynamic conditions?

McHenry compares the maximum tangential velocity of the kicking leg for levers with different geometry. Because all models generate the same maximum speed, but different forces (i.e. different accelerations of constant mass), McHenry infers that geometry of the lever ‘does not present a trade-off between force and velocity’. McHenry attributes this result to dynamic conditions, specifically to the inertia of the leg and the capacity of the spring to overcome this inertia.

We assert that the fundamental action of a rigid, frictionless and massless lever does not change under dynamic conditions. When any such lever performs work, the output force and velocity are related to the input force and velocity via mechanical advantage of the lever, A. This force–velocity trade-off follows from balancing moments on the lever:

1.1

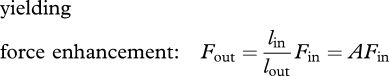1.2

1.3

F_in, F_out, v_in, v_out are the components of force and velocity perpendicular to the lever (figure 1). These equations are consistent with McHenry's model, and they hold under quasi-static and dynamic conditions, regardless of whether the forces vary with time. Importantly, power input equals power output at each instant:

1.4

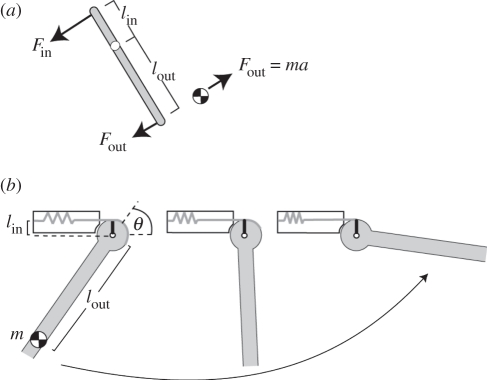
Figure 1.

Lever analogy for a locust leg with spring–mass dynamics. Equations (1.1)–(1.4) were derived from a free-body diagram of a lever (a, in contrast to McHenry's fig. 1a) acting to move mass m with acceleration a. We assumed F_in = F_spring and F_out = ma (b), simplifying McHenry's nonlinear geometry. The dynamics were simulated using parameters similar to those of McHenry: k = 0.3 N mm^–1, L_o (spring stretch at θ_o) =1.5 mm, l_in = 0.75 mm, l_out = 20 mm, m = 20 mg, θ_o = 6°, and 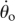= 0° s^–1. We varied l_out (10–40 mm) alone and in combination with the mass (8–80 mg) to assess the effects of geometry on force, velocity and power.

It appears McHenry made differing assumptions about the input forces and speeds when analysing lever mechanics under quasi-static and dynamic conditions. When comparing levers under quasi-static conditions, McHenry assumed that the input speeds were identical, but not the input forces. Under dynamic conditions, he assumed that the input (length-dependent) forces were identical, but not the input speeds. These assumptions and their implications for power transmission (not spring–mass dynamics) explain McHenry's result that levers with high A ‘generate more force and achieve high speed more quickly’ under dynamic conditions, when compared with quasi-static ones.

2. Does McHenry's model exhibit a force–velocity trade-off?

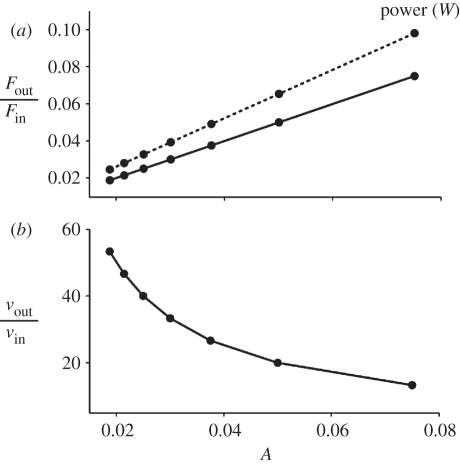
We conducted simulations of a lever with spring–mass dynamics (figure 1, similar to McHenry's model), and we compared the time-varying forces, velocities and powers associated with the input (spring) and output (mass) for levers with different geometry. We used equation (1.1) assuming F_out = ma (incorporating all of the leg's mass into F_out, as McHenry did) and F_in = F_spring (simplifying McHenry's geometry such that the spring force acted perpendicular to the lever throughout time). These simulations confirm that equations (1.2) and (1.3) hold: while F_out/F_in increases with A, v_out/v_in decreases with A (figure 2). Therefore, a force–velocity trade-off indeed exists.

Figure 2.

Force–velocity trade-off for a lever with spring–mass dynamics. As predicted from equations (1.2) and (1.3), the ratio of maximum forces [F_out/F_in]_max = A (a) while the ratio of maximum speeds [v_out/v_in]_max = 1/A (b). Note that peak power of the spring increases with A (a, dashed line) for any given output mass.

3. Does output force or velocity alone characterize lever mechanics?

Levers exhibit a force–velocity trade-off because they transmit power (equation (1.4)). In McHenry's analysis, the spring's input power is not the same in each simulation. This is because the rate of energy release from the spring depends on the inertial resistance of the mass (which depends on l_out). By accelerating the same mass with the same length-dependent (but not rate-dependent) force, McHenry guarantees that his higher A lever transmits greater power (figure 2 and electronic supplementary material, figure S1). Though arguably interesting, this approach makes it difficult to infer general principles about the effects of lever geometry—especially if only the output force and velocity are considered. To accurately characterize the mechanics of a lever, ratios of both the input and output forces and velocities must be analysed (equations (1.2)–(1.4)). Although maximum velocity may be relevant for McHenry's example, the function of many musculoskeletal levers is to transmit power (e.g. [2,3]).

A force–velocity trade-off is most easily discerned when the levers being compared have identical input force and velocity, and therefore identical power. Under identical power, levers with different A clearly exhibit a trade-off between output force and speed (electronic supplementary material, figure S2).

4. What determines the mass's maximum velocity?

McHenry concludes that no force–velocity trade-off exists for his model, in part, because the maximum speed of the kicking leg does not depend on geometry. We concur that the mass's maximum speed is independent of A—but this fact should not be associated with lever mechanics. As McHenry noted, the mass's maximum speed is determined by the principle of energy conservation, and thus depends only on the mass being accelerated and the elastic energy available (assuming no energy loss and negligible lever inertia). If the same energy accelerated the mass along a horizontal, frictionless table, the mass would reach the same speed, with no lever involved.

5. Do all levers show the same force–velocity trade-off?

We affirm McHenry's overarching message that lever systems with more complex dynamics—such as those with velocity-dependent input forces (e.g. actuated by muscles), or those with non-negligible inertia, energy dissipation, or compliance—may exhibit more complex relationships between forces and speeds than predicted by equations (1.1)–(1.4). Therefore, as McHenry notes, caution is warranted when modelling and analysing biological levers. For example, representing a limb as a point mass accelerated by a ‘massless, frictionless lever’ may be insufficient for some applications. When a lever has inertia and transmits power at a non-steady rate, equations (1.1) and (1.2) must be modified:

1.5

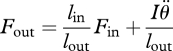1.6

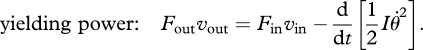1.7

In this case, output power equals input power minus the change in rotational kinetic energy of the lever. Output velocity is still related to input velocity, and output force to input force, but in a more complicated manner, since some of the input energy is converted to kinetic energy of the lever itself. These equations may be applicable when modelling a limb that transmits forces to its environment, or when it physically does not make sense to lump all of a limb's mass into the output force. Equations (1.5)–(1.7) are relevant to biologists, since many musculoskeletal structures have inertia and undergo substantial accelerations, acting as levers to transmit power.

In closing, we thank Matt McHenry for stimulating this discussion. We hope this commentary further clarifies the principles that govern rigid levers with spring–mass dynamics.