Age dynamics in scientific creativity

Abstract

Data on Nobel Laureates show that the age–creativity relationship varies substantially more over time than across fields. The age dynamics within fields closely mirror field-specific shifts in (i) training patterns and (ii) the prevalence of theoretical contributions. These dynamics are especially pronounced in physics and coincide with the emergence of quantum mechanics. Taken together, these findings show fundamental shifts in the life cycle of research productivity, inform theories of the age–creativity relationship, and provide observable predictors for the age at which great achievements are made.

At what age do scientists tend to produce great ideas? Focusing on great scientific achievements of the 20th century, this article shows that the age–creativity relationship demonstrates much greater variation over time than across fields. Moreover, field-specific dynamics in the age–creativity relationship are closely associated with variation in other field-specific characteristics, including the prevalence of theoretical contributions, educational duration, and citation patterns. These dynamics were especially pronounced in physics during the 1920s and 1930s, when quantum mechanics was developing. Thus, although the iconic image of the young, great mind making critical breakthroughs was a good description of physics at that time, it turns out to be a poor descriptor of age–creativity patterns more generally or even of physics today, where the mean age of Nobel Prize winning achievements since 1980 is 48 y.

This article makes two contributions to research on the age–creativity relationship. First, existing work—dating from the 19th century and spanning multiple disciplines—has emphasized differences in when creativity peaks across various fields, assuming that each field shows a fixed age–creativity pattern (1–8). In contrast, this article shows that such cross-field age differences are small compared with cross-time differences within fields. Moreover, the field-specific dynamics are large enough that the traditional rank ordering of fields by the age at which breakthrough contributions are made is unstable. Second, this article shows that these age dynamics are closely associated with several observable metrics. This analysis draws together two strands of work on the age–creativity relationship, which have only been studied separately in prior work, including our own: (i) how the training requirements related to acquiring foundational knowledge may explain the age at which scientific careers begin (9, 10), and (ii) the distinction between conceptual and experimental work in explaining creative peaks across the life cycle (11, 12). Although we do not identify causal mechanisms, we show that measures drawn from this prior work, in addition to a measure of foundational knowledge based on backward citation ages, all move in a striking and intuitive way with shifts in the tendency for scientific contributions by the young. These collective dynamics are especially pronounced in physics during the early 20th century.

Our primary analysis focuses on the complete set of Nobel Prizes given between 1901 and 2008 in physics, chemistry, and medicine. Through extensive historical and biographical analysis, we determined the years (and hence ages) at which each Nobel Prize winner produced their Nobel Prize-winning work, providing a dataset of 525 Nobel Prize winners, with 182 in physics, 153 in chemistry, and 190 in medicine. Alternative data sources are also considered below. (Data-collection methods, raw data, and summary statistics are described in detail in SI Appendix.)

Results

The image of the young, great mind making critical breakthroughs is iconic in the hard sciences. Moreover, traditional analyses of Nobel Prize winners show that, on average, physicists have made important contributions at earlier ages than chemists or medical scientists (5, 7, 8). Our first results reconsider this evidence, studying differences in the age of peak creativity between fields and changes over time within fields.

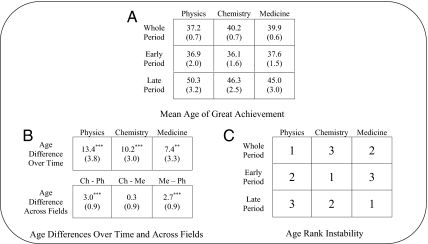
Fig. 1A presents the mean age at which Nobel Laureates did their prize-winning work, showing field averages for the whole sample period and separately for an early period (prize-winning work before 1905) and late period (prize-winning work after 1985). Two key patterns emerge. First, shifts in the mean age over time are large. As summarized in Fig. 1B, the mean age of prize-winning work increased by 7.4 y (P < 0.05) in medicine, 10.2 y (P < 0.01) in chemistry, and 13.4 y (P < 0.01) in physics. These magnitudes are much larger than the cross-field differences, the largest of which in the whole sample is 3.0 y (P < 0.01) between chemistry and physics. Second, the traditional cross-field comparisons are highly unstable. As summarized in Fig. 1C, the rank ordering of fields from youngest to oldest interchanges. Physics, for example, has the oldest mean age at great achievement in the late period, even though it is the youngest field over the period as a whole. A variance decomposition further demonstrates the relative importance of shifts over time. Not surprisingly given the wide range of ages at which individuals make important contributions, most of the variation cannot be explained by field or year effects. Nevertheless, static, cross-field differences only account for 2.48% of the overall variance in ages but within field dynamics account for 12.33% or five times the variance explained by the cross-field differences focused on in the literature. (The share of the variance explained by cross-field differences is the R² of a regression of age at great achievement on field dummy variables. The share of the variance explained by within-field dynamics is the R² of a regression of age at great achievement on field-specific fractional polynomial regressions.)

Fig. 1.

Age of great achievement over time and by field. (A) The mean age at which Nobel Laureates produced their prize-winning work in physics, chemistry, and medicine across all years (Whole) and in the early period (through 1905) and late period (from 1985). (B Upper) The change in mean age of great achievement between the early and late periods within each field. (B Lower) For each pair of fields, the difference between fields in the mean age over all years. (C) The rank ordering of the fields by mean age for the whole period, early period, and late period (1 indicates lowest mean age, and 3 indicates highest mean age). SEs are given in parentheses. **significance at 5%; ***significance at 1%.

Figs. 2 (physics), 3 (chemistry), and 4 (medicine) detail the field-specific age-dynamics and examine factors relating to these shifts. Figs. 2A, 3A, and 4A show the percentage of great achievements produced by ages 30 and 40. The predicted values and indicated 95% confidence intervals are given by logistic fractional polynomial regressions (13). (Here and below, we estimate second-degree logistic fractional polynomial regressions for physics and medicine and a third-degree logistic fractional polynomial model for chemistry, which shows more complicated dynamics.) Letting Achievement Age_i and Year_i denote the age and year in which laureate i made his or her great achievement, the (second-degree) regressions are from models of the form (Eq. 1)

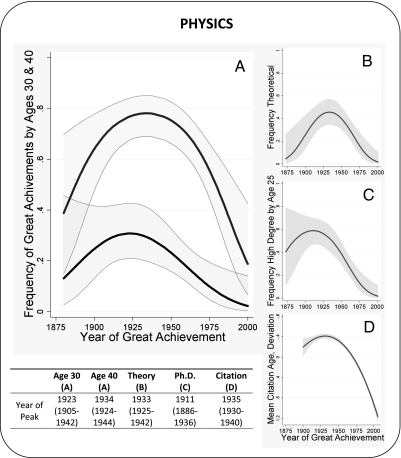
Fig. 2.

Dynamics in physics. The figure presents dynamics in age and associated factors for Nobel Prize-winning achievements in Physics. (A) The evolution over time in the frequency of prize-winning contributions by ages 30 (Lower) and 40 (Upper). Shaded regions indicate 95% confidence intervals. (B–D) Related dynamics: the frequency of prize-winning achievements with an important theoretical component (B), the frequency of Nobel Laureates who received their PhD by age 25 (C), and the backward citation age for the top papers in physics (D, inverted y axis), as defined in the text. The table summarizes the year when each measure peaks, with 95% confidence intervals, further indicating similar dynamics across the measures.

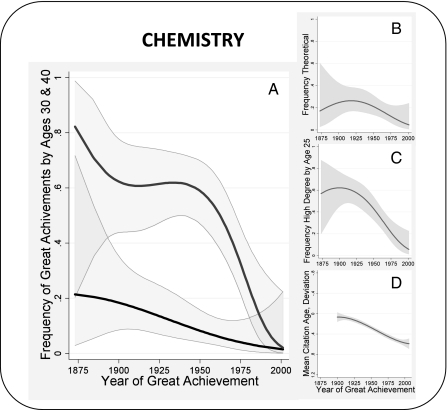
Fig. 3.

Dynamics in chemistry. Age and associated factors for Nobel Prize winning achievements in Chemistry. (A) The evolution over time in the frequency of prize-winning contributions by ages 30 (Lower) and 40 (Upper). (B\x{2013}D) Related dynamics: the frequency of prize-winning achievements with an important theoretical component (B), the frequency of Nobel Laureates who received their PhD by age 25 (C), and the backward citation age for the top papers in Chemistry (D, inverted y axis), as defined in the text. Shaded regions indicate 95% confidence intervals.

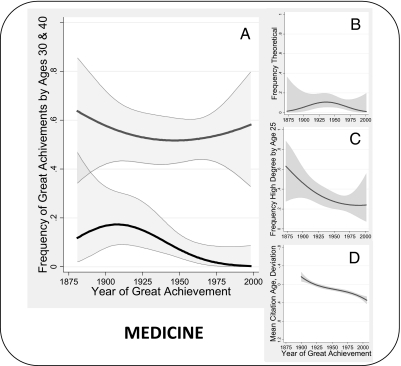
Fig. 4.

Dynamics in medicine. Age and associated factors for Nobel Prize winning achievements in Medicine. (A) The evolution over time in the frequency of prize-winning contributions by ages 30 (Lower) and 40 (Upper). (B\x{2013}D) Related dynamics: the frequency of prize-winning achievements with an important theoretical component (B), the frequency of Nobel Laureates who received their PhD by age 25 (C), and the backward citation age for the top papers in Medicine (D, inverted y axis), as defined in the text. Shaded regions indicate 95% confidence intervals.

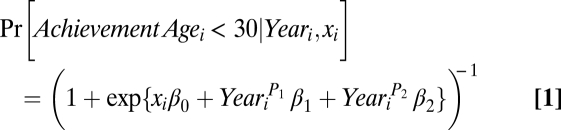where P_j ∈ {−2, −1, −1/2, 0, 1, 2, 3} for j = 1,2. The estimation procedure searches over values of P_j to obtain the best fit to the data. This approach smoothes the data in the way that a polynomial regression does but, by searching over functional forms, it provides a more flexible relationship between Year_i and the dependent variable than a polynomial regression.

In the early years, great achievement at young ages is common in all three fields. Before 1905, 69% of chemists, 63% of medical scientists, and 60% of physicists did their prize-winning work before age 40, and prize-winning work done before age 30 accounted for ∼20% of cases. The ensuing 100 y exhibit large dynamics, with two key features. First, in all three fields, great achievement before age 30 becomes increasingly rare, converging toward 0% of cases by the end of the century. This shift away from the very young also extends to higher age thresholds in physics and chemistry. In physics, great achievement by age 40 occurs in only 19% of cases by the year 2000, less than one-third its rate in 1900. In chemistry, great achievement by age 40 converges toward 0% by 2000, but it accounted for 66% of cases in 1900. SI Appendix, Fig. S1 A and B presents the underlying data and additional nonparametric estimates that show similar patterns. SI Appendix, Figs. S2 and S3 and Table S2, together with associated analysis in SI Appendix drawing on (10), show that underlying demographic shifts do not explain the dynamics in early life-cycle innovation.

Beyond the general aging pattern over the 20th century as a whole, a notable and exceptional dynamic appears in the initial increase in the frequency of young achievement in physics. The share of physics Nobel Laureates who did their prize-winning work at young ages rises sharply, peaking at 31% in 1923 (with a 95% confidence interval of 1905–1942) for work done by age 30 and peaking at 78% in 1934 (with a 95% confidence interval of 1924–1944) for work done by age 40, before declining over the remainder of the century. The shift in physics stands out from both chemistry, where young achievement declines more consistently, and medicine, which shows a decline in achievement before age 30 but otherwise no substantial trends. The lower average age for physics over the whole period (Fig. 1) thus arises from the temporarily increased incidence of contributions by young physicists during the first third of the 20th century but does not represent a stable feature of physics. SI Appendix, Fig. S4 shows similar age shifts in physics when using sources other than the Nobel Prize, and SI Appendix, Fig. S5 shows that the physics pattern is robust to controlling for region of birth.

The substantial shift toward youthful achievements in early 20th century physics occurs in a similar period as the development of quantum mechanics. Historians of physics often identify 1900 to 1927 as the key period in this development, starting with Planck's introduction of quanta in 1900 and continuing through the formulation of consistent theoretical foundations in 1925 to 1927 (14–16). Fig. 2A shows that the probabilities of great achievement by ages 30 and 40 peak during this period.

Werner Heisenberg, who developed his matrix mechanics in 1925 at age 23 and his uncertainty principle 2 y later, may provide a useful window into early career contributions during this period. Strikingly, Heisenberg did not seem particularly young for an important physicist at the time; Pauli and Dirac made contemporary, prize-winning contributions at ages 25 and 26. In the previous 10 y, the majority of Nobel Prizes in physics had been given to individuals for work done by their early 30s, and Dirac and Einstein suggested that, by age 30, a physicist was effectively dead (17, 18). However, as Figs. 2A, 3A, and 4A show, one cannot make similar claims about chemistry or medicine at that time or about physics today.

Heisenberg's example points toward two features of this period that may illuminate the age dynamics: the prevalence of abstract/deductive work and the obsolescence of existing knowledge. One line of age-creativity research has emphasized that abstract/deductive contributions tend to come at earlier ages than inductive contributions, which draw more heavily on accumulated knowledge (11, 12). Kuhn (14) points to the role of theoretical contributions like Heisenberg's in this episode. Thus, there may have been a shift to theoretical work, which tends to be abstract and deductive, in physics at this time. A second line of age–creativity research has emphasized that the expansion of foundational knowledge in a field may increase training requirements, making contributions at younger ages more difficult (9, 10). From this perspective, age dynamics might be associated with changes in a field's foundational knowledge, which may typically expand with time but may also contract in a case where new knowledge devalues old knowledge. Heisenberg, for example, nearly failed his PhD examinations at age 21, because he knew little of classic electromagnetism (19); his contributions in the subsequent 4 y suggest that training in classic physics may have become less salient.

To examine the importance of theoretical work, we classified all Nobel Prize-winning achievements according to whether the work had an important theoretical component (see SI Appendix for methods and data). To examine the relationship between the age dynamics and shifts in training, we identified the age at which each Nobel laureate received his or her highest degree (a doctorate in 98% of cases).

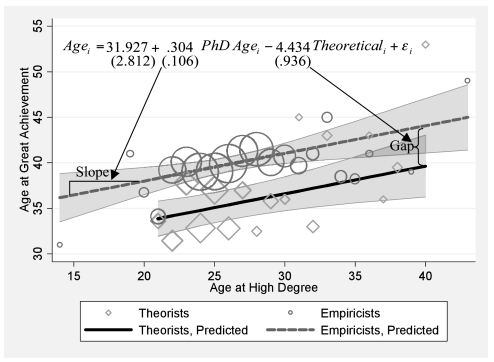
Fig. 5 shows how the mean age at Nobel Laureates’ great achievements is jointly related to the theoretical vs. empirical content of their contribution and their age at high degree. Fig. 5 summarizes these relationships using an ordinary least-squares regression with a linear term in age at high degree and a categorical variable for a theoretical great achievement. Theorists make their great achievements 4.434 (SE = 0.936) years earlier than empiricists on average, and a 1-y increase in the Nobel Laureate's age at highest degree is associated with a 0.304-y (SE = 0.106) increase in the average age of the Laureate's great achievement. SI Appendix, Table S3 presents regression estimates for a range of specifications (including the one in Fig. 5, reported in SI Appendix, Table S3B), showing that (i) training duration and (ii) theoretical research are independent, robust, and powerful predictors for the age at which great scientific achievements are made.

Fig. 5.

Predictors of age at great achievement. The figure shows how the age at which a Nobel Laureate produces prize-winning work is related to the Laureate's age at highest degree and whether the great achievement had a theoretical component. Each square (circle) represents the average achievement age for the Nobel Laureates who received their high degree at a given age and the Nobel Prize for theoretical (empirical) work. They are scaled in proportion to the number of Nobel Laureates in that cell. SI Appendix, Table S3 reports regressions of achievement age on the nature of work (theoretical vs. empirical) and age at high degree for a range of specifications. The regression and 95% confidence intervals are based on the specification shown in column 3 of SI Appendix, Table S3B.

Given that the nature of a Nobel Laureate's work and the length of the Laureate's training are strong independent predictors for the age at prize-winning contributions, we turn to how they comove over time with the age at prize-winning contributions. Fractional polynomial estimates of the dynamics for theoretical contributions are presented in Figs. 2B, 3B, and 4B. SI Appendix, Fig. S1C presents the underlying data and additional nonparametric estimates that show similar patterns. In physics, the prevalence of theoretical contributions is hump-shaped over the 20th century (Fig. 2B), showing a striking association with the age dynamics (Fig. 2A). The probability a contribution is theoretical peaks at 46% in 1933 (with a 95% confidence interval of 1925–1942). (A portion of the shift to younger ages in the early part of the 20th century is reflected in a shift to more theoretical work. At the same time, SI Appendix, Fig. S6 shows that the shift to the young in early 20th century physics and the ensuing aging phenomenon persist when looking within theorists and within empiricists.) The dynamics in theoretical contributions in chemistry (Fig. 3B) and medicine (Fig. 4B) also resemble their respective age dynamics, although the temporal shifts in these fields are less precisely estimated.

Figs. 2C, 3C, and 4C present fractional polynomial estimates of the shares of Nobelists who received their highest degree by age 25. The training patterns also mirror the achievement–age patterns closely. Although the majority of Nobel Laureates received their degrees by age 25 in the early 20th century, all three fields show substantial declines in this tendency, with physics and chemistry converging toward 0% of cases by the end of the century. Furthermore, in physics, the tendency to receive a PhD at young ages follows the same inverted U-shape. The dynamics in chemistry and medicine, though less precisely estimated, match closely with the dynamics in the propensity for great achievement by age 40. SI Appendix, Fig. S1C presents the underlying data and additional nonparametric estimates that show similar patterns.

To further examine shifts in foundational knowledge, we have developed a measure based on backward citations to prior work. Specifically, we take the top 100 most-cited papers published annually over the 20th century in each of the three Nobel fields and in an “other” category (comprising all other science and engineering fields). The data are drawn from the Thomson Reuters–Institute for Scientific Information's Century of Science (covering 1900–1955) and its Web of Science data (covering 1955 to the present). We measure the mean age of each of these highly cited papers’ backward citations. To eliminate background trends in citation age dynamics, we study the difference of the mean age of backward citations in each of the three Nobel fields from the mean age in the other category and normalize this difference by the SD in the other category. Note that the Institute for Scientific Information data are independent of the Nobel Prize data and allow more precise estimation of the dynamics because of greater sample size.

We use this citation age measure (i.e., the temporal distance to prior work) to examine knowledge dynamics, where a tendency to cite older papers suggests that top research draws on longer-established knowledge and a tendency to cite recent papers suggests that top research primarily draws on recent work. (See SI Appendix for citation methods and data details.) Figs. 2D, 3D, and 4D present fractional polynomial estimates of the evolution in backward citation age for physics, chemistry, and medicine. SI Appendix, Fig. S1D presents the underlying data and additional nonparametric estimates that show similar patterns. Again, the citation age dynamics match closely the achievement age dynamics. In physics, the tendency toward recent citations peaks in 1935 (with a 95% confidence interval of 1930–1940), which is close to the peak in youthful achievement. (SI Appendix, Table S4 shows that the temporary reduction in citation age in physics was not driven by new scholars entering the field. Rather, the humped-shaped phenomenon in Fig. 2D persists when looking at changes over time within individuals' careers, indicating that existing scholars also started citing more recent work.) Citation-age dynamics in chemistry and medicine also reflect age-creativity patterns in those fields. Overall, the dynamics in achievement age appear similar to the citation age dynamics.

Summary and Conclusions

This article demonstrates that the frequency of great achievement at young ages is more a function of time than field. The analysis further shows strong, independent associations between age dynamics within fields and both the prevalence of theoretical work and measures of the stock of foundational knowledge. Further work is needed to assess causal mechanisms underlying these empirical relationships and consider alternative forces, possibly emanating from the norms and institutions of science or the scale of the scientific enterprise (20, 21). Notably, the dynamics in age at great achievement, prevalence of theory, PhD age, and mean citation age are especially pronounced in physics and are coincident with the development quantum mechanics, which Kuhn placed at the center of his analysis of scientific revolutions (12, 22). The findings thus may provide candidate, quantitative markers to help identify such revolutionary events, providing an intriguing direction for future research.