Abstract
Fluid secretion relies on a close interplay between Ca2+-activated Cl and K channels. Salivary acinar cells contain both large conductance, BK, and intermediate conductance, IK1, K channels. Physiological fluid secretion occurs with only modest (<500 nM) increases in intracellular Ca2+ levels but BK channels in many cell types and in heterologous expression systems require very high concentrations for significant activation. We report here our efforts to understand this apparent contradiction. We determined the Ca2+ dependence of IK1 and BK channels in mouse parotid acinar cells. IK1 channels activated with an apparent Ca2+ affinity of about 350 nM and a hill coefficient near 3. Native parotid BK channels activated at similar Ca2+ levels unlike the BK channels in other cell types. Since the parotid BK channel is encoded by an uncommon splice variant, we examined this clone in a heterologous expression system. In contrast to the native parotid channel, activation of this expressed “parslo” channel required very high levels of Ca2+. In order to understand the functional basis for the special properties of the native channels, we analyzed the parotid BK channel in the context of the horrigan-Aldrich model of BK channel gating. We found that the shifted activation of parotid BK channels resulted from a hyperpolarizing shift of the voltage dependence of voltage sensor activation and channel opening and included a large change in the coupling of these two processes.
Key words: ion channels, Ca2+-activated K channels, maxi-K channels, IK1 channels
Introduction
There are three general classes of Ca2+-activated K channels. The large conductance, BK channel (KCa1.1, Slo) is the prominent member of the first group and is activated by depolarized membrane potentials as well as by Ca2+ ions. These channels are ubiquitously expressed throughout the body from secretory cells to the brain. The small conductance, SK, channels (KCa2.1, KCa2.2 and KCa2.3) are activated by Ca2+ but not by membrane potential and are widely expressed throughout the central nervous system. The third class has only a single member: the intermediate conductance IK1 (KCa2.3) channel. These channels are also activated by Ca2+ and not by voltage and are less wide spread than the other channels. They are quite prominent in red blood cells and T-lymphocytes and in organs involved in salt and fluid secretion including the colon and salivary glands.
IK1 and maxi-K channels are the only K channels expressed in mouse parotid acinar cells.1–3 There is extremely little variation in the IK1 protein sequence in different cell types and across species. The mouse parotid IK1 protein is essentially identical to the human variant. In contrast there are several BK channel variants expressed in different cell types and across species. The mouse parotid BK protein is highly homologous with the human form and differs at five, generally conserved, single sites and in the last eight conceptually translated amino acids.1 There are much larger differences between the mouse parotid BK isoform and the “standard” mouse version (mSlo)4 that is the common subject of electrophysiogical studies using heterologous gene expression. ParSlo does not have the IYF motif at splice site 3 that is present in mSlo. There are differences at splice site 6 including a retained exon in parSlo that contains a premature stop codon.
While BK channel activation is both Ca2+- and voltage-dependent, these channels can be activated in low (even nominally zero) Ca2+ but this activation requires extremely depolarized potentials—well beyond the physiologically accessible range.4,5 Cell Ca2+ levels increase in response to several stimulatory factors but the average, global Ca2+ levels are usually below a few µM, too little to cause significant BK channel activation except at very depolarized potentials. This problem is overcome in many cell types, particularly neurons and muscle cells, by positioning BK channels very near sources of Ca2+ that can produce very high Ca2+ levels in local microdomains.6–10
As noted above, IK and BK channels are expressed in salivary glands including parotid acinar cells. The physiological role of these cells in stimulating fluid secretion involves a muscarinic receptor-mediated increase in intracellular Ca2+ and the subsequent activation of the IK1 and BK channels in concert with Ca2+-activated Cl channels. The increase in Ca2+ in these cells does not exhibit the “hot spots” associated with the microdomains more typical in excitable cells and the maximal global Ca2+ is usually less than 1 µM.11–14 If the BK channels in the parotid cells have the same Ca2+ and voltage sensitivity as their counterparts in other cell types, they would not be activated by these low levels of Ca2+ and yet muscarinic stimulation that results in only modest levels of intracellular Ca2+ clearly activates BK channels.15 Very early studies suggested that a large conductance K channel in salivary glands is activated at low Ca2+ concentrations16 so the BK channels in secretory cells may have properties that differ from those in excitable tissues. We report here our efforts to understand the apparent difference between BK channels in salivary glands and the similar channels in neurons and muscles.
In this study we investigated the Ca2+ dependence of IK1 and BK channel activation in mouse and human parotid acinar cells. Our focus was on intact, whole cell measurements with physiologically relevant solutions. The Ca2+ dependence of parotid acinar IK1 channels was quite similar to the same channels in heterologous expression systems. In contrast we found a considerable difference in the Ca2+ dependence of native parotid BK and previously published studies with heterologously expressed mSlo or hSlo channels. Since, as noted above, the mouse parotid BK channel differs from mSlo and hSlo and the previously published studies utilized different experimental techniques and solutions, we also examined the Ca2+ dependence of heterologously expressed parSlo using the same techniques as for the native cells. Finally, in an effort to understand the functional differences between the expressed channels and those in native parotid cells, we analyzed our results in the context of the Horrigan-Aldrich (HA) model,17 which has been shown to provide insight into the mechanisms underlying the voltage- and Ca2+-sensitive properties of BK channels.
Results
As described in Introduction, salivary gland acinar cells express two types of Ca2+-activated K channels: the voltage- and time-independent IK1 channel and a particular, parotid-specific splice variant of BK channels. We have determined the Ca2+ dependence of the activation of these channels in their native context and with physiologically-relevant aqueous solutions.
Ca2+ activation of IK1 channels.
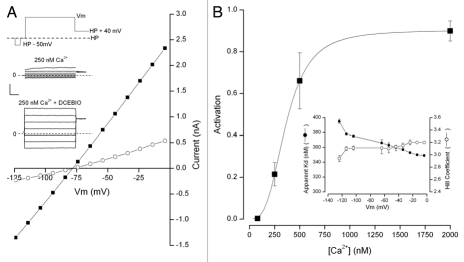
The results of our investigation of parotid IK1 channels are illustrated in Figure 1. The inset of part A shows the voltage clamp protocol used. Raw currents elicited by this protocol from a parotid acinar cell with 250 nM intracellular Ca2+ are also illustrated in the inset and the currents from the same cell with 20 µM of the IK1 activator DCEBIO added. The current-voltage relations from these data are shown in the main part of Figure 1A and these are zero near the K+ equilibrium potential as expected. Since this concentration of DCEBIO under these conditions produces maximal activation, the ratio between the currents in the presence and absence of DCEBIO allows a reasonable estimate of the fractional IK1 channel activation. Using this procedure we determined the amount of IK1 channel activation at negative voltages emphasizing the physiologically relevant range. The results of this analysis are illustrated in part B of Figure 1.
Figure 1.
Ca2+ sensitivity of IK1 channel activation. (A) inset: voltage protocol used (top) and currents recorded with 250 nM Ca2+ in the absence (middle) and presence of 20 µM DCEBIO. Main: IK1 channel current-voltage relation in the absence (◯) and presence (■) of 20 µM DCEBIO. (B) Main: mean IK1 channel activation determined at −54 mV as a function of internal Ca2+. The line is a fit of the standard Hill equation with a Kd value of 360 nM and a Hill coefficient, N, of 3.1. Inset: Kd (●) and N (◯) values obtained at the indicated Ca2+ concentrations. Data from 6–8 cells at each Ca2+ level.
The main part of Figure 1B illustrates the Ca2+ dependence of IK1 channel activation obtained at −54 mV. These data are fit (see Materials and Methods) by the Hill equation (line) which provides a reasonable description of these data. The apparent Kd and N values obtained from this fit are 360 nM and 3.1, respectively. The Kd values exhibited a small voltage sensitivity (note expanded scale in the inset) ranging from about 340 nM near 0 mV and almost 400 nM at very negative potentials. In contrast the Hill coefficient had little or no voltage dependence (also an expanded scale) with values between about 3.0 and 3.2. The behavior of these parotid IK1 channels are quite similar to heterologously expressed IK1 channels (see Discussion).
Ca2+ and BK channel activation.
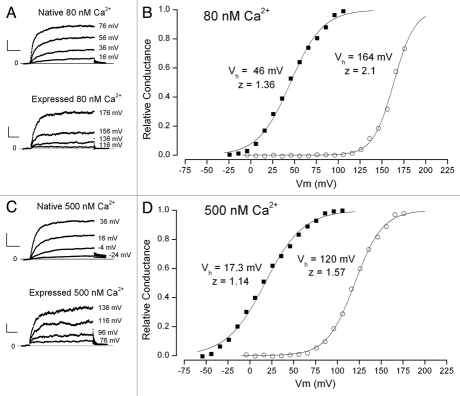
The Ca2+ dependence of BK channel activation is more complex than that of IK1 channels and examples of this are illustrated in Figure 2. Part A of this figure shows raw BK channel currents recorded at the indicated potentials with the same protocol illustrated in the inset of Figure 1A. Shown are currents from a native parotid acinar cell (upper) and the parotid BK channel variant, parSlo, expressed in CHO cells— note the different range of potentials shown. Both cells in these examples were patched with 80 nM Ca2+. This concentration of Ca2+ was chosen since it is close to the resting concentration in parotid acinar cells.12–14 The relative conductance-voltage relations from these two cells are shown in Figure 2B along with fits of the Boltzmann equation. It is apparent that the activation of BK channels in the parotid cells occurred at potentials approximately 120 mV more hyperpolarized than these same channels in the heterologous expression system. There also appeared to be a somewhat smaller voltage sensitivity of activation of the native channels as judged by the small decrease in the z parameter.
Figure 2.
Comparison of the Ca2+ sensitivity of the voltage dependence of native BK channels in parotid acinar cells and the parSlo channel expressed in CHO cells. (A) Raw BK currents recorded from a native cell (upper) and transfected CHO cells (lower) with 80 nM free Ca2+. The voltages used are shown at the right of each current record. (B) Voltage dependence of relative BK channel conductance with 80 nM Ca2+ from a native parotid cell (■) and from a CHO cell transfected with parSlo (◯). Lines are fits of the standard Boltzmann equation with apparent valence and Vh values of 1.36 and 46 mV for the native cell data and 2.1 and 164 mV for the heterologously expressed BK channel. (C) Raw BK currents recorded from a native cell (upper) and transfected CHO cells (lower) with 500 nM free Ca2+. The voltages used are shown at the right of each current record. (D) Voltage dependence of relative BK channel conductance with 500 nM Ca2+ from a native parotid cell (■) and from a CHO cell transfected with parSlo (◯). Lines are fits of the standard Boltzmann equation with apparent valence and Vh values of 1.14 and 17 mV for the native cell data and 1.57 and 120 mV for the heterologously expressed BK channel.
In several such experiments with 80 nM Ca2+ we found a mean value for the Vh values of BK channels in native cells of 46.3 ± 1.6 mV (n = 8) and, about 113 mV more depolarized, 159 ± 2.0 mV (5) for the heterologously expressed channels. The effective valence, z, also differed between the native and expressed channels but not nearly as dramatically: mean values of 1.2 ± 0.06 and 1.8 ± 0.09, respectively. The very hyperpolarized activation range of mouse parotid BK channels was also found in human parotid acinar cells. The difficulty of obtaining human tissue limits the amount of data that can be obtained but in 5 cells from two subjects the mean Vh and z values were 61 ± 3.5 mV and 1.6 ± 0.11, respectively. This property was not confined to the parotid gland: acinar cells from the mouse submandibular gland (SMG) exhibited a Vh value of 37 ± 1.8 mV (9), even more hyperpolarized than parotid cells, and a z value of 1.79 ± 0.06 (9). The human parotid and mouse SMG data were obtained in the absence of crown ether (see Materials and Methods) but this is not likely to be a major issue at this low Ca2+ concentration.
The large difference between the activation voltage ranges of native channels and those expressed in the CHO cells continued as the internal Ca2+ was increased to 500 nM. Figure 2C shows raw currents recorded at this concentration from a native parotid cell (upper) and a cell in which the channels were heterologously expressed (note the different voltage ranges illustrated). Part D of the figure contains the relative conductance-voltage relation from these two cells. The large difference between the activation voltage ranges is apparent with mid point values of 17 and 120 mV for the channels in the native cell and those in the expression system. The steepness of these two relations also differed (z values of 1.14 and 1.57, respectively) and both appeared smaller than their counterparts at 80 nM Ca2+. Pooled data from many cells confirmed these observations: mean Vh values for native cells was 16.5 ± 2.2 mV (12) and 124 ± 1.8 mV (4) and mean z values of 0.96 ± 0.047 (12) and 1.6 ± 0.11 (4), respectively.
Similar experiments were carried out over a range of Ca2+ concentrations from near zero to 2 µM, which encompasses the physiological levels of Ca2+ in these cells.11–14 The pooled results from these experiments are illustrated in Figure 3. The activation curves from BK channels in the native cells are shown with filled symbols and those from heterologously expressed channels are in open symbols. The large difference between the activation range of channels in these two cell systems is apparent. At all concentrations of Ca2+, the activation range of the native channels occurred at substantially more hyperpolarized values than for expressed channels.
Figure 3.
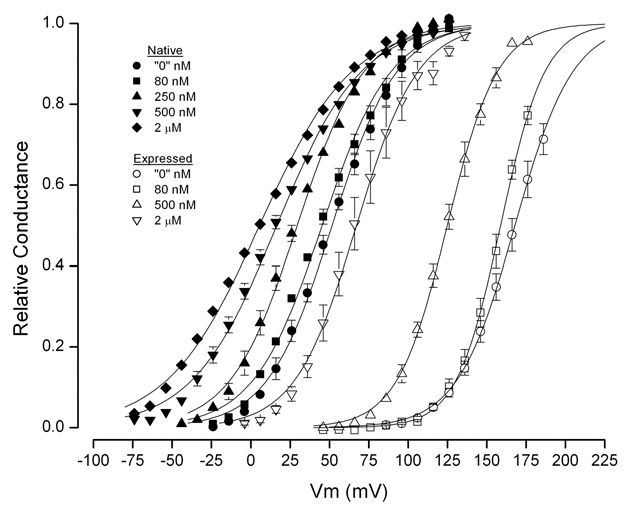
Voltage- and Ca2+-dependence of mean BK channel relative conductance from native cells and from cells expressing parSlo. Data from 4–12 cells at each Ca2+ level. Lines are fits of the standard Boltzmann equation.
It is useful to compare the voltage dependence of BK channel activation in the nominal absence of Ca2+ in order to remove complications from Ca2+-dependent processes. At these “zero” Ca levels the mid-point of parotid cell BK channel activation and activation of the same channels expressed in CHO cells were 51.4 ± 2.0 mV (5) and 168 ± 3.2 mV (5), respectively. That is, the native channels activated at potentials almost 120 mV more hyperpolarized than the heterologously expressed channels. The effective valence, z, was much more similar: 1.24 ± 0.088 (5) and 1.42 ± 0.076 (5) for the native and expressed channel, respectively. These data are listed in Table 1 along with the range of published data from heterologously expressed mSlo and hSlo channels. These data show that, in the nominal absence of Ca2+, the voltage activation of expressed parSlo BK channels was quite similar to the mSlo and hSlo variants and all of these are substantially more depolarized than the channels in native parotid cells.
In many cells BK channels are heteromeric complexes with one of four types of accessory subunits, β1 to β4. The presence of these subunits alters the activation voltage range18,19,21,23–27 so it is possible that the hyperpolarized shift of the native channels could be due to the presence of one of these subunits. Parotid BK channels are not likely to express β2 or β3 subunits since these induce an inactivation in the channels not seen in the native channels. The lack of β2 and β3 transcripts in parotid cells is confirmed by PCR analysis of tissue from the parotid gland showing the presence of only β1 and β4.1 In general these subunits produce a depolarizing shift of BK channel activation in low Ca2+ so their presence should not be responsible for the hyperpolarized activation of native BK channels seen in low Ca2+. In order to verify this assertion, we generated mice with both the β1 and β4 genes ablated. We compared the BK channel activation in these β1/β4-null mice to that in mice with the same genetic background but with both beta subunit genes intact. We found mean values of Vh and z of BK channels in parotid cells from the β1/β4-null mice of 44 ± 1.1 mV (6) and 1.25 ± 0.04 (6), respectively. These values are not statistically different from their gene-matched control group and very similar to the values described above for BK channels from our standard animals. These data are included in Table 1.
Table 1.
BK channel activation parameters in nominally zero Ca2+
| Channel | Vh (mV) | z |
| Native parotid BK | 51.4 ± 2.0 (5) | 1.24 ± 0.088 (5) |
| Expressed parSlo | 168 mV ± 3.2 mV (5) | 1.42 ± 0.076 (5) |
| Expressed mSlo/hSlo | 161–209 mV | 0.95–1.3 |
| β1/β4 (+/+, +/+) | 39.2 ± 5.1 (5) | 1.14 ± 0.11 (5) |
| β1/β4 (×/−, −/−) | 44 ± 2.6 (6) | 1.25 ± 0.089 (6) |
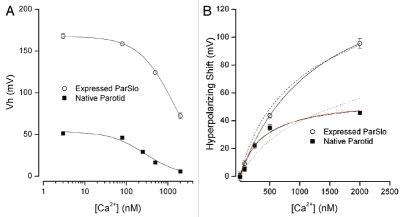
Figure 4A provides a summary of the Vh values from native BK channels and the parSlo channels expressed in CHO cells over the range of Ca2+ examined. The large difference between the activation voltage range of BK channels in the native cells and in the heterologous expression system is apparent. These data seem to indicate that the Ca2+-induced shift of Vh values of native channels was different than those in the expression system. This suggestion is confirmed by the data in Figure 4B where the shift in activation (from the “zero” Ca2+ level) produced by the indicated Ca2+ concentrations is shown for channels in native cells (■) and those in the expression system (○). At least over this range of Ca2+, these two sets of data can each be well-described by a standard single Ca2+ binding isotherm with apparent Ca2+ affinities of 385 nM and 1.3 µM for native parotid channels and those in the expression system, respectively. The actual involvement of Ca2+ in the activation of BK channels is more complicated than implied by this analysis (see below) and the close fits should not be construed as representing a specific mechanism. Nevertheless, the greater than 8-fold difference in the apparent affinities for the Ca2+-induced shift of native BK channels and these same channels in a heterologous expression system illustrate another dramatic difference between these channels. The solid red and dashed lines represent a more detailed analysis of the Ca2+ dependent properties of these channels in the context of the Horrigan-Aldrich model discussed below.
Figure 4.
Shift of the voltage-dependent activation of BK channels in parotid cells and heterologously expressed. (A) Vh values from fits of the Boltzmann equation to BK channel activation in native cells (■) and in cells heterologously expressing parSlo (◯). Mean and SEM limits (if larger than symbols) from 4–12 cells at each Ca2+ concentration. The solid lines serve only to smoothly connect the data points. (B) Magnitude of the hyperpolarizing shift induced by the indicated internal Ca2+ levels. The shift is measured from the lowest Ca2+ value (near 3 nM) and data from native cells (■) and CHO cells expressing parSlo (◯). Solid black lines: fits of standard binding isotherm with apparent Kd values of 158 nM and 1,300 nM for native cell and CHO cell data, respectively. Solid and dashed red lines discussed in text.
The functional basis for parotid BK channel activation.
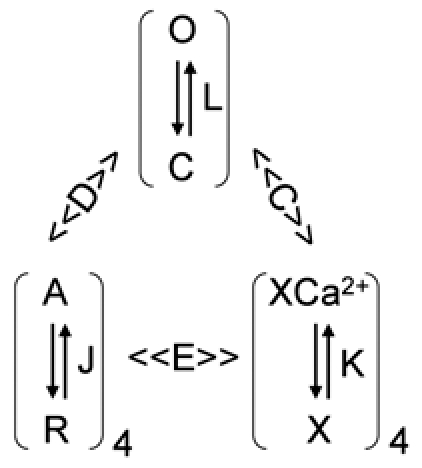
It would be instructive to understand the mechanistic basis for the difference in the activation voltage range between the BK channels in native parotid acinar cells and these same channels in the heterologous expression system. The diagram below represents the current knowledge of the Ca2+- and voltage-dependent behavior of BK channels derived from many studies over several years28–31 which has been given its most complete, quantitative description by Horrigan and Aldrich.17 In this Horrigan-Aldrich (HA) gating scheme, the voltage sensors of each of the four subunits undergoes a transition between a resting (R) and an activated (A) conformation governed by a voltage dependent equilibrium constant, J. The actual channel opening is conferred by a transition from a closed (C) to an open (O) conformation with an equilibrium constant, L, that is also voltage-dependent. The Ca2+ dependence of activation results from the binding of Ca2+ ions to a receptor (X) in each of the four subunits with equilibrium constant, K. D, C and E are allosteric factors that control the synergy among these various gating components. Values of unity for these parameters represent no cooperativity; the larger the value the stronger the coupling. For example, the C-O equilibrium constant L increases D-fold for each voltage sensor that is activated. There are similar allosteric interactions between Ca2+-binding and channel opening (C) and Ca2+-binding and voltage sensor activation (E).
The mathematical description of the activation of the channels with this gating scheme is given by:
where
VJ sets the voltage range of voltage sensor activation; it is the half-activation voltage for each voltage sensor in the absence of Ca2+. The parameter zJ controls the voltage sensitivity for activation of the voltage sensors and zL controls the voltage sensitivity of the closed-open conformational change. Kd is the dissociation constant for a single calcium binding site.
In this scheme there are three parameters that strongly control the voltage-dependent activation of the channel in zero Ca2+: VJ, L0 and D. It has been shown17 that the voltage at which the channel activation time constant has its maximal value provides a rough estimate of the VJ value. Figure 5A shows the measured activation time constants for native BK channels (■) and for heterologously expressed parSlo channels (○). Similar to data obtained from expressed hSlo and mSlo channels,17–19 we found that the time constants for expressed parSlo channels has a maximum at very positive potentials—near 150 mV. In sharp contrast, the time constants for activation of BK channels in parotid cells peaks at much lower voltages—near zero mV. Thus, it is immediately clear that at least one of the mechanisms underlying the strongly hyperpolarized activation range of native BK is that the voltage sensors appear to operate at much more hyperpolarized potentials.
Figure 5.
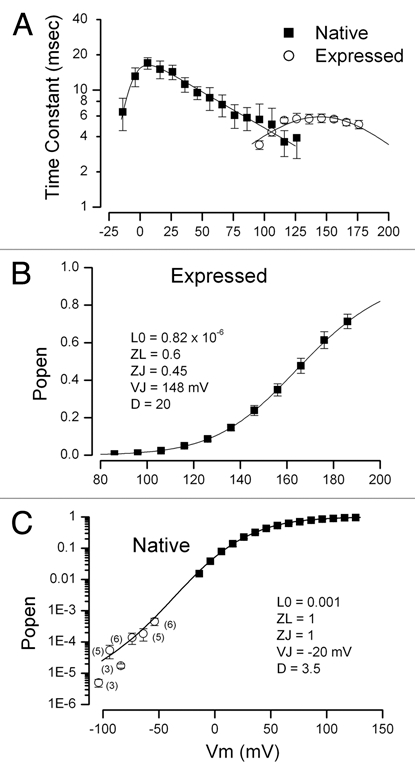
BK channel activation properties in zero Ca2+. (A) The voltage dependence of the time constants from single exponential fits to the activation phase of BK channels in parotid acinar cells (■) and channels expressed in CHO cells (◯). SEM limits for three of the native cell data have been omitted for clarity. Solid lines are fits of the expectation of single close-open transition with voltage dependent forward and backward rate constants given by: 1/(a0.exp(zaVm/RT) + b0·exp(zbVm/RT)). (B) Average voltage-dependent activation of parSlo channels expressed in CHO cells. Mean and SEM limits (where larger than symbols) from five cells. The solid line is computed from the HA model with the indicated parameters. (C) Average voltage dependent activation of BK channels in parotid acinar cells. Solid symbols indicate data obtained at positive membrane voltages. Mean and SEM limits (where larger than symbols) from five cells. The open symbols represent data from whole cell, single channel measurements (in 135 mM K). Mean and SE M limits illustrated from the indicated number of measurements. The solid line is computed from the HA model with the indicated parameters.
It is more difficult to determine the other two parameters (L0 and D) controlling the voltage range of channel activation. However, since it appears that the Ca2+ and voltage sensitivities of heterologously expressed parSlo channels are quite similar to hSlo and mSlo channels we can use previously determined values for these parameters (and for zJ and zL) as starting points for our analysis of expressed parSlo channels. Figure 5B shows the voltage dependence of expressed parSlo channel open probability (relative conductance) in nominally zero Ca2+ (■) (from Fig. 3). The solid line is from the HA model with the indicated parameters. These parameter values are listed in Table 2 along with the range of those published for hSlo and mSlo channels. It is apparent that the values of these parameters from expressed parSlo channels were generally within the range of published values. One exception was the steepness of the closed-open conformational change (zL) which was a bit larger in parSlo channels. Thus the HA model provided an excellent description of heterologously expressed parSlo channels with properties very similar to those of expressed hSlo and mSlo channels previously reported.
Table 2.
Horrigan-Aldrich parameters for BK channels
| Parameter | Expressed parSlo | Expressed mSlo/hSlo | Native BK |
| L0 | 0.82 × 10−6 | 0.2é2.2 × 10−6 | 0.001 |
| zL | 0.6 | 0.31–0.41 | 1 |
| VJ | 148 mV | 120–150 mV | −20 mV |
| zJ | 0.45 | 0.5–0.58 | 1 |
| D | 20 | 16.8–40 | 3.5 |
| Kd | 8 µM | 8.2–21.8 µM | 0.8 µM |
| C | 11 | 8–14 | 2.3 |
| E | 1.8 | 2.4–6 | 1 |
Shown are the HA parameters for the channels indicated. The various parameters are described in the text.
We then turned our attention to the determination of the HA model parameters for the native BK channels. The time constant data of Figure 5 indicates that the VJ parameter will be in the vicinity of 0 mV. Determining the L0 and zL parameters is more difficult. One approach involves measuring gating charge movements.17,18 We were unable to detect gating currents in the native cells most likely because of the relatively few channels expressed in these cells. An alternate method to estimate these parameters is to measure the channel open probability at very negative potentials. At such voltages there is a very low channel open probability and the voltage sensors are all in their resting state. Under these conditions channel activation is described by:
For L << 1 (again at negative potentials where Po is very small) this becomes:
Thus, L0 and zL can be estimated from measurements of channel open probability at very negative potentials where Po is very small. Under these conditions the open channel probability will be a simple exponential function of Vm and so these will be linearly related on a semi-logarithmic plot. We determined the BK channel open probability of native BK channels at very negative potentials by measuring single BK channel activity in whole cell mode as described in Materials and Methods. We found an approximately linear relationship between Po and V m (open symbols in Fig. 5C) and a best fit to the data was obtained with L0 and zL values of 0.02 and 1.78, respectively. It should be emphasized that these are only rough estimates for at least two reasons: (1) the inherent difficulty and potential technical problems in determining the open probability of single channels in whole cell, patch clamp mode and (2) the possibility that the conditions necessary for the analysis are not met. In particular, the voltage range used must be sufficiently negative to assure the voltage sensors are in the resting state. The data in Figure 5A shows that voltage sensor activation of native BK channels was substantially more hyperpolarized than of the expressed channels which raises concern about the rigorous validity of the assumption. Data could not be obtained at more negative voltages because of deleterious effects on the cells. Nevertheless, the values of L0 and zL obtained with this method should still provide a reasonable approximation of the true values.
We used these estimates for the values of L0 and zL and the estimate for VJ, as described above, as starting points for determining a complete set of HA parameters that could describe the native channel open probability over the full range of experimentally accessible membrane potentials. The results of this analysis are illustrated in Figure 5C. The filled symbols are the open channel probability data (relative conductance) from Figure 3 for zero Ca2+. The open symbols represent the channel open probability obtained from single channel measurements in whole cell mode. The solid line is from the HA model with the indicated parameters and these are included in Table 2.
The results of these analyses show that the BK channels in native parotid acinar cells exhibited three significant differences from their heterologously expressed parSlo counterparts: (1) much larger L0 values which means the closed-open transition will occur at more negative potentials; (2) much more hyper-polarized VJ values, which means the voltage sensors operate at more negative values; and (3) a much smaller allosteric parameter D. A small allosteric coupling would, by itself, cause channel activation to shift to more positive potentials because channel activation would rely more on the open-closed conformational change which occurs at potentials relatively positive to the voltage range for voltage sensor activation. Thus, the very hyperpolarized activation of native BK channels is due to the fact that both voltage sensor activation and the closed-open conformational change occur at more hyperpolarized potentials—in spite of a decreased coupling between these two processes.
The data that we have obtained on the Ca2+-dependent shift of Vh (Fig. 4) should allow us to define the Ca2+-dependent model parameters Kd, C and E. We expected to be able to describe the expressed parSlo data with parameters similar to the published values. This was indeed the case as can be seen from the red line in Figure 4 that provides a good description of the data from the expressed channels. The Kd, C and E parameters for this description are listed in Table 2 and are quite similar to previously published values for hSlo and mSlo.
In contrast we were not able to obtain a good fit to the data from the native cells with any set of Ca2+-dependent parameters as long as the Kd value was within the published range. The best version is the dashed red line in Figure 4B associated with the data from the native cells and with values for Kd and allosteric parameters, C and E, of 8 µM, 5, and 1.8, respectively. In order to provide an accurate description of the Ca2+ dependence of the BK channels in native cells (solid red line in Fig. 4B) a 10-fold lower Kd value of 0.8 µM was required along with C and E parameters of 2.3 and 1, respectively. These values are included in the summary in Table 2.
Discussion
Parotid IK1 channel Ca2+ sensitivity.
In this study we examined the Ca2+ dependence of the activation of IK1 and BK channels in parotid acinar cells. Parotid IK1 channels were activated by Ca2+ with an apparent Kd value near 360 nM and a Hill coefficient of about 3. These values are similar to those for IK1 channels in other native cells and in heterologous expression systems systems.32–35 Thus, the Ca2+ sensitivity of IK1 channel activation appears quite similar across species and cell types.
Parotid BK channel Ca2+ sensitivity.
In contrast to the global uniformity of the properties of IK1 channels in native cells and in expression systems, we found that the Ca2+ and voltage sensitivity of BK channels in mouse parotid acinar cells were quite different than the analogous channels in other native cells (e.g., skeletal muscle,5 arterial smooth muscle6 and neurons7) and expression systems.4,20,28 Specifically, in very low intracellular Ca2+ the BK channels in parotid acinar cells activated at potentials more than 100 mV more hyperpolarized than BK channels in native excitable cells and the different Slo variants in heterologous expression systems. This property of BK channels is not restricted to mouse parotid acinar cells—human parotid and mouse submandibular cells have a similarly hyperpolarized voltage activation range (see Table 1). We found that BK channels in mouse distal colon crypt cells have that same property (unpublished observations). Cochlear inner hair cells also express BK channels36 with very hyperpolarized activation in low Ca2+ as do channels in a prostate cancer cell line.37 This shared property suggests that it may be generally true that cells of epithelial origin all express native BK channels with a hyperpolarized activation voltage range.
We also investigated the voltage dependence of parotid BK channels with increased levels of Ca2+. We found that the hyper-polarizing activation shift caused by increased Ca2+ tended to saturate at values near 40 mV for Ca2+ levels of a few µM whereas expressed parSlo (like mSlo and hSlo) shifted near 100 mV for these modest Ca2+ levels and was not nearing saturation.
Functional basis for the hyperpolarized voltage activation range of parotid BK channels.
In order to understand the origin of the differences between BK channels in parotid cells and in heterologous expression systems, we analyzed our results in the context of the Horrigan-Aldrich (HA) description of BK gating. There is a cooperative relationship among three processes in BK channel activation (see Results): a voltage dependent activation of the four voltage sensors, the voltage dependent opening of the channels, and the Ca2+ binding to receptors on the four channel subunits. The hyperpolarized activation of parotid BK channels could result from enhanced activation of the voltage sensors (an increase in the HA J parameter), an enhanced likelihood of channel opening (L), or the allosteric parameter (D) that couples these two processes. Our analysis revealed that the parotid BK channels have a strongly reduced (6-fold) coupling between the voltage sensor activation and channel opening. This property on its own would result in a depolarized shift of BK activation but both the voltage sensor activation and channel opening process were very much shifted to more hyperpolarized potentials.
We found that the HA model could accurately describe the full range of the voltage dependence of parotid acinar cell BK channels and expressed parSlo channels at low Ca2+. We also showed that this model could account for the shift of Vh with increased Ca2+ for both these channels—by increasing the apparent Ca2+ affinity of the native channels. While the HA model accurately described the voltage steepness in nominally zero Ca2+, it could not account for the observed small decrease in this parameter with increased Ca2+ without an accompanying change in the parameters that control voltage steepness. In contrast, while the fits are not perfect, there are examples of the application of the HA model to expressed BK channels that can reasonably account for the small, Ca2+-dependent increase in voltage steepness.17–19,21 We do not know the origin of the inability of the HA model to accurately describe the voltage steepness of parotid BK channel activation over a range of Ca2+ concentrations but there are examples of the same inability for BK channels expressed with a beta subunit.18,19,21
Thus, compared with their expressed channel counterparts, the parotid BK channels seem to have (1) a very different voltage range for activation of the voltage sensors; (2) a very different voltage range for channel opening; (3) a large reduction in the coupling between voltage sensors and channel opening; (4) an increased Ca2+ binding affinity; (5) some other, more minor, alteration that accounts for the inability of the HA model to accurately describe the Ca2+-induced changes in the steepness of the voltage dependence of activation. The net result of these differences is BK channels that are seemingly optimized to operate in the physiological range of intracellular Ca2+ in parotid acinar cells—without the need for these channels to be in microdomains of very high Ca2+ levels. It would be instructive to determine if the functional basis for the low Ca2+, hyperpolarized activation of BK channels in the other cell types is similar to that for the BK channels in native parotid cells.
Molecular basis for the hyperpolarized activation of parotid BK channels.
We do not know the molecular basis for the hyperpolarized activation range of parotid BK channels but possibilities include interaction with auxiliary proteins, post-translational modifications, phosphorylation, oxidation-reduction effects, and the actions of other modulatory agents. The category of auxiliary proteins could include the four known BK channel subunits. Of these, parotid tissue shows evidence of expression of only β1 and β4.1 All four subunits alter the relationship between voltage and Ca2+ activation but they actually make the BK channels less active at low Ca2+ levels and so would not likely underlie the high activity of parotid BK channels in low Ca2+. Nevertheless, we tested the BK channels in parotid acinar cells from animals with both the β1 and β4 genes ablated and these had the same hyperpolarized gating range in low Ca2+ as wild-type animals. Thus, the high activity of native parotid BK channels in low Ca2+ cannot be explained by the presence of one or both of these two β subunits. Note that upregulation of one or both of the other two β subunits can be ruled out since each of these subunits causes clear inactivation of the BK channel which is not seen in the parotid channels.
BK channels are subject to posttranslational modification,38 phosphorylation by serine-threonine kinases,39 tyrosine kinases,40 hormones,41 and the actions of redox agents42 and other small molecules.43 The effects of all these modifications and reagents result in altered activation of BK channels. However, these changes are quite modest compared to the 120 mV differences between native parotid BK channels and most other BK channels.
The modest effects of posttranslational modifications and modulation by kinases or small molecules would seem to make it unlikely that anything similar would be able to account for BK activation at relatively hyperpolarized potentials. While, as noted above, the known β subunits produce a shift of the voltage dependence of BK channel activation in the opposite direction needed, all but β3 can cause up to a 60 mV hyperpolarizing shift in elevated Ca2+.25,27 Thus, while we do not know the actual basis for the hyperpolarized activation of parotid (and other epithelial) BK channels, we speculate that it may be produced by association with a protein or other large molecule that has not yet been shown to modify BK channel gating.
Parotid BK channel physiology.
The fluid secretion process requires a movement of Cl− ions through Ca2+-activated Cl channels (CaCCs) in the apical membrane of secretory acinar cells. Without the concomitant activation of K channels, secretion would come to an immediate halt as the increased Cl− conductance would drive the membrane potential to the Cl− equilibrium potential and so shut off Cl− movement. In addition to their Ca2+ sensitivity CaCCs are also activated by depolarizing voltages.44 If excessive K+ conductance is activated, the cell membrane voltage will approach the K+ equilibrium potential (near −85 mV) and inhibit the Cl channels. Thus, sustained fluid secretion relies on a careful balance of both Cl− and K+ conductances. The apparent Ca2+ affinity for CaCC activation is strongly voltage-dependent and at physiological voltages is near 300 nM.45,46 In this study we found a value of about 350 nM for the Ca2+ affinity of parotid IK1 channels at physiological voltages. We also found that a reasonable fraction of BK channels would be activated at a few hundred nM of Ca2+ even at physiological voltage levels. While the fraction of BK channels activated under these circumstances is not large, single BK channels have about 8 times the conductance of IK1 channels and about 50 times the conductance of the CaCCs1,45,47,48 so even a relative few BK channels can exert a significant influence on the physiology of secretory cells. We showed in this study that the particular biophysical properties of salivary BK and IK1 channels endow them with the necessary characteristics to coordinate with CaCCs in order accomplish their physiological roles in fluid and electrolyte secretion.
Materials and Methods
Ethical approval.
The procedures for animal handling, maintenance and surgery were approved by the University of Rochester Committee on Animal Resources. All animals used in this study were housed in a pathogen-free area at the University of Rochester. Mice were sacrificed by exsanguination following exposure to CO2.
Human parotid tissue was obtained from healthy subjects (30–70 years of age) undergoing surgical removal of a pleomorphic adenoma. Excess normal tissue surrounding the tumor is not used for diagnostic evaluation of the sample. This discarded tissue was collected immediately after surgical excision and transported to the laboratory in ice-cold physiological saline and acinar cells were isolated for electrophysiological recordings. These procedures were approved by the University of Rochester Institutional Review Board.
Parotid acinar cell preparations.
Detailed descriptions of the procedures for producing single parotid acinar cells have been previously described.49 Briefly, surgically removed parotid glands were finely minced and digested with trypsin after which the cells were dissociated with Liberase and, finally, plated onto 5 mm diameter glass coverslips. All dissociation solutions were gassed continuously with 95% O2 + 5% CO2 and maintained at 37°C. Several strains of mice were used in this study. Most of the data were obtained from BlackSwiss x 129/SvJ hybrid animals. Some data were collected from mice in which genes encoding both the BK β1 and β4 subunits were ablated. These animals were the result of breeding β1(−/−) and β4(−/−) mice (each in a C57 background) in the laboratory of James E. Melvin of this institution.
Heterologous expression.
The mouse parotid KCa1.1 variant (parSlo) was cotransfected (0.5 µg or 1 µg) into CHO-K1 cells using a Nucleofector machine (Amaxa Biosystems, MD) according to the manufacturer's instructions or via Lipofectamine LTX (Invitrogen, CA) along with 0.25 µg of the fluorescent vector EYFPpdc315 for transfected cell identification. Cells were used between 24 and 48 h after transfection. The CHO-K1 cells were obtained from ATCC and grown in Ham's F-12K medium + L-glutamine (ATTC, VA) with 10% FBS and maintained in a CO2 incubator with 5% CO2.
Electrophysiological recordings.
Membrane currents were acquired (at 20–22°C) using Axopatch 200B amplifiers and a Digidata 1320A digitizer (Axon Instruments, Foster City, CA) or a 12-bit analog/digital converter system of our own design. Membrane voltages were corrected for the measured junction potential between the external and internal solutions. Pipettes from quartz patch glass (Garner Glass Co., Claremont CA) were used and measured access resistance values were between 2.5 and 8.5 MΩ with a mean value of 5.7 ± 0.19 (SEM, n = 59). Owing to the large macroscopic currents in the native cells special attention was paid to series resistance compensation. A minimum value of 90% was used with most values greater than 95%. The external solution contained (in mM): 150 Na-glutamate, 5 K-glutamate, 2 CaCl2, 2 MgCl2, 10 HEPES, pH 7.2. The internal solution contained 135 mM K-glutamate buffered to pH 7.2 with 10 mM HEPES and various free Ca2+ concentrations designed with MaxChelator Sliders (Chris Patton) (http://maxchelator.stanford.edu). The Ca2+ buffers used for the various free concentrations were: 10 mM BAPTA (80 nM); 2.5 mM EGTA/2.5 EDTA mM (500 nM); and 5 mM HEDTA (2 µM). A nominally 0 Ca2+ solution contained 10 mM BAPTA with no added Ca2+ with an estimated free Ca2+ level of 3 nM. All internal solutions contained 50 µmol/l crown ether to chelate potential Ba2+ contamination. The use of glutamate as the main anion in these solutions was used to reduce Cl− channel currents in the native parotid cells to insignificant levels.
The external solutions used to isolate currents through BK channels in the parotid acinar cells included maurotoxin (Sigma) and TRAM-34 (Sigma) to eliminate currents through IK1 channels.50,51 The TRAM-34 concentration used was 1 µM from a 10 mM stock in DMSO. Maurotoxin was used at a final concentration of 50 nM in the presence of 0.01% BSA from a 50 µM stock solution in 15 mM Na2HPO4. Maurotoxin concentrations as high as 1 µM have a negligible effect on BK channels50 which we have confirmed (unpublished observations) with mouse parotid cells with the IK1 gene ablated.2 In addition TRAM-34 and the related compound clotrimazole have a negligible effect on BK channel currents.49 Finally, since negligible IK1 currents are activated in low Ca2+ (80 nM or less) control experiments were performed with these low Ca2+ levels and showed an insignificant effect of these concentrations of maurotoxin and TRAM-34 on BK channel currents (data not shown).
IK1 channels are voltage- and time-independent and so we measured the currents through these channels from the instantaneous change in current (immediately after the settling of the capacity transient) at each test potential before significant activation of the time-dependent BK channel. Negligible IK1 channel currents are activated at Ca2+ concentrations below 100 nM.32,33 IK1 channels are activated by an increase in the open channel probability by the chemical compound DCEBIO with a Kd value of about 840 nM.52 The DCEBIO activation of IK1 channels is independent of intracellular Ca2+ levels above 100 nM.53 Thus, in our experiments we determined the relative level of IK1 activation from currents recorded at a given level of intracellular Ca2+ and the current level induced by 20 µM DCEBIO.
Data analysis.
BK channel steady state currents (ISS) were used to compute conductance-voltage (G–V) relation: GBK = ISS/(Vm − VK) where Vm is the membrane voltage and VK is the K+ equilibrium potential. Relative channel activation was determined as the relative BK conductance. The G–V relations in low Ca2+ did not reach a saturating level at reasonably accessible voltages so in these cases the fit of a Boltzmann function was used to estimate the maximal level:
where Gmax is the maximum conductance, z is the effective valence that controls the slope of the relation, Vh is the voltage at which the conductance reaches half its maximum value, and F, R and T have their usual thermodynamic meanings.
The analysis of the native BK channel properties with the Horrigan-Aldrich model (see text) requires channel activation data at very low open probability values with 0 Ca2+. The rather small number of BK channels in these parotid cells requires that these low open probability values be obtained by measuring single BK channel activity. This was done in the whole cell, patch clamp mode with an external solution consisting of (in mM): 135 K-glutamate, 2 CaCl2, 2 MgCl2, 10 HEPES, pH 7.2. In these experiments we measured the fractional single channel open time and, with the measured single channel current level, computed the conductance. The maximum BK conductance was obtained in the same cell via macroscopic current measurements and the relative conductance computed as the ratio of these.
The various mathematical functions were computed or fit to data by several software programs and algorithms. Exponential fitting was done in Clampfit 9.2 and software of our own design using Levenberg-Marquardt, Simplex and sum of squares methods. Boltzmann functions and the predictions of the Horrigan-Aldrich model were made in Origin 6.1 or 7. The Ca2+ dependence of some parameters was fit by the Hill equation:
where Kd is the apparent Ca2+ binding affinity and N is the Hill coefficient, necessary for an accurate description of situations involving more than a single Ca2+ binding site.
Unless otherwise indicated pooled data are reported as the mean with standard error of the mean values. Tests of statistical significance of paired groups of data were performed with the independent t-test in Origin with p ≤ 0.05 considered significant.
Acknowledgements
This work was supported by NIH grants: RO1 DE016960 and R01 DE019245. We thank Richard W. Aldrich (University of Texas at Austin; Austin, TX) and R. Brenner (University of Texas Health Science Center; San Antonio, TX) for the β1 and β4 knock-out mice. We are grateful to James E. Melvin for continuing discussions during this work and for critically reading the manuscript. We also thank Laurie Koeck and Yasna Jaramillo of the Melvin lab for maintaining the mouse colonies and for breeding the β1 and β4 double knock-out mice.
Abbreviation
- BK
a big conductance
- Ca2+-activated K channel
IK1
- an intermediate conductance
Ca2+-activated K channel
Footnotes
Previously published online: www.landesbioscience.com/journals/channels/article/12197
References
- 1.Nehrke K, Quinn CC, Begenisich T. Molecular identification of Ca2+-activated K+ channels in parotid acinar cells. Am J Physiol Cell Physiol. 2003;284:535–546. doi: 10.1152/ajpcell.00044.2002. [DOI] [PubMed] [Google Scholar]
- 2.Begenisich T, Nakamoto T, Ovitt CE, Nehrke K, Brugnara C, Alper SL, et al. Physiological roles of the intermediate conductance, Ca2+-activated potassium channel Kcnn4. J Biol Chem. 2004;279:47681–47687. doi: 10.1074/jbc.M409627200. [DOI] [PubMed] [Google Scholar]
- 3.Romanenko V, Nakamoto T, Srivastava A, Melvin JE, Begenisich T. Molecular identification and physiological roles of parotid acinar cell maxi-K channels. J Biol Chem. 2006;281:27964–27972. doi: 10.1074/jbc.M603871200. [DOI] [PubMed] [Google Scholar]
- 4.Butler A, Tsunoda S, McCobb DP, Wei A, Salkoff L. mSlo, a complex mouse gene encoding “maxi” calcium-activated potassium channels. Science. 1993;261:221–224. doi: 10.1126/science.7687074. [DOI] [PubMed] [Google Scholar]
- 5.Pallotta BS, Magleby KL, Barrett JN. Single channel recordings of Ca2+-activated K+ currents in rat muscle cell culture. Nature. 1981;293:471–474. doi: 10.1038/293471a0. [DOI] [PubMed] [Google Scholar]
- 6.Brayden JE, Nelson MT. Regulation of arterial tone by activation of calcium-dependent potassium channels. Science. 1992;256:532–535. doi: 10.1126/science.1373909. [DOI] [PubMed] [Google Scholar]
- 7.Robitaille R, Garcia ML, Kaczorowski GJ, Charlton MP. Functional colocalization of calcium and calcium-gated potassium channels in control of transmitter release. Neuron. 1993;11:645–655. doi: 10.1016/0896-6273(93)90076-4. [DOI] [PubMed] [Google Scholar]
- 8.Nelson MT, Cheng H, Rubart M, Santana LF, Bonev AD, Knot HJ, et al. Relaxation of arterial smooth muscle by calcium sparks. Science. 1995;270:633–637. doi: 10.1126/science.270.5236.633. [DOI] [PubMed] [Google Scholar]
- 9.Brenner R, Perez GJ, Bonev AD, Eckman DM, Kosek JC, Wiler SW, et al. Vasoregulation by the beta1 subunit of the calcium-activated potassium channel. Nature. 2000;407:870–876. doi: 10.1038/35038011. [DOI] [PubMed] [Google Scholar]
- 10.Zhuge R, Fogarty KE, Tuft RA, Walsh JV., Jr Spontaneous transient outward currents arise from microdomains where BK channels are exposed to a mean Ca2+ concentration on the order of 10 microM during a Ca2+ spark. J Gen Physiol. 2002;120:15–27. doi: 10.1085/jgp.20028571. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 11.Sneyd J, Tsaneva-Atanasova K, Yule DI, Thompson JL, Shuttleworth TJ. Control of calcium oscillations by membrane fluxes. Proc Natl Acad Sci USA. 2004;101:1392–1396. doi: 10.1073/pnas.0303472101. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 12.Gray PT. Oscillations of free cytosolic calcium evoked by cholinergic and catecholaminergic agonists in rat parotid acinar cells. J Physiol. 1988;406:35–53. doi: 10.1113/jphysiol.1988.sp017367. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 13.Foskett JK, Melvin JE. Activation of salivary secretion: coupling of cell volume and [Ca2+]i in single cells. Science. 1989;244:1582–1585. doi: 10.1126/science.2500708. [DOI] [PubMed] [Google Scholar]
- 14.Foskett JK, Gunter-Smith PJ, Melvin JE, Turner RJ. Physiological localization of an agonist-sensitive pool of Ca2+ in parotid acinar cells. Proc Natl Acad Sci USA. 1989;86:167–171. doi: 10.1073/pnas.86.1.167. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 15.Hayashi T, Poronnik P, Young JA, Cook DI. The AChevoked, Ca2+-activated whole-cell K+ current in mouse mandibular secretory cells. Whole-cell and fluorescence studies. J Membr Biol. 1996;152:253–259. doi: 10.1007/s002329900103. [DOI] [PubMed] [Google Scholar]
- 16.Maruyama Y, Gallacher DV, Petersen OH. Voltage and Ca2+-activated K+ channel in baso-lateral acinar cell membranes of mammalian salivary glands. Nature. 1983;302:827–829. doi: 10.1038/302827a0. [DOI] [PubMed] [Google Scholar]
- 17.Horrigan FT, Aldrich RW. Coupling between voltage sensor activation, Ca2+ binding and channel opening in large conductance (BK) potassium channels. J Gen Physiol. 2002;120:267–305. doi: 10.1085/jgp.20028605. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 18.Bao L, Cox DH. Gating and ionic currents reveal how the BKCa channel's Ca2+ sensitivity is enhanced by its beta1 subunit. J Gen Physiol. 2005;126:393–412. doi: 10.1085/jgp.200509346. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 19.Orio P, Latorre R. Differential effects of beta1 and beta2 subunits on BK channel activity. J Gen Physiol. 2005;125:395–411. doi: 10.1085/jgp.200409236. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 20.Koval OM, Fan Y, Rothberg BS. A role for the S0 transmembrane segment in voltage-dependent gating of BK channels. J Gen Physiol. 2007;129:209–220. doi: 10.1085/jgp.200609662. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 21.Wang B, Rothberg BS, Brenner R. Mechanism of beta4 subunit modulation of BK channels. J Gen Physiol. 2006;127:449–465. doi: 10.1085/jgp.200509436. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 22.Hou S, Horrigan FT, Xu R, Heinemann SH, Hoshi T. Comparative effects of H+ and Ca2+ on large-conductance Ca2+- and voltage-gated Slo1 K+ channels. Channels (Austin) 2009;3:249–258. doi: 10.4161/chan.3.4.9253. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 23.Sweet TB, Cox DH. Measuring the influence of the BKCa {beta}1 subunit on Ca2+ binding to the BKCa channel. J Gen Physiol. 2009;133:139–150. doi: 10.1085/jgp.200810129. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 24.Dworetzky SI, Boissard CG, Lum-Ragan JT, McKay MC, Post-Munson DJ, Trojnacki JT, et al. Phenotypic alteration of a human BK (hSlo) channel by hSlo beta subunit coexpression: changes in blocker sensitivity, activation/relaxation and inactivation kinetics, and protein kinase A modulation. J Neurosci. 1996;16:4543–4550. doi: 10.1523/JNEUROSCI.16-15-04543.1996. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 25.Brenner R, Jegla TJ, Wickenden A, Liu Y, Aldrich RW. Cloning and functional characterization of novel large conductance calcium-activated potassium channel beta subunits, hKCNMB3 and hKCNMB4. J Biol Chem. 2000;275:6453–6461. doi: 10.1074/jbc.275.9.6453. [DOI] [PubMed] [Google Scholar]
- 26.Weiger TM, Holmqvist MH, Levitan IB, Clark FT, Sprague S, Huang WJ, et al. A novel nervous system beta subunit that downregulates human large conductance calcium-dependent potassium channels. J Neurosci. 2000;20:3563–3570. doi: 10.1523/JNEUROSCI.20-10-03563.2000. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 27.Behrens R, Nolting A, Reimann F, Schwarz M, Waldschutz R, Pongs O. hKCNMB3 and hKCNMB4, cloning and characterization of two members of the large-conductance calcium-activated potassium channel beta subunit family. FEBS Lett. 2000;474:99–106. doi: 10.1016/s0014-5793(00)01584-2. [DOI] [PubMed] [Google Scholar]
- 28.Cui J, Cox DH, Aldrich RW. Intrinsic voltage dependence and Ca2+ regulation of mslo large conductance Ca-activated K+ channels. J Gen Physiol. 1997;109:647–673. doi: 10.1085/jgp.109.5.647. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 29.Horrigan FT, Cui J, Aldrich RW. Allosteric voltage gating of potassium channels I. Mslo ionic currents in the absence of Ca2+ J Gen Physiol. 1999;114:277–304. doi: 10.1085/jgp.114.2.277. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 30.Horrigan FT, Aldrich RW. Allosteric voltage gating of potassium channels II. Mslo channel gating charge movement in the absence of Ca2+ J Gen Physiol. 1999;114:305–336. doi: 10.1085/jgp.114.2.305. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 31.Rothberg BS, Magleby KL. Voltage and Ca2+ activation of single large-conductance Ca2+-activated K+ channels described by a two-tiered allosteric gating mechanism. J Gen Physiol. 2000;116:75–99. doi: 10.1085/jgp.116.1.75. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 32.Ishii TM, Silvia C, Hirschberg B, Bond CT, Adelman JP, Maylie J. A human intermediate conductance calcium-activated potassium channel. Proc Natl Acad Sci USA. 1997;94:11651–11656. doi: 10.1073/pnas.94.21.11651. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 33.Logsdon NJ, Kang J, Togo JA, Christian EP, Aiyar J. A novel gene, hKCa4, encodes the calcium-activated potassium channel in human T lymphocytes. J Biol Chem. 1997;272:32723–32726. doi: 10.1074/jbc.272.52.32723. [DOI] [PubMed] [Google Scholar]
- 34.Takahata T, Hayashi M, Ishikawa T. SK4/IK1-like channels mediate TEA-insensitive, Ca2+-activated K+ currents in bovine parotid acinar cells. Am J Physiol Cell Physiol. 2003;284:127–144. doi: 10.1152/ajpcell.00250.2002. [DOI] [PubMed] [Google Scholar]
- 35.Grissmer S, Nguyen AN, Cahalan MD. Calcium-activated potassium channels in resting and activated human T lymphocytes. Expression levels, calcium dependence, ion selectivity and pharmacology. J Gen Physiol. 1993;102:601–630. doi: 10.1085/jgp.102.4.601. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 36.Thurm H, Fakler B, Oliver D. Ca2+-independent activation of BKCa channels at negative potentials in mammalian inner hair cells. J Physiol. 2005;569:137–151. doi: 10.1113/jphysiol.2005.094680. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 37.Gessner G, Schonherr K, Soom M, Hansel A, Asim M, Baniahmad A, et al. BKCa channels activating at resting potential without calcium in LNCaP prostate cancer cells. J Membr Biol. 2005;208:229–240. doi: 10.1007/s00232-005-0830-z. [DOI] [PubMed] [Google Scholar]
- 38.Braun AP. Distinct post-translational modifications regulate BK channel activity: the interplay between protein palmitoylation and phosphorylation. Channels (Austin) 2009;3:144–145. doi: 10.4161/chan.3.3.9257. [DOI] [PubMed] [Google Scholar]
- 39.Schubert R, Nelson MT. Protein kinases: tuners of the BKCa channel in smooth muscle. Trends Pharmacol Sci. 2001;22:505–512. doi: 10.1016/s0165-6147(00)01775-2. [DOI] [PubMed] [Google Scholar]
- 40.Alioua A, Mahajan A, Nishimaru K, Zarei MM, Stefani E, Toro L. Coupling of c-Src to large conductance voltage- and Ca2+-activated K+ channels as a new mechanism of agonist-induced vasoconstriction. Proc Natl Acad Sci USA. 2002;99:14560–14565. doi: 10.1073/pnas.222348099. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 41.Valverde MA, Rojas P, Amigo J, Cosmelli D, Orio P, Bahamonde MI, et al. Acute activation of Maxi-K channels (hSlo) by estradiol binding to the beta subunit. Science. 1999;285:1929–1931. doi: 10.1126/science.285.5435.1929. [DOI] [PubMed] [Google Scholar]
- 42.Soto MA, Gonzalez C, Lissi E, Vergara C, Latorre R. Ca2+-activated K+ channel inhibition by reactive oxygen species. Am J Physiol Cell Physiol. 2002;282:461–471. doi: 10.1152/ajpcell.00167.2001. [DOI] [PubMed] [Google Scholar]
- 43.Hou S, Heinemann SH, Hoshi T. Modulation of BKCa channel gating by endogenous signaling molecules. Physiology (Bethesda) 2009;24:26–35. doi: 10.1152/physiol.00032.2008. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 44.Arreola J, Melvin JE, Begenisich T. Activation of calcium-dependent chloride channels in rat parotid acinar cells. J Gen Physiol. 1996;108:35–47. doi: 10.1085/jgp.108.1.35. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 45.Nakamoto T, Srivastava A, Romanenko VG, Ovitt CE, Perez-Cornejo P, Arreola J, et al. Functional and molecular characterization of the fluid secretion mechanism in human parotid acinar cells. Am J Physiol Regul Integr Comp Physiol. 2007;292:2380–2390. doi: 10.1152/ajpregu.00591.2006. [DOI] [PubMed] [Google Scholar]
- 46.Giovannucci DR, Bruce JI, Straub SV, Arreola J, Sneyd J, Shuttleworth TJ, et al. Cytosolic Ca2+ and Ca2+-activated Cl- current dynamics: insights from two functionally distinct mouse exocrine cells. J Physiol. 2002;540:469–484. doi: 10.1113/jphysiol.2001.013453. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 47.Romanenko VG, Nakamoto T, Srivastava A, Begenisich T, Melvin JE. Regulation of membrane potential and fluid secretion by Ca2+-activated K+ channels in mouse submandibular glands. J Physiol. 2007;581:801–817. doi: 10.1113/jphysiol.2006.127498. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 48.Piper AS, Large WA. Multiple conductance states of single Ca2+-activated Cl- channels in rabbit pulmonary artery smooth muscle cells. J Physiol. 2003;547:181–196. doi: 10.1113/jphysiol.2002.033688. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 49.Thompson J, Begenisich T. Membrane-delimited inhibition of maxi-K channel activity by the intermediate conductance Ca2+-activated K channel. J Gen Physiol. 2006;127:159–169. doi: 10.1085/jgp.200509457. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 50.Castle NA, London DO, Creech C, Fajloun Z, Stocker JW, Sabatier JM. Maurotoxin: a potent inhibitor of intermediate conductance Ca2+-activated potassium channels. Mol Pharmacol. 2003;63:409–418. doi: 10.1124/mol.63.2.409. [DOI] [PubMed] [Google Scholar]
- 51.Wulff H, Miller MJ, Hansel W, Grissmer S, Cahalan MD, Chandy KG. Design of a potent and selective inhibitor of the intermediate-conductance Ca2+activated K+ channel, IKCa1: a potential immunosuppressant. Proc Natl Acad Sci USA. 2000;97:8151–8156. doi: 10.1073/pnas.97.14.8151. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 52.Singh S, Syme CA, Singh AK, Devor DC, Bridges RJ. Benzimidazolone activators of chloride secretion: potential therapeutics for cystic fibrosis and chronic obstructive pulmonary disease. J Pharmacol Exp Ther. 2001;296:600–611. [PubMed] [Google Scholar]
- 53.Pedersen KA, Schroder RL, Skaaning-Jensen B, Strobaek D, Olesen SP, Christophersen P. Activation of the human intermediate-conductance Ca2+-activated K+ channel by 1-ethyl-2-benzimidazolinone is strongly Ca2+-dependent. Biochim Biophys Acta. 1999;1420:231–240. doi: 10.1016/s0005-2736(99)00110-8. [DOI] [PubMed] [Google Scholar]